Simulation and Experimental Activity for the Evaluation of the Filling Capability in External Gear Pumps
Abstract
:1. Introduction
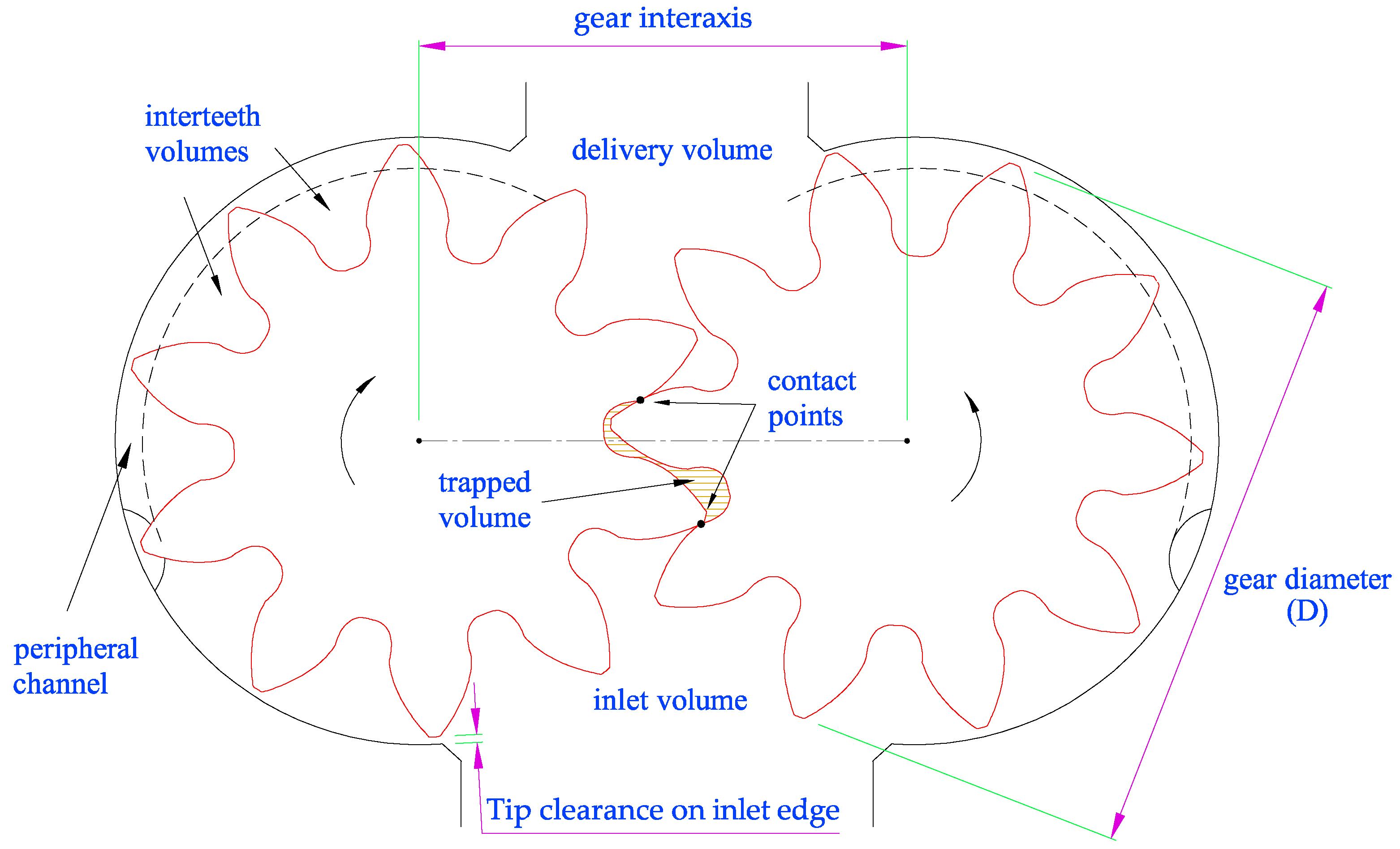
2. Component Description
3. Description of the Simulation Model
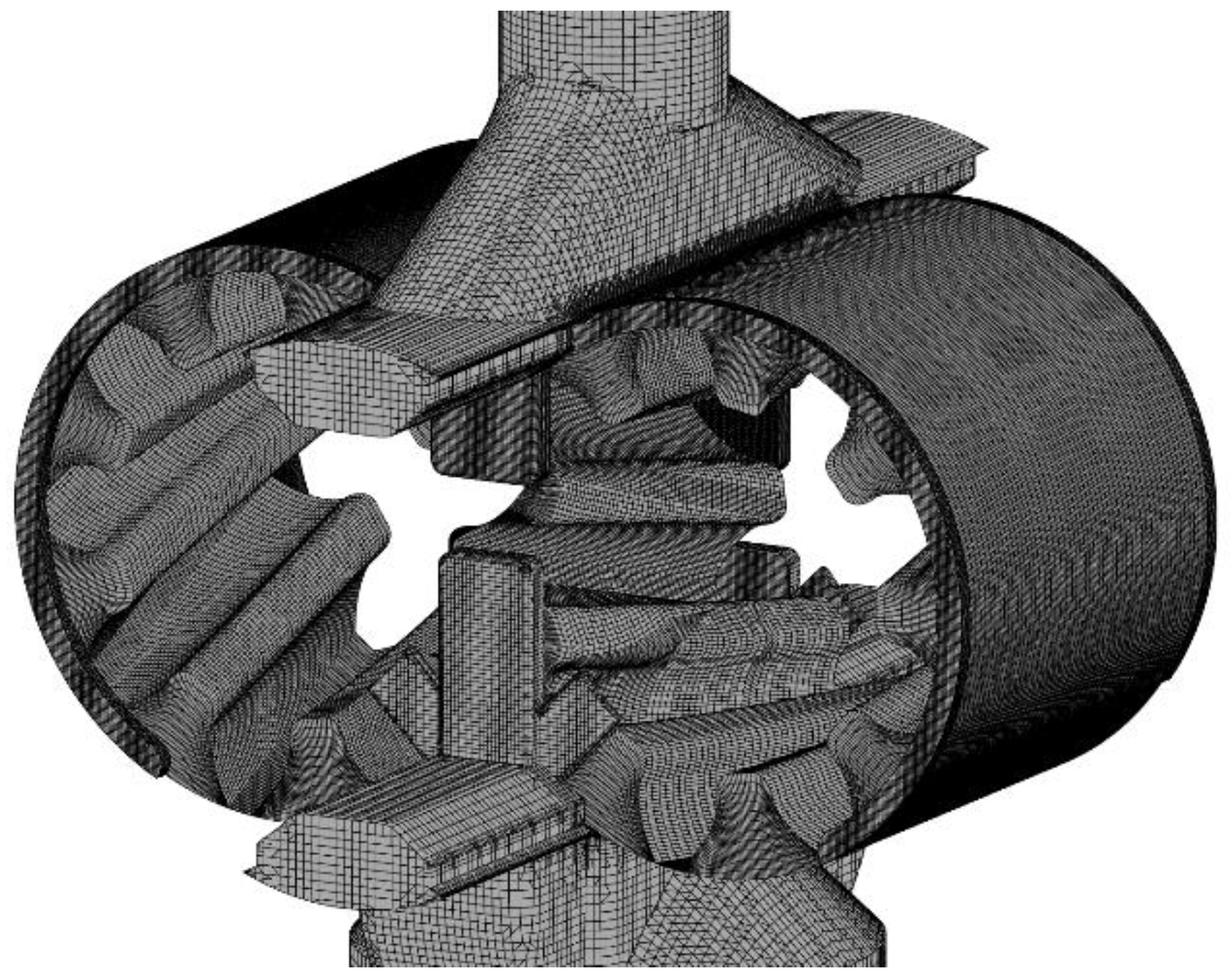
3.1. Model Structure
3.2. Model Optimization
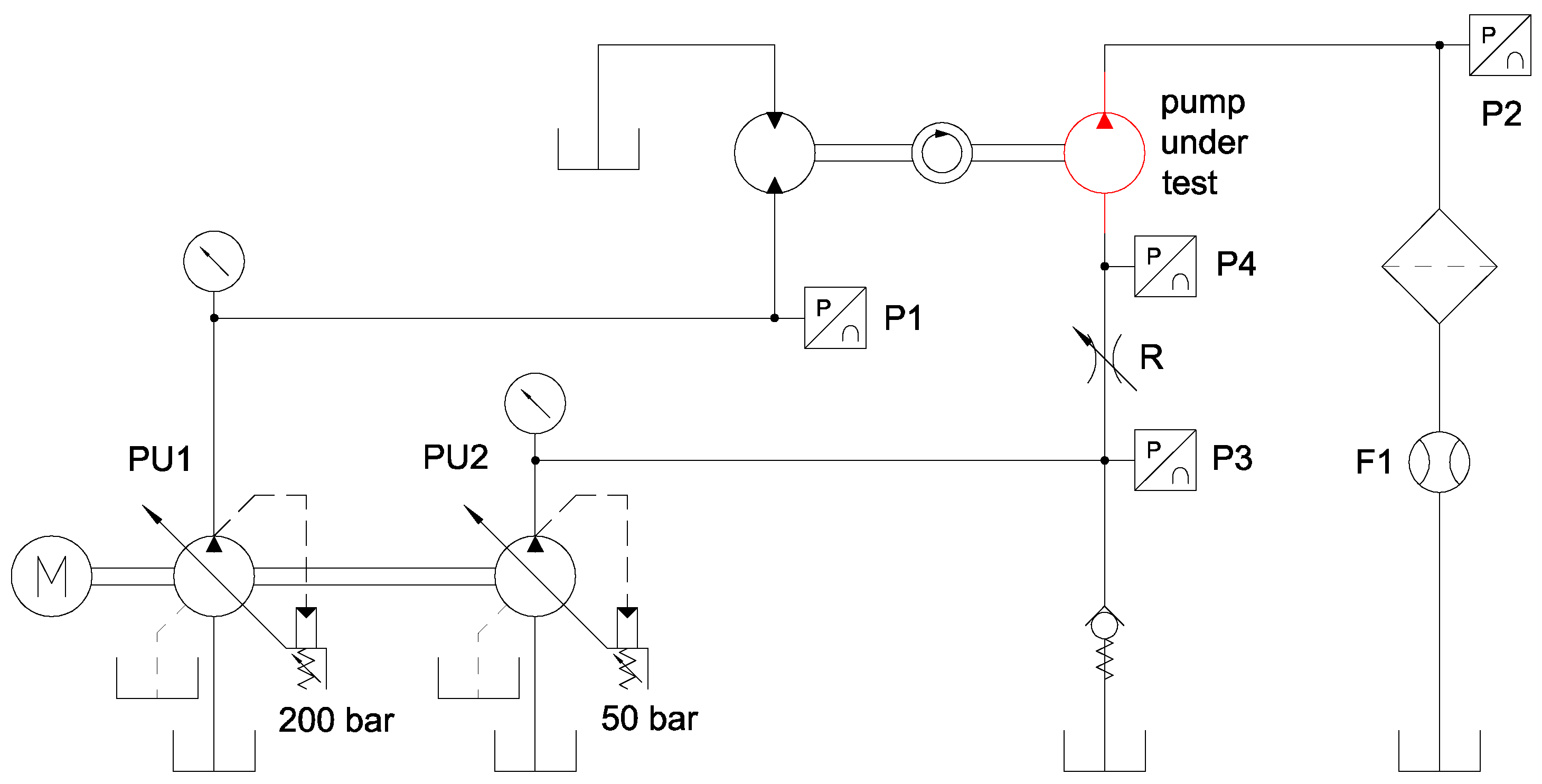
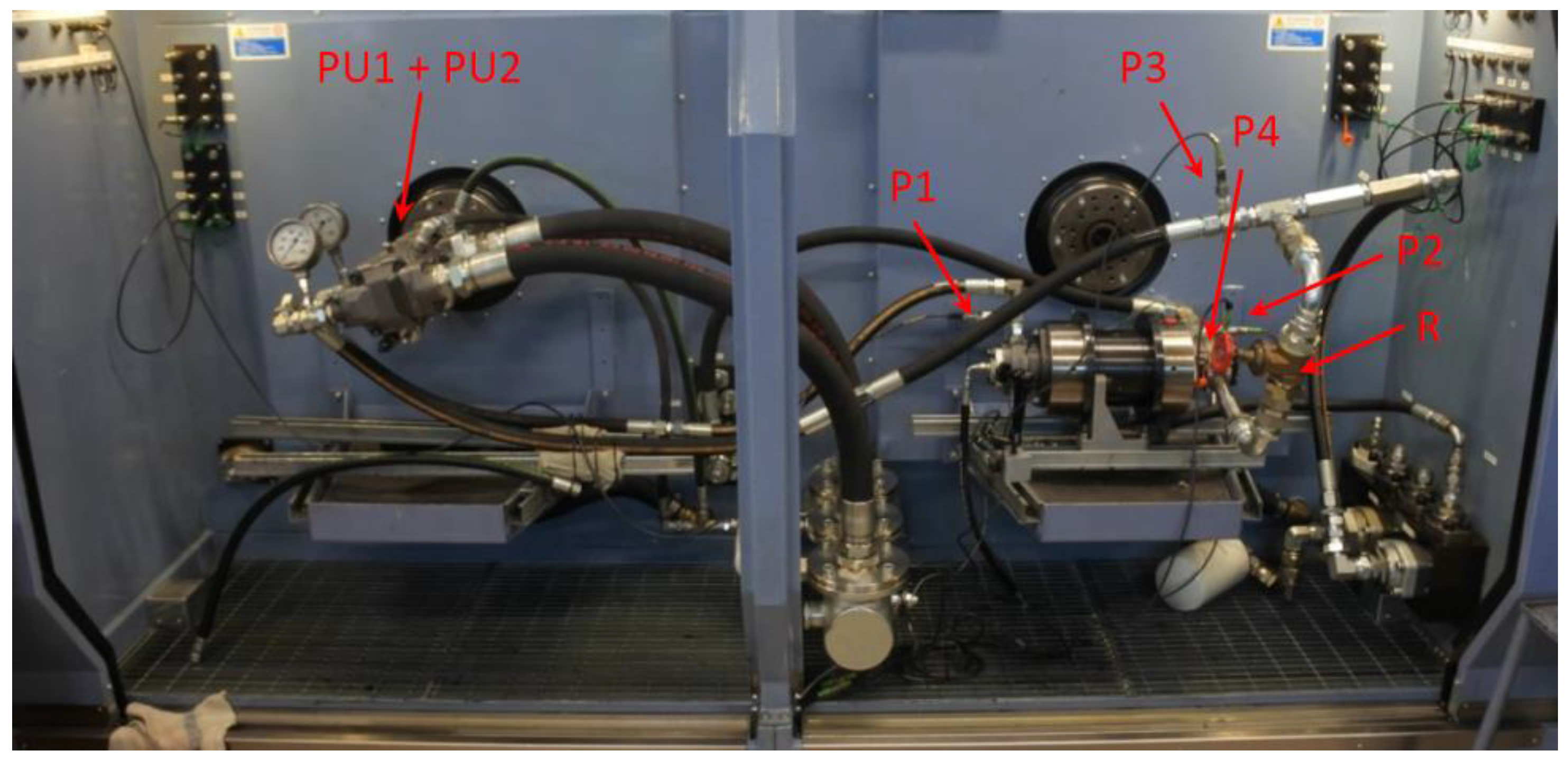
4. Test Rig Layout
5. Results and Discussion
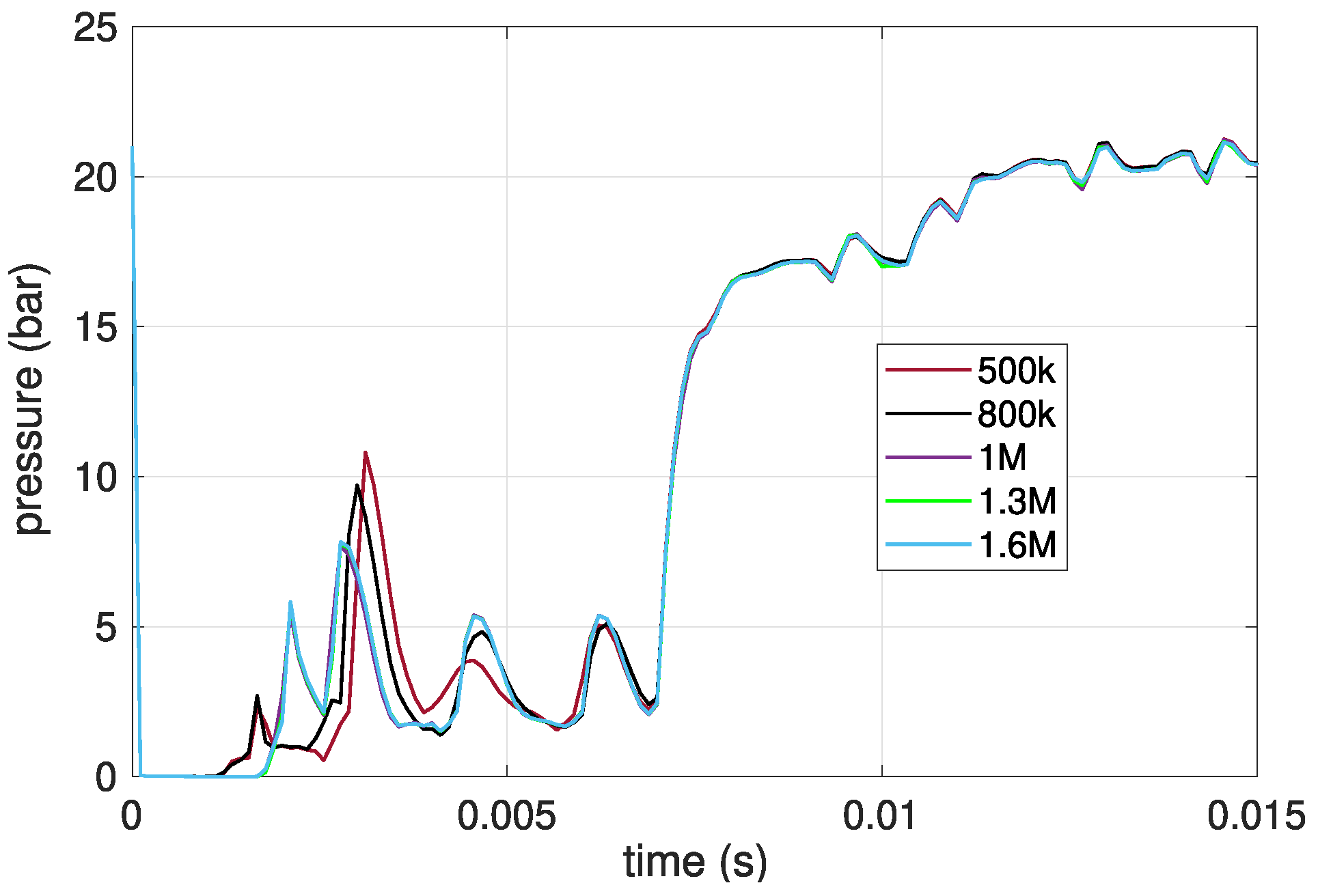
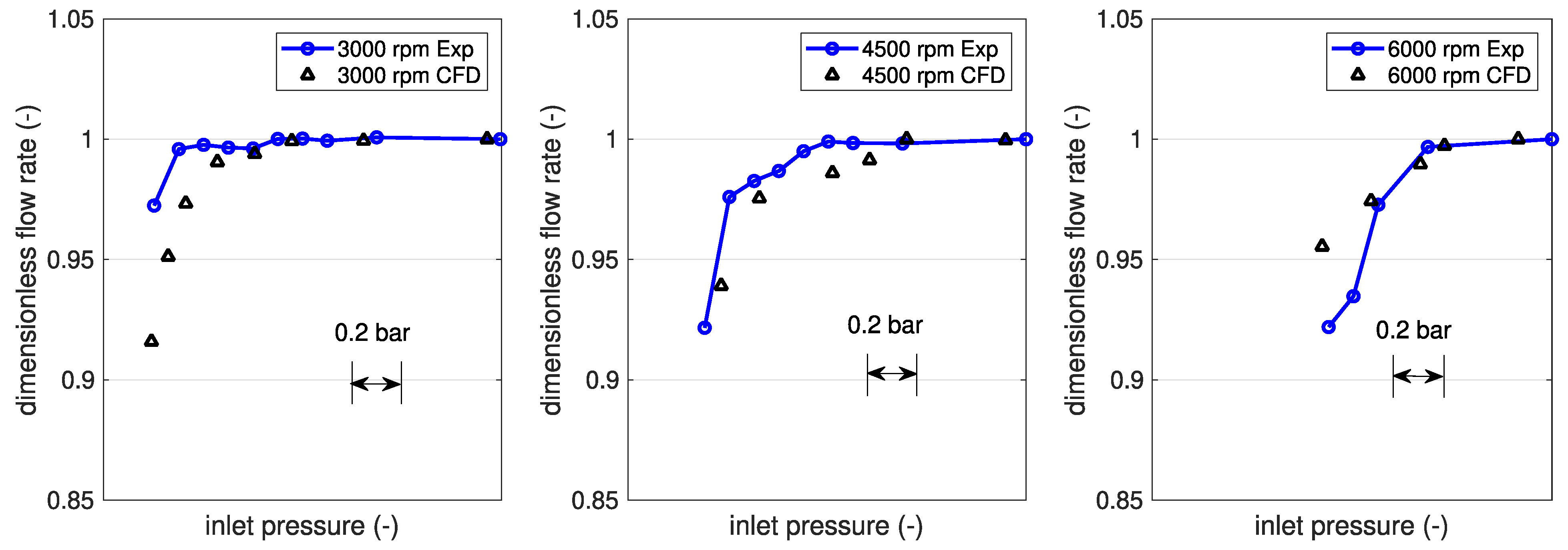
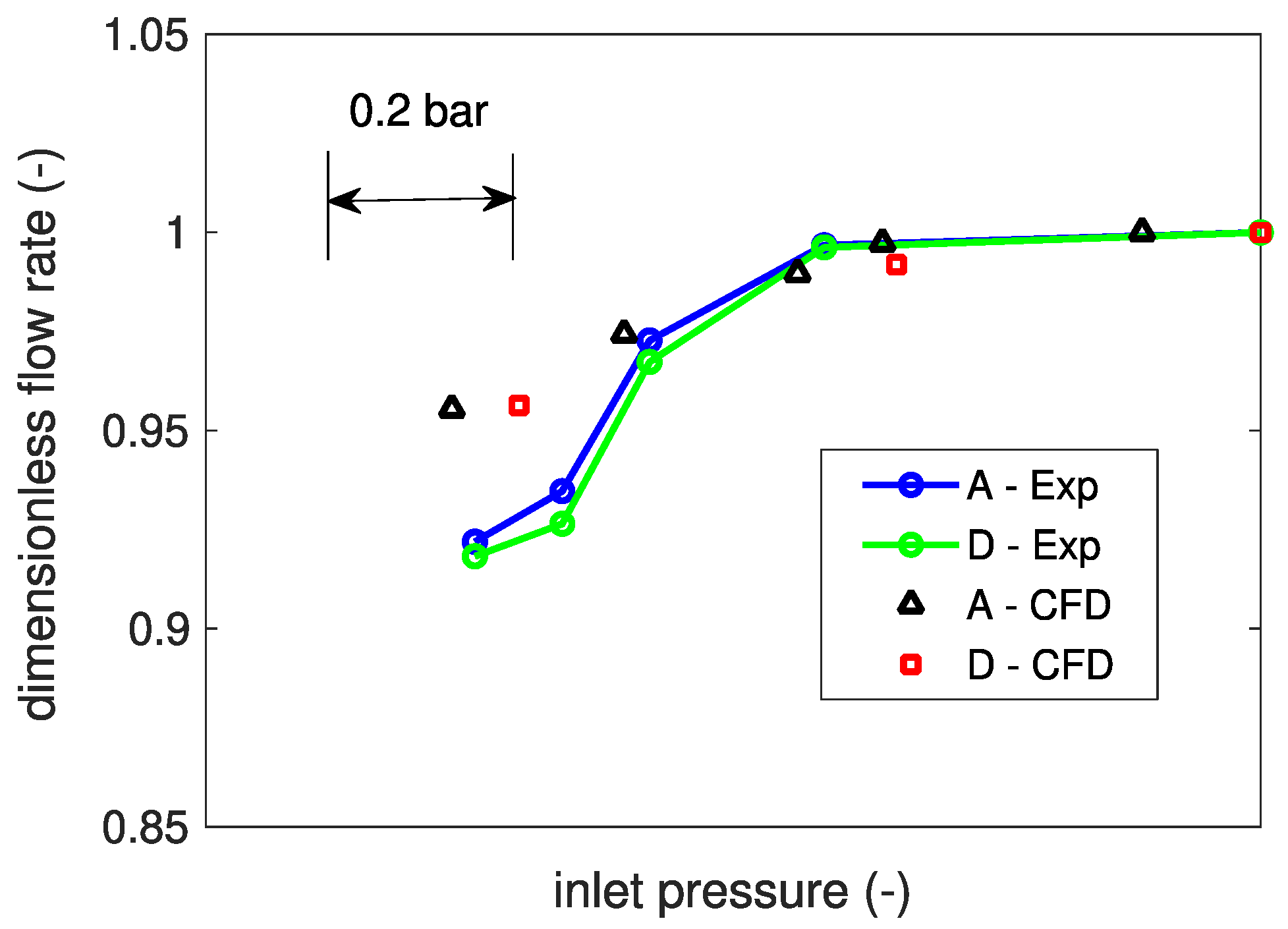
5.1. Steady-State Characteristics
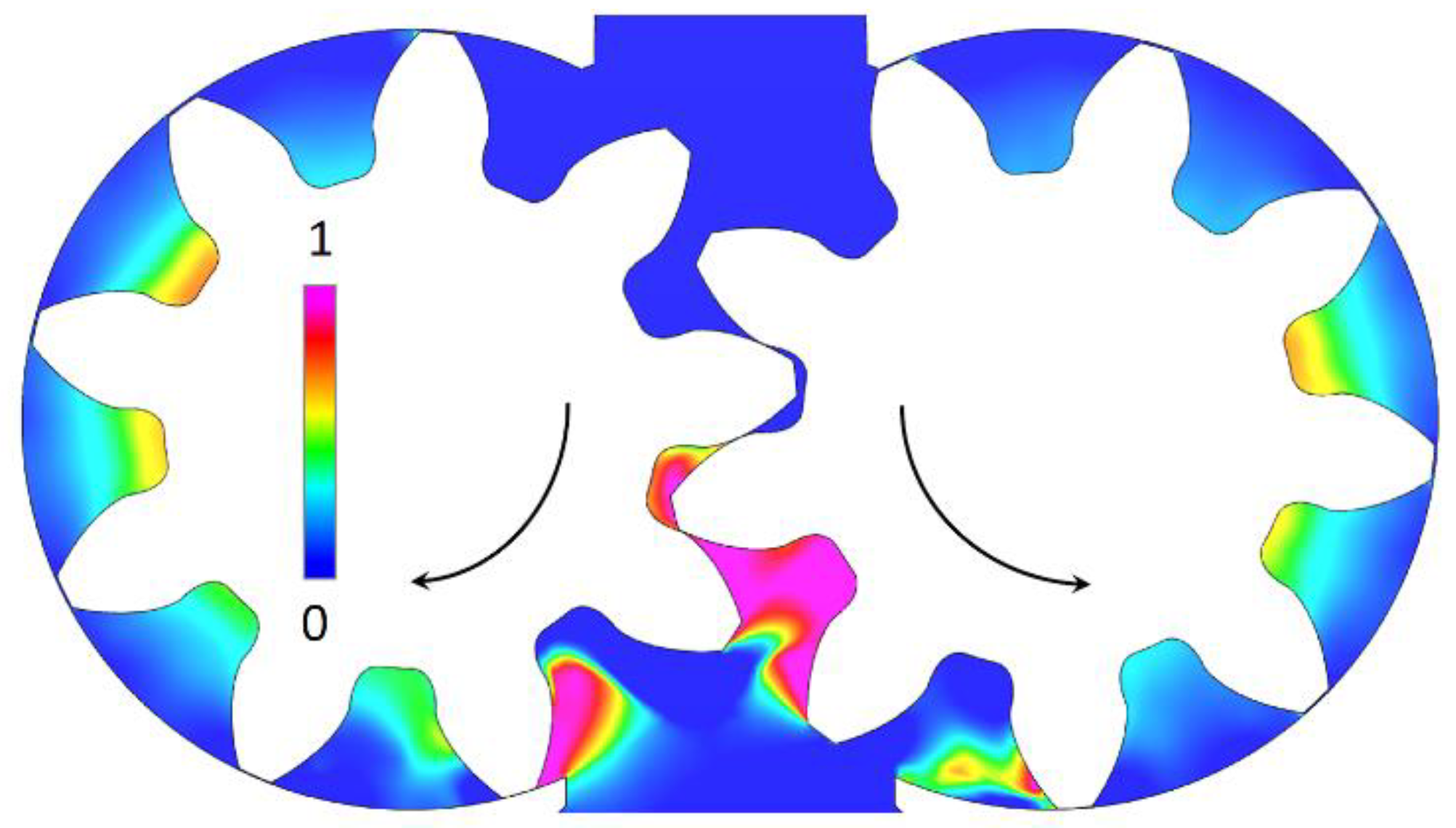
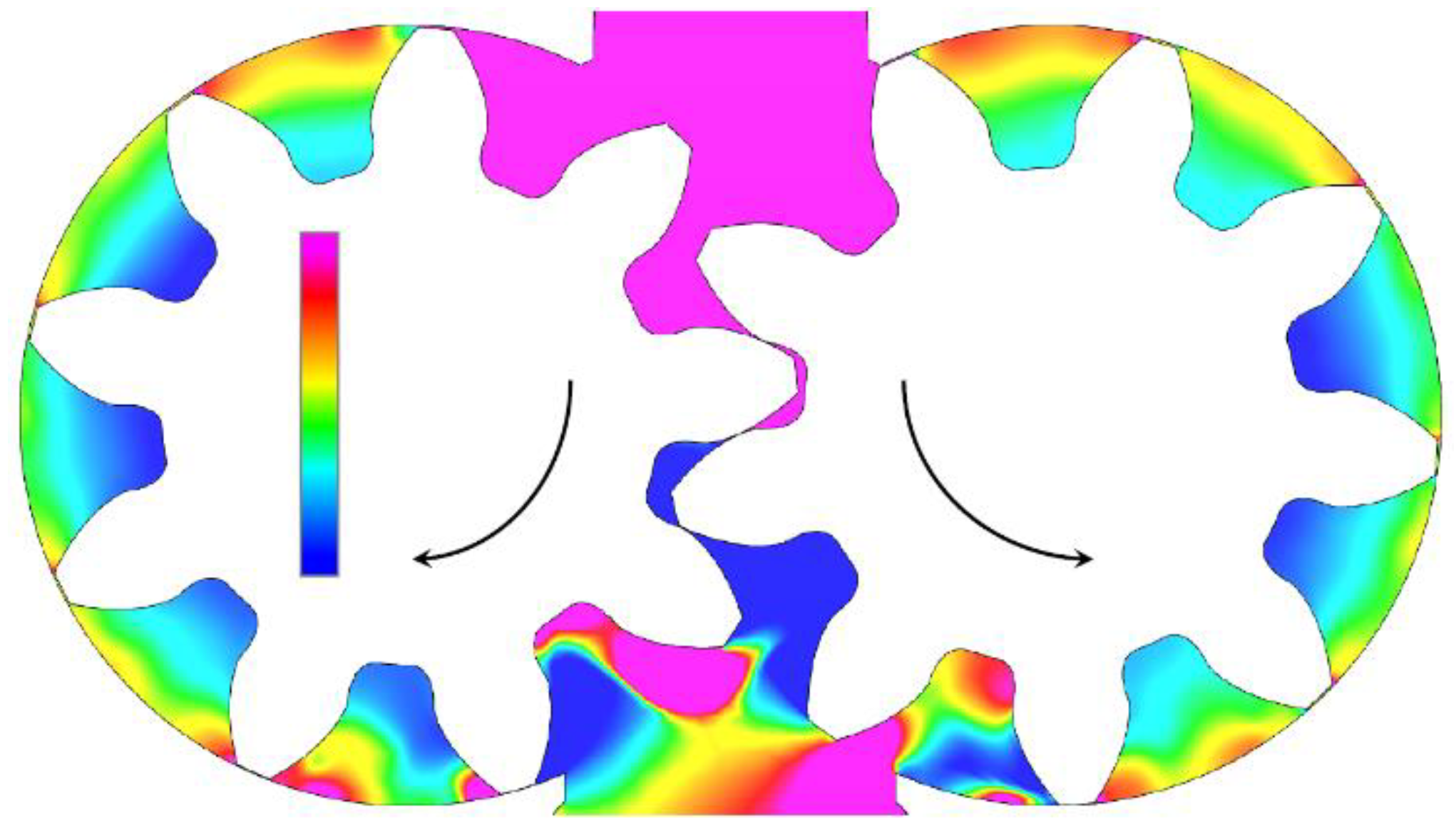
5.2. Evolution of the Free Air Fraction
- The derivative of the chamber volume becomes zero as tooth “c” completely exits the space between “a” and “b”;
- The connection area between the chamber and the suction volume has become quite large, since the distance between the tips of tooth “b” and “c” has significantly increased;
- The chamber becomes favorably oriented in the direction of the incoming flow.
5.3. Spur Gear Pump Type A
5.4. Different Ratio Axial Width/Diameter for Spur Gear Types B and C
- External gear diameter +/− 5%;
- Gear inter-axis +/− 5%;
- The same number of teeth;
- The same displacement.
5.5. Increment in the Diameter of the Inlet Port for Spur Gear Type D
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ketelsen, S.; Padovani, D.; Andersen, T.O.; Ebbesen, M.K.; Schmidt, L. Classification and review of pump-controlled differential cylinder drives. Energies 2019, 12, 1293. [Google Scholar] [CrossRef]
- Qu, S.; Fassbender, D.; Vacca, A.; Busquets, E. A high-efficient solution for electro-hydraulic actuators with energy regeneration capability. Energy 2021, 216, 119291. [Google Scholar] [CrossRef]
- Bedotti, A.; Pastori, M.; Casoli, P. Modelling and energy comparison of system layouts for a hydraulic excavator. Energy Procedia 2018, 148, 26–33. [Google Scholar] [CrossRef]
- Casoli, P.; Scolari, F.; Minav, T.; Rundo, M. Comparative Energy Analysis of a Load Sensing System and a Zonal Hydraulics for a 9-Tonne Excavator. Actuators 2020, 9, 39. [Google Scholar] [CrossRef]
- Fresia, P.; Rundo, M.; Padovani, D.; Altare, G. Combined speed control and centralized power supply for hybrid energy-efficient mobile hydraulics. Autom. Constr. 2022, 140, 104337. [Google Scholar] [CrossRef]
- Padovani, D.; Ketelsen, S.; Schmidt, L. Downsizing the electric motors of energy-efficient self-contained electro-hydraulic systems by using hybrid technologies. In Proceedings of the BATH/ASME 2020 Symposium on Fluid Power and Motion Control, Virtual, Online, 9–11 September 2020. [Google Scholar] [CrossRef]
- Altare, G.; Padovani, D.; Nervegna, N. A Commercial Excavator: Analysis, Modelling and Simulation of the Hydraulic Circuit; SAE Technical Paper 2012-01-2040; SAE: Warrendale, PA, USA, 2012. [Google Scholar] [CrossRef]
- Altare, G.; Padovani, D.; Nervegna, N. A Close-up View of a Load Sensing “Hybrid” PDCV. Trans. Jpn. Fluid Power Syst. Soc. 2013, 44, 64–73. [Google Scholar] [CrossRef]
- Casoli, P.; Scolari, F.; Vescovini, C.M.; Mesturini, D.; Zannoni, F. Excavator hydraulic circuit solution to reduce dissipations and fuel consumption. In E3S Web of Conferences; EDP Sciences: Les Ulis, France, 2021; Volume 312. [Google Scholar] [CrossRef]
- Gaiola, A.; Zardin, B.; Casoli, P.; Borghi, M.; Mazzali, F.; Pintore, F.; Fiorati, S. The Hydraulic Power Generation and Transmission on Agricultural Tractors: Feasible architectures to reduce dissipation and fuel consumption-Part 2. In E3S Web of Conferences; EDP Sciences: Les Ulis, France, 2020; Volume 197. [Google Scholar] [CrossRef]
- Bedotti, A.; Campanini, F.; Pastori, M.; Riccò, L.; Casoli, P. Energy saving solutions for a hydraulic excavator. Energy Procedia 2017, 126, 1099–1106. [Google Scholar] [CrossRef]
- Sun, F.; Ji, H.; Yang, S.; Li, C. Numerical Investigation on Pairing Solutions of Non-Positive Displacement Pumps and Internal Gear Pump for High-Speed Design. Fluids 2023, 8, 178. [Google Scholar] [CrossRef]
- Rundo, M. Theoretical flow rate in crescent pumps. Simul. Model. Pract. Theory 2017, 71, 1–14. [Google Scholar] [CrossRef]
- Marinaro, G.; Frosina, E.; Senatore, A. A Numerical Analysis of an Innovative Flow Ripple Reduction Method for External Gear Pumps. Energies 2021, 14, 471. [Google Scholar] [CrossRef]
- Ivantysyn, J.; Ivantysynova, M. Hydrostatic Pumps and Motors; Akademia Books International: New Delhi, India, 2001. [Google Scholar]
- Ransegnola, T.; Zhao, X.; Vacca, A. A comparison of helical and spur external gear machines for fluid power applications: Design and optimization. Mech. Mach. Theory 2019, 142, 103604. [Google Scholar] [CrossRef]
- Zardin, B.; Natali, E.; Borghi, M. Evaluation of the Hydro—Mechanical Efficiency of External Gear Pumps. Energies 2019, 12, 2468. [Google Scholar] [CrossRef]
- Rituraj, R.; Vacca, A.; Rigosi, M. Modeling and validation of hydro-mechanical losses in pressure compensated external gear machines. Mech. Mach. Theory 2021, 161, 104310. [Google Scholar] [CrossRef]
- Zhao, X.; Vacca, A. Multi-domain simulation and dynamic analysis of the 3D loading and micromotion of continuous-contact helical gear pumps. Mech. Syst. Signal Process. 2022, 163, 108116. [Google Scholar] [CrossRef]
- Ransegnola, T.; Vacca, A. Virtual Design and Analysis of the Balancing Element of an External Gear Machine Considering Cavitation and Mixed Lubrication Effects. Int. J. Fluid Power 2023, 24, 77–98. [Google Scholar] [CrossRef]
- Mazzei, P.; Frosina, E.; Senatore, A. Helical Gear Pump: A Comparison between a Lumped Parameter and a Computational Fluid Dynamics-Based Approaches. Fluids 2023, 8, 193. [Google Scholar] [CrossRef]
- Orlandi, F.; Muzzioli, G.; Milani, M.; Paltrinieri, F.; Montorsi, L. Development of a Numerical Approach for the CFD Simulation of a Gear Pump under Actual Operating Conditions. Fluids 2023, 8, 244. [Google Scholar] [CrossRef]
- Qu, S.; Zappaterra, F.; Vacca, A.; Liu, Z.; Busquets, E. Experimental Verification of An Electro-Hydraulic Actuation System Driven by An Integrated Electro-Hydraulic Unit. Int. J. Fluid Power 2023, 24, 327–360. [Google Scholar] [CrossRef]
- Padovani, D.; Ketelsen, S.; Hagen, D.; Schmidt, L. A self-contained electro-hydraulic cylinder with passive load-holding capability. Energies 2019, 12, 292. [Google Scholar] [CrossRef]
- Paltrinieri, F.; Milani, M.; Montorsi, L. Testing the performance of an innovative high speed external gear pump as a reliable hydraulic power unit for automotive robotized transmissions. In AIP Conference Proceedings; AIP Publishing: Melville, NY, USA, 2019; Volume 2191. [Google Scholar] [CrossRef]
- Chao, Q.; Xu, Z.; Tao, J.; Liu, C.; Zhai, J. Cavitation in a high-speed aviation axial piston pump over a wide range of fluid temperatures. Proc. Inst. Mech. Eng. Part A J. Power Energy 2022, 236, 727–737. [Google Scholar] [CrossRef]
- Buono, D.; Cola, F.D.S.D.; Senatore, A.; Frosina, E.; Buccilli, G.; Harrison, J. Modelling Approach on a Gerotor Pump Working in Cavitation Conditions. Energy Procedia 2016, 101, 701–709. [Google Scholar] [CrossRef]
- Del Campo, D.; Castilla, R.; Raush, G.A.; Gamez Montero, P.J.; Codina, E. Numerical analysis of external gear pumps including cavitation. J. Fluids Eng. 2012, 134, 081105. [Google Scholar] [CrossRef]
- Frosina, E.; Senatore, A.; Rigosi, M. Study of a high-pressure external gear pump with a computational fluid dynamic modeling approach. Energies 2017, 10, 1113. [Google Scholar] [CrossRef]
- Mithun, M.-G.; Koukouvinis, P.; Karathanassis, I.K.; Gavaises, M. Numerical simulation of three-phase flow in an external gear pump using immersed boundary approach. Appl. Math. Model. 2019, 72, 682–699. [Google Scholar] [CrossRef]
- Altare, G.; Rundo, M. Computational Fluid Dynamics Analysis of Gerotor Lubricating Pumps at High-Speed: Geometric Features Influencing the Filling Capability. J. Fluids Eng. 2016, 138, 111101. [Google Scholar] [CrossRef]
- Rundo, M.; Altare, G.; Casoli, P. Simulation of the filling capability in vane pumps. Energies 2019, 12, 283. [Google Scholar] [CrossRef]
- Corvaglia, A.; Rundo, M.; Casoli, P.; Lettini, A. Evaluation of Tooth Space Pressure and Incomplete Filling in External Gear Pumps by Means of Three-Dimensional CFD Simulations. Energies 2021, 14, 342. [Google Scholar] [CrossRef]
- Borghi, M.; Zardin, B. Axial balance of external gear pumps and motors: Modelling and discussing the influence of elastohydrodynamic lubrication in the axial gap. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Houston, TX, USA, 13–19 November 2015. [Google Scholar] [CrossRef]
- Torrent, M.; Gamez-Montero, P.J.; Codina, E. Motion Modelling of the Floating Bushing in an External Gear Pump Using Dimensional Analysis. Actuators 2023, 12, 338. [Google Scholar] [CrossRef]
- Vacca, A.; Guidetti, M. Modelling and experimental validation of external spur gear machines for fluid power applications. Simul. Model. Pract. Theory 2011, 19, 2007–2031. [Google Scholar] [CrossRef]
- Dhar, S.; Vacca, A.; Lettini, A. A novel fluid-structure-thermal interaction model for the analysis of the lateral lubricating gap flow in external gear machines. In Proceedings of the ASME/BATH 2013 Symposium on Fluid Power and Motion Control, Sarasota, FL, USA, 6–9 October 2013. [Google Scholar] [CrossRef]
- Rundo, M.; Squarcini, R.; Furno, F. Modelling of a Variable Displacement Lubricating Pump with Air Dissolution Dynamics. SAE Int. J. Engines 2018, 11, 111–126. [Google Scholar] [CrossRef]
- Stuppioni, U.; Suman, A.; Pinelli, M.; Blum, A. Computational fluid dynamics modeling of gaseous cavitation in lubricating vane pumps: An approach based on dimensional analysis. J. Fluids Eng. 2020, 142, 071206. [Google Scholar] [CrossRef]
- Rundo, M.; Altare, G. Lumped Parameter and Three-Dimensional Computational Fluid Dynamics Simulation of a Variable Displacement Vane Pump for Engine Lubrication. J. Fluids Eng. 2018, 140, 061101. [Google Scholar] [CrossRef]
- Schlichting, H.; Gersten, K. Onset of Turbulence (Stability Theory). In Boundary-Layer Theory; Springer: Berlin, Germany, 2016; pp. 415–416. [Google Scholar] [CrossRef]
- Ding, H.; Visser, F.C.; Jiang, Y.; Furmanczyk, M. Demonstration and validation of a 3D CFD simulation tool predicting pump performance and cavitation for industrial applications. J. Fluids Eng. 2011, 133, 011101. [Google Scholar] [CrossRef]
- Altare, G.; Rundo, M. Advances in simulation of gerotor pumps: An integrated approach. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2017, 231, 1221–1236. [Google Scholar] [CrossRef]





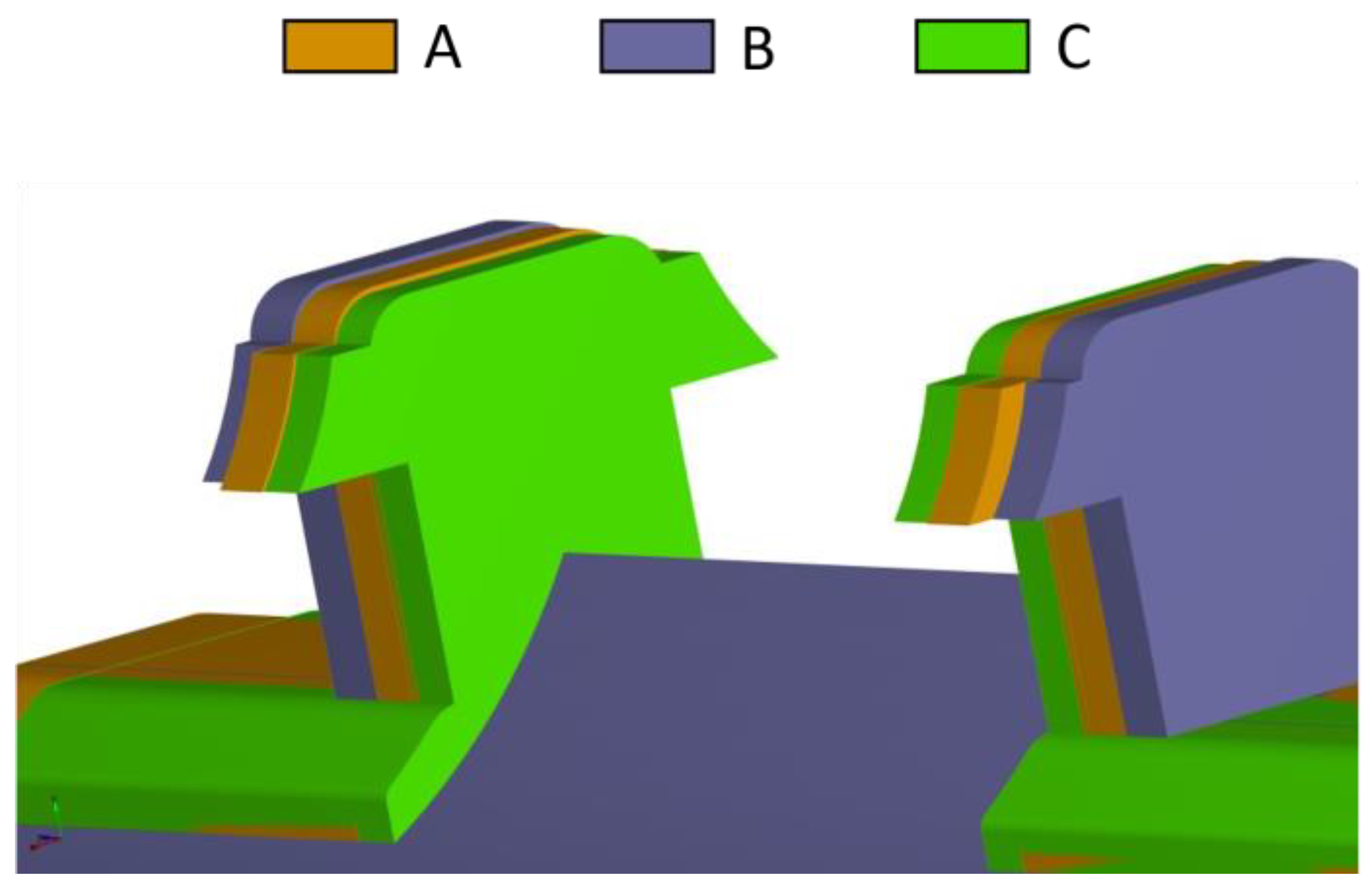


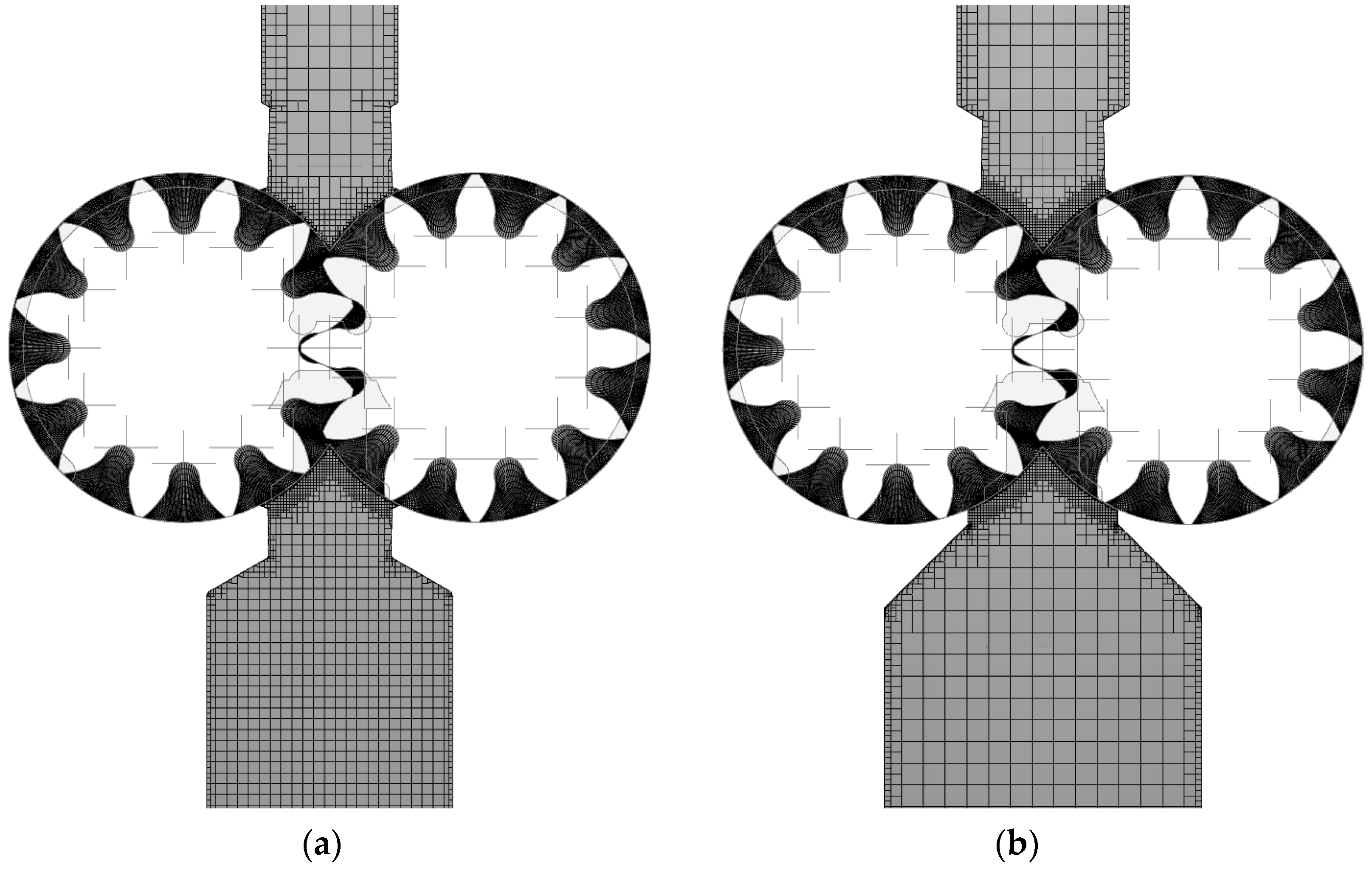
| Configuration | Description |
|---|---|
| Helical gear | Reference pump |
| Spur gear A | Standard straight-teeth pump |
| Spur gear B | Higher ratio thickness/diameter with respect to spur gear A |
| Spur gear C | Lower ratio thickness/diameter with respect to spur gear A |
| Spur gear D | As spur gear A with larger inlet port |
| Configuration | Volumetric Efficiency | CPU Time (h/rev) |
|---|---|---|
| 0.5 deg—residual drop 0.1 | 94.3% | 3.5 |
| 0.5 deg—residual drop 0.01 | 96.7% | 16.5 |
| 1 deg—residual drop 0.1 | 90.3% | 3 |
| 1 deg—residual drop 0.01 | 96.7% | 7 |
| Volume | Number of Cells |
|---|---|
| Two rotors | 792,000 |
| Delivery volume | 760,143 |
| Inlet volume | 181,732 |
| Suction duct | 22,800 |
| Total | 1,756,675 |
| Quantity | Transducer | Range | Accuracy |
|---|---|---|---|
| Pressures P1, P2 | Trafag 8253 | 0 ÷ 400 bar | ±0.3% F.S. |
| Pressures P3, P4 | Trafag 8254 | −1 ÷ 4 bar | ±0.3% F.S. |
| Flow rate | VSE VS 4 | 1 ÷ 250 L/min | ±0.3% Reading |
| Parameter | A Version | B Version | C Version |
|---|---|---|---|
| Minimum flow area | - | +6% | −7% |
| Rotor–inlet interface area | - | +6% | −7% |
| Tooth–flank gap | 22 μm | 19 μm | 16 μm |
| Tip clearance on inlet edge | 7 μm | 6 μm | 4 μm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Corvaglia, A.; Rundo, M.; Bonati, S.; Rigosi, M. Simulation and Experimental Activity for the Evaluation of the Filling Capability in External Gear Pumps. Fluids 2023, 8, 251. https://doi.org/10.3390/fluids8090251
Corvaglia A, Rundo M, Bonati S, Rigosi M. Simulation and Experimental Activity for the Evaluation of the Filling Capability in External Gear Pumps. Fluids. 2023; 8(9):251. https://doi.org/10.3390/fluids8090251
Chicago/Turabian StyleCorvaglia, Alessandro, Massimo Rundo, Sara Bonati, and Manuel Rigosi. 2023. "Simulation and Experimental Activity for the Evaluation of the Filling Capability in External Gear Pumps" Fluids 8, no. 9: 251. https://doi.org/10.3390/fluids8090251
APA StyleCorvaglia, A., Rundo, M., Bonati, S., & Rigosi, M. (2023). Simulation and Experimental Activity for the Evaluation of the Filling Capability in External Gear Pumps. Fluids, 8(9), 251. https://doi.org/10.3390/fluids8090251








