Modeling and Visualization of Coolant Flow in a Fuel Rod Bundle of a Small Modular Reactor
Abstract
1. Introduction
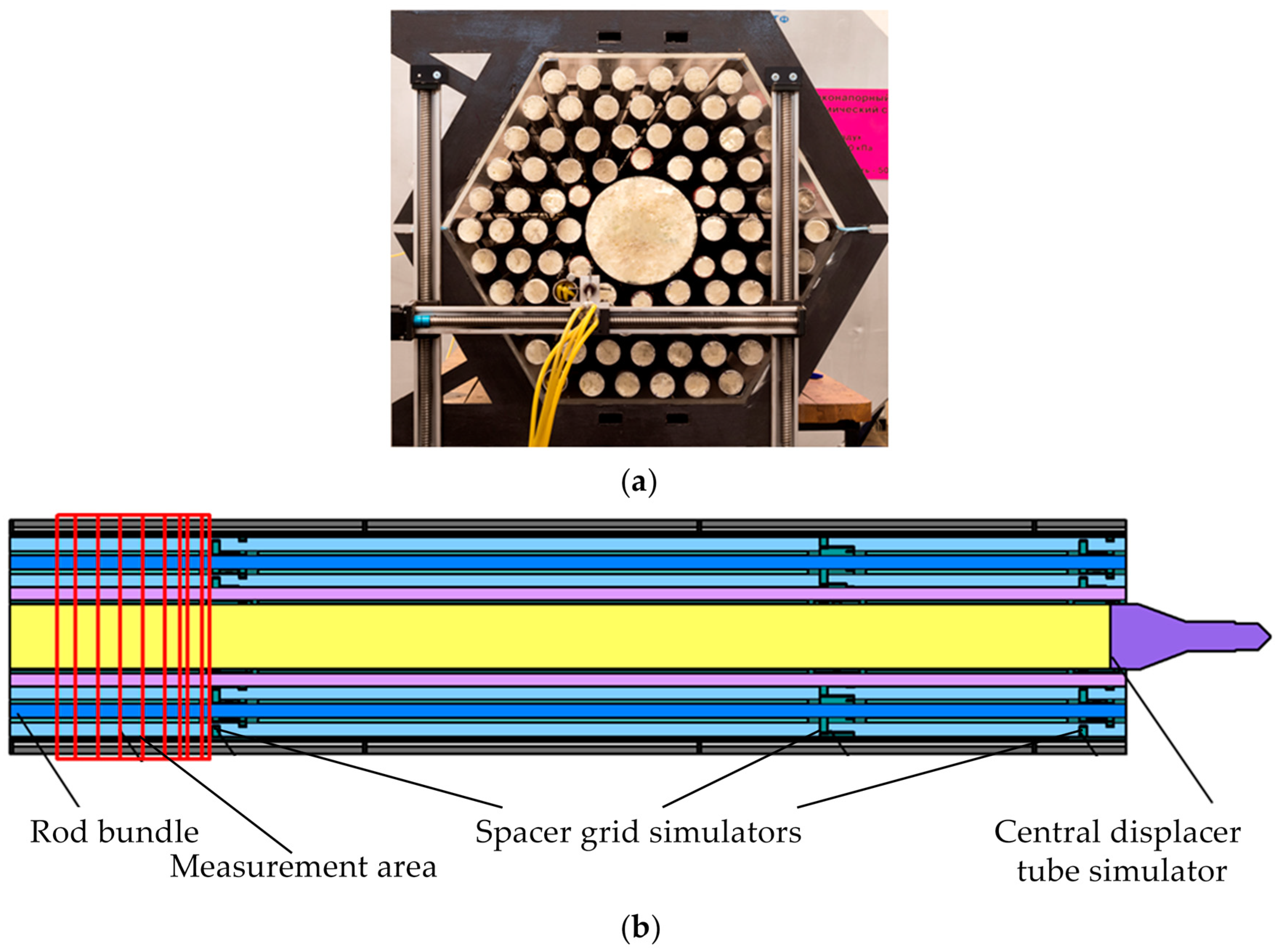
2. Research Facility and Experimental Model
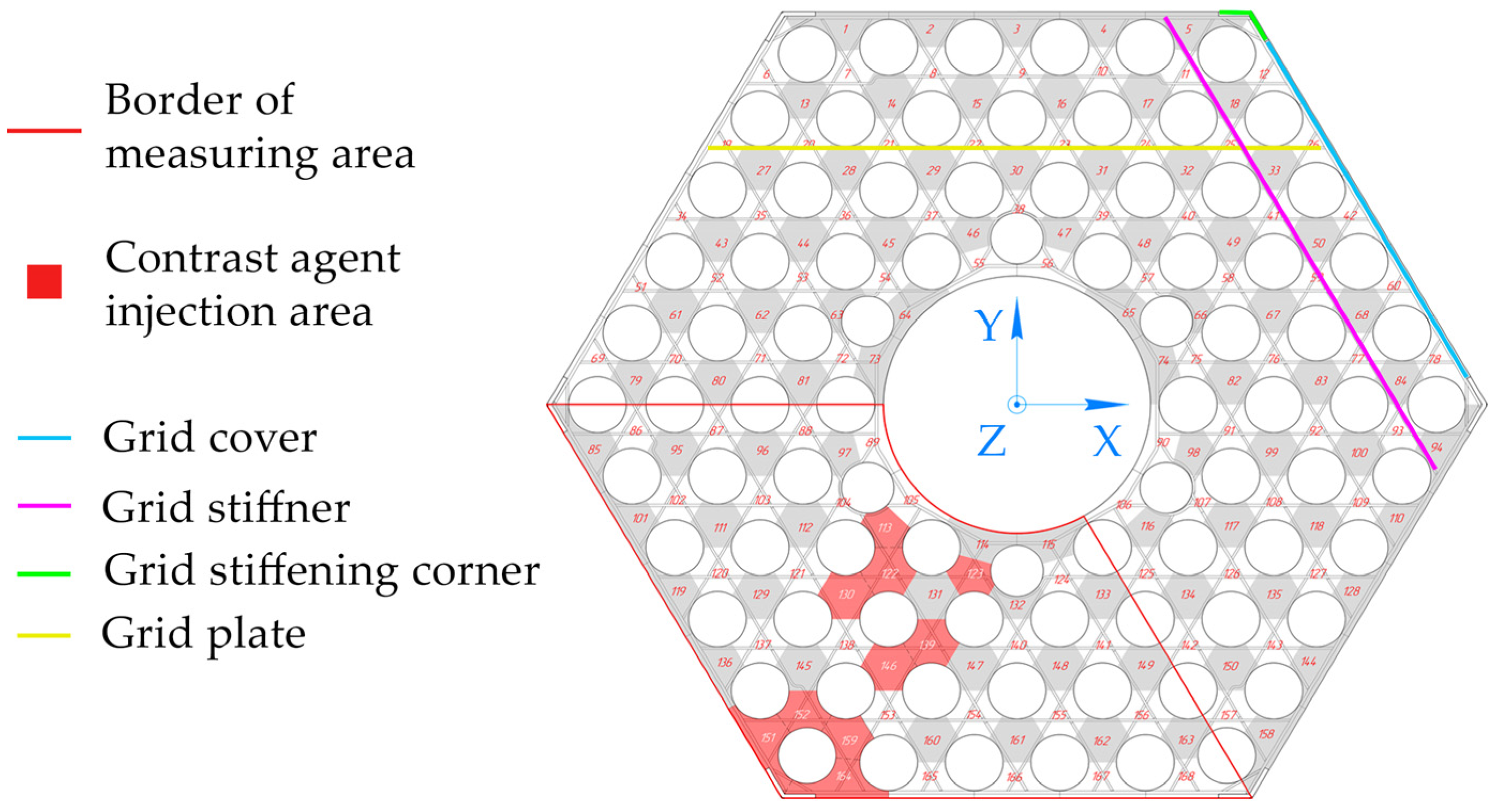
3. The Representativeness of the Study
4. Experimental Methodology
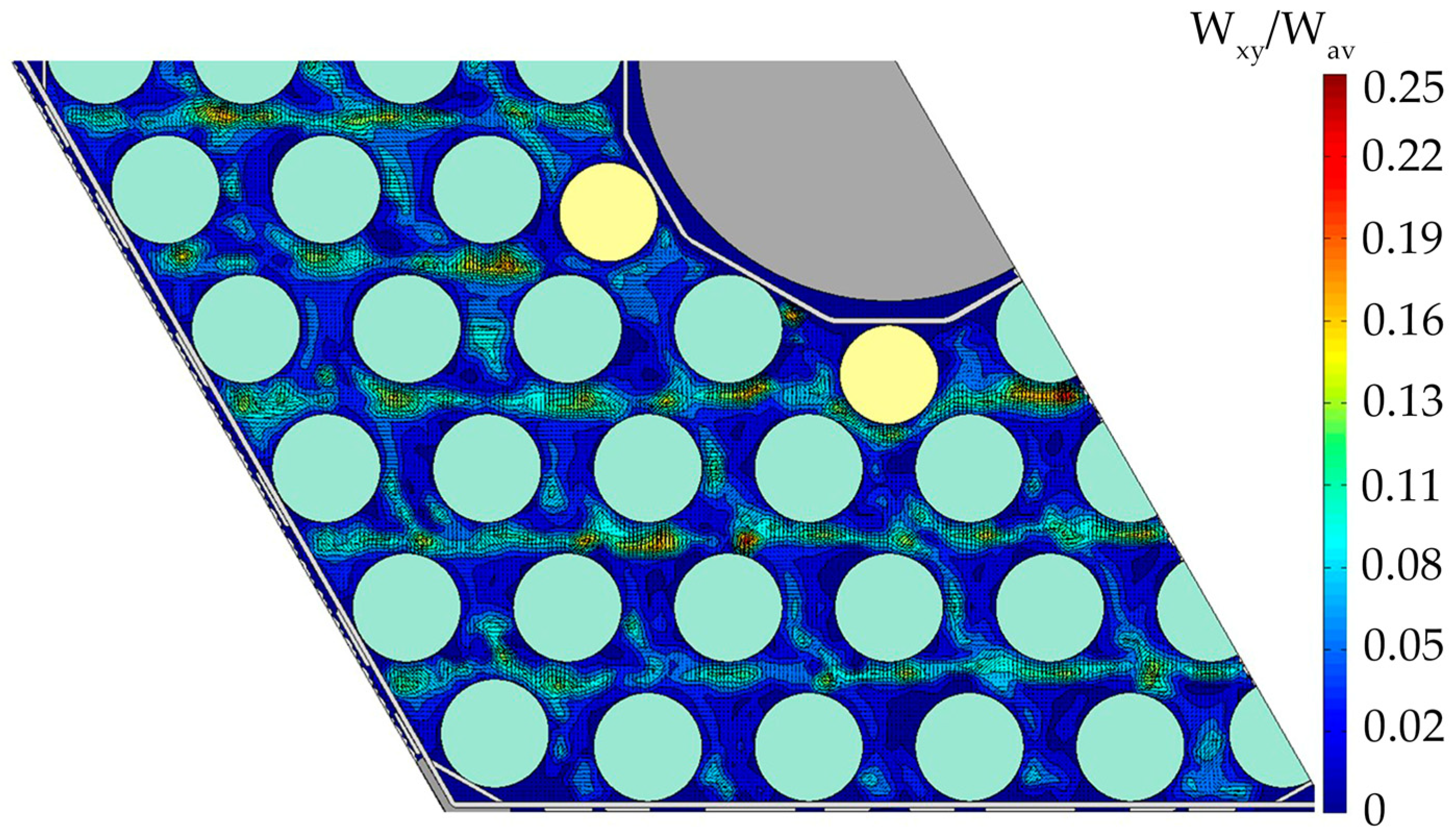
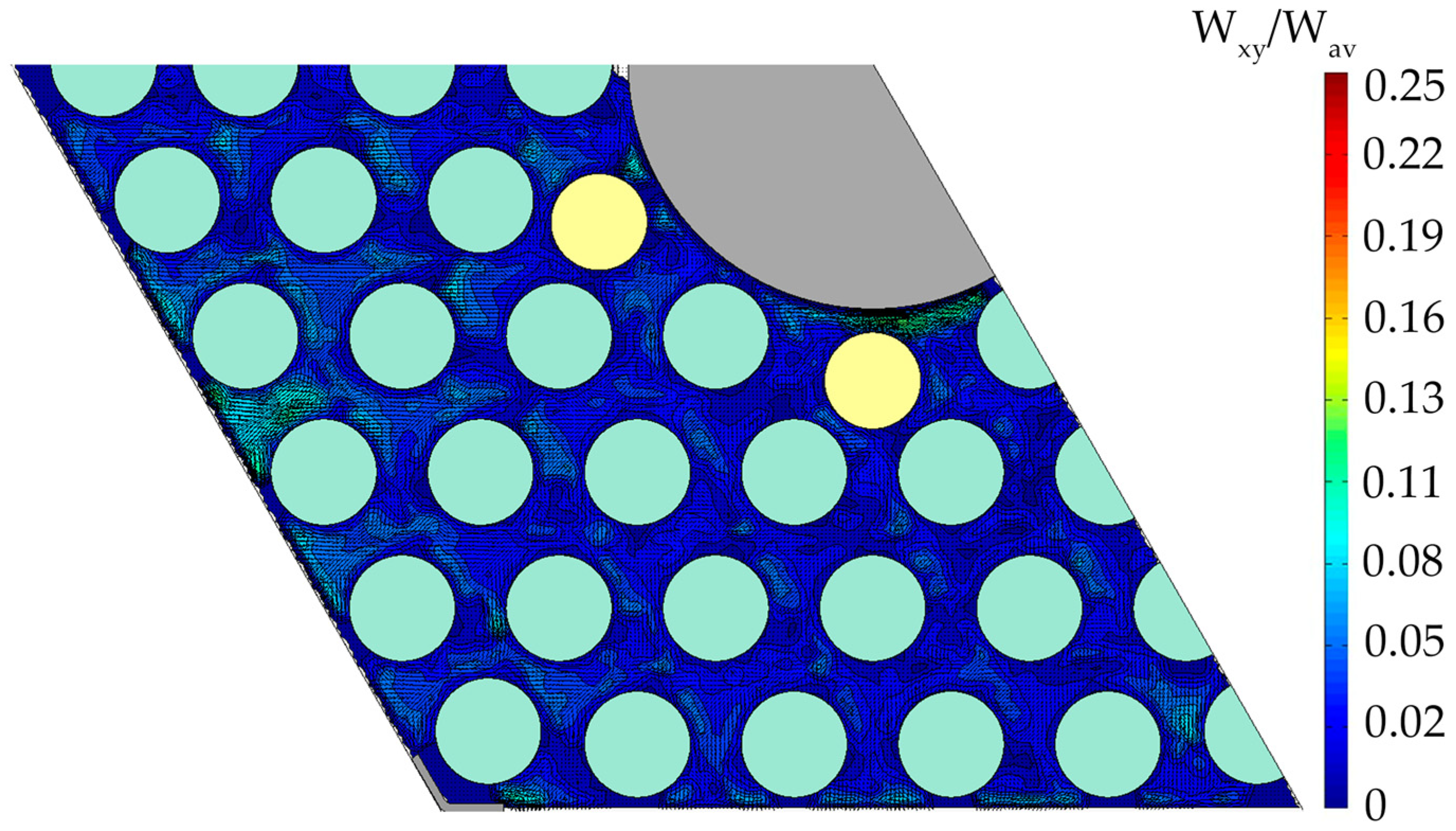
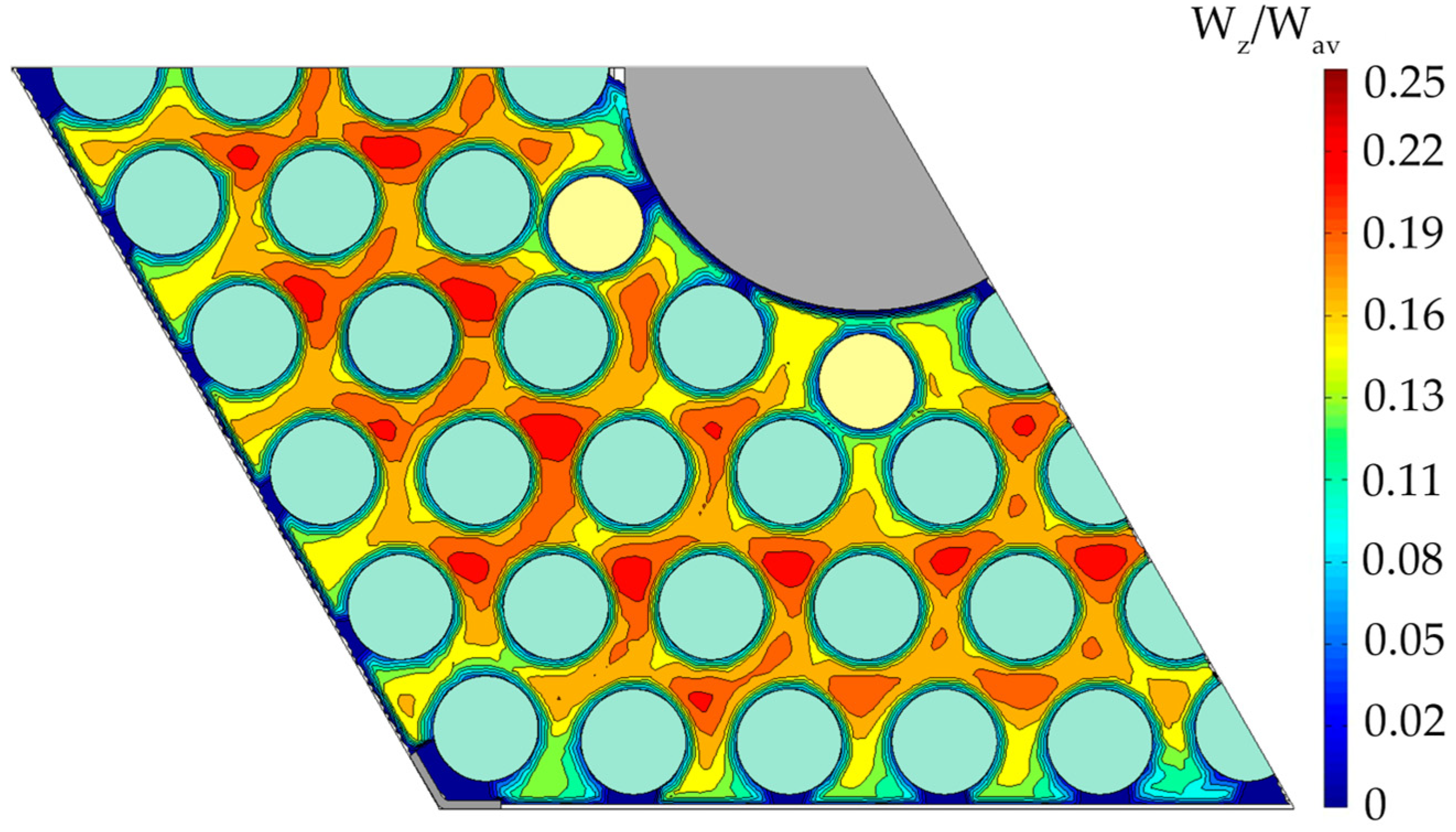
5. The Results of the Study on the Hydrodynamics of the Coolant Using the Pneumometry Technique
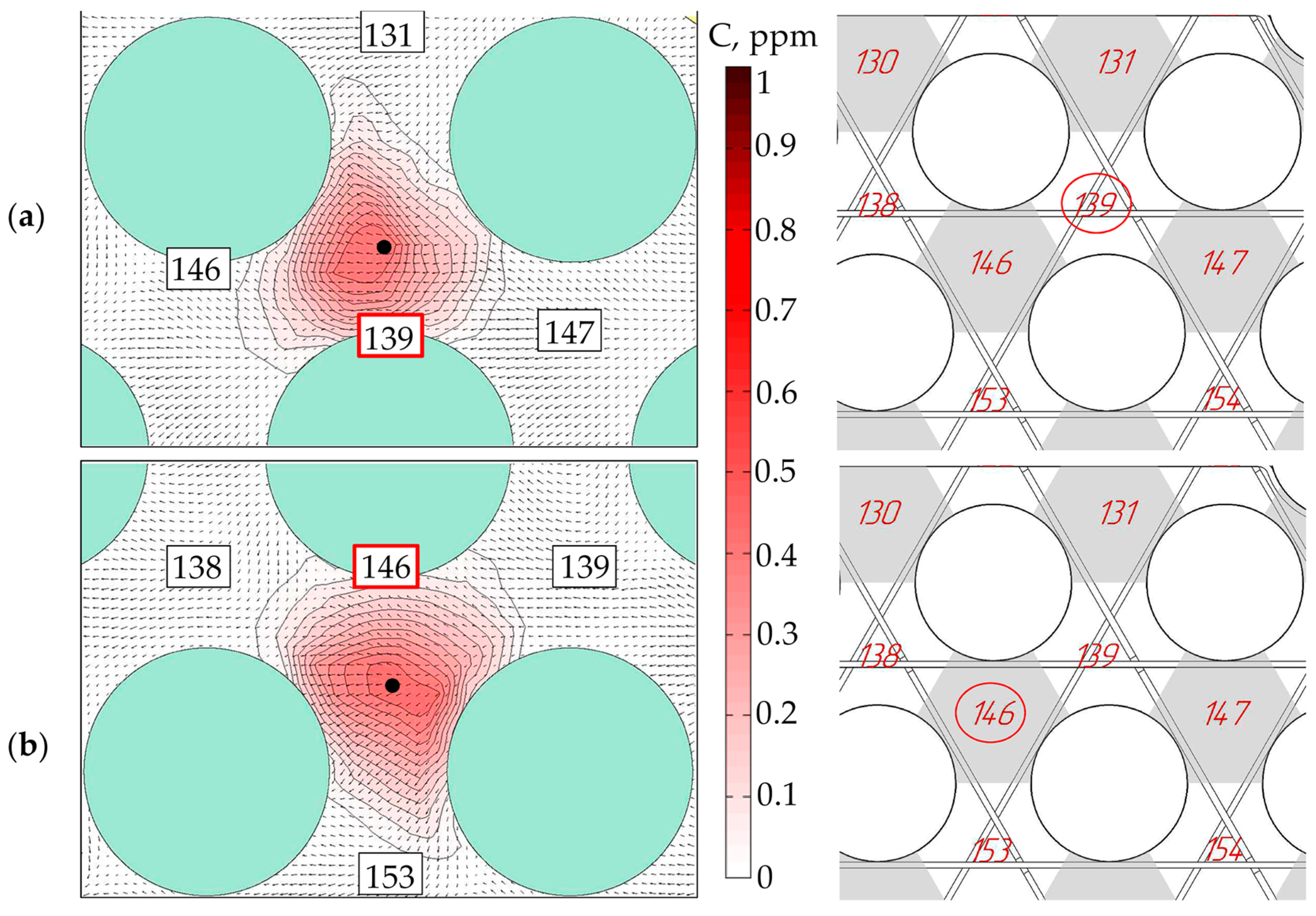
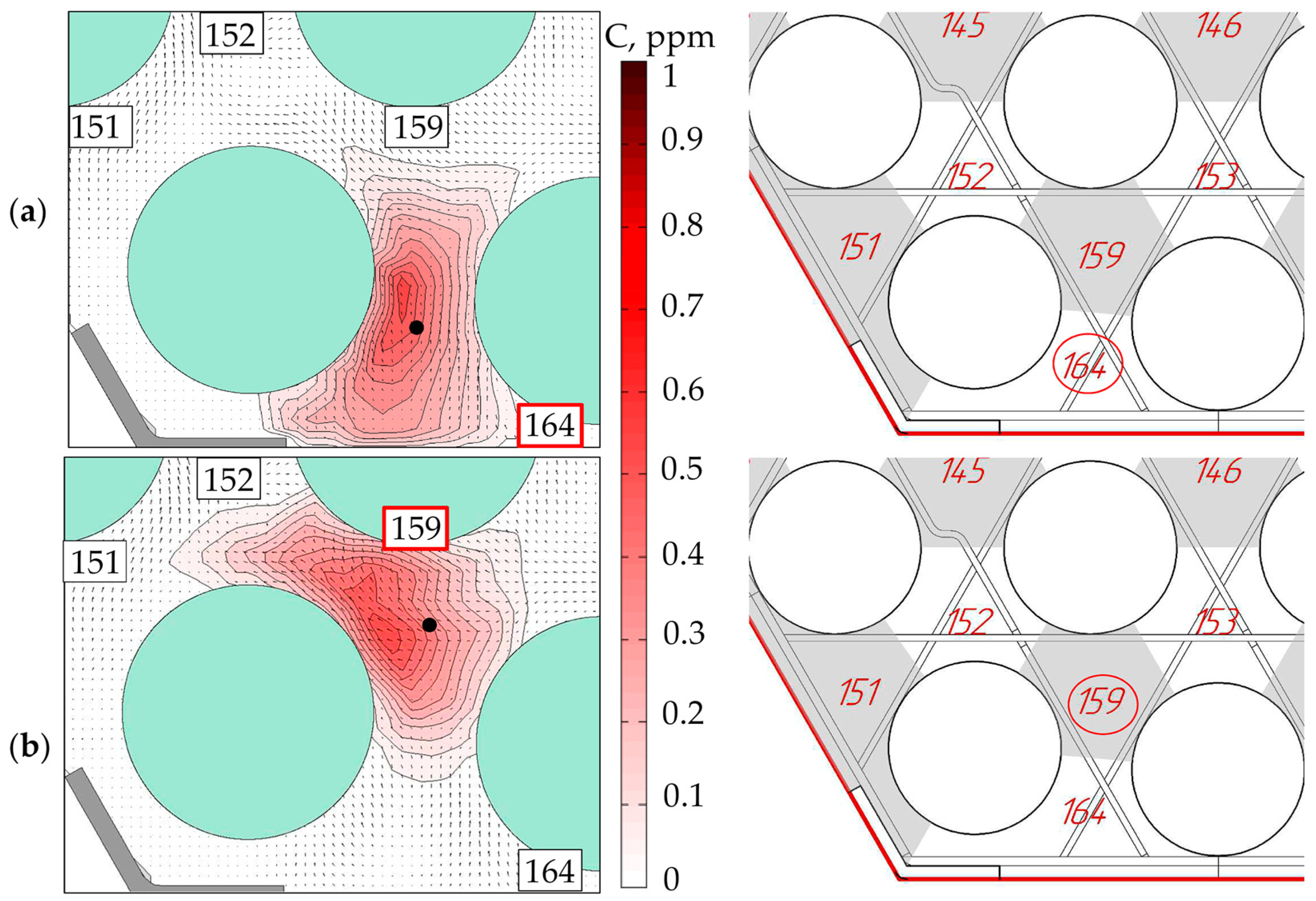
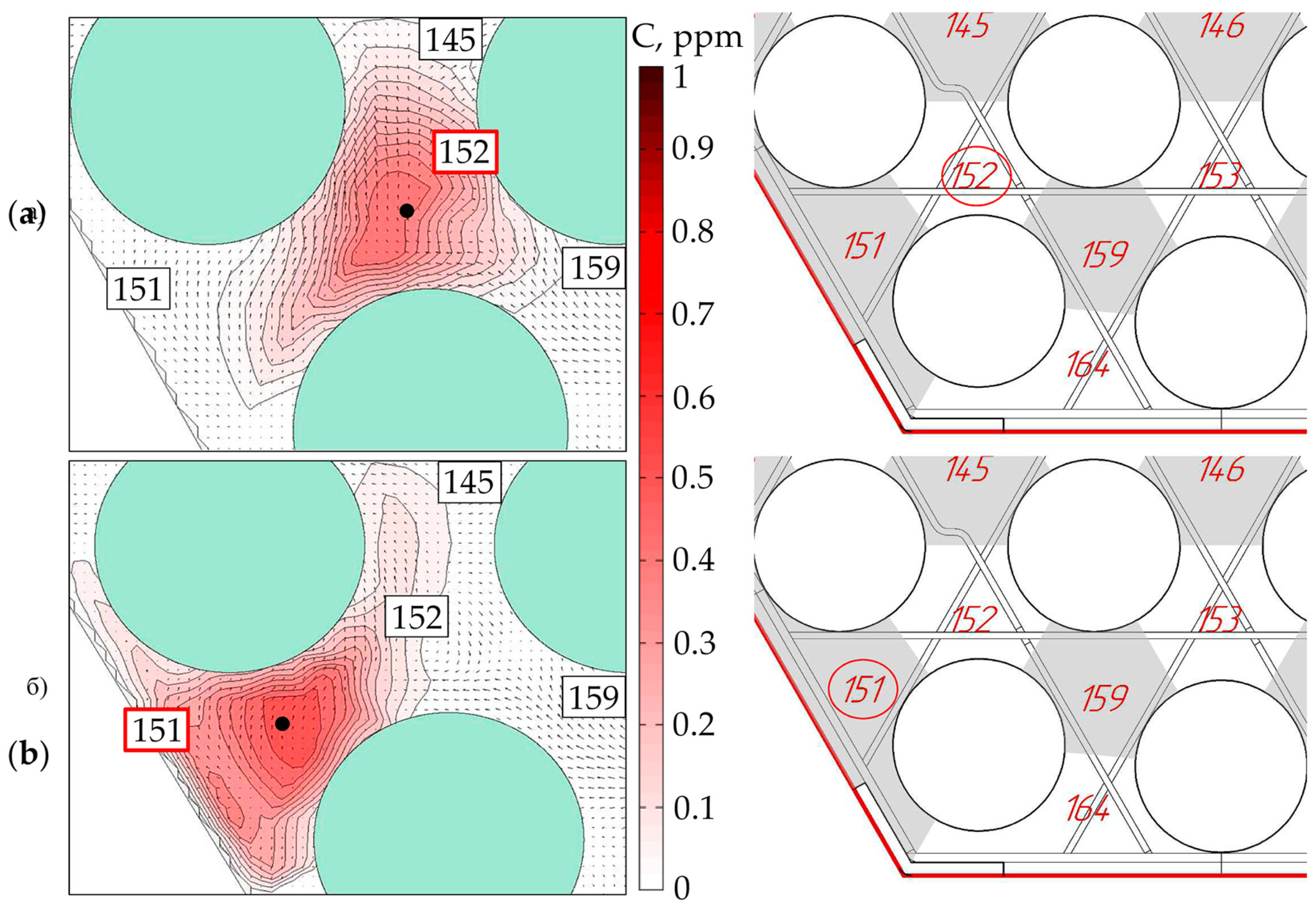
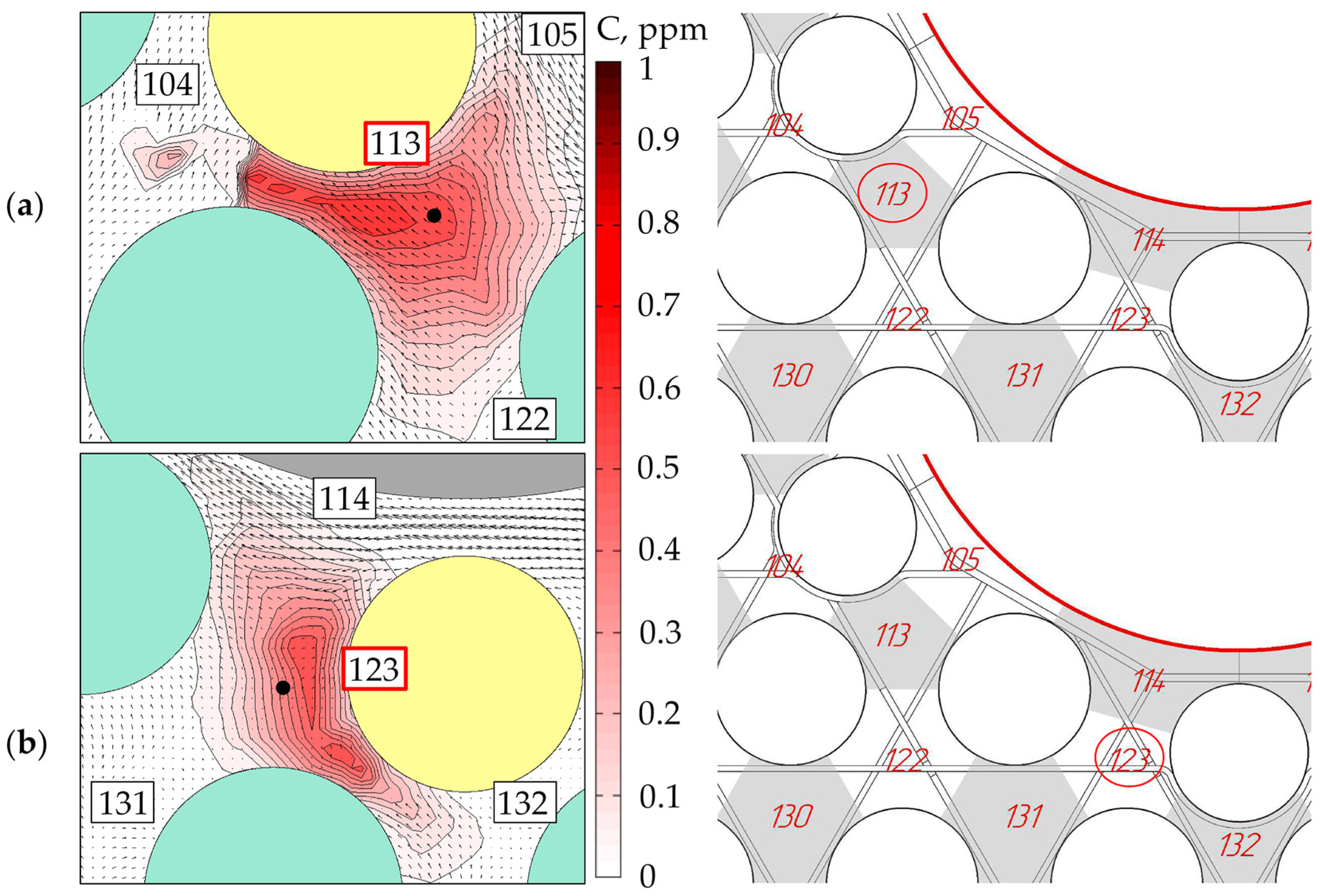
6. The Results of the Study on the Hydrodynamics of the Coolant Using the Contrast Agent Injection Method
7. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Petrunin, V.V.; Sheshina, N.V.; Fateev, S.A.; Kurachenkov, A.V.; Shchekin, D.V.; BrykaloV, S.M.; Bezrukov, A.A. Scientific and technical aspects of developing a RITM-200N innovative reactor for SNPPs. At. Energy 2023, 134, 1–10. [Google Scholar] [CrossRef]
- Filippov, S.P.; Veselov, F.V.; Pankrushina, T.G. Prospects of SNPPs in the electric power industry. At. Energy 2023, 134, 11–20. [Google Scholar] [CrossRef]
- Zverev, D.L.; Fadeev, Y.P.; Pakhomov, A.N.; Galitskikh, V.Y.; Polunichev, V.I.; Veshnyakov, K.B.; Kabin, S.V.; Turusov, A.Y. Reactor Installations for Nuclear Icebreakers: Origination Experience and Current Status. At. Energy 2020, 129, 18–26. [Google Scholar] [CrossRef]
- Belyaev, V.M.; Bol’shukhin, M.A.; Pakhomov, A.N.; Khizbullin, A.M.; Lepekhin, A.N.; Polunichev, V.I.; Veshnyakov, K.B.; Sokolov, A.N.; Turusov, A.Y. The World’s First Floating NPP: Origination and Direction of Future Development. At. Energy 2020, 129, 27–34. [Google Scholar] [CrossRef]
- Zakharychev, A.A.; Iksanova, G.S.; Kupriyanov, A.V.; Osin, A.B.; Petrunin, V.V.; Samoilov, O.B.; Shipov, D.L. Methodological Issues and Results of Experimental and Computational Studies of Critical Heat Flows in RITM-200 Reactor Fuel Assemblies for Small NPP. At. Energy 2021, 130, 63–68. [Google Scholar] [CrossRef]
- Korolev, V.I. Analysis of New Technical Solutions for the RITM-200 Reactor Plant in Project 22220 Universal Nuclear Icebreakers. Vestn. Gos. Univ. Morskogo Rechn. Flot. Im. Admirala S. O. Makarova 2022, 14, 945–960. [Google Scholar] [CrossRef]
- Tan, S.; Cheng, S.; Wang, K.; Liu, X.; Cheng, H.; Wang, J. The development of micro and small modular reactor in the future energy market. Front. Energy Res. 2023, 11, 1149127. [Google Scholar] [CrossRef]
- Crețulescu, A. Small Modular Reactors in Romania’s Energy Future. Two Essential Perspectives: Capital Costs and Public Perception. Proc. Int. Conf. Bus. Excell. 2024, 18, 1765–1775. [Google Scholar] [CrossRef]
- Deng, C.; Zhu, S.; He, Y.; Wu, Y.; He, K.; Zhang, J.; Su, G. Analysis of Gas-Cooled Micro Modular Reactor (MMR) Fuel. J. Nucl. Mater. 2024, 598, 155191. [Google Scholar] [CrossRef]
- Trupp, A.C.; Azad, R.S. The Structure of Turbulent Flow in Triangular Array Rod Bundles. Nucl. Eng. Des. 1975, 32, 47–84. [Google Scholar] [CrossRef]
- Caraghiaur, D. Experimental Study and Modeling of Spacer Grid Influence on Flow in Nuclear Fuel Assemblies; Technical Report; KTH Stockholm: Stockholm, Sweden, 2009. [Google Scholar]
- Bakosi, J.; Christon, M.A.; Lowrie, R.B.; Pritchett-Sheats, L.A.; Nourgaliev, R.R. Large-eddy simulations of turbulent flow for grid-to-rod fretting in nuclear reactors. Nucl. Eng. Des. 2013, 262, 544–561. [Google Scholar] [CrossRef]
- Peña-Monferrer, C.; Muñoz-Cobo, J.L.; Chiva, S. CFD turbulence study of PWR spacer-grids in a rod bundle. Sci. Technol. Nucl. Install. 2014, 2014, 635651. [Google Scholar] [CrossRef]
- Sergeev, D.A.; Kandaurov, A.A.; Troitskaya, Y.I. The particular use of PIV methods for the modelling of heat and hydrophysical processes in the nuclear power plants. J. Phys. Conf. Ser. 2017, 891, 012088. [Google Scholar] [CrossRef]
- Yu, H.; Cai, J.; He, S.; Li, X. Analysis of neutron physics and thermal hydraulics for fuel assembly of small modular reactor loaded with ATFs. Ann. Nucl. Energy 2020, 152, 107957. [Google Scholar] [CrossRef]
- He, S.; Cai, J.; Chen, Z.; Liu, R. Thermal hydraulic analysis of accident tolerant fuels for Reactivity-Initiated-Accident in PWR with different coolant channel geometries. Nucl. Eng. Des. 2019, 351, 131–142. [Google Scholar] [CrossRef]
- Cong, T.; Zhang, X. Numerical Study of Bubble Coalescence and Breakup in the Reactor Fuel Channel with a Vaned Grid. Energies 2018, 11, 256. [Google Scholar] [CrossRef]
- Tian, Z.; Yang, L.; Han, S.; Yuan, X.; Lu, H.; Li, S.; Liu, L. Numerical Investigation on the Flow Characteristics in a 17 × 17 Full-Scale Fuel Assembly. Energies 2020, 13, 397. [Google Scholar] [CrossRef]
- Lee, I.S.; Yoon, D.H.; Bang, Y.S.; Kim, T.H.; Kim, Y.C. Assessment of Realistic Departure from Nucleate Boiling Ratio (DNBR) Considering Uncertainty Quantification of Core Flow Asymmetry. Energies 2021, 14, 1504. [Google Scholar] [CrossRef]
- Vlasov, M.N.; Merinov, I.G. Application of an Integral Turbulence Model to Close the Model of an Anisotropic Porous Body as Applied to Rod Structures. Fluids 2022, 7, 77. [Google Scholar] [CrossRef]
- Kim, K.; Kim, W.-S.; Choi, H.-S.; Seol, H.; Lim, B.-J.; Euh, D.-J. An Experimental Evaluation of the APR1000 Core Flow Distribution Using a 1/5 Scale Model. Energies 2024, 17, 2714. [Google Scholar] [CrossRef]
- Beliavskii, S.; Alhassan, S.; Danilenko, V.; Karvan, R.; Nesterov, V. Effect of changing the outer fuel element diameter on ther-mophysical parameters of KLT-40S reactor unit. Ann. Nucl. Energy 2023, 190, 109877. [Google Scholar] [CrossRef]
- Hidayatullah, H.; Susyadi, S.; Hadid Subki, M. Design and technology development for small modular reactors—Safety expectations, prospects and impediments of their deployment. Prog. Nucl. Energy 2015, 79, 127–135. [Google Scholar] [CrossRef]
- Bhowmik, P.K.; Sabharwall, P.; Johnson, J.T.; Retamales, M.E.T.; Wang, C.; O’Brien, J.E.; Lietwiler, C.; Wu, Q. Scaling methodologies and similarity analysis for thermal hydraulics test facility development for water-cooled small modular reactor. Nucl. Eng. Des. 2024, 424, 113235. [Google Scholar] [CrossRef]
- Ren, L.; Minjun, P.; Genglei, X.; Lin, S. The natural circulation flow characteristic of the core in floating nuclear power plant in rolling motion. Ann. Nucl. Energy 2020, 142, 107385. [Google Scholar] [CrossRef]
- Alam, S.B.; de Oliveira, R.G.G.; Goodwin, C.S.; Parks, G.T. Coupled neutronic/thermal-hydraulic hot channel analysis of high power density civil marine SMR cores. Ann. Nucl. Energy 2019, 127, 400–411. [Google Scholar] [CrossRef]
- Samoilov, O.B.; Shipov, D.L.; Kupriyanov, A.V.; Sholin, E.V.; Vishneva, T.Y.; Molodtsov, A.A.; Osin, A.B.; Dmitriev, S.M.; Khrobostov, A.E.; Doronkov, D.V.; et al. Efficiency studies of heat-transfer intensifier grids in TVSA-T model fuel assemblies. At. Energy 2020, 128, 17–23. [Google Scholar] [CrossRef]
- Dmitriev, S.M.; Gerasimov, A.V.; Dobrov, A.A.; Doronkov, D.V.; Pronin, A.N.; Solntsev, D.N.; Khrobostov, A.E.; Shvetsov, Y.K.; Shipov, D.L. Hydrodynamics and mixing of a coolant in the core of the VVER with fuel assemblies of different designs. Thermophys. Aeromech. 2019, 26, 845–860. [Google Scholar] [CrossRef]
- Gerasimov, A.V.; Dmitriev, S.M.; Dobrov, A.A.; Doronkov, D.V.; Pronin, A.N.; Ryazanov, A.V.; Solntsev, D.N.; Khrobostov, A.E. Computational-experimental investigations of the processes of motion of a coolant flow in the region of the guide channel behind mixing grids of fuel assemblies. J. Eng. Phys. Thermophys. 2020, 93, 145–154. [Google Scholar] [CrossRef]
- Samoilov, O.B.; Noskov, A.S.; Shipov, D.L.; Dmitriev, S.M.; Dobrov, A.A.; Doronkov, D.V.; Legchanov, M.A.; Pronin, A.N.; Solntsev, D.N.; Sorokin, V.D.; et al. Hydrodynamic features of the flow downstream from the mixing spacer grid in a Kvadrat fuel assembly in PWRs. Therm. Eng. 2019, 66, 243–248. [Google Scholar] [CrossRef]
- Dmitriev, S.M.; Dobrov, A.A.; Doronkov, D.V.; Doronkova, D.S.; Legchanov, M.A.; Pronin, A.N.; Ryazanov, A.V.; Khrobostov, A.E. Studies of the hydrodynamics of the coolant behind the mixing intensifier grids of the fuel assemblies of a PWR reactor. High Temp. 2022, 60, 358–365. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dmitriev, S.; Demkina, T.; Dobrov, A.; Doronkov, D.; Kuritsin, D.; Nikolaev, D.; Pronin, A.; Riazanov, A.; Solntsev, D. Modeling and Visualization of Coolant Flow in a Fuel Rod Bundle of a Small Modular Reactor. Fluids 2024, 9, 235. https://doi.org/10.3390/fluids9100235
Dmitriev S, Demkina T, Dobrov A, Doronkov D, Kuritsin D, Nikolaev D, Pronin A, Riazanov A, Solntsev D. Modeling and Visualization of Coolant Flow in a Fuel Rod Bundle of a Small Modular Reactor. Fluids. 2024; 9(10):235. https://doi.org/10.3390/fluids9100235
Chicago/Turabian StyleDmitriev, Sergei, Tatiyana Demkina, Aleksandr Dobrov, Denis Doronkov, Daniil Kuritsin, Danil Nikolaev, Alexey Pronin, Anton Riazanov, and Dmitriy Solntsev. 2024. "Modeling and Visualization of Coolant Flow in a Fuel Rod Bundle of a Small Modular Reactor" Fluids 9, no. 10: 235. https://doi.org/10.3390/fluids9100235
APA StyleDmitriev, S., Demkina, T., Dobrov, A., Doronkov, D., Kuritsin, D., Nikolaev, D., Pronin, A., Riazanov, A., & Solntsev, D. (2024). Modeling and Visualization of Coolant Flow in a Fuel Rod Bundle of a Small Modular Reactor. Fluids, 9(10), 235. https://doi.org/10.3390/fluids9100235






