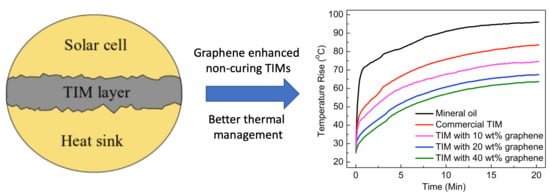
Non-Curing Thermal Interface Materials with Graphene Fillers for Thermal Management of Concentrated Photovoltaic Solar Cells
Abstract
1. Introduction
2. Material Preparation and Characterization
3. Solar Cell Testing Procedures
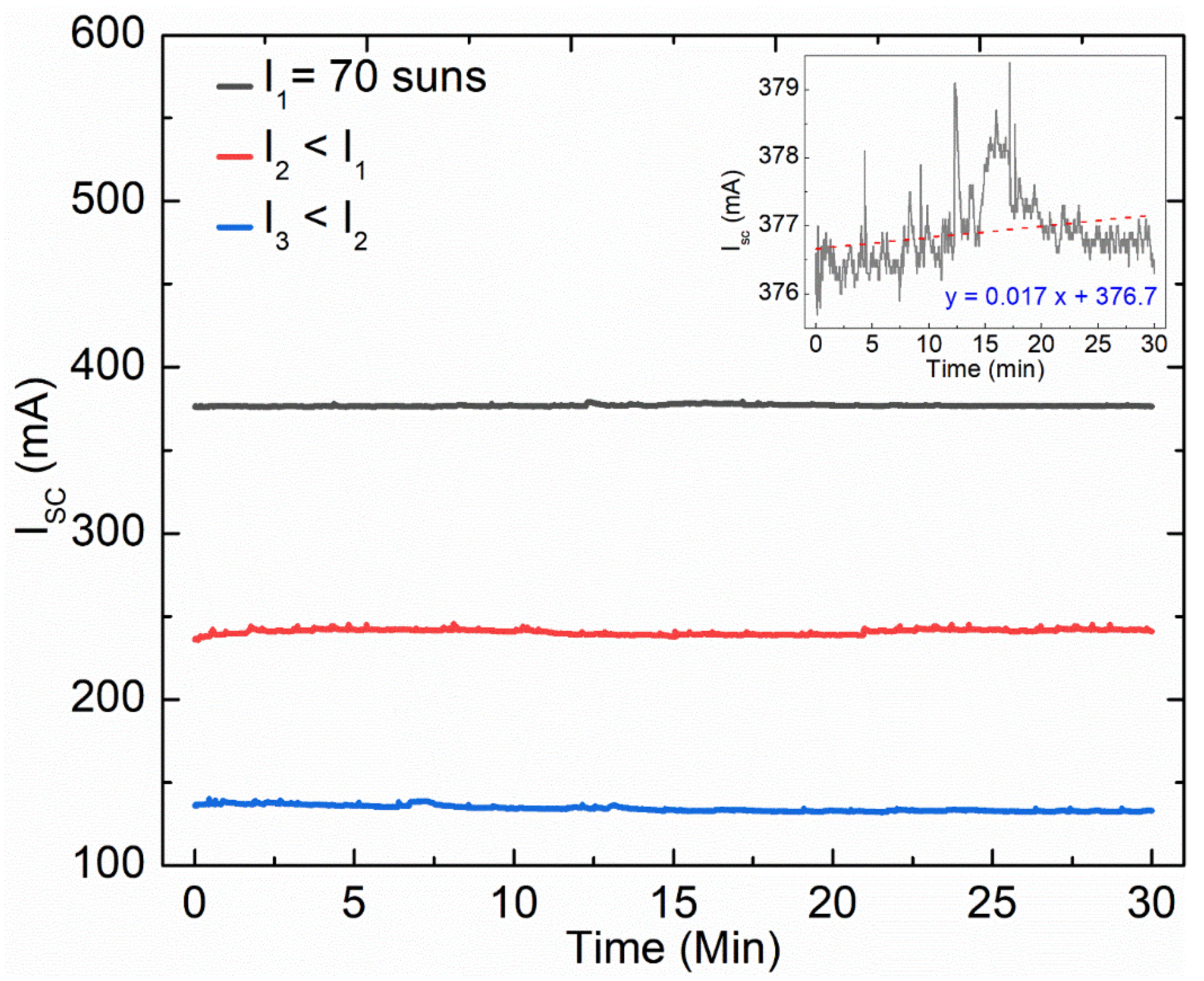
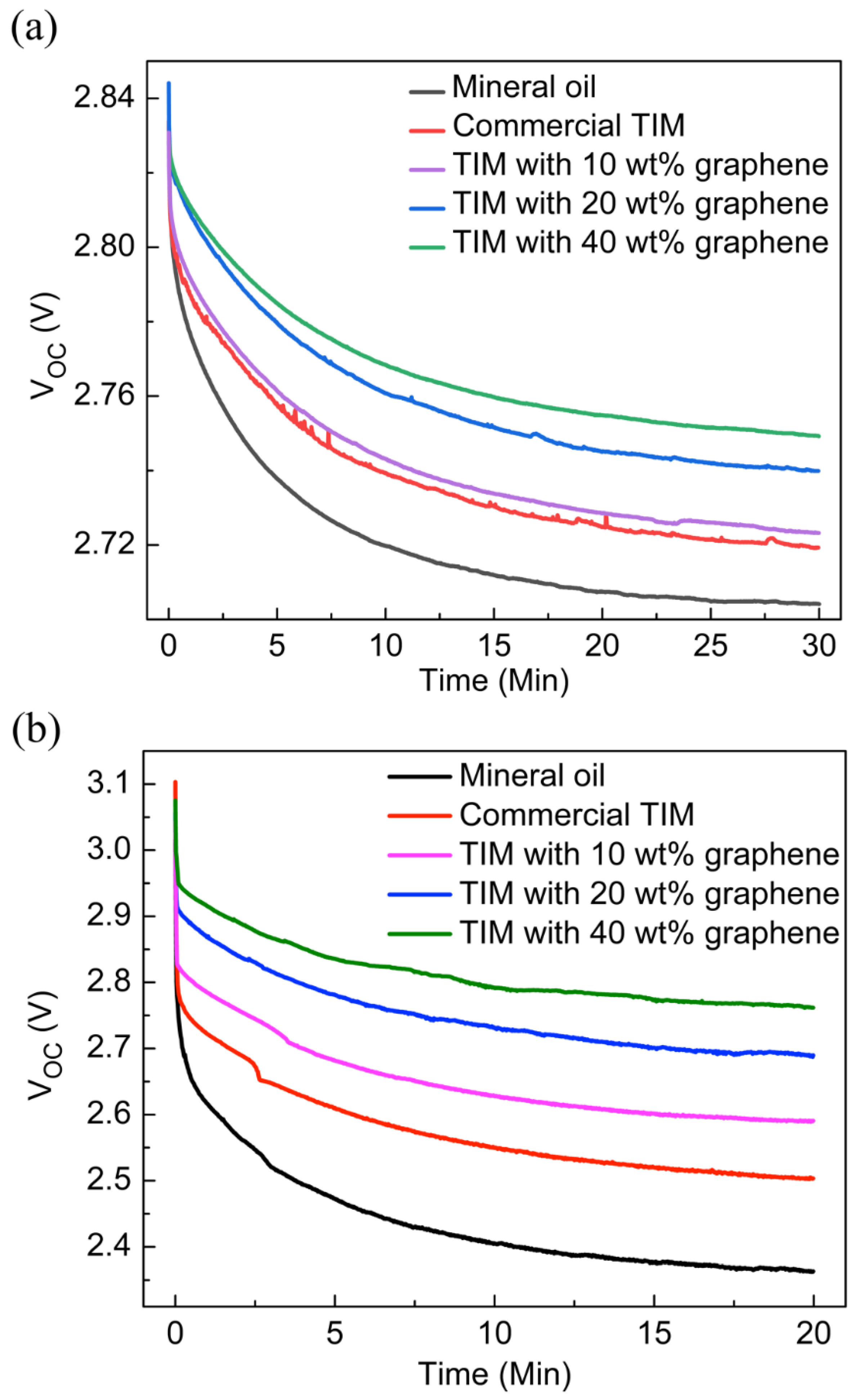
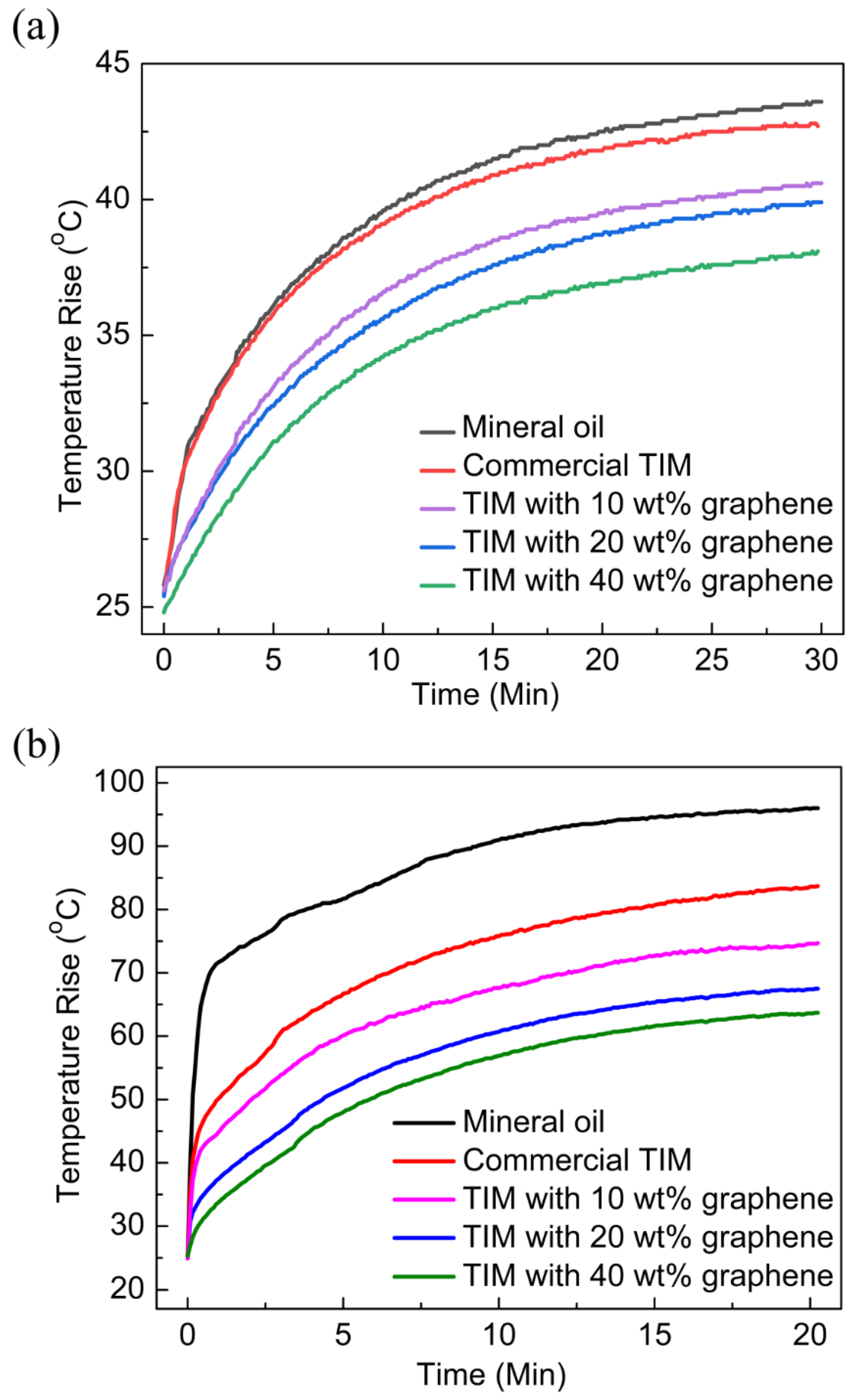
4. Results and Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Ponce, F.A. Novel Semiconductors For Sustainable Solar Energy Technologies. J. Phys. Conf. Ser. 2019, 1173, 012001. [Google Scholar] [CrossRef]
- Tyagi, H.; Agarwal, A.K.; Chakraborty, P.R.; Powar, S. Introduction to Advances in Solar Energy Research, 1st ed.; Springer Neture Singapore: Singapore, 2019; pp. 3–11. [Google Scholar]
- Oaneill, M.; McDanal, A.J.; Piszczor, M.; Peshek, T.; Myers, M.; McPheeters, C.; Steinfeldt, J.; Heintz, B.; Sharps, P.; Puglia, M.; et al. Advanced Development of Space Photovoltaic Concentrators Using Robust Lenses, Multi-Junction Cells, Graphene Radiators. In Proceedings of the 2018 IEEE 7th World Conference Photovoltaic Energy Conversion, Waikoloa Village, HI, USA, 10–15 June 2018. [Google Scholar] [CrossRef]
- Yang, D.; Yang, R.; Priya, S.; Liu, S. (Frank) Recent Advances in Flexible Perovskite Solar Cells: Fabrication and Applications. Angew. Chem. Int. Ed. 2019, 58, 4466–4483. [Google Scholar] [CrossRef] [PubMed]
- Xue, Q.; Xia, R.; Brabec, C.J.; Yip, H.L. Recent Advances in Semi-Transparent Polymer and Perovskite Solar Cells for Power Generating Window Applications. Energy Environ. Sci. 2018, 11, 1688–1709. [Google Scholar] [CrossRef]
- Rodríguez-Seco, C.; Cabau, L.; Vidal-Ferran, A.; Palomares, E. Advances in the Synthesis of Small Molecules as Hole Transport Materials for Lead Halide Perovskite Solar Cells. Acc. Chem. Res. 2018, 51, 869–880. [Google Scholar] [CrossRef]
- Di Carlo Rasi, D.; Janssen, R.A.J. Advances in Solution-Processed Multijunction Organic Solar Cells. Adv. Mater. 2019, 31. [Google Scholar] [CrossRef]
- Lu, L.; Zheng, T.; Wu, Q.; Schneider, A.M.; Zhao, D.; Yu, L. Recent Advances in Bulk Heterojunction Polymer Solar Cells. Chem. Rev. 2015, 115, 12666–12731. [Google Scholar] [CrossRef]
- Zuo, C.; Bolink, H.J.; Han, H.; Huang, J.; Cahen, D.; Ding, L. Advances in Perovskite Solar Cells. Adv. Sci. 2016, 3, 1–16. [Google Scholar] [CrossRef]
- Yu, M.; Long, Y.Z.; Sun, B.; Fan, Z. Recent Advances in Solar Cells Based on One-Dimensional Nanostructure Arrays. Nanoscale 2012, 4, 2783–2796. [Google Scholar] [CrossRef]
- Gledhill, L.K.M. High Efficiency Multi-Junction Space Solar Cells. In Proceedings of the Space Programs and Technologies Conference, Hunstville, AL, USA, 26–28 September 1995; Volume 6, p. 3540. [Google Scholar]
- King, R.R.; Law, D.C.; Edmondson, K.M.; Fetzer, C.M.; Kinsey, G.S.; Yoon, H.; Sherif, R.A.; Karam, N.H. 40% Efficient Metamorphic GaInP/GaInAs/Ge Multijunction Solar Cells. Appl. Phys. Lett. 2007, 90, 90–93. [Google Scholar] [CrossRef]
- Takamoto, T.; Kaneiwa, M.; Imaizumi, M.; Yamaguchi, M. InGaP/GaAs-Based Multijunction Solar Cells. Prog. Photovolt. Res. Appl. 2005, 13, 495–511. [Google Scholar] [CrossRef]
- Law, D.C.; King, R.R.; Yoon, H.; Archer, M.J.; Boca, A.; Fetzer, C.M.; Mesropian, S.; Isshiki, T.; Haddad, M.; Edmondson, K.M.; et al. Future Technology Pathways of Terrestrial III-V Multijunction Solar Cells for Concentrator Photovoltaic Systems. Sol. Energy Mater. Sol. Cells 2010, 94, 1314–1318. [Google Scholar] [CrossRef]
- Cotal, H.; Fetzer, C.; Boisvert, J.; Kinsey, G.; King, R.; Hebert, P.; Yoon, H.; Karam, N. III-V Multijunction Solar Cells for Concentrating Photovoltaics. Energy Environ. Sci. 2009, 2, 174–192. [Google Scholar] [CrossRef]
- Geisz, J.F.; Steiner, M.A.; Jain, N.; Schulte, K.L.; France, R.M.; McMahon, W.E.; Perl, E.E.; Friedman, D.J. Building a Six-Junction Inverted Metamorphic Concentrator Solar Cell. IEEE J. Photovolt. 2018, 8, 626–632. [Google Scholar] [CrossRef]
- Green, M.A.; Dunlop, E.D.; Levi, D.H.; Hohl-Ebinger, J.; Yoshita, M.; Ho-Baillie, A.W.Y. Solar Cell Efficiency Tables (Version 54). Prog. Photovolt. Res. Appl. 2019, 27, 565–575. [Google Scholar] [CrossRef]
- Du, Y.; Le, N.C.H.; Chen, D.; Chen, H.; Zhu, Y. Thermal Management of Solar Cells Using a Nano-Coated Heat Pipe Plate: An Indoor Experimental Study. Int. J. Energy Res. 2017, 41, 867–876. [Google Scholar] [CrossRef]
- Ciulla, G.; Lo Brano, V.; Moreci, E. Forecasting the Cell Temperature of PV Modules with an Adaptive System. Int. J. Photoenergy 2013, 2013, 10. [Google Scholar] [CrossRef]
- Alonso García, M.C.; Balenzategui, J.L. Estimation of Photovoltaic Module Yearly Temperature and Performance Based on Nominal Operation Cell Temperature Calculations. Renew. Energy 2004, 29, 1997–2010. [Google Scholar] [CrossRef]
- Radziemska, E. The Effect of Temperature on the Power Drop in Crystalline Silicon Solar Cells. Renew. Energy 2003, 28, 1–12. [Google Scholar] [CrossRef]
- Mageed, H.M.A. Temperature Effects on the Electrical Performance of Large Area Multicrystalline Silicon Solar Cells Using the Current Shunt Measuring Technique. Engineering 2010, 2, 888–894. [Google Scholar] [CrossRef][Green Version]
- Brinkworth, B.J.; Cross, B.M.; Marshall, R.H.; Yang, H. Thermal Regulation of Photovoltaic Cladding. Sol. Energy 1997, 61, 169–178. [Google Scholar] [CrossRef]
- Brinkworth, B.J.; Sandberg, M. Design Procedure for Cooling Ducts to Minimise Efficiency Loss Due to Temperature Rise in PV Arrays. Sol. Energy 2006, 80, 89–103. [Google Scholar] [CrossRef]
- Sadewasser, S.; Salomé, P.M.P.; Rodriguez-Alvarez, H. Materials Efficient Deposition and Heat Management of CuInSe2 Micro-Concentrator Solar Cells. Sol. Energy Mater. Sol. Cells 2017, 159, 496–502. [Google Scholar] [CrossRef]
- Ling, Z.; Zhang, Z.; Shi, G.; Fang, X.; Wang, L.; Gao, X.; Fang, Y.; Xu, T.; Wang, S.; Liu, X. Review on Thermal Management Systems Using Phase Change Materials for Electronic Components, Li-Ion Batteries and Photovoltaic Modules. Renew. Sustain. Energy Rev. 2014, 31, 427–438. [Google Scholar] [CrossRef]
- Mousavi Baygi, S.R.; Sadrameli, S.M. Thermal Management of Photovoltaic Solar Cells Using Polyethylene Glycol1000 (PEG1000) as a Phase Change Material. Therm. Sci. Eng. Prog. 2018, 5, 405–411. [Google Scholar] [CrossRef]
- Radwan, A.; Ookawara, S.; Ahmed, M. Thermal Management of Concentrator Photovoltaic Systems Using Two-Phase Flow Boiling in Double-Layer Microchannel Heat Sinks. Appl. Energy 2019, 241, 404–419. [Google Scholar] [CrossRef]
- Li, D.; Xuan, Y.; Yin, E.; Li, Q. Conversion Efficiency Gain for Concentrated Triple-Junction Solar Cell System Through Thermal Management. Renew. Energy 2018, 126, 960–968. [Google Scholar] [CrossRef]
- Khan, S.A.; Sezer, N.; Ismail, S.; Koç, M. Design, Synthesis and Nucleate Boiling Performance Assessment of Hybrid Micro-Nano Porous Surfaces for Thermal Management of Concentrated Photovoltaics (CPV). Energy Convers. Manag. 2019, 195, 1056–1066. [Google Scholar] [CrossRef]
- Reddy, S.R.; Ebadian, M.A.; Lin, C.X. A review of PV-T systems: Thermal Management and Efficiency with Single Phase Cooling. Int. J. Heat Mass Transf. 2015, 91, 861–871. [Google Scholar] [CrossRef]
- Sun, X.; Silverman, T.J.; Zhou, Z.; Khan, M.R.; Bermel, P.; Alam, M.A. Optics-Based Approach to Thermal Management of Photovoltaics: Selective-Spectral and Radiative Cooling. IEEE J. Photovolt. 2017, 7, 566–574. [Google Scholar] [CrossRef]
- Chauhan, A.; Tyagi, V.V.; Anand, S. Futuristic Approach for Thermal Management in Solar PV/Thermal Systems with Possible Applications. Energy Convers. Manag. 2018, 163, 314–354. [Google Scholar] [CrossRef]
- Rabie, R.; Emam, M.; Ookawara, S.; Ahmed, M. Thermal Management of Concentrator Photovoltaic Systems Using New Configurations of Phase Change Material Heat Sinks. Sol. Energy 2019, 183, 632–652. [Google Scholar] [CrossRef]
- Sharma, S.; Micheli, L.; Chang, W.; Tahir, A.A.; Reddy, K.S.; Mallick, T.K. Nano-Enhanced Phase Change Material for Thermal Management of BICPV. Appl. Energy 2017, 208, 719–733. [Google Scholar] [CrossRef]
- Preet, S. Water and Phase Change Material Based Photovoltaic Thermal Management Systems: A review. Renew. Sustain. Energy Rev. 2018, 82, 791–807. [Google Scholar] [CrossRef]
- Ma, T.; Yang, H.; Zhang, Y.; Lu, L.; Wang, X. Using Phase Change Materials in Photovoltaic Systems for Thermal Regulation and Electrical Efficiency Improvement: A Review and Outlook. Renew. Sustain. Energy Rev. 2015, 43, 1273–1284. [Google Scholar] [CrossRef]
- Browne, M.C.; Norton, B.; McCormack, S.J. Phase Change Materials for Photovoltaic Thermal Management. Renew. Sustain. Energy Rev. 2015, 47, 762–782. [Google Scholar] [CrossRef]
- Du, Y. Advanced Thermal Management of a Solar Cell by a Nano-Coated Heat Pipe Plate: A Thermal Assessment. Energy Convers. Manag. 2017, 134, 70–76. [Google Scholar] [CrossRef]
- Sargunanathan, S.; Elango, A.; Mohideen, S.T. Performance Enhancement of Solar Photovoltaic Cells Using Effective Cooling Methods: A review. Renew. Sustain. Energy Rev. 2016, 64, 382–393. [Google Scholar] [CrossRef]
- Ye, Z.; Li, Q.; Zhu, Q.; Pan, W. The Cooling Technology of Solar Cells Under Concentrated System. In Proceedings of the 2009 IEEE 6th International Power Electronics and Motion Control Conference, Wuhan, China, 17–20 May 2009; Volume 3, pp. 2193–2197. [Google Scholar]
- Hasan, A.; McCormack, S.J.; Huang, M.J.; Norton, B. Evaluation of Phase Change Materials for Thermal Regulation Enhancement of Building Integrated Photovoltaics. Sol. Energy 2010, 84, 1601–1612. [Google Scholar] [CrossRef]
- Huang, M.J.; Eames, P.C.; Norton, B. Phase Change Materials for Limiting Temperature Rise in Building Integrated Photovoltaics. Sol. Energy 2006, 80, 1121–1130. [Google Scholar] [CrossRef]
- Du, D.; Darkwa, J.; Kokogiannakis, G. Thermal Management Systems for Photovoltaics (PV) Installations: A Critical Review. Sol. Energy 2013, 97, 238–254. [Google Scholar] [CrossRef]
- Prasher, R.; Chiu, C.P. Thermal interface materials. Mater. Adv. Packag. Second Ed. 2016, 10, 511–535. [Google Scholar]
- Zhang, K.; Chai, Y.; Yuen, M.M.F.; Xiao, D.G.W.; Chan, P.C.H. Carbon Nanotube Thermal Interface Material for High-Brightness Light-Emitting-Diode Cooling. Nanotechnology 2008, 19, 205706. [Google Scholar] [CrossRef] [PubMed]
- Renteria, J.; Legedza, S.; Salgado, R.; Balandin, M.P.; Ramirez, S.; Saadah, M.; Kargar, F.; Balandin, A.A. Magnetically-Functionalized Self-Aligning Graphene Fillers for High-Efficiency Thermal Management Applications. Mater. Des. 2015, 88, 214–221. [Google Scholar] [CrossRef]
- Malekpour, H.; Chang, K.H.; Chen, J.C.; Lu, C.Y.; Nika, D.L.; Novoselov, K.S.; Balandin, A.A. Thermal Conductivity of Graphene Laminate. Nano Lett. 2014, 14, 5155–5161. [Google Scholar] [CrossRef] [PubMed]
- Yan, Z.; Nika, D.L.; Balandin, A.A. Thermal Properties of Graphene and Few-Layer Graphene: Applications in Electronics. IET Circuits Devices Syst. 2015, 9, 4–12. [Google Scholar] [CrossRef]
- Goli, P.; Legedza, S.; Dhar, A.; Salgado, R.; Renteria, J.; Balandin, A.A. Graphene-Enhanced Hybrid Phase Change Materials for Thermal Management of Li-Ion Batteries. J. Power Sources 2014, 248, 37–43. [Google Scholar] [CrossRef]
- Renteria, J.; Nika, D.; Balandin, A. Graphene Thermal Properties: Applications in Thermal Management and Energy Storage. Appl. Sci. 2014, 4, 525–547. [Google Scholar] [CrossRef]
- Li, X.; Yang, R. Effect of Lattice Mismatch on Phonon Transmission and Interface Thermal Conductance Across Dissimilar Material Interfaces. Phys. Rev. B Condens. Matter Mater. Phys. 2012, 86. [Google Scholar] [CrossRef]
- Shahil, K.M.F.; Balandin, A.A. Thermal Properties of Graphene and Multilayer Graphene: Applications in Thermal Interface Materials. Solid State Commun. 2012, 152, 1331–1340. [Google Scholar] [CrossRef]
- Shahil, K.M.F.; Balandin, A.A. Graphene-Multilayer Graphene Nanocomposites as Highly Efficient Thermal Interface Materials. Nano Lett. 2012, 12, 861–867. [Google Scholar] [CrossRef]
- Goyal, V.; Balandin, A.A. Thermal Properties of the Hybrid Graphene-Metal Nano-Micro-Composites: Applications in Thermal Interface Materials. Appl. Phys. Lett. 2012, 100. [Google Scholar] [CrossRef]
- Nika, D.L.; Balandin, A.A. Phonons and Thermal Transport in Graphene and Graphene-Based Materials. Rep. Prog. Phys. 2017, 80. [Google Scholar] [CrossRef] [PubMed]
- Balandin, A.A.; Nika, D.L.; Ghosh, S.; Pokatilov, E.P. Lattice Thermal Conductivity of Graphene Flakes: Comparison with Bulk Graphite. Appl. Phys. Lett. 2009, 94, 3–5. [Google Scholar]
- Ghosh, S.; Bao, W.; Nika, D.L.; Subrina, S.; Pokatilov, E.P.; Lau, C.N.; Balandin, A.A. Dimensional Crossover of Thermal Transport In Few-Layer Graphene. Nat. Mater. 2010, 9, 555–558. [Google Scholar] [CrossRef]
- Ghosh, S.; Nika, D.L.; Pokatilov, E.P.; Balandin, A.A. Heat Conduction in Graphene: Experimental Study and Theoretical Interpretation. New J. Phys. 2009, 11, 095012. [Google Scholar] [CrossRef]
- Balandin, A.A.; Ghosh, S.; Nika, D.L.; Pokatilov, E.P. Thermal Conduction in Suspended Graphene Layers. Fuller. Nanotub. Carbon Nanostruct. 2010, 18, 474–486. [Google Scholar] [CrossRef]
- Balandin, A.A. Thermal Properties of Graphene and Nanostructured Carbon Materials. Nat. Mater. 2011, 10, 569–581. [Google Scholar] [CrossRef]
- Balandin, A. The Heat Is On: Graphene Applications. IEEE Nanotechnol. Mag. 2011, 5, 15–19. [Google Scholar] [CrossRef]
- Nika, D.L.; Pokatilov, E.P.; Askerov, A.S.; Balandin, A.A. Phonon Thermal Conduction in Graphene: Role of Umklapp and Edge Roughness Scattering. Phys. Rev. B Condens. Matter Mater. Phys. 2009, 79, 1–12. [Google Scholar] [CrossRef]
- Saadah, M.; Hernandez, E.; Balandin, A.A. Thermal Management of Concentrated Multi-Junction Solar Cells with Graphene-Enhanced Thermal Interface Materials. Appl. Sci. 2017, 7, 589. [Google Scholar] [CrossRef]
- McNamara, A.J.; Joshi, Y.; Zhang, Z.M. Characterization of Nanostructured Thermal Interface Materials—A Review. Int. J. Therm. Sci. 2012, 62, 2–11. [Google Scholar] [CrossRef]
- Lv, P.; Tan, X.W.; Yu, K.H.; Zheng, R.L.; Zheng, J.J.; Wei, W. Super-Elastic Graphene/Carbon Nanotube Aerogel: A Novel Thermal Interface Material with Highly Thermal Transport Properties. Carbon 2016, 99, 222–228. [Google Scholar] [CrossRef]
- Loeblein, M.; Tsang, S.H.; Pawlik, M.; Phua, E.J.R.; Yong, H.; Zhang, X.W.; Gan, C.L.; Teo, E.H.T. High-Density 3D-Boron Nitride and 3D-Graphene for High-Performance Nano-Thermal Interface Material. ACS Nano 2017, 11, 2033–2044. [Google Scholar] [CrossRef] [PubMed]
- Tang, B.; Hu, G.; Gao, H.; Hai, L. Application of Graphene as Filler to Improve Thermal Transport Property of Epoxy Resin for Thermal Interface Materials. Int. J. Heat Mass Transf. 2015, 85, 420–429. [Google Scholar] [CrossRef]
- Park, W.; Guo, Y.; Li, X.; Hu, J.; Liu, L.; Ruan, X.; Chen, Y.P. High-Performance Thermal Interface Material Based on Few-Layer Graphene Composite. J. Phys. Chem. C 2015, 119, 26753–26759. [Google Scholar] [CrossRef]
- Kargar, F.; Barani, Z.; Balinskiy, M.; Magana, A.S.; Lewis, J.S.; Balandin, A.A. Dual-Functional Graphene Composites for Electromagnetic Shielding and Thermal Management. Adv. Electron. Mater. 2019, 5, 35–39. [Google Scholar] [CrossRef]
- Kargar, F.; Barani, Z.; Salgado, R.; Debnath, B.; Lewis, J.S.; Aytan, E.; Lake, R.K.; Balandin, A.A. Thermal Percolation Threshold and Thermal Properties of Composites with High Loading of Graphene and Boron Nitride Fillers. ACS Appl. Mater. Interfaces 2018, 10, 37555–37565. [Google Scholar] [CrossRef]
- Lewis, J.S.; Barani, Z.; Magana, A.S.; Kargar, F.; Balandin, A.A. Thermal and Electrical Conductivity Control in Hybrid Composites with Graphene and Boron Nitride Fillers. Mater. Res. Express 2019, 6. [Google Scholar] [CrossRef]
- Barani, Z.; Mohammadzadeh, A.; Geremew, A.; Huang, C.Y.; Coleman, D.; Mangolini, L.; Kargar, F.; Balandin, A.A. Thermal Properties of the Binary-Filler Hybrid Composites with Graphene and Copper Nanoparticles. Adv. Funct. Mater. 2019. [Google Scholar] [CrossRef]
- Incropera, F.P.; Dewitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer, 6th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2006. [Google Scholar]
- Hirst, L.C.; Ekins-Daukes, N.J. Fundamental Losses in Solar Cells. Prog. Photovolt. Res. Appl. 2011, 19, 286–293. [Google Scholar] [CrossRef]
- Singh, P.; Ravindra, N.M. Temperature Dependence of Solar Cell Performance—An Analysis. Sol. Energy Mater. Sol. Cells 2012, 101, 36–45. [Google Scholar] [CrossRef]
- Matsushita, S.; Sugawara, S.; Isobe, T.; Nakajima, A. Temperature Dependence of a Perovskite-Sensitized Solar Cell: A Sensitized “Thermal” Cell. ACS Appl. Energy Mater. 2019, 2, 13–18. [Google Scholar] [CrossRef]
- Vadiee, E.; Fang, Y.; Zhang, C.; Fischer, A.M.; Williams, J.J.; Renteria, E.J.; Balakrishnan, G.; Honsberg, C.B. Temperature Dependence of GaSb and AlGaSb Solar Cells. Curr. Appl. Phys. 2018, 18, 752–761. [Google Scholar] [CrossRef]
- Taguchi, M.; Maruyama, E.; Tanaka, M. Temperature Dependence of Amorphous/Crystalline Silicon Heterojunction Solar Cells. Jpn. J. Appl. Phys. 2008, 47, 814–818. [Google Scholar] [CrossRef]
- Tvingstedt, K.; Deibel, C. Temperature Dependence of Ideality Factors in Organic Solar Cells and the Relation to Radiative Efficiency. Adv. Energy Mater. 2016, 6, 1–13. [Google Scholar] [CrossRef]
- Singh, P.; Singh, S.N.; Lal, M.; Husain, M. Temperature Dependence of I-V Characteristics and Performance Parameters of Silicon Solar Cell. Sol. Energy Mater. Sol. Cells 2008, 92, 1611–1616. [Google Scholar] [CrossRef]
- Wysocki, J.J.; Rappaport, P. Effect of Temperature on Photovoltaic Solar Energy Conversion. J. Appl. Phys. 1960, 31, 571–578. [Google Scholar] [CrossRef]
- Kinsey, G.S.; Hebert, P.; Barbour, K.E.; Krut, D.D.; Cotal, H.L.; Sherif, R.A. Concentrator Multifunction Solar Cell Characteristics Under Variable Intensity and Temperature. Prog. Photovolt. Res. Appl. 2008, 16, 503–508. [Google Scholar] [CrossRef]

| Sample | Bulk Thermal Conductivity (W/mK) |
|---|---|
| Mineral oil | 0.27 |
| Mineral oil with 10 wt% graphene | 3.05 |
| Mineral oil with 20 wt% graphene | 4.82 |
| Mineral oil with 30 wt% graphene | 5.51 |
| Mineral oil with 40 wt% graphene | 6.74 |
| Commercial TIM (Ice Fusion) | 1.34 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahadevan, B.K.; Naghibi, S.; Kargar, F.; Balandin, A.A. Non-Curing Thermal Interface Materials with Graphene Fillers for Thermal Management of Concentrated Photovoltaic Solar Cells. C 2020, 6, 2. https://doi.org/10.3390/c6010002
Mahadevan BK, Naghibi S, Kargar F, Balandin AA. Non-Curing Thermal Interface Materials with Graphene Fillers for Thermal Management of Concentrated Photovoltaic Solar Cells. C. 2020; 6(1):2. https://doi.org/10.3390/c6010002
Chicago/Turabian StyleMahadevan, Barath Kanna, Sahar Naghibi, Fariborz Kargar, and Alexander A. Balandin. 2020. "Non-Curing Thermal Interface Materials with Graphene Fillers for Thermal Management of Concentrated Photovoltaic Solar Cells" C 6, no. 1: 2. https://doi.org/10.3390/c6010002
APA StyleMahadevan, B. K., Naghibi, S., Kargar, F., & Balandin, A. A. (2020). Non-Curing Thermal Interface Materials with Graphene Fillers for Thermal Management of Concentrated Photovoltaic Solar Cells. C, 6(1), 2. https://doi.org/10.3390/c6010002