Magnetically Induced Two-Phonon Blockade in a Hybrid Spin–Mechanical System
Abstract
:1. Introduction
2. Theoretical Model
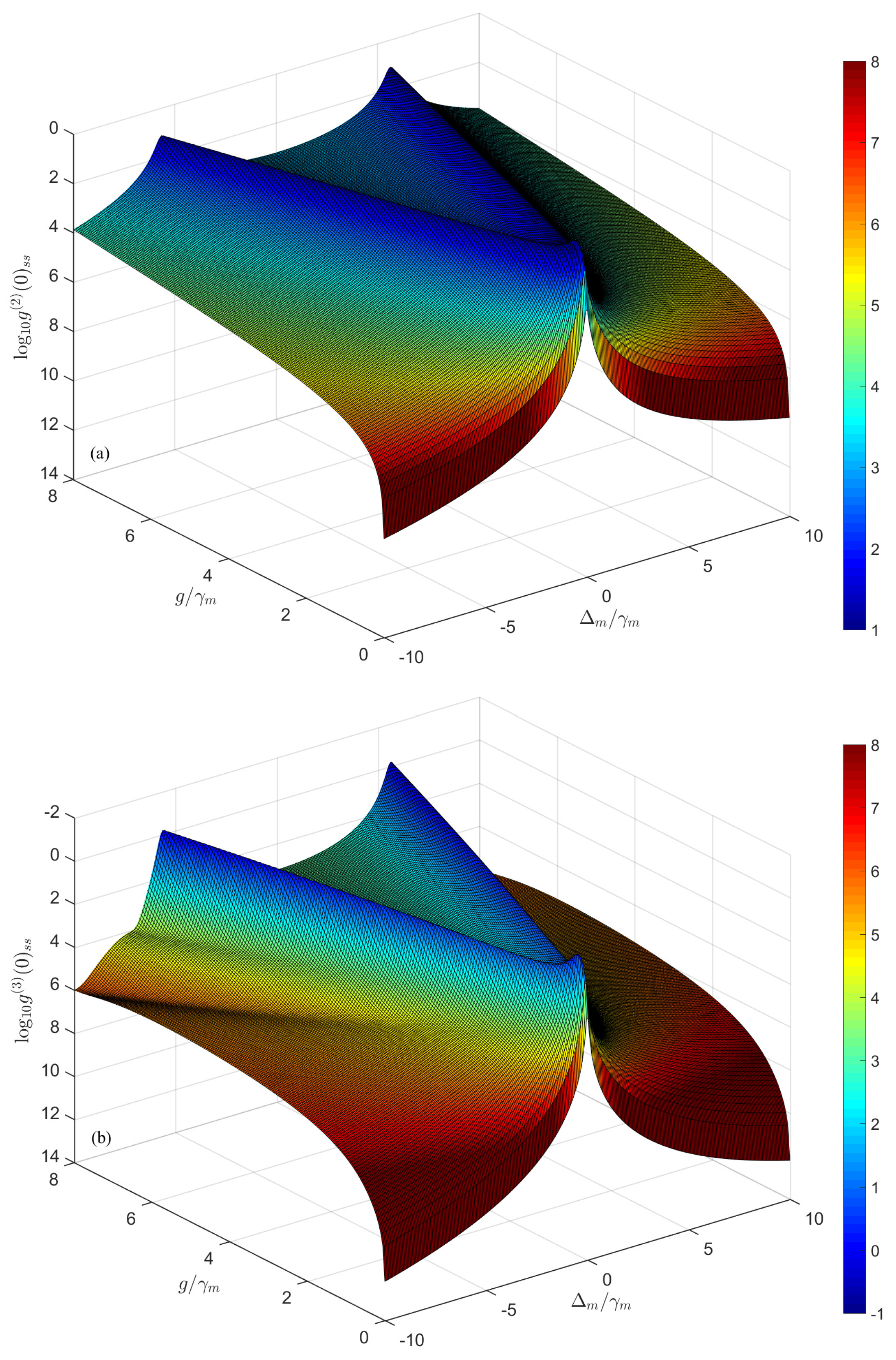
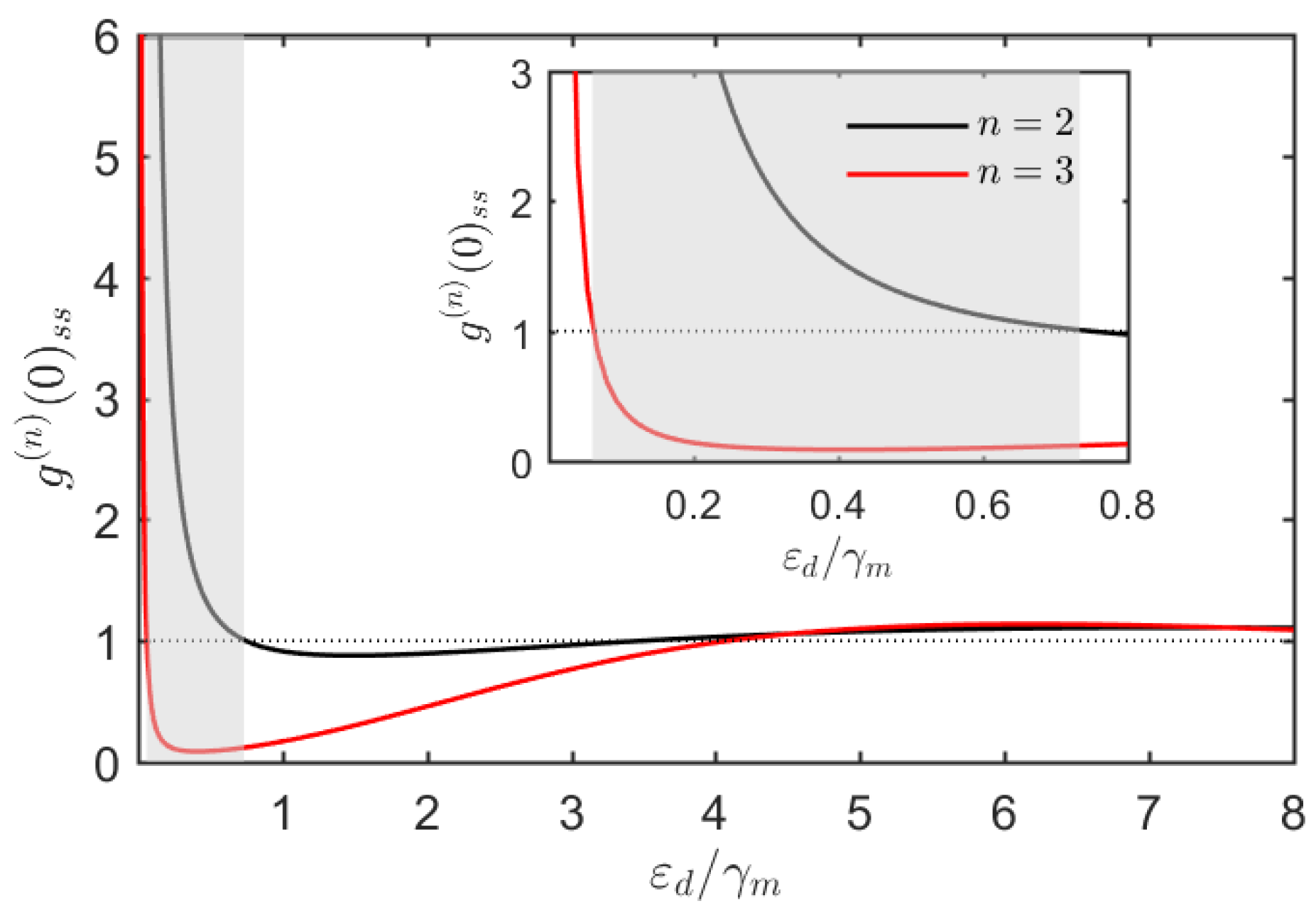
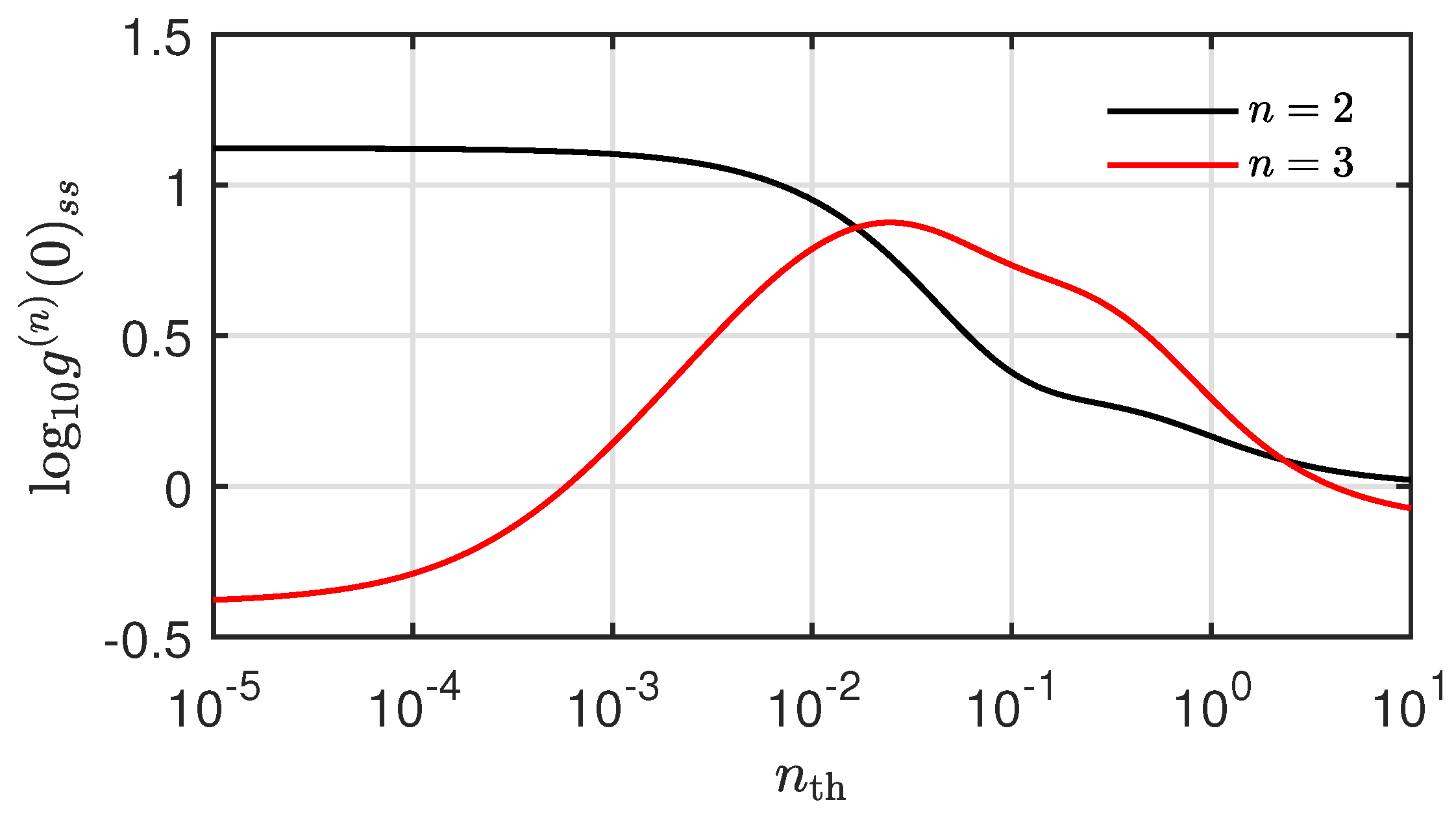
3. The Two-Phonon Coupling Induced the Two-Phonon Blockade via Driving the Effective Two-Level System
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Poot, M.; van der Zant, H.S. Mechanical systems in the quantum regime. Phys. Rep. 2012, 511, 273–335. [Google Scholar] [CrossRef]
- Treutlein, P.; Genes, C.; Hammerer, K.; Poggio, M.; Rabl, P. Hybrid Mechanical Systems. In Cavity Optomechanics; Springer: Berlin/Heidelberg, Germany, 2014; pp. 327–351. [Google Scholar]
- Stannigel, K.; Komar, P.; Habraken, S.J.M.; Bennett, S.D.; Lukin, M.D.; Zoller, P.; Rabl, P. Optomechanical quantum information processing with photons and phonons. Phys. Rev. Lett. 2012, 109, 013603. [Google Scholar] [CrossRef] [PubMed]
- Habraken, S.J.M.; Stannigel, K.; Lukin, M.D.; Zoller, P.; Rabl, P. Continuous mode cooling and phonon routers for phononic quantum networks. New J. Phys. 2012, 14, 115004. [Google Scholar] [CrossRef]
- Patel, R.N.; Sarabalis, C.J.; Jiang, W.; Hill, J.T.; Safavi-Naeini, A.H. Engineering phonon leakage in nanomechanical resonators. Phys. Rev. Appl. 2017, 8, 041001. [Google Scholar] [CrossRef]
- Lemonde, M.-A.; Meesala, S.; Sipahigil, A.; Schuetz, M.J.A.; Lukin, M.D.; Loncar, M.; Rabl, P. Phonon networks with silicon-vacancy centers in diamond waveguides. Phys. Rev. Lett. 2018, 120, 213603. [Google Scholar] [CrossRef]
- Kuzyk, M.C.; Wang, H. Scaling phononic quantum networks of solid-state spins with closed mechanical subsystems. Phys. Rev. X 2018, 8, 041027. [Google Scholar] [CrossRef]
- Braginsky, V.B.; Khalili, F.Y. Quantum Measurements; Cambridge University Press: Cambridge, UK, 1992. [Google Scholar]
- O’Connell, A.D.; Hofheinz, M.; Ansmann, M.; Bialczak, R.C.; Lenander, M.; Lucero, E.; Neeley, M.; Sank, D.; Wang, H.; Weides, M.; et al. Quantum ground state and single-phonon control of a mechanical resonator. Nature 2010, 464, 697–703. [Google Scholar] [CrossRef] [PubMed]
- Teufel, J.D.; Donner, T.; Li, D.; Harlow, J.W.; Allman, M.S.; Cicak, K.; Sirois, A.J.; Whittaker, J.D.; Lehnert, K.W.; Simmonds, R.W. Sideband cooling of micromechanical motion to the quantum ground state. Nature 2011, 475, 359–363. [Google Scholar] [CrossRef] [PubMed]
- Chan, J.; Alegre, T.P.M.; Safavi-Naeini, A.H.; Hill, J.T.; Krause, A.; Gröblacher, S.; Aspelmeyer, M.; Painter, O. Laser cooling of a nanomechanical oscillator into its quantum ground state. Nature 2011, 478, 89–92. [Google Scholar] [CrossRef]
- Clark, J.B.; Lecocq, F.; Simmonds, R.W.; Aumentado, J.; Teufel, J.D. Sideband cooling beyond the quantum backaction limit with squeezed light. Nature 2017, 541, 191–195. [Google Scholar] [CrossRef]
- Mancini, S.; Man’ko, V.I.; Tombesi, P. Ponderomotive control of quantum macroscopic coherence. Phys. Rev. A 1997, 55, 3042. [Google Scholar] [CrossRef]
- Bose, S.; Jacobs, K.; Knight, P.L. Preparation of nonclassical states in cavities with a moving mirror. Phys. Rev. A 1997, 56, 4175. [Google Scholar] [CrossRef]
- Marshall, W.; Simon, C.; Penrose, R.; Bouwmeester, D. Towards quantum superpositions of a mirror. Phys. Rev. Lett. 2003, 91, 130401. [Google Scholar] [CrossRef]
- Kómár, P.; Bennett, S.D.; Stannigel, K.; Habraken, S.J.M.; Rabl, P.; Zoller, P.; Lukin, M.D. Single-photon nonlinearities in two-mode optomechanics. Phys. Rev. A 2013, 87, 013839. [Google Scholar] [CrossRef]
- Lü, X.-Y.; Liao, J.-Q.; Tian, L.; Nori, F. Steady-state mechanical squeezing in an optomechanical system via Duffing nonlinearity. Phys. Rev. A 2015, 91, 013834. [Google Scholar] [CrossRef]
- Wollman, E.E.; Lei, C.U.; Weinstein, A.J.; Suh, J.; Kronwald, A.; Marquardt, F.; Clerk, A.A.; Schwab, K.C. Quantum squeezing of motion in a mechanical resonator. Science 2015, 349, 952–955. [Google Scholar] [CrossRef]
- Pirkkalainen, J.-M.; Damskägg, E.; Brandt, M.; Massel, F.; Sillanpää, M.A. Squeezing of quantum noise of motion in a micromechanical resonator. Phys. Rev. Lett. 2015, 115, 243601. [Google Scholar] [CrossRef]
- Wang, M.; Lü, X.-Y.; Wang, Y.-D.; You, J.Q.; Wu, Y. Macroscopic quantum entanglement in modulated optomechanics. Phys. Rev. A 2016, 94, 053807. [Google Scholar] [CrossRef]
- Imamoḡlu, A.; Schmidt, H.; Woods, G.; Deutsch, M. Strongly interacting photons in a nonlinear cavity. Phys. Rev. Lett. 1997, 79, 1467. [Google Scholar] [CrossRef]
- Birnbaum, K.M.; Boca, A.; Miller, R.; Boozer, A.D.; Northup, T.E.; Kimble, H.J. Photon blockade in an optical cavity with one trapped atom. Nature 2005, 436, 87–90. [Google Scholar] [CrossRef]
- Rabl, P. Photon blockade effect in optomechanical systems. Phys. Rev. Lett. 2011, 107, 063601. [Google Scholar] [CrossRef] [PubMed]
- Kronwald, A.; Ludwig, M.; Marquardt, F. Full photon statistics of a light beam transmitted through an optomechanical system. Phys. Rev. A 2013, 87, 013847. [Google Scholar] [CrossRef]
- Liao, J.-Q.; Nori, F. Photon blockade in quadratically coupled optomechanical systems. Phys. Rev. A 2013, 88, 023853. [Google Scholar] [CrossRef]
- Wang, H.; Gu, X.; Liu, Y.-X.; Miranowicz, A.; Nori, F. Tunable photon blockade in a hybrid system consisting of an optomechanical device coupled to a two-level system. Phys. Rev. A 2015, 92, 033806. [Google Scholar] [CrossRef]
- Bin, Q.; Lü, X.-Y.; Bin, S.-W.; Wu, Y. Two-photon blockade in a cascaded cavity-quantum-electrodynamics system. Phys. Rev. A 2018, 98, 043858. [Google Scholar] [CrossRef]
- Miranowicz, A.; Paprzycka, M.; Liu, Y.-X.; Bajer, J.; Nori, F. Two-photon and three-photon blockades in driven nonlinear systems. Phys. Rev. A 2013, 87, 023809. [Google Scholar] [CrossRef]
- Deng, W.-W.; Li, G.-X.; Qin, H. Enhancement of the two-photon blockade in a strong-coupling qubit-cavity system. Phys. Rev. A 2015, 91, 043831. [Google Scholar] [CrossRef]
- Hamsen, C.; Tolazzi, K.N.; Wilk, T.; Rempe, G. Two-Photon Blockade in an Atom-Driven Cavity QED System. Phys. Rev. Lett. 2017, 118, 133604. [Google Scholar] [CrossRef] [PubMed]
- Kowalewska-Kudłaszyk, A.; Abo, S.I.; Chimczak, G.; Peřina, J., Jr.; Nori, F.; Miranowicz, A. Two-photon blockade and photon-induced tunneling generated by squeezing. Phys. Rev. A 2019, 100, 053857. [Google Scholar] [CrossRef]
- Zou, F.; Zhang, X.-Y.; Xu, X.-W.; Huang, J.-F.; Liao, J.-Q. Multiphoton blockade in the two-photon Jaynes-Cummings model. Phys. Rev. A 2020, 102, 053710. [Google Scholar] [CrossRef]
- Feng, L.-J.; Gong, S.-Q. Two-photon blockade generated and enhanced by mechanical squeezing. Phys. Rev. A 2021, 103, 043509. [Google Scholar] [CrossRef]
- Seok, H.; Wright, E.M. Antibunching in an optomechanical oscillator. Phys. Rev. A 2017, 95, 053844. [Google Scholar] [CrossRef]
- Xie, H.; Liao, C.G.; Shang, X.; Ye, M.Y.; Lin, X.M. Phonon blockade in a quadratically coupled optomechanical system. Phys. Rev. A 2017, 96, 013861. [Google Scholar] [CrossRef]
- Xie, H.; Liao, C.G.; Shang, X.; Chen, Z.H.; Lin, X.M. Optically induced phonon blockade in an optomechanical system with second-order nonlinearity. Phys. Rev. A 2018, 98, 023819. [Google Scholar] [CrossRef]
- Zheng, L.-L.; Yin, T.-S.; Bin, Q.; Lü, X.-Y.; Wu, Y. Single-photon-induced phonon blockade in a hybrid spin-optomechanical system. Phys. Rev. A 2019, 99, 013804. [Google Scholar] [CrossRef]
- Liu, Y.X.; Miranowicz, A.; Gao, Y.B.; Bajer, J.; Sun, C.P.; Nori, F. Qubit-induced phonon blockade as a signature of quantum behavior in nanomechanical resonators. Phys. Rev. A 2010, 82, 032101. [Google Scholar] [CrossRef]
- Wang, X.; Miranowicz, A.; Li, H.R.; Nori, F. Method for observing robust and tunable phonon blockade in a nanomechanical resonator coupled to a charge qubit. Phys. Rev. A 2016, 93, 063861. [Google Scholar] [CrossRef]
- Xu, X.-W.; Chen, A.-X.; Liu, Y.-X. Phonon blockade in a nanomechanical resonator resonantly coupled to a qubit. Phys. Rev. A 2016, 94, 063853. [Google Scholar] [CrossRef]
- Ramos, T.; Sudhir, V.; Stannigel, K.; Zoller, P.; Kippenberg, T.J. Nonlinear Quantum Optomechanics via Individual Intrinsic Two-Level Defects. Phys. Rev. Lett. 2013, 110, 193602. [Google Scholar] [CrossRef]
- Yin, T.-S.; Bin, Q.; Zhu, G.-L.; Gin, G.-R.; Chen, A. Phonon blockade in a hybrid system via the second-order magnetic gradient. Phys. Rev. A 2019, 100, 063840. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, J.-L.; Han, J.-X.; Xia, Y.; Jiang, Y.-Y.; Song, J. Enhanced Phonon Blockade in a Weakly Coupled Hybrid System via Mechanical Parametric Amplification. Phys. Rev. Appl. 2022, 17, 024009. [Google Scholar] [CrossRef]
- Guan, S.; Bowen, W.P.; Liu, C.; Duan, Z. Phonon antibunching effect in coupled nonlinear micro/nanomechanical resonator at finite temperature. Euro Phys. Lett. 2017, 119, 58001. [Google Scholar] [CrossRef]
- Sarma, B.; Sarma, A.K. Tunable phonon blockade in weakly nonlinear coupled mechanical resonators via Coulomb interaction. Sci. Rep. 2018, 8, 14583. [Google Scholar] [CrossRef]
- Shi, H.Q.; Zhou, X.T.; Xu, X.W.; Liu, N.H. Tunable phonon blockade in quadratically coupled optomechanical systems. Sci. Rep. 2018, 8, 2212. [Google Scholar] [CrossRef] [PubMed]
- Shi, H.Q.; Xu, X.W.; Liu, N.H. Phonon blockade in a nanomechanical resonator quadratically coupled to a two-level system. Sci. Rep. 2019, 9, 8754. [Google Scholar] [CrossRef] [PubMed]
- Wang, M.; Yin, T.-S.; Sun, Z.-Y.; Cheng, H.-G.; Zhan, B.-F.; Zheng, L.-L. Unconventional phonon blockade via atom-photon-phonon interaction in hybrid optomechanical systems. Opt. Express 2022, 30, 10251–10268. [Google Scholar] [CrossRef]
- Miranowicz, A.; Bajer, J.; Lambert, N.; Liu, Y.-X.; Nori, F. Tunable multiphonon blockade in coupled nanomechanical resonators. Phys. Rev. A 2016, 93, 013808. [Google Scholar] [CrossRef]
- Aspelmeyer, M.; Kippenberg, T.J.; Marquardt, F. Cavity optomechanics. Rev. Mod. Phys. 2014, 86, 1391. [Google Scholar] [CrossRef]
- Xiong, H.; Si, L.-G.; Lü, X.-Y.; Yang, X.-X.; Wu, Y. Review of cavity optomechanics in the weak-coupling regime: From linearization to intrinsic nonlinear interactions. Sci. China Phys. Mech. Astron. 2015, 58, 1–13. [Google Scholar] [CrossRef]
- Zhu, G.-L.; Lü, X.-Y.; Wan, L.-L.; Yin, T.-S.; Bin, Q.; Wu, Y. Controllable nonlinearity in a dual-coupling optomechanical system under a weak-coupling regime. Phys. Rev. A 2018, 97, 033830. [Google Scholar] [CrossRef]
- Lü, X.-Y.; Zhu, G.-L.; Zheng, L.-L.; Wu, Y. Entanglement and quantum superposition induced by a single photon. Phys. Rev. A 2018, 97, 033807. [Google Scholar] [CrossRef]
- Lü, X.-Y.; Zheng, L.-L.; Zhu, G.-L.; Wu, Y. Single-Photon-Triggered Quantum Phase Transition. Phys. Rev. Appl. 2018, 9, 064006. [Google Scholar] [CrossRef]
- Bin, Q.; Lü, X.-Y.; Yin, T.-S.; Li, Y.; Wu, Y. Collective radiance effects in the ultrastrong-coupling regime. Phys. Rev. A 2009, 99, 033809. [Google Scholar] [CrossRef]
- Xiang, Z.-L.; Ashhab, S.; You, J.Q.; Nori, F. Hybrid quantum circuits: Superconducting circuits interacting with other quantum systems. Rev. Mod. Phys. 2013, 85, 623–653. [Google Scholar] [CrossRef]
- LaHaye, M.D.; Suh, J.; Echternach, P.M.; Schwab, K.C.; Roukes, M.L. Nanomechanical measurements of a superconducting qubit. Nature 2009, 459, 960–964. [Google Scholar] [CrossRef] [PubMed]
- Pirkkalainen, J.-M.; Cho, S.U.; Li, J.; Paraoanu, G.S.; Hakonen, P.J.; Sillanpää, M.A. Hybrid circuit cavity quantum electrodynamics with a micromechanical resonator. Nature 2013, 494, 211–215. [Google Scholar] [CrossRef] [PubMed]
- Hunger, D.; Camerer, S.; Hänsch, T.W.; König, D.; Kotthaus, J.P.; Reichel, J.; Treutlein, P. Resonant coupling of a Bose-Einstein condensate to a micromechanical oscillator. Phys. Rev. Lett. 2010, 104, 143002. [Google Scholar] [CrossRef] [PubMed]
- Kurizki, G.; Bertet, P.; Kubo, Y.; Mølmer, K.; Petrosyan, D.; Rabl, P.; Schmiedmayer, J. Quantum technologies with hybrid systems. Proc. Natl. Acad. Sci. USA 2015, 112, 3866–3873. [Google Scholar] [CrossRef] [PubMed]
- Balasubramanian, G.; Neumann, P.; Twitchen, D.; Markham, M.; Kolesov, R.; Mizuochi, N.; Isoya, J.; Achard, J.; Beck, J.; Tissler, J.; et al. Ultralong spin coherence time in isotopically engineered diamond. Nat. Mater. 2009, 8, 383–387. [Google Scholar] [CrossRef]
- Bar-Gill, N.; Pham, L.M.; Jarmola, A.; Budker, D.; Walsworth, R.L. Solid-state electronic spin coherence time approaching one second. Nat. Commun. 2013, 4, 1743. [Google Scholar] [CrossRef]
- Bennett, S.D.; Yao, N.Y.; Otterbach, J.; Zoller, P.; Rabl, P.; Lukin, M.D. Phonon-Induced Spin-Spin Interactions in Diamond Nanostructures: Application to Spin Squeezing. Phys. Rev. Lett. 2013, 110, 156402. [Google Scholar] [CrossRef]
- Kepesidis, K.V.; Bennett, S.D.; Portolan, S.; Lukin, M.D.; Rabl, P. Phonon cooling and lasing with nitrogen-vacancy centers in diamond. Phys. Rev. B 2013, 88, 064105. [Google Scholar] [CrossRef]
- Ovartchaiyapong, P.; Lee, K.W.; Myers, B.A.; Jayich, A.C.B. Dynamic strain-mediated coupling of a single diamond spin to a mechanical resonator. Nat. Commun. 2014, 5, 4429. [Google Scholar] [CrossRef] [PubMed]
- Teissier, J.; Barfuss, A.; Appel, P.; Neu, E.; Maletinsky, P. Strain Coupling of a Nitrogen-Vacancy Center Spin to a Diamond Mechanical Oscillator. Phys. Rev. Lett. 2014, 113, 020503. [Google Scholar] [CrossRef] [PubMed]
- MacQuarrie, E.R.; Gosavi, T.A.; Jungwirth, N.R.; Bhave, S.A.; Fuchs, G.D. Mechanical Spin Control of Nitrogen-Vacancy Centers in Diamond. Phys. Rev. Lett. 2013, 111, 227602. [Google Scholar] [CrossRef] [PubMed]
- Rabl, P.; Cappellaro, P.; Dutt, M.V.G.; Jiang, L.; Maze, J.R.; Lukin, M.D. Strong magnetic coupling between an electronic spin qubit and a mechanical resonator. Phys. Rev. B 2009, 79, 041302. [Google Scholar] [CrossRef]
- Arcizet, O.; Jacques, V.; Siria, A.; Poncharal, P.; Vincent, P.; Seidelin, S. A single nitrogen-vacancy defect coupled to a nanomechanical oscillator. Nat. Phys. 2011, 7, 879–883. [Google Scholar] [CrossRef]
- Kolkowitz, S.; Jayich, A.C.B.; Unterreithmeier, Q.P.; Bennett, S.D.; Rabl, P.; Harris, J.G.E.; Lukin, M.D. Coherent sensing of a mechanical resonator with a single-spin qubit. Science 2012, 335, 1603–1606. [Google Scholar] [CrossRef] [PubMed]
- Pigeau, B.; Rohr, S.; De Lépinay, L.M.; Gloppe, A.; Jacques, V.; Arcizet, O. Observation of a phononic Mollow triplet in a multimode hybrid spin-nanomechanical system. Nat. Commun. 2015, 6, 8603. [Google Scholar] [CrossRef]
- Yin, Z.Q.; Zhao, N.; Li, T.C. Hybrid opto-mechanical systems with nitrogen-vacancy centers. Sci. China Phys. Mech. Astron. 2015, 58, 050303. [Google Scholar] [CrossRef]
- Li, P.-B.; Xiang, Z.-L.; Rabl, P.; Nori, F. Hybrid Quantum Device with Nitrogen-Vacancy Centers in Diamond Coupled to Carbon Nanotubes. Phys. Rev. Lett. 2016, 117, 015502. [Google Scholar] [CrossRef] [PubMed]
- Ma, Y.; Yin, Z.Q.; Huang, P.; Yang, W.L.; Du, J. Cooling a mechanical resonator to the quantum regime by heating it. Phys. Rev. A 2016, 94, 053836. [Google Scholar] [CrossRef]
- Cai, K.; Wang, R.X.; Yin, Z.Q.; Long, G.L. Second-order magnetic field gradient-induced strong coupling between nitrogen-vacancy centers and a mechanical oscillator. Sci. China Phys. Mech. Astron. 2017, 60, 070311. [Google Scholar] [CrossRef]
- Muñoz, C.S.; Lara, A.; Puebla, J.; Nori, F. Hybrid Systems for the Generation of Nonclassical Mechanical States via Quadratic Interactions. Phys. Rev. Lett. 2018, 121, 123604. [Google Scholar] [CrossRef] [PubMed]
- Ohashi, K.; Rosskopf, T.; Watanabe, H.; Loretz, M.; Tao, Y.; Hauert, R.; Tomizawa, S.; Ishikawa, T.; Ishi-Hayase, J.; Shikata, S.; et al. Negatively Charged Nitrogen-Vacancy Centers in a 5 nm Thin 12C Diamond Film. Nano Lett. 2013, 13, 4733–4738. [Google Scholar] [CrossRef]
- Peterson, R.W.; Purdy, T.P.; Kampel, N.S.; Andrews, R.W.; Yu, P.-L.; Lehnert, K.W.; Regal, C.A. Laser Cooling of a Micromechanical Membrane to the Quantum Backaction Limit. Phys. Rev. Lett. 2016, 116, 063601. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, H.-Y.; Yin, T.-S.; Chen, A. Magnetically Induced Two-Phonon Blockade in a Hybrid Spin–Mechanical System. Magnetochemistry 2024, 10, 41. https://doi.org/10.3390/magnetochemistry10060041
Liu H-Y, Yin T-S, Chen A. Magnetically Induced Two-Phonon Blockade in a Hybrid Spin–Mechanical System. Magnetochemistry. 2024; 10(6):41. https://doi.org/10.3390/magnetochemistry10060041
Chicago/Turabian StyleLiu, Hong-Yue, Tai-Shuang Yin, and Aixi Chen. 2024. "Magnetically Induced Two-Phonon Blockade in a Hybrid Spin–Mechanical System" Magnetochemistry 10, no. 6: 41. https://doi.org/10.3390/magnetochemistry10060041
APA StyleLiu, H.-Y., Yin, T.-S., & Chen, A. (2024). Magnetically Induced Two-Phonon Blockade in a Hybrid Spin–Mechanical System. Magnetochemistry, 10(6), 41. https://doi.org/10.3390/magnetochemistry10060041





