Collective Magnetic Behavior of 11 nm Photo-Switchable CsCoFe Prussian Blue Analogue Nanocrystals: Effect of Dilution and Light Intensity
Abstract
:1. Introduction
2. Results and Discussion
2.1. Materials and Methods
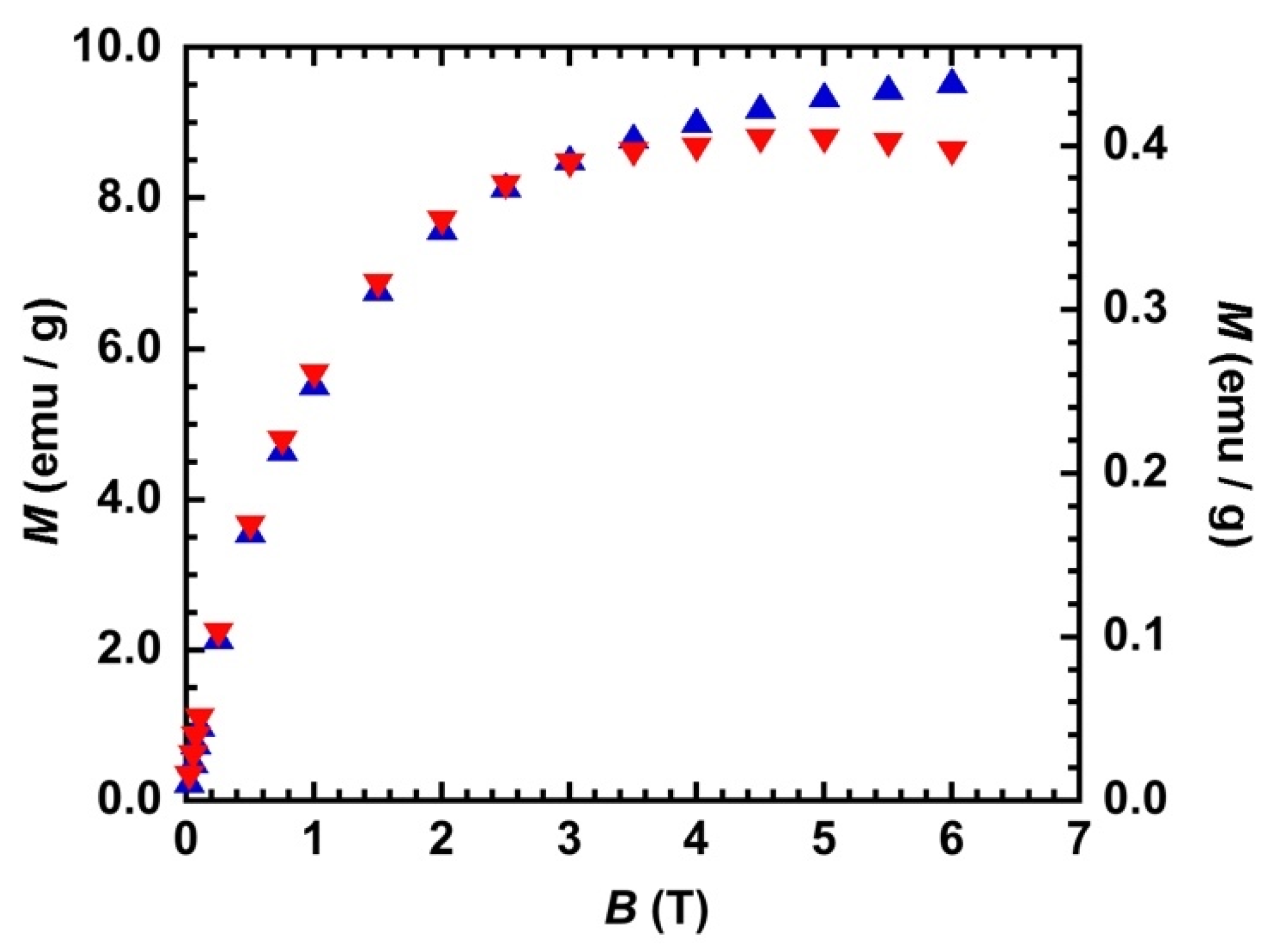
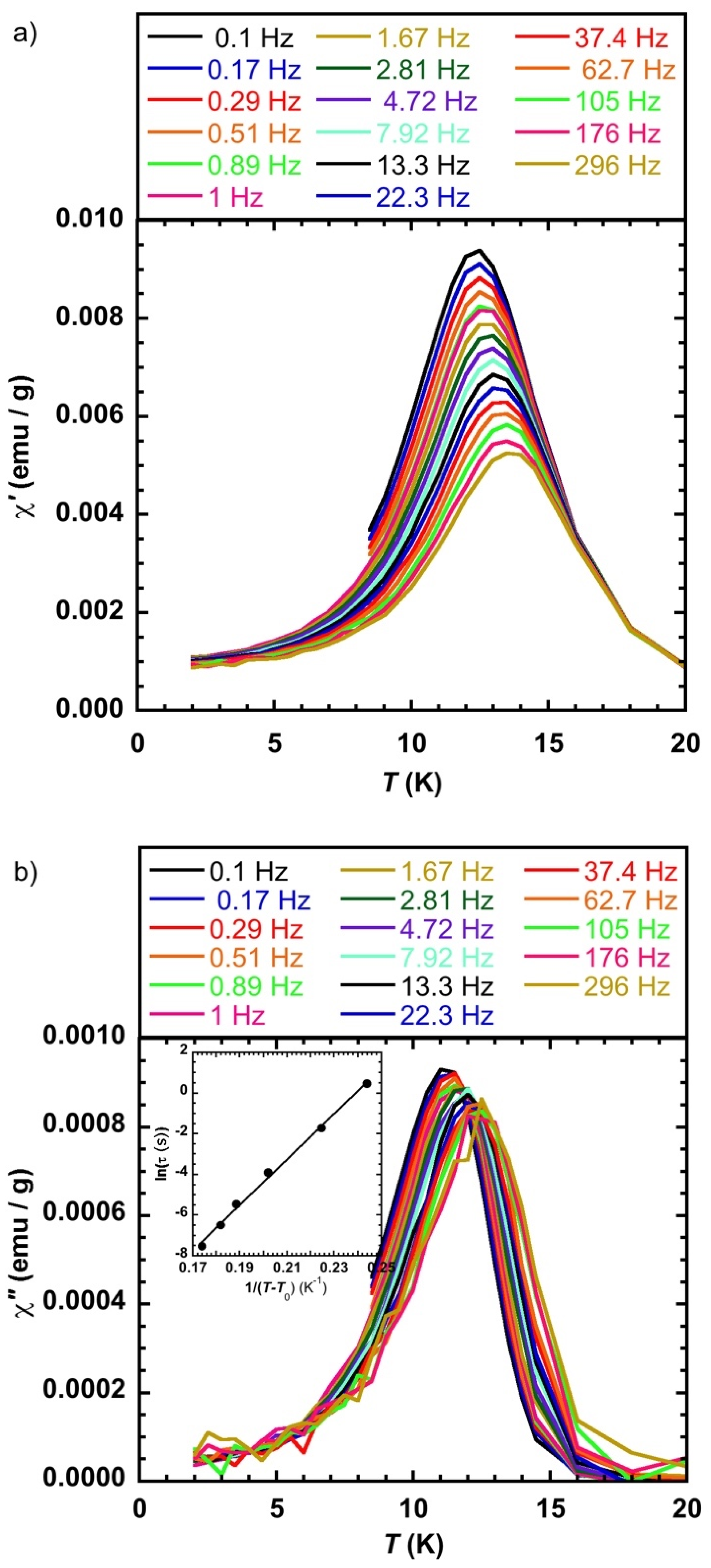
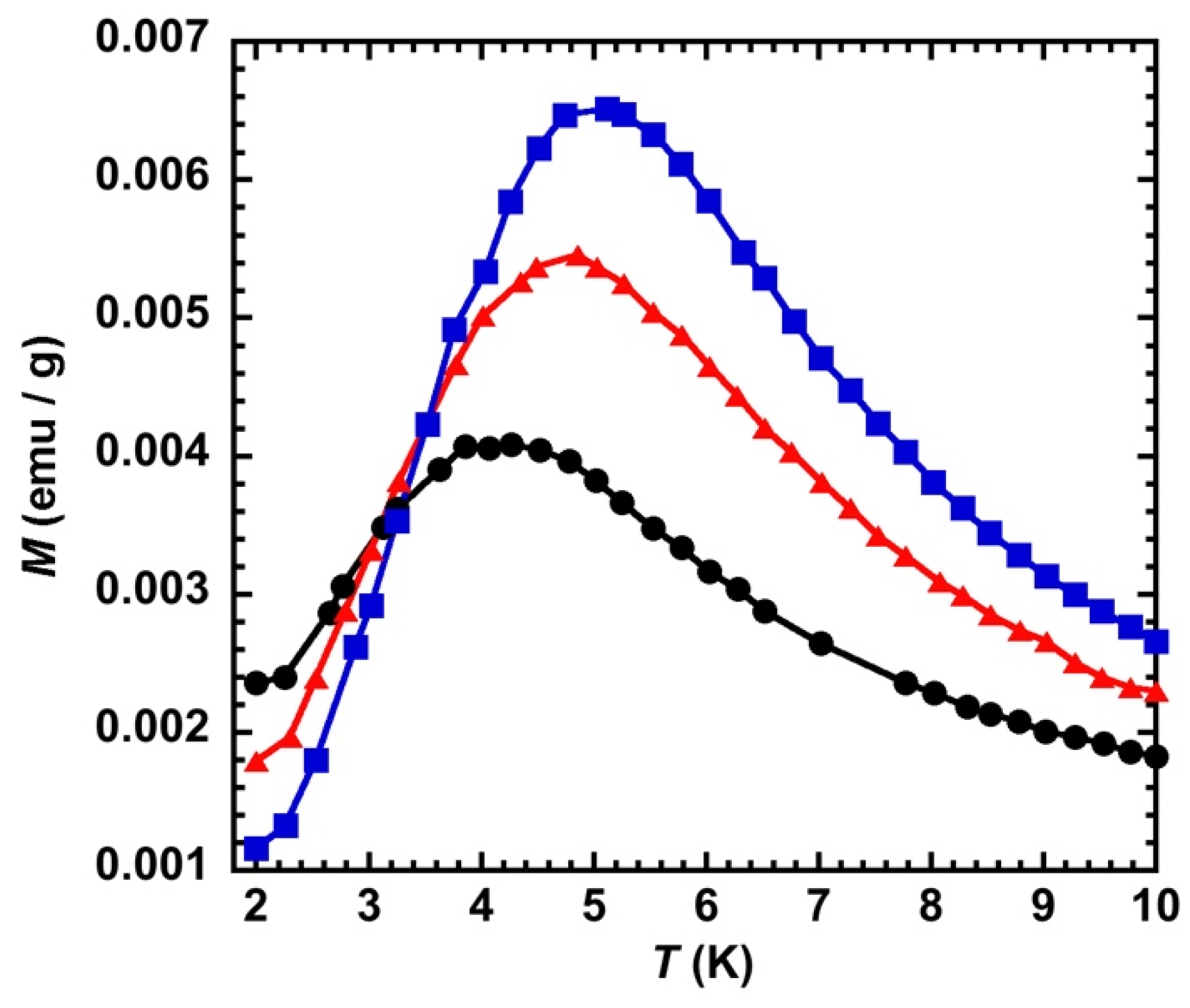
2.2. Magnetic Behavior of CsCoFe_CTA
2.3. Magnetic Behavior of CsCoFe_PVP
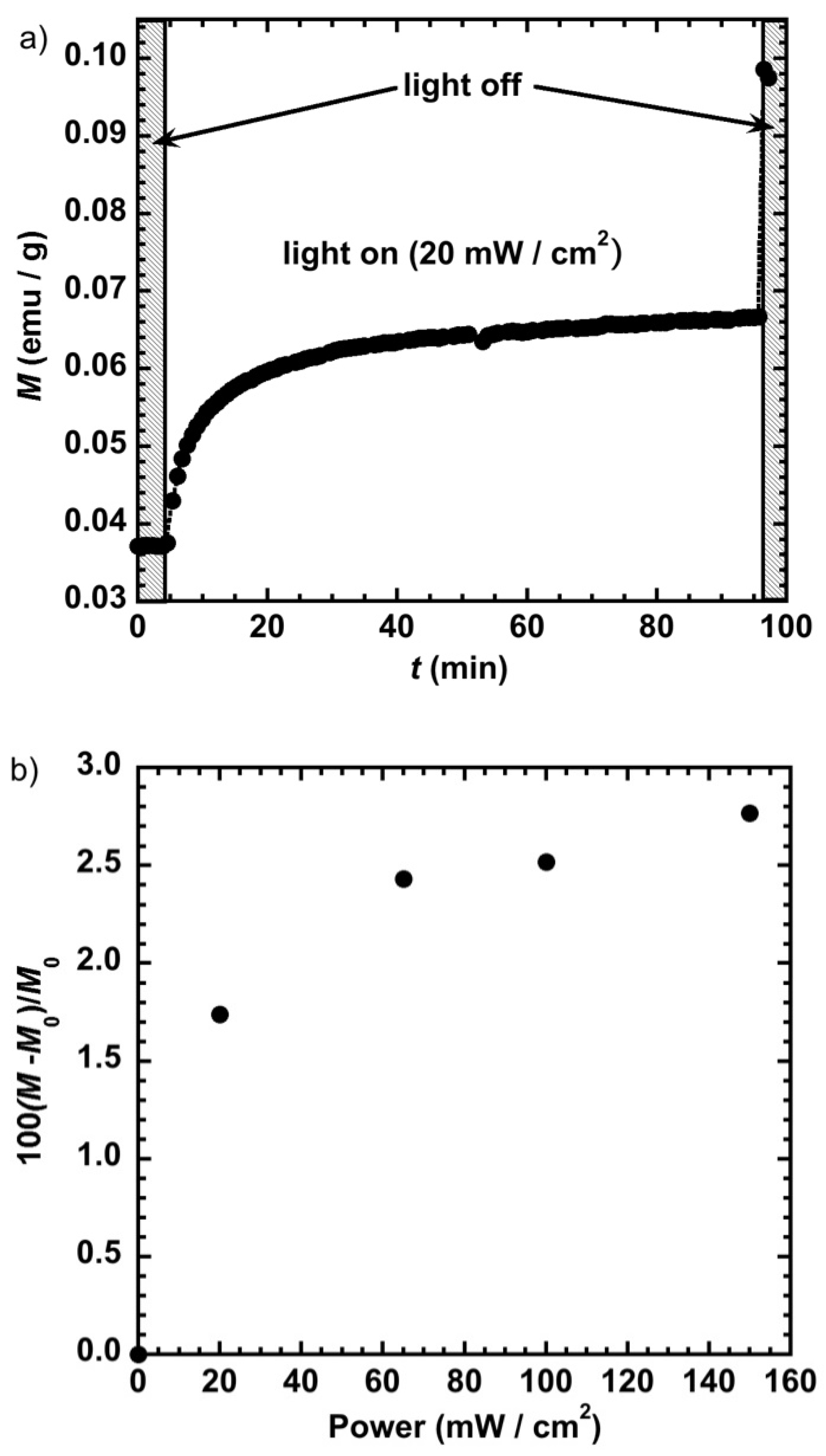
2.4. Effect of the Power of Light Irradiation
3. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sato, O.; Iyoda, T.; Fujishima, A.; Hashimoto, K. Photoinduced magnetization of a cobalt-iron cyanide. Science 1996, 272, 704–705. [Google Scholar] [CrossRef]
- Sato, O.; Einaga, Y.; Iyoda, T.; Fujishima, A.; Hashimoto, K. Cation-driven electron transfer involving a spin transition at room temperature in a cobalt iron cyanide thin film. J. Phys. Chem. B 1997, 101, 3903–3905. [Google Scholar] [CrossRef]
- Yokoyama, T.; Ohta, T.; Sato, O.; Hashimoto, K. Characterization of magnetic CoFe cyanides by x-ray-absorption fine-structure spectroscopy. Phys. Rev. B 1998, 58, 8257–8266. [Google Scholar] [CrossRef]
- Sato, O.; Einaga, Y.; Fujishima, A.; Hashimoto, K. Photoinduced long-range magnetic ordering of a cobalt-iron cyanide. Inorg. Chem. 1999, 38, 4405–4412. [Google Scholar] [CrossRef]
- Champion, G.; Escax, V.; Moulin, C.C.D.; Bleuzen, A.; Villain, F.O.; Baudelet, F.; Dartyge, E.; Verdaguer, N. Photoinduced ferrimagnetic systems in prussian blue analogues (CxCo4)-Co-I[Fe(CN)(6)](y) (C-I = alkali cation). 4. Characterization of the ferrimagnetism of the photoinduced metastable state in Rb1.8Co4[Fe(CN)(6)](3.3)center dot 13H(2)O by K edges X-ray magnetic circular dichroism. J. Am. Chem. Soc. 2001, 123, 12544–12546. [Google Scholar] [PubMed]
- Escax, V.; Bleuzen, A.; Moulin, C.C.D.; Villain, F.; Goujon, A.; Varret, F.; Verdaguer, M. Photoinduced ferrimagnetic systems in prussian blue analogues (CxCo4)-Co-I[Fe(CN)(6)](y) (C-I = alkali cation). 3. Control of the photo- and thermally induced electron transfer by the [Fe(CN)(6)] vacancies in cesium derivatives. J. Am. Chem. Soc. 2001, 123, 12536–12543. [Google Scholar] [CrossRef] [PubMed]
- Shimamoto, N.; Ohkoshi, S.; Sato, O.; Hashimoto, K. Control of charge-transfer-induced spin transition temperature on cobalt-iron Prussian blue analogues. Inorg. Chem. 2002, 41, 678–684. [Google Scholar] [CrossRef] [PubMed]
- Trinh, L.; Zerdane, S.; Mazerat, S.; Dia, N.; Dragoe, D.; Herrero, C.; Riviere, E.; Catala, L.; Cammarata, M.; Collet, E.; et al. Photoswitchable 11 nm CsCoFe Prussian Blue Analogue Nanocrystals with High Relaxation Temperature. Inorg. Chem. 2020, 59, 13153–13161. [Google Scholar] [CrossRef] [PubMed]
- Brinzei, D.; Catala, L.; Louvain, N.; Rogez, G.; Stephan, O.; Gloter, A.; Mallah, T. Spontaneous stabilization and isolation of dispersible bimetallic coordination nanoparticles of CsxNi[Cr(CN)(6)](y). J. Mater. Chem. 2006, 16, 2593–2599. [Google Scholar] [CrossRef]
- Cammarata, M.; Zerdane, S.; Balducci, L.; Azzolina, G.; Mazerat, S.; Exertier, C.; Trabuco, M.; Levantino, M.; Alonso-Mori, R.; Glownia, J.M.; et al. Charge transfer driven by ultrafast spin transition in a CoFe Prussian blue analogue. Nat. Chem. 2021, 13, 10–14. [Google Scholar] [CrossRef]
- Bonnet, R.; Lenfant, S.; Mazerat, S.; Mallah, T.; Vuillaume, D. Long-range electron transport in Prussian blue analog nanocrystals. Nanoscale 2020, 12, 20374–20385. [Google Scholar] [CrossRef] [PubMed]
- Prado, Y.; Arrio, M.A.; Volatron, F.; Otero, E.; Moulin, C.C.D.; Sainctavit, P.; Catala, L.; Mallah, T. Magnetic Anisotropy of Cyanide-Bridged Core and CoreShell Coordination Nanoparticles Probed by X-ray Magnetic Circular Dichroism. Chem. Eur. J. 2013, 19, 6685–6694. [Google Scholar] [CrossRef] [PubMed]
- Dormann, J.L.; Spinu, L.; Tronc, E.; Jolivet, J.P.; Lucari, F.; D’Orazio, F. Effect of interparticle interactions on the dynamical properties of gamma-Fe2O3 nanoparticles. J. Magn. Magn. Mater. 1998, 183, L255–L260. [Google Scholar] [CrossRef]
- Prado, Y.; Mazerat, S.; Riviere, E.; Rogez, G.; Gloter, A.; Stephan, O.; Catala, L.; Mallah, T. Magnetization Reversal in (CsNiCrIII)-Cr-II(CN)(6) Coordination Nanoparticles: Unravelling Surface Anisotropy and Dipolar Interaction Effects. Adv. Funct. Mater. 2014, 24, 5402–5411. [Google Scholar] [CrossRef]
- Dormann, J.L.; Cherkaoui, R.; Spinu, L.; Nogues, M.; Lucari, F.; D’Orazio, F.; Fiorani, D.; Garcia, A.; Tronc, E.; Jolivet, J.P. From pure superparamagnetic regime to glass collective state of magnetic moments in gamma-Fe2O3 nanoparticle assemblies. J. Magn. Magn. Mater. 1998, 187, L139–L144. [Google Scholar] [CrossRef]
- Brinzei, D.; Catala, L.; Rogez, G.; Gloter, A.; Mallah, T. Magnetic behaviour of negatively charged nickel(II) hexacyanoferrate(III) coordination nanoparticles. Inorg. Chim. Acta 2008, 361, 3931–3936. [Google Scholar] [CrossRef]
- Folch, B.; Guari, Y.; Larionova, J.; Luna, C.; Sangregorio, C.; Innocenti, C.; Caneschi, A.; Guerin, C. Synthesis and behaviour of size controlled cyano-bridged coordination polymer nanoparticles within hybrid mesoporous silica. New J. Chem. 2008, 32, 273–282. [Google Scholar] [CrossRef]
- Pajerowski, D.M.; Frye, F.A.; Talham, D.R.; Meisel, M.W. Size dependence of the photoinduced magnetism and long-range ordering in Prussian blue analogue nanoparticles of rubidium cobalt hexacyanoferrate. New J. Phys. 2007, 9, 222. [Google Scholar] [CrossRef]
- Moulin, R.; Delahaye, E.; Bordage, A.; Fonda, E.; Baltaze, J.P.; Beaunier, P.; Riviere, E.; Fornasieri, G.; Bleuzen, A. Ordered Mesoporous Silica Monoliths as a Versatile Platform for the Study of Magnetic and Photomagnetic Prussian Blue Analogue Nanoparticles. Eur. J. Inorg. Chem. 2017, 1303–1313. [Google Scholar] [CrossRef]
- Fornasieri, G.; Bordage, A.; Bleuzen, A. Magnetism and Photomagnetism of Prussian Blue Analogue Nanoparticles Embedded in Porous Metal Oxide Ordered Nanostructures. Eur. J. Inorg. Chem. 2018, 259–271. [Google Scholar] [CrossRef] [Green Version]
- Rave, W.; Fabian, K.; Hubert, A. Magnetic states of small cubic particles with uniaxial anisotropy. J. Magn. Magn. Mater. 1998, 190, 332–348. [Google Scholar] [CrossRef]
- Mallah, T.; Auberger, C.; Verdaguer, M.; Veillet, P. A Heptanuclear Criiiniii(6) Complex with a Low-Lying S = 15/2 Ground-State. J. Chem. Soc. Chem. Commun. 1995, 61–62. [Google Scholar] [CrossRef]
- Gadet, V.; Mallah, T.; Castro, I.; Verdaguer, M.; Veillet, P. High-Tc Molecular-Based Magnets–A Ferromagnetic Bimetallic Chromium(Iii) Nickel(Ii) Cyanide with Tc = 90-K. J. Am. Chem. Soc. 1992, 114, 9213–9214. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trinh, L.; Rivière, E.; Mazerat, S.; Catala, L.; Mallah, T. Collective Magnetic Behavior of 11 nm Photo-Switchable CsCoFe Prussian Blue Analogue Nanocrystals: Effect of Dilution and Light Intensity. Magnetochemistry 2021, 7, 99. https://doi.org/10.3390/magnetochemistry7070099
Trinh L, Rivière E, Mazerat S, Catala L, Mallah T. Collective Magnetic Behavior of 11 nm Photo-Switchable CsCoFe Prussian Blue Analogue Nanocrystals: Effect of Dilution and Light Intensity. Magnetochemistry. 2021; 7(7):99. https://doi.org/10.3390/magnetochemistry7070099
Chicago/Turabian StyleTrinh, Linh, Eric Rivière, Sandra Mazerat, Laure Catala, and Talal Mallah. 2021. "Collective Magnetic Behavior of 11 nm Photo-Switchable CsCoFe Prussian Blue Analogue Nanocrystals: Effect of Dilution and Light Intensity" Magnetochemistry 7, no. 7: 99. https://doi.org/10.3390/magnetochemistry7070099
APA StyleTrinh, L., Rivière, E., Mazerat, S., Catala, L., & Mallah, T. (2021). Collective Magnetic Behavior of 11 nm Photo-Switchable CsCoFe Prussian Blue Analogue Nanocrystals: Effect of Dilution and Light Intensity. Magnetochemistry, 7(7), 99. https://doi.org/10.3390/magnetochemistry7070099






