Next Article in Journal
New Manufacturing Process for Granular Texture Management in Polycrystalline BaM Hexaferrites through the Goethite Crystallite Laths Aspect Ratio, and a Specialized Law of Approach to the Magnetic Saturation for Partly Polarized Uniaxial Materials
Previous Article in Journal
Energetic Neutral Atom Imaging of the Earth’s Ring Current and Some Results from the Chinese Double Star Program
Previous Article in Special Issue
On the Concurrent Bipartite Entanglement of a Spin-1 Heisenberg Diamond Cluster Developed for Tetranuclear Nickel Complexes
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Magnetochemistry
Volume 9
Issue 1
10.3390/magnetochemistry9010031
magnetochemistry-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Something You Need Might Be under Your Feet: Molecular Magnetism of Heavy Kramers Lanthanide Hydrated Chlorides and Their Complexes with Polydentate Terpy Ligand

by
Svetlana P. Petrosyants
,
Konstantin A. Babeshkin
,
Andrey B. Ilyukhin
,
Pavel S. Koroteev
* and
Nikolay N. Efimov
N.S. Kurnakov Institute of General and Inorganic Chemistry, Russian Academy of Sciences, Leninsky Prosp. 31, 119991 Moscow, Russia
*
Author to whom correspondence should be addressed.
Magnetochemistry 2023, 9(1), 31; https://doi.org/10.3390/magnetochemistry9010031
Submission received: 1 December 2022 / Revised: 8 January 2023 / Accepted: 9 January 2023 / Published: 12 January 2023
(This article belongs to the Special Issue Molecular Magnetic Materials)
Browse Figures
Versions Notes

Abstract

:
A study of the molecular magnetism of the hydrated salts [Ln(H2O)6Cl2]Cl (Ln = Gd (1Gd), Dy (1Dy), Er (1Er), Yb (1Yb)) and lanthanide chloride complexes with 2,2′;6′,2″-terpyridine (terpy) synthesized on their basis, [Ln(H2O)4(terpy)Cl]Cl2·3H2O (Ln = Gd (2Gd), Dy (2Dy), Er (2Er), and Yb (2Yb), was carried out. It was found that both the initial hydrated chlorides containing Dy, Er, Yb, and their derivatives with terpyridine exhibit the properties of single-molecule magnets. For the complexes with terpyridine, the values of the remagnetization barriers increase from Er to Dy, while for the aquachlorides, the corresponding values increase in the series Ueff (1Dy) < Ueff (1Er). It was found that magnetic relaxation in Yb complexes mostly proceeds according to the Raman mechanism.

1. Introduction

The search for new single-molecule magnets (SMMs) is one of the most topical problems in the study of lanthanide complexes. SMMs are the compounds able to retain residual magnetization when an external magnetic field is turned off. Unlike nanomagnets obtained by dispersion or deposition of ferromagnetic materials, monomolecular magnets are synthesized according to certain methods, and they are isolated and purified as individual chemical compounds. Their phase purity can be easily confirmed by X-ray diffraction methods. The molecules of such compounds are identical in size, structure, and magnetic properties. These technically valuable qualities make it possible to consider such compounds as components of information storage devices [1,2,3,4], such as in spintronics [5] and in other areas of research on magnetic materials [6]. The choice of lanthanide complexes as a search area for SMMs is not accidental. The internal structure of f-orbitals makes it possible to use them to create systems with interesting magnetic properties. Currently, the main interest in the field of magnetochemistry of lanthanide compounds is focused on the development of strategies for improving the quality of SMMs based on them. The preparation and study of new molecular systems with paramagnetic REE ions is topical because it creates a scientific basis for the design of modern functional materials. The fundamental goal is to search for new effective approaches to the directed synthesis of lanthanide complexes with specific magnetic characteristics, and to study the magnetic behavior of the obtained compounds comprehensively. To reach it, the design of new coordination compounds of lanthanides, taking into account the abidance of the required conditions for manifestation of the SMM properties by them, is necessary. Such conditions are the bistability of the ground electronic state of the ion (i.e., the ion should belong to so-called Kramers ions, which contain an odd number of f-electrons) and the presence of a significant magnetic anisotropy of the Ln3+ ion. In addition, a high-spin ground state of the rare-earth ion is also a preferred property, which manifests itself in a significant value of the magnetic moment. One of the ions that combines these conditions is Dy3+, which determines its popularity as an object of magnetochemical research. At the same time, similar qualities are also inherent in some other lanthanide ions, such as in Yb3+, which has been much less studied.
According to the results of world studies, the interest in the field of molecular magnets has currently shifted to obtaining mononuclear complexes [7]. However, while there are no problems with the choice of the central atom in the complex, the selection of ligands is the most interesting task at the moment. Of particular interest is the study of the influence of the coordination environment of ions and of the complexes’ structures on specifics of their magnetic properties in order to search for promising magnetoactive materials and the study of the possibility for constructing new families of lanthanide coordination compounds by combining ligands with different geometry and denticity to obtain original complexes with pronounced SMM properties.
Previously, for preparation of mononuclear compounds of lanthanides, chelating ligands were most often used, among which the anions of bidentate β-diketones [8,9] and carboxylic acids [10,11], Schiff bases [12,13,14,15,16], and the classical neutral polydentate ligands bpy, phen, and terpy [17,18,19,20,21,22] were the most popular. The study of the magnetic properties of lanthanide aquathiocyanates with heterocyclic diimines has shown that the substitution of coordinated water molecules by a polydentate ligand [23,24,25] leads to deterioration of the SMM properties. Therefore, it was interesting to consider the effect of another acidoligand—the chloride ion—on the magnetic characteristics of aqua complexes of lanthanides with polydentate ligands.
It should be noted that chemists around the world are trying to synthesize increasingly complicated new lanthanide complexes. The pursuit of novelty sometimes leads to the ignorance of the properties of long-known compounds. At the same time, sometimes something you need can be right under your feet. It is surprising that in the modern scientific literature, there are no detailed magnetochemical data for such simple compounds as lanthanide aquachlorides, which are used as precursors for the synthesis of many SMMs, including the record holders in the magnitude of the remagnetization barrier [26]. Due to the extremely high sensitivity of AC magnetic susceptibility at low temperatures (~2 K), knowledge of the dynamics of magnetic behavior can make it possible to determine the presence of microimpurities of initial reagents in the final products. Therefore, it is necessary to know the properties of all substances involved in the work, including the initial and the intermediate components of the reaction. In order to fill the knowledge gap in the field of the magnetic properties of lanthanide aquachlorides, the present study was carried out.
Terpyridine, a commercially available N-donor, was chosen as a polydentate ligand able to form stable complexes with lanthanide ions and to modify their magnetic properties. Terpyridines have attracted great interest as ligands in the last decade due to the possibility of creating a wide variety of coordination polymers and two-dimensional structures; many possibilities of this series of compounds were considered in the work [27].
In this work, we determined the magnetic characteristics of the known LnCl3·6H2O (1Ln) salts in comparison with ones of the [Ln(H2O)4(terpy)Cl]Cl2·3H2O (2Ln) complexes derived from them. A detailed analysis of the experimental data on the dynamic magnetic behavior is carried out, taking into account theoretical calculations that make it possible to choose the most probable path for the magnetic relaxation of the system.

2. Results and Discussion

2.1. Synthesis and Structure Features

Hydrated chlorides of the Ln3+ ions are the most in-demand starting reagents for syntheses of Ln3+ complexes in coordination chemistry, which is due to the stability of the oxidative state of the Ln3+ cation and good solubility of these chlorides in organic solvents. LnCl3·6H2O salts are isostructural with the yttrium analog [Y(H2O)6Cl2]Cl [28]: in the mononuclear cationic aquachloride complex [Ln(H2O)6Cl2]+, the coordination number (CN) of the complexing agent is 8, and the coordination site is LnO6Cl2 (Figure 1a). Ligands, of the molecular (H2O) and anionic (Cl−) varieties, form a polyhedron close in geometry to a square antiprism (D4d). XRD analysis of commercial LnCl3·6H2O (Ln = Gd, Dy, Er, Yb) samples used in the work have shown that all the substances are single-phase and isomorphic with [Y(H2O)6Cl2]Cl [28] (Figure S1).
Self-assembly of aquachlorides with terpy ligands in water–alcohol solutions is accompanied by the formation of cationic complexes [Ln(H2O)4(terpy)Cl]Cl2·3H2O (2Ln) containing one terpy molecule. The outer sphere of the complexes contains two Cl− ions, which compensate for the charge of the cationic complex ion, and three water molecules. The coordination of the tridentate terpy ligand to the lanthanide ion occurs with the substitution of the coordinated water molecules and of one of the coordinated chloride ions; as a result, the charge of the cations in 2Ln complexes increases to 2+. In the 2Ln complexes, the CN of the complexing ion is 8, as in the initial 1Ln aquachlorides; the coordination site is converted to LnO4N3Cl, with the geometry of the environment close to a triangular dodecahedron or a two-capped triangular prism. The coordination sphere of Ln3+ is filled with four water molecules, a chloride anion, and a terpyridine ligand (Figure 1b). The expected composition of the compounds corresponds to the data obtained from elemental analysis.
To date, the existence of seven phases (25 compounds in CCDC [29], CSD version 5.43, updates March 2022) has been discovered in the Ln-terpy-Cl-H2O system. Only one of the phases, [Ln(terpy)(H2O)xCl]Cl2·3H2O (x = 4–5; space group Cm, Ln = La, Ce, Nd, Sm-Er, Lu [30]; Pr [31]), is present for almost the entire series of the lanthanides. The authors of [30] note that the formation of this phase was not observed for Yb, Tm, or Y, but it can be assumed that this statement is applied only to the isolation of single crystals suitable for XRD. This is confirmed by the single-phase complex [Yb(terpy)(H2O)4Cl]Cl2·3H2O isolated by us (Figure 1).
Such a stability of the crystal structure is presumably determined by the fact that four of five (Ln = La-Nd) or three of four (Ln = Tb-Lu) coordinated H2O molecules are directed to a large cavity containing Cl− anions and solvate H2O molecules. A slight shift of coordinated H2O molecules is compensated by shifts of anions and solvate H2O molecules with the preservation of the crystal architecture. For Ln = Sm-Gd, the complexes with coordination numbers of 8 and 9 coexist in the crystal [30]. For 2Dy and 2Er complexes, a disordering of one of the coordinated water molecules is observed [32,33,34].
In accordance with the results of X-ray phase analysis, the complexes of 1Ln (Figure S1) and 2Ln (Figure S2) series are single-phase and isostructural with each other in the series.

2.2. Magnetic Properties of the Complexes

Magnetic susceptibility of complexes of the composition LnCl3·6H2O (Ln = Gd (1Gd), Dy (1Dy), Er (1Er), Yb (1Yb)) and [Ln(H2O)4(terpy)Cl]Cl2·3H2O (Ln = Gd (2Gd), Dy (2Dy), Er (2Er), and Yb (2Yb)) was studied in a temperature range of 2–300 K, in a magnetic field of 5000 Oe. The corresponding χT (T) dependences are shown in Figure 2, and the characteristic χT values are given in Table 1. The values of χT at 300 K are close to the theoretical values for isolated ions of the corresponding lanthanides. For the Gd, Dy, and Yb complexes, the plots of χT (T) dependences typical of mononuclear complexes of the corresponding lanthanides are observed.
The magnetic behavior of the 1Gd and 2Gd complexes in a constant magnetic field is typical of most gadolinium complexes. The χT value remains almost unchanged down to 7 K (1Gd) or 10 K (2Gd), then a sharp drop down to the minimum values at 2 K is observed. This behavior may take place due to the saturation effect in an external magnetic field (Zeeman effect), as well as due to the presence of weak intermolecular antiferromagnetic interactions between the Gd3+ ions.
For Dy and Er complexes, the χT values remain almost unchanged upon cooling from 300 to 100 K, then an initially slow but accelerating decrease in χT is observed with a minimum value at 2 K. This behavior can be caused both by the depopulation of mj sublevels and by weak antiferromagnetic intermolecular interactions of Dy3+ or Er3+ ions.
In the case of Yb (1Yb, 2Yb) complexes, the same picture is observed: a gradual decrease in the value of χT from 300 to 4 K. At temperatures from 4 to 2 K, a noticeably faster decrease of the χT value is noted. In general, the decrease in χT with the temperature lowering is probably determined by the Zeeman effect.
It is well known that the complexes of heavy lanthanides can exhibit slow relaxation of the magnetization, i.e., manifest the properties of SMMs. For all the obtained complexes, the magnetic measurements were carried out in an alternating field in order to determine their ability to be SMMs. Among the studied complexes, it was not possible to detect slow magnetic relaxation for gadolinium compounds. In a zero magnetic field, for all the complexes, no significant values of the out-of-phase component of the magnetic susceptibility χ″ are observed, which indicates a high relaxation rate under these conditions. As it is known, the application of an external magnetic field suppresses the process of quantum tunneling of the magnetization and, as a consequence, it can lead to a slowdown in the relaxation of the magnetization, which was observed for the studied complexes (Figures S3–S8). The optimal value of the external field strength at which the maximum in the frequency dependence of the out-of-phase component of AC magnetic susceptibility χ″(ν) is located in the region of lower frequencies corresponds to the longest magnetization relaxation times, which was experimentally established. The optimal magnetic field strength for the 2Dy complex is 1500 Oe, while for the 1Dy, 1Er, 1Yb, 2Er, and 2Yb complexes, the corresponding value is 1000 Oe. At optimal values of the magnetic field strength, the measurements of the AC magnetic susceptibility were carried out in various temperature ranges. For the 1Dy complex, in contrast to the other studied compounds, an increase in the χ″(ν) dependence, along with the temperature growth, is observed. A similar behavior was observed earlier for the {[Tb(H2O)2][Co(CN)6]·2H2O}n complex in our recent work [35] and in a number of studies by other authors [36,37,38]; such effect may take place due to collective behavior caused by weak dipole-dipole or exchange interactions between the Ln3+ ions.
The obtained χ″(ν) isotherms were approximated by the generalized Debye model (Figure 3, Figures S9 and S10). The Cole–Cole plots were built (Figures S11–S16) and the values of the α parameters, which determine the width of the distribution of relaxation times, were calculated (Tables S1–S6). The maximum value of the α parameter did not exceed 0.22, which suggests a narrow distribution of magnetic relaxation. Based on the data obtained, the corresponding dependences of the relaxation time on the reciprocal temperature τ(1/T) were plotted (Figure 4). In order to determine the relaxation mechanisms, the high-temperature parts of the dependences τ(1/T) were approximated by an Arrhenius equation (τ = τ0 · exp(Ueff/kBT)) that corresponds to the Orbach relaxation mechanism (Figure S17, Table S7). In all of these cases, a significant deviation from the linear behavior of the dependences τ(1/T) in semilogarithmic coordinates indicates the presence of relaxation mechanisms different from the Orbach one.
Without knowledge of the electronic structure and positions of the Kramers doublets of lanthanide ions, the further analysis and correct description of the mechanisms of magnetization relaxation in the compounds under study was difficult. For the correct analysis of the experimental dependences of the relaxation time on temperature, ab initio calculations were carried out (Table S8, Figures S18 and S19). A comparison of the values of the effective energy remagnetization barriers Ueff obtained by approximating the high-temperature part of the experimental dependences of relaxation times on the reciprocal temperature by the Arrhenius equation with the positions of the excited states (Kramers doublets) obtained from ab initio calculations, which showed a good agreement between the Ueff values (28, 36, and 21 cm−1) and energies of the first excited state KD2 (30, 36, and 26 cm−1) for the 1Er, 2Dy, and 2Er complexes, respectively (Table S8). This coincidence indicates that the priority relaxation of the magnetization in the complexes 1Er, 2Dy, and 2Er occurs through the first excited state, which implies thermally stimulated quantum tunneling. In the case of complexes 1Dy, 1Yb, and 2Yb, the Ueff values (22, 54, and 20 cm−1), are much smaller than the KD2 energies (54, 193, and 84 cm−1, resp.), which makes it possible to assume that, apparently, the main relaxation mechanism in the 1Dy, 1Yb, and 2Yb complexes is the Raman mechanism. It should be noted that in most of the cases reported in the literature, Yb3+ complexes relax through a Raman process [39,40,41,42,43,44,45,46,47,48].
To determine the parameters of the relaxation processes for 1Dy, 1Yb, and 2Yb complexes, the τ(1/T) dependences were approximated using the equation describing the Raman relaxation (τRaman−1 = CRaman · Tn_Raman; Table S9). However, for the 1Dy complex, it was not possible to satisfactorily approximate the experimental data in a whole temperature range by the Raman mechanism only, which indicates the presence of relaxation mechanisms additional to the Raman mechanism. The direct relaxation mechanism (τdirect−1 = AdirectHn_directT), which manifests itself in non-zero magnetic fields, can be one of such additional mechanisms. When approximating the experimental τ(1/T) dependence of 1Dy complex by the sum of Raman and direct relaxation mechanisms, a good agreement between the theoretical curve and experimental data was obtained. Similarly, for 1Er, 2Dy, and 2Er complexes, a satisfactory agreement between the theoretical curves and the experimental data was obtained by approximation using the sum of the Orbach and the direct relaxation mechanisms. The fit by sum of Raman and Orbach mechanisms lead to overparameterization (see Table S10).
The parameters of the best approximations of all studied complexes exhibiting slow magnetic relaxation are given in Table 2.
For the 2Yb compound, nRaman values are close to those for the systems with low-lying thermally populated excited states (nRaman = 5). For complex 1Yb, the values of the Raman scattering index is between the values characteristic of non-Kramers ions (nRaman = 7) and Kramers ions (nRaman = 9). This may be due to perturbations caused by the influence of the crystal field of ligands, as well as the distribution of donor centers relative to the central metal ion.
Even taking into account the fact that relaxation in ytterbium complexes mostly occurs according to the Raman mechanism, and that the Ueff values for them are phenomenological characteristics, the discovery of one of the highest values of the magnetization reversal barrier among the Yb compounds, namely, Ueff/kB = 78 K for the 1Yb complex, can be considered as one of the important points of this article. The latest published record value of Ueff/kB for Yb complex is nearly the same, 77 K (53.6 cm−1) [48]; however, the complex [Yb2(5-Me-L)2(DMF)2(NO3)2]·DMF (L = 5-Me-LH2 = N′-(2-hydroxy-5-methylbenzylidene)picolinohydrazide) studied in that work required synthetic efforts to be prepared, unlike the easily accessible aquachloride 1Yb.
It is usually considered that the Dy3+ ion has the best properties for the formation of SMMs, but earlier, we obtained two series of complexes in which the Yb derivatives exhibited higher Ueff values than the dysprosium ones, similar to the 1Ln series [35,49]. It is important to note a possibility of influence of the geometry of the magnetic center local environment and of the ligands’ mutual arrangement on the slow magnetic relaxation [39]. Analysis of the results of magnetic property measurements and of ab initio calculations for 1Dy and 2Dy complexes shows a significant difference in the predominant way of magnetization relaxation. This is probably due to fixation of a more favorable mutual arrangement of donor centers in 2Dy in comparison to the “freedom” in 1Dy, which leads to a significant admixture of the ±9/2 state to the ±15/2 ground state in the case of 1Dy (Figure S18). The latter, apparently, leads to the relaxation of the magnetization according to the Raman mechanism.
In the case of the Dy3+ ion, considering its highest magnitude mJ state, the electron cloud of 4f orbitals has an oblate shape, while in the case of the Er3+ ion, it is prolate, and even more prolate in Yb3+ case [50]. For ions having prolate and oblate shapes of the electron cloud, the different dependences of the change in relaxation parameters on the geometry of the local ion environment should be expected. When passing from complexes of 1Ln series to complexes of 2Ln series, the coordination polyhedron changes from a square antiprism (Table S11) to an intermediate polyhedron between a triangular dodecahedron and a two-capped triangular prism (Table S12); this fact was confirmed by calculations using the SHAPE program [51]. Respectively, the same change in the coordination environment of the ion has the opposite effect on the SMM properties of the corresponding derivatives of these lanthanides.
For 2Ln series of complexes, the coordination polyhedron contains four water oxygens, three nitrogen atoms of the terpy ligand, and one Cl− ion, while for the 1Ln series, the coordination polyhedron includes six O atoms of water and two Cl− ions. The heterogeneity of the coordination polyhedron in passing from the starting 1Ln compounds to 2Ln complexes increases significantly, and it is also a factor influencing the values of the magnetization reversal barriers. In our case, the effective magnetization reversal barrier for the Dy complexes increases, while for the Er and Yb complexes it decreases (Table S7). It was also shown in [52] that a more heterogeneous coordination environment can positively affect the magnitude of the remagnetization barrier for Dy complexes. Comparison of the results of our earlier works and the data of the present work together with analysis of the literature data shows that for Yb complexes, an increase in the inhomogeneity of the environment does not affect the Ueff value unambiguously [39,53,54]. However, for Er complexes, the decrease in the magnetization reversal barrier with an increase in the heterogeneity of the environment is a rule rather than an exception [35].
Comparison of the relaxation times τ found for 1Ln and 2Ln complexes reveals a changing trend similar to that of the effective barrier value Ueff. The magnetization relaxation time for the oblate Dy3+ ion at the same temperature (for example, at 4 K) in the 2Dy molecule (2.3 × 10−3 s) is almost two orders of magnitude greater than the analogous value for 1Dy (3.7 × 10−5 s). At the same time, for Er and Yb complexes containing the ions with the overall prolate shape of the electron density, an inverse dependence is observed: the geometry of the local environment and the strength of the ligand field in the 1Ln structure (5.6 × 10−3 and 6.8 × 10−4 s for Ln = Er and Yb, respectively) contribute to an increase in relaxation time by an order of magnitude with respect to 2Ln (1.0 × 10−4 and 1.4 × 10−4 s for Ln = Er and Yb, resp). The experimental data obtained are in good agreement with the conclusions of the work of Rinehart and Long [50].
In order to better understand the influence of the geometry of the complexes on their SMM properties, the anisotropy axes were calculated ab initio for the studied complexes (Figures S20–S25). The angles between the anisotropy axes and the elements of symmetry of the coordination polyhedra were found (Table S13). Among the complexes of 1Ln series, the smallest angle between the magnetic anisotropy axis and the twofold symmetry axis (60°) is observed for the 1Yb complex, while for the complexes 1Dy and 1Er, it is 90°. This relatively small angle, in comparison with 1Dy and 1Er complexes, may serve as an additional explanation for the high remagnetization barrier found for 1Yb complex, since the SMM properties are favored by the smallest angle between the symmetry axis and the easy magnetization one [53]. In the complexes of 2Ln series, the coordination polyhedron is irregular and lacks an axis of symmetry; therefore, it is difficult to draw conclusions based on the symmetry properties of the coordination polyhedron in this case.

3. Materials and Methods

The following commercial reagents and solvents were used as purchased: LnCl3·6H2O (Ln = Gd, Dy, Er, Yb) (1Ln), terpy (Aldrich), MeOH, EtOH. All operations were carried out in the air. The powder X-ray diffraction patterns were recorded on a Bruker D8 ADVANCE X-Ray Diffractometer (CuKα, Ni-filter, LYNXEYE detector, reflection geometry). Elemental analyses were carried out using standard techniques on a EUROEA 3000 analyzer.
Magnetic susceptibility measurements were performed using a Quantum Design PPMS-9 susceptometer. This instrument works in the temperature range of 2–300 K under applied DC fields from −9 to 9 T. For AC susceptibility measurements, an oscillating AC field of 5, 3, and 1 Oe amplitude for 10–100 Hz, 100–1000 Hz, and 10–10,000 Hz ranges of oscillating frequency, respectively, was applied. All the magnetic measurements were performed on polycrystalline samples sealed in polyethylene bags and covered with mineral oil in order to prevent the field-induced orientation of crystallites. The paramagnetic components of the magnetic susceptibility χ were determined by taking into account the diamagnetic contribution evaluated from Pascal’s constants and the contributions of the sample holder and mineral oil.

3.1. Synthesis of [Ln(H2O)4(terpy)Cl]Cl2·3H2O (2Ln) Compounds

The complexes were obtained according to the following procedure: LnCl3·6H2O (0.25–0.5 mmol) was dissolved in 10 mL EtOH; the solution formed was added to a heated (~50 °C) solution of terpy (0.5–1.0 mmol) in 40 mL EtOH. The resulting mixture was stirred for ~45 min, then the homogeneous solution was transferred into a crystallizer for concentration at room temperature, the solid phase was separated on a filter, washed with EtOH, and dried in air. The single-phase state of the obtained samples was confirmed by powder XRD. The exact quantities of the starting substances used and yields are indicated below for each case.
Complex 2Gd: Complex 1Gd (0.0920 g, 0.248 mmol) and terpy (0.1114 g, 0.478 mmol) were taken. Yield of 2Gd 0.0896 g, 58% with respect to Gd. Calculated for C15H25N3O7Cl3Gd: C 28.92; H 4.04; N 6.75. Found %: C 28.60; H 3.91; N 6.44.
Complex 2Dy: Complex 1Dy (0.0935 g, 0.248 mmol) and terpy (0.1211 g, 0.519 mmol) were taken. Yield of 2Dy 0.091 g, 58% with respect to Dy. Calculated for C15H25Cl4N3O7Dy: C 27.14; H 3.80; N 6.33. Found %: C 27.38; H 3.75; N 5.95.
Complex 2Er: Complex 1Er (0.095 g, 0.249 mmol) and terpy (0.1156 g, 0.495 mmol) were taken. Yield of 2Er 0.0872 g, 55% with respect to Er. Calculated for C15H25N3O7Cl3Er (M 632.93), %: C 28.46; H 3.98; N 6.64. Found %: C 28.38; H 3.75; N 6.80.
Complex 2Yb: Complex 1Yb (0.1995 g, 0.514 mmol) and terpy (0.2102 g, 0.901 mmol) were taken. Yield of 2Yb 0.2216 g, 67.5% with respect to Yb. Calculated for C15H25N3O7Cl3Yb (M 638.77), %: C 28.20, H 3.94, N 6.57. Found %: C 28.08; H 3.93; N 6.99.

3.2. Computations

A CASSCF/RASSI-SO/SINGLE_ANISO post Hartree-Fock ab initio calculation of 1Ln and 2Ln has been performed using OpenMolcas package [55,56]. The relativistic effect of Ln ion has been taken into account by DKH Hamiltonian [57]. The disk space of the two-electron integral has been reduced by Cholesky decomposition technique [58]. We have used an ANO-RCC VTZP basis set for Ln, N, O, Cl, and a VDZP basis set for C and H. The CASSCF calculation has been performed by considering thirteen (Yb), eleven (Er), and nine (Dy) electrons in seven 4f orbitals, i.e., using CAS (n, 7) active space, where n = 13, 11, 9, respectively. We have computed 7 doublets for Yb, 35 quartet and 112 doublets for Er, and 21 sextets for Dy complexes configuration state functions (CSFs) using this active space. These CSFs have been mixed by RASSI-SO to compute spin-orbit energy levels [59]. Finally, the g-tensor, magnetic susceptibility, molar magnetization, and barrier height for magnetization reversal have been calculated using the SINGLE_ANISO, which interfaced with RASSI-SO energies [60].

4. Conclusions

Thus, for two series of lanthanide complexes containing the Ln3+ ions in square-antiprismatic coordination, [Ln(H2O)6Cl2]Cl (Ln = Gd (1Gd), Dy (1Dy), Er (1Er), Yb (1Yb)), and, in the irregular average between the triangular-dodecahedral and double-capped-triangular-prismatic coordination, [Ln(H2O)4(terpy)Cl]Cl2·3H2O (Ln = Gd (2Gd), Dy (2Dy), Er (2Er), Yb (2Yb)), the magnetic properties were studied and compared. The studies of compounds of the 1Ln series in an alternating magnetic field has shown an increase in the values of effective magnetization reversal barriers on going from 1Dy to 1Er. For compounds of the 2Ln series, the inverse dependence is observed: the effective magnetization reversal barrier of the 2Dy complex is significantly higher than the barrier of 2Er complex. Thus, the multidirectional effect of the introduction of terpy ligand into the coordination sphere of the lanthanide on the SMM properties of the complexes, depending on the conformation of the f-electron shell of the Ln3+ ion—namely, the weakening of the SMM properties for the Dy3+ ion having the oblate shape of the electron cloud, and the strengthening of them for the prolate Er3+ ion—was demonstrated. It was shown that for Yb complexes, the predominant mechanism of magnetic relaxation is the Raman mechanism.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/magnetochemistry9010031/s1, Figure S1: Powder XRD patterns of complexes 1Gd-1Yb in comparison with the calculated powder XRD pattern of ErCl3·6H2O; Figure S2: Powder XRD patterns of complexes 2Gd-2Yb in comparison with the calculated powder XRD pattern of [Y(H2O)4(terpy)Cl]Cl2·3H2O; Figure S3: Frequency dependencies of in-phase, χ′ (left) and out-of-phase, χ″ (right) components of dynamic magnetic susceptibility for complex 1Dy at T = 2 K under various dc magnetic fields. Solid lines are visual guides; Figure S4: Frequency dependencies of in-phase, χ′ (left) and out-of-phase, χ″ (right) components of dynamic magnetic susceptibility for complex 1Er at T = 2 K under various dc magnetic fields. Solid lines are visual guides; Figure S5: Frequency dependencies of in-phase, χ′ (left) and out-of-phase, χ″ (right) components of dynamic magnetic susceptibility for complex 1Yb at T = 2 K under various dc magnetic fields. Solid lines are visual guides; Figure S6: Frequency dependencies of in-phase, χ′ (left) and out-of-phase, χ″ (right) components of dynamic magnetic susceptibility for complex 2Dy at T = 2 K under various dc magnetic fields. Solid lines are visual guides; Figure S7: Frequency dependencies of in-phase, χ′ (left) and out-of-phase, χ″ (right) components of dynamic magnetic susceptibility for complex 2Er at T = 2 K under various dc magnetic fields. Solid lines are visual guides; Figure S8: Frequency dependencies of in-phase, χ′ (left) and out-of-phase, χ″ (right) components of dynamic magnetic susceptibility for complex 2Yb at T = 2 K under various dc magnetic fields. Solid lines are visual guides; Figure S9: Frequency dependencies of the in-phase, χ′ (top) and out-of-phase, χ″ (bottom) components of the AC susceptibility for 1Dy in 1000 Oe DC field (left) and for 2Dy in 1500 Oe DC field (right). Solid lines were fitted using the generalized Debye model; Figure S10: Frequency dependencies of the in-phase, χ′ (top) and out-of-phase, χ″ (bottom) components of the AC susceptibility between 2.5 and 5 K for 1Er (left) and between 2 and 4 K for 2Er (right) in 1000 Oe DC field. Solid lines were fitted using the generalized Debye model; Figure S11: The Cole–Cole plot for 1Dy; Table S1: The values of the α parameters obtained by approximating the dependences χ′(ν) and χ″(ν) for the 1Dy complex by the generalized Debye model; Figure S12: The Cole–Cole plot for 2Dy; Table S2: The values of the α parameters obtained by approximating the dependences χ′(ν) and χ″(ν) for the 2Dy complex by the generalized Debye model; Figure S13: The Cole–Cole plot for 1Er; Table S3: The values of the α parameters obtained by approximating the dependences χ′(ν) and χ″(ν) for the 1Er complex by the generalized Debye model; Figure S14: The Cole–Cole plot for 2Er; Table S4: The values of the α parameters obtained by approximating the dependences χ′(ν) and χ″(ν) for the 2Er complex by the generalized Debye model; Figure S15: The Cole–Cole plot for 1Yb; Table S5: The values of the α parameters obtained by approximating the dependences χ′(ν) and χ″(ν) for the 1Yb complex by the generalized Debye model; Figure S16: The Cole–Cole plot for 2Yb; Table S6: The values of the α parameters obtained by approximating the dependences χ′(ν) and χ″(ν) for the 2Yb complex by the generalized Debye model; Figure S17: Plots of the τ(1/T) dependences for 1Dy, 1Er, 1Yb, 2Dy, 2Er, and 2Yb in a DC field of optimal intensity. The blue dotted lines represent the best-fit of experimental data by the Arrhenius equation (Orbach mechanism) at high temperatures. The green solid lines represent the best-fit of experimental data by the Raman relaxation mechanism only. The red solid lines represent the best-fit of experimental data by sum of Raman and Orbach relaxation mechanisms.; Table S7: Parameters of the Orbach relaxation processes for the complexes obtained by approximating the high-temperature part of the temperature dependences of the relaxation time; Table S8: The ab initio computed energy levels (cm−1) with the associated g-tensors of the four lowest KDs for 1Ln and 2Ln; Table S9: Parameters of the Raman relaxation processes for the complexes obtained by approximating the temperature dependences of the relaxation time; Table S10: Parameters for approximation by the sum Raman and Orbach relaxation processes for the complexes obtained by approximating the temperature dependences of the relaxation time (red solid lines on Figure S17). Figure S18: Mechanisms of magnetic relaxation of complexes 1Ln and 2Ln. The red line indicates the QTM and TA-QTM via ground KD and excited KD respectively. The olive line indicates the transition probability between KD1 and KD2. The blue line indicates mechanism of Orbach relaxation. The blue characters indicate the mJ composition of each KD; Figure S19: Comparison of the computed magnetic susceptibility of 1Ln and 2Ln with experiment. Points correspond to the experimental magnetic susceptibility data. The solid lines are the computed magnetic susceptibilities; Table S11: Shape Measures calculation for [Y(H2O)6Cl2]Cl, isostructural with complexes 1Ln; Table S12: Shape Measures calculation for [Lu(H2O)4(terpy)Cl]Cl2·3H2O, isostructural with complexes 2Ln; Figure S20: The orientation of the anisotropic axis for the ground state for 1Dy according to ab initio calculations; Figure S21: The orientation of the anisotropic axis for the ground state for 1Er according to ab initio calculations; Figure S22: The orientation of the anisotropic axis for the ground state for 1Yb according to ab initio calculations; Figure S23: The orientation of the anisotropic axis for the ground state for 2Dy according to ab initio calculations; Figure S24: The orientation of the anisotropic axis for the ground state for 2Er according to ab initio calculations; Figure S25: The orientation of the anisotropic axis for the ground state for 2Yb according to ab initio calculations; Table S13: Calculation of the angles between the magnetic anisotropy axes and the axes of symmetry of the coordination polyhedrons in 1Ln and 2Ln complexes.

Author Contributions

Conceptualization, S.P.P. and P.S.K.; synthesis, S.P.P.; structural studies, A.B.I.; magnetic measurements and calculations, N.N.E. and K.A.B.; writing—original draft preparation, S.P.P., K.A.B. and A.B.I.; writing—review and editing, P.S.K., N.N.E. and K.A.B.; supervision, N.N.E. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by the Russian Science Foundation, grant number 22-23-00574.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

The data presented in this study are available on request from the corresponding author.

Acknowledgments

This research was performed using the equipment of the JRC PMR IGIC RAS.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Vaz, R.C.; Esteves, I.O.; Oliveira, W.X.; Honorato, J.; Martins, F.T.; Marques, L.F.; dos Santos, G.L.; Ricardo, O.; Freire, R.O.; Jesus, L.T.; et al. Mononuclear lanthanide(III)-oxamate complexes as new photoluminescent field-induced single-molecule magnets: Solid-state photophysical and magnetic properties. Dalton Trans. 2020, 49, 16106–16124. [Google Scholar] [CrossRef] [PubMed]
  2. Zhu, Z.; Guo, M.; Li, X.L.; Tang, J. Molecular magnetism of lanthanide: Advances and perspectives. Coord. Chem. Rev. 2019, 378, 350–364. [Google Scholar] [CrossRef]
  3. Zabala-Lekuona, A.; Seco, J.M.; Colacio, E. Single-Molecule Magnets: From Mn12-ac to dysprosium metallocenes, a travel in time. Coord. Chem. Rev. 2021, 441, 213984. [Google Scholar] [CrossRef]
  4. Parmar, V.S.; Mills, D.P.; Winpenny, R.E.P. Mononuclear Dysprosium Alkoxide and Aryloxide Single-Molecule Magnets. Chem. Eur. J. 2021, 27, 7625–7645. [Google Scholar] [CrossRef] [PubMed]
  5. Lu, J.; Guo, M.; Tang, J. Recent Developments in Lanthanide Single-Molecule Magnets. Chem. Asian J. 2017, 12, 2772–2779. [Google Scholar] [CrossRef]
  6. Cador, O.; Le Guennic, B.; Pointillart, F. Electro-activity and magnetic switching in lanthanide-based single-molecule magnets. Inorg. Chem. Front. 2019, 6, 3398–3417. [Google Scholar] [CrossRef] [Green Version]
  7. Casanovas, B.; Porcar, O.; Speed, S.; Vicente, R.; Font-Bardía, M.; El Fallah, M.S. Field-Induced SMM and Vis/NIR Luminescence on Mononuclear Lanthanide Complexes with 9-Anthracenecarboxylate and 2,2′:6,2″-Terpyridine. Magnetochemistry 2021, 7, 124. [Google Scholar] [CrossRef]
  8. Canaj, A.B.; Sing, M.K.; Wilson, C.; Rajaraman, G.; Murrie, M. Chemical and in silico tuning of the magnetisation reversal barrier in pentagonal bipyramidal Dy(III) single-ion magnets. Chem. Comm. 2018, 54, 8273–8276. [Google Scholar] [CrossRef] [Green Version]
  9. Li, L.L.; Su, H.D.; Liu, S.; Xu, Y.C.; Wang, W.Z. A new air- and moisture-stable pentagonal-bipyramidal Dy III single-ion magnet based on the HMPA ligand. Dalton Trans. 2019, 48, 2213–2219. [Google Scholar] [CrossRef]
  10. Gavrikov, A.V.; Efimov, N.N.; Dobrokhotova, Z.V.; Ilyukhin, A.B.; Vasilyev, P.N.; Novotortsev, V.M. Novel mononuclear Ln complexes with pyrazine-2-carboxylate and acetylacetonate co-ligands: Remarkable single molecule magnet behavior of a Yb derivative. Dalton Trans. 2017, 46, 11806–11816. [Google Scholar] [CrossRef]
  11. Jiménez, J.R.; Diaz-Ortega, I.F.; Ruiz, E.; Aravena, D.; Pope, S.J.A.; Colacio, E.; Herrera, J.M. Lanthanide Tetrazolate Complexes Combining Single-Molecule Magnet and Luminescence Properties: The Effect of the Replacement of Tetrazolate N3 by β-Diketonate Ligands on the Anisotropy Energy Barrier. Chem. Eur. J. 2016, 22, 14548–14559. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  12. Sugita, M.; Ishikawa, N.; Ishikawa, T.; Koshihara, S.Y.; Kaizu, Y. Static Magnetic-Field-Induced Phase Lag in the Magnetization Response of Tris(dipicolinato)lanthanides. Inorg. Chem. 2006, 45, 1299–1304. [Google Scholar] [CrossRef] [PubMed]
  13. Boulon, M.E.; Cucinotta, G.; Luzon, J.; Degl’Innocenti, C.; Perfetti, M.; Bernot, K.; Calvez, G.; Caneschi, A.; Sessoli, R. Magnetic Anisotropy and Spin-Parity Effect Along the Series of Lanthanide Complexes with DOTA. Angew. Chem. Int. Ed. 2013, 52, 350–354. [Google Scholar] [CrossRef] [PubMed]
  14. Mylonas-Margaritis, I.; Maniaki, D.; Mayans, J.; Ciammaruchi, L.; Bekiari, V.; Raptopoulou, C.P.; Psycharis, V.; Christodoulou, S.; Escuer, A.; Perlepes, S.P. Mononuclear Lanthanide(III)-Salicylideneaniline Complexes: Synthetic, Structural, Spectroscopic, and Magnetic Studies. Magnetochemistry 2018, 4, 45. [Google Scholar] [CrossRef] [Green Version]
  15. Liu, J.L.; Yuan, K.; Leng, J.D.; Ungur, L.; Wernsdorfer, W.; Guo, F.S.; Chibotaru, L.F.; Tong, M.L. A Six-Coordinate Ytterbium Complex Exhibiting Easy-Plane Anisotropy and Field-Induced Single-Ion Magnet Behavior. Inorg. Chem. 2012, 51, 8538–8544. [Google Scholar] [CrossRef]
  16. Zhang, L.; Zhang, Y.Q.; Zhang, P.; Zhao, L.; Guo, M.; Tang, J. Single-Molecule Magnet Behavior Enhanced by Synergic Effect of Single-Ion Anisotropy and Magnetic Interactions. Inorg. Chem. 2017, 56, 7882–7889. [Google Scholar] [CrossRef]
  17. Bar, A.K.; Kalita, P.; Sutter, J.P.; Chandrasekhar, V. Pentagonal-Bipyramid Ln(III) Complexes Exhibiting Single-Ion-Magnet Behavior: A Rational Synthetic Approach for a Rigid Equatorial Plane. Inorg. Chem. 2018, 57, 2398–2401. [Google Scholar] [CrossRef]
  18. Saha, R.; Goswami, S.; Biswas, S.; Steele, I.M.; Dey, K.; Jana, A.D.; Kumar, S. A dynamic metal–organic supramolecular host based on weak π-stacking interactions incorporating 2D water-chloride-methanolic supramolecular sheet. Inorg. Chim. Acta 2014, 423, 123–132. [Google Scholar] [CrossRef]
  19. Puntus, L.N.; Lyssenko, K.A.; Antipin, M.Y.; Bünzli, J.C.G. Role of Inner- and Outer-Sphere Bonding in the Sensitization of Eu III-Luminescence Deciphered by Combined Analysis of Experimental Electron Density Distribution Function and Photophysical Data. Inorg. Chem. 2008, 47, 2008–11095. [Google Scholar] [CrossRef]
  20. Puntus, L.N.; Lyssenko, K.A.; Pekareva, I.S.; Bünzli, J.C.G. Intermolecular Interactions as Actors in Energy-Transfer Processes in Lanthanide Complexes with 2,2′-Bipyridine. J. Phys. Chem. B 2009, 113, 9265–9277. [Google Scholar] [CrossRef]
  21. Lhoste, J.; Henry, N.; Loiseau, T.; Abraham, F. Molecular assemblies of trichloride neodymium and europium complexes chelated by 1,10-phenanthroline. Polyhedron 2011, 30, 1289–1294. [Google Scholar] [CrossRef]
  22. Alfi, N.; Khorasani-Motlagh, M.; Rezvani, A.R.; Noroozifar, M.; Molčanov, K. Synthesis, characterization, crystal structure, DNA/BSA binding ability and antibacterial activity of asymmetric europium complex based on 1,10-phenanthroline. J. Mol. Struct. 2017, 1137, 771–783. [Google Scholar] [CrossRef]
  23. Petrosyants, S.P.; Ilyukhin, A.B.; Gavrikov, A.V.; Mikhlina, Y.A.; Puntus, L.N.; Varaksina, E.A.; Efimov, N.N.; Novotortsev, V.M. Luminescent and magnetic properties of mononuclear lanthanide thiocyanates with terpyridine as auxiliary ligand. Inorg. Chim. Acta 2019, 486, 499–505. [Google Scholar] [CrossRef]
  24. Petrosyants, S.P.; Dobrokhotova, Z.V.; Ilyukhin, A.B.; Efimov, N.N.; Gavrikov, A.V.; Vasilyev, P.N.; Novotortsev, V.M. Mononuclear Dysprosium Thiocyanate Complexes with 2,2′-Bipyridine and 1,10-Phenanthroline: Synthesis, Crystal Structures, SIM Behavior, and Solid-Phase Transformations. Eur. J. Inorg. Chem. 2017, 2017, 3561–3569. [Google Scholar] [CrossRef]
  25. Petrosyants, S.P.; Ilyukhin, A.B.; Efimov, N.N.; Gavrikov, A.V.; Novotortsev, V.M. Self-assembly and SMM properties of lanthanide cyanocobaltate chain complexes with terpyridine as blocking ligand. Inorg. Chim. Acta 2018, 482, 813–820. [Google Scholar] [CrossRef]
  26. Giansiracus, M.J.; Al-Badran, S.; Kostopoulos, A.K.; Whitehead, G.F.S.; Collison, D.; Tuna, F.; Winpenny, R.E.P.; Chilton, N.F. A Large Barrier Single-Molecule Magnet without Magnetic Memory. Dalton Trans. 2019, 48, 10795–107981. [Google Scholar] [CrossRef]
  27. Housecroft, C.E.; Constable, E.C. The terpyridine isomer game: From chelate to coordination network building block. Chem. Commun. 2020, 56, 10786–10794. [Google Scholar] [CrossRef]
  28. Bell, M.T.; Smith, A.J. Structure of hexaaquadichloroyttrium(III) chloride. Acta Crystallogr. Sect. C 1990, 46, 960–962. [Google Scholar] [CrossRef]
  29. Groom, C.R.; Bruno, I.J.; Lightfoot, M.P.; Ward, S.C. The Cambridge Structural Database. Acta Crystallogr. Sect. B 2016, 72, 171–179. [Google Scholar] [CrossRef]
  30. Kepert, C.; Lu, W.; Skelton, B.; White, A. Structural Systematics of Rare Earth Complexes. V. The Hydrated 1: 1 Adducts of 2,2′:6′,2″-Terpyridine With the Lanthanoid(III) Chlorides. Aust. J. Chem. 1994, 47, 365–384. [Google Scholar] [CrossRef]
  31. Glick, M.D.; Radonovich, L.J. Stereochemistry of the chloropentaaquoterpyridylpraseodymium(III) ion. Inorg. Chem. 1971, 10, 1463–1468. [Google Scholar] [CrossRef]
  32. Lhoste, J.; Pérez-Campos, A.; Henry, N.; Loiseau, T.; Rabu, P.; Abraham, F. Chain-like and dinuclear coordination polymers in lanthanide (Nd, Eu) oxochloride complexes with 2,2′:6′,2″-terpyridine: Synthesis, XRD structure and magnetic properties. Dalton Trans. 2011, 40, 9136–9144. [Google Scholar] [CrossRef] [PubMed]
  33. Curnock, E.; Levason, W.; Light, M.E.; Luthra, S.K.; McRobbie, G.; Monzittu, F.M.; Reid, G.; Williams, R.N. Group 3 metal trihalide complexes with neutral N-donor ligands-exploring their affinity towards fluoride. Dalton Trans. 2018, 7, 6059–6068. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  34. Kepert, C.J.; Skeleton, B.W.; White, A.H. Structural Systematics of Rare Earth Complexes. VII. Crystal Structure of Bis(2,2′/6′,2ESC-Terpyridinium) Octaaquaterbium(III) Heptachloride Hydrate. Aust. J. Chem. 1994, 47, 391–396. [Google Scholar] [CrossRef]
  35. Babeshkin, K.A.; Gavrikov, A.V.; Petrosyants, S.P.; Ilyukhin, A.B.; Belova, E.V.; Efimov, N.N. Unexpected Supremacy of Non-Dysprosium Single-Ion Magnets within a Series of Isomorphic Lanthanide Cyanocobaltate(III) Complexes. Eur. J. Inorg. Chem. 2000, 46, 4380–4390. [Google Scholar] [CrossRef]
  36. Feng, M.; Tong, M.L. Single Ion Magnets from 3d to 5f: Developments and Strategies. Chem. Eur. J. 2018, 24, 7574–7594. [Google Scholar] [CrossRef]
  37. Mamontova, E.; Long, J.; Ferreira, R.; Botas, A.; Luneau, D.; Guari, Y.; Carlos, L.; Larionova, J. Magneto-Luminescence Correlation in the Textbook Dysprosium(III) Nitrate Single-Ion Magnet. Magnetochemistry 2016, 2, 41. [Google Scholar] [CrossRef] [Green Version]
  38. Habib, F.; Brunet, G.; Vieru, V.; Korobkov, I.; Chibotaru, L.F.; Murugesu, M. Significant Enhancement of Energy Barriers in Dinuclear Dysprosium Single-Molecule Magnets through Electron-Withdrawing Effects. J. Am. Chem. Soc. 2013, 135, 13242–13245. [Google Scholar] [CrossRef]
  39. Petrosyants, S.P.; Babeshkin, K.A.; Gavrikov, A.V.; Ilyukhin, A.B.; Belova, E.V.; Efimov, N.N. Towards comparative investigation of Er- and Yb-based SMMs: The effect of the coordination environment configuration on the magnetic relaxation in the series of heteroleptic thiocyanate complexes. Dalton Trans. 2019, 48, 12644–12655, and references therein. [Google Scholar] [CrossRef]
  40. Pointillart, F.; Cador, O.; Le Guennic, B.; Ouahab, L. Uncommon Lanthanide Ions in Purely 4f Single Molecule Magnets. Coord. Chem. Rev. 2017, 346, 150–175. [Google Scholar] [CrossRef]
  41. Borah, A.; Murugavel, R. Magnetic relaxation in single-ion magnets formed by less-studied lanthanide ions Ce(III), Nd(III), Gd(III), Ho(III), Tm(II/III) and Yb(III). Coord. Chem. Rev. 2022, 453, 214288, and references therein. [Google Scholar] [CrossRef]
  42. Mondal, A.; Konar, S. Strong Equatorial Crystal Field Enhances the Axial Anisotropy and Energy Barrier for Spin Reversal Process in Yb2 Single Molecule Magnets. Chem. Eur. J. 2021, 27, 3449–3456. [Google Scholar] [CrossRef]
  43. Leng, J.D.; Hua, Q.Y.; Liu, W.T.; Tao, Z.X.; Tan, N.W.; Wang, Y.F.; Lin, W.Q. Slow magnetic relaxation of mononuclear complexes based on uncommon Kramers lanthanide ions Ce III, Sm III and Yb III. Dalton Trans. 2022, 51, 12661–12669. [Google Scholar] [CrossRef]
  44. Handzlik, G.; Magott, M.; Arczyński, M.; Sheveleva, A.M.; Tuna, F.; Baran, S.; Pinkowicz, D. Identical anomalous Raman relaxation exponent in a family of Single Ion Magnets: Towards reliable Raman relaxation determination. Dalton Trans. 2020, 49, 11942–11949. [Google Scholar] [CrossRef]
  45. Fondo, M.; Corredoira-Vázquez, J.; García Deibe, A.M.; Matalobos, J.S.; Amoza, M.; Botas, A.M.P.; Ferreira, R.A.S.; Carlos, L.D.; Colacio, E. Field-Induced slow magnetic relaxation and luminescence thermometry in a mononuclear ytterbium complex. Inorg. Chem. Front. 2020, 7, 3019–3029. [Google Scholar] [CrossRef]
  46. Han, L.Z.; Jiao, C.Q.; Chen, W.C.; Shao, K.Z.; Jin, L.Y.; Su, Z.M. Assembly of tetra-nuclear Yb III-containing selenotungstate clusters: Synthesis, structures, and magnetic properties. Dalton Trans. 2021, 50, 11535–11541. [Google Scholar] [CrossRef]
  47. Shmelev, M.A.; Polunin, R.A.; Gogoleva, N.V.; Evstifeev, I.S.; Vasilyev, P.N.; Dmitriev, A.A.; Varaksina, E.A.; Efimov, N.N.; Taydakov, I.V.; Sidorov, A.A.; et al. Cadmium-Inspired Self-Polymerization of {LnIIICd2} Units: Structure, Magnetic and Photoluminescent Properties of Novel Trimethylacetate 1D-Polymers (Ln = Sm, Eu, Tb, Dy, Ho, Er, Yb). Molecules 2021, 26, 4296. [Google Scholar] [CrossRef]
  48. Mondal, A.; Konar, S. A remarkable energy barrier for spin reversal in a field induced dinuclear ytterbium single molecule magnet. Dalton Trans. 2021, 50, 13666–13670. [Google Scholar] [CrossRef]
  49. Gavrikov, A.V.; Efimov, N.N.; Ilyukhin, A.B.; Dobrokhotova, Z.V.; Novotortsev, V.M. Yb3+ can be much better than Dy3+: SMM properties and controllable self-assembly of novel lanthanide 3,5-dinitrobenzoate-acetylacetonate complexes. Dalton Trans. 2018, 47, 6199–6209. [Google Scholar] [CrossRef]
  50. Rinehart, J.D.; Long, J.R. Exploiting single-ion anisotropy in the design of f-element single-molecule magnets. Chem. Sci. 2011, 2, 2078–2085. [Google Scholar] [CrossRef]
  51. Llunell, M.; Casanova, D.; Cirera, J.; Alemany, P.; Alvarez, S. SHAPE, v2.1; University of Barcelona: Barcelona, Spain; The Hebrew University of Jerusalem: Jerusalem, Israel, 2013. [Google Scholar]
  52. Aravena, D.; Ruiz, E. Shedding Light on the Single-Molecule Magnet Behavior of Mononuclear Dy III Complexes. Inorg. Chem. 2013, 52, 13770–13778. [Google Scholar] [CrossRef] [PubMed]
  53. Petrosyants, S.P.; Babeshkin, K.A.; Ilyukhin, A.B.; Belova, E.V.; Efimov, N.N. Complexes of Lanthanide (Dy, Er, Yb) Thiocyanates with Tetramethylphenanthroline. Synthesis, Thermolysis, and SMM Properties. Russ. J. Coord. Chem. 2021, 47, 244–252. [Google Scholar] [CrossRef]
  54. Petrosyants, S.P.; Babeshkin, K.A.; Ilyukhin, A.B.; Efimov, N.N. Molecular Magnets Based on Mononuclear Aqua and Aqua-Chloro Lanthanide (Tb, Dy, Er, Yb) Complexes with Bipyridine. Russ. J. Coord. Chem. 2021, 47, 165–173. [Google Scholar] [CrossRef]
  55. Galván, I.F.; Vacher, M.; Alavi, A.; Angeli, C.; Aquilante, F.; Autschbach, J.; Bao, J.J.; Bokarev, S.I.; Bogdanov, N.A.; Carlson, R.K.; et al. OpenMolcas: From Source Code to Insight. J. Chem. Theory Comput. 2019, 15, 5925–5964. [Google Scholar] [CrossRef]
  56. Aquilante, F.; Autschbach, J.; Baiardi, A.; Battaglia, S.; Borin, V.A.; Chibotaru, L.F.; Conti, I.; De Vico, L.; Delcey, M.; Galván, I.F.; et al. Modern quantum chemistry with [Open] Molcas. J. Chem. Phys. 2020, 152, 214117. [Google Scholar] [CrossRef]
  57. Reiher, M. Relativistic Douglas-Kroll-Hess theory. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2012, 2, 139–149. [Google Scholar] [CrossRef]
  58. Wolf, A.; Reiher, M.; Hess, B.A. The generalized Douglas–Kroll transformation. J. Chem. Phys. 2002, 117, 9215–9226. [Google Scholar] [CrossRef] [Green Version]
  59. Chibotaru, L.F.; Ungur, L.; Soncini, A. The Origin of Nonmagnetic Kramers Doublets in the Ground State of Dysprosium Triangles: Evidence for a Toroidal Magnetic Moment. Angew. Chem. Int. Ed. 2008, 47, 4126–4129. [Google Scholar] [CrossRef] [Green Version]
  60. Chibotaru, L.F.; Ungur, L. Ab initio calculation of anisotropic magnetic properties of complexes. I. Unique definition of pseudospin Hamiltonians and their derivation. J. Chem. Phys. 2012, 137, 064112. [Google Scholar] [CrossRef]
Magnetochemistry 09 00031 g001 550
Figure 1. Structures of [Y(H2O)6Cl2]+ complex in compound 1Yb (a) and of [Yb(terpy)(H2O)4Cl]2+ complex in 2Yb compound (b). Hydrogen atoms of water molecules in the structure of [Yb(terpy)(H2O)4Cl]2+ are not shown for clarity.
Figure 1. Structures of [Y(H2O)6Cl2]+ complex in compound 1Yb (a) and of [Yb(terpy)(H2O)4Cl]2+ complex in 2Yb compound (b). Hydrogen atoms of water molecules in the structure of [Yb(terpy)(H2O)4Cl]2+ are not shown for clarity.
Magnetochemistry 09 00031 g001
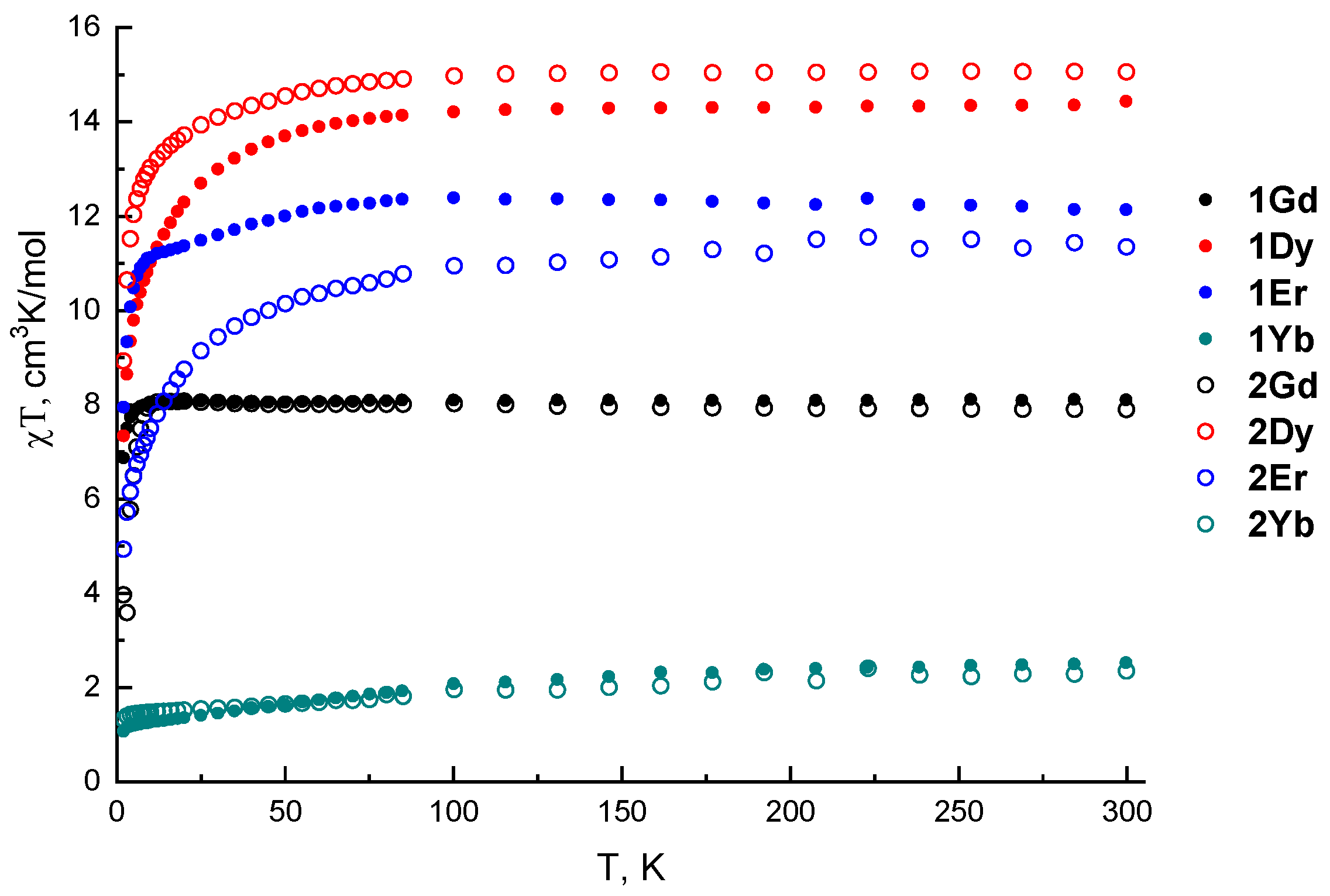
Magnetochemistry 09 00031 g002 550
Figure 2. χT vs. T dependences for the studied complexes in 5000 Oe DC field.
Figure 2. χT vs. T dependences for the studied complexes in 5000 Oe DC field.
Magnetochemistry 09 00031 g002
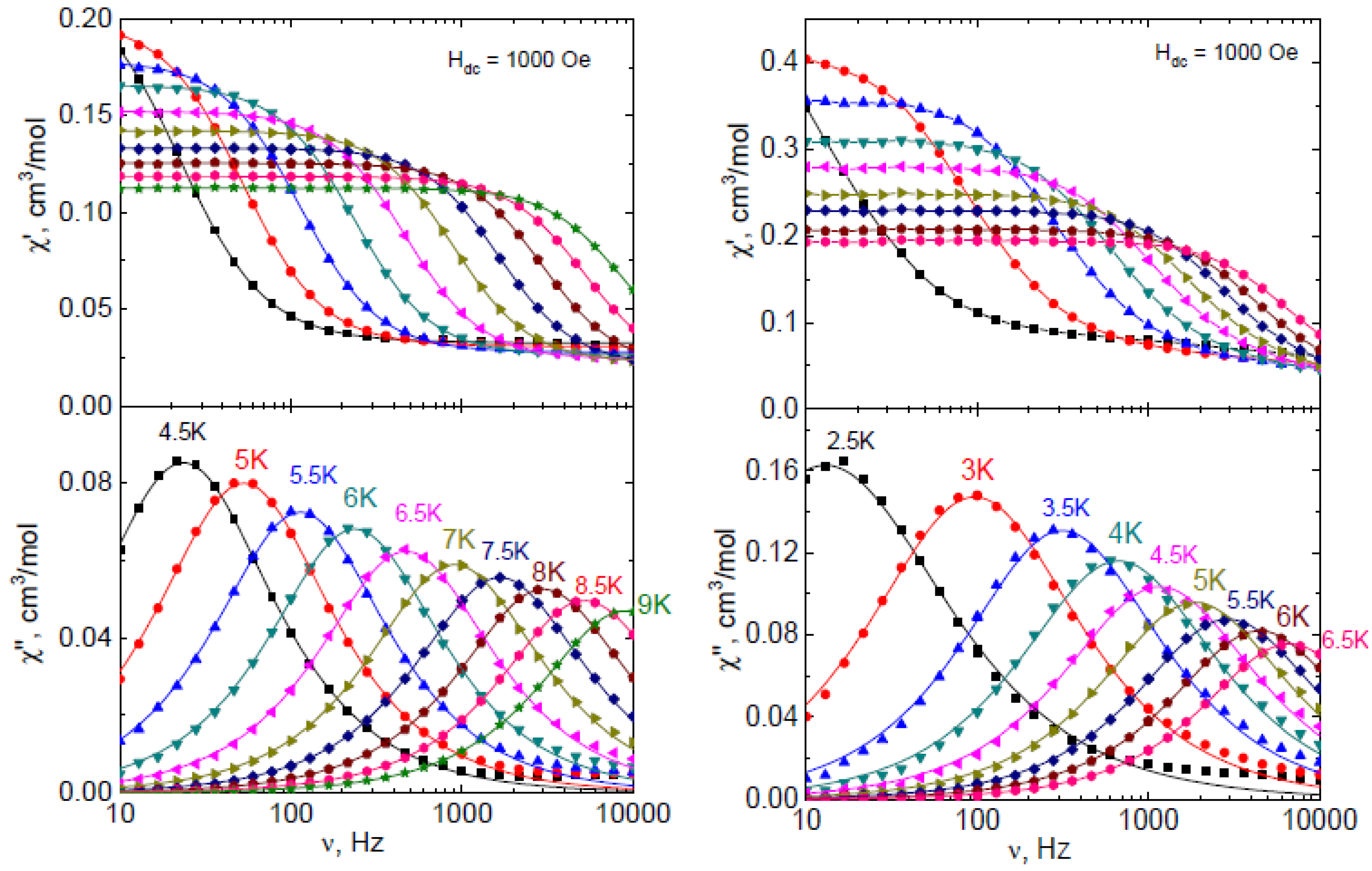
Magnetochemistry 09 00031 g003 550
Figure 3. Frequency dependences of the in-phase (χ′) and out-of-phase (χ″) components of the AC magnetic susceptibility of complexes 1Yb (left) and 2Yb (right) in a DC magnetic field of optimal intensity.
Figure 3. Frequency dependences of the in-phase (χ′) and out-of-phase (χ″) components of the AC magnetic susceptibility of complexes 1Yb (left) and 2Yb (right) in a DC magnetic field of optimal intensity.
Magnetochemistry 09 00031 g003
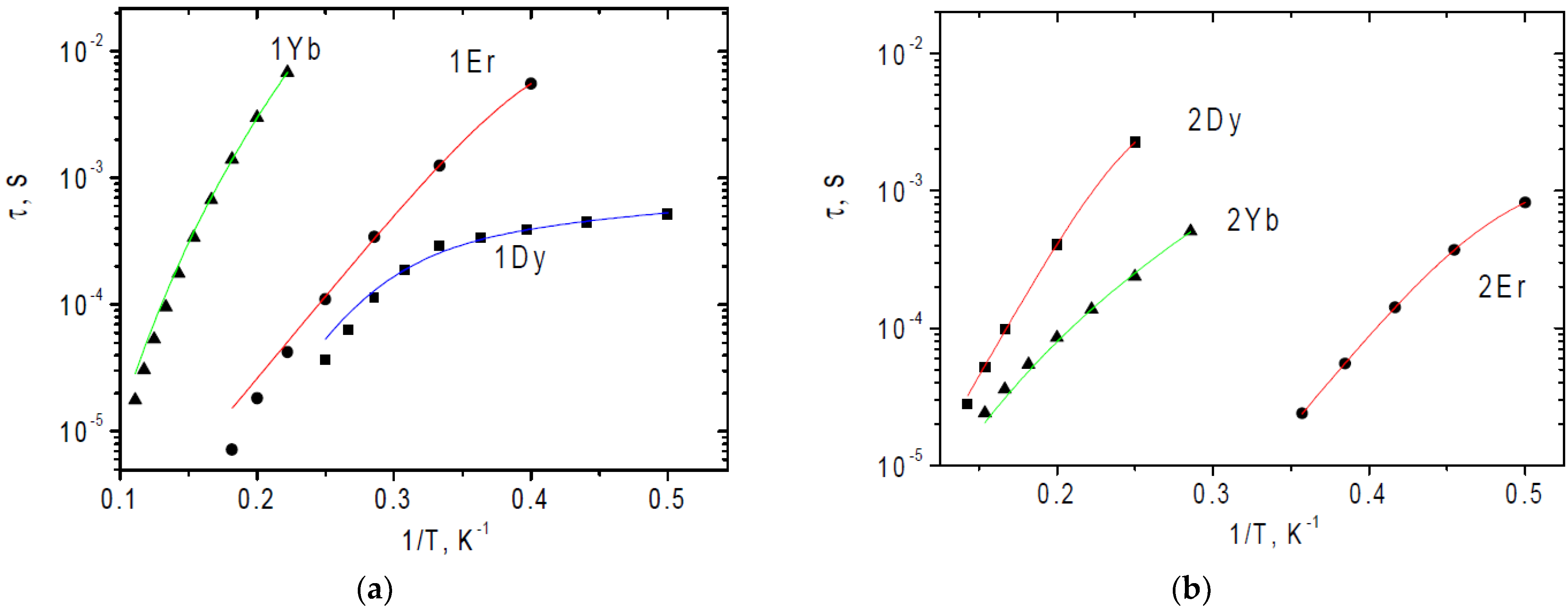
Magnetochemistry 09 00031 g004 550
Figure 4. Plots of the τ(1/T) dependences for 1Dy, 1Er, 1Yb (a) and 2Dy, 2Er, 2Yb (b) in a DC field of optimal intensity. The green lines represent the best approximation by the Raman mechanism (for 1Yb and 2Yb). The red lines represent the best approximation by the sum of the Orbach and direct mechanisms (for 1Er, 2Dy and 2Er). The blue line represents the best approximation by the sum of the Raman and direct mechanisms (for 1Dy).
Figure 4. Plots of the τ(1/T) dependences for 1Dy, 1Er, 1Yb (a) and 2Dy, 2Er, 2Yb (b) in a DC field of optimal intensity. The green lines represent the best approximation by the Raman mechanism (for 1Yb and 2Yb). The red lines represent the best approximation by the sum of the Orbach and direct mechanisms (for 1Er, 2Dy and 2Er). The blue line represents the best approximation by the sum of the Raman and direct mechanisms (for 1Dy).
Magnetochemistry 09 00031 g004
Table
Table 1. χT values (cm3·mol−1·K) for the complexes at 300 and at 2 K.
Table 1. χT values (cm3·mol−1·K) for the complexes at 300 and at 2 K.
ComplexχT (300 K)χT Theor.χT (2 K)
1Gd8.107.886.87
1Dy14.4414.177.34
1Er12.1411.487.95
1Yb2.532.571.07
2Gd7.907.883.96
2Dy15.0614.178.93
2Er11.3511.484.94
2Yb2.352.571.33
Table
Table 2. Parameters of the most probable magnetic relaxation processes for the studied complexes.
Table 2. Parameters of the most probable magnetic relaxation processes for the studied complexes.
Complex1Dy1Er1Yb2Dy2Er2Yb
Field, Oe100010001000150010001000
T range, K2–42.5–5.54.5–94–72–43.5–6.5
RamanC, K−n_Raman·s−10.057 *-0.001--3.0
nRaman9 **-7.9--5.2
DirectAdirect, K−1 Oe−n_direct s−19.3 × 10−10 *3.4 × 10−11 *-1.1 × 10−11 *3.9 × 10−10 *-
ndirect4 **4 **-4 **4 **-
OrbachUeff/kB, K (Ueff, cm−1)-30 * (21)-46 * (32)32 * (22)-
τ0, s-6.5 × 10−8 *-4.3 × 10−8 *2.8 × 10−10 *-
*—the parameter values are obtained for approximation by the sum of mechanisms. **—the parameters were fixed at indicated values during fitting in order to prevent overparametrization.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Petrosyants, S.P.; Babeshkin, K.A.; Ilyukhin, A.B.; Koroteev, P.S.; Efimov, N.N. Something You Need Might Be under Your Feet: Molecular Magnetism of Heavy Kramers Lanthanide Hydrated Chlorides and Their Complexes with Polydentate Terpy Ligand. Magnetochemistry 2023, 9, 31. https://doi.org/10.3390/magnetochemistry9010031

AMA Style

Petrosyants SP, Babeshkin KA, Ilyukhin AB, Koroteev PS, Efimov NN. Something You Need Might Be under Your Feet: Molecular Magnetism of Heavy Kramers Lanthanide Hydrated Chlorides and Their Complexes with Polydentate Terpy Ligand. Magnetochemistry. 2023; 9(1):31. https://doi.org/10.3390/magnetochemistry9010031

Chicago/Turabian Style

Petrosyants, Svetlana P., Konstantin A. Babeshkin, Andrey B. Ilyukhin, Pavel S. Koroteev, and Nikolay N. Efimov. 2023. "Something You Need Might Be under Your Feet: Molecular Magnetism of Heavy Kramers Lanthanide Hydrated Chlorides and Their Complexes with Polydentate Terpy Ligand" Magnetochemistry 9, no. 1: 31. https://doi.org/10.3390/magnetochemistry9010031

APA Style

Petrosyants, S. P., Babeshkin, K. A., Ilyukhin, A. B., Koroteev, P. S., & Efimov, N. N. (2023). Something You Need Might Be under Your Feet: Molecular Magnetism of Heavy Kramers Lanthanide Hydrated Chlorides and Their Complexes with Polydentate Terpy Ligand. Magnetochemistry, 9(1), 31. https://doi.org/10.3390/magnetochemistry9010031

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop