State-of-Charge Monitoring and Battery Diagnosis of Different Lithium Ion Chemistries Using Impedance Spectroscopy
Abstract
1. Introduction
1.1. Battery State Indicators
1.2. State-of-Health Indicators
1.3. Pseudocapacitance and Pseudocharge
2. Experimental Setup
- A.
- VoltSolar: 18,650 type, 3.2 V, 1.4–1.5 Ah, charge max. 3.65 V, cut-off voltage 2.0 V.
- B.
- Sony: US18650FT cylindrical, 3.2 V, 1.05–1.1 Ah,
- C.
- LithiumWerks (formerly A123) 26,650 cell, ANR26650M1B: 3.3 V, 2.6 Ah.
2.1. Test Procedure
- Capacity determination by coulomb-counting: Each cell was first charged at 1 C rate (CC) to the upper cutoff voltage, then at constant voltage (CV) until the current dropped below 0.1 A. The discharge took place at 25 °C and 1 C rate under a constant current load until the cut-off voltage was reached. Q is the withdrawn electrical charge. Then the battery was recharged as above.
- Impedance measurements were taken 20 min after each constant-current discharge step, from SOC 100%, 98%, 96%, down to 30%. The VoltSolar cell was tested down to 50% SOC. The EIS measurement took 80 s for six values per frequency decade in the frequency range from 1 kHz to 0.1 Hz. The measuring arrangement is schematically shown in Figure 2.
2.2. Measurement Parameters
3. Results and Discussion
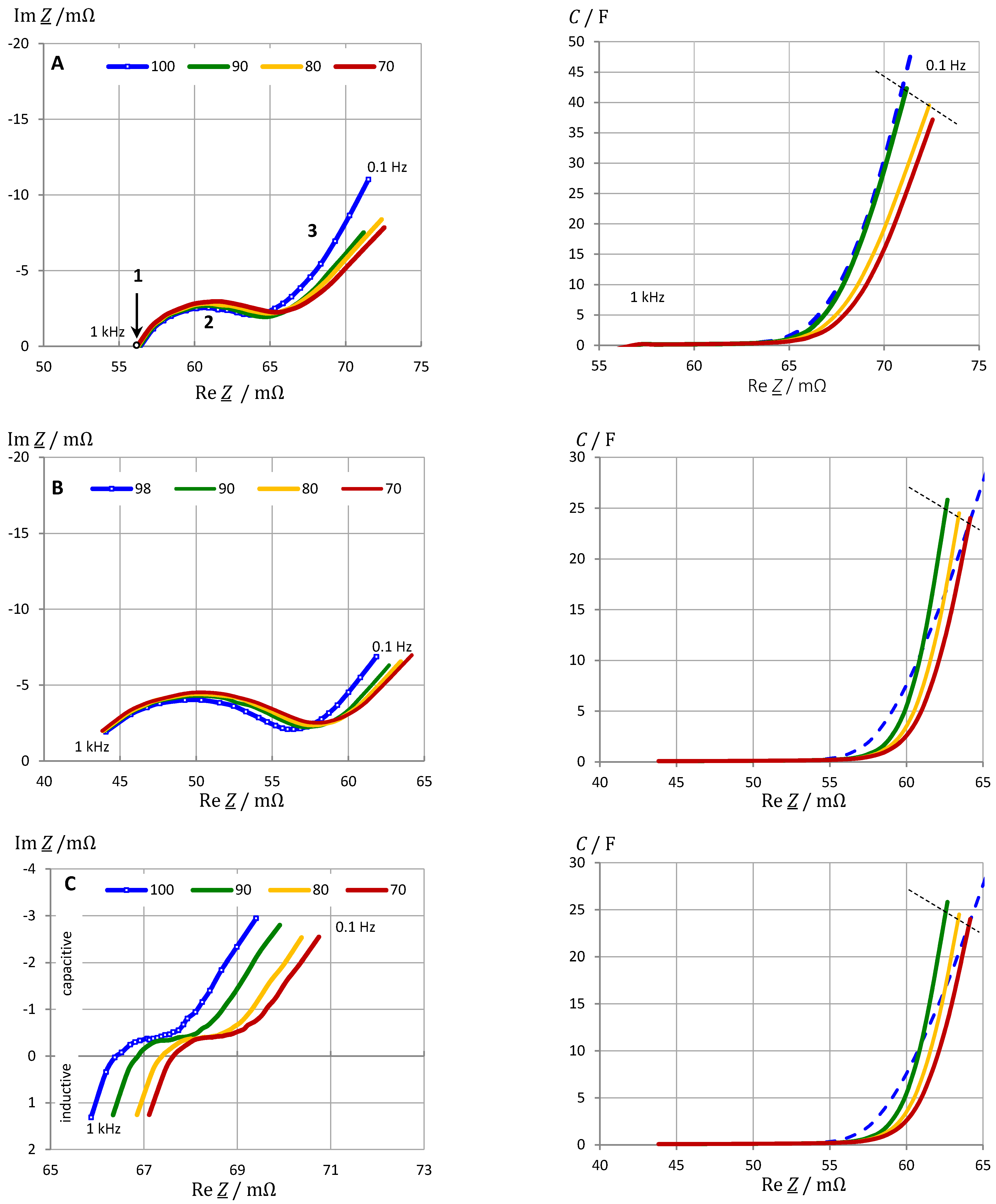
3.1. Frequency Response of Pseudocapacitance
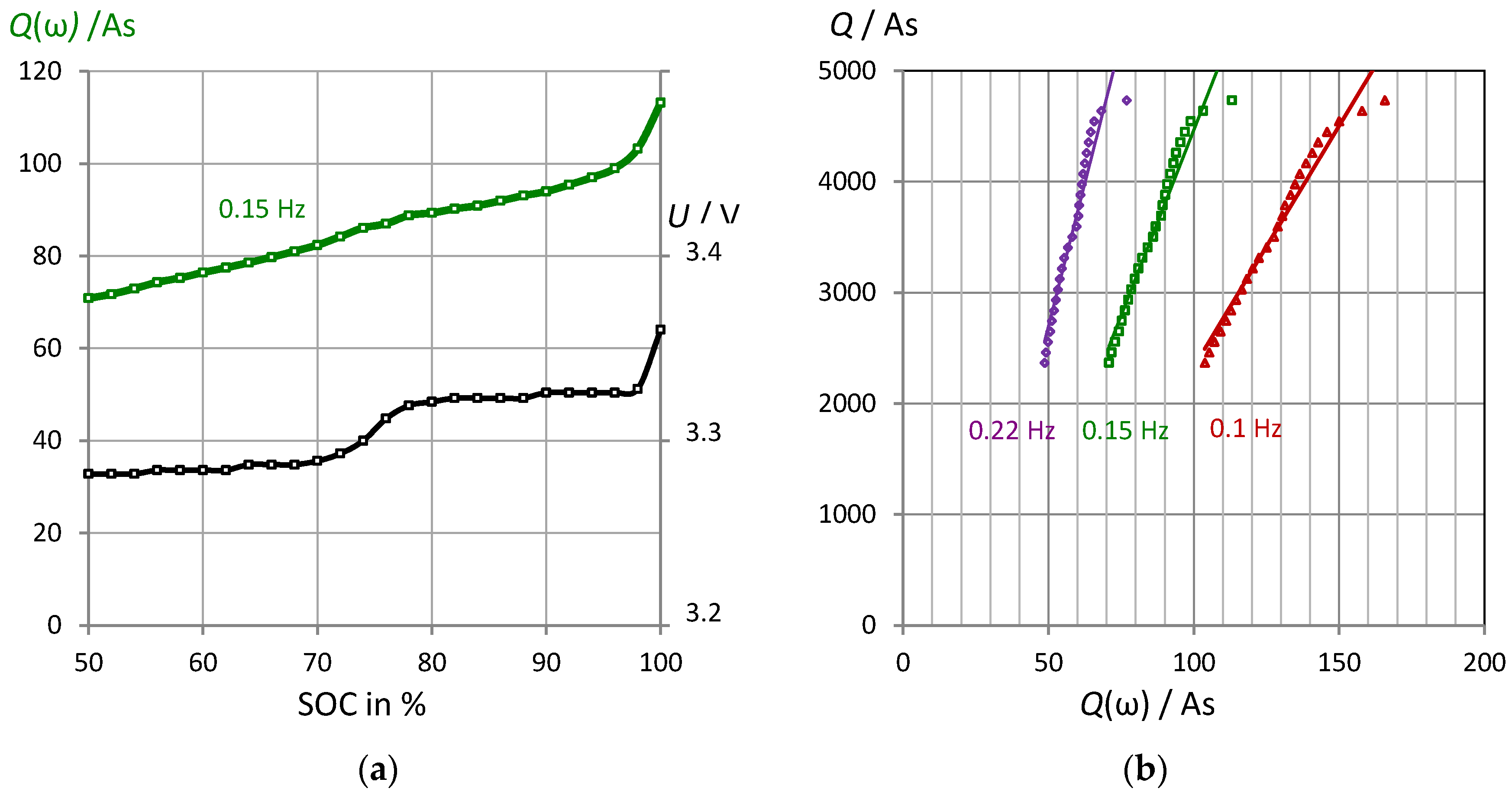
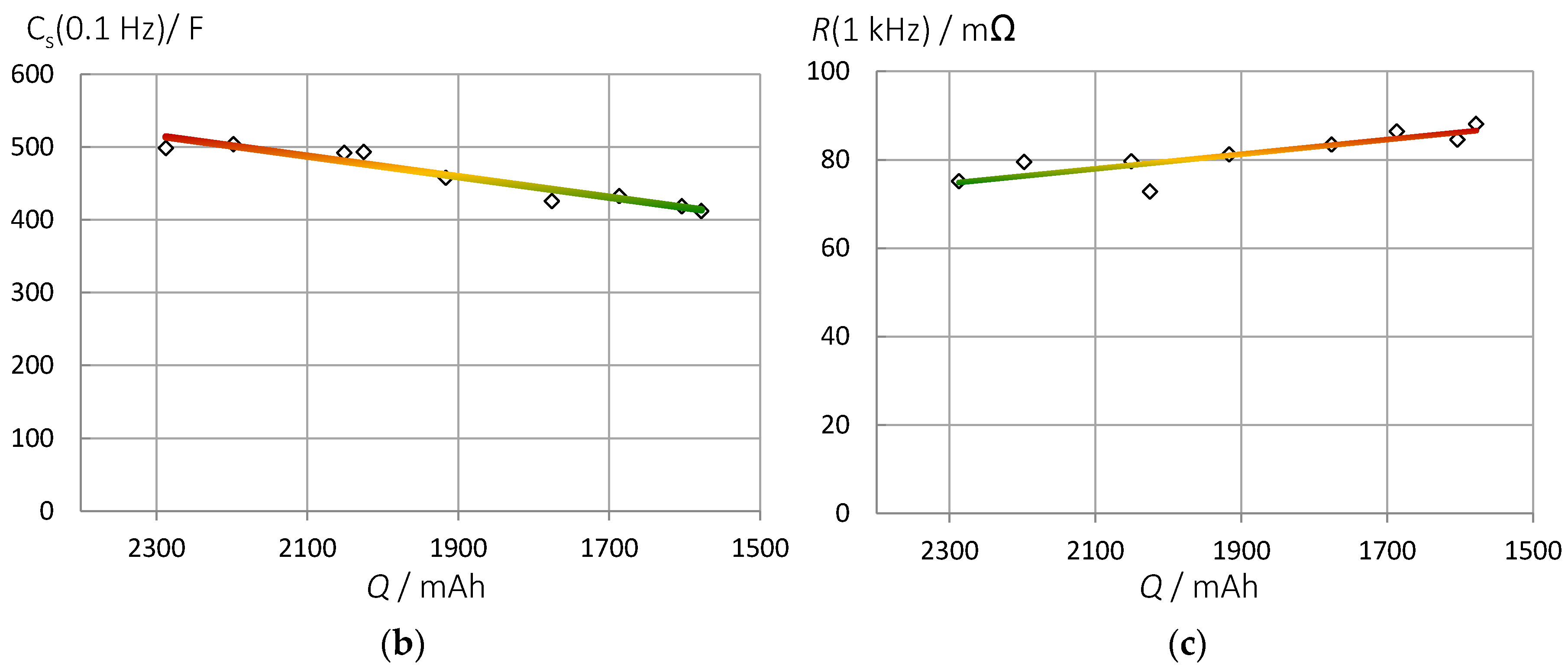
3.2. Correlation of Pseudocapacitance and Battery Capacity
3.3. Verification and Validation of the Pseudocapacitance Model
3.4. Properties of Pseudocharge
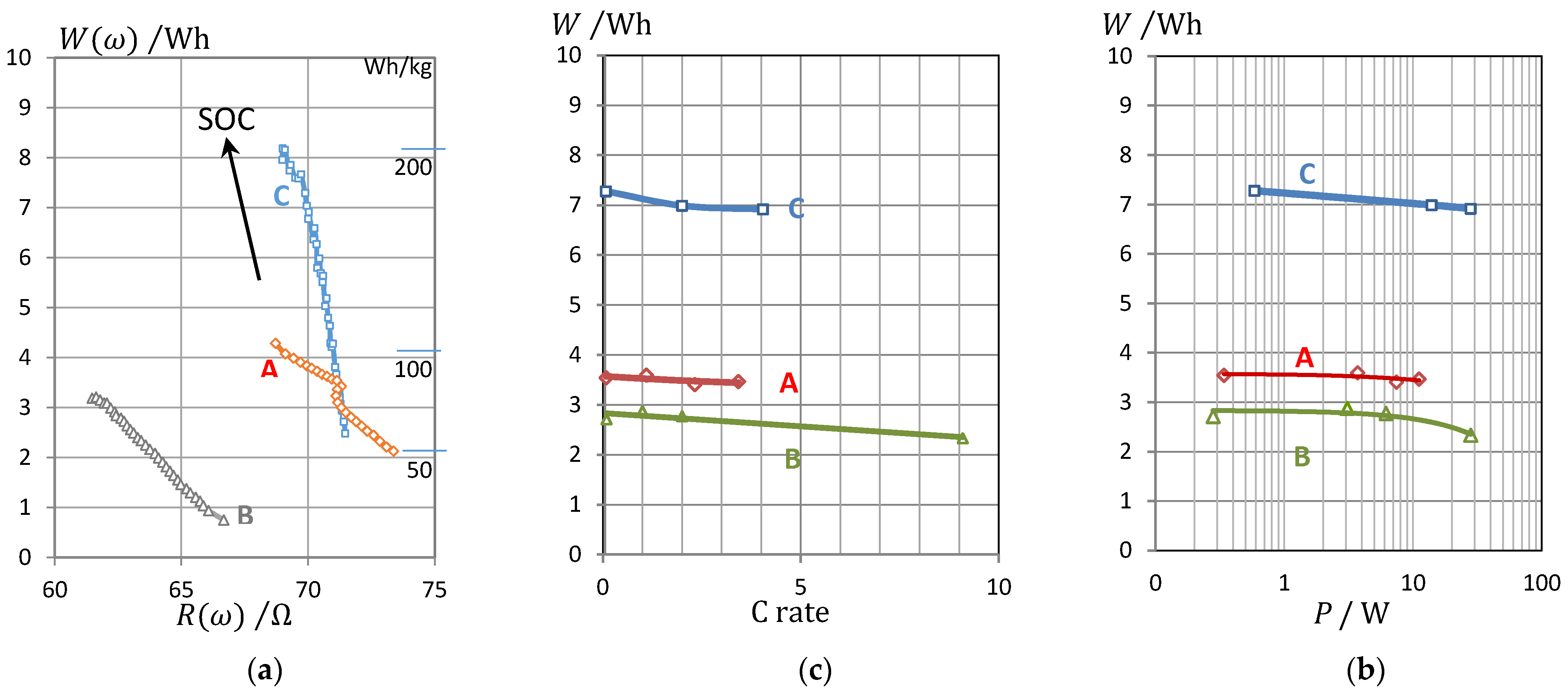
3.5. Conversion of Impedance Data to Energy and Power
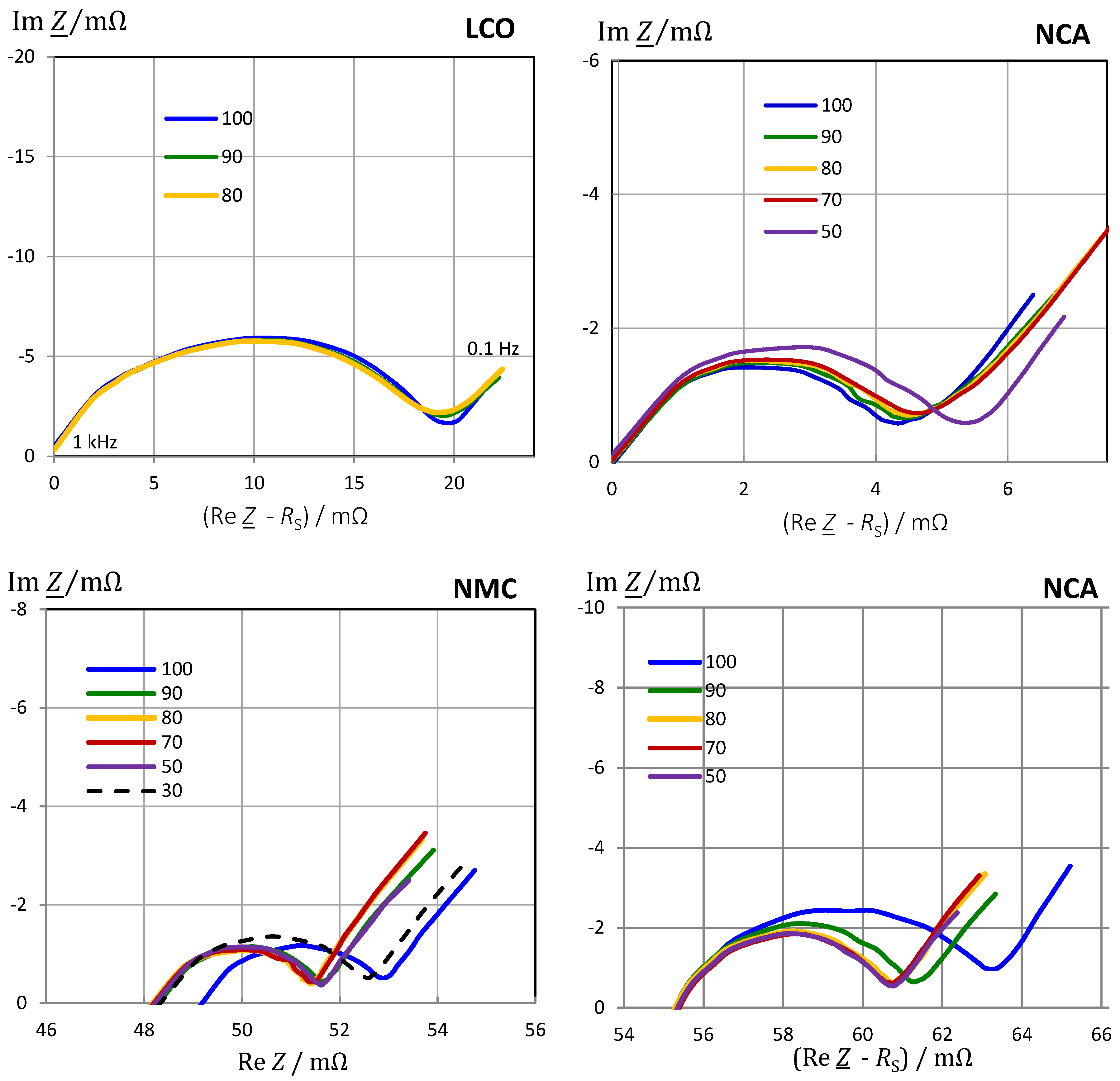
3.6. Impact of Cell Chemistry
- Electrolyte and solid-electrolyte interface (SEI) at high frequencies;
- Charge-transfer at medium frequencies;
- Pore diffusion and intercalation at frequencies below 0.01 Hz.
3.7. Impact of Aging
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Conflicts of Interest
List of Symbols and Abbreviations
| C | pseudocapacitance (F) |
| f | frequency (Hz) |
| Q | electric charge, battery capacity (Ah) |
| Q0 | capacity of a fully charged battery (Ah) |
| R | ohmic resistance, real part of impedance (Ω) |
| RS | electrolyte resistance (Ω) |
| U | cell voltage (V) |
| Y | complex admittance: Y = Z–1 (Ω–1) |
| Z | complex impedance (Ω) |
| ω | angular frequency: ω = 2πf (s–1) |
| Ah | Ampere hour: 1 Ah = 3600 C |
| j | imaginary operator: |
| LCO | lithium cobalt oxide |
| LMO | lithium manganese spinel |
| LFP | lithium iron phosphate |
| N | subscript: nominal value |
| NCA | nickel cobalt aluminum |
| NMC | nickel manganese cobalt |
| SOC, α | state-of-charge |
| SOH, β | state-of-health |
References
- Kurzweil, P.; Shamonin, M. State-of-Charge Monitoring by Impedance Spectroscopy during Long-Term Self-Discharge of Supercapacitors and Lithium-Ion Batteries. Batteries 2018, 4, 35. [Google Scholar] [CrossRef]
- Kurzweil, P.; Scheuerpflug, W. State-of-Charge Monitoring and Battery Diagnosis of NiCd Cells Using Impedance Spectroscopy. Batteries 2020, 6, 4. [Google Scholar] [CrossRef]
- Barsoukov, E.; Macdonald, J.R. Impedance Spectroscopy: Theory, Experiment, and Applications; Wiley: Hoboken, NJ, USA, 2018. [Google Scholar]
- Rodrigues, S.; Munichandraiah, N.; Shukla, A.K. A review of state-of-charge indication of batteries by means of a.c. impedance measurements. J. Power Sources 2000, 87, 12–20. [Google Scholar] [CrossRef]
- Osaka, T.; Mukoyama, D.; Nara, H. Review—Development of Diagnostic Process for Commercially Available Batteries, Especially Lithium Ion Battery, by Electrochemical Impedance Spectroscopy. J. Electrochem. Soc. 2015, 162, A2529. [Google Scholar] [CrossRef]
- Huang, J.; Gao, Y.; Luo, J.; Wang, S.; Li, C.; Chen, S.; Zhang, J. Editors’ Choice—Review—Impedance Response of Porous Electrodes: Theoretical Framework, Physical Models and Applications. J. Electrochem. Soc. 2020, 167, 166503. [Google Scholar] [CrossRef]
- La Rue, A.; Weddle, P.J.; Ma, M.; Hendricks, C.; Kee, R.J.; Vincent, T.L. State-of-Charge Estimation of LiFePO4–Li4Ti5O12 Batteries using History-Dependent Complex-Impedance. J. Electrochem. Soc. 2019, 166, A404. [Google Scholar]
- Cai, Y.; Huang, D.; Ma, Z.; Wang, H.; Huang, Y.; Wu, X.; Li, Q. Construction of highly conductive network for improving electrochemical performance of lithium iron phosphate. Electrochim. Acta 2019, 305, 563–570. [Google Scholar] [CrossRef]
- Spingler, F.B.; Naumann, M.; Jossen, A. Capacity Recovery Effect in Commercial LiFePO4/Graphite Cells. J. Electrochem. Soc. 2020, 167, 040526. [Google Scholar] [CrossRef]
- Klein, M.P.; Park, J.W. Current Distribution Measurements in Parallel-Connected Lithium-Ion Cylindrical Cells under Non-Uniform Temperature Conditions. J. Electrochem. Soc. 2017, 164, A1893–A1906. [Google Scholar] [CrossRef]
- Wang, J.; Liu, P.; Hicks-Garner, J.; Sherman, E.; Soukiazian, S.; Verbrugge, M.; Tataria, H.; Musser, J.; Finamore, P. Cycle-life model for graphite-LiFePO4 cells. J. Power Sources 2011, 196, 3942–3948. [Google Scholar] [CrossRef]
- Wenzl, H. Capacity. In Encyclopedia of Electrochemical Power Sources; Dyer, J.G.C., Moseley, P., Ogumi, Z., Rand, D., Scrosati, B., Eds.; Elsevier: Amsterdam, The Netherlands, 2009; Volume 1, pp. 395–400. [Google Scholar]
- Waag, W.; Sauer, D.U. State-of-Charge/Health. In Encyclopedia of Electrochemical Power Sources; Garche, J., Dyer, C., Moseley, P., Ogumi, Z., Rand, D., Scrosati, B., Eds.; Elsevier: Amsterdam, The Netherlands, 2009; Volume 4, pp. 793–804. [Google Scholar]
- Piller, S.; Perrin, M.; Jossen, A. A Methods for state-of-charge determination and their applications. J. Power Sources 2001, 96, 113–120. [Google Scholar] [CrossRef]
- Bergveld, J.J.; Danilov, D.; Notten, P.H.L.; Pop, V.; Regtien, P.P.L. Adaptive State-of-charge determination. In Encyclopedia of Electrochemical Power Sources; Garche, J., Dyer, C., Moseley, P., Ogumi, Z., Rand, D., Scrosati, B., Eds.; Elsevier: Amsterdam, The Netherlands, 2009; Volume 1, pp. 450–477. [Google Scholar]
- Hung, M.H.; Lin, C.H.; Lee, L.C.; Wang, C.M. State-of-charge and state-of-health estimation for lithium-ion batteries based on dynamic impedance technique. J. Power Sources 2014, 268, 861–873. [Google Scholar] [CrossRef]
- Dowgiallo, E.J. Method for Determining Battery State of Charge by Measuring A.C. Electrical Phase Angle Change. U.S. Patent 3984762A, 1975. [Google Scholar]
- Finger, E.P.; Sands, E.A. Method and Apparatus for Measuring the State of Charge of a Battery by Monitoring Reductions in Voltage. U.S. Patent 4193026A, 1978. [Google Scholar]
- Kikuoka, T.; Yamamoto, H.; Sasaki, N.; Wakui, K.; Murakami, K.; Ohnishi, K.; Kawamura, G.; Noguchi, H.; Ukigaya, F. System for Measuring State of Charge of Storage Battery. U.S. Patent 4377787A, 1979. [Google Scholar]
- Seyfang, G.R. Battery State of Charge indicator. U.S. Patent 4,949,046, 1985. [Google Scholar]
- Peled, E.; Yamin, H.; Reshef, I.; Kelrich, D.; Rozen, S. Method and Apparatus for Determining the State-of-Charge of Batteries Particularly Lithium Batteries. U.S. Patent 4,725,784 A, 1988. [Google Scholar]
- Hahn, M.; Schindler, S.; Triebs, L.C.; Danzer, M.A. Optimized Process Parameters for a Reproducible Distribution of Relaxation Times Analysis of Electrochemical Systems. Batteries 2019, 5, 43. [Google Scholar] [CrossRef]
- Wang, X.; Wei, X.; Zhu, J.; Dai, H.; Zheng, Y.; Xu, X.; Chen, Q. A review of modeling, acquisition, and application of lithium-ion battery impedance for onboard battery management. eTransportation 2021, 7, 100093. [Google Scholar] [CrossRef]
- Eddahech, A.; Briat, O.; Woirgard, E.; Vinassa, J.M. Remaining useful life prediction of lithium batteries in calendar ageing for automotive applications. Microelectron. Reliab. 2012, 52, 2438–2442. [Google Scholar] [CrossRef]
- Galeotti, M.; Cinà, L.; Giammanco, C.; Cordiner, S.; Di Carlo, A. Performance analysis and SOH (state of health) evaluation of lithium polymer batteries through electrochemical impedance spectroscopy. Energy 2015, 89, 678–686. [Google Scholar] [CrossRef]
- Howey, D.A.; Mitcheson, P.D.; Yufit, V.; Offer, G.J.; Brandon, N.P. Online Measurement of Battery Impedance Using Motor Controller Excitation. IEEE Trans. Veh. Technol. 2014, 63, 2557–2566. [Google Scholar] [CrossRef]
- Spielbauer, M.; Berg, P.; Ringat, M.; Bohlen, O.; Jossen, A. Experimental study of the impedance behavior of 18650 lithium-ion battery cells under deforming mechanical abuse. J. Energy Storage 2019, 26, 101039. [Google Scholar] [CrossRef]
- Srinivasan, R.; Demirev, P.A.; Carkhuff, B.G. Rapid monitoring of impedance phase shifts in lithium-ion batteries for hazard prevention. J. Power Sources 2018, 405, 30–36. [Google Scholar] [CrossRef]
- Kurzweil, P.; Fischle, H.J. A new monitoring method for electrochemical aggregates by impedance spectroscopy. J. Power Sources 2004, 127, 331–340. [Google Scholar] [CrossRef]
- Kurzweil, P.; Ober, J.; Wabner, D.W. Method for Correction and Analysis of Impedance Spectra. Electrochim. Acta 1989, 34, 1179–1185. [Google Scholar] [CrossRef]
- Huang, J.; Li, Z.; Liaw, B.Y.; Zhang, J. Graphical analysis of electrochemical impedance spectroscopy data in Bode and Nyquist representations. J. Power Sources 2016, 309, 82–98. [Google Scholar] [CrossRef]






| Chemistry | Cell | Voltage U (V) | Capacity Q (Ah) | C Rate Charge and Discharge | V per 100 % | Correlation of Pseudocapacitance (in F) and Capacity (in Ah) | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | LTO | BE Power 18,650 (Li4Ti5O12) | 2.8 … 1.5 | 1.3 | 6 | 1.5 | – | – | – | – | – |
| 2 | LFP | SONY US18650 FTC | 3.6 … 2 | 1.1 | 1.1 | 30 | 0.21 | – | – | – | – |
| 3 | LithiumWerks ANR26650M1B | 3.6 … 2 | 2.6 | 4 | 70 | 0.18 | – | – | – | – | |
| 4 | VoltSolar 18,650 IFR | 3.6 … 2 | 1.4 | – | 3 | – | – | – | – | – | |
| 5 | LMO | Samsung INR18650-20R (LiNiCoMnO2) | 4.2 … 2.5 | 2 | 4 | 20 | 1.15 | 2.73 – | −12.5 – | 19.3 302 | −9.34 6.2 |
| 6 | NMC | Samsung ICR18650-22P (MnNi) | 4.2 … 2.5 | 2.15 | 2.15 | 10 | – | – | – | – | – |
| 7 | Samsung INR18650-25R (NiMn) | 4.2 … 2.5 | 2.5 | 4 | 20 | – | – | – | – | – | |
| 8 | LG ICR18650HE2 (Ni Mn Co) | 4.2 … 2.0 | 2.5 | 4 | 20 | 1.0 | 1018 – | −5798 – | 11,032 182 | −6540 132 | |
| 9 | LG 18650-HG2 (Co Ni Mn) | 4.2 … 2.5 | 3 | 4 | 20 | – | – | – | – | – | |
| 10 | LCO | Panasonic UR18650 FK | 4.2 … 2.5 | 2.5 | 1.75 | 5 | 0.81 | 516 – | −2644 – | 4599 231 | −2349 −59 |
| 11 | NCA | SONY US18650VTC5 | 4.2 … 2.5 | 2.6 | 2.5 | 20 | 1.0 | – | – | – | – |
| 12 | LG INR18650MH1 | 4.2 … 2.5 | 3.2 | 3.1 | 10 | – | – | – | – | – | |
| 13 | Panasonic NCR18650GA | 4.2 … 2.5 | 3.3 | 10 | 10 | 0.93 | – | – | – | – | |
| 14 | Samsung INR18650-35E LiNiCoAlO2 | 4.2 … 2.65 | 3.35 | 2 | 8 | – | – | – | – | – | |
| 15 | Panasonic NCR18650 B | 4.2 … 2.5 | 3.4 | 1.62 | 3.4 | – | – | – | – | – | |
| Battery | Battery 1 | Battery 2 | Battery 3 | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Measurement | 1 | 2 | 3 | 1 | 2 | 3 | 1 | 2 | 3 |
| Repeatability of | 1.42 | 1.37 | 1.36 | 1.48 | 1.15 | 1.36 | 1.56 | 1.35 | 1.34 |
| slope ΔSOC/ΔC | 1.38 ± 0.02 | 1.33 ± 0.13 | 1.41 ± 0.09 | ||||||
| Linear model | SOC = 1.412⋅C − 220.4 or C = 0.6995⋅SOC − 152.8 for SOC in % and C in F. | ||||||||
| Quadratic model | SOC = −0.01120⋅C2 + 5.877⋅C − 657.6 or C = 0.003748⋅SOC2 + 0.2198⋅SOC + 166.6 | ||||||||
| Cell # | Full Charge SOC > 0.9 | Medium State-of-Charge SOC ≈ 0.5 | Low State-of-Charge SOC < 0.5 | ||
|---|---|---|---|---|---|
| 5 | LMO | No significant impact on resistance. C ∼ SOC | R < 40 mΩ drops slightly. Reactance increases: X ∼ SOC. | R ≈ 40 mΩ drops slightly. X(0.1 Hz) = constant. | |
| 10 | LCO | No significant impact on resistance | R and X are slightly higher when SOC is lower. | R and X are high when SOC is low. Steeper U(Q) curve. | |
| 1113 | NCA | Resistance and impedance increase: R ∼ SOC | R and X are slightly higher when SOC is lower. | R and X are high when SOC is low. Steeper U(Q) curve. | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kurzweil, P.; Scheuerpflug, W. State-of-Charge Monitoring and Battery Diagnosis of Different Lithium Ion Chemistries Using Impedance Spectroscopy. Batteries 2021, 7, 17. https://doi.org/10.3390/batteries7010017
Kurzweil P, Scheuerpflug W. State-of-Charge Monitoring and Battery Diagnosis of Different Lithium Ion Chemistries Using Impedance Spectroscopy. Batteries. 2021; 7(1):17. https://doi.org/10.3390/batteries7010017
Chicago/Turabian StyleKurzweil, Peter, and Wolfgang Scheuerpflug. 2021. "State-of-Charge Monitoring and Battery Diagnosis of Different Lithium Ion Chemistries Using Impedance Spectroscopy" Batteries 7, no. 1: 17. https://doi.org/10.3390/batteries7010017
APA StyleKurzweil, P., & Scheuerpflug, W. (2021). State-of-Charge Monitoring and Battery Diagnosis of Different Lithium Ion Chemistries Using Impedance Spectroscopy. Batteries, 7(1), 17. https://doi.org/10.3390/batteries7010017





