AI-Optimized Lattice Structures for Biomechanics Scaffold Design
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
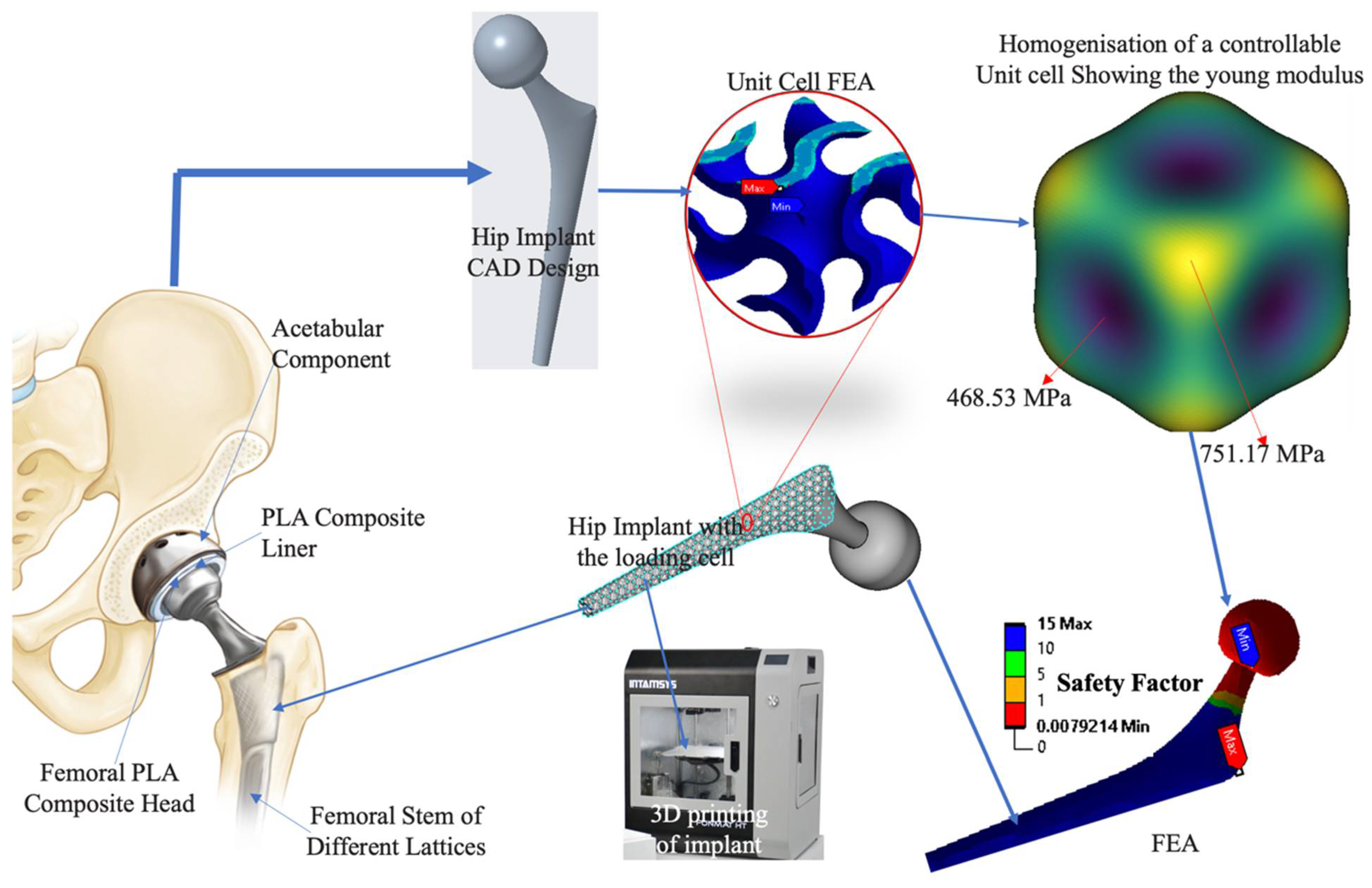
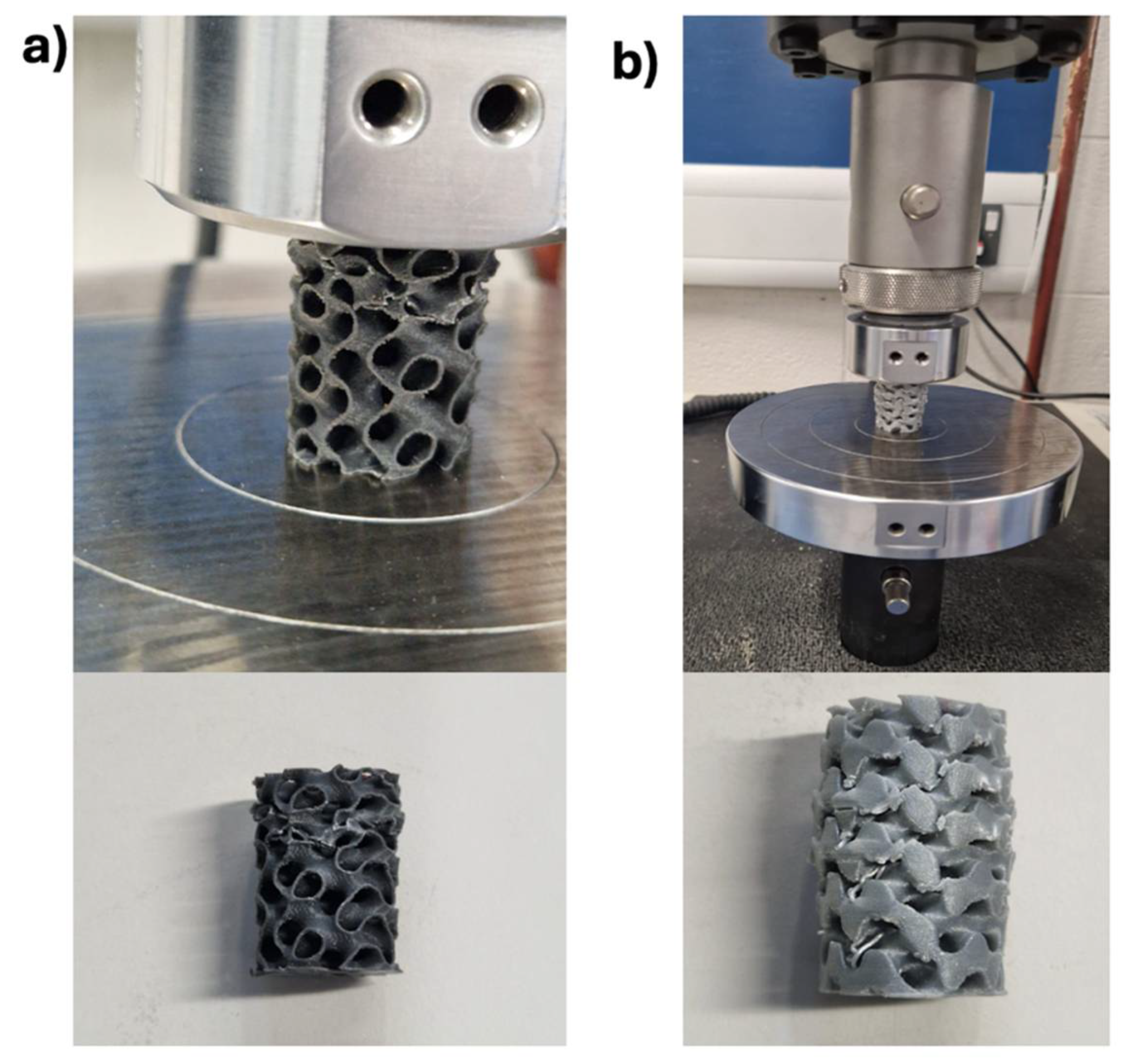
2.2. Lattice Design and Fabrication
2.3. Homogeneity and FEA Analysis of Lattices
2.4. Mechanical Characterization of Composites for Orthopedic
3. Results
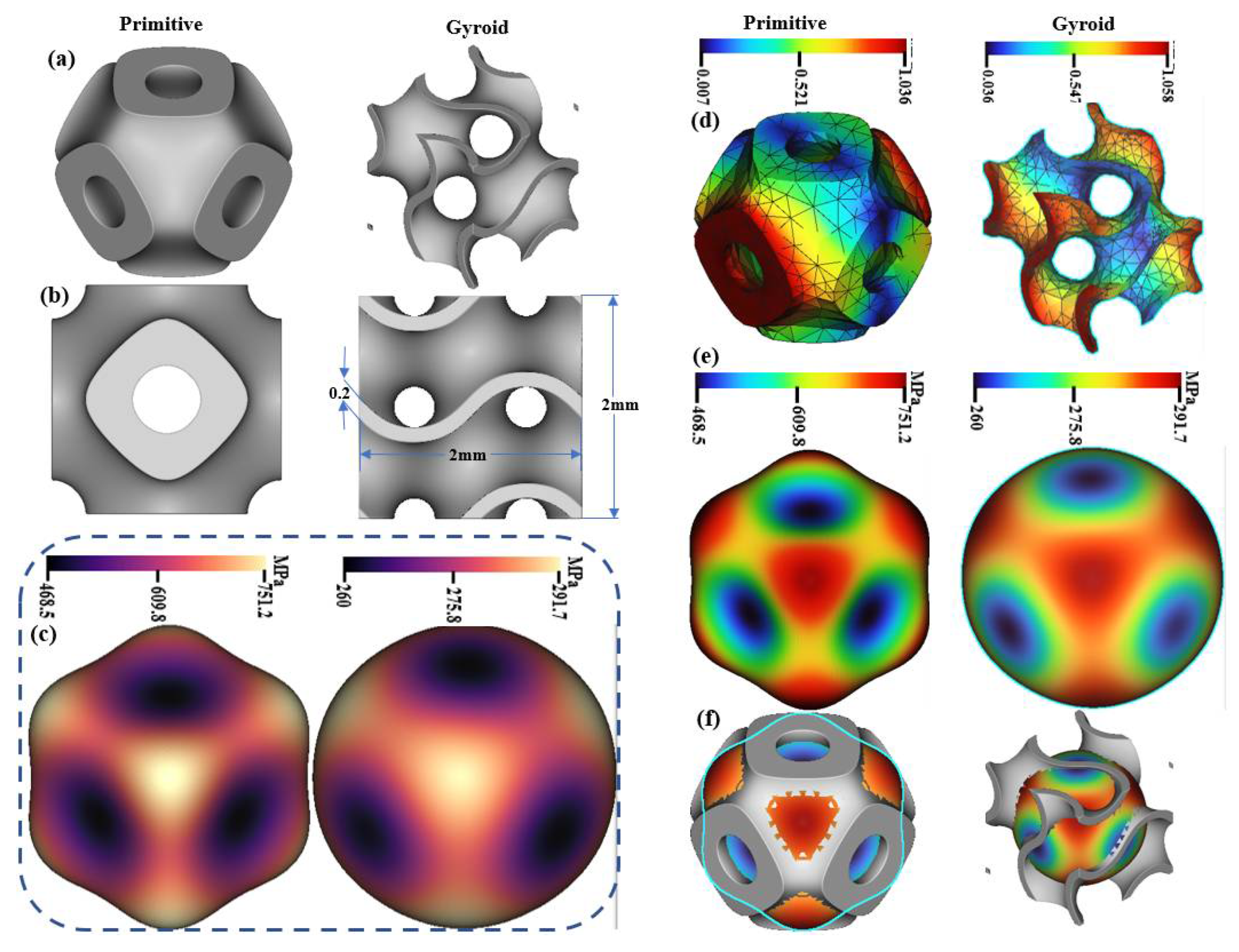
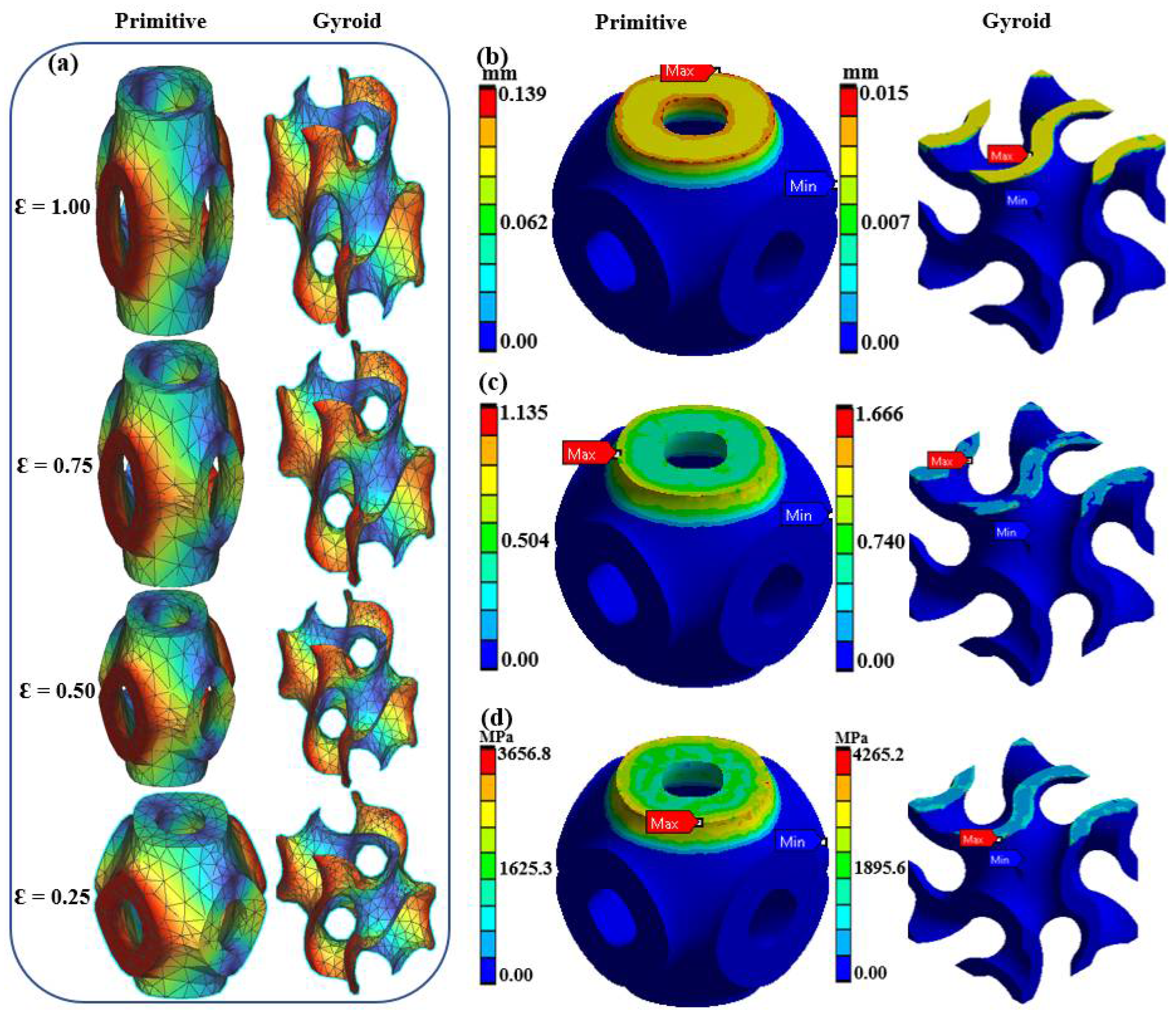
3.1. Homogeneity of Lattice Structures
3.2. Comparison of Schwartz Primitive and Gyroid Lattices
3.3. FEA of Scaffold Models
3.4. Mechanical Properties
3.5. Thermal and Chemical Characterization
3.6. Thermal Properties and Crystallization Behavior of the Composites
3.7. Analysis of FTIR Spectra and Surface Morphology
4. Discussion
4.1. Thermal and Chemical Analysis
4.2. Validation of the Research
4.3. Discussion on Validation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Oladapo, B.I.; Zahedi, S.A.; Ismail, S.O.; Olawade, D.B. Recent advances in biopolymeric composite materials: Future sustainability of bone-implant. Renew. Sustain. Energy Rev. 2021, 150, 111505. [Google Scholar] [CrossRef]
- Xu, Y.; Zhang, F.; Zhai, W.; Cheng, S.; Li, J.; Wang, Y. Unraveling of Advances in 3D-Printed Polymer-Based Bone Scaffolds. Polymers 2022, 14, 566. [Google Scholar] [CrossRef] [PubMed]
- Balabanov, S.V.; Makogon, A.I.; Sychev, M.M.; Evstratov, A.A.; Regazzi, A.; Lopez-Cuesta, J.M. 3D printing and mechanical properties of polyamide products with Schwartz primitive topology. Tech. Phys. 2020, 65, 211–215. [Google Scholar] [CrossRef]
- Long, T.; Song, X.; Han, B.; Suo, Y.; Jia, L. In Situ Magnetic Field Compensation Method for Optically Pumped Magnetometers Under Three-Axis Nonorthogonality. IEEE Trans. Instrum. Meas. 2024, 73, 9502112. [Google Scholar] [CrossRef]
- Overview of Materials for Polylactic Acid (PLA) Biopolymer. Available online: https://www.matweb.com/search/DataSheet.aspx?MatGUID=ab96a4c0655c4018a8785ac4031b9278 (accessed on 14 November 2024).
- Luan, S.; Yu, X.; Lei, S.; Ma, C.; Wang, X.; Xue, X.; Ding, Y.; Ma, T.; Zhu, B. Deep learning for fast super-resolution ultrasound microvessel imaging. Phys. Med. Biol. 2023, 68, 245023. [Google Scholar] [CrossRef]
- Yang, X. Hydroxyapatite: Design with Nature. In Orthopedic Biomaterials: Advances and Applications; Springer: Cham, Switzerland, 2017; pp. 141–165. [Google Scholar] [CrossRef]
- Wang, K.; Boonpratatong, A.; Chen, W.; Ren, L.; Wei, G.; Qian, Z.; Lu, X.; Zhao, D. The Fundamental Property of Human Leg During Walking: Linearity and Nonlinearity. IEEE Trans. Neural Syst. Rehabil. Eng. 2023, 31, 4871–4881. [Google Scholar] [CrossRef]
- Omigbodun, F.T.; Oladapo, B.I. Enhanced Mechanical Properties and Degradation Control of Poly(Lactic) Acid/Hydroxyapatite/Reduced Graphene Oxide Composites for Advanced Bone Tissue Engineering Application. Biomimetics 2024, 9, 651. [Google Scholar] [CrossRef]
- Zhao, Y.; Liu, Y.; Kang, S.; Sun, D.; Liu, Y.; Wang, X.; Lu, L. Peripheral nerve injury repair by electrical stimulation combined with graphene-based scaffolds. Front. Bioeng. Biotechnol. 2024, 12, 1345163. [Google Scholar] [CrossRef]
- Omigbodun, F. Manufacturing and Mechanical Characterisation of PLA/cHAP/rGO Composite for Bone Implants; Loughborough University: Loughborough, UK, 2024. [Google Scholar] [CrossRef]
- Javaid, M.; Haleem, A. 3D printed tissue and organ using additive manufacturing: An overview. Clin. Epidemiol. Glob. Health 2019, 8, 586–594. [Google Scholar] [CrossRef]
- Xu, J.; Chang, L.; Chen, T.; Ren, T.; Zhang, Y.; Cai, Z. Study of the bending properties of variable stiffness chain mail fabrics. Compos. Struct. 2023, 322, 117369. [Google Scholar] [CrossRef]
- Viet, N.V.; Zaki, W. Artificial neural network model of the mechanical behaviour of shape memory alloy Schwartz primitive lattice architectures. Mech. Mater. 2023, 183, 104680. [Google Scholar] [CrossRef]
- Wang, J.; Sarkar, D.; Mohan, A.; Lee, M.; Ma, Z.; Chortos, A. Deep Learningfor Strain Field Customization inBioreactor with Dielectric Elastomer Actuator Array. Cyborg Bionic Syst. 2024, 5, 0155. [Google Scholar] [CrossRef] [PubMed]
- Maconachie, T.; Tino, R.; Lozanovski, B.; Watson, M.; Jones, A.; Pandelidi, C.; Alghamdi, A.; Almalki, A.; Downing, D.; Brandt, M.; et al. The compressive behaviour of ABS gyroid lattice structures manufactured by fused deposition modelling. Int. J. Adv. Manuf. Technol. 2020, 107, 4449–4467. [Google Scholar] [CrossRef]
- He, X.; Jiang, Z.; Akakuru, O.U.; Li, J.; Wu, A. Nanoscale covalent organic frameworks: From controlled synthesis to cancer therapy. Chem. Commun. 2021, 57, 12417–12435. [Google Scholar] [CrossRef]
- Hayashi, K.; Kishida, R.; Tsuchiya, A.; Ishikawa, K. Superiority of triply periodic minimal surface gyroid structure to strut-based grid structure in both strength and bone regeneration. ACS Appl. Mater. Interfaces 2023, 15, 34570–34577. [Google Scholar] [CrossRef]
- Li, C.; Bian, Y.; Zhao, Z.; Liu, Y.; Guo, Y. Advances in Biointegrated Wearable and Implantable Optoelectronic Devices for Cardiac Healthcare. Cyborg Bionic Syst. 2024, 5, 0172. [Google Scholar] [CrossRef]
- Berman, J. Homogeneous lattices and lattice-ordered groups. Colloq. Math. 1974, 1, 13–24. Available online: https://www.infona.pl/resource/bwmeta1.element.zamlynska-4e5aeaae-e4c1-444f-ae36-a31e5a94712d/content/partDownload/4c833e38-7cb8-35ed-a389-a5bcf7506891 (accessed on 28 November 2024). [CrossRef]
- Uesugi, K.; Mayama, H.; Morishima, K. Analysis of Rowing Force of the Water Strider Middle Leg by Direct Measurement Using a Bio-Appropriating Probe and by Indirect Measurement Using Image Analysis. Cyborg Bionic Syst. 2023, 4, 0061. [Google Scholar] [CrossRef]
- Soro, N.; Attar, H.; Wu, X.; Dargusch, M.S. Investigation of the structure and mechanical properties of additively manufactured Ti-6Al-4V biomedical scaffolds designed with a Schwartz primitive unit-cell. Mater. Sci. Eng. A 2018, 745, 195–202. [Google Scholar] [CrossRef]
- Li, Z.; Xu, Q. Multi-Section Magnetic Soft Robot with Multirobot Navigation System for Vasculature Intervention. Cyborg Bionic Syst. 2024, 5, 0188. [Google Scholar] [CrossRef]
- Chouhan, G.; Murali, G.B. Designs, advancements, and applications of three-dimensional printed gyroid structures: A review. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2024, 238, 965–987. [Google Scholar] [CrossRef]
- Joyce, M.; Hodgkinson, T.; Lemoine, M.; González-Vázquez, A.; Kelly, D.J.; O’Brien, F.J. Development of a 3D-printed bioabsorbable composite scaffold with mechanical properties suitable for treating large, load-bearingarticular cartilage defects. Eur. Cells Mater. 2023, 45, 158–172. [Google Scholar] [CrossRef] [PubMed]
- Maskery, I.; Sturm, L.; Aremu, A.O.; Panesar, A.; Williams, C.B.; Tuck, C.J.; Wildman, R.D.; Ashcroft, I.; Hague, R.J.M. Insights into the mechanical properties of several triply periodic minimal surface lattice structures made by polymer additive manufacturing. Polymer 2018, 152, 62–71. [Google Scholar] [CrossRef]
- Jiang, Z.; Shi, H.; Tang, X.; Qin, J. Recent advances in droplet microfluidics for single-cell analysis. TrAC Trends Anal. Chem. 2023, 159, 116932. [Google Scholar] [CrossRef]
- Zhang, L.; Feih, S.; Daynes, S.; Chang, S.; Wang, M.Y.; Wei, J.; Lu, W.F. Energy absorption characteristics of metallic triply periodic minimal surface sheet structures under compressive loading. Addit. Manuf. 2018, 23, 505–515. [Google Scholar] [CrossRef]
- Maskery, I.; Aremu, A.O.; Parry, L.; Wildman, R.D.; Tuck, C.J.; Ashcroft, I.A. Effective design and simulation of surface-based lattice structures featuring volume fraction and cell type grading. Mater. Des. 2018, 155, 220–232. [Google Scholar] [CrossRef]
- Wang, H.; Wang, Y.; Yan, S.; Du, X.; Gao, Y.; Liu, H. Merge-and-Split Graph Convolutional Network for Skeleton-Based Interaction Recognition. Cyborg Bionic Syst. 2024, 5, 0102. [Google Scholar] [CrossRef]
- Lee, S.S.; Du, X.; Kim, I.; Ferguson, S.J. Scaffolds for bone tissue engineering. Matter 2022, 5, 2722–2759. [Google Scholar] [CrossRef]
- Cui, Q.; Ding, Z.; Chen, F. Hybrid Directed Hypergraph Learning and Forecasting of Skeleton-Based Human Poses. Cyborg Bionic Syst. 2024, 5, 0093. [Google Scholar] [CrossRef]
- da Silva Siqueira, A.; Braga, N.F.; Muñoz, P.A.R.; de Freitas, L.F.; Ferreira, A.H.; Fechine, G.J.M. Biodegradable scaffold: Integration of polylactic acid, hydroxyapatite, and graphene oxide via FDM 3D printing. Express Polym. Lett. 2024, 18, 656–672. [Google Scholar] [CrossRef]
- Xue, R.; Yuan, P.; Zhao, B.; Jing, F.; Kou, X.; Yue, W.; Wang, Y.; Wang, D.; Sewvandi, G.A.; Hu, D. DLP printing of BT/HA nanocomposite ceramic scaffolds using low refractive index BT crystals. J. Mater. 2024, 10, 1036–1048. [Google Scholar] [CrossRef]
- Gomez, J.; Villaro, E.; Perez, J.; Haidar, B.A. Preparation of electrically conductive PLA/rGO nanocomposite filaments. Graphene Technol. 2020, 5, 41–48. [Google Scholar] [CrossRef]
- Peng, X.; Han, Y.; Liu, G.; Li, J.; Yi, B.; Sa, G.; Jiang, S. Effect of manufacturing process parameters on the compression and energy absorption properties of 4D-printed deformable honeycomb structure. Smart Mater. Struct. 2024, 33, 075035. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, L.; Daynes, S.; Zhang, H.; Feih, S.; Wang, M.Y. Design of graded lattice structure with optimised mesostructures for additive manufacturing. Mater. Des. 2018, 142, 114–123. [Google Scholar] [CrossRef]
- Jiang, Z.; Han, X.; Zhao, C.; Wang, S.; Tang, X. Recent Advance in Biological Responsive Nanomaterials for Biosensing and Molecular Imaging Application. Int. J. Mol. Sci. 2022, 23, 1923. [Google Scholar] [CrossRef]
- Omigbodun, F.T.; Oladapo, B.I.; Osa-Uwagboe, N. Exploring the frontier of Polylactic Acid/Hydroxyapatite composites in bone regeneration and their revolutionary biomedical applications—A review. J. Reinf. Plast. Compos. 2024. [Google Scholar] [CrossRef]
- Wu, Y.; Chiu, G. An Improved Model of Height Profile for Drop-On-Demand Print of Ultraviolet Curable Ink. ASME Lett. Dyn. Syst. Control 2021, 1, 031010. [Google Scholar] [CrossRef]
- Zhang, B.; Wang, L.; Song, P.; Pei, X.; Sun, H.; Wu, L.; Zhou, C.; Wang, K.; Fan, Y.; Zhang, X. 3D printed bone tissue regenerative PLA/HA scaffolds with comprehensive performance optimisations. Mater. Des. 2021, 201, 109490. [Google Scholar] [CrossRef]
- Wu, Y.; Chiu, G. Modeling Height Profile for Drop-on-Demand Print of UV Curable Ink. In Proceedings of the ASME 2019 Dynamic Systems and Control Conference, Park City, UT, USA, 8–11 October 2019. V002T13A006. [Google Scholar] [CrossRef]
- Raquez, J.M.; Habibi, Y.; Murariu, M.; Dubois, P. Polylactide (PLA)-based nanocomposites. Prog. Polym. Sci. 2013, 38, 1504–1542. [Google Scholar] [CrossRef]
- Wu, Y.; Chiu, G. An Improved Height Difference Based Model of Height Profile for Drop-on-Demand 3D Printing with UV Curable Ink. In Proceedings of the 2021 American Control Conference (ACC), New Orleans, LA, USA, 25–28 May 2021; pp. 491–495. [Google Scholar] [CrossRef]
- Marques, P.A.A.P.; Gonçalves, G.; Singh, M.K.; Grácio, J. Graphene oxide and hydroxyapatite as fillers of polylactic acid nanocomposites: Preparation and characterisation. J. Nanosci. Nanotechnol. 2012, 12, 6686–6692. [Google Scholar] [CrossRef]
- Zhu, L.; Bai, M.; Xiao, S.; Liu, Y.; Zhu, Q.; Wang, Z.; Zhao, J.; Zhang, W.; Chen, D. In-situ monitoring of cellular H2O2 within 3D cell clusters using conductive scaffolds. Talanta 2024, 279, 126559. [Google Scholar] [CrossRef]
- Liu, C.; Wong, H.M.; Yeung, K.W.K.; Tjong, S.C. Novel Electrospun Polylactic Acid Nanocomposite Fiber Mats with Hybrid Graphene Oxide and Nanohydroxyapatite Reinforcements Having Enhanced Biocompatibility. Polymers 2016, 8, 287. [Google Scholar] [CrossRef]
- Wu, Y.; Chiu, G. Error Diffusion based Feedforward Height Control for Inkjet 3D Printing. In Proceedings of the 2023 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Seattle, WA, USA, 28–30 June 2023; pp. 125–131. [Google Scholar] [CrossRef]






| Lattice Type | Strut Diameter (mm) | Porosity (%) | Lattice Volume (mm3) |
|---|---|---|---|
| Schwartz Primitive | 0.6 | 75.09 | 660.04 |
| High-Density Schwartz Primitive | 0.6 | 0 | 2650.72 |
| Gyroid | 0.6 | 44.24 | 1477.91 |
| High-Density Gyroid | 0.6 | 0 | 2650.72 |
| PLA (%) | cHAP (%) | rGO (%) | Young Modulus (E) GPa | Shear Modulus (G) GPa | Bulk Modulus (GPa) | Poisson Ratio (ϑ) | Density (ῤ) kg/m3 |
|---|---|---|---|---|---|---|---|
| 89.9 | 10 | 0.1 | 5.42 | 2.02 | 5.65 | 0.34 | 1494.95 |
| 89.7 | 10 | 0.3 | 5.68 | 2.12 | 5.92 | 0.34 | 1496.64 |
| 89.5 | 10 | 0.5 | 5.99 | 2.22 | 6.66 | 0.35 | 1495.72 |
| Sample | Initial Mass (g) | Mass After Mixing (g) | Yield Stress (MPa) | Rz (µm) | Ra (µm) |
|---|---|---|---|---|---|
| 100/0-PLA/cHAP/rGO | 120.00 | 106.70 | 88.90 | 12.10 | 0.94 |
| 90/10-PLA/cHAP/rGO | 119.91 | 113.11 | 94.17 | 20.00 | 4.32 |
| 80/20-PLA/cHAP/rGO | 120.23 | 110.90 | 92.23 | 26.00 | 4.50 |
| Lattice Type | Stress (MPa) | Displacement (mm) | Elastic Strain | Energy (J) |
|---|---|---|---|---|
| Schwartz Primitive | 16.5 | 0.045 | 0.012 | 1.35 |
| Gyroid | 10.2 | 0.028 | 0.008 | 1.72 |
| High-Density Schwartz Primitive | 18.7 | 0.038 | 0.014 | 1.20 |
| High-Density Gyroid | 12.1 | 0.030 | 0.009 | 1.65 |
| TPMS Structure | PLA MPa | PLA/cHAP/rGO 0.1% MPa | PLA/cHAP/rGO 0.3% MPa | PLA/cHAP/rGO 0.5% MPa |
|---|---|---|---|---|
| Schwartz Primitive | 38.30 | 45.10 | 48.13 | 58.61 |
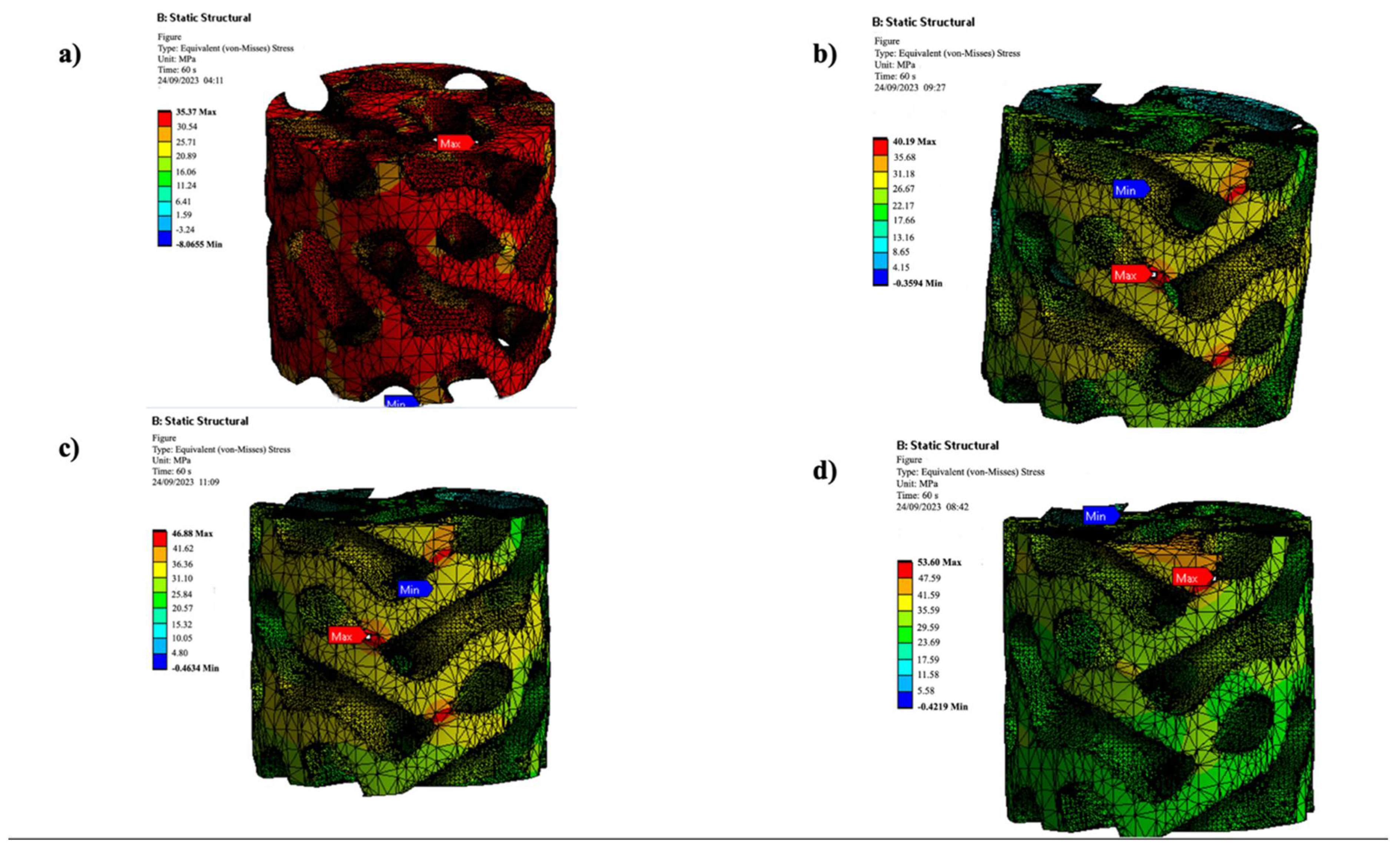
| Gyroid | 35.37 | 40.19 | 46.88 | 53.60 |
| Composite Type | Modulus of Elasticity (GPa) | UTS (MPa) | Compressive Strength (MPa) |
|---|---|---|---|
| Bulk | Schwartz Primitive | Gyroid | |
| PLA | 3.42 | 1.78 | 1.77 |
| PLA-10% cHAP | 4.86 | 2.98 | 2.56 |
| PLA-10% cHAP-0.1% rGO | 5.42 | 3.53 | 3.49 |
| PLA-10% cHAP-0.3% rGO | 5.68 | 3.82 | 3.79 |
| PLA-10% cHAP-0.5% rGO | 5.99 | 4.05 | 4.02 |
| Sample | Tg (°C) | Tm (°C) | Tc (°C) | ΔHm (J/g) | ΔHc (J/g) | Xc (%) |
|---|---|---|---|---|---|---|
| PLA/cHAP (10 wt%) | 61 | 159 | 114.8 | 25.1 | −25.9 | 30.1 |
| PLA/cHAP/rGO (0.1%) | 65 | 170 | 109 | 37.1 | 23.4 | 21.1 |
| PLA/cHAP/rGO (0.3%) | 65 | 170 | 109 | 36.4 | 22.7 | 21.4 |
| PLA/cHAP/rGO (0.5%) | 64 | 169 | 108 | 26.7 | 15.6 | 19.4 |
| Source | Material Used | Tensile Test (MPa) | Compressive Test (MPa) | Thermal Property | Key Findings |
|---|---|---|---|---|---|
| Omigbodun et al. [11] | PLA/cHAP/rGO | 56.78 | 107 | >300 °C | Enhanced mechanical properties and thermal stability |
| Balabanov et al. [3] | Polyamide with Schwartz Primitive topology | N/A | 50 | N/A | Improved strength for 3D-printed polyamide products |
| Maconachie et al. [16] | ABS Gyroid lattice structures | N/A | 44 | N/A | Superior strength in Gyroid lattice structures |
| Li et al. [19] | Graphene-based scaffolds for nerve repair | N/A | Not specified | N/A | Enhanced electrical conductivity and mechanical support |
| Omigbodun et al. [9] | PLA/Hydroxyapatite/Reduced Graphene Oxide | 29.17 | 53.60 | >200 °C | Confirmation and extension of the mechanical improvements |
| This Research | PLA/cHAP/rGO with Gyroid and Schwartz Lattices | 56.78 | 107 (Gyroid: 53.60, Schwartz: 58.61) | >300 °C | Use of Human systems to predict optimal lattice structures. Superior Gyroid lattice design for load-bearing applications. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Omigbodun, F.T.; Oladapo, B.I. AI-Optimized Lattice Structures for Biomechanics Scaffold Design. Biomimetics 2025, 10, 88. https://doi.org/10.3390/biomimetics10020088
Omigbodun FT, Oladapo BI. AI-Optimized Lattice Structures for Biomechanics Scaffold Design. Biomimetics. 2025; 10(2):88. https://doi.org/10.3390/biomimetics10020088
Chicago/Turabian StyleOmigbodun, Francis T., and Bankole I. Oladapo. 2025. "AI-Optimized Lattice Structures for Biomechanics Scaffold Design" Biomimetics 10, no. 2: 88. https://doi.org/10.3390/biomimetics10020088
APA StyleOmigbodun, F. T., & Oladapo, B. I. (2025). AI-Optimized Lattice Structures for Biomechanics Scaffold Design. Biomimetics, 10(2), 88. https://doi.org/10.3390/biomimetics10020088







