Bio-Inspired Design of a Porous Resorbable Scaffold for Bone Reconstruction: A Preliminary Study
Abstract
:1. Introduction
2. The Model Employed to Describe Bone Remodeling
2.1. Mechanical Formulation
- the bulk displacement vector, ,
- the Lagrangian porosity, ,
- 1.
- the finite strain tensor, , whose components are
- 2.
- and being the Lagrangian porosity corresponding to the current and the reference configuration, respectively. We can also express by the equationwhere is the average fluid-displacement vector defined in such a way that the volume of fluid displaced through unit areas is and represents the flow of the fluid relative to the solid but measured in terms of volume per unit area of the bulk medium.
2.2. Growth/Resorption Process Formulation
3. An Illustrative Theoretical Case: Numerical Implementation
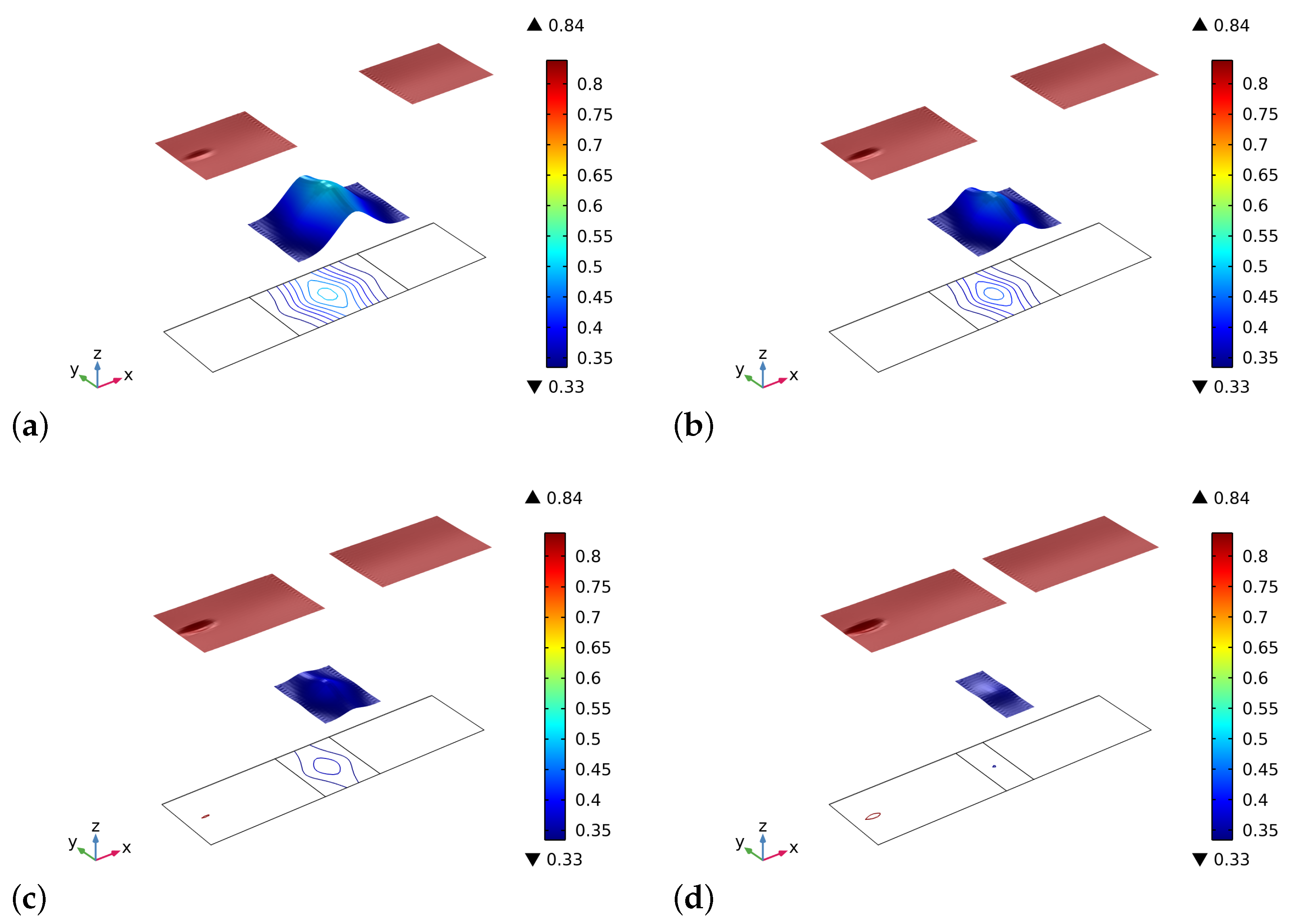
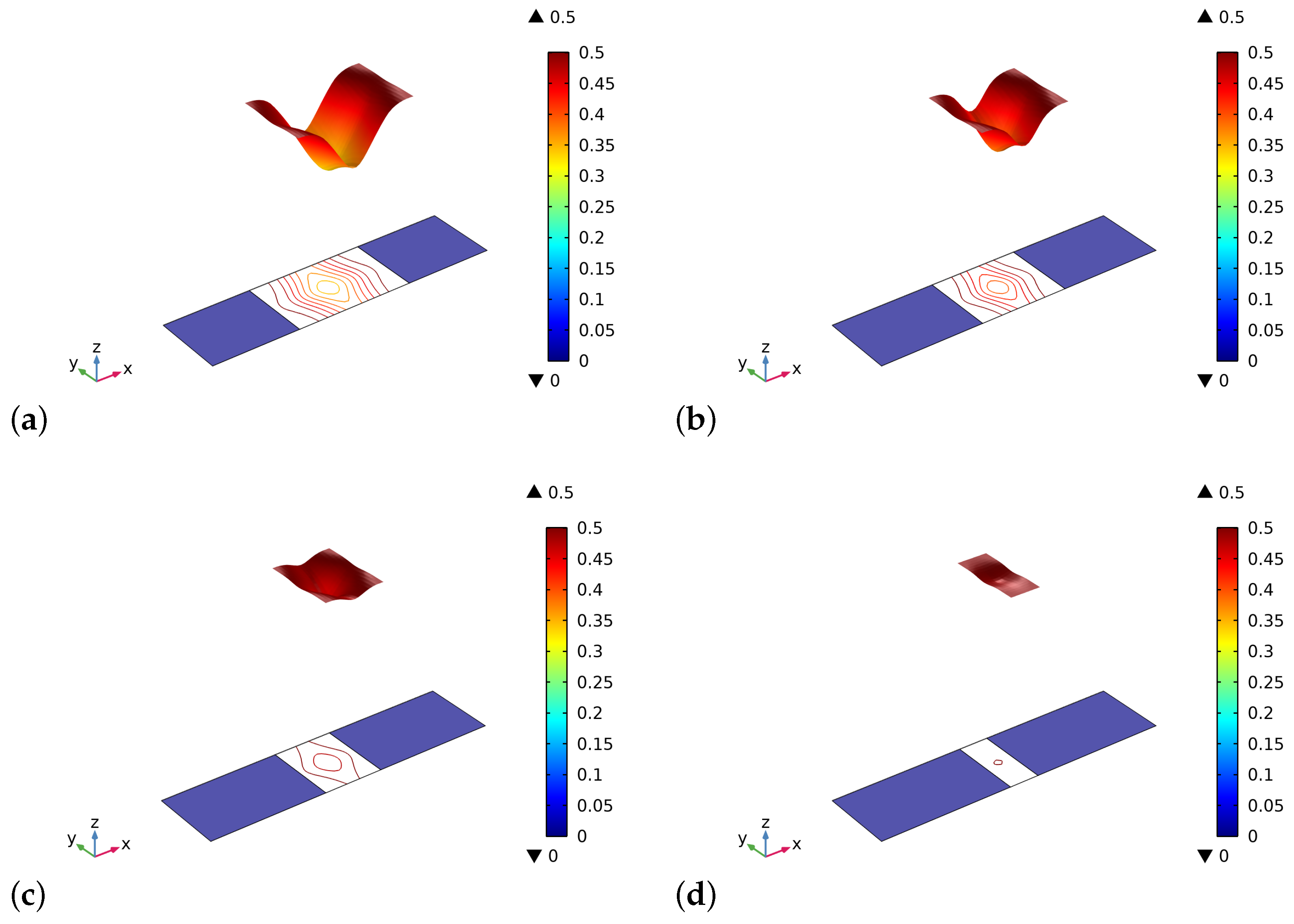
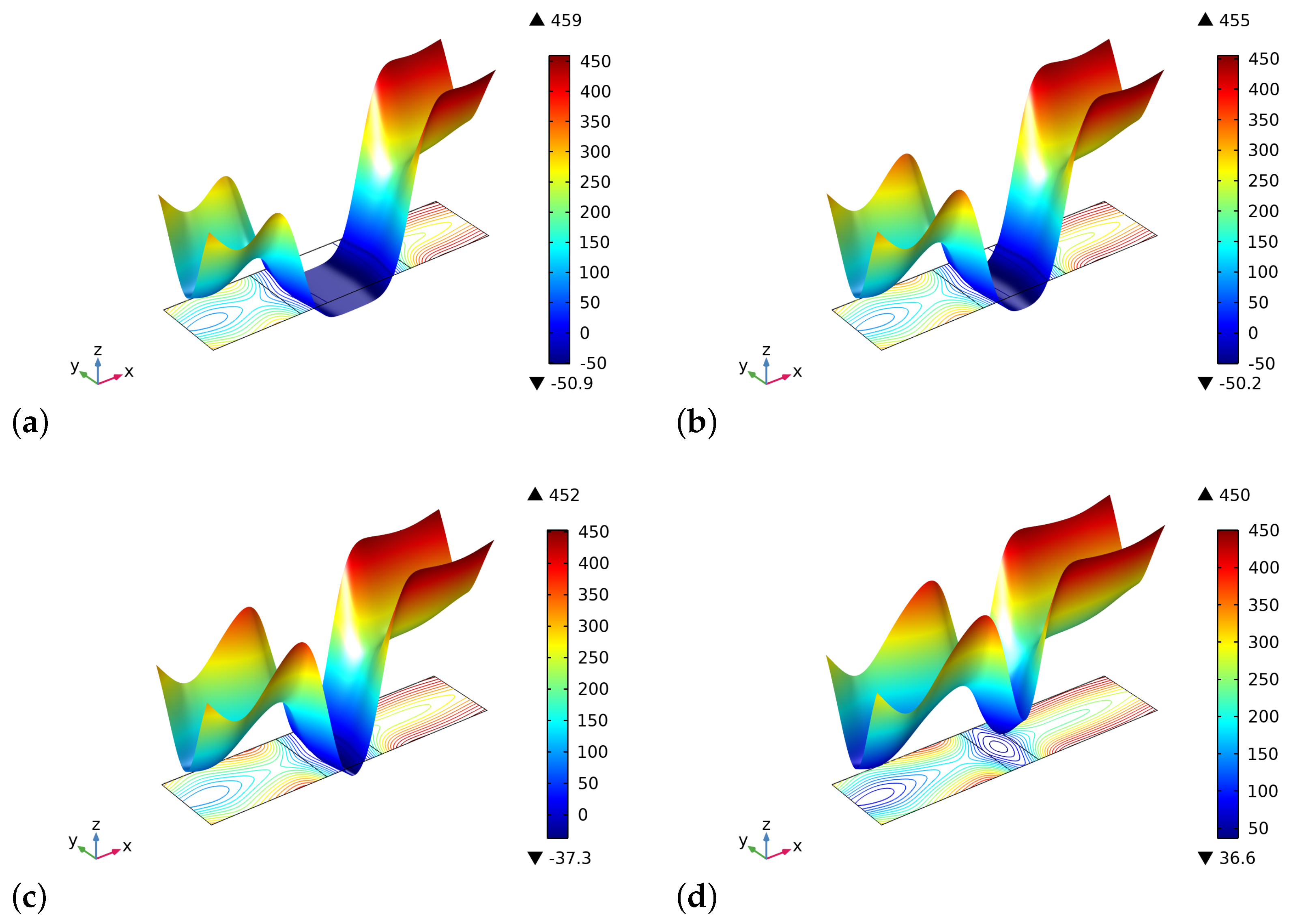
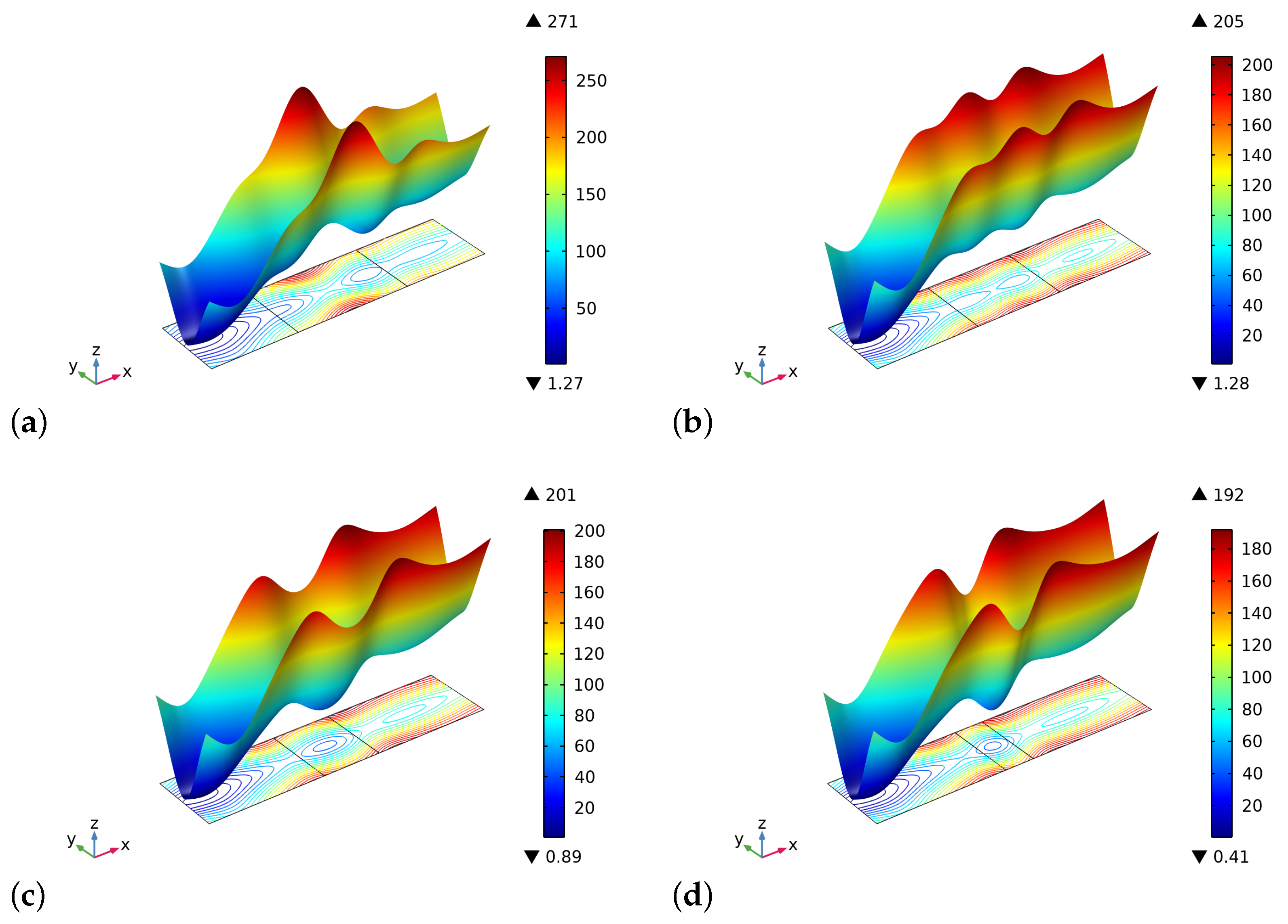
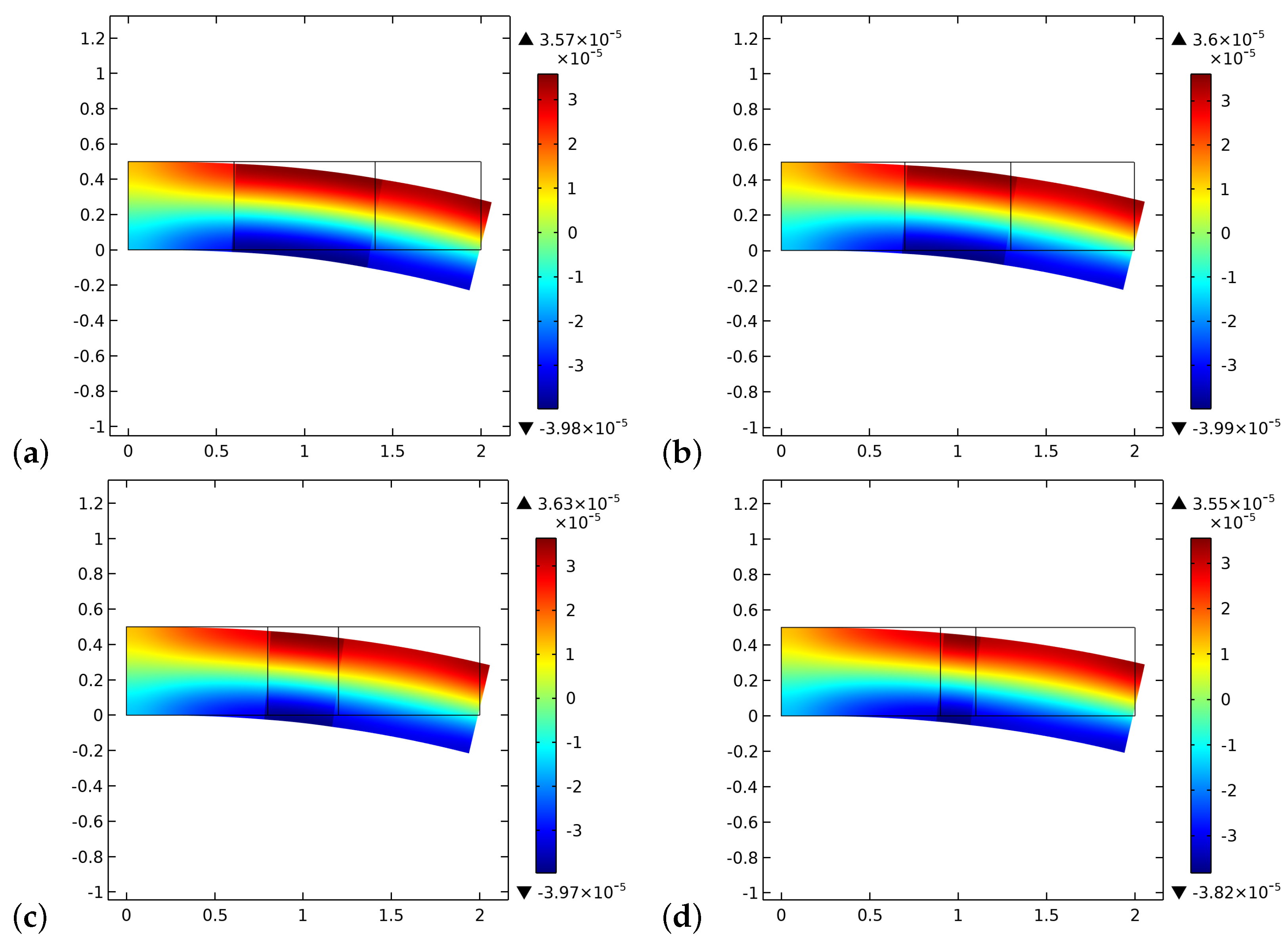
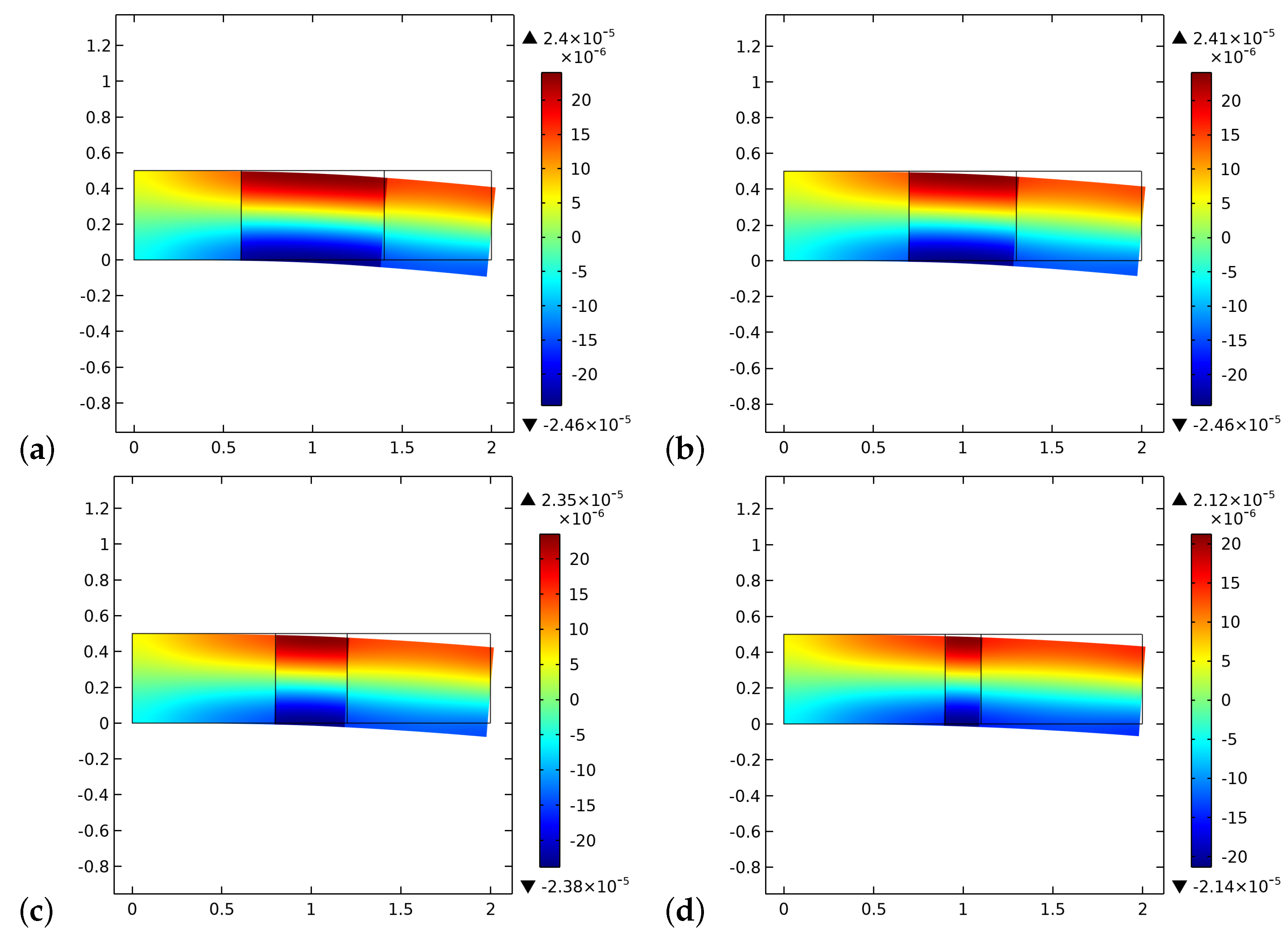
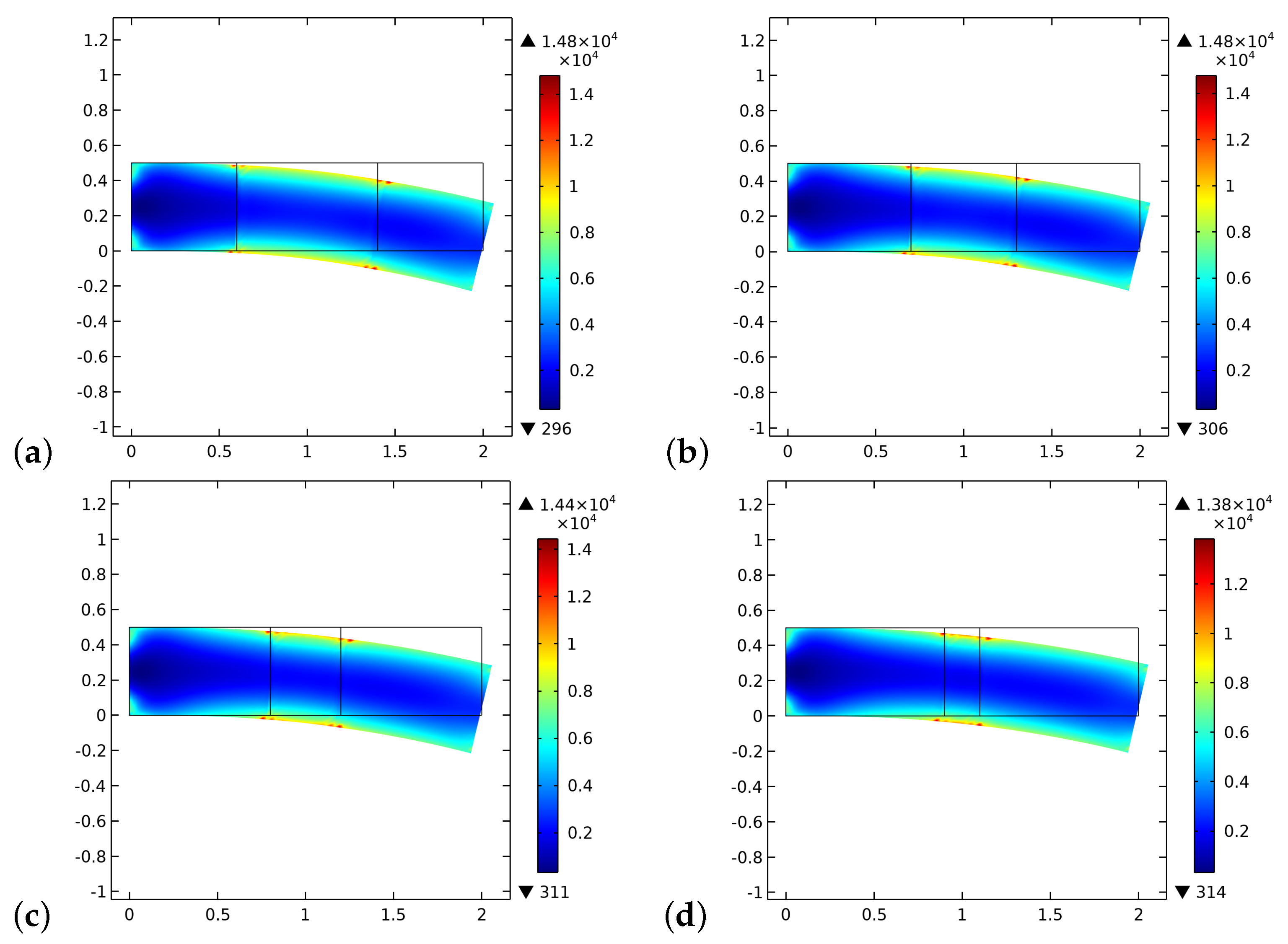
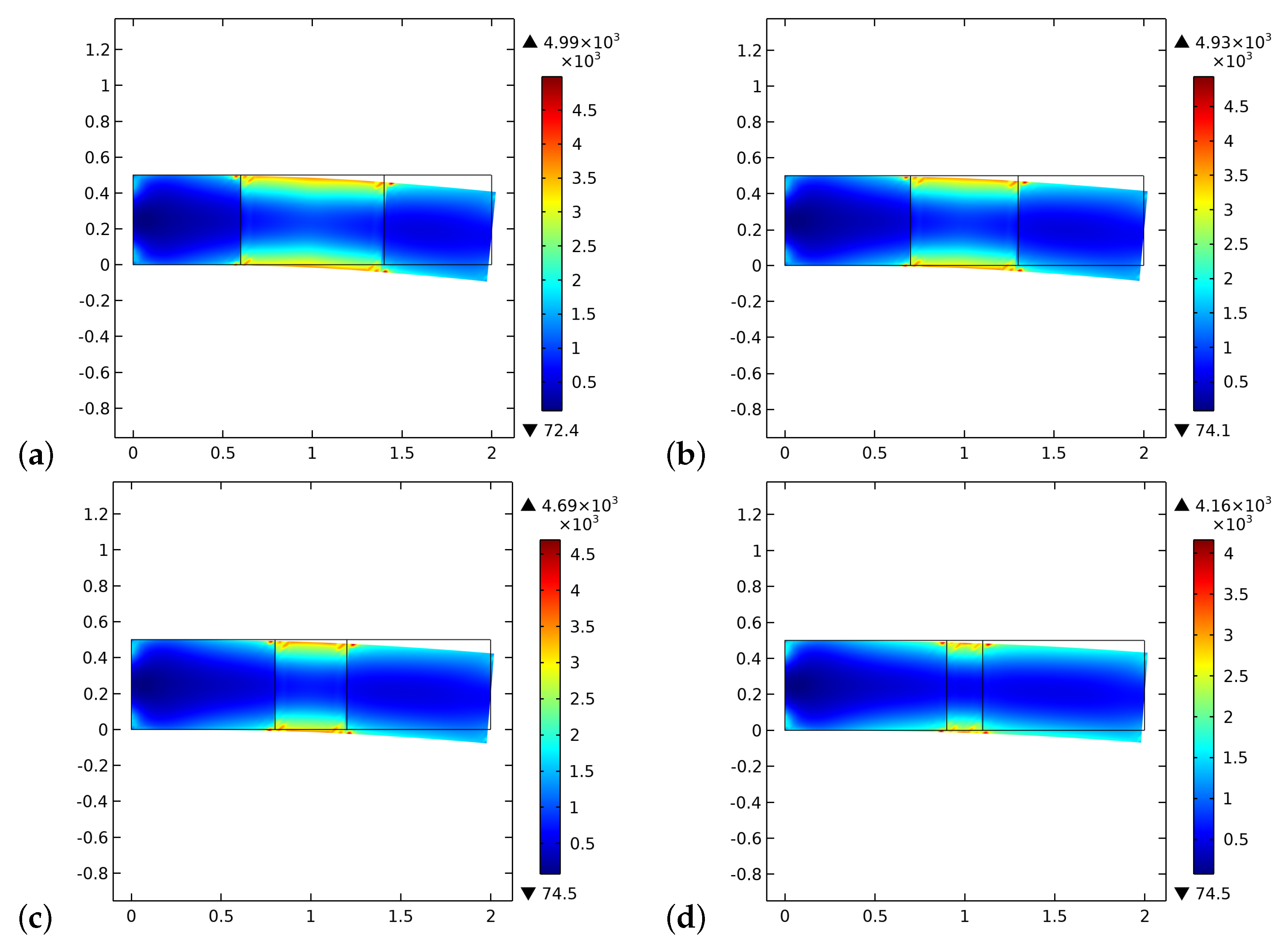
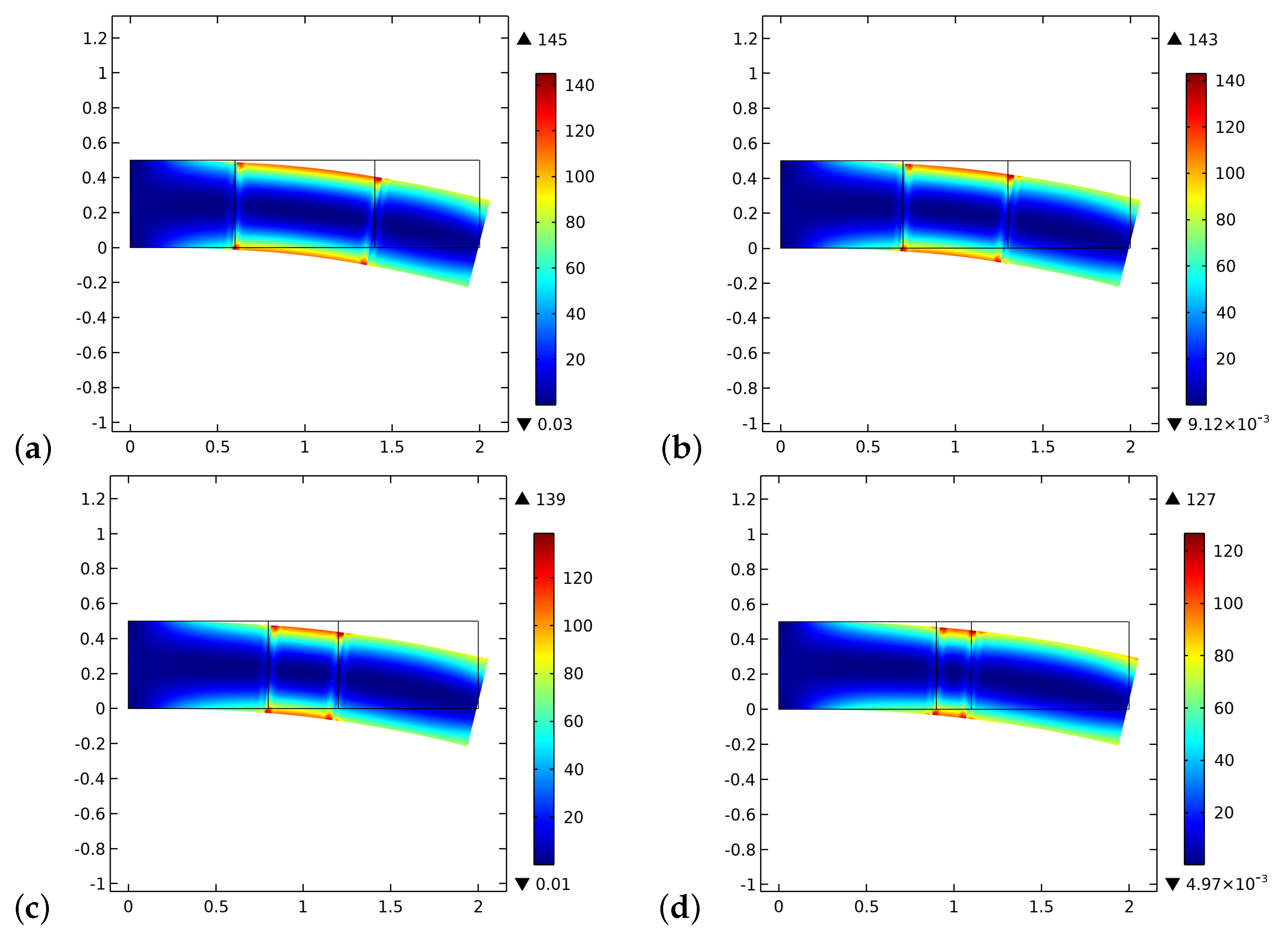
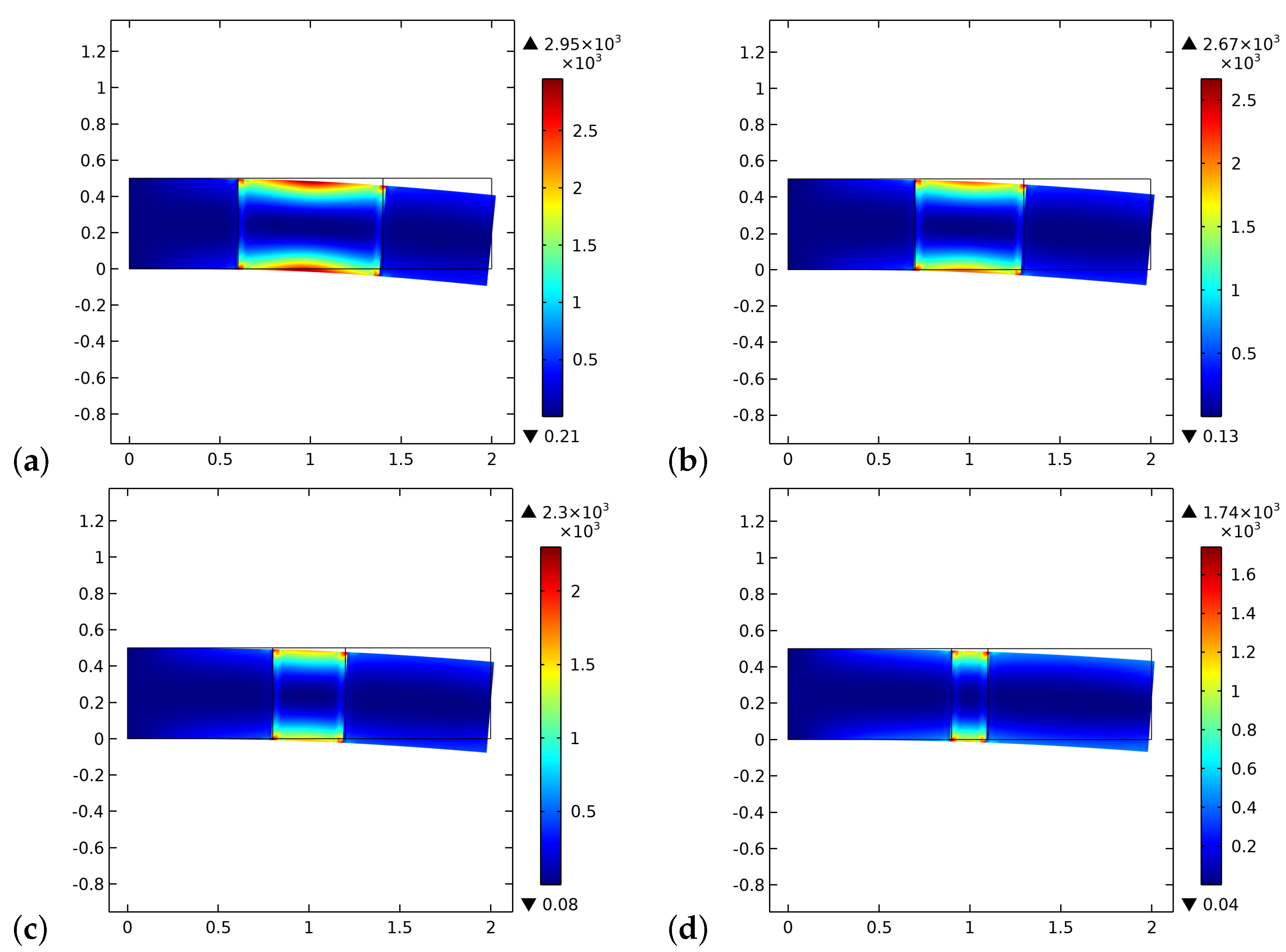
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| RMS | root mean square |
| PD | proportional derivative |
| bone-graft-bone |
References
- Cho, S.H.; Andersson, H.M.; White, S.R.; Sottos, N.R.; Braun, P.V. Polydimethylsiloxane-based self-healing materials. Adv. Mater. 2006, 18, 997–1000. [Google Scholar] [CrossRef]
- Toohey, K.S.; Sottos, N.R.; Lewis, J.A.; Moore, J.S.; White, S.R. Self-healing materials with microvascular networks. Nat. Mater. 2007, 6, 581–585. [Google Scholar] [CrossRef]
- Eremeyev, V.A.; Skrzat, A.; Vinakurava, A. Application of the micropolar theory to the strength analysis of bioceramic materials for bone reconstruction. Strength Mater. 2016, 48, 573–582. [Google Scholar] [CrossRef]
- Eremeyev, V.A.; Pietraszkiewicz, W. Material symmetry group and constitutive equations of micropolar anisotropic elastic solids. Math. Mech. Solids 2016, 21, 210–221. [Google Scholar] [CrossRef]
- Eremeyev, V.A.; Pietraszkiewicz, W. Material symmetry group of the non-linear polar-elastic continuum. Int. J. Solids Struct. 2012, 49, 1993–2005. [Google Scholar] [CrossRef] [Green Version]
- Madeo, A.; dell’Isola, F.; Darve, F. A continuum model for deformable, second gradient porous media partially saturated with compressible fluids. J. Mech. Phys. Solids 2013, 61, 2196–2211. [Google Scholar] [CrossRef] [Green Version]
- Rosi, G.; Placidi, L.; dell’Isola, F. “Fast” and “slow” pressure waves electrically induced by nonlinear coupling in Biot-type porous medium saturated by a nematic liquid crystal. Z. Angew. Math. Und Phys. 2017, 68, 51. [Google Scholar] [CrossRef] [Green Version]
- Alibert, J.J.; Seppecher, P.; dell’Isola, F. Truss modular beams with deformation energy depending on higher displacement gradients. Math. Mech. Solids 2003, 8, 51–73. [Google Scholar] [CrossRef]
- Pideri, C.; Seppecher, P. A second gradient material resulting from the homogenization of an heterogeneous linear elastic medium. Contin. Mech. Thermodyn. 1997, 9, 241–257. [Google Scholar] [CrossRef] [Green Version]
- Abdoul-Anziz, H.; Seppecher, P. Strain gradient and generalized continua obtained by homogenizing frame lattices. Math. Mech. Complex Syst. 2018, 6, 213–250. [Google Scholar] [CrossRef] [Green Version]
- George, D.; Allena, R.; Remond, Y. A multiphysics stimulus for continuum mechanics bone remodeling. Math. Mech. Complex Syst. 2018, 6, 307–319. [Google Scholar] [CrossRef]
- George, D.; Allena, R.; Bourzac, C.; Pallu, S.; Bensidhoum, M.; Portier, H.; Rémond, Y. A new comprehensive approach for bone remodeling under medium and high mechanical load based on cellular activity. Math. Mech. Complex Syst. 2020, 8, 287–306. [Google Scholar] [CrossRef]
- Hernandez-Rodriguez, Y.; Lekszycki, T. Novel description of bone remodelling including finite memory effect, stimulation and signalling mechanisms. Contin. Mech. Thermodyn. 2020, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Giorgio, I.; dell’Isola, F.; Andreaus, U.; Alzahrani, F.; Hayat, T.; Lekszycki, T. On mechanically driven biological stimulus for bone remodeling as a diffusive phenomenon. Biomech. Model. Mechanobiol. 2019, 18, 1639–1663. [Google Scholar] [CrossRef] [PubMed]
- Gross, T.S.; Edwards, J.L.; Mcleod, K.J.; Rubin, C.T. Strain gradients correlate with sites of periosteal bone formation. J. Bone Miner. Res. 1997, 12, 982–988. [Google Scholar] [CrossRef] [PubMed]
- Judex, S.; Gross, T.S.; Zernicke, R.F. Strain gradients correlate with sites of exercise-induced bone-forming surfaces in the adult skeleton. J. Bone Miner. Res. 1997, 12, 1737–1745. [Google Scholar] [CrossRef] [PubMed]
- Barchiesi, E.; Spagnuolo, M.; Placidi, L. Mechanical metamaterials: A state of the art. Math. Mech. Solids 2019, 24, 212–234. [Google Scholar] [CrossRef]
- dell’Isola, F.; Seppecher, P.; Alibert, J.J.; Lekszycki, T.; Grygoruk, R.; Pawlikowski, M.; Steigmann, D.; Giorgio, I.; Andreaus, U.; Hild, F.; et al. Pantographic metamaterials: An example of mathematically driven design and of its technological challenges. Contin. Mech. Thermodyn. 2019, 31, 851–884. [Google Scholar] [CrossRef] [Green Version]
- Di Cosmo, F.; Laudato, M.; Spagnuolo, M. Acoustic metamaterials based on local resonances: Homogenization, optimization and applications. In Generalized Models and Non-Classical Approaches in Complex Materials 1; Springer: Berlin/Heidelberg, Germany, 2018; pp. 247–274. [Google Scholar]
- Spagnuolo, M. Circuit analogies in the search for new metamaterials: Phenomenology of a mechanical diode. In Nonlinear Wave Dynamics of Materials and Structures; Springer: Berlin/Heidelberg, Germany, 2020; pp. 411–422. [Google Scholar]
- Yildizdag, M.E.; Tran, C.A.; Barchiesi, E.; Spagnuolo, M.; dell’Isola, F.; Hild, F. A multi-disciplinary approach for mechanical metamaterial synthesis: A hierarchical modular multiscale cellular structure paradigm. In State of the Art and Future Trends in Material Modeling; Springer: Berlin/Heidelberg, Germany, 2019; pp. 485–505. [Google Scholar]
- dell’Isola, F.; Seppecher, P.; Spagnuolo, M.; Barchiesi, E.; Hild, F.; Lekszycki, T.; Giorgio, I.; Placidi, L.; Andreaus, U.; Hayat, T.; et al. Advances in pantographic structures: Design, manufacturing, models, experiments and image analyses. Contin. Mech. Thermodyn. 2019, 31, 1231–1282. [Google Scholar] [CrossRef] [Green Version]
- Vangelatos, Z.; Melissinaki, V.; Farsari, M.; Komvopoulos, K.; Grigoropoulos, C. Intertwined microlattices greatly enhance the performance of mechanical metamaterials. Math. Mech. Solids 2019, 24, 2636–2648. [Google Scholar] [CrossRef]
- Yildizdag, M.E.; Barchiesi, E.; dell’Isola, F. Three-point bending test of pantographic blocks: Numerical and experimental investigation. Math. Mech. Solids 2020, 25, 1965–1978. [Google Scholar] [CrossRef]
- Turco, E.; Misra, A.; Sarikaya, R.; Lekszycki, T. Quantitative analysis of deformation mechanisms in pantographic substructures: Experiments and modeling. Contin. Mech. Thermodyn. 2019, 31, 209–223. [Google Scholar] [CrossRef]
- Turco, E. How the properties of pantographic elementary lattices determine the properties of pantographic metamaterials. In New Achievements in Continuum Mechanics and Thermodynamics; Springer: Berlin/Heidelberg, Germany, 2019; pp. 489–506. [Google Scholar]
- Turco, E.; Misra, A.; Pawlikowski, M.; dell’Isola, F.; Hild, F. Enhanced Piola–Hencky discrete models for pantographic sheets with pivots without deformation energy: Numerics and experiments. Int. J. Solids Struct. 2018, 147, 94–109. [Google Scholar] [CrossRef] [Green Version]
- Eugster, S.; dell’Isola, F.; Steigmann, D. Continuum theory for mechanical metamaterials with a cubic lattice substructure. Math. Mech. Complex Syst. 2019, 7, 75–98. [Google Scholar] [CrossRef] [Green Version]
- Della Corte, A.; Giorgio, I.; Scerrato, D. A review of recent developments in mathematical modeling of bone remodeling. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2020, 234, 273–281. [Google Scholar] [CrossRef]
- Giorgio, I.; Spagnuolo, M.; Andreaus, U.; Scerrato, D.; Bersani, A.M. In-depth gaze at the astonishing mechanical behavior of bone: A review for designing bio-inspired hierarchical metamaterials. Math. Mech. Solids 2020. [Google Scholar] [CrossRef]
- Coussy, O. Poromechanics; John Wiley & Sons: Hoboken, NJ, USA, 2004. [Google Scholar]
- Biot, M.A. Mechanics of deformation and acoustic propagation in porous media. J. Appl. Phys. 1962, 33, 1482–1498. [Google Scholar] [CrossRef]
- Morgan, E.F.; Yeh, O.C.; Chang, W.C.; Keaveny, T.M. Nonlinear behavior of trabecular bone at small strains. J. Biomech. Eng. 2001, 123, 1–9. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sansalone, V.; Martin, M.; Haïat, G.; Pivonka, P.; Lemaire, T. A new model of bone remodeling and turnover set up in the framework of generalized continuum mechanics. Math. Mech. Solids 2021. [Google Scholar] [CrossRef]
- Lekszycki, T.; dell’Isola, F. A mixture model with evolving mass densities for describing synthesis and resorption phenomena in bones reconstructed with bio-resorbable materials. Z. Angew. Math. Mech. 2012, 92, 426–444. [Google Scholar] [CrossRef] [Green Version]
- Lu, Y.; Lekszycki, T. Modeling of an initial stage of bone fracture healing. Contin. Mech. Thermodyn. 2015, 27, 851–859. [Google Scholar] [CrossRef] [Green Version]
- Lu, Y.; Lekszycki, T. Modelling of bone fracture healing: Influence of gap size and angiogenesis into bioresorbable bone substitute. Math. Mech. Solids 2017, 22, 1997–2010. [Google Scholar] [CrossRef]
- Bednarczyk, E.; Lekszycki, T. A novel mathematical model for growth of capillaries and nutrient supply with application to prediction of osteophyte onset. Z. Angew. Math. Und Phys. 2016, 67, 1–14. [Google Scholar] [CrossRef]
- Frasca, P.; Harper, R.; Katz, J.L. Strain and frequency dependence of shear storage modulus for human single osteons and cortical bone microsamples—Size and hydration effects. J. Biomech. 1981, 14, 679–690. [Google Scholar] [CrossRef]
- Madeo, A.; George, D.; Lekszycki, T.; Nierenberger, M.; Rémond, Y. A second gradient continuum model accounting for some effects of micro-structure on reconstructed bone remodelling. C R Mec. 2012, 340, 575–589. [Google Scholar] [CrossRef]
- dell’Isola, F.; Andreaus, U.; Placidi, L. At the origins and in the vanguard of peridynamics, non-local and higher-gradient continuum mechanics: An underestimated and still topical contribution of Gabrio Piola. Math. Mech. Solids 2015, 20, 887–928. [Google Scholar] [CrossRef]
- Auffray, N.; dell’Isola, F.; Eremeyev, V.A.; Madeo, A.; Rosi, G. Analytical continuum mechanics à la Hamilton–Piola least action principle for second gradient continua and capillary fluids. Math. Mech. Solids 2015, 20, 375–417. [Google Scholar] [CrossRef] [Green Version]
- Germain, P. The method of virtual power in the mechanics of continuous media, I: Second-gradient theory. Math. Mech. Complex Syst. 2020, 8, 153–190. [Google Scholar] [CrossRef]
- Epstein, M.; Smelser, R. An appreciation and discussion of Paul Germain’s “The method of virtual power in the mechanics of continuous media, I: Second-gradient theory”. Math. Mech. Complex Syst. 2020, 8, 191–199. [Google Scholar] [CrossRef]
- Cowin, S.C. Bone poroelasticity. J. Biomech. 1999, 32, 217–238. [Google Scholar] [CrossRef]
- Giorgio, I.; Andreaus, U.; dell’Isola, F.; Lekszycki, T. Viscous second gradient porous materials for bones reconstructed with bio-resorbable grafts. Extrem. Mech. Lett. 2017, 13, 141–147. [Google Scholar] [CrossRef]
- Mindlin, R.D. Micro-structure in linear elasticity. Arch. Ration. Mech. Anal. 1964, 16, 51–78. [Google Scholar] [CrossRef]
- Toupin, R.A. Elastic materials with couple-stresses. Arch. Rational Mech. Anal. 1962, 11, 385–414. [Google Scholar] [CrossRef] [Green Version]
- Allena, R.; Cluzel, C. Heterogeneous directions of orthotropy in three-dimensional structures: Finite element description based on diffusion equations. Math. Mech. Complex Syst. 2018, 6, 339–351. [Google Scholar] [CrossRef]
- Cluzel, C.; Allena, R. A general method for the determination of the local orthotropic directions of heterogeneous materials: Application to bone structures using μCT images. Math. Mech. Complex Syst. 2018, 6, 353–367. [Google Scholar] [CrossRef]
- Peng, L.; Bai, J.; Zeng, X.; Zhou, Y. Comparison of isotropic and orthotropic material property assignments on femoral finite element models under two loading conditions. Med. Eng. Phys. 2006, 28, 227–233. [Google Scholar] [CrossRef]
- Goda, I.; Assidi, M.; Belouettar, S.; Ganghoffer, J.F. A micropolar anisotropic constitutive model of cancellous bone from discrete homogenization. J. Mech. Behav. Biomed. Mater. 2012, 16, 87–108. [Google Scholar] [CrossRef]
- Alibert, J.J.; Della Corte, A. Homogenization of nonlinear inextensible pantographic structures by Γ-convergence. Math. Mech. Complex Syst. 2019, 7, 1–24. [Google Scholar] [CrossRef] [Green Version]
- Giorgio, I.; Harrison, P.; dell’Isola, F.; Alsayednoor, J.; Turco, E. Wrinkling in engineering fabrics: A comparison between two different comprehensive modelling approaches. Proc. R. Soc. Math. Phys. Eng. Sci. 2018, 474, 20180063. [Google Scholar] [CrossRef]
- Abali, B.E.; Wu, C.C.; Müller, W.H. An energy-based method to determine material constants in nonlinear rheology with applications. Contin. Mech. Thermodyn. 2016, 28, 1221–1246. [Google Scholar] [CrossRef]
- Rosi, G.; Placidi, L.; Auffray, N. On the validity range of strain-gradient elasticity: A mixed static-dynamic identification procedure. Eur. J. Mech. A/Solids 2018, 69, 179–191. [Google Scholar] [CrossRef] [Green Version]
- De Angelo, M.; Placidi, L.; Nejadsadeghi, N.; Misra, A. Non-standard Timoshenko beam model for chiral metamaterial: Identification of stiffness parameters. Mech. Res. Commun. 2020, 103, 103462. [Google Scholar] [CrossRef]
- Placidi, L. A variational approach for a nonlinear 1-dimensional second gradient continuum damage model. Continuum. Mech. Therm. 2015, 27, 623–638. [Google Scholar] [CrossRef]
- Placidi, L. A variational approach for a nonlinear one-dimensional damage-elasto-plastic second-gradient continuum model. Continuum. Mech. Therm. 2016, 28, 119–137. [Google Scholar] [CrossRef]
- Misra, A.; Singh, V. Micromechanical model for viscoelastic materials undergoing damage. Continuum. Mech. Therm. 2013, 25, 343–358. [Google Scholar] [CrossRef]
- Placidi, L.; Barchiesi, E.; Misra, A. A strain gradient variational approach to damage: A comparison with damage gradient models and numerical results. Math. Mech. Complex Syst. 2018, 6, 77–100. [Google Scholar] [CrossRef]
- Placidi, L.; Misra, A.; Barchiesi, E. Two-dimensional strain gradient damage modeling: A variational approach. Z. Angew. Math. Phys. 2018, 69, 1–19. [Google Scholar] [CrossRef]
- Placidi, L.; Barchiesi, E. Energy approach to brittle fracture in strain-gradient modelling. Proc. R. Soc. Math. Phys. A Eng. Sci. 2018, 474, 20170878. [Google Scholar] [CrossRef]
- Timofeev, D.; Barchiesi, E.; Misra, A.; Placidi, L. Hemivariational continuum approach for granular solids with damage-induced anisotropy evolution. Math. Mech. Solids 2020, 1081286520968149. [Google Scholar] [CrossRef]
- Giorgio, I.; Andreaus, U.; Scerrato, D.; dell’Isola, F. A visco-poroelastic model of functional adaptation in bones reconstructed with bio-resorbable materials. Biomech. Model. Mechanobiol. 2016, 15, 1325–1343. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Garner, E.; Lakes, R.; Lee, T.; Swan, C.; Brand, R. Viscoelastic dissipation in compact bone: Implications for stress-induced fluid flow in bone. J. Biomech. Eng. 2000, 122, 166–172. [Google Scholar] [CrossRef] [Green Version]
- Cowin, S.C.; Nunziato, J.W. Linear elastic materials with voids. J. Elast. 1983, 13, 125–147. [Google Scholar] [CrossRef]
- Biot, M.A. Generalized theory of acoustic propagation in porous dissipative media. J. Acoust. Soc. Am. 1962, 34, 1254–1264. [Google Scholar] [CrossRef]
- Giorgio, I.; Andreaus, U.; Scerrato, D.; Braidotti, P. Modeling of a non-local stimulus for bone remodeling process under cyclic load: Application to a dental implant using a bioresorbable porous material. Math. Mech. Solids 2017, 22, 1790–1805. [Google Scholar] [CrossRef] [Green Version]
- Aretusi, G.; Ciallella, A. An Application of Coulomb-Friction Model to Predict Internal Dissipation in Concrete. In Mathematical Applications in Continuum and Structural Mechanics; Marmo, F., Sessa, S., Barchiesi, E., Spagnuolo, M., Eds.; Springer Nature Switzerland AG: Cham, Switzerland, 2021; Volume 127, Advanced Structured Materials. [Google Scholar]
- Heinemann, P.; Kasperski, M. Damping Induced by Walking and Running. Procedia Eng. 2017, 199, 2826–2831. [Google Scholar] [CrossRef]
- Eriksen, E.F. Cellular mechanisms of bone remodeling. Rev. Endocr. Metab. Disord. 2010, 11, 219–227. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Green, A.E.; Rivlin, R.S. Multipolar continuum mechanics. Arch. Ration. Mech. Anal. 1964, 17, 113–147. [Google Scholar] [CrossRef]
- Polizzotto, C. A note on the higher order strain and stress tensors within deformation gradient elasticity theories: Physical interpretations and comparisons. Int. J. Solids Struct. 2016, 90, 116–121. [Google Scholar] [CrossRef]
- Andreaus, U.; Giorgio, I.; Madeo, A. Modeling of the interaction between bone tissue and resorbable biomaterial as linear elastic materials with voids. Z. Angew. Math. Phys. 2015, 66, 209–237. [Google Scholar] [CrossRef] [Green Version]
- Kumar, C.; Jasiuk, I.; Dantzig, J. Dissipation energy as a stimulus for cortical bone adaptation. J. Mech. Mater. Struct. 2011, 6, 303–319. [Google Scholar] [CrossRef] [Green Version]
- Andreaus, U.; Colloca, M.; Iacoviello, D. Optimal bone density distributions: Numerical analysis of the osteocyte spatial influence in bone remodeling. Comput. Methods Programs Biomed. 2014, 113, 80–91. [Google Scholar] [CrossRef]
- Andreaus, U.; Colloca, M.; Iacoviello, D. An optimal control procedure for bone adaptation under mechanical stimulus. Control. Eng. Pract. 2012, 20, 575–583. [Google Scholar] [CrossRef]
- Andreaus, U.; Colloca, M.; Iacoviello, D.; Pignataro, M. Optimal-tuning PID control of adaptive materials for structural efficiency. Struct. Multidiscip. Optim. 2011, 43, 43–59. [Google Scholar] [CrossRef]
- Carriero, A.; Pereira, A.; Wilson, A.J.; Castagno, S.; Javaheri, B.; Pitsillides, A.; Marenzana, M.; Shefelbine, S.J. Spatial relationship between bone formation and mechanical stimulus within cortical bone: Combining 3D fluorochrome mapping and poroelastic finite element modelling. Bone Rep. 2018, 8, 72–80. [Google Scholar] [CrossRef]
- Tiwari, A.K.; Prasad, J. Cortical Bone Adaptation to Mechanical Environment: Strain Energy Density Versus Fluid Motion. In Biomanufacturing; Springer: Berlin/Heidelberg, Germany, 2019; pp. 241–271. [Google Scholar]
- Hambli, R.; Kourta, A. A theory for internal bone remodeling based on interstitial fluid velocity stimulus function. Appl. Math. Model. 2015, 39, 3525–3534. [Google Scholar] [CrossRef]
- Forest, S. Micromorphic approach for gradient elasticity, viscoplasticity, and damage. J. Eng. Mech. 2009, 135, 117–131. [Google Scholar] [CrossRef]
- Cazzani, A.; Malagù, M.; Turco, E. Isogeometric analysis of plane-curved beams. Math. Mech. Solids 2016, 21, 562–577. [Google Scholar] [CrossRef]
- Cazzani, A.; Malagù, M.; Turco, E.; Stochino, F. Constitutive models for strongly curved beams in the frame of isogeometric analysis. Math. Mech. Solids 2016, 21, 183–209. [Google Scholar] [CrossRef]
- Greco, L.; Cuomo, M.; Contrafatto, L. A reconstructed local B formulation for isogeometric Kirchhoff–Love shells. Comput. Methods Appl. Mech. Eng. 2018, 332, 462–487. [Google Scholar] [CrossRef]
- Greco, L.; Cuomo, M.; Contrafatto, L. Two new triangular G1-conforming finite elements with cubic edge rotation for the analysis of Kirchhoff plates. Comput. Methods Appl. Mech. Eng. 2019, 356, 354–386. [Google Scholar] [CrossRef]
- Balobanov, V.; Niiranen, J. Locking-free variational formulations and isogeometric analysis for the Timoshenko beam models of strain gradient and classical elasticity. Comput. Methods Appl. Mech. Eng. 2018, 339, 137–159. [Google Scholar] [CrossRef]
- Yildizdag, M.E.; Ardic, I.T.; Demirtas, M.; Ergin, A. Hydroelastic vibration analysis of plates partially submerged in fluid with an isogeometric FE-BE approach. Ocean. Eng. 2019, 172, 316–329. [Google Scholar] [CrossRef]
- Yildizdag, M.E.; Demirtas, M.; Ergin, A. Multipatch discontinuous Galerkin isogeometric analysis of composite laminates. Contin. Mech. Thermodyn. 2020, 32, 607–620. [Google Scholar] [CrossRef]
- dell’Erba, R. Swarm robotics and complex behaviour of continuum material. Contin. Mech. Thermodyn. 2019, 31, 989–1014. [Google Scholar] [CrossRef] [Green Version]
- dell’Erba, R. Position-based dynamic of a particle system: A configurable algorithm to describe complex behaviour of continuum material starting from swarm robotics. Contin. Mech. Thermodyn. 2018, 30, 1069–1090. [Google Scholar] [CrossRef] [Green Version]

| (GPa) | (GPa) | (kg/m3) | (kg/m3) | |
|---|---|---|---|---|
| 17 | 13.6 | 0.3 | 1800 | 1800 |
| (GPa) | (N) | (N/m3) | (N/m) | (N/m) |
| 1.7 | ||||
| (N s/m2) | (N s/m2) | (s/m2) | (s/m2) | (s/m2) |
| D (mm) | (N/m2) | (N/m2) | ||
| 1 | 1 | 1 | 50.97 | 56.33 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Scerrato, D.; Bersani, A.M.; Giorgio, I. Bio-Inspired Design of a Porous Resorbable Scaffold for Bone Reconstruction: A Preliminary Study. Biomimetics 2021, 6, 18. https://doi.org/10.3390/biomimetics6010018
Scerrato D, Bersani AM, Giorgio I. Bio-Inspired Design of a Porous Resorbable Scaffold for Bone Reconstruction: A Preliminary Study. Biomimetics. 2021; 6(1):18. https://doi.org/10.3390/biomimetics6010018
Chicago/Turabian StyleScerrato, Daria, Alberto Maria Bersani, and Ivan Giorgio. 2021. "Bio-Inspired Design of a Porous Resorbable Scaffold for Bone Reconstruction: A Preliminary Study" Biomimetics 6, no. 1: 18. https://doi.org/10.3390/biomimetics6010018
APA StyleScerrato, D., Bersani, A. M., & Giorgio, I. (2021). Bio-Inspired Design of a Porous Resorbable Scaffold for Bone Reconstruction: A Preliminary Study. Biomimetics, 6(1), 18. https://doi.org/10.3390/biomimetics6010018








