Perspectives of Gas Phase Ion Chemistry: Spectroscopy and Modeling
Abstract
:1. Introduction
2. Methodology
2.1. Experiments at Elettra Synchrotron
2.2. Computational Methodology
3. Atom/Ion Transfer Reactions
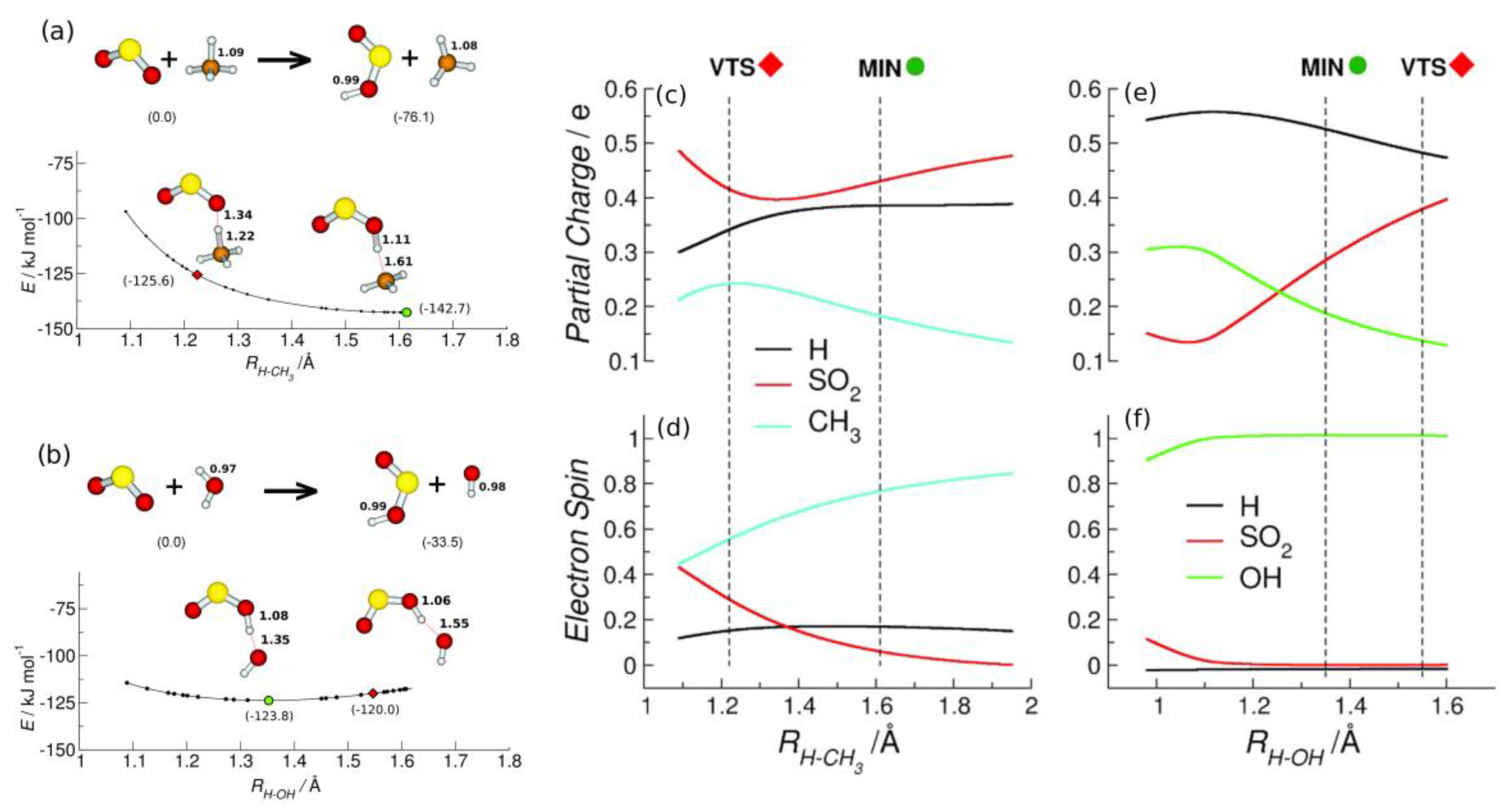
3.1. SO2-CH4/H2O
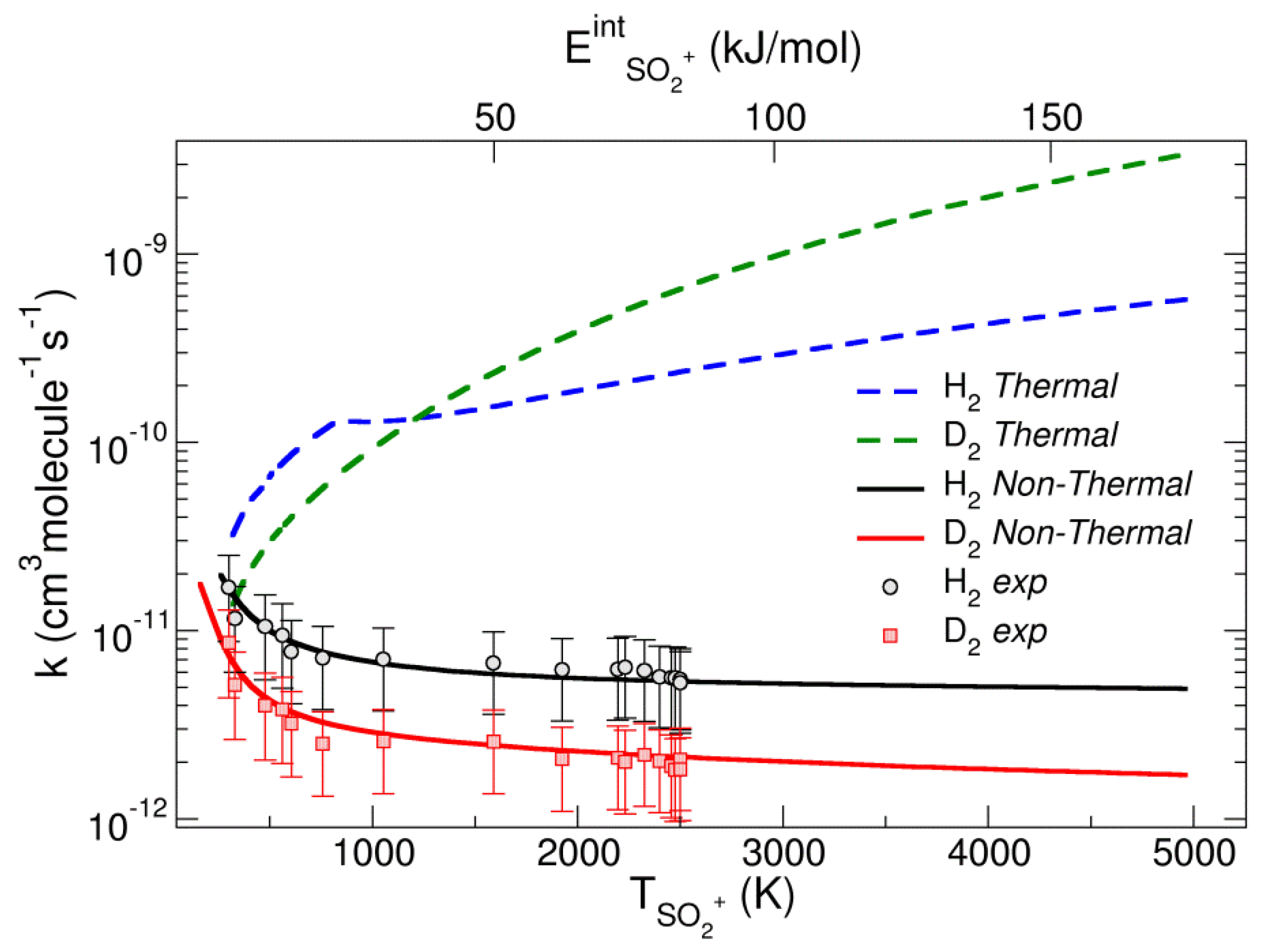
3.2. SO2/H2
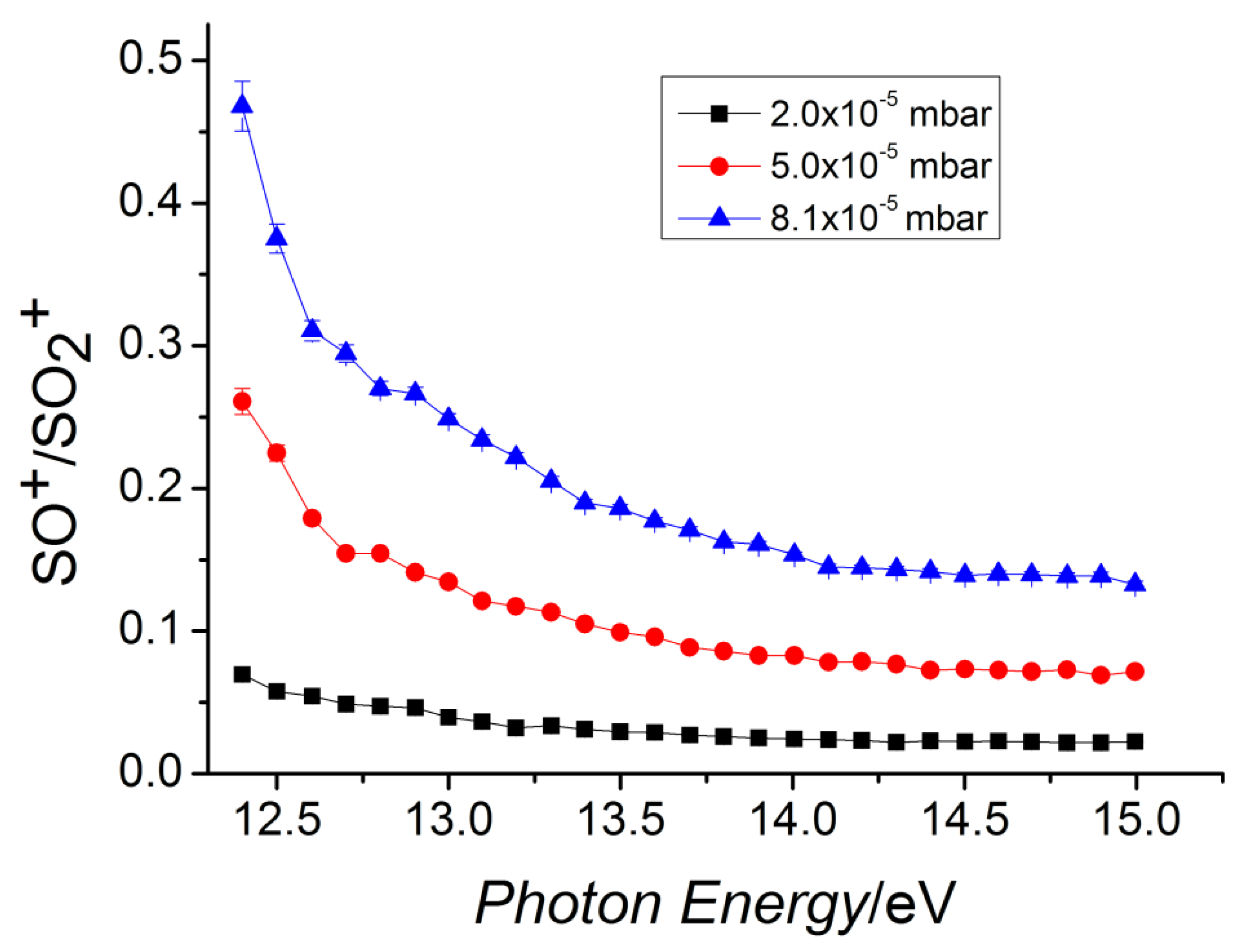
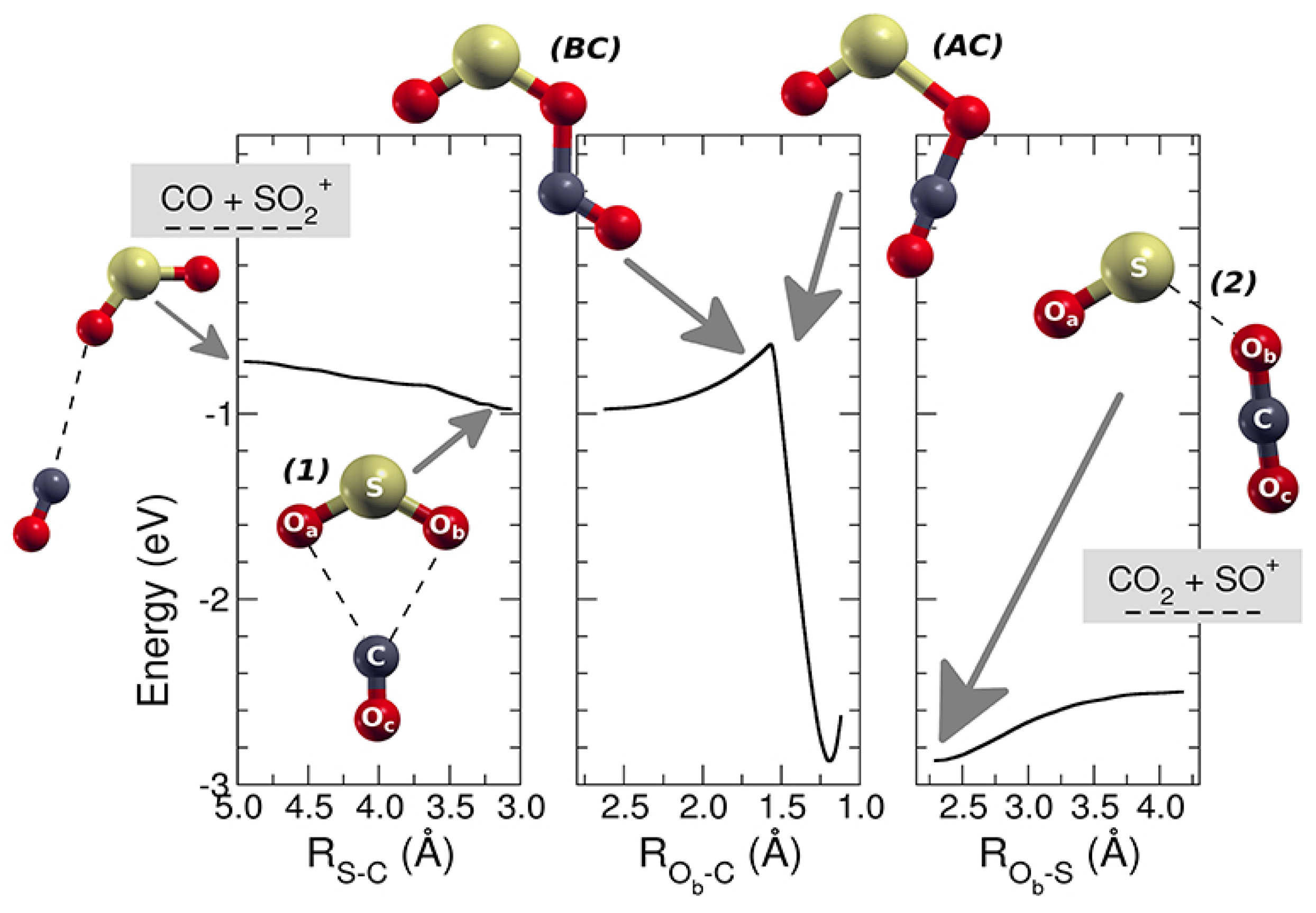
3.3. SO2/CO
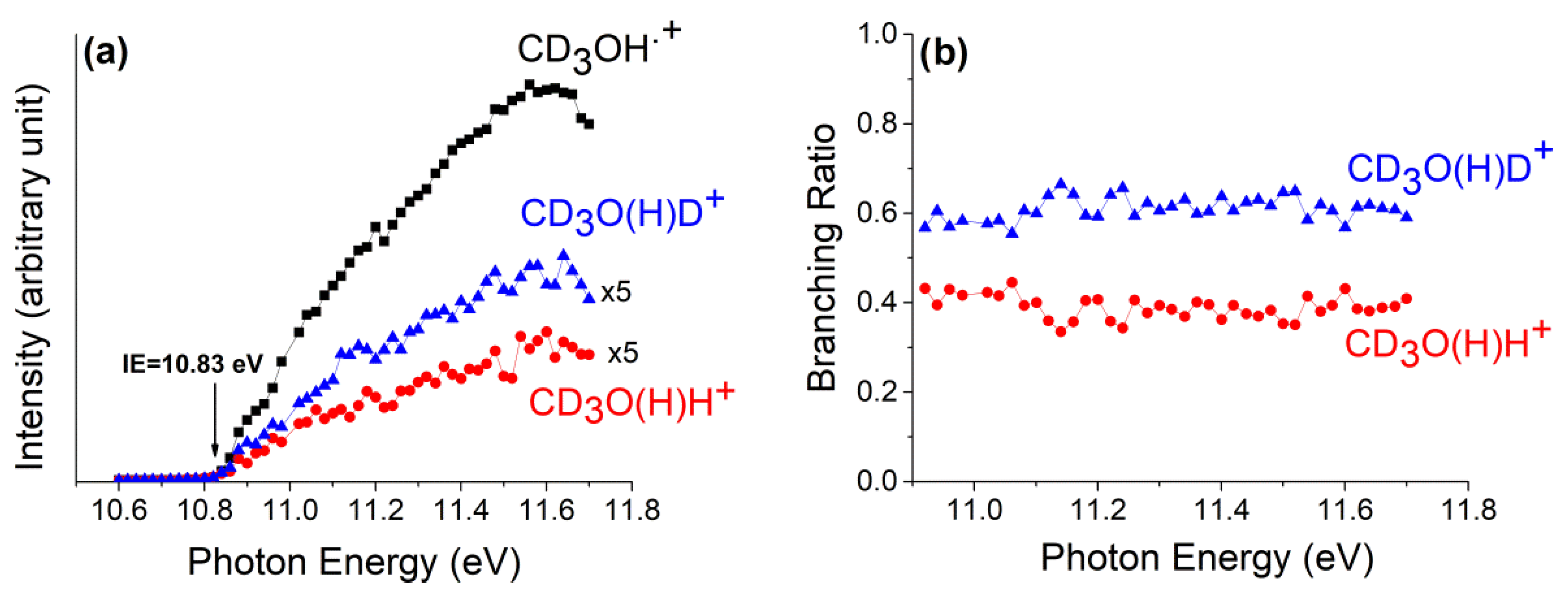
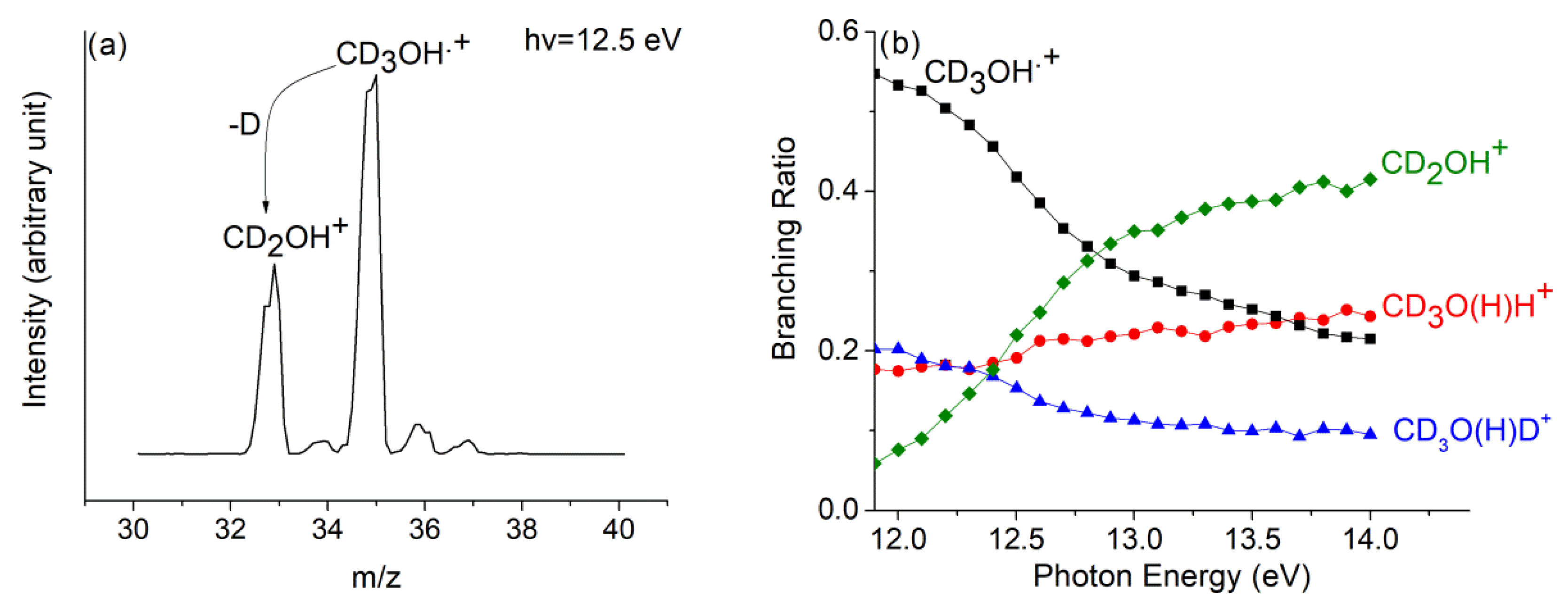
3.4. CH3OH+-CH3OH
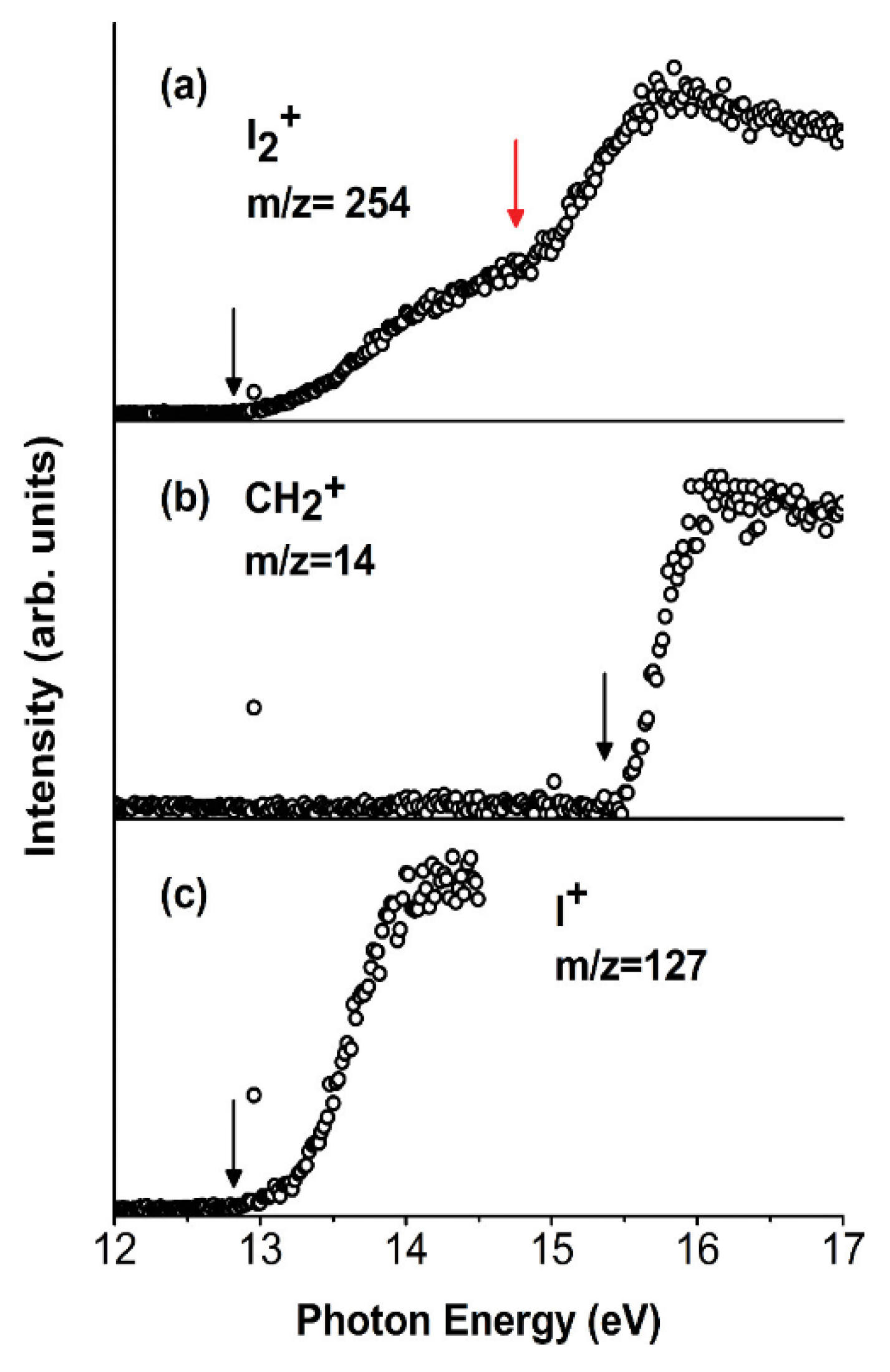
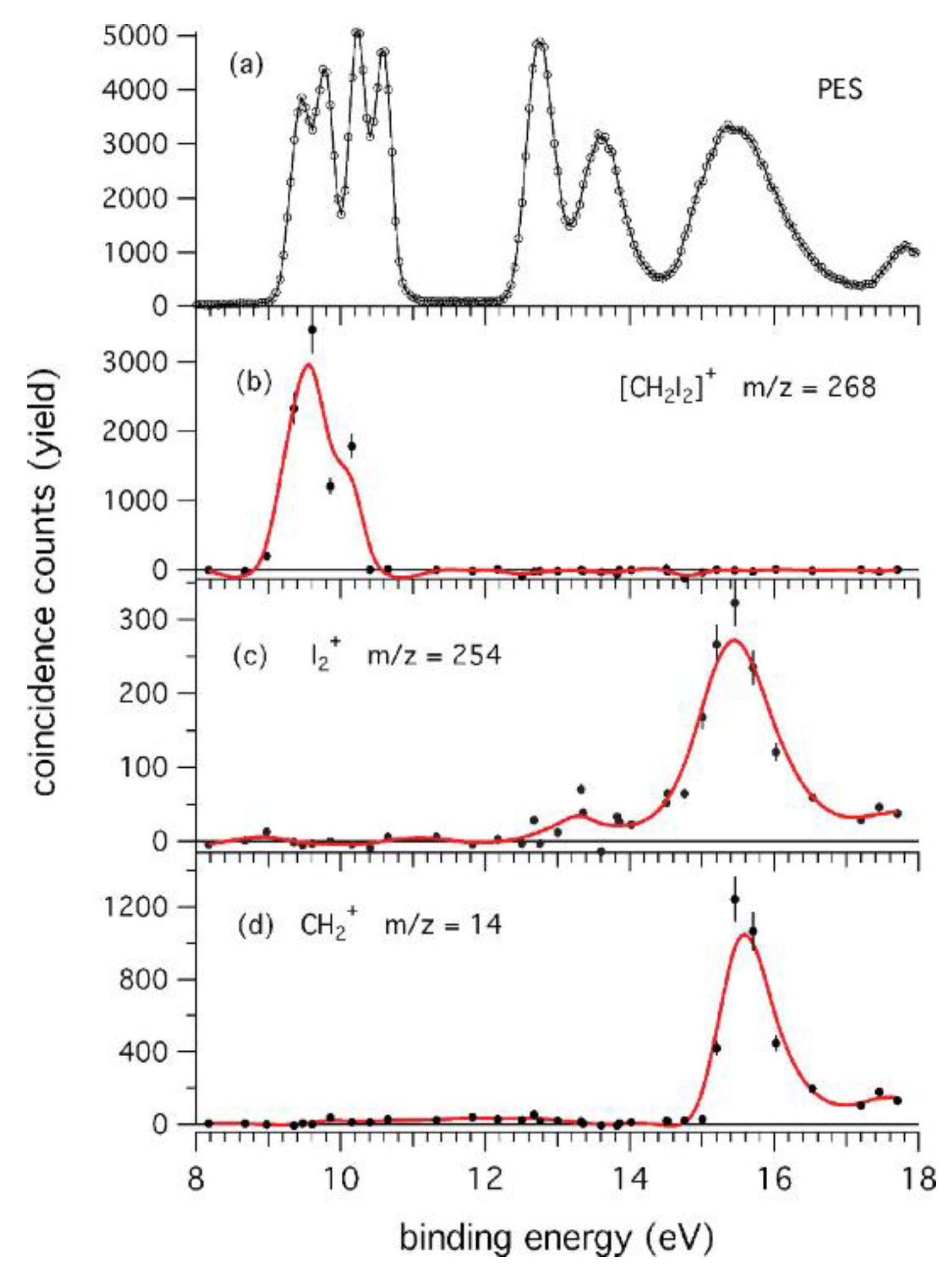
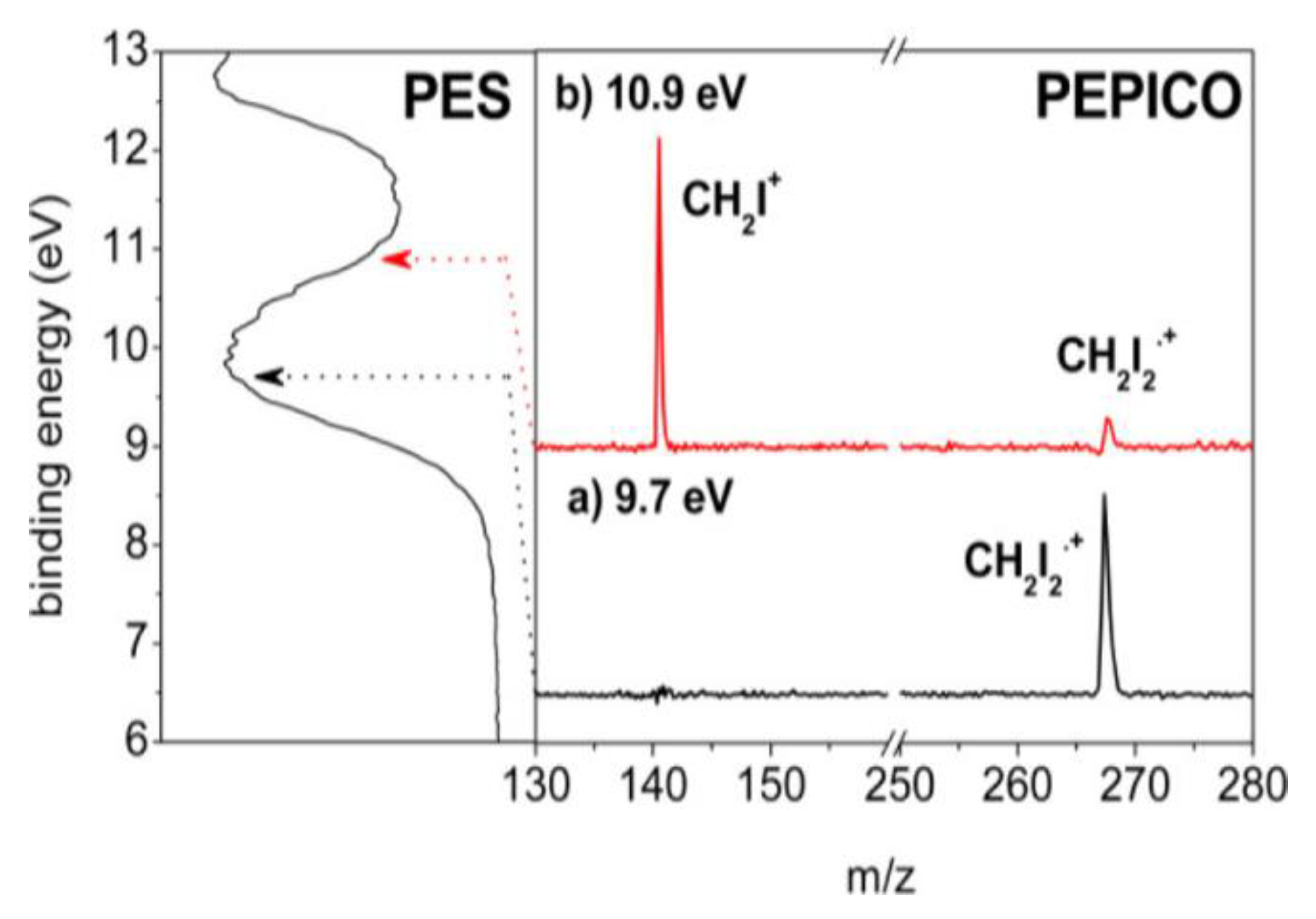
4. Photofragmentations of Halomethanes
5. Perspectives
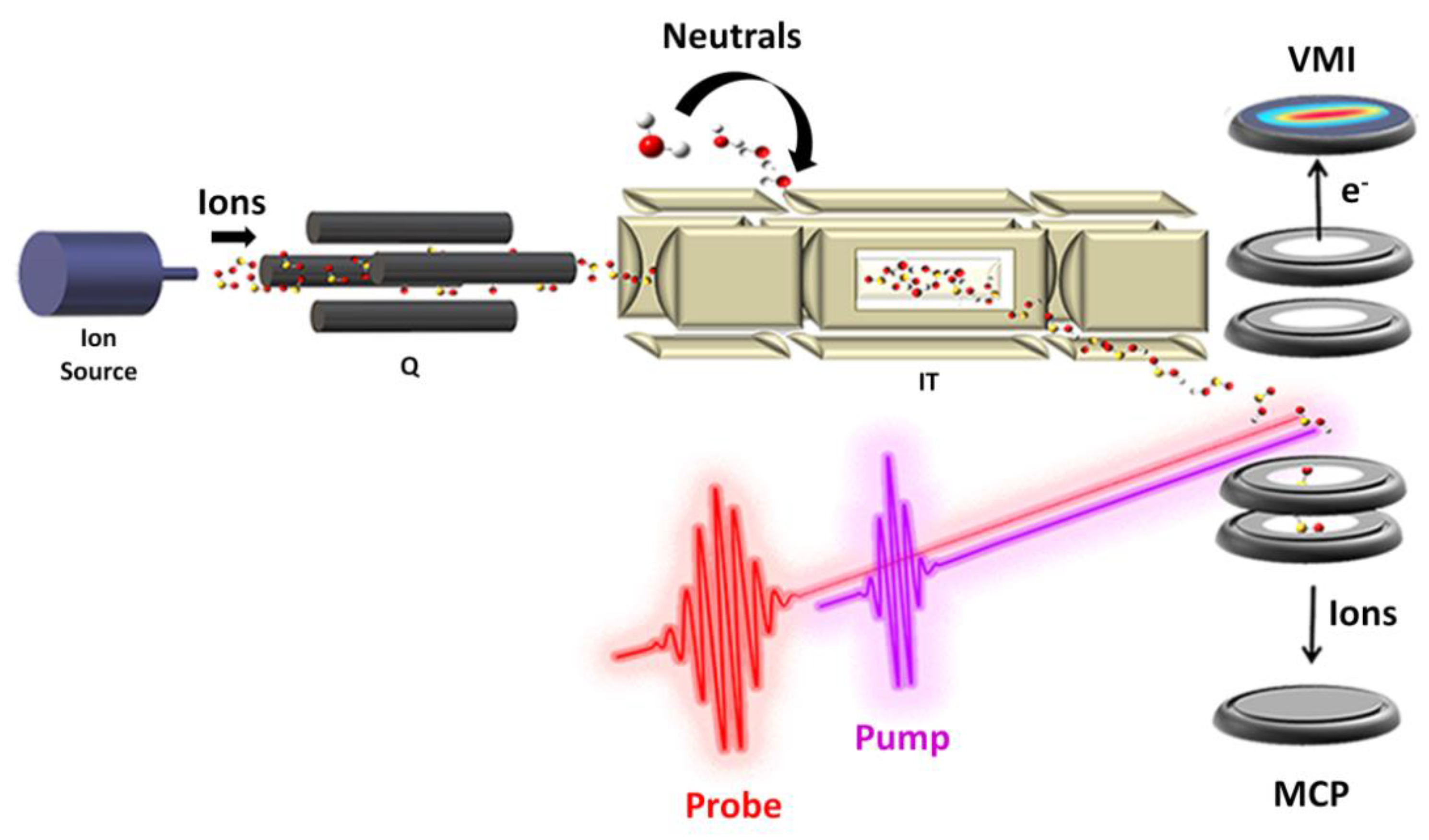
5.1. Planning Experiments at the FEL Facilities
5.1.1. Ultrafast Reaction Dynamics
5.1.2. Study the Intermediate State of a Reaction: A Challenge Still Open
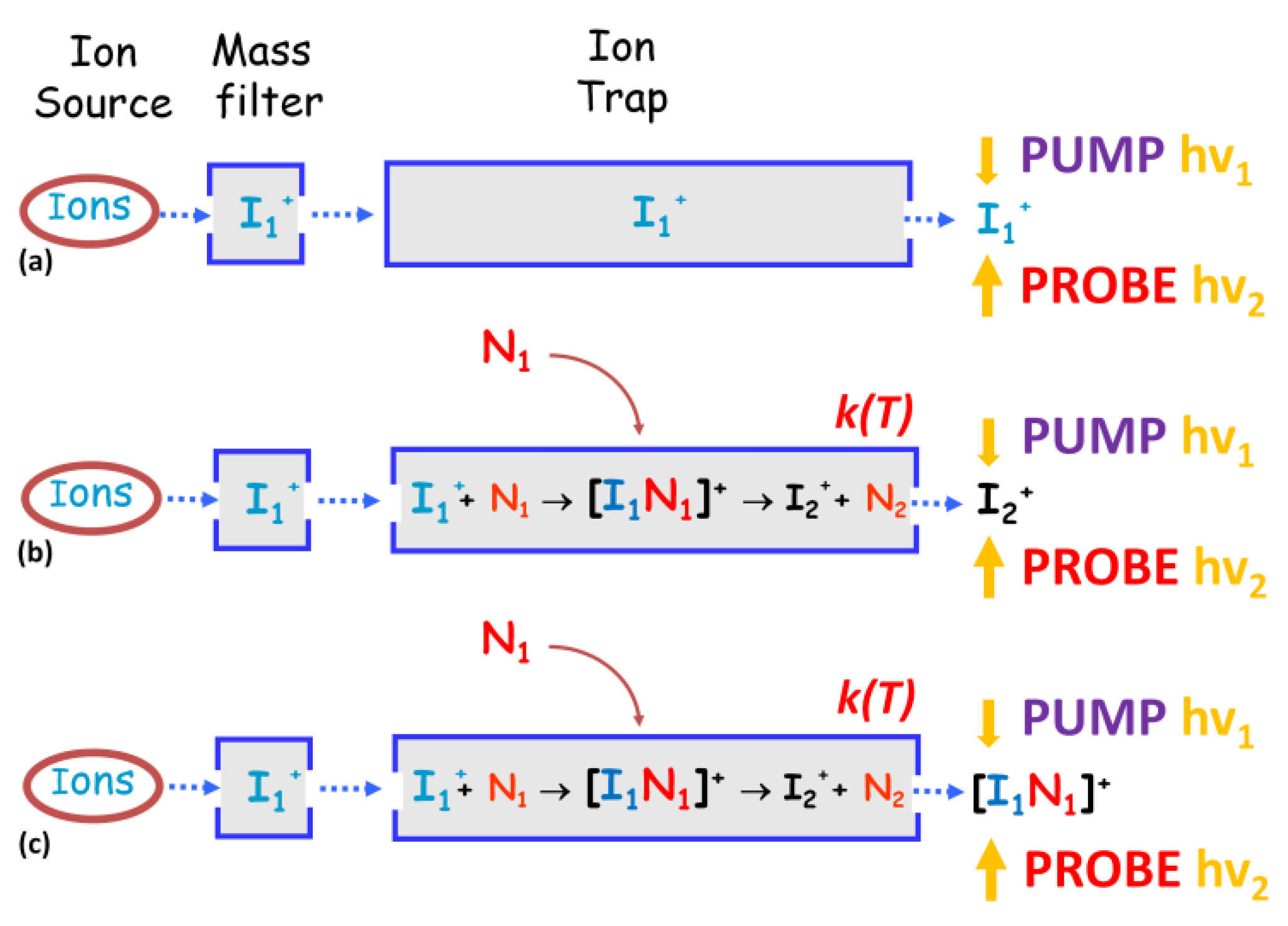
5.1.3. Time-Resolved Ion-Molecule Reactions in Gas Phase
5.2. Improvement in Modeling the Ion–Molecule Reactions
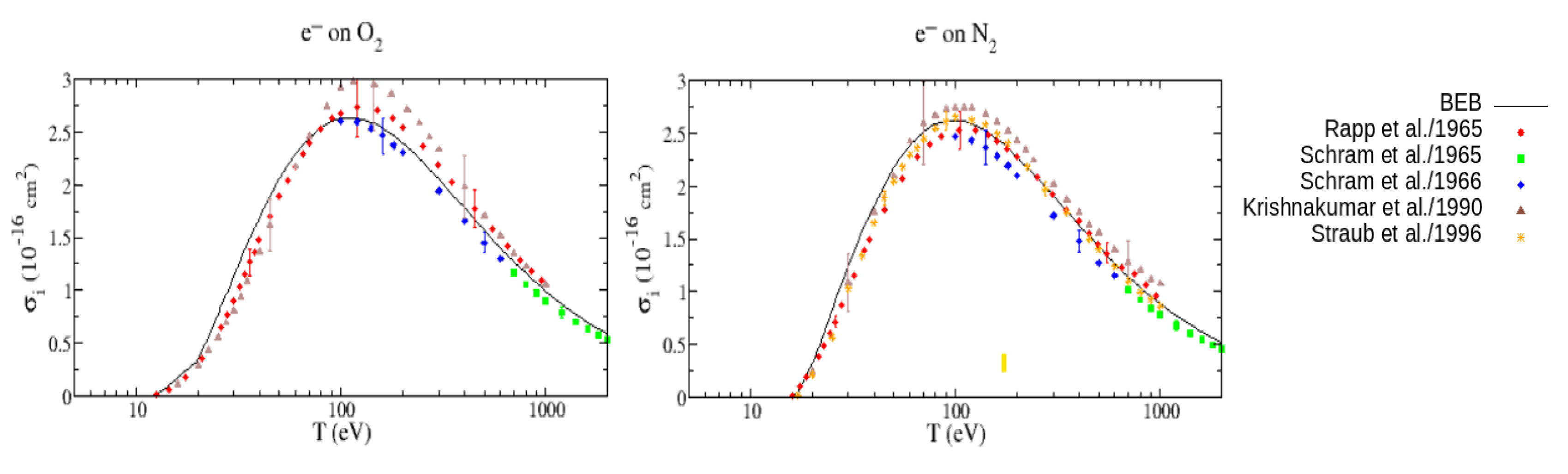
5.2.1. Ionisation
5.2.2. Elastic Scattering
5.2.3. Electronic Excitation
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Larsson, M.; Geppert, W.D.; Nyman, G. Ion chemistry in space. Rep. Prog. Phys. 2012, 75, 066901. [Google Scholar] [CrossRef] [PubMed]
- Mollah, M.Y.A.; Schennach, R.; Patscheider, J.; Promreuk, S.; Cocke, D.L. Plasma Chemistry as a Tool for Green Chemistry, Environmental Analysis and Waste Management. J. Hazard. Mater. 2000, 79, 301–320. [Google Scholar] [CrossRef]
- Castrovilli, M.C.; Markush, P.; Bolognesi, P.; Rousseau, P.; Maclot, S.; Cartoni, A.; Delaunay, R.; Domaracka, A.; Kočišek, J.; Huber, B.A.; et al. Fragmentation of pure and hydrated clusters of 5Br-uracil by low energy carbon ions: Observation of hydrated fragments. Phys. Chem. Chem. Phys. 2017, 19, 19807–19814. [Google Scholar] [CrossRef] [PubMed]
- Geppert, W.D.; Larsson, M. Experimental Investigations into Astrophysically Relevant Ionic Reactions. Chem. Rev. 2013, 113, 8872–8905. [Google Scholar] [CrossRef]
- de Petris, G.; Cartoni, A.; Troiani, A.; Angelini, G.; Ursini, O. Water activation by SO2+ ions: An effective source of OH radicals. Phys. Chem. Chem. Phys. 2009, 11, 9976. [Google Scholar] [CrossRef]
- Ravishankara, A.R.; Randall, D.A.; Hurrell, J.W. Complex and yet predictable: The message of the 2021 Nobel Prize in Physics. Proc. Natl. Acad. Sci. USA 2022, 119, e2120669119. [Google Scholar] [CrossRef]
- Board, O.S. ; National Research Council. Climate Intervention: Reflecting Sunlight to Cool Earth; National Academies Press: Washington, DC, USA, 2015. [Google Scholar]
- Satta, M.; Casavola, A.R.; Cartoni, A.; Castrovilli, M.C.; Catone, D.; Chiarinelli, J.; Borocci, S.; Avaldi, L.; Bolognesi, P. “Ionization of 2- and 4(5)-Nitroimidazoles Radiosensitizers: A “Kinetic Competition” Between NO2 and NO Losses” Chem. Phys. Chem. 2021, 22, 2387–2391. [Google Scholar] [CrossRef]
- Casavola, A.R.; Cartoni, A.; Castrovilli, M.C.; Borocci, S.; Bolognesi, P.; Chiarinelli, J.; Catone, D.; Avaldi, L. VUV Photofragmentation of Chloroiodomethane: The Iso-CH2I-Cl and Iso-CH2Cl-I Radical Cation Formation. J. Phys. Chem. A 2020, 124, 7491–7499. [Google Scholar] [CrossRef]
- Ball, J.A.; Gottlieb, C.A.; Lilley, A.E.; Radford, H.E. Detection of Methyl Alcohol in Sagittarius. Astrophys. J. 1970, 162, L203. [Google Scholar] [CrossRef]
- Available online: http://astrochymist.org (accessed on 5 May 2022).
- Burkholder, J.B.; Abbatt, J.P.D.; Barnes, I.; Roberts, J.M.; Melamed, M.L.; Ammann, M.; Bertram, A.K.; Cappa, C.D.; Carlton, A.G.; Carpenter, L.J.; et al. The Essential Role for Laboratory Studies in Atmospheric Chemistry. Environ. Sci. Technol. 2017, 51, 2519–2528. [Google Scholar] [CrossRef] [Green Version]
- Derossi, A.; Lama, F.; Piacentini, M.; Prosperi, T.; Zema, N. High flux and High Resolution Beamline for Elliptically Polarized Radiation in the Vacuum Ultraviolet and Soft X-ray Regions. Rev. Sei. Instrum. 1995, 66, 1718–1720. [Google Scholar] [CrossRef]
- Cartoni, A.; Casavola, A.R.; Bolognesi, P.; Castrovilli, M.C.; Catone, D.; Chiarinelli, J.; Richter, R.; Avaldi, L. Insights into 2- and 4(5)-Nitroimidazole Decomposition into Relevant Ions and Molecules Induced by VUV Ionization. J. Phys. Chem. A 2018, 122, 4031–4041. [Google Scholar] [CrossRef] [PubMed]
- Marr, G.V.; West, J.B. Absolute Photoionization Cross-Section Tables for Helium, Neon, Argon, and Krypton in the VUV Spectral Regions. At. Data Nucl. Data Tables 1976, 18, 497–508. [Google Scholar] [CrossRef]
- Parr, R.G.; Yang, W. Density-Functional Theory of Atoms and Molecule; Oxford University Press: Oxford, UK, 1995. [Google Scholar]
- Bao, J.L.; Truhlar, D.G. Variational Transition State Theory: Theoretical Framework and Recent Developments. Chem. Soc. Rev. 2017, 46, 7548–7596. [Google Scholar] [CrossRef]
- Peterson, K.A.; Shepler, B.C.; Figgen, D.; Stoll, H. On the spectroscopic and thermochemical properties of ClO, BrO, IO, and their anions. J. Phys. Chem. A 2006, 110, 13877. [Google Scholar] [CrossRef]
- Available online: http://www.uni-stuttgart.de/ (accessed on 19 June 2022).
- Raghavachary, K.; Trucks, G.W.; Pople, J.A.; Head-Gordon, M. A fifth-order perturbation comparison of electron correlation theories. Chem. Phys. Lett. 1989, 157, 479. [Google Scholar] [CrossRef]
- Bauernschmitt, R.; Häser, M.; Treutler, O.; Ahlrichs, R. Calculation of excitation energies within time-dependent density functional theory using auxiliary basis set expansions. Chem. Phys. Lett. 1997, 264, 573. [Google Scholar] [CrossRef]
- Su, T.; Chesnavich, W.J. Parametrization of the ion–polar molecule collision rate constant by trajectory calculations. J. Chem. Phys. 1982, 76, 5183–5185. [Google Scholar] [CrossRef]
- Viggiano, A.A.; Morris, R.A.; Paschkewitz, J.S.; Paulson, J.F. Kinetics of the gas-phase reactions of chloride anion, Cl-with CH3Br and CD3Br: Experimental evidence for nonstatistical behavior? J. Am. Chem. Soc. 1992, 114, 10477–10482. [Google Scholar] [CrossRef]
- Liu, A.J.; Anderson, S.L. Dynamical control of ‘statistical’ ion–molecule reactions. Int. J. Mass Spectrom. 2005, 241, 173–184. [Google Scholar] [CrossRef]
- Satta, M.; Catone, D.; Castrovilli, M.C.; Bolognesi, P.; Avaldi, L.; Zema, N.; Cartoni, A. Ion Chemistry of Carbon Dioxide in Nonthermal Reaction with Molecular Hydrogen. J. Phys. Chem. A 2022, 126, 3463–3471. [Google Scholar] [CrossRef] [PubMed]
- Cartoni, A.; Catone, D.; Bolognesi, P.; Satta, M.; Markus, P.; Avaldi, L. HSO2+ Formation from Ion-Molecule Reactions of SO2⋅+ with Water and Methane: Two Fast Reactions with Reverse Temperature-Dependent Kinetic Trend. Chem. Eur. J. 2017, 23, 6772–6780. [Google Scholar] [CrossRef] [PubMed]
- Satta, M.; Cartoni, A.; Catone, D.; Castrovilli, M.C.; Bolognesi, P.; Zema, N.; Avaldi, L. The Reaction of Sulfur Dioxide Radical Cation with Hydrogen and its Relevance in Solar Geoengineering Models. Chem. Phys. Chem. 2020, 21, 1146–1156. [Google Scholar] [CrossRef]
- Anicich, V.G. An Index of the Literature for Bimolecular Gas Phase Cation-Molecule Reaction Kinetics; JPL Publication 03-19: Pasadena, CA, USA, 2003. [Google Scholar]
- Catone, D.; Satta, M.; Cartoni, A.; Castrovilli, M.C.; Bolognesi, P.; Turchini, S.; Avaldi, L. Gas Phase Oxidation of Carbon Monoxide by Sulfur Dioxide Radical Cation: Reaction Dynamics and Kinetic Trend with the Temperature. Front. Chem. 2019, 7, 140. [Google Scholar] [CrossRef] [Green Version]
- Schwarz, H.; Shaik, S.; Li, J. Electronic effects on room temperature, gas-phase C–H bond activations by cluster oxides and metal carbides: The methane challenge. J. Am. Chem. Soc. 2017, 139, 17201–17212. [Google Scholar] [CrossRef] [PubMed]
- Linstrom, P.J.; Mallard, W.G. NIST Chemistry WebBook, NIST Standard Reference Database. Gaithersburg, MD: National Institute of Standards and Technology, 20899. 2005. Available online: http://webbook.nist.gov (accessed on 19 June 2022).
- Crabtree, R.H. C–H bond activation: A radical non-metal solution. Nat. Chem. 2009, 1, 348–349. [Google Scholar] [CrossRef] [PubMed]
- Speidel, M.; Nau, R.; Arnold, F.; Schlager, H.; Stohl, A. Sulfur dioxide measurements in the lower, middle and upper troposphere: Deployment of an aircraft-based chemical ionization mass spectrometer with permanent in-flight calibration. Atmos. Environ. 2007, 41, 2427–2437. [Google Scholar] [CrossRef] [Green Version]
- Cacace, F.; de Petris, G. Mass spectrometric study of simple main group molecules and ions important in atmospheric processes. Int. J. Mass Spectrom. 2000, 194, 1–10. [Google Scholar] [CrossRef]
- Lias, S.G.; Bartmess, J.E.; Liebman, J.F.; Holmes, J.L.; Levin, R.D.; Mallard, W.G. Gas-Phase Ion and Neutral Thermochemistry. J. Phys. Chem. Ref. Data 1988, 17, 872. [Google Scholar]
- Fehsenfeld, F.C.; Ferguson, E.E. Atmospheric atomic sulfur ion reactions. J. Geophys. Res. 1973, 78, 1699–1701. [Google Scholar] [CrossRef]
- Truhlar, D.G.; Garrett, B.C. Variational transition state theory. Annu. Rev. Phys. Chem. 1984, 35, 159–189.1146. [Google Scholar] [CrossRef]
- Bowers, M.T. Gas Phase Ion Chemistry; Accademic Press: New York, NY, USA, 1979; Volume 1. [Google Scholar]
- Carpenter, L.J.; Nightingale, P.D. Chemistry and release of gases from the surface ocean. Chem. Rev. 2015, 115, 4015–4034. [Google Scholar] [CrossRef] [PubMed]
- Catone, D.; Satta, M.; Castrovilli, M.C.; Bolognesi, P.; Avaldi, L.; Cartoni, A. Photoionization of Methanol: A Molecular Source for The Prebiotic Chemistry. Chem. Phys. Lett. 2021, 771, 138467. [Google Scholar] [CrossRef]
- Sandford, A.; Nuevo, M.; Bera, P.P.; Lee, T.J. Prebiotic Astrochemistry and the Formation of Molecules of Astrobiological Interest in Interstellar Clouds and Protostellar Disks. Chem. Rev. 2020, 120, 4616–4659. [Google Scholar] [CrossRef] [PubMed]
- Herbst, E. The Synthesis of Large Interstellar Molecules. Int, Rev. Phys. Chem. 2017, 36, 287–331. [Google Scholar] [CrossRef]
- Bouma, W.J.; Nobes, R.H.; Radom, L. Methylenoxonium Radical Cation CH2OH2 +: A Surprisingly Stable Isomer of the Methanol Radical Cation. J. Am. Chem. Soc. 1982, 104, 2929–2930. [Google Scholar] [CrossRef]
- Ma, N.L.; Smith, B.J.; Pople, J.A.; Radom, L. Rearrangement and Dissociative Reactions of the Methanol Radical Cation (CH3OH.+): A Comparison of Theory and Experiment. J. Am. Chem. Soc. 1991, 113, 7903–7912. [Google Scholar] [CrossRef]
- Wagner, J.P.; Bartlett, M.A.; Allen, W.D.; Duncan, M.A. Tunneling Isomerizations on the Potential Energy Surfaces of Formaldehyde and, Methanol Radical Cations. ACS Earth Space Chem. 2017, 1, 361–367. [Google Scholar] [CrossRef]
- Ruscic, B.; Berkowitz, J. Heat of formation of hydroxymethyl and methanol D0(H CH2OH). J. Phys. Chem. 1993, 97, 11451–11455. [Google Scholar] [CrossRef]
- Olah, G.A.; Mathew, T.; Prakash, G.K.S.; Rasul, G. Chemical Aspects of Astrophysically Observed Extraterrestrial Methanol, Hydrocarbon Derivatives, and Ions. J. Am. Chem. Soc. 2016, 138, 1717–1722. [Google Scholar] [CrossRef]
- Blanksby, S.J.; Ellison, G.B. Dissociation Energies of Organic Molecules. Acc. Chem. Res. 2003, 36, 255–263. [Google Scholar] [CrossRef] [PubMed]
- Borkar, S.; Sztaraya, B.; Bodi, A. Bond Dissociative photoionization mechanism of methanol isotopologues (CH3OH, CD3OH, CH3OD and CD3OD) by iPEPICO: Energetics, statistical and non-statistical kinetics and isotope effects. Phys. Chem. Chem. Phys. 2011, 13, 13009–13020. [Google Scholar] [CrossRef] [PubMed]
- Okada, S.; Matsumoto, A.; Dohmaru, T.; Taniguchi, S.; Hayakawa, T. Ion-molecule reactions in acetaldehyde and methanol. Mass Spectrom. 1972, 20, 311–319. [Google Scholar] [CrossRef]
- Herbst, E. Journal Proceedings of the NASA LAW 2006. In Proceedings of the 2006 NASA Workshop on Laboratory Astrophysics, Las Vegas, NV, USA, 14–16 February 2006. [Google Scholar]
- Rowland, F.S.; Molina, M.J. Chlorofluoromethanes in the environment. Rev. Geophys. 1975, 13, 1–35. [Google Scholar] [CrossRef]
- Mössinger, J.C.; Shallcross, D.E.; Cox, R.A. UV–VIS absorption cross-sections and atmospheric lifetimes of CH2Br2, CH2I2 and CH2BrI. J. Chem. Soc., Faraday Trans. 1998, 94, 1391–1396. [Google Scholar] [CrossRef]
- O’ Dowd, C.D.; Jimenez, J.L.; Bahreini, R.; Flagan, R.C.; Seinfeld, J.H.; Hämeri, K.; Pirjola, L.; Kulmala, M.; Jennings, S.G.; Hoffmann, T. Marine aerosol formation from biogenic iodine emissions. Nature 2002, 417, 632. [Google Scholar] [CrossRef]
- Saiz-Lopez, A.; Plane, J.M.C.; Baker, A.R.; Carpenter, L.J.; von Glasow, R.; Gómez Martín, J.C.; McFiggans, G.; Saunders, R.W. Atmospheric chemistry of iodine. Chem. Rev. 2012, 112, 1773. [Google Scholar] [CrossRef]
- Tegtmeier, S.; Krüger, K.; Quack, B.; Atlas, E.L.; Pisso, I.; Stohl, A.; Yang, X. Emission and transport of bromocarbons: From the West Pacific ocean into the stratosphere. Atmos. Chem. Phys. 2012, 12, 10633. [Google Scholar] [CrossRef] [Green Version]
- Liu, Z.H.; Wang, Y.Q.; Ma, J.J.; Wang, L.; He, G.Z. Concerted elimination of CH2I2 and CH2ICl under intense femtosecond laser excitation. Chem. Phys. Lett. 2004, 383, 198. [Google Scholar] [CrossRef]
- Alcantara, K.F.; Wolff, W.; Gomes, A.H.A.; Sigaud, L.; Soriano, S.; Oliveira, V.; Rocha, A.B.; Santos, A.C.F. Fragmentation of the CH2Cl2 molecule by proton impact and VUV photons. J. Phys. B At., Mol. Opt. Phys. 2011, 44, 165205. [Google Scholar] [CrossRef]
- Lago, A.F.; Kercher, J.P.; Bödi, A.; Sztáray, B.; Miller, B.; Wurzelmann, D.; Baer, T. Dissociative Photoionization and Thermochemistry of Dihalomethane Compounds Studied by Threshold Photoelectron Photoion Coincidence Spectroscopy. J. Phys. Chem. A 2005, 109, 1802. [Google Scholar] [CrossRef] [PubMed]
- Harvey, J.; Tuckett, R.P.; Bodi, A. A halomethane thermochemical network from iPEPICO experiments and quantum chemical calculations. J. Phys. Chem. A 2012, 116, 9696. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cartoni, A.; Bolognesi, P.; Fainelli, E.; Avaldi, L. Photofragmentation Spectra of Halogenated Methanes in The VUV Photon Energy Range, J. Chem. Phys. 2014, 140, 184307. [Google Scholar] [CrossRef] [PubMed]
- Satta, M.; Bolognesi, P.; Cartoni, A.; Casavola, A.R.; Catone, D.; Markus, P.; Avaldi, L. A joint theoretical an experimental study on diiodomethane: Ions and neutrals in the gas phase J. Chem. Phys. 2015, 143, 244312. [Google Scholar] [CrossRef] [PubMed]
- Cartoni, A.; Casavola, A.R.; Bolognesi, P.; Borocci, S.; Avaldi, L. VUV Photofragmentation of CH2I2: The [CH2I-I].+ Iso-diiodomethane Intermediate in the I-loss Channel from [CH2I2].+. J. Phys. Chem. A. 2015, 119, 3704. [Google Scholar] [CrossRef]
- Rebholz, M.; Thomas, D.; Despré, V.; Aufleger, L.; Hartmann, M.; Meyer, K.; Stooß, V.; Magunia, A.; Wachs, D.; Birk, P.; et al. All-XUV Pump-Probe Transient Absorption Spectroscopy of the Structural Molecular Dynamics of Di-iodomethane. Phys. Rev. X 2021, 11, 031001. [Google Scholar] [CrossRef]
- Krausz, F.; Ivanov, M. Attosecond physics. Rev. Mod. Phys. 2009, 81, 163–234. [Google Scholar] [CrossRef] [Green Version]
- Gallmann, L.; Cirelli, C.; Keller, U. Attosecond dynamics. Ann. Rev. Phys. Chem. 2012, 63, 447–469. [Google Scholar] [CrossRef] [Green Version]
- Miller, R.J.D. Femtosecond crystallography with ultrabright electrons and X-rays: Capturing chemistry in action. Science 2014, 343, 1108–1116. [Google Scholar] [CrossRef]
- Fang, L.; Osipov, T.; Murphy, B.F.; Rudenko, A.; Rolles, D.; Petrovic, V.S.; Bostedt, C.; Bozek, J.D.; Bucksbaum, P.H.; Berrah, N. Probing ultrafast electronic and molecular dynamics with free-electron lasers. J. Phys. B At. Mol. Opt. Phys. 2014, 47, 124006. [Google Scholar] [CrossRef] [Green Version]
- Barty, A.; Küpper, J.; Chapman, H.N. Molecular imaging using X-ray free-electron lasers. Annu. Rev. Phys. Chem. 2013, 64, 415–435. [Google Scholar] [CrossRef] [PubMed]
- Feldhaus, J.; Krikunova, M.; Meyer, M.; Möller, T.; Moshammer, R.; Rudenko, A.; Tschentscher, T.; Ullrich, J. AMO science at the FLASH and European XFEL free-electron laser facilities. J. Phys. B At. Mol. Opt. Phys. 2013, 46, 164002. [Google Scholar] [CrossRef]
- Wolf, A.; Pedersen, H.B.; Lammich, L.; Jordon-Thaden, B.; Altevogt, S.; Domesle, C.; Hergenhahn, U.; Förstel, M.; Heber, O. Soft-x-ray fragmentation studies of molecular ions. J. Phys. B At. Mol. Opt. Phys. 2010, 43, 194007. [Google Scholar] [CrossRef]
- Feldhaus, J. FLASH—the first soft x-ray free electron laser (FEL) user facility. J. Phys. B: At. Mol. Opt. Phys. 2010, 43, 194002. [Google Scholar] [CrossRef]
- Emma, P.; Akre, R.; Arthur, J.; Bionta, R.; Bostedt, C.; Bozek, J.; Brachmann, A.; Bucksbaum, P.; Coffee, R.; Decker, F.-J.; et al. First lasing and operation of an angstrom-wavelength free electron laser. Nat. Photonics 2010, 4, 641–647. [Google Scholar] [CrossRef]
- Allaria, E.; Appio, R.; Badano, L.; Barletta, W.A.; Bassanese, S.; Biedron, S.G.; Borga, A.; Busetto, E.; Castronovo, D.; Cinquegrana, P.; et al. Highly coherent and stable pulses from the FERMI seeded free-electron laser in the extreme ultraviolet. Nat. Photonics 2012, 6, 699–704. [Google Scholar] [CrossRef]
- Yabashi, M.; Tanaka, H.; Tanaka, T.; Tomizawa, H.; Togashi, T.; Nagasono, M.; Ishikawa, T.; Harries, J.R.; Hikosaka, Y.; Hishikawa, A.; et al. Compact XFEL and AMO sciences: SACLA and SCSS. J. Phys. B At. Mol. Opt. Phys. 2013, 46, 164001. [Google Scholar] [CrossRef]
- Grguraš, I.; Maier, A.R.; Behrens, C.; Mazza, T.; Kelly, T.J.; Radcliffe, P.; Düsterer, S.; Kazansky, A.K.; Kabachnik, N.M.; Tschentscher, T.; et al. Ultrafast X-ray pulse characterization at free-electron lasers. Nat. Photonics 2012, 6, 852–857. [Google Scholar] [CrossRef]
- Bolognesi, P.; Castrovilli, M.C.; O’Keeffe, P.; Casavola, A.R.; Catone, D.; Turchini, S.; Avaldi, L. Photofragmentation of organic molecules of biological interest: The pyrimidine and 2Br-pyrimidine cases. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. At. 2012, 279, 118–123. [Google Scholar] [CrossRef]
- Bolognesi, P.; Carravetta, V.; Sementa, L.; Barcaro, G.; Monti, S.; Mishra, P.M.; Cartoni, A.; Castrovilli, M.C.; Chiarinelli, J.; Tosic, S.; et al. Core shell investigation of 2-nitroimidazole. Front. Chem. 2019, 7, 151. [Google Scholar]
- Castrovilli, M.C.; Bolognesi, P.; Casavola, A.; Cartoni, A.; Catone, D.; O’Keeffe, P.; Avaldi, L. Insights into 2-Chloropyrimidine fragmentation through a thermochemical analysis of the ionic fragments. Eur. Phys. J. D 2014, 68, 253. [Google Scholar] [CrossRef]
- Chiarinelli, J.; Casavola, A.R.; Castrovilli, M.C.; Bolognesi, P.; Cartoni, A.; Wang, F.; Richter, R.; Catone, D.; Tosic, S.; Bratislav, P.; et al. Radiation Damage Mechanisms of Chemotherapeutically Active Nitroimidazole Derived Compounds. Front. Chem. 2019, 7, 329. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zettergren, H.; Domaracka, A.; Schlathölter, T.; Bolognesi, P.; Diaz-Tendero, S.; Labuda, M.; Tosic, S.; Maclot, S.; Johnsson, P.; Steber, A.; et al. Roadmap on dynamics of molecules and clusters in the gas phase. Eur. Phys. J. D 2021, 75, 152. [Google Scholar] [CrossRef]
- Chiarinelli, J.; Bolognesi, P.; Domaracka, A.; Rousseau, P.; Castrovilli, M.C.; Richter, R.; Chatterjee, S.; Wang, F.; Avaldi, L. Insights into the dissociative ionization of glycine by PEPICO experiments. Phys. Chem. Chem. Phys 2018, 20, 22841–22848. [Google Scholar] [CrossRef] [PubMed]
- Månsson, E.P.; De Camillis, S.; Castrovilli, M.C.; Galli, M.; Nisoli, M.; Calegari, F.; Greenwood, J.B. Ultrafast dynamics in the DNA building blocks thymidine and thymine initiated by ionizing radiation. Phys.Chem.Chem.Phys. 2017, 19, 19815. [Google Scholar] [CrossRef] [Green Version]
- Liu, Q.; Seiffert, L.; Trabattoni, A.; Castrovilli, M.C.; Galli, M.; Rupp, P.; Frassetto, F.; Poletto, L.; Nisoli, M.; Rühl, E.; et al. Attosecond streaking metrology with isolated nanotargets. J. Opt. 2018, 20, 024002. [Google Scholar] [CrossRef] [Green Version]
- Castrovilli, M.C.; Trabattoni, A.; Bolognesi, P.; O’Keeffe, P.; Avaldi, L.; Nisoli, M.; Calegari, F.; Cireasa, R. Ultrafast Hydrogen Migration in Photoionized Glycine. J. Phys. Chem. Lett. 2018, 9, 6012–6016. [Google Scholar] [CrossRef] [Green Version]
- Månsson, E.P.; Latini, S.; Covito, F.; Wanie, V.; Galli, M.; Perfetto, E.; Stefanucci, G.; Hübener, H.; De Giovannini, U.; Castrovilli, M.C.; et al. Real-time observation of a correlation-driven sub 3 fs charge migration in ionised adenine. Commun. Chem. 2021, 4, 73. [Google Scholar] [CrossRef]
- Ueda, K.; Sokell, E.; Schippers, S.; Aumayr, F.; Sadeghpour, H.; Burgdörfer, J.; Lemell, C.; Tong, X.-M.; Pfeifer, T.; Calegari, F.; et al. Roadmap on photonic, electronic and atomic collision physics: I. Light–matter interaction. J. Phys. B: At. Mol. Opt. Phys. 2019, 52, 171001. [Google Scholar] [CrossRef]
- Leone, S.R.; McCurdy, C.W.; Burgdörfer, J.; Cederbaum, L.S.; Chang, Z.; Dudovich, N.; Feist, J.; Greene, C.H.; Ivanov, M.; Kienberger, R.; et al. What will it take to observe processes in ‘real time’? Nat. Photon. 2014, 8, 162. [Google Scholar] [CrossRef]
- Vager, Z.; Naaman, R.; Kanter, E.P. Coulomb explosion imaging of small molecules. Science 1989, 244, 426–431. [Google Scholar] [CrossRef] [PubMed]
- Ullrich, J.; Moshammer, R.; Dorn, A.; Dörner, R.; Schmidt, L.P.H.; Schmidt-Böcking, H. Recoil-ion and electron momentum spectroscopy: Reaction-microscopes. Rep. Prog. Phys. 2003, 66, 1463–1545. [Google Scholar] [CrossRef]
- Kurka, M.; Rudenko, A.; Foucar, L.; Kuhnel, K.U.; Jiang, Y.H.; Ergler, T.; Havermeier, T.; Smolarski, M.; Schossler, S.; Cole, K.; et al. Two-photon double ionization of Ne by free-electron laser radiation: A kinematically complete experiment. J. Phys. B At. Mol. Opt. Phys. 2009, 42, 141002. [Google Scholar] [CrossRef]
- Domesle, C.; Dziarzhytski, S.; Guerassimova, N.; Harbo, L.S.; Heber, O.; Lammich, L.; Jordon-Thaden, B.; Treusch, R.; Wolf, A.; Pedersen, H.B. Photoionization and fragmentation of H3O+ under XUV irradiation. Phys. Rev. A 2013, 88, 043405. [Google Scholar] [CrossRef] [Green Version]
- Takanashi, T.; Golubev, N.V.; Callegari, C.; Fukuzawa, H.; Motomura, K.; Iablonskyi, D.; Kumagai, Y.; Mondal, S.; Tachibana, T.; Nagaya, K.; et al. Time-resolved measurement of interatomic Coulombic decay induced by two-photon double excitation of Ne2. Phys. Rev. Lett. 2017, 118, 033202. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Iablonskyi, D.; Nagaya, K.; Fukuzawa, H.; Motomura, K.; Kumagai, Y.; Mondal, S.; Tachibana, T.; Takanashi, T.; Nishiyama, T.; Matsunami, K.; et al. Slow Interatomic Coulombic Decay of Multiply Excited Ne Clusters. Phys. Rev. Lett. 2016, 117, 276806. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Schnorr, K.; Senftleben, A.; Kurka, M.; Rudenko, A.; Foucar, L.; Schmid, G.; Broska, A.; Pfeifer, T.; Meyer, K.; Anielski, D.; et al. Time-resolved measurement of interatomic coulombic decay in Ne2. Phys. Rev. Lett. 2013, 111, 093402. [Google Scholar] [CrossRef] [Green Version]
- Schnorr, K.; Senftleben, A.; Kurka, M.; Rudenko, A.; Schmid, G.; Pfeifer, T.; Meyer, K.; Kübel, M.; Kling, M.F.; Jiang, Y.H.; et al. Electron rearrangement dynamics in dissociating I2n+ molecules accessed by extreme ultraviolet pump-probe experiments. Phys. Rev. Lett. 2014, 113, 073001. [Google Scholar] [CrossRef] [Green Version]
- Erk, B.; Boll, R.; Trippel, S.; Anielski, D.; Foucar, L.; Rudek, B.; Epp, S.W.; Coffee, R.; Carron, S.; Schorb, S.; et al. Imaging charge transfer in iodomethane upon x-ray photoabsorption. Science 2014, 345, 288–291. [Google Scholar] [CrossRef]
- Jiang, Y.H.; Rudenko, A.; Herrwerth, O.; Foucar, L.; Kurka, M.; Kühnel, K.U.; Lezius, M.; Kling, M.F.; van Tilborg, J.; Belkacem, A.; et al. Ultrafast extreme ultraviolet induced isomerization of acetylene cations. Phys. Rev. Lett. 2010, 105, 263002. [Google Scholar] [CrossRef]
- Küpper, J.; Stern, S.; Holmegaard, L.; Filsinger, F.; Rouzée, A.; Rudenko, A.; Johnsson, P.; Martin, A.V.; Adolph, M.; Aquila, A.; et al. X-ray diffraction from isolated and strongly aligned gas-phase molecules with a free-electron laser. Phys. Rev. Lett. 2014, 112, 083002. [Google Scholar] [CrossRef] [Green Version]
- Scherer, N.F.; Khundkar, L.R.; Bernstein, R.B.; Zewail, A.H. Real-time picosecond clocking of the collision complex in a bimolecular reaction: The birth of OH from H+CO2. J. Chem. Phys. 1987, 87, 1451. [Google Scholar] [CrossRef] [Green Version]
- Hu, M.G.; Liu, Y.; Grimes, D.D.; Lin, Y.W.; Gheorghe, A.H.; Vexiau, R.; Bouloufa-Maafa, N.; Dulieu, O.; Rosenband, T.; Ni, K.K. Direct observation of bimolecular reactions of ultracold KRb molecules. Science 2019, 366, 1111–1115. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ferrari, A.; Sala, P.R.; Fasso, A.; Ranft, J. FLUKA: A Multi-Particle Transport Code (Program Version 2005); CERN: Geneva, Switzerland, 2005. [Google Scholar]
- Engel, R.; Heck, D.; Huege, T.; Pierog, T.; Reininghaus, M.; Riehn, F.; Ulrich, R.; Unger, M.; Veberič, D. Towards A Next Generation of CORSIKA: A Framework for the Simulation of Particle Cascades in Astroparticle Physics. Comput. Softw. Big Sci. 2019, 3, 2. [Google Scholar] [CrossRef] [Green Version]
- Agostinelli, S.; Allison, J.; Allison, J.; Apostolakis, J.; Araujo, H.; Arce, P.; Asai, M.; Axen, D.; Banerjee, S.; Barrand, G.; et al. Geant4 -a simulation toolkit. Nucl. Instrum. Methods Phys. Res. A 2003, 506, 250–303. [Google Scholar] [CrossRef] [Green Version]
- Allison, J.; Amako, K.; Apostolakis, J.; Araujo, H.; Dubois, P.A.; Asai, M.; Barrand, G.; Capra, R.; Chauvie, S.; Chytracek, R.; et al. Geant4 developments and applications. IEEE Trans. Nucl. Sci. 2006, 53, 270–278. [Google Scholar] [CrossRef] [Green Version]
- Allison, J.; Amako, K.; Apostolakis, J.; Arce, P.; Asai, M.; Aso, T.; Bagli, E.; Bagulya, A.; Banerjee, S.; Barrand, G.; et al. Recent developments in Geant4. Nucl. Instrum. Methods Phys. Res. A 2016, 835, 186–225. [Google Scholar] [CrossRef]
- Velinov, P.; Buchvarova, M.; Mateev, L.; Ruder, H. Determination of electron production rates caused by cosmic ray particles in ionospheres of terrestrial planets. Adv. Space Res. 2001, 27, 1901–1908. [Google Scholar] [CrossRef]
- Velinov, P.; Mishev, A. Cosmic ray induced ionization in the atmosphere estimated with CORSIKA code simulations, C. R. Acad. Bulg. Sci. 2007, 60, 493–500. [Google Scholar]
- Desorgher, L.; Fluckiger, E.O.; Gurtner, M.; Moser, M.R.; Butikofer, R. Atmocosmics: A GEANT 4 code for computing the intercation of cosmic rays with the Earth’s atmopshere. Int. J. Mod. Phys. A 2005, 20, 6802–6804. [Google Scholar] [CrossRef]
- Usoskin, I.G.; Kovaltsov, G.A. Cosmic ray induced ionization in the atmosphere: Full modeling and practical applications. J. Geophys. Res. 2006, 111, D21206. [Google Scholar] [CrossRef] [Green Version]
- Banjac, S.; Heber, B.; Herbst, K.; Berger, L.; Burmeister, S. On-the-Fly Calculation of Absorbed and Equivalent Atmospheric Radiation Dose in A Water Phantom with the Atmospheric Radiation Interaction Simulator (AtRIS). J. Geophys. Res. Space Phys. 2019, 124, 9774–9790. [Google Scholar] [CrossRef] [Green Version]
- Kim, Y.-K.; Irikura, K.K.; Rudd, M.E.; Ali, M.A.; Stone, P.M.; Chang, J.; Coursey, J.S.; Dragoset, R.A.; Kishore, A.R.; Olsen, K.J.; et al. Electron-Impact Ionization Cross Section for Ionization and Excitation Database (Version 3.0). Available online: http://physics.nist.gov/ionxsec (accessed on 14 June 2022).
- Kim, Y.K.; Rudd, M.E. Binary-encounter-dipole model for electron-impact ionization. Phys. Rev. 1994, A50, 3954–3967. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mott, N.F. The collision between two electrons Proc. R. Soc. London. 1930, A126, 259–267. [Google Scholar]
- Vriens, L.; Mac-Daniel, E.W. Case Studies in Atomic Collision Physics; Mac-Daniel, E.W., McDowell, M.R.C., Eds.; North Holland Publishing Co.: Amsterdam, The Netherlands, 1974; Volume 1, p. 335. [Google Scholar]
- Bethe, H. Zur theorie des durchgangs schneller korpuskularstrahlen durch materie Ann. Phys. 1930, 397, 325–400. [Google Scholar]
- Kim, Y.K.; Irikura, K.K. Electron-impact ionization cross sections for polyatomic molecules, radicals, and ions AIP. Conf. Proc. 2000, 543, 220–241. [Google Scholar]
- Hwang, W.; Kim, Y.K.; Rudd, M.E. New model for electron-impact ionization cross sections of molecules. J. Chem. Phys. 1996, 104, 2956–2966. [Google Scholar] [CrossRef] [Green Version]
- Kim, Y.K.; Irikura, K.K.; Rudd, M.E.; Ali, M.A.; Stone, P.M.; Chang, J.; Coursey, J.S.; Dragoset, R.A.; Kishore, A.R.; Olsen, K.J.; et al. Electron-impact cross Sections for Ionization and Excitation Database NIST Physical Measurement Laboratory. 1997. Available online: https://www.nist.gov/pml/electron-impact-cross-sections-ionization-and-excitation-database (accessed on 19 May 2022).
- Kim, Y.K.; Hwang, W. Electron-impact ionization cross sections of atmospheric molecules J. Chem. Phys. 1997, 106, 1026–1033. [Google Scholar] [CrossRef] [Green Version]
- Bull, J.N.; Harland, P.W.; Vallance, C. Absolute Total Electron Impact Ionization Cross-Sections for Many-Atom Organic and Halocarbon Species. J. Phys. Chem. 2012, A116, 767–777. [Google Scholar] [CrossRef]
- Bull, J.N.; Lee, J.W.L.; Vallance, C. Absolute electron total ionization cross-sections: Molecular analogues of DNA and RNA nucleobase and sugar constituents. Phys. Chem. Chem. Phys. 2014, 16, 10743. [Google Scholar] [CrossRef]
- Bordage, M.C.; Bordes, J.; Edel, S.; Terrissol, M.; Franceries, X.; Bardies, M.; Lampe, N.; Incerti, S. Implementation of new physics models for low energy electrons in liquid water in Geant4-DNA. Physica Med. 2016, 32, 1833–1840. [Google Scholar] [CrossRef] [PubMed]
- Kim, Y.; Santos, J.; Parente, F. Extension of the Binary-Encounter-Dipole Model to Relativistic Incident Electrons. Phys. Rev. 2000, A62, 052710. [Google Scholar] [CrossRef] [Green Version]
- Edel, S. Modelisation du Transport des Photons et de s’Electrons Dans l’ADN Plasmide. PhD Thesis, Universite Toulouse III-Paul Sabatier, Toulouse, France, 2006. [Google Scholar]
- Rapp, D.; Englander Golden, P. Total Cross Sections for Ionization and Attachment in Gases by Electron Impact. I. Positive Ionization. J. Chem. Phys. 1965, 43, 1464. [Google Scholar] [CrossRef]
- Schram, B.I.; De Heer, F.J.; Van Der Wiel, M.J.; Kistemaker, J. Ionization cross sections for electrons (0.6-20 keV) in noble and diatomic gases. Physica 1965, 31, 94–112. [Google Scholar] [CrossRef]
- Schram, B.I.; Moustafa, H.R.; Schutten, J.; De Heer, F.J. Ionization cross sections for electrons (100-600eV) in noble and diatomic gases. Physica 1966, 32, 734–740. [Google Scholar] [CrossRef]
- Krishnakumar, E.; Srivastava, S.K. Cross sections for the production of N+2, N++ N2+2 and N2+ by electron impact on N2. J. Phys. B At. Mol. Opt. Phys. 1990, 23, 1893. [Google Scholar] [CrossRef]
- Straub, H.C.; Renault, P.; Lindsay, B.G.; Smith, K.A.; Stebbings, R.F. Absolute partial cross sections for electron-impact ionization of H2, N2, and O2 from threshold to 1000 eV. Phys. Rev. A 1996, 54, 2146–2153. [Google Scholar] [CrossRef]
- Raj, D. A note on the use of the additivity rule for electron-molecule elastic scattering. Phys. Lett. A 1991, 160, 571–574. [Google Scholar] [CrossRef]
- Mott, N.F.; Massey, H.S.W. The Theory of Atomic Collisions; Clarendon Press: Oxford, UK, 1965. [Google Scholar]
- Salvat, F.; Jablonski, A.; Powell, C.J. ELSEPA—Dirac partial-wave calculation of elastic scattering of electrons and positrons by atoms, positive ions and molecules. Comput. Phys. Commun. 2005, 165, 157–190. [Google Scholar] [CrossRef]
- Blanco, F.; García, G. A screening-corrected additivity rule for the calculation of electron scattering from macro-molecules. J. Phys. B At. Mol. Opt. Phys. 2009, 42, 145203. [Google Scholar] [CrossRef]
- Blanco, F.; García, G. Screening corrections for calculation of electron scattering differential cross sections from polyatomic molecules. Phys. Lett. A 2004, 330, 230–237. [Google Scholar] [CrossRef]
- Limao-Vieira, P.; Blanco, F.; Oller, J.C.; Munoz, A.; Pèrez, J.M.; Vinodkumar, M.; Garcìa, G.; Mason, N. Electron scattering cross sections for SF6 and SF5CF3 at intermediate and high energies 100–10,000 eV. J. Phys. Rev. A 2005, 71, 032720. [Google Scholar] [CrossRef] [Green Version]
- Limao-Vieira, P.; Horie, M.H.; Kato Hoshino, M.; Blanco, F.; Garcìa, G.; Buckman, S.J.; Tanaka, H. Differential elastic electron scattering cross sections for CCl4 by 1.5–100 eV energy electron impact. J. Chem. Phys. 2011, 135, 234309. [Google Scholar] [CrossRef] [Green Version]
- Kato, H.; Suga, A.; Hoshino, M.; Blanco, F.; Garcìa, G.; Limao-Vieira, P.; Brunger, M.J.; Tanaka, H. Elastic cross sections for electron scattering from GeF4: Predominance of atomic-F in the high-energy collision dynamics. J. Chem. Phys. 2012, 136, 13. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Khandker, M.H.; Arony, N.T.; Haque, A.K.F.; Maaza, M.; Billah, M.M.; Uddin, M.A. Scattering of e± from N2 in the energy range 1eV–10keV. Mol. Phys. 2019, 118, 1699183. [Google Scholar] [CrossRef]
- Raj, D.; Kumar, A. Elastic scattering of electrons by molecular oxygen. Phys. Lett. A 2001, 282, 284–287. [Google Scholar] [CrossRef]
- Shyn, T.W.; Sharp, W.E. Angular distribution of electrons elastic scattered from O2: 2.0-200 eV impact energy. Phys. Rev. A 1982, 26, 3. [Google Scholar] [CrossRef]
- Vukalović, J.; Maljković, J.B.; Tökési, K.; Predojević, B.; Marinković, B.P. Elastic Electron Scattering from Methane Molecule in the Energy Range from 50–300 eV. Int. J. Mol. Sci. 2021, 22, 647. [Google Scholar] [CrossRef]
- Billah, M.M.; Billah, M.M.; Khatun, M.M.; Haque, M.M.; Ali, M.Y.; Khandker, M.H.; Haque, A.K.F.; Watabe, H.; Uddin, M.A. A Theoretical Study of Scattering of Electrons and Positrons by CO2 Molecule. Atoms 2022, 10, 31. [Google Scholar] [CrossRef]
- Gote, M.; Ehrhardt, H. Rotational excitation of diatomic molecules at intermediate energy: Absolute differential state-to-state transition cross sections for electron scattering from N2, Cl2, CO and HCl. J. Phys. B At. Mol. Opt. Phys. 1995, 28, 3957. [Google Scholar] [CrossRef]
- Shyn, T.W.; Carignan, G.R. Angular Nstribution of electrons elastically scattered from gases: 1.5-400 eV on N2. II. Phy. Rev. A 1980, 22, 923–929. [Google Scholar] [CrossRef]
- Nickel, J.C.; Mott, C.; Kanik, I.; McCollum, D.C. Absolute elastic differential electron scattering cross sections for carbon monoxide and molecular nitrogen in the intermediate energy region. J. Phys. B At. Mol. and Opt. Phys. 1988, 21, 1867–1877. [Google Scholar] [CrossRef]
- Srivastava, S.K. Absolute elastic differential electron scattering cross sections in the intermediate energy region. J. Chem. Phys. 1976, 64, 1340. [Google Scholar] [CrossRef]
- Jansen, R.H.J.; De Heer, F.J.; Luyken, H.J.; Van Wingerden, B.; Blaauw, H.J. Absolute differential cross sections for elastic scattering of electrons by helium, neon, argon and molecular nitrogen. J. Phys. B: Atom. Mol. Phys. 1976, 9, 185. [Google Scholar] [CrossRef]
- Kim, Y.-K. Scaling of plane-wave Born cross sections for electron-impact excitation of neutral atoms. Phys. Rev. A 2001, 64, 032713. [Google Scholar] [CrossRef]
- Kim, Y.-K. Scaled Born cross sections for excitations of H2 by electron impact. J. Chem. Phys. 2007, 126, 064305. [Google Scholar] [CrossRef]
- Suzuki, D.; Kato, H.; Ohkawa, M.; Anzai, K.; Tanaka, H.; Limão-Vieira, P.; Brunger, M.J.J. Electron excitation of the Schumann–Runge continuum, longest band, and second band electronic states in O2. Chem. Phys. 2011, 134, 064311. [Google Scholar] [CrossRef]
- Tanaka, H.; Brunger, M.J.; Campbell, L.; Kato, H.; Hoshino, M.; Rau, A.R.P. Scaled plane-wave Born cross sections for atoms and molecules. Rev. Mod. Phys. 2016, 88, 025004. [Google Scholar] [CrossRef]
- Bartschat, K.; Tennyson, J.; Zatsarinny, O. Quantum-Mechanical Calculations of Cross Sections for Electron Collisions with Atoms and Molecules. Plasma Processes. Polym. 2016, 14, 1600093. [Google Scholar] [CrossRef] [Green Version]
- Isaacs, W.A.; McCurdy, C.W.; Rescigno, T.N. Theoretical support for a Ramsauer-Townsend minimum in electron-CF4 scattering. Phys. Rev. A 1998, 58, 309. [Google Scholar] [CrossRef]
- Winstead, C.; McKoy, V.; Sun, Q. Low-energy elastic electron scattering by tetrafluoromethane (CF4). J. Chem. Phys. 1993, 98, 1105–1109. [Google Scholar] [CrossRef] [Green Version]
- Tennyson, J. Electron–molecule collision calculations using the -matrix method. Phys. Rep. 2010, 491, 29–76. [Google Scholar] [CrossRef]
- Burke, P.G.; Noble, C.J.; Scott, P. R-matrix theory of electron scattering at intermediate energies. A. Math. Phys. Sci. 1987, 410, 289–310. [Google Scholar]
- Mašín, Z.; Benda, J.; Gorfinkiel, J.D.; Harvey, A.G.; Tennyson, J. A suite for modeling electronic processes in molecules interacting with electrons, positrons and photons using the R-matrix method. Comput. Phys. Commun. 2019, 249, 107092. [Google Scholar] [CrossRef] [Green Version]
- Carr, J.M.; Galiatsatos, P.; Gorfinkiel, J.; Harvey, A.; Lysaght, M.; Madden, D.; Masın, Z.; Plummer, M.; Tennyson, J.; Varambhia, H. UKRmol: A low-energy electron- and positron-molecule scattering suite. Eur. Phys. J. D 2012, 66, 58–69. [Google Scholar] [CrossRef]
- Tashiro, M.; Morokuma, K.; Tennyson, J. R-matrix calculation of differential cross sections for low-energy electron collisions with ground-state and electronically excited-state O2 molecules. Phy. Rev. A: At. Mol. Opt. Phys. 2006, 74, 022706. [Google Scholar] [CrossRef] [Green Version]
- Tashiro, M.; Morokuma, K.; Tennyson, J. R-matrix calculation of electron collisions with electronically excited O2 molecules. Phy. Rev. A At. Mol. Opt. Phys. 2006, 73, 052707. [Google Scholar] [CrossRef] [Green Version]
- Su, H.; Cheng, X.; Zhang, H.; Tennyson, J. Electron collisions with molecular nitrogen in its ground and electronically excited states using the R-matrix method. J. Phys. B At. Mol. Opt. Phys. 2021, 54, 115203. [Google Scholar] [CrossRef]
- Meltzer, T.; Tennyson, J.; Mašín, Z.; Zammit, M.C.; Scarlett, L.H.; Fursa, D.V.; Bray, I. Benchmark calculations of electron impact electronic excitation of the hydrogen molecule. J. Phys. B At. Mol. Opt. Phys. 2020, 53, 145204. [Google Scholar] [CrossRef] [Green Version]
- Incerti, S.; Kyriakou, I.; Bernal, M.A.; Bordage, M.C.; Francis, Z.; Guatelli, S.; Ivanchenko, V.; Karamitros, M.; Lampe, N.; Lee, S.B.; et al. BrownGeant4-DNA example applications for track structure simulations in liquid water: A report from the Geant4-DNA Project. Med. Phys. 2018, 45, e722–e739. [Google Scholar] [CrossRef] [Green Version]
- Bernal, M.A.; Bordage, M.C.; Brown, J.M.C.; Davídková, M.; Delage, E.; El Bitar, Z.; Enger, S.A.; Francis, Z.; Guatelli, S.; Ivanchenko, V.N.; et al. Track structure modeling in liquid water: A review of the Geant4-DNA very low energy extension of the Geant4 Monte Carlo simulation toolkit. Phys. Med. 2015, 31, 861–874. [Google Scholar] [CrossRef] [PubMed]
- Incerti, S.; Baldacchino, G.; Bernal, M.; Capra, R.; Champion, C.; Francis, Z.; Guatelli, S.; Guèye, P.; Mantero, A.; Mascialino, B.; et al. The Geant4-DNA project. Int. J. Model. Simul. Sci. Comput. 2010, 1, 157–178. [Google Scholar] [CrossRef]
- Incerti, S.; Ivanchenko, A.; Karamitros, M.; Mantero, A.; Moretto, P.; Tran, H.N.; Mascialino, B.; Champion, C.; Ivanchenko, V.N.; Bernal, M.A.; et al. Comparison of Geant4 very low energy cross section models with experimental data in water. Med. Phys. 2010, 37, 4692–4708. [Google Scholar] [CrossRef] [PubMed]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Satta, M.; Castrovilli, M.C.; Nicolanti, F.; Casavola, A.R.; Mancini Terracciano, C.; Cartoni, A. Perspectives of Gas Phase Ion Chemistry: Spectroscopy and Modeling. Condens. Matter 2022, 7, 46. https://doi.org/10.3390/condmat7030046
Satta M, Castrovilli MC, Nicolanti F, Casavola AR, Mancini Terracciano C, Cartoni A. Perspectives of Gas Phase Ion Chemistry: Spectroscopy and Modeling. Condensed Matter. 2022; 7(3):46. https://doi.org/10.3390/condmat7030046
Chicago/Turabian StyleSatta, Mauro, Mattea Carmen Castrovilli, Francesca Nicolanti, Anna Rita Casavola, Carlo Mancini Terracciano, and Antonella Cartoni. 2022. "Perspectives of Gas Phase Ion Chemistry: Spectroscopy and Modeling" Condensed Matter 7, no. 3: 46. https://doi.org/10.3390/condmat7030046
APA StyleSatta, M., Castrovilli, M. C., Nicolanti, F., Casavola, A. R., Mancini Terracciano, C., & Cartoni, A. (2022). Perspectives of Gas Phase Ion Chemistry: Spectroscopy and Modeling. Condensed Matter, 7(3), 46. https://doi.org/10.3390/condmat7030046





