The Shrinking Fermi Liquid Scenario for Strange-Metal Behavior from Overdamped Optical Phonons
Abstract
:1. Introduction
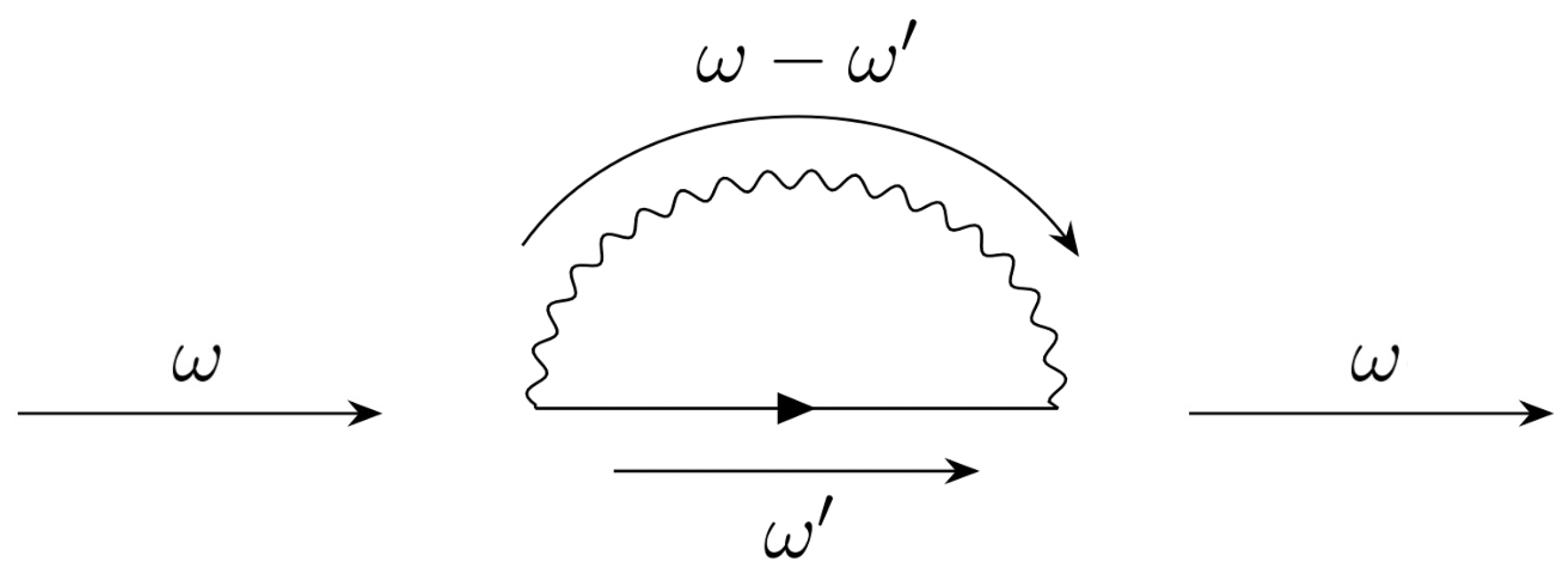
2. Self-Energy from a Simple Local Propagator
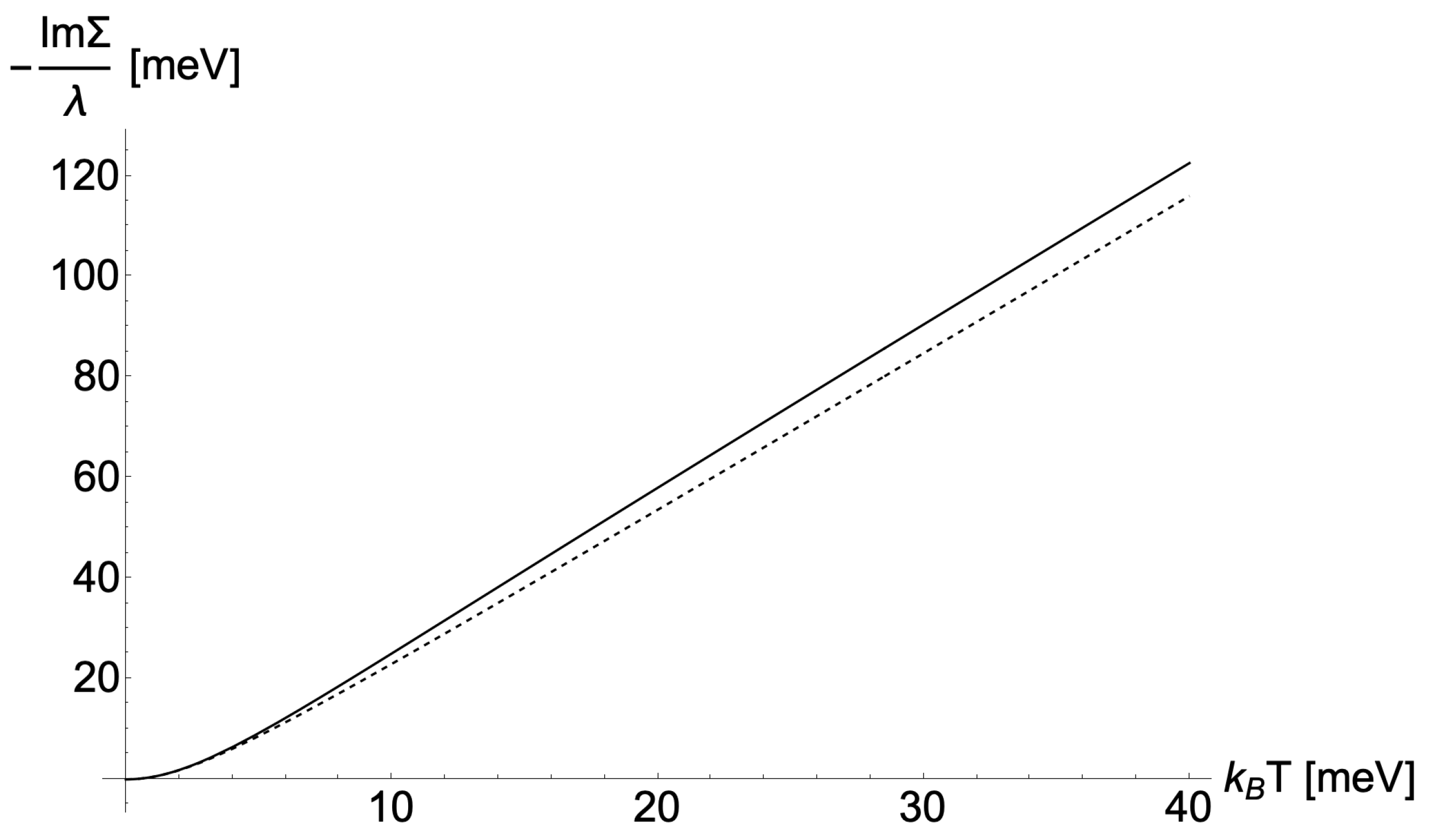
2.1. Zero Frequency
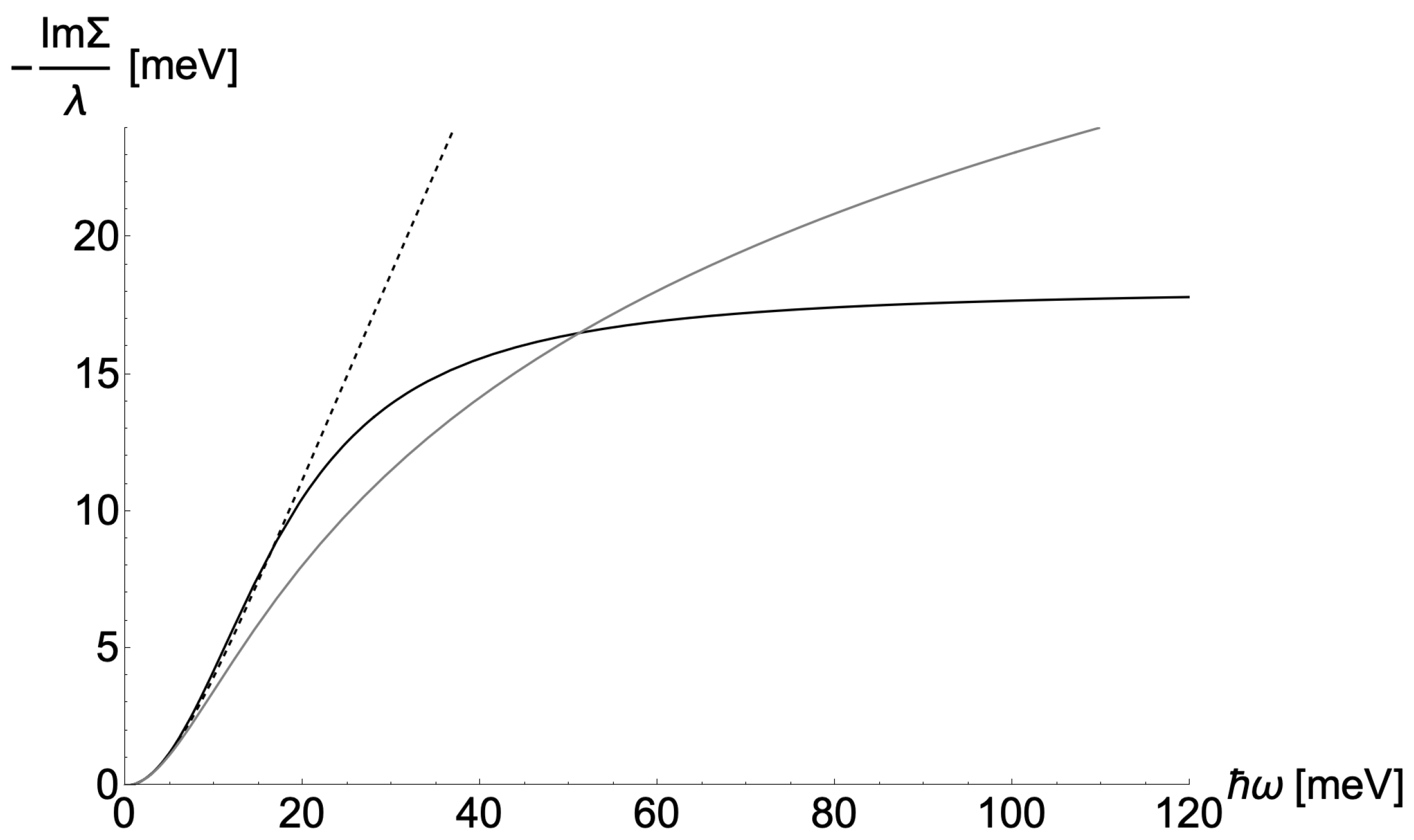
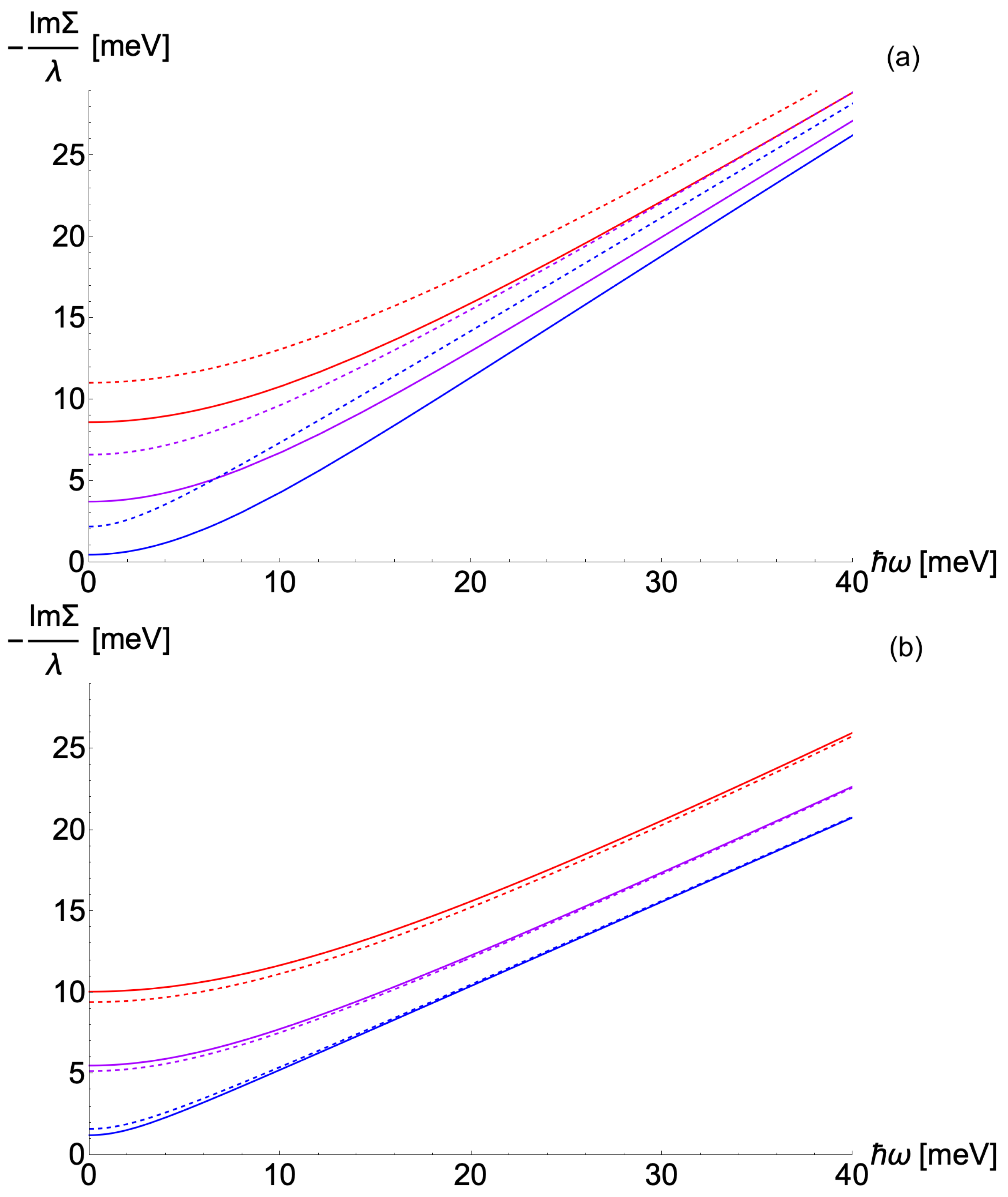
2.2. Zero Temperature
3. Discussion and Conclusions
3.1. The Main Features of the Overdamped Optical Phonon Model
3.2. Connection with the Shrinking Fermi Liquid Scenario
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| MFL | Marginal Fermi Liquid |
| CDF | Charge Density Fluctuations |
| SFL | Shrinking Fermi Liquid |
Appendix A
Appendix B
References
- Gurvitch, T.; Fiory, A.T. Resistivity of La1.825Sr0.175CuO4 and YBa2Cu3O7 to 1100 K: Absence of saturation and its implications. Phys. Rev. Lett. 1987, 59, 1337. [Google Scholar] [CrossRef]
- Hussey, N.E. Phenomenology of the normal state in-plane transport properties of high-Tc cuprates. J. Phys. Condens. Matter 2008, 20, 123201. [Google Scholar] [CrossRef]
- Proust, C.; Taillefer, L.P. The remarkable underlying ground states of cuprate superconductors. Annu. Rev. Condens. Matter Phys. 2019, 10, 409. [Google Scholar] [CrossRef]
- Varma, C.M. Colloquium: Linear in temperature resistivity and associated mysteries including high temperature superconductivity. Rev. Mod. Phys. 2020, 92, 031001. [Google Scholar] [CrossRef]
- Marel, D.V.D.; Molegraaf, H.J.A.; Zaanen, J.; Nussinov, Z.; Carbone, F.; Damascelli, A.; Eisaki, H.; Greven, M.; Kes, P.H.; Li, M. Quantum critical behaviour in a high-Tc superconductor. Nature 2003, 425, 271–274. [Google Scholar] [CrossRef]
- Michon, B.; Berthod, C.; Rischau, C.W.; Ataei, A.; Chen, L.; Komiya, S.; Ono, S.; Taillefer, L.; van der Marel, D.; Georges, A. Reconciling scaling of the optical conductivity of cuprate superconductors with Planckian resistivity and specific heat. Nat. Commun. 2023, 14, 3033. [Google Scholar] [CrossRef]
- Michon, B.; Girod, C.; Badoux, S.; Kačmarčík, J.; Ma, Q.; Dragomir, M.; Dabkowska, H.A.; Gaulin, B.D.; Zhou, J.S.; Pyon, S.; et al. Thermodynamic signatures of quantum criticality in cuprate superconductors. Nature 2019, 567, 218–222. [Google Scholar] [CrossRef] [PubMed]
- Legros, A.; Benhabib, S.; Tabis, W.; Laliberté, F.; Dion, M.; Lizaire, M.; Vignolle, B.; Vignolles, D.; Raffy, H.; Li, Z.Z.; et al. Universal T-linear resistivity and Planckian dissipation in overdoped cuprates. Nat. Phys. 2019, 15, 142. [Google Scholar] [CrossRef]
- Varma, C.M.; Littlewood, P.B.; Schmitt-Rink, S.; Abrahams, E.; Ruckenstein, A.E. Phenomenology of the normal state of Cu-O high-temperature superconductors. Phys. Rev. Lett. 1989, 63, 1996. [Google Scholar] [CrossRef] [PubMed]
- Ruckenstein, A.E.; Varma, C.M. A theory of marginal fermi-liquids. Phys. C Supercond. 1991, 134, 185–189. [Google Scholar] [CrossRef]
- Littlewood, P.B.; Varma, C.M. Phenomenology of the normal and superconducting states of a marginal Fermi liquid. J. Appl. Phys. 1991, 69, 4979–4984. [Google Scholar] [CrossRef]
- Littlewood, P.B.; Varma, C.M. Phenomenology of the superconductive state of a marginal Fermi liquid. Phys. Rev. B 1992, 46, 405. [Google Scholar] [CrossRef]
- Sire, C.; Varma, C.M.; Ruckenstein, A.E.; Giamarchi, T. Theory of the marginal-Fermi-liquid spectrum and pairing in a local copper oxide model. Phys. Rev. Lett. 1994, 72, 2478. [Google Scholar] [CrossRef]
- Shekhter, A.; Varma, C.M. Long-wavelength correlations and transport in a marginal Fermi liquid. Phys. Rev. B 2009, 79, 045117. [Google Scholar] [CrossRef]
- Littlewood, P.B.; Zaanen, J.; Aeppli, G.; Monien, H. Spin fluctuations in a two-dimensional marginal Fermi liquid. Phys. Rev. B 1993, 48, 487. [Google Scholar] [CrossRef]
- Abrahams, E.; Varma, C.M. Hall effect in the marginal Fermi liquid regime of high-Tc superconductors. Phys. Rev. B 2003, 68, 094502. [Google Scholar] [CrossRef]
- Kokalj, J.; Hussey, N.E.; McKenzie, R.H. Transport properties of the metallic state of overdosed cuprate superconductors from an Anisotropic marginal Fermi liquid model. Phys. Rev. B 2012, 86, 045132. [Google Scholar] [CrossRef]
- Hwang, J.; Timusk, T.; Puchkov, A.V.; Wann, N.L.; Gu, G.D. Marginal Fermi liquid analysis of 300 K reflectance of Bi2Sr2Cu2O8+δ. Phys. Rev. B 2004, 69, 094520. [Google Scholar] [CrossRef]
- Hlubina, R.; Rice, T.M. Resistivity as a function of temperature for models with hot spots on the Fermi surface. Phys. Rev. B 1995, 51, 9253. [Google Scholar] [CrossRef] [PubMed]
- Wen, J.-J.; Huang, H.; Lee, S.-J.; Jang, H.; Knight, J.; Lee, Y.S.; Fujita, M.; Suzuki, K.M.; Asano, S.; Kivelson, S.A.; et al. Observation of two types of charge-density-wave orders in superconducting La2−xSrxCuO4. Nat. Commun. 2019, 10, 3269. [Google Scholar] [CrossRef] [PubMed]
- Lin, J.Q.; Miao, H.; Mazzone, D.G.; Gu, G.D.; Nag, A.; Walters, A.C.; Garcia-Fernandez, M.; Barbour, A.; Pelliciari, J.; Jarrige, I.; et al. Strongly correlated charge density wave in La2−xSrxCuO4 evidenced by doping-dependent phonon anomaly. Phys. Rev. Lett. 2020, 124, 207005. [Google Scholar] [CrossRef]
- Miao, H.; Fabbris, G.; Koch, R.J.; Mazzone, D.G.; Nelson, C.S.; Acevedo-Esteves, R.; Li, Y.; Gu, G.D.; Yilmaz, T.; Kaznatcheev, K.; et al. Charge density waves in cuprate superconductors beyond the critical doping. NPJ Quantum Mater. 2021, 6, 31. [Google Scholar] [CrossRef]
- Miao, H.; Lorenzana, J.; Seibold, G.; Peng, Y.Y.; Amorese, A.; Yakhou-Harris, F.; Kummer, K.; Brookes, N.B.; Konik, R.M.; Thampy, V.; et al. High-temperature charge density wave correlations in La1.875Ba0.125CuO4 without spin-charge locking. Proc. Natl. Acad. Sci. USA 2017, 114, 12430–12435. [Google Scholar] [CrossRef]
- Miao, H.; Fumagalli, R.; Rossi, M.; Lorenzana, J.; Seibold, G.; Yakhou-Harris, F.; Kummer, K.; Brookes, N.B.; Gu, G.D.; Braicovich, L.; et al. Formation of incommensurate charge density waves in cuprates. Phys. Rev. X 2019, 9, 031042. [Google Scholar] [CrossRef]
- Wang, Q.; Horio, M.; von Arx, K.; Shen, Y.; John Mukkattukavil, D.; Sassa, Y.; Ivashko, O.; Matt, C.E.; Pyon, S.; Takayama, T.; et al. High-temperature charge-stripe correlations in La1.675Eu0.2Sr0.125CuO4. Phys. Rev. Lett. 2020, 124, 187002. [Google Scholar] [CrossRef]
- Yu, B.; Tabis, W.; Bialo, I.; Yakhou, F.; Brookes, N.B.; Anderson, Z.; Tang, Y.; Yu, G.; Greven, M. Unusual dynamic charge correlations in simple-tetragonal HgBa2CuO4+δ. Phys. Rev. X 2020, 10, 021059. [Google Scholar] [CrossRef]
- Arpaia, R.; Ghiringhelli, G. Charge Order at High Temperature in Cuprate Superconductors. J. Phys. Soc. Jpn. 2021, 90, 111005. [Google Scholar] [CrossRef]
- Castellani, C.; Castro, C.D.; Grilli, M. Singular Quasiparticle Scattering in the Proximity of Charge Instabilities. Phys. Rev. Lett. 1995, 75, 4650. [Google Scholar] [CrossRef] [PubMed]
- Andergassen, S.; Caprara, S.; Castro, C.D.; Grilli, M. Anomalous Isotopic Effect Near the Charge-Ordering Quantum Criticality. Phys. Rev. Lett. 2001, 87, 056401. [Google Scholar] [CrossRef] [PubMed]
- Arpaia, R.; Caprara, S.; Fumagalli, R.; De Vecchi, G.; Peng, Y.Y.; Andersson, E.; Betto, D.; De Luca, G.M.; Brookes, N.B.; Lombardi, F.; et al. Dynamical charge density fluctuations pervading the phase diagram of a Cu-based high-Tc superconductor. Science 2019, 365, 906–910. [Google Scholar] [CrossRef] [PubMed]
- Seibold, G.; Arpaia, R.; Peng, Y.Y.; Fumagalli, R.; Braicovich, L.; Di Castro, C.; Grilli, M.; Ghiringhelli, G.C.; Caprara, S. Strange metal behaviour from charge density fluctuations in cuprates. Commun. Phys. 2021, 4, 7. [Google Scholar] [CrossRef]
- Caprara, S.; Castro, C.D.; Mirarchi, G.; Seibold, G.; Grilli, M. Dissipation-driven strange metal behavior. Commun. Phys. 2022, 5, 10. [Google Scholar] [CrossRef]
- Mirarchi, G.; Seibold, G.; Di Castro, C.; Grilli, M.; Caprara, S. The Strange-Metal Behavior of Cuprates. Condens. Matter 2022, 7, 29. [Google Scholar] [CrossRef]
- Devereaux, T.P.; Cuk, T.; Shen, Z.-X.; Nagaosa, N. Anisotropic Electron-Phonon Interaction in the Cuprates. Phys. Rev. Lett. 2004, 93, 117004. [Google Scholar] [CrossRef]
- Mazza, G.; Grilli, M.; Castro, C.D.; Caprara, S. Evidence for phonon-like charge and spin fluctuations from an analysis of angle-resolved photoemission spectra of La2−xSrxCuO4 superconductors. Phys. Rev. B 2013, 87, 014511. [Google Scholar] [CrossRef]
- Grilli, M.; Di Castro, C.; Mirarchi, G.; Seibold, G.; Caprara, S. Dissipative Quantum Criticality as a Source of Strange Metal Behavior. Symmetry 2023, 15, 569. [Google Scholar] [CrossRef]
- Engelsberg, S.; Schrieffer, J.R. Coupled Electron-Phonon System. Phys. Rev. 1963, 131, 993. [Google Scholar] [CrossRef]
- Arpaia, R.; Martinelli, L.; Sala, M.M.; Caprara, S.; Nag, A.; Brookes, N.B.; Camisa, P.; Li, Q.; Gao, Q.; Zhou, X.; et al. Signature of quantum criticality in cuprates by charge density fluctuations. Nat. Commun. 2023, 14, 7198. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mirarchi, G.; Grilli, M.; Seibold, G.; Caprara, S. The Shrinking Fermi Liquid Scenario for Strange-Metal Behavior from Overdamped Optical Phonons. Condens. Matter 2024, 9, 14. https://doi.org/10.3390/condmat9010014
Mirarchi G, Grilli M, Seibold G, Caprara S. The Shrinking Fermi Liquid Scenario for Strange-Metal Behavior from Overdamped Optical Phonons. Condensed Matter. 2024; 9(1):14. https://doi.org/10.3390/condmat9010014
Chicago/Turabian StyleMirarchi, Giovanni, Marco Grilli, Götz Seibold, and Sergio Caprara. 2024. "The Shrinking Fermi Liquid Scenario for Strange-Metal Behavior from Overdamped Optical Phonons" Condensed Matter 9, no. 1: 14. https://doi.org/10.3390/condmat9010014
APA StyleMirarchi, G., Grilli, M., Seibold, G., & Caprara, S. (2024). The Shrinking Fermi Liquid Scenario for Strange-Metal Behavior from Overdamped Optical Phonons. Condensed Matter, 9(1), 14. https://doi.org/10.3390/condmat9010014







