Electroluminescence and Electron Avalanching in Two-Phase Detectors
Abstract
:1. Introduction
2. Basic Concepts of Signal Amplification in Two-Phase Detectors
3. Light Signal Amplification in the Gas Phase of a Two-Phase Detector, Using Proportional Electroluminescence
3.1. Three EL Mechanisms
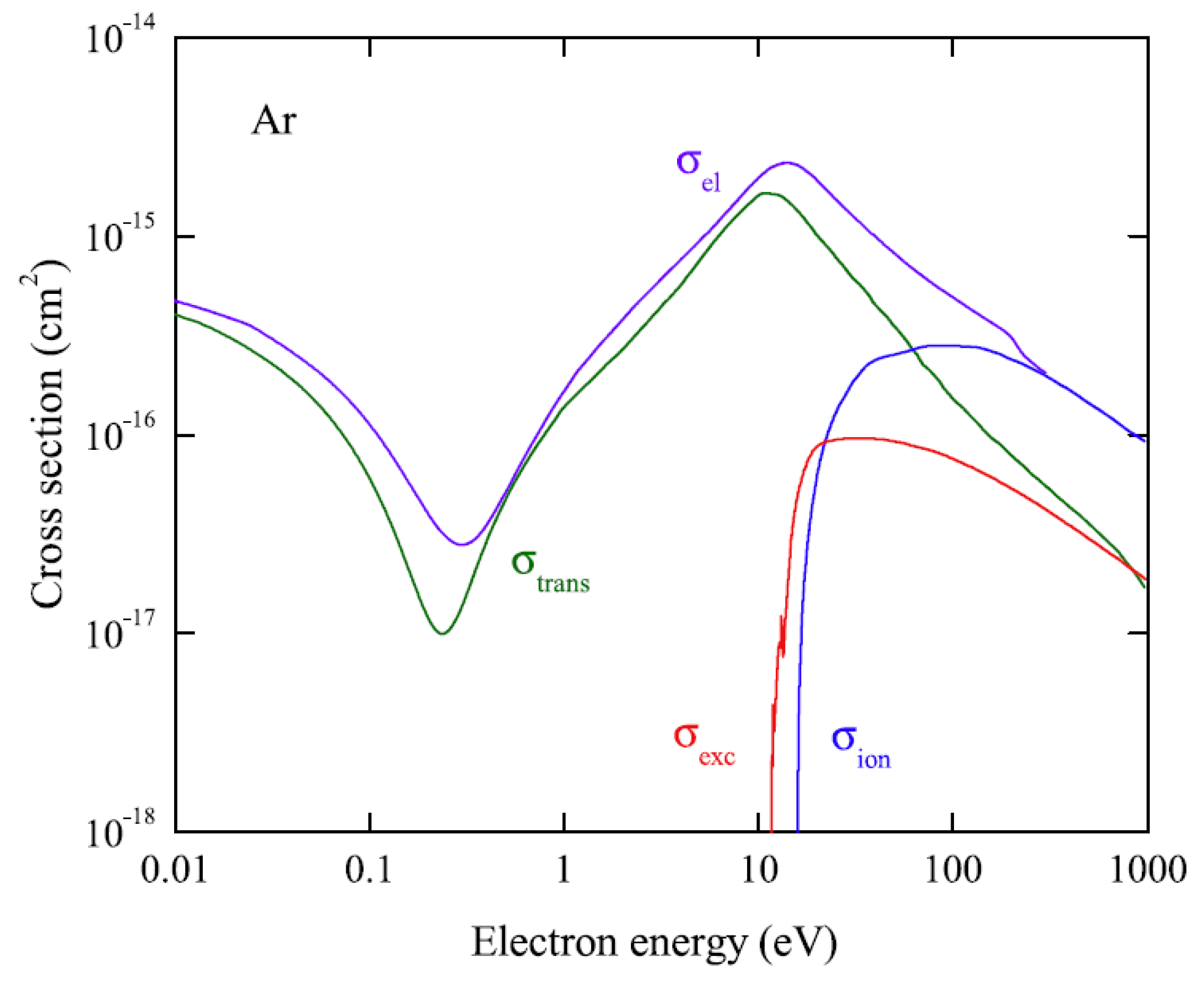
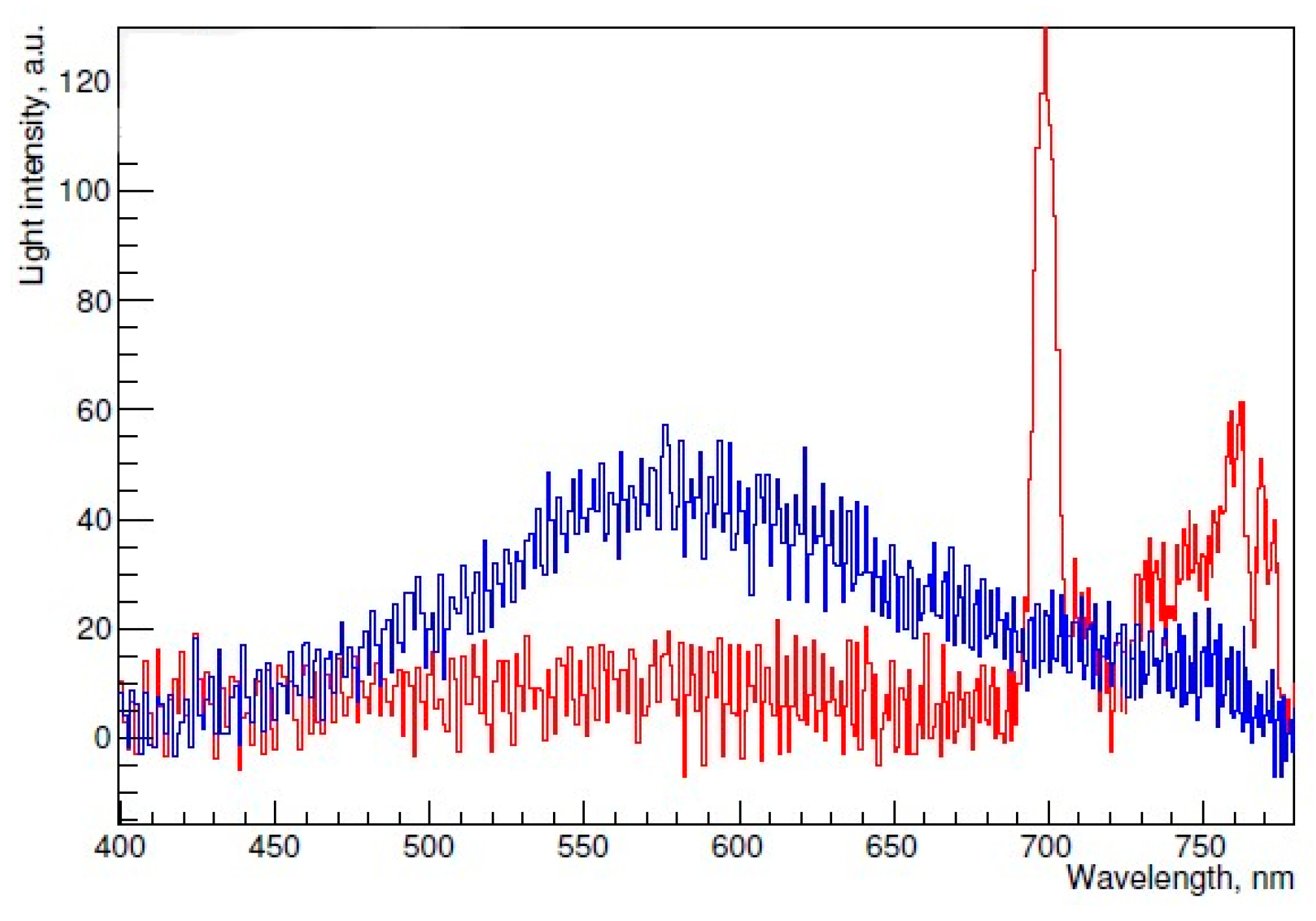
3.2. Electroluminescence Due to Excimer Emission
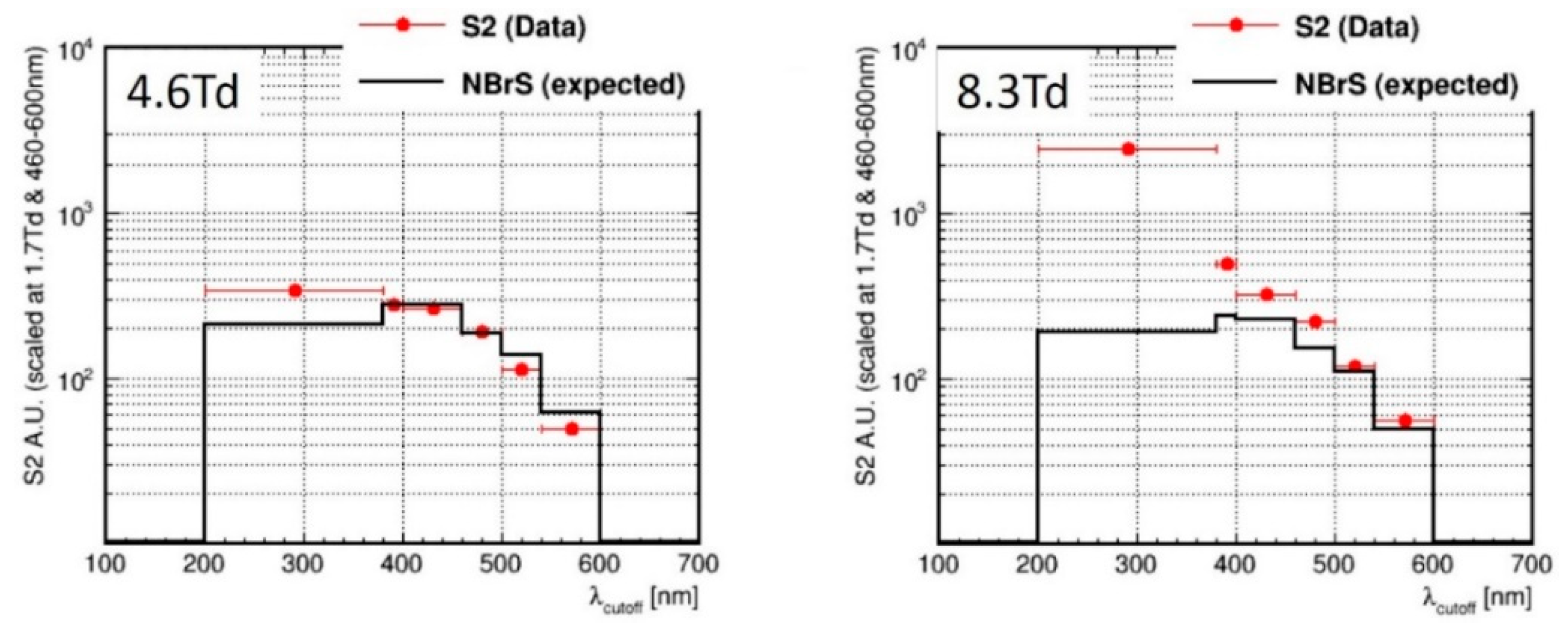
3.3. Electroluminescence Due Neutral Bremsstrahlung Effect
3.4. Electroluminescence Due to Atomic Transitions in the NIR
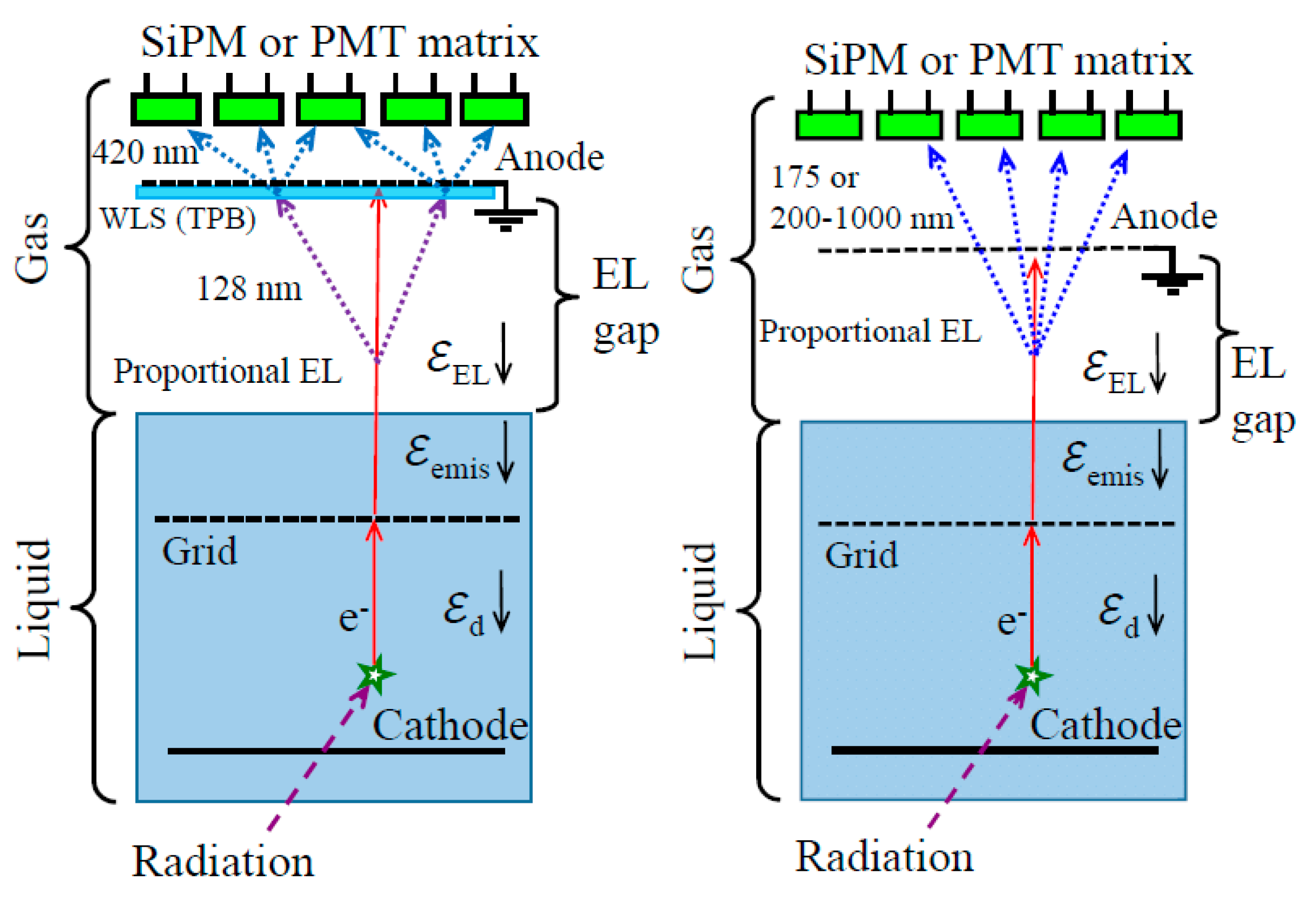
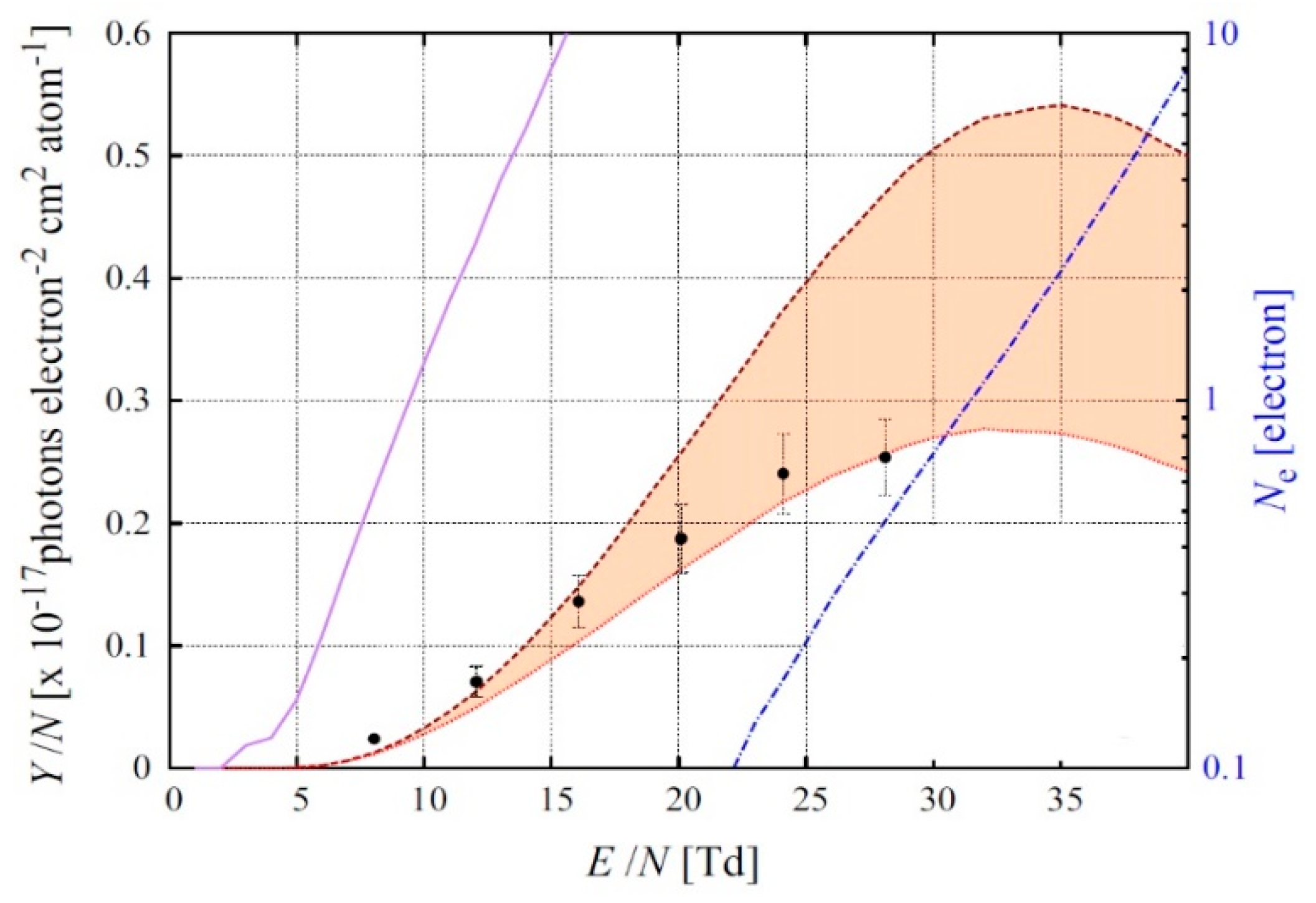
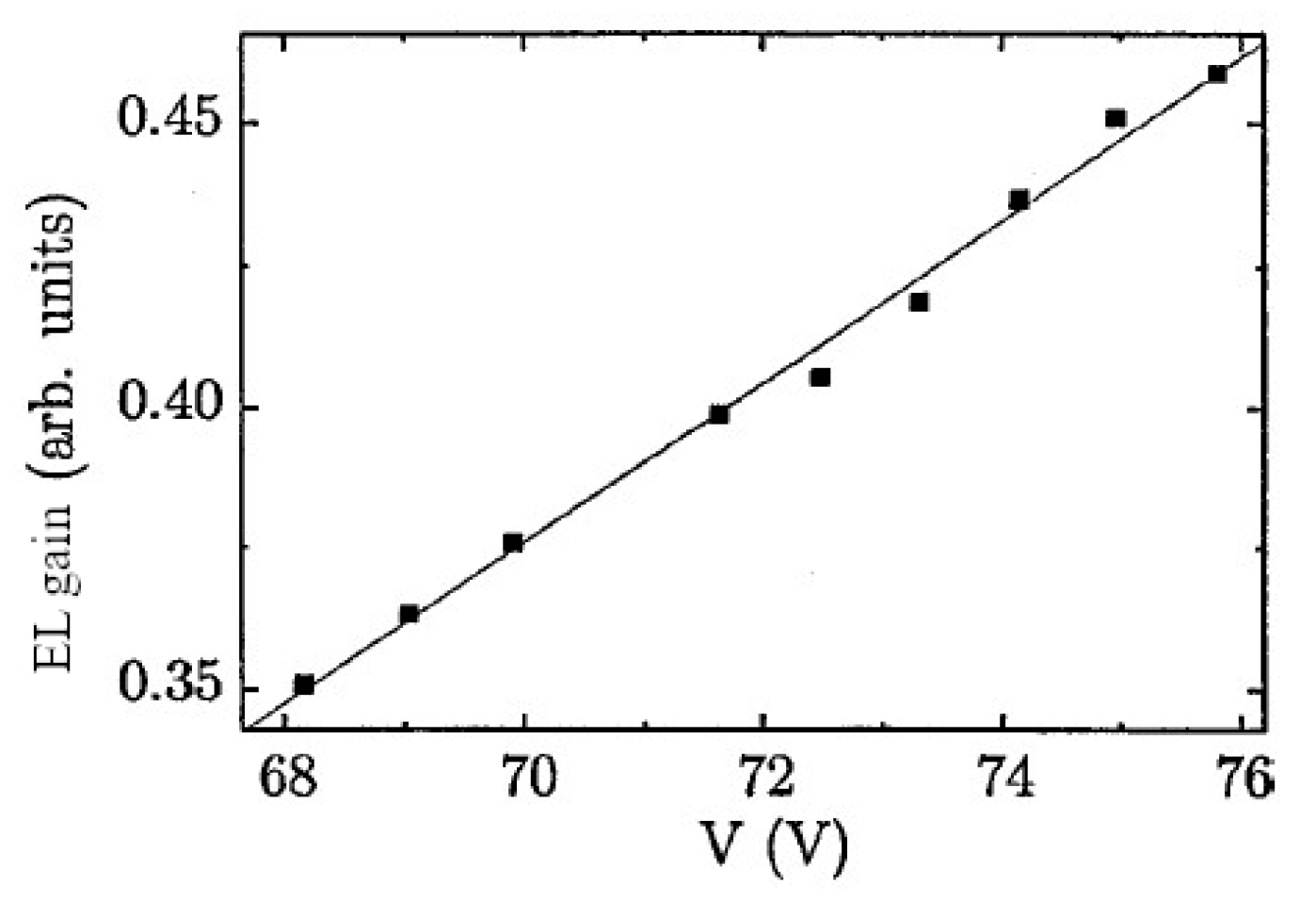
3.5. Concepts of Light Signal Amplification
4. Charge Signal Amplification in the Gas Phase of Two-Phase Detector, Using Electron Avalanching
4.1. Charge Signal Amplification Concepts at Cryogenic Temperatures
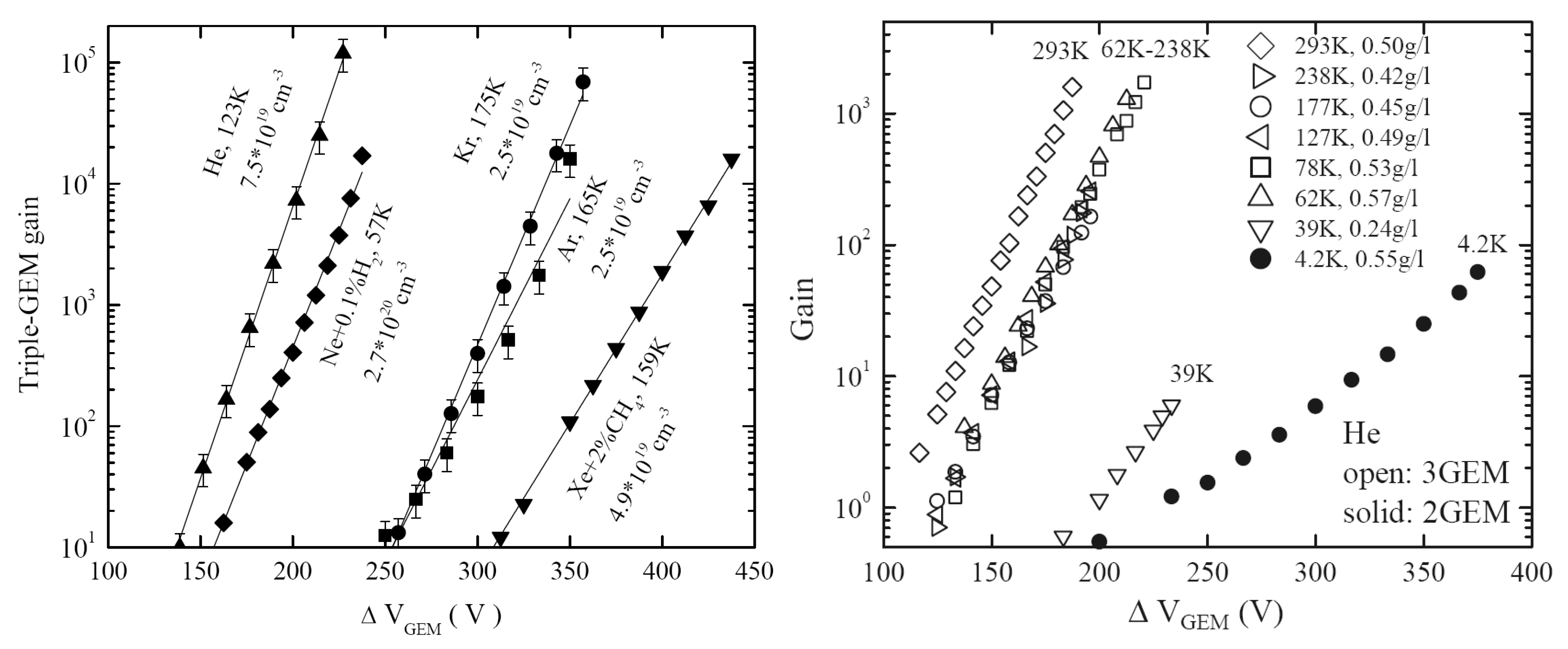
4.2. GEM Operation in Pure Noble Gases at Cryogenic Temperatures
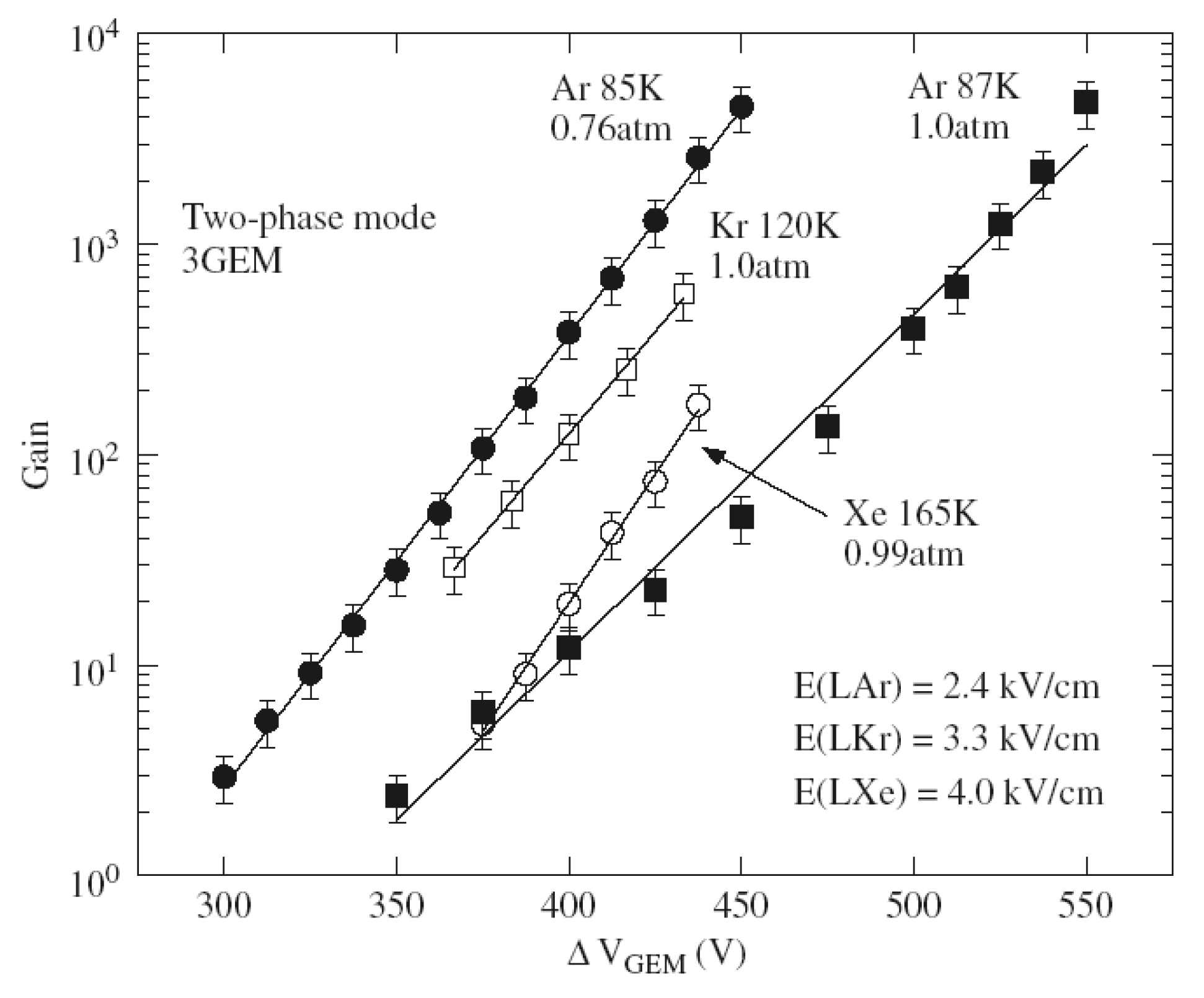
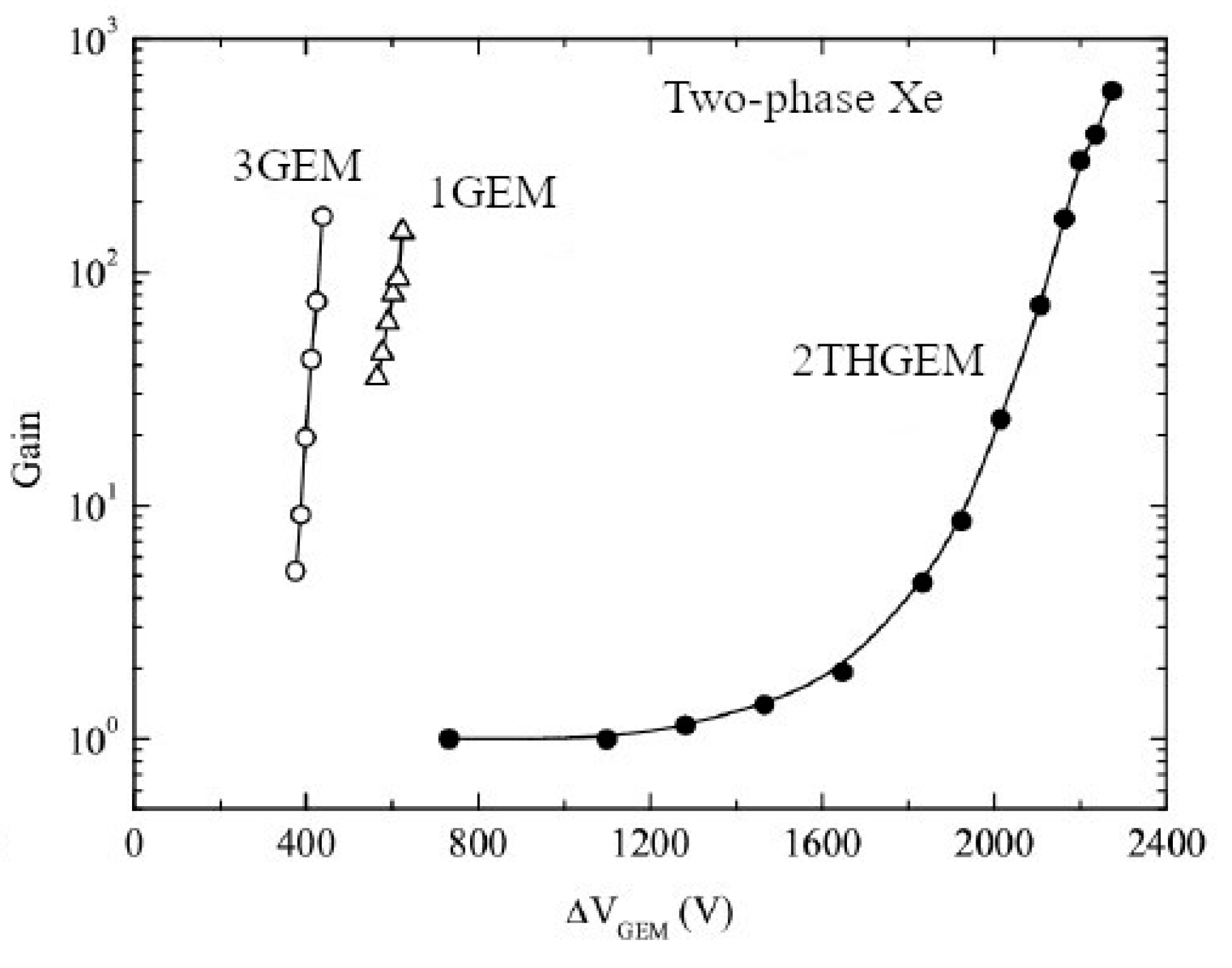
4.3. Two-Phase Detectors with GEM Multipliers
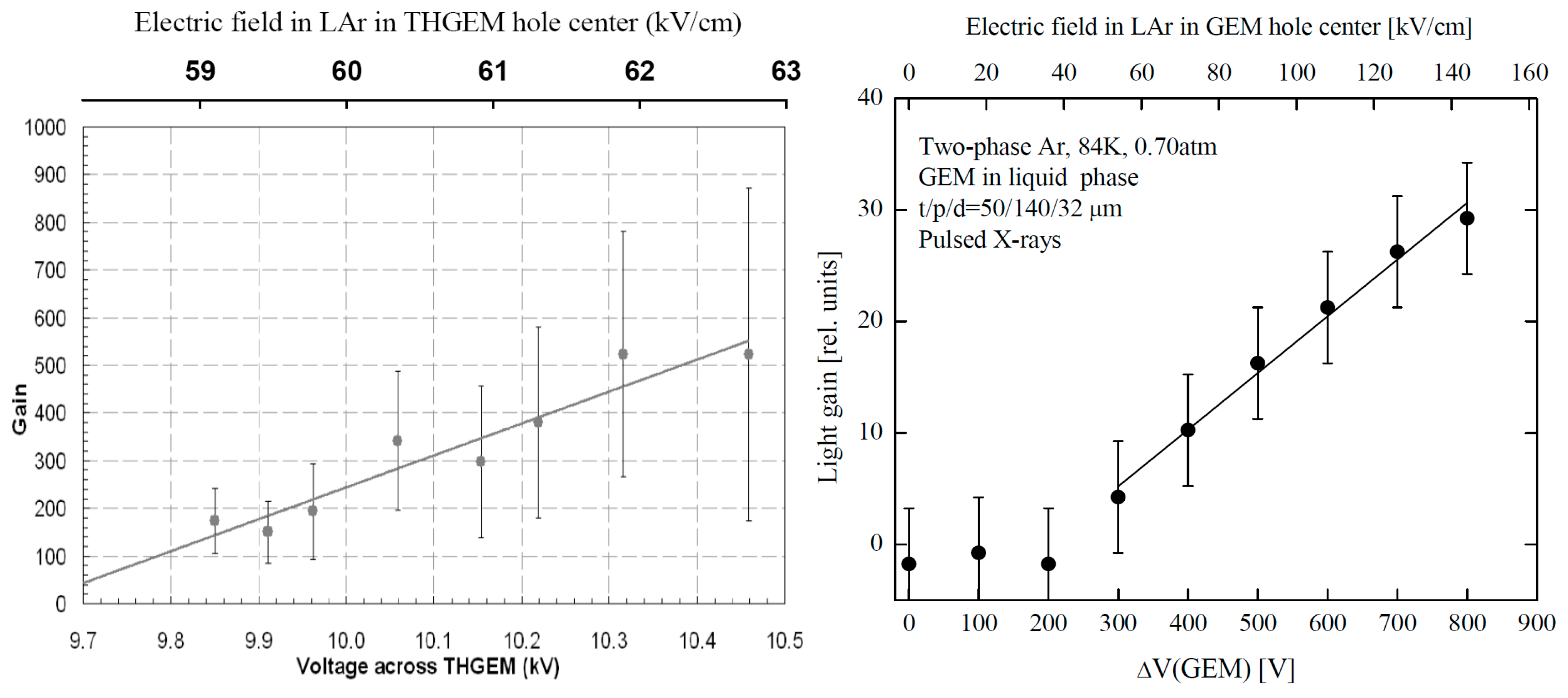
4.4. Two-Phase Detectors with THGEM Multipliers
4.5. Gain Limit, Gain Stability and Discharge-Resistance Problems in Two-Phase Detectors with GEM and THGEM Multipliers
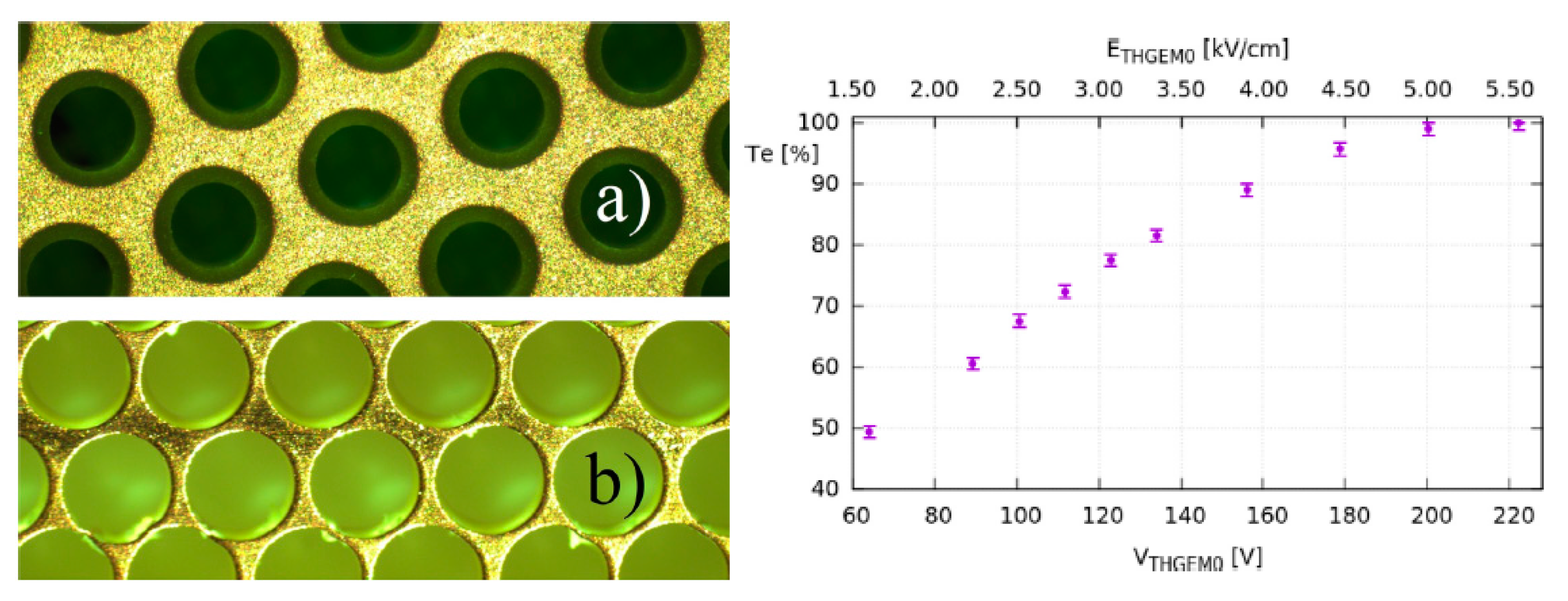
4.6. THGEM as Interface Grid in Two-Phase Detectors
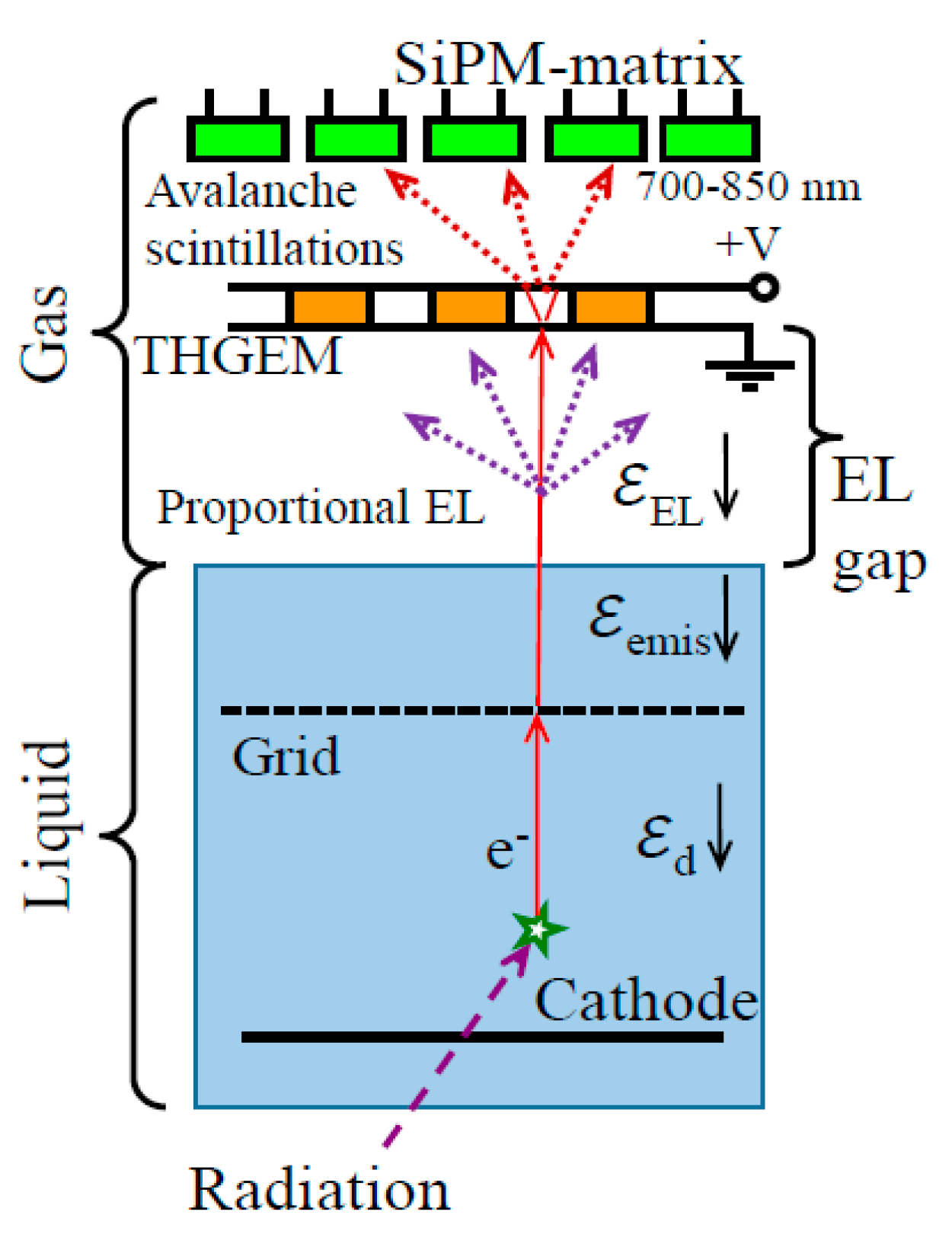
5. Combined Charge/Light Signal Amplification in the Gas Phase of Two-Phase Detector, Using Avalanche Scintillations
5.1. Two-Phase Ar Detector with Combined THGEM/SiPM-Matrix Multiplier
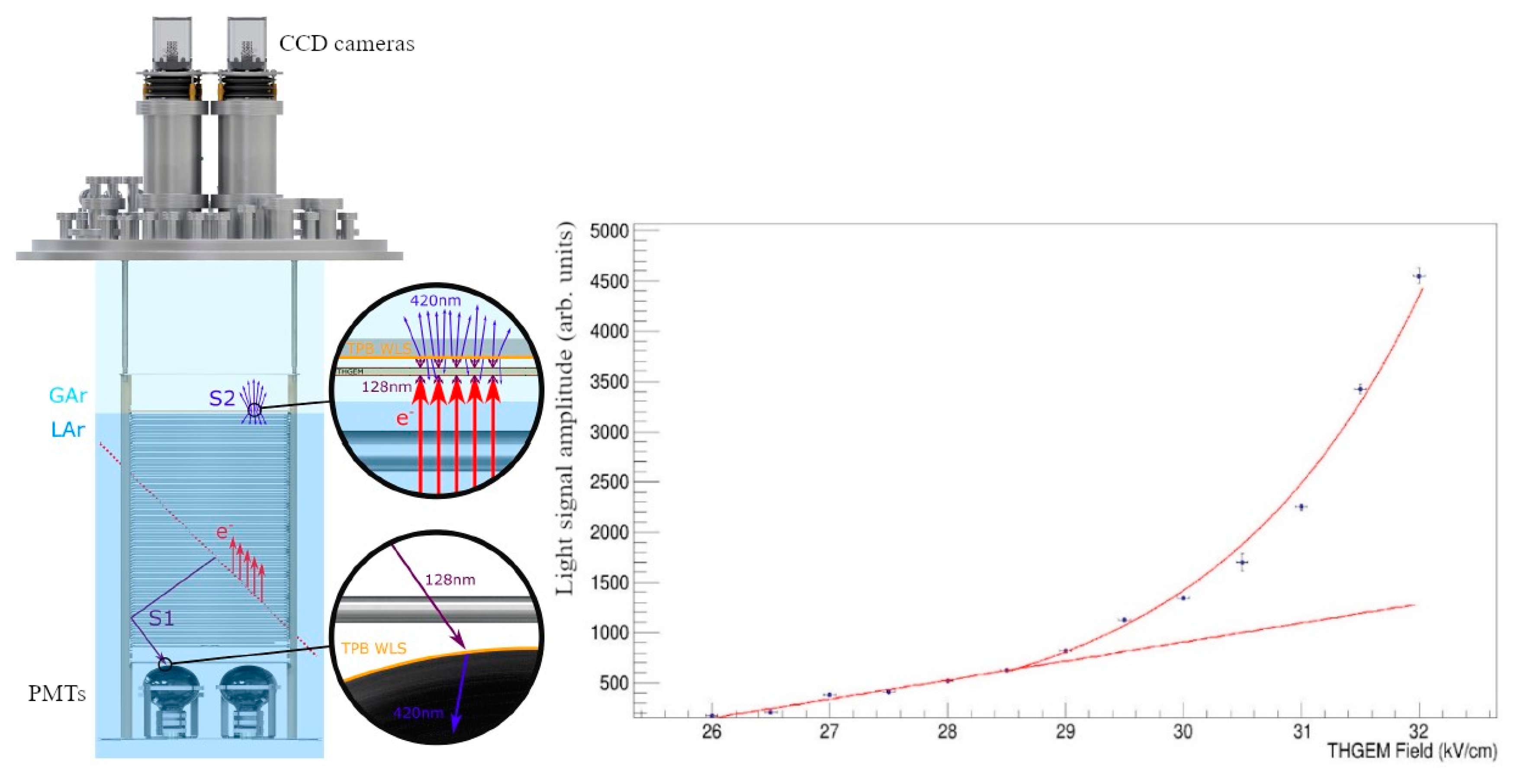
5.2. Two-Phase Ar Detector with Combined THGEM/CCD-Camera Multiplier
6. Charge and Light Signal Amplification in the Liquid Phase
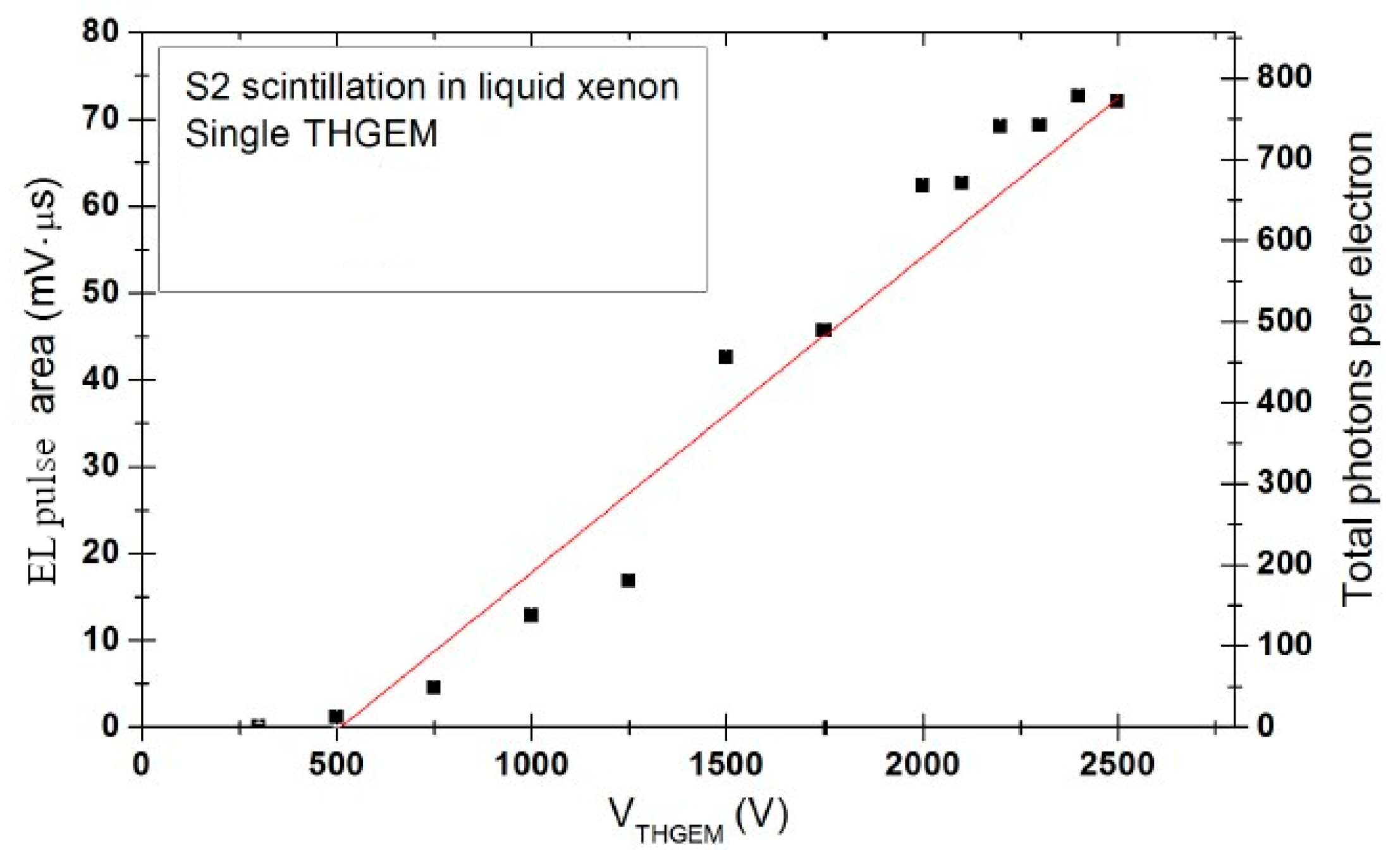
6.1. Charge and Light Signal Amplification in Liquid Xe and Liquid Ar Using wires, Strips and Needles
6.2. Light Signal Amplification in Liquid Ar Using THGEMs and GEMs
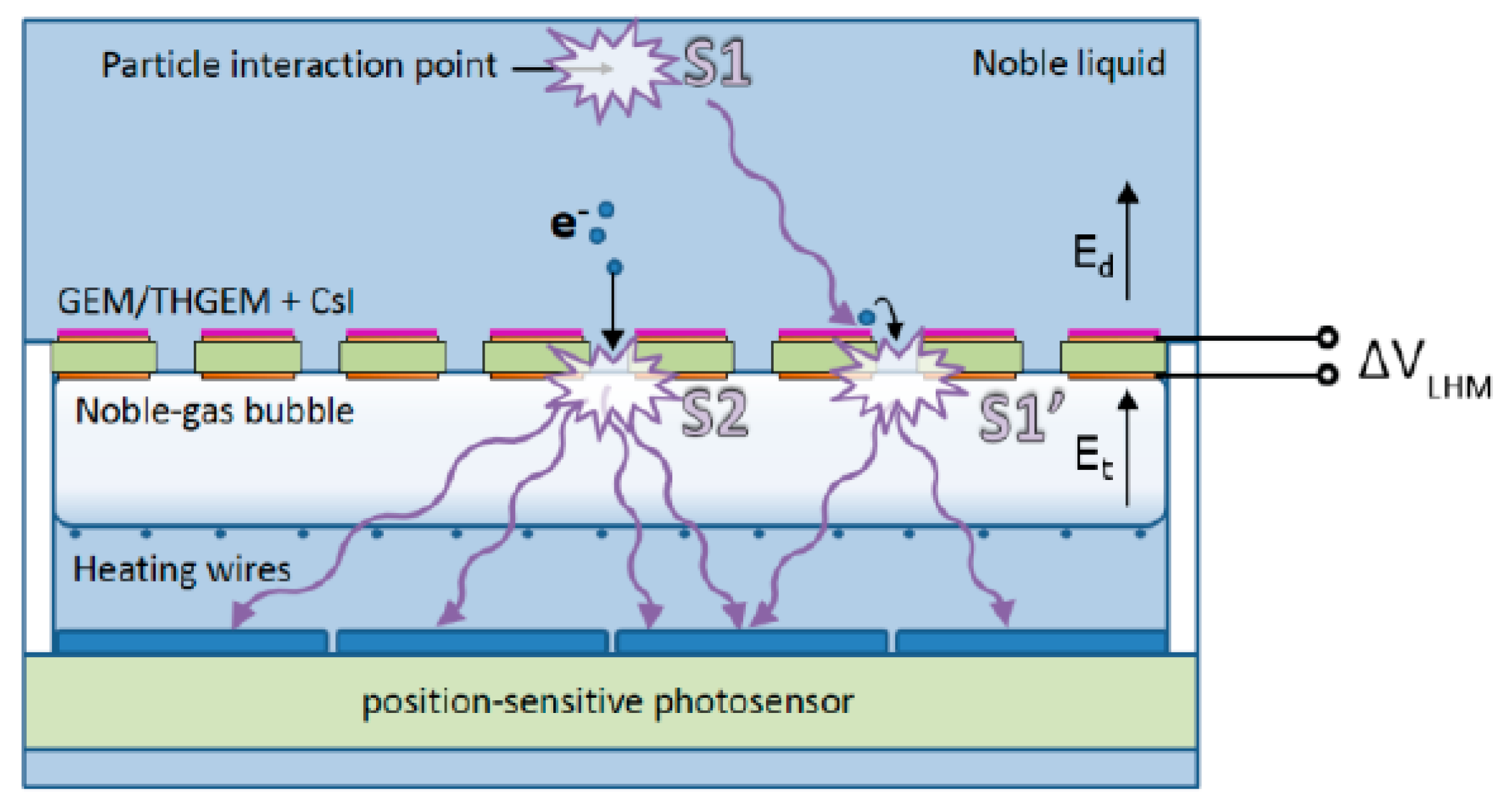
6.3. Liquid-Hole Multipliers in Liquid Ar and Liquid Xe
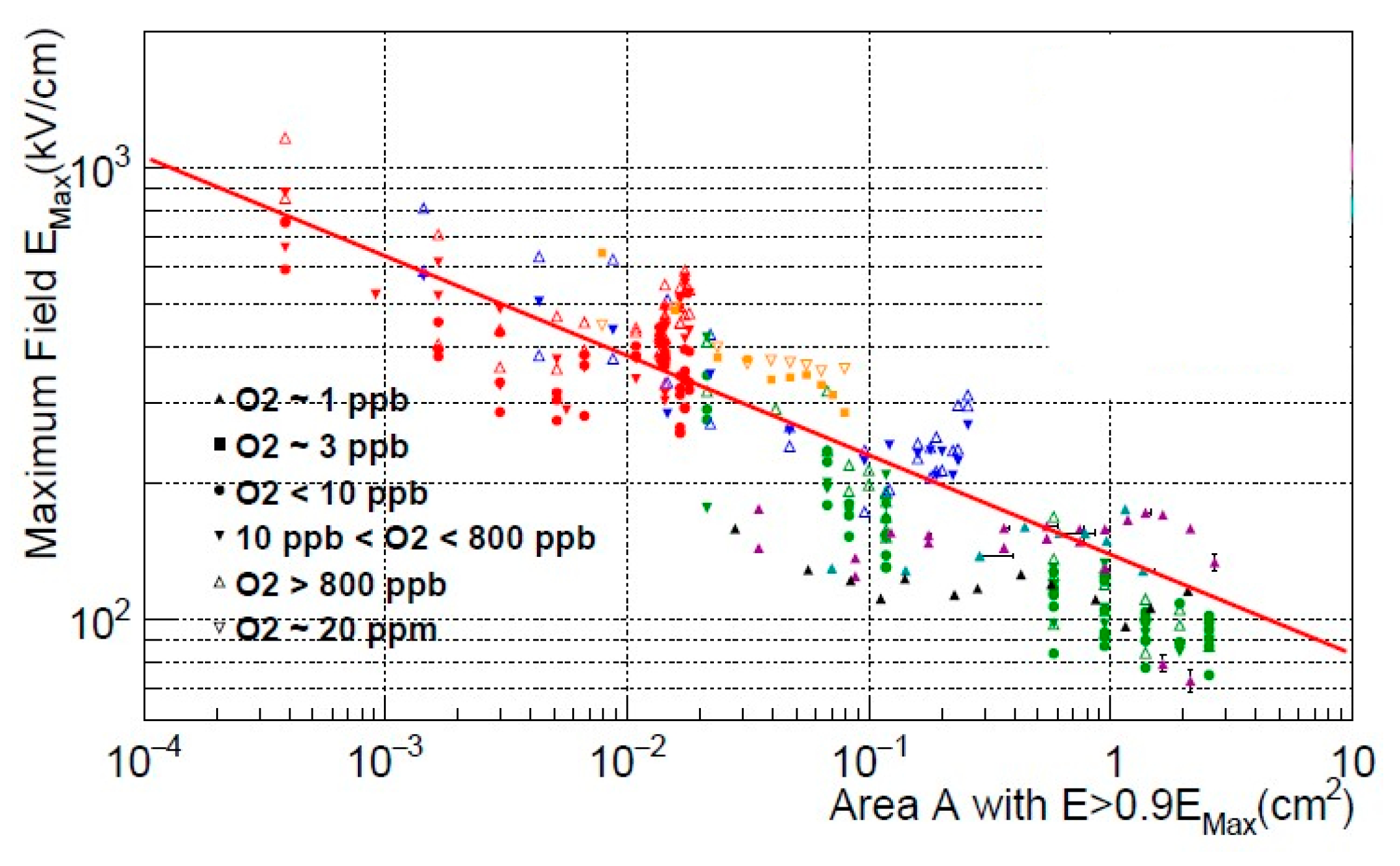
6.4. Breakdowns in Noble-Gas Liquids
7. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Dolgoshein, B.A.; Lebedenko, V.N.; Rodionov, B.U. New method of registration of ionizing-particle tracks in condensed matter. JETP Lett. 1970, 11, 351. [Google Scholar]
- Aprile, E.; Bolotnikov, A.E.; Bolozdynya, A.; Doke, T. Noble Gas Detectors; Wiley-VCH: Weinheim, Germany, 2006. [Google Scholar]
- Bolozdynya, A. Emission Detectors; World Scientific Publishing: Singapore, 2010. [Google Scholar]
- Buzulutskov, A. Advances in cryogenic avalanche detectors. J. Instrum. 2012, 7, C02025, arxiv: 1112.6153. [Google Scholar] [CrossRef] [Green Version]
- Chepel, V.; Araujo, H. Liquid noble gas detectors for low energy particle physics. J. Instrum. 2013, 8, R04001. [Google Scholar] [CrossRef] [Green Version]
- González-Díaz, D.; Monrabal, F.; Murphy, S. Gaseous and dual-phase time projection chambers for imaging rare processes. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2018, 878, 200–255. [Google Scholar] [CrossRef] [Green Version]
- Buzulutskov, A. Photon emission and atomic collision processes in two-phase argon doped with xenon and nitrogen. Europhys. Lett. 2017, 117, 39002. [Google Scholar] [CrossRef] [Green Version]
- Morozov, A.; Heindl, T.; Krücken, R.; Ulrich, A.; Wieser, J. Conversion efficiencies of electron beam energy to vacuum ultraviolet light for Ne, Ar, Kr, and Xe excited with continuous electron beams. J. Appl. Phys. 2008, 103, 103301. [Google Scholar] [CrossRef]
- Buzulutskov, A.; Shemyakina, E.; Bondar, A.; Dolgov, A.; Frolov, E.; Nosov, V.; Oleynikov, V.; Shekhtman, L.; Sokolov, À. Revealing neutral bremsstrahlung in two-phase argon electroluminescence. Astropart. Phys. 2018, 103, 29–40. [Google Scholar] [CrossRef] [Green Version]
- Lindblom, P.; Solin, O. Atomic infrared noble gas scintillations I: Optical spectra. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 1998, 268, 204–208. [Google Scholar] [CrossRef]
- Fraga, M.M.; Fetal, S.; Fraga, F.; Antunes, E.; Goncalves, J.; Bueno, C.C.; Marques, R.; Policarpo, A.J.P.L. A Study of scintillation light from microstructure based detectors. IEEE Trans. Nucl. Sci. 2000, 47, 933–938. [Google Scholar] [CrossRef]
- The DarkSide Collaboration; Aalseth, C.E.; Abdelhakim, S.; Agnes, P.; Ajaj, R.; Albuquerque, I.F.M.; Alexander, T.; Alici, A.; Alton, A.K.; Amaudruz, P.; et al. SiPM-matrix readout of two-phase argon detectors using electroluminescence in the visible and near infrared range. arXiv 2020, arXiv:2004.02024. [Google Scholar]
- Bondar, A.; Buzulutskov, A.; Dolgov, A.; Frolov, E.; Nosov, V.; Oleynikov, V.; Shemyakina, E.; Sokolov, A. Neutral bremsstrahlung in two-phase argon electroluminescence: Further studies and possible applications. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2020, 958, 162432. [Google Scholar] [CrossRef] [Green Version]
- Monteiro, C.M.B.; Matias-Lopes, J.; Veloso, J.F.C.A.; Dos Santos, J.M.F. Secondary scintillation yield in pure argon. Phys. Lett. B 2008, 668, 167–170. [Google Scholar] [CrossRef] [Green Version]
- Buzulutskov, A.; Bondar, A.; Grebenuk, A. Infrared scintillation yield in gaseous and liquid argon. Europhys. Lett. 2011, 94, 520011. [Google Scholar] [CrossRef]
- Oliveira, C.; Schindler, H.; Veenhof, R.; Biagi, S.; Monteiro, C.M.B.; dos Santos, J.M.F.; Ferreira, A.; Veloso, J.F.C.A. A simulation toolkit for electroluminescence assessment in rare event experiments. Phys. Lett. B 2011, 703, 217–222. [Google Scholar] [CrossRef] [Green Version]
- Monteiro, C.M.B.; Fernandes, L.; Matias-Lopes, J.; Coelho, L.; Veloso, J.F.C.A.; dos Santos, J.M.F.; Giboni, K.; Aprile, E. Secondary scintillation yield in pure xenon. J. Instrum. 2007, 2, P05001. [Google Scholar] [CrossRef]
- Mano, R.D.P.; Mano, R.; Barata, E.; Fernandes, L.; Freitas, E. A gas proportional scintillation counter with krypton filling. In Proceedings of the Light detection in noble elements (LIDINE), Manchester, UK, 28–30 August 2019. [Google Scholar]
- Tanaka, M.; Aoyama, K.; Kimura, M.; Takeda, T.; Yorita, K. Studies on liquid argon S1 and S2 properties for low mass WIMP search experiments. J. Phys. Conf. Ser. 2020, 1468, 012052. [Google Scholar] [CrossRef]
- al Samarai, I.; Berat, C.; Deligny, O.; Letessier-Selvon, A.; Montanet, F.; Settimo, M.; Stassi, P. Molecular bremsstrahlung radiation at GHz frequencies in air. Phys. Rev. D 2016, 93, 052004. [Google Scholar] [CrossRef] [Green Version]
- Dyachkov, L.G.; Kobzev, G.A.; Norman, G.E. Effect of resonances in elastic scattering on the bremsstrahlung of electrons in an atomic field. Sov. Phys. JETP 1974, 38, 697–700. [Google Scholar]
- Agnes, P.; Alexander, T.; Alton, A.; Arisaka, K.; Back, H.; Baldin, B.; Biery, K.; Bonfini, G.; Bossa, M.; Brigatti, A.; et al. First results from the darkside-50 dark matter experiment at Laboratori Nazionali del Gran Sasso. Phys. Lett. B 2015, 743, 456–466. [Google Scholar] [CrossRef]
- Oliveira, C.A.B.; Correia, P.M.M.; Ferreira, A.; Biagi, S.; Veenhof, R.; Veloso, J.F.C. Simulation of gaseous Ar and Xe electroluminescence in the near infrared range. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2013, 722, 1–4. [Google Scholar] [CrossRef]
- Bondar, A.; Buzulutskov, A.; Dolgov, A.; Grebenuk, A.; Peleganchuk, S.; Porosev, V.; Shekhtman, L.; Shemyakina, E.; Sokolov, A. Study of infrared scintillations in gaseous and liquid argon. Part II: Light yield and possible applications. J. Instrum. 2012, 7, P06014. [Google Scholar] [CrossRef]
- Bondar, A.; Buzulutskov, A.; Grebenuk, A.; Sokolov, A.; Akimov, D.; Alexandrov, I.; Breskin, A. Direct observation of avalanche scintillations in a THGEM-based two-phase Ar avalanche detector using Geiger-mode APD. J. Instrum. 2010, 5, P08002. [Google Scholar] [CrossRef]
- Asaadi, J.; Jones, B.; Tripathi, A.; Parmaksiz, I.; Sullivan, H.; Williams, Z. Emanation and bulk fluorescence in liquid argon from tetraphenyl butadiene wavelength shifting coatings. J. Instrum. 2019, 14, P02021. [Google Scholar] [CrossRef] [Green Version]
- Derenzo, S.E.; Mast, T.S.; Zaklad, H.; Muller, R.A. Electron avalanche in liquid xenon. Phys. Rev. A 1974, 9, 2582–2591. [Google Scholar] [CrossRef] [Green Version]
- Masuda, K.; Takasu, S.; Doke, T.; Takahashi, T.; Nakamoto, A.; Kubota, S.; Shibamura, E. A liquid xenon proportional scintillation counter. Nucl. Instrum. Methods 1979, 160, 247–253. [Google Scholar] [CrossRef]
- Policarpo, A.; Chepel, V.; Lopes, I.; Peskov, V.; Geltenbort, P.; Marques, R.; Araujo, H.; Fraga, F.; Alves, M.; Fonte, P.; et al. Observation of electron multiplication in liquid xenon with a Microstrip plate. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 1995, 365, 568–571. [Google Scholar] [CrossRef]
- Aprile, E.; Contreras, H.; Goetzke, L.W.; Fernandez, A.J.M.; Messina, M.; Naganoma, J.; Plante, G.; Rizzo, A.; Shagin, P.; Wall, R. Measurements of proportional scintillation and electron multiplication in liquid xenon using thin wires. J. Instrum. 2014, 9, P11012. [Google Scholar] [CrossRef] [Green Version]
- Kim, J.; Dardin, S.; Kadel, R.; Kadyk, J.; Peskov, V.; Wenzel, W. Electron avalanches in liquid argon mixtures. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2004, 534, 376–396. [Google Scholar] [CrossRef] [Green Version]
- Grebinnik, V.G.; Dodokhov, V.K.; Zhukov, V.A. High-pressure proportional counter at low temperatures. Instr. Exp. Tech. 1978, 21, 1225. [Google Scholar]
- Masaoka, S.; Katano, R.; Kishimoto, S.; Isozumi, Y. A model for the operation of helium-filled proportional counter at low temperatures near 4.2 K. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 2000, 171, 360–372. [Google Scholar] [CrossRef]
- Dolgoshein, B.A.; Kruglov, A.A.; Lebedenko, V.N.; Miroshnichenko, V.P. Electronic particle detection method for two-phase systems: Liquid-gas. Sov. J. Part. Nucl. 1973, 4, 70. [Google Scholar]
- Buzulutskov, A.; Bondar, A.; Shekhtman, L.; Snopkov, R.; Tikhonov, Y. First results from cryogenic avalanche detectors based on gas electron multipliers. IEEE Trans. Nucl. Sci. 2003, 50, 2491–2493. [Google Scholar] [CrossRef]
- Sauli, F. The gas electron multiplier (GEM): Operating principles and applications. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2016, 805, 2–24. [Google Scholar] [CrossRef]
- Breskin, A.; Alon, R.; Cortesi, M.; Chechik, R.; Miyamoto, J.; Dangendorf, V.; Maia, J.; dos Santos, J.M.F. A concise review on THGEM detectors. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2009, 598, 107–111. [Google Scholar] [CrossRef] [Green Version]
- Buzulutskov, A.; Breskin, A.; Chechik, R.; Garty, G.; Sauli, F.; Shekhtman, L. The GEM photomultiplier operated with noble gas mixtures. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2000, 443, 164–180. [Google Scholar] [CrossRef] [Green Version]
- Buzulutskov, A.F. Radiation detectors based on gas electron multipliers (review). Instrum. Exp. Tech. 2007, 50, 287–310. [Google Scholar] [CrossRef]
- Bondar, A.; Buzulutskov, A.; Grebenuk, A.; Pavlyuchenko, D.; Snopkov, R.; Tikhonov, Y. Two-phase argon and xenon avalanche detectors based on Gas Electron Multipliers. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2006, 556, 273–280. [Google Scholar] [CrossRef] [Green Version]
- Buzulutskov, A.; Dodd, J.; Galea, R.; Ju, Y.; Leltchouk, M.; Rehak, P.; Tcherniatine, V.; Willis, W.; Bondar, A.; Pavlyuchenko, D.; et al. GEM operation in helium and neon at low temperatures. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2005, 548, 487–498. [Google Scholar] [CrossRef] [Green Version]
- Galea, R.; Dodd, J.; Ju, Y.; Leltchouk, M.; Willis, W.; Rehak, P.; Tcherniatine, V.; Buzulutskov, A.; Pavlyuchenko, D. Gas purity effect on GEM performance in He and Ne at low temperatures. IEEE Trans. Nucl. Sci. 2006, 53, 2260–2263. [Google Scholar] [CrossRef] [Green Version]
- Periale, L.; Peskov, V.; Iacobaeus, C.; Francke, T.; Lund-Jensen, B.; Pavlopoulos, P.; Picchi, P.; Pietropaolo, F.; Tokanai, F. The successful operation of hole-type gaseous detectors at cryogenic temperatures. IEEE Trans. Nucl. Sci. 2005, 52, 927–931. [Google Scholar] [CrossRef] [Green Version]
- Rubbia, A. Experiments for cp-violation: A giant liquid argon scintillation, cerenkov and charge imaging experiment. J. Phys. Conf. Ser. 2006, 39, 129. [Google Scholar] [CrossRef] [Green Version]
- Buzulutskov, A.; Bondar, A. Electric and Photoelectric Gates for ion backflow suppression in multi-GEM structures. J. Instrum. 2006, 1, P08006. [Google Scholar] [CrossRef] [Green Version]
- Ju, Y.; Dodd, J.; Galea, R.; Leltchouk, M.; Willis, W.; Jia, L.; Rehak, P.; Tcherniatine, V. Cryogenic design and operation of liquid helium in an electron bubble chamber towards low energy solar neutrino detectors. Cryogenics 2007, 47, 81–88. [Google Scholar] [CrossRef]
- Gai, M.; McKinsey, D.N.; Ni, K.; Rubin, D.A.R.; Wongjirad, T.; Alon, R.; Breskin, A.; Cortesi, M.; Miyamoto, J. Toward application of a thick gas electron multiplier (THGEM) readout for a dark matter detector. arXiv 2007, arXiv:0706.1106. [Google Scholar]
- Bondar, A.; Buzulutskov, A.; Grebenuk, A.; Pavlyuchenko, D.; Tikhonov, Y.; Breskin, A. Thick GEM versus thin GEM in two-phase argon avalanche detectors. J. Instrum. 2008, 3, P07001. [Google Scholar] [CrossRef]
- Lightfoot, P.K.; Barker, G.J.; Mavrokoridis, K.; Ramachers, Y.A.; Spooner, N.J.C. Optical readout tracking detector concept using secondary scintillation from liquid argon generated by a thick gas electron multiplier. J. Instrum. 2009, 4, P04002. [Google Scholar] [CrossRef] [Green Version]
- McConkey, N.; Barker, G.; Bennieston, A.; Harrison, P.; Lightfoot, P.K.; Morgan, B.; Stewart, D.; Spooner, N.J.C.; Ramachers, Y.A. Optical readout technology for large volume liquid argon detectors. Nucl. Phys. B Proc. Suppl. 2011, 215, 255–257. [Google Scholar] [CrossRef]
- Akimov, D.; Bondar, A.; Burenkov, A.; Buzulutskov, A. Detection of reactor antineutrino coherent scattering off nuclei with a two-phase noble gas detector. J. Instrum. 2009, 4, P06010. [Google Scholar] [CrossRef]
- Duval, S.; Breskin, A.; Carduner, H.; Cussonneau, J.-P.; Lamblin, J.; Le Ray, P.; Morteau, E.; Oger, T.; Stutzmann, J.-S.; Thers, D. MPGDs in Compton imaging with liquid-xenon. J. Instrum. 2009, 4, P12008. [Google Scholar] [CrossRef] [Green Version]
- Akimov, D. Techniques and results for the direct detection of dark matter (review). Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2011, 628, 50–58. [Google Scholar] [CrossRef]
- Duval, S.; Breskin, A.; Budnik, R.; Chen, W.T.; Carduner, H.; Cortesi, M.; Cussonneau, J.P.; Donnard, J.; Lamblin, J.; Le Ray, P.; et al. On the operation of a micropattern gaseous UV-photomultiplier in liquid-Xenon. J. Instrum. 2011, 6, P04007. [Google Scholar] [CrossRef]
- Bondar, A.; Buzulutskov, A.; Dolgov, A.; Grebenuk, A.; Shemyakina, E.; Sokolov, A.; Breskin, A.; Thers, D. First demonstration of THGEM/GAPD-matrix optical readout in a two-phase Cryogenic Avalanche Detector in Ar. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2013, 732, 213–216. [Google Scholar] [CrossRef]
- Akimov, D.Y.; Akindinov, A.; Alexandrov, I.S.; Belov, V.A.; Bolozdynya, A.I.; Burenkov, A.; Buzulutskov, A.F.; Danilov, M.; Efremenko, Y.V.; Kirsanov, M.A.; et al. Two-phase xenon emission detector with electron multiplier and optical readout by multipixel avalanche Geiger photodiodes. J. Instrum. 2013, 8, P05017. [Google Scholar] [CrossRef] [Green Version]
- Breskin, A. Liquid Hole-Multipliers: A potential concept for large single-phase noble-liquid TPCs of rare events. J. Phys. Conf. Ser. 2013, 460, 012020. [Google Scholar] [CrossRef]
- Arazi, L.; Erdal, E.; Coimbra, A.; Rappaport, M.; Vartsky, D.; Chepel, V.; Breskin, A. Liquid hole multipliers: Bubble-assisted electroluminescence in liquid xenon. J. Instrum. 2015, 10, P08015. [Google Scholar] [CrossRef]
- Erdal, E.; Arazi, L.; Breskin, A.; Shchemelinin, S.; Roy, A.; Tesi, A.; Vartsky, D.; Bressler, S. Bubble-assisted liquid hole multipliers in LXe and LAr: Towards “local dual-phase TPCs”. J. Instrum. 2020, 15, C04002. [Google Scholar] [CrossRef] [Green Version]
- Mavrokoridis, K.; Ball, F.; Carroll, J.; Lazos, M.; McCormick, K.J.; Smith, N.A.; Touramanis, C.; Walker, J. Optical readout of a two phase liquid argon TPC using CCD camera and THGEMs. J. Instrum. 2014, 9, P02006. [Google Scholar] [CrossRef] [Green Version]
- Hollywood, D.; Majumdar, K.; Mavrokoridis, K.; McCormick, K.; Philippou, B.; Powell, S.; Roberts, A.; Smith, N.; Stavrakis, G.; Touramanis, C.; et al. ARIADNE—A novel optical LArTPC: Technical design report and initial characterisation using a secondary beam from the CERN PS and cosmic muons. J. Instrum. 2020, 15, P03003. [Google Scholar] [CrossRef]
- Ye, T.; Giboni, K.; Ji, X. Initial evaluation of proportional scintillation in liquid Xenon for direct dark matter detection. J. Instrum. 2014, 9, P12007. [Google Scholar] [CrossRef]
- Bressan, A.; Buzulutskov, A.; Ropelewski, L.; Sauli, F.; Shekhtman, L. High gain operation of GEM in pure argon. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 1999, 423, 119–124. [Google Scholar] [CrossRef] [Green Version]
- Bondar, A.; Buzulutskov, A.; Shekhtman, L. High pressure operation of the triple-GEM detector in pure Ne, Ar and Xe. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2002, 481, 200–203. [Google Scholar] [CrossRef] [Green Version]
- Bondar, A.; Buzulutskov, A.; Shekhtman, L.; Snopkov, V.; Vasiljev, A. Triple GEM operation in compressed He and Kr. Nucl. Instrum. Methods Phys. Res. Sect. A: Accel. Spectrom. Detect. Assoc. Equip. 2002, 493, 8–15. [Google Scholar] [CrossRef] [Green Version]
- Bondar, A.; Buzulutskov, A.; Shekhtman, L.; Snopkov, R.; Tikhonov, Y. Cryogenic avalanche detectors based on gas electron multipliers. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2004, 524, 130–141. [Google Scholar] [CrossRef] [Green Version]
- Bondar, A.; Buzulutskov, A.; Grebenuk, A.; Pavlyuchenko, D.; Tikhonov, Y. Recent results on the properties of two-phase argon avalanche detectors. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2009, 598, 121–125. [Google Scholar] [CrossRef] [Green Version]
- Bondar, A.; Buzulutskov, A.; Grebenuk, A.; Pavlyuchenko, D.; Snopkov, R.; Tikhonov, Y.; Kudryavtsev, V.; Lightfoot, P.; Spooner, N. A two-phase argon avalanche detector operated in a single electron counting mode. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2007, 574, 493–499. [Google Scholar] [CrossRef] [Green Version]
- Bondar, A.; Buzulutskov, A.; Grebenuk, A.; Pavlyuchenko, D.; Tikhonov, Y. Electron emission properties of two-phase argon and argon-nitrogen avalanche detectors. J. Instrum. 2009, 4, P09013. [Google Scholar] [CrossRef]
- Bondar, A.; Buzulutskov, A.; Grebenuk, A.; Shemyakina, E.; Sokolov, A.; Akimov, D.; Alexandrov, I.; Breskin, A. On the low-temperature performances of THGEM and THGEM/G-APD multipliers in gaseous and two-phase Xe1. J. Instrum. 2011, 6, P07008. [Google Scholar] [CrossRef] [Green Version]
- Balau, F.; Solovov, V.; Chepel, V.; Pereira, A.; Lopes, M.; Lopes, I. GEM operation in double-phase xenon. Nucl. Instrum. Methods Phys. Res. Sect. A: Accel. Spectrom. Detect. Assoc. Equip. 2009, 598, 126–129. [Google Scholar] [CrossRef] [Green Version]
- Bondar, A.; Buzulutskov, A.; Dolgov, A.; Grebenuk, A.; Shemyakina, E.; Sokolov, A.; Akimov, D.; Breskin, A.; Thers, D. Two-phase cryogenic avalanche detectors with THGEM and hybrid THGEM/GEM multipliers operated in Ar and Ar+N2. J. Instrum. 2013, 8, P02008. [Google Scholar] [CrossRef] [Green Version]
- Badertscher, A.; Curioni, A.; Knecht, L.; Lussi, D.; Marchionni, A.; Natterer, G.; Resnati, F.; Rubbia, A.; Viant, T. First operation of a double phase LAr large electron multiplier time projection chamber with a 2D projective readout anode. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2011, 641, 48–57. [Google Scholar] [CrossRef] [Green Version]
- Badertscher, A.; Curioni, A.; Degunda, U.; Epprecht, L.; Gendotti, A.; Horikawa, S.; Knecht, L.; Lussi, D.; Natterer, G.; Nguyen, K.; et al. First operation and performance of a 200 lt double phase LAr LEM-TPC with a 40 × 76 cm2 readout. J. Instrum. 2013, 8, P04012. [Google Scholar] [CrossRef] [Green Version]
- Cantini, C.; Epprecht, L.; Gendotti, A.; Horikawa, S.; Periale, L.; Murphy, S.; Natterer, G.; Regenfus, C.; Resnati, F.; Sergiampietri, F.; et al. Performance study of the effective gain of the double phase liquid Argon LEM Time Projection Chamber. J. Instrum. 2015, 10, P03017. [Google Scholar] [CrossRef] [Green Version]
- Aimard, B.; Alt, C.; Asaadi, J.; Auger, M.; Aushev, V.; Autiero, D.; Badoi, M.; Balaceanu, A.; Balik, G.; Balleyguier, L.; et al. A 4 tonne demonstrator for large-scale dual-phase liquid argon time projection chambers. J. Instrum. 2018, 13, P11003. [Google Scholar] [CrossRef]
- Buzulutskov, A.; (Budker Institute of Nuclear Physics, Novosibirsk, Russia). Personal communication, 2020.
- Lightfoot, P.K.; Hollingworth, R.; Spooner, N.; Tovey, D. Development of a double-phase Xenon cell using micromegas charge readout for applications in dark matter physics. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2005, 554, 266–285. [Google Scholar] [CrossRef]
- Bondar, A.; Buzulutskov, A.; Frolov, E.; Oleynikov, V.; Shemyakina, E.; Sokolov, A. Electron transport and electric field simulations in two-phase detectors with THGEM electrodes. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2019, 943, 162431. [Google Scholar] [CrossRef] [Green Version]
- Schüssler, A.S.; Burghorn, J.; Wyder, P.; Lembrikov, B.I.; Baptist, R. Observation of excimer luminescence from electron-excited liquid xenon. Appl. Phys. Lett. 2000, 77, 2786–2788. [Google Scholar] [CrossRef]
- Stewart, D.Y.; Barker, G.J.; Bennieston, A.J.; Harrison, P.F.; Lightfoot, P.K.; McConkey, N.; Morgan, B.; Ramachers, Y.A.; Robinson, M.; Spooner, N.J.C.; et al. Modelling electroluminescence in liquid argon. J. Instrum. 2010, 5, P10005. [Google Scholar] [CrossRef] [Green Version]
- Auger, M.; Blatter, A.; Ereditato, A.; Goeldi, D.; Janos, S.; Kreslo, I.; Luethi, M.; Von Rohr, C.R.; Strauss, T.; Weber, M. On the electric breakdown in liquid argon at centimeter scale. J. Instrum. 2016, 11, P03017. [Google Scholar] [CrossRef] [Green Version]
- Arazi, L.; Coimbra, A.; Itay, R.; Landsman, H.; Levinson, L.; Pasmantirer, B.; Rappaport, M.L.; Vartsky, D.; Breskin, A. First observation of liquid-xenon proportional electroluminescence in THGEM holes. J. Instrum. 2013, 8, C12004. [Google Scholar] [CrossRef] [Green Version]
- Erdal, E.; Arazi, L.; Chepel, V.; Rappaport, M.; Vartsky, D.; Breskin, A. Direct observation of bubble-assisted electroluminescence in liquid xenon. J. Instrum. 2015, 10, P11002. [Google Scholar] [CrossRef]
- Aalseth, C.E.; Acerbi, F.; Agnes, P.; Albuquerque, I.F.M.; Alexander, T.; Alici, A.; Alton, A.K.; Antonioli, P.; Arcelli, S.; Ardito, R.; et al. DarkSide-20k: A 20 tonne two-phase LAr TPC for direct dark matter detection at LNGS. Eur. Phys. J. Plus 2018, 133, 131. [Google Scholar] [CrossRef]
- Gerhold, J.; Hubmann, M.; Telser, E. Gap size effect on liquid helium breakdown. Cryogenics 1994, 34, 579–586. [Google Scholar] [CrossRef]
- Acciarri, R.; Carls, B.; James, C.; Johnson, B.; Jöstlein, H.; Lockwitz, S.; Lundberg, B.; Raaf, J.; Rameika, R.; Rebel, B.; et al. Liquid argon dielectric breakdown studies with the MicroBooNE purification system. J. Instrum. 2014, 9, P11001. [Google Scholar] [CrossRef] [Green Version]
- Tvrznikova, L.; Bernard, E.; Kravitz, S.; O’Sullivan, K.; Richardson, G.; Riffard, Q.; Waldron, W.; Watson, J.; McKinsey, D. Direct comparison of high voltage breakdown measurements in liquid argon and liquid xenon. J. Instrum. 2019, 14, P12018. [Google Scholar] [CrossRef] [Green Version]
- Heindl, T.; Dandl, T.; Hofmann, M.; Krücken, R.; Oberauer, L.; Potzel, W.; Wieser, J.; Ulrich, A. The scintillation of liquid argon. Europhys. Lett. 2010, 91, 62002. [Google Scholar] [CrossRef] [Green Version]


















© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Buzulutskov, A. Electroluminescence and Electron Avalanching in Two-Phase Detectors. Instruments 2020, 4, 16. https://doi.org/10.3390/instruments4020016
Buzulutskov A. Electroluminescence and Electron Avalanching in Two-Phase Detectors. Instruments. 2020; 4(2):16. https://doi.org/10.3390/instruments4020016
Chicago/Turabian StyleBuzulutskov, Alexey. 2020. "Electroluminescence and Electron Avalanching in Two-Phase Detectors" Instruments 4, no. 2: 16. https://doi.org/10.3390/instruments4020016