1. Introduction
In the framework of various challenges human society is currently facing, e.g., climate change, the extinction of species, or the rapidly increasing population on earth, it is crucial to deeply understand complex biological, physical, and chemical processes in nature to find solutions for the challenges. As synchrotron radiation sources allow the investigation of structures, materials, and processes in different time and length scales in situ/in vivo, they are essential to create a deeper understanding of nature [
1]. With the help of synchrotrons, for example, large membrane protein complexes have been investigated [
2], the novel coronavirus SARS-CoV-2 has been studied [
3], and the rhizosphere chemistry was analysed [
4]. However, also the detailed investigation of technical structures, materials, and fabrication processes is fundamental to improve technology. The synchrotron radiation technology plays a key role in engineering science, permitting, for example, a detailed analysis of the structure solidification process of alloys [
5] or the in situ analyses of metal additive manufacturing [
6]. Aside from using the synchrotron radiation sources in different areas of investigation, synchrotron radiation facilities involve many other research fields, e.g., the interdisciplinary research related to the installation and the setup of the accelerator machine and the beamlines. The present study contributes to the research on the accelerator machine setup, which is very important to ensure a stable particle beam. Altogether, synchrotron radiation facilities are used in many different disciplines and are very important to find solutions for today’s challenges.
The heart of a synchrotron radiation facility is a storage ring, in which electrons circle at a constant energy. Deflecting their trajectory using magnetic fields generates electromagnetic waves, i.e., synchrotron radiation. For more information about the setup and the functionality of synchrotron radiation facilities, please refer to [
7].
All synchrotron radiation sources worldwide are limited in spectral brightness [
1]. However, a new (fourth) generation of synchrotron sources has been developed based on stronger focusing attices (‘Multi-Bend Achromats’), which generate highly parallel and narrow particle beams characterised by high-intensity X-rays [
7]: highly brilliant light sources. Several synchrotron radiation facilities are already working with new or upgraded machines, e.g., MAX IV (Lund, Sweden), SIRIUS (Campinas, Sao Paolo, Brazil) or ESRF-EBS (Grenoble, France). Many other facilities ike Diamond (Oxfordshire, England), SOLEIL (Saint-Aubin, France), SPring-8 (Sayo Town, Hyōgo Prefecture, Japan) or DESY (German Electron Synchrotron, Hamburg, Germany) are discussing upgrades [
8].
At DESY, the current PETRA IV project aims at upgrading the present synchrotron radiation source PETRA III to PETRA IV, which will be an ultralow-emittance source and diffraction limited up to X-rays of 10 keV. Thus, its X-rays will be used for 3D microscopy of biological, chemical, and physical structures and processes under realistic conditions considering time scales down to the sub-nanosecond regime. The analysed length scales will vary from atomic dimensions to millimetres [
1].
The PETRA IV project started in spring 2016. After finishing the conceptual design phase by publishing the conceptual design report in November 2019, the technical design phase will probably last until December 2022. The construction work is planned to be initiated at the beginning of 2025 and PETRA IV is expected to start operation in January 2027. For more information about the PETRA IV project and its current status please refer to [
9,
10,
11,
12].
The currently operating PETRA III synchrotron is installed in a tunnel built in 1976, which has a large circumference of 2.304 km [
1,
11]. This tunnel will also be used for the upgraded machine, which is why the dimensions of the girder structures studied here followed the PETRA III girder geometry. The layout of PETRA IV consists of eight arcs of 201.6 m length each and eight long sections, of which four are 108 m long and the remaining four 64.5 m (
Figure 1). One arc is composed of eight identical cells, each of 26.2 m length. In each cell, five girders of 1 m, 3 m, and 6 m length are planned to support the magnets (
Figure 2) [
11]. The present study exemplarily focussed on the 3 m girder.
A high particle beam stability is essential to obtain a low-emittance and diffraction limited storage ring [
14]. It depends, aside from the thermal issues, on the transfer function of the ground vibration to the particle beam, in which the girder plays a key role. This vibration transmission path is illustrated in
Figure 3. Ground motions are inevitable. However, the vibrations reaching the particle beam
should be less than 10% of the particle beam size
to obtain the required particle beam stability and can be calculated as follows [
14]:
Thus,
depends on the ground vibrations
and on the transfer functions from the ground to the slab
, from the slab to the girder
, from the girder to the magnet
, and on the transfer function amplification due to the attice design
. The latter can be positively influenced by a fast orbit feedback factor
. In addition, in the case of low magnet–girder assembly eigenfrequencies, passive damping mechanisms (damping pads or materials) can reduce high vibration amplifications by a factor
as applied to the APS (Argonne National Laboratory, Lemont, IL, USA) [
15] or to the ESRF (Grenoble, France) [
16].
With regard to damping,
Figure 4 shows the vibration transmissibility of a sinusoidal excitation depending on the ratio of the exciting frequency
to the eigenfrequency
for different damping ratios
[
17]. Very soft supports lead to a
/
value larger than
, where the transmissibility is very low and the structure is isolated from the ground motion. Nevertheless, soft supports cannot be implemented in the accelerator machine because of the low tolerances between neighbouring girders. Damping mechanisms can be effectively applied to intermediate-stiffness supports that show
/
values around 1. However, if the structural eigenfrequency is significantly larger than the excitation frequency (
/
1), a high structural stiffness is present and damping mechanisms are not necessary because of the already low transmissibility [
17]. Therefore, stiff magnet–girder assemblies with a high 1st eigenfrequency are inevitable to reach a high particle beam stability.
A published overview on magnet–girder assemblies of different accelerator machines worldwide showed that the 1st eigenfrequencies of the magnet–girder assemblies vary from about 10 Hz of the 3rd generation machines (ESRF, APS) and about 20 Hz for SPring-8 or SSRF (Shanghai, People’s Republic of China) to above 40 Hz for the new-generation light sources (SOLEIL, ESRF-EBS) [
14]. Measurements of the currently installed PETRA III magnet–girder assembly revealed a 1st eigenfrequency of about 35 Hz and a 1st magnet mode at 25 Hz [
18]. An equipped PETRA III girder installed in the PETRA tunnel is displayed in
Figure 5.
Regarding the girder design and its boundary conditions, a compromise between stability (vibration, temperature) and adjustability (alignment) has to be made. On the one hand, a large number of girder support points increases the stiffness and thus the 1st eigenfrequency [
19]. On the other hand, an over-determined system of more than three support points (assuming that at each support point, all six degrees of freedom are fixed) is difficult to align, especially considering the small tolerances. In addition, the thermal deformation of the girder tends to increase with a rising support point number [
14]. While the vertical position of the girder is well defined by three points, more than three support points lead to an over-defined system and may hinder a symmetrical horizontal expansion. However, thermal issues are not addressed within this study. Regarding the girder alignment systems, motorised jacks and cam movers (e.g., applied to the PETRA III girder) have been used. In the latest magnet–girder assemblies, manual adjustments are more present, e.g., wedge jacks (APS, SSRF [
14]) or precision levellers made by AirLoc AG (
www.airloc.com, accessed on 31 August 2021) (e.g., SOLEIL [
20] or ESRF-EBS [
21]). Nevertheless, a support system allowing a girder adjustment at any time generally shows a lower stiffness than a non-adjustable support.
Summing up, there are many different specifications and demands that have to be taken into account to develop a new girder design. However, it is often not clearly understood how strongly the different specifications affect the stability of the magnet–girder assemblies. Therefore, different structural components and boundary conditions of a magnet–girder assembly were investigated in the present parametric study. The objective was to understand and quantify the impact of the varied parameters on the magnet–girder eigenfrequencies, before initiating the actual girder design process. Two different girder designs were taken into account. It was focused on the following parameters: the positioning and the mass of the magnets, the connection stiffness between the magnets and the girder and between the girder and the bases, the support point position of the girder, and the material properties of the girder and the bases. Based on the findings, the definition of the studied parameters can be improved to efficiently design magnet–girder assemblies with high eigenfrequencies required for a high particle beam stability.
The girder design and the boundary conditions followed the specifications defined for PETRA IV. It has to be noted, though, that the specifications considered here have been changed due to new insights of the PETRA IV project. Nevertheless, the findings of the parametric study are still relevant for future girder design processes in different synchrotron radiation facilities.
2. Materials and Methods
In the following, the finite element method (FEM) model of the studied magnet–girder assembly considering the specifications of the 3 m long PETRA IV girder is described. The parametric models were generated using the software Rhinoceros (version 6 SR10, Robert McNeel & Associates, Seattle, WA, USA) with its Plug-In Grasshopper (version 1.0.0007, Robert McNeel & Associates). All numerical models were solved using the solver OptiStruct (Altair Engineering Inc., Troy, MI, USA).
As the PETRA IV machine will be installed in the already available PETRA tunnel, the design space for the girders followed the dimensions of the currently installed PETRA III girders (cf.,
Figure 5).
Figure 6 shows the girder design space, which was equipped with eight magnets and connected to three bases at three points with the
x coordinates 0.05
, 0.50
, and 0.95
(
= 2900 mm, the girder length). The connection was realised using beam elements (CBEAM) with a diameter of 50 mm and a length of 20 mm, which were connected to the girder and the bases via rigid body elements (RBE3). The lower surface of the bases was considered as mounted.
For the girder, the bases, and the three connection beams, S235 was specified as a material characterised by a Young’s modulus E of 210,000 N , a density of 7.83 , and a Poisson’s ratio of 0.3.
The girder was equipped with eight magnets varying in width (cf.,
Figure 6) and mass (
Table 1), which were fixed to the upper girder surface. As the volume of each magnet
was fixed, its targeted mass
was obtained by defining an artificial density
for each magnet. In addition, a mass factor
q was defined as:
Since the magnet masses listed in
Table 1 were only first estimations, it was decided to set the factor
q equal to 1.15 in order to conservatively assume a slightly higher magnet mass, as the heavy magnets have a strong impact on the overall eigenfrequencies. However, in the present studies, the factor
q was varied to quantify the impact of the magnet mass on the magnet–girder eigenfrequencies.
Hereinafter, the impact of different components and boundary conditions of the magnet–girder assembly on the eigenfrequencies was analysed. Two different girder geometries were taken into account to assess the influence of the girder design on the analysed parameters (
Figure 7): (1) a hollow box girder that corresponded to the defined girder design space and possessed a wall thickness of 50 mm and (2) the PETRA III girder currently installed in the PETRA III machine, which has seven inner vertical ribs. The geometry of the 4200 mm long PETRA III girder was adapted to obtain a girder length of 2900 mm equal to the box girder length.
A mesh study of the FEM model varying the volume element (CTETRA) size from 10 mm to 45 mm in step sizes of 2.5 mm identified a sufficient element size applied to both the girder structures and the magnets. In this mesh study, only the girder structure equipped with magnets was considered. Thus, as the bases were neglected, a fixed support was defined at the three support point locations, i.e., all translations and rotations were inhibited. The element size, which showed result differences compared to the three previous element size steps of maximum 5%, was declared as sufficient.
During the parametric study, the following components and boundary conditions were altered and their impact on the first six eigenfrequencies of the magnet–girder assembly was investigated:
Magnet position height and connection;
Stiffness of the magnet–girder connection;
Magnet mass;
Girder support point position;
Stiffness of the girder support;
Material properties of the girder and the bases.
Table 2 lists the model components that were considered for each modal analysis, which required the definition of a modal load case.
2.1. Magnets
2.1.1. Magnet Position Height and Connection
The distance between the lower magnet surface and the girder was altered from 15 mm to 215 mm with a step size of 25 mm. In addition, the distances 0 mm—magnets fixed to the girder—and 290 mm were analysed. Similar to the magnet–girder assembly currently installed in the PETRA III accelerator (cf.,
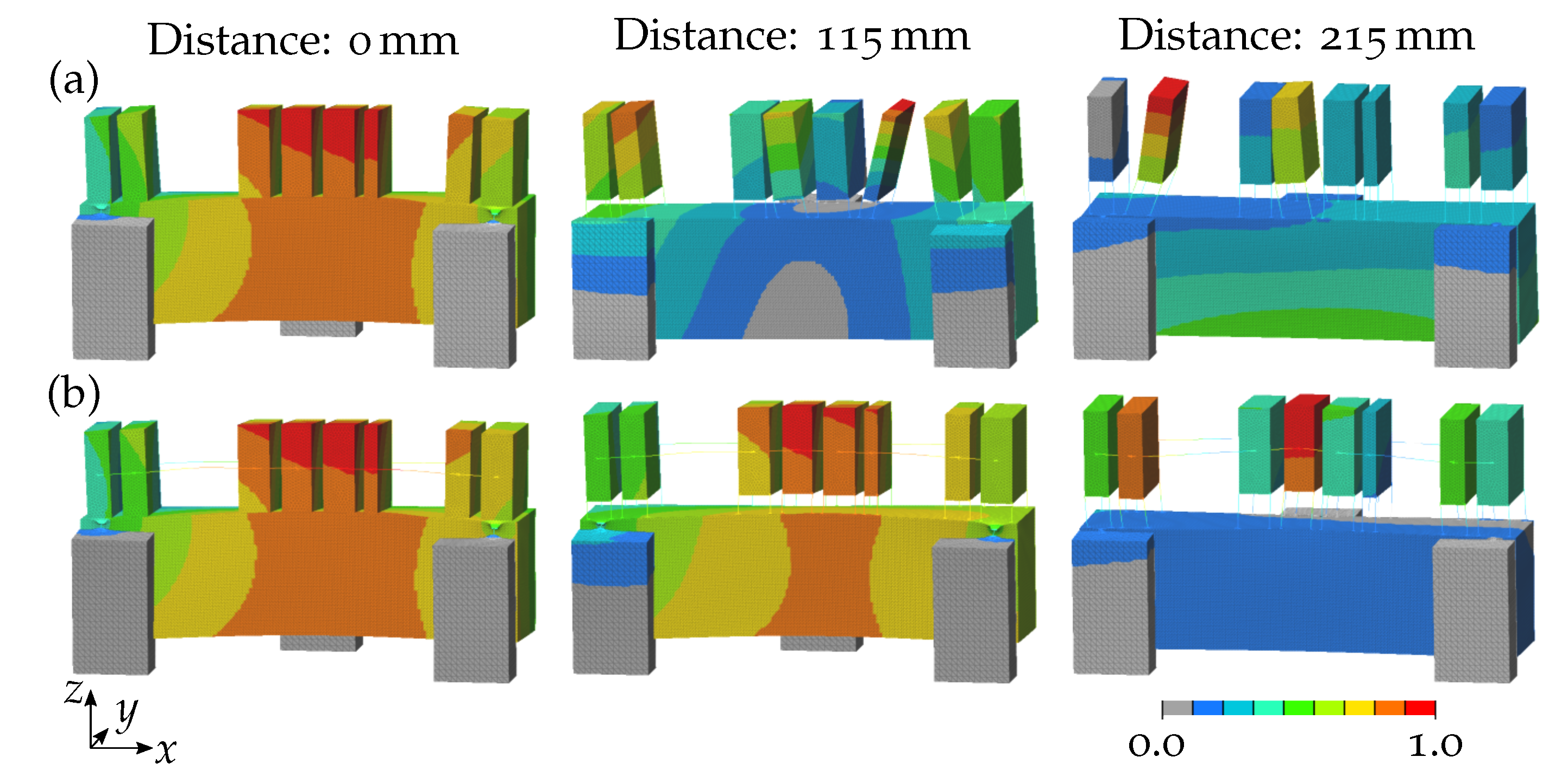
Figure 5), in which each magnet is connected to the girder via four screws embedded in resin, the magnets were positioned on the girder using four beams of 50 mm diameter each. The analyses were conducted for both the magnets positioned individually and the magnets connected to each other. The latter was realised using beams of 50 mm diameter that were fixed to the middle node of the front and back side of each magnet. All beams were connected to the magnets and the girder via RBE3.
Figure 8 shows exemplarily the model assembly for the position height study of the connected magnets.
2.1.2. Stiffness of the Connection between Girder and Magnet
The diameter of the beams connecting the magnets to the girder was varied from 5 mm to 100 mm with a step size of 5 mm to investigate the impact of the connection stiffness on the magnet–girder assembly eigenfrequencies. A distance between magnets and girder of 215 mm was assumed following the magnet position height of the PETRA III girder.
The fixation of a magnet on the girder can be abstracted as a frame composed of four elastic posts (height
, bending stiffness
, with
I denoting the second moment of inertia) and a rigid bar, on which a mass
m is fixed that can only move horizontally (
Figure 9). The stiffness
of one post depends on the applied force
F and the deflection
w [
22]:
As the four posts are connected side-by-side (i.e., parallel), the resulting stiffness of the frame
is the sum of the four single stiffnesses:
Thus, the 1st eigenfrequency of the frame
is
Based on these equations, the horizontal stiffness of the connection between girder and magnet was estimated using Equation (
4), in which the stiffness is multiplied by 4, since one magnet is supported by four parallel beams. The lowest frequency of a horizontal rigid magnet mode was estimated using Equation (
5) considering the mass of the heaviest magnet SF2AH (cf.,
Table 1). Regarding both equations, the magnets were abstracted as point masses on top of the beam neglecting the beam masses and the connection stiffness of the beam to the girder was assumed as infinitely stiff.
To estimate the vertical stiffness of the magnet support, the support was abstracted as four parallel massless rods, each of a length
and an extensional stiffness
provided with a mass
m at its end.
A is the cross section area of the rod.
Figure 10 shows one of these rods. A movement of the mass leads to an equal extension
of the rods. Thus, a restoring force acts on the mass. The total stiffness of the four rods is four times the rod stiffness
defined as [
22]:
2.1.3. Magnet Mass
To investigate the impact of the magnet mass on the magnet–girder assembly, the factor
q (cf., Equation (
2)) was varied from 0.10 to 2.05 with a step size of 0.15. The magnets were considered as fixed to the upper girder surface.
2.2. Girder Support
2.2.1. Support Point Position
In first published studies, the impact of the number and location of the girder support points on the eigenfrequencies were investigated [
23]. The results showed a significant 1st eigenfrequency increase with a rising support point number. At each support point, all six degrees of freedom were fixed. Consequently, installing more than three support points would result in an over-determined system, which makes an accurate adjustment of the equipped girder in the tunnel difficult or even impossible. Thus, different positions of only three support points were analysed here.
Since both investigated girder geometries showed a thin wall compared to the girder length, a consideration of the girder as a shell body with the same element size that was obtained in the mesh study was possible. Due to the high number of analysed combinations of support point positions, a solid girder would have involved very time-consuming computational effort. In contrast, the magnets were still considered as solids to consider not only their masses, but also their moments of inertia.
The x values of the support points were varied during the parametric study from 0.05 to 0.35 for point 1, from 0.65 to 0.95 for point 2, and from 0.3 to 0.7 for point 3 in step sizes of 0.1 , whereby symbolises the girder length. In addition, the study was conducted three times with different z values of the support points considering 900 mm, 700 mm, and 500 mm, which resulted in a total of 243 analysed support point position combinations.
Figure 11 shows all support points that were considered. The front and back wall of the PETRA III girder were positioned at
y = 185.5 mm and
y = 462.5 mm, i.e., closer to the girder centre (cf.,
Figure 7a). Consequently, the support points at heights of 500 mm and 700 mm were moved by 185.5 mm in the
y direction (support points 1 and 2) and in the negative
y direction (support point 3) to be defined on the girder wall.
The support point combination which led to the highest 1st eigenfrequency was chosen. Subsequently, the model was calculated with the girder considered as a solid body to estimate the deviation of the shell model from the volume model.
2.2.2. Stiffness of the Girder Support
To study how the girder support stiffness affects the overall magnet–girder assembly eigenfrequencies, the diameter of the beams connecting girder and bases was varied from 5 mm to 100 mm in 5 mm steps.
Since only shear forces were relevant owing to the shortness of the beams, the shear stiffness
of the connection between girder and bases was estimated based on a cantilever beam with a point load
F (
Figure 12). The maximum deflection
at
x =
l is [
24]:
As the stiffness depends on the force and the deflection (cf., Equation (
3)), it is defined as:
where
G symbolises the shear modulus and
is defined as 3/4 for the circular cross section presented here with the area
A [
25].
2.3. Material Properties
In the previous studies, S235 was considered as a material for the girder and the bases. Here, the impact of the material properties on the eigenfrequencies was analysed by considering the materials aluminium, grey cast iron, spheroidal cast iron, and mineral cast for the girder and the bases (
Table 3). The results were compared to those obtained for the girder and bases made out of S235. Additionally, the ratio
defined as:
was considered as it can be seen as a parameter of how the Young’s modulus and the density, which are significant material properties, affect the eigenfrequency.
4. Discussion
The parametric study revealed a strong impact of all analysed components and boundary conditions on the eigenfrequencies of the magnet–girder assembly. Generally, the majority of the analysed relationships between the components and boundary conditions and the overall eigenfrequencies were independent of the studied girder geometries. In the following, the obtained results are discussed.
4.1. Magnets
The impact of the magnet position height and connection, the magnet connection stiffness, and the magnet mass on the magnet–girder assembly eigenfrequencies was studied. In the following, some comments on the obtained results are given.
Concerning the magnet position height and connection study, the strong eigenfrequency decrease from 0 mm to 15 mm height for the PETRA III girder resulted from the girder geometry. The beams connecting the magnets to the girder were fixed to the girder at the outer part of the upper surface that was not directly supported by the vertical girder walls, since those were slightly moved towards the girder centre (cf.,
Figure 7a). Consequently, already a slight ift of the magnets above the girder surface resulted in high stresses—and thus high deformations—in these areas. The connection stiffness of the magnets was reduced and so were the eigenfrequencies. This was not the case for the box girder, because the vertical girder walls were at the very ends of the upper girder surface, which is why the connection area to the beams was directly supported.
Regarding the study on the magnet connection stiffness, the analytical calculation of the magnet mode shape frequency was in the same order of the numerically obtained value, but still differed (5.7 Hz vs. 1.1 Hz). The differences can be explained by the simplification of the analytical model, in which the magnet volume was not considered. Consequently, the centre of mass was assumed to be lower than that in the numerical model and the magnet mass inertia was also neglected. In addition, the girder had a certain stiffness, which was abstracted to be infinite in the analytical model. However, since both obtained frequency values were still in the same order, the numerical value can be seen as plausible.
The analyses of the magnet mass impact on the eigenfrequencies showed that the 1st box girder eigenfrequency did not vary with increasing magnet mass. This highlights that the girder eigenfrequency can be independent of the magnet mass, if the corresponding mode shape is characterised by a global rotation around an axis as close as possible to the magnet axis (i.e., to the particle beam).
In summary, the study indicated that the following aspects positively influence (i.e., increase) the magnet–girder assembly eigenfrequencies:
A low position of the magnets (i.e., as close as possible to the upper girder surface);
Connecting the magnets to each other;
A high stiffness of the connection between girder and magnets (i.e., a high stiffness of the magnet alignment system);
A low magnet mass;
Mode shapes that show a global rotation around an axis close to the particle beam.
The currently installed PETRA III girder is equipped with magnets positioned high above the upper girder surface using an alignment system for each magnet (cf.,
Figure 5), which leads to a decrease of the overall eigenfrequencies as shown in the parametric study. For PETRA IV, a different magnet alignment system is planned to increase the magnet support stiffness and to avoid low frequency magnet eigenmodes.
Recently, the alignment system EASy has been invented at DESY [
26]. The EASy type A3 is discussed to be placed between the PETRA IV magnets and the girder. The alignment component is 234 mm high [
26], which implies a distance between magnets and girder similar to the maximum value of 215 mm that was analysed in the present parametric study. First experiments on the EASy A3 revealed a minimum horizontal stiffness of 50
and a vertical stiffness of slightly more than 1.0
[
27]. In contrast, the parametric study model, in which each magnet was connected to the girder using four beams with a cross section diameter of 50 mm, showed a magnet support stiffness of 311
horizontally and 7.7
vertically. These values are significantly higher than the measured stiffness for the EASy A3. Moreover, considering the analytical calculations based on Equation (
6), a vertical stiffness of 1000
would correspond to a beam diameter of about 18.1 mm. Following the relation between the beam cross section diameter and the 1st eigenfrequency displayed in
Figure 17, a cross section diameter of 18.1 mm would result in a 1st eigenfrequency of the magnet–girder assembly of about 13 Hz. However, it shall be noted again that this calculation is based on a simplified analytical model, which is why the estimated eigenfrequency value, as well as the calculated stiffness value of the magnet support have to be interpreted with caution. In further studies, the connection stiffness of the magnets positioned on the girder should be measured to ensure that reliable values are discussed.
At the MAX IV synchrotron radiation facility aboratory, all magnets positioned on one girder are linked in one large box to avoid low frequency magnet bending modes [
28]. The parametric study results also indicated that connecting the magnets raises the overall eigefrequencies, because rigid magnet modes at low frequencies are prevented. However, the implementation of a magnet connection is probably not possible for PETRA IV, because an easy and individual adjustment of all magnet installed in the tunnel is required. Consequently, the low frequency magnet bending modes have to be avoided by a very high stiffness of the alignment system.
4.2. Girder Support
The study of the girder support position and stiffness indicated that the following aspects increase the eigenfrequencies:
A high position of the support points;
A horizontal support point positioning in form of a large (isosceles) triangle;
A high girder support stiffness.
In different synchrotron machines worldwide, girders are fixed by using more than three support points (e.g., SOLEIL [
20] or NSLS-II [
29]). However, as already mentioned in
Section 2.2.1, more than three support points result in an over-determined system, which makes the girder alignment in the tunnel almost impossible. In addition, due to a possible ground settlement during the accelerator operation for many years, a girder alignment might be necessary. Therefore, only three support points were considered here.
Based on the results, the support points should be positioned in a large triangle involving an increased support span to obtain a higher stability and thus higher eigenfrequencies, which was also mentioned in a study on the APS-U girder design [
30]. Moreover, the support points should be placed as high as possible, which was also obtained in previous studies [
19,
23]. low support point locations would decrease the eigenfrequencies and increase the vibration amplitudes of the magnets in the case of resonance, which should be avoided to guarantee a high functionality of the particle accelerator.
In addition to the support point position, a high stiffness of the girder support system is essential, which is why an appropriate girder alignment system should be chosen. Apparently, wedge jacks and AirLoc AG’s precision levellers allow a high girder support stiffness, because they have been used in the latest magnet–girder assemblies (cf.,
Section 1). Regarding the APS-U girder, the implementation of stiff lateral pushers also rose the eigenfrequencies significantly [
31]. Once a girder is aligned, an additional clamping system can further increase the support stiffness and the magnet–girder assembly eigenfrequencies, as applied to the SOLEIL girder [
20,
32].
It is also important to note that a high stiffness of the bases, on which the girder is positioned, is necessary to obtain high eigenfrequencies. As shown in previous studies, a low base stiffness can strongly reduce eigenfrequencies [
23]. For this reason, the here considered bases were heavy and stable solid blocks, which can also be utilised for PETRA IV.
4.3. Material Properties
The material properties strongly influence the eigenfrequencies of the magnet–girder assembly. As eigenfrequencies are known to be proportional to the Young’s modulus and anti-proportional to the mass [
22], the observed high dependency of the 1st eigenfrequency on the ratio
was expectable. However, this effect seems to only fully apply to the simple box girder. The PETRA IV girder shows a more complex geometry involving an irregular material (mass) distribution. Consequently, not only the ratio
, but also the irregular mass distribution has a significant effect on the eigenfrequencies. Thus, it can be concluded that the consideration of the ratio
is very useful as a first approach to evaluate the effectiveness of a girder (bases) material, especially for simple girder structures, before further detailed analyses shall be carried out.
Aside from the ratio
, further material properties have to be taken into account for the decision on the girder/bases material: Girders placed next to an undulator should have a very low magnetisability to avoid an undesired impact on the undulator magnetic field. Furthermore, the temperature stability and corrodibility of a girder and bases material are important characteristics, because variations in the tunnel air temperature and humidity can cause corrosion and structural deformations. Although the PETRA IV tunnel temperature will be stabilised to ±1 K [
11], an outage is always possible, which is why the girder (and bases) material should show a high temperature stability. However, the temperature stability will not be analysed in this work.
Girders of different synchrotron facilities are made of materials such as carbon steel (ESRF-EBS [
21]) or ductile cast iron (APS-U [
31]). As the PETRA IV girder or bases might be made of grey or spheroidal cast iron, both materials were studied here. They showed a ratio
similar to S235 (cf.,
Table 3) and the small deviations in the ratio correlate with the 1st eigenfrequency deviations. Consequently, based on the eigenfrequency results using a steel girder, the eigenfrequency values for a cast iron girder can be estimated.
4.4. Outlook
In the following, different issues that should be investigated in further studies are mentioned.
As ground vibrations decrease with increasing frequency [
33], one of the common girder design objectives is to maximise the 1st eigenfrequency, which is why this study focused on this issue. However, in some cases, specific frequency values are precarious, e.g., due to the vibration of other components of the synchrotron radiation facility. Thus, the magnet–girder eigenfrequencies have to be shifted out of the range of these exciting frequencies in order to avoid resonance phenomena. In continuative studies, this issue could be addressed, e.g., by more explicitly investigating the sensitivity of the 1st magnet–girder eigenfrequency to varying boundary conditions or components.
In the majority of the modern synchrotron radiation facilities, the girders are not fixed at each support point. Instead, there are different girder support (alignment) systems that have more than three support points, but do not imply an over-constrained system, e.g., by using cam movers [
34]. Thus, an easy alignment is still possible. As there are different girder support systems utilised in synchrotron radiation facilities worldwide, a detailed investigation of the impact of the different support systems on the magnet–girder eigenfrequencies would perfectly complement the present study.
Another issue that could be analysed in further studies is the impact of horizontal movements (parallel to the particle beam) of the magnets on the magnet–girder eigenfrequencies. Oftentimes, the girder design process starts, although the magnet attice is not yet fully defined. Therefore, studying the sensitivity of the eigenfrequencies to lateral magnet movements might be interesting for many engineers working on girder designs.