Pressure Capacity Assessment of L-PBF-Produced Microchannel Heat Exchangers
Abstract
:1. Introduction
Motivation, Aims, and Objective
2. Literature Review
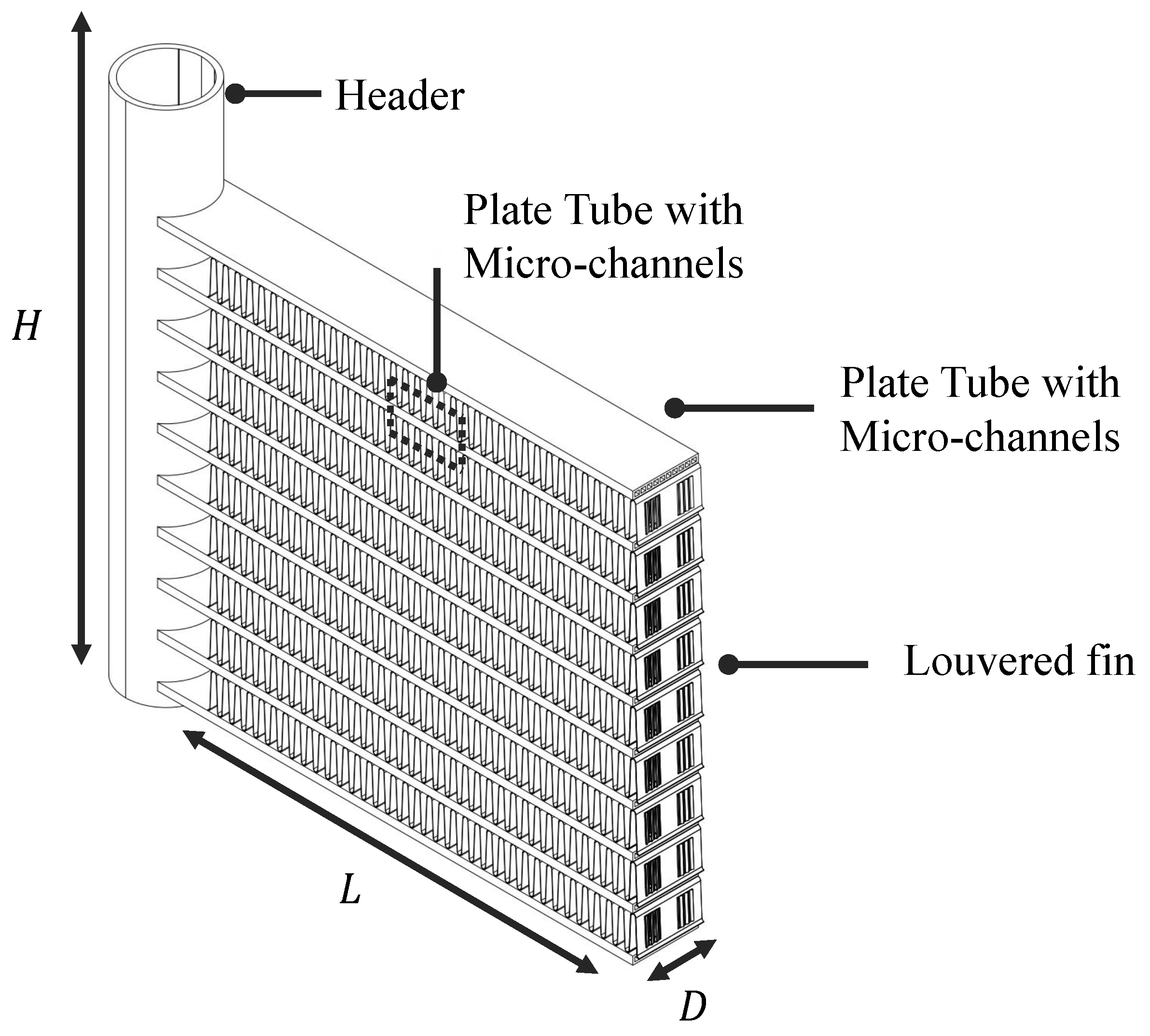
2.1. Introduction to MCHX
2.2. Introduction to L-PBF
2.3. Introduction to Powder Material AlSi10Mg Properties
3. Experiment Design and Methodology
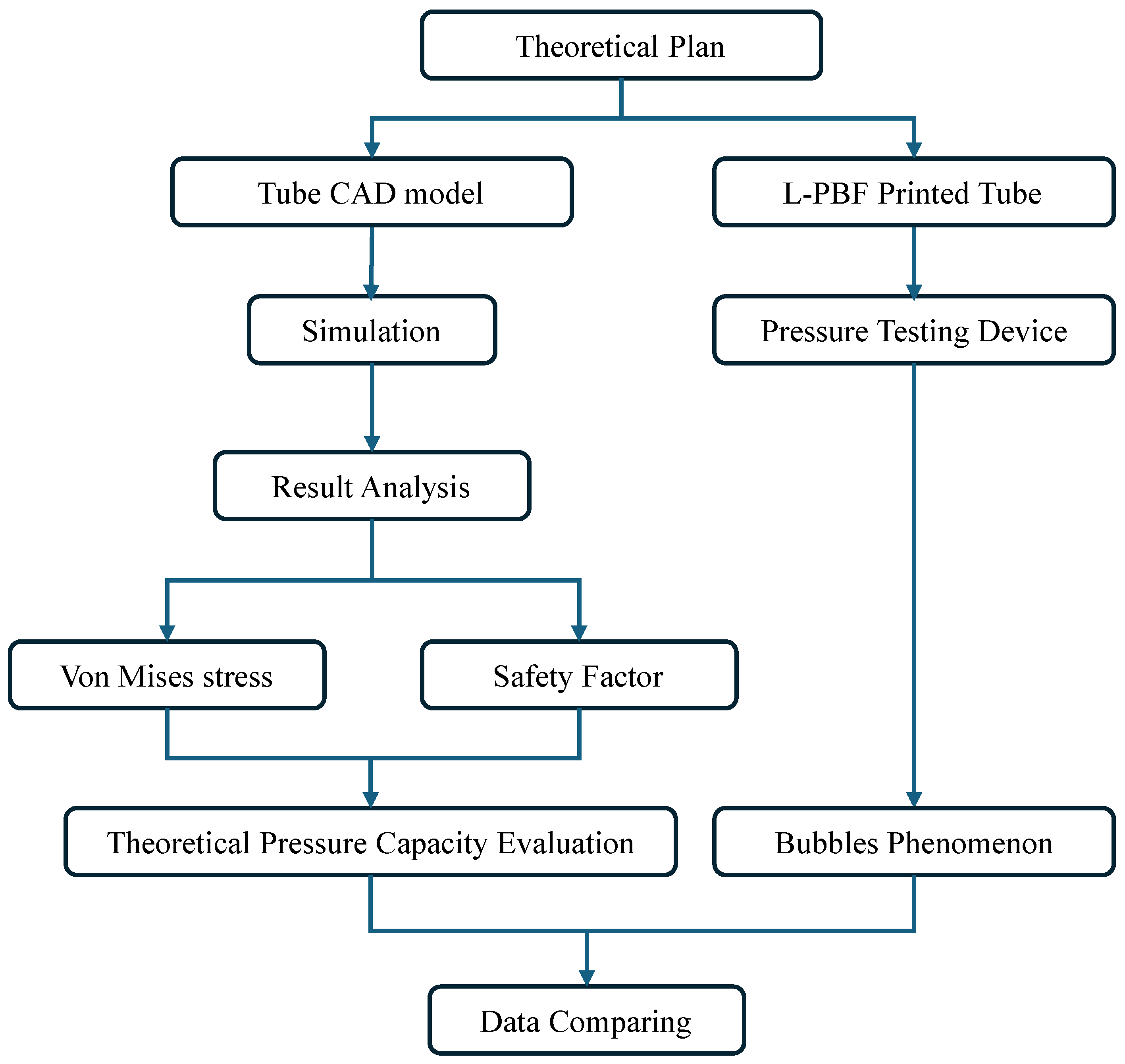
3.1. Experiment Workflow
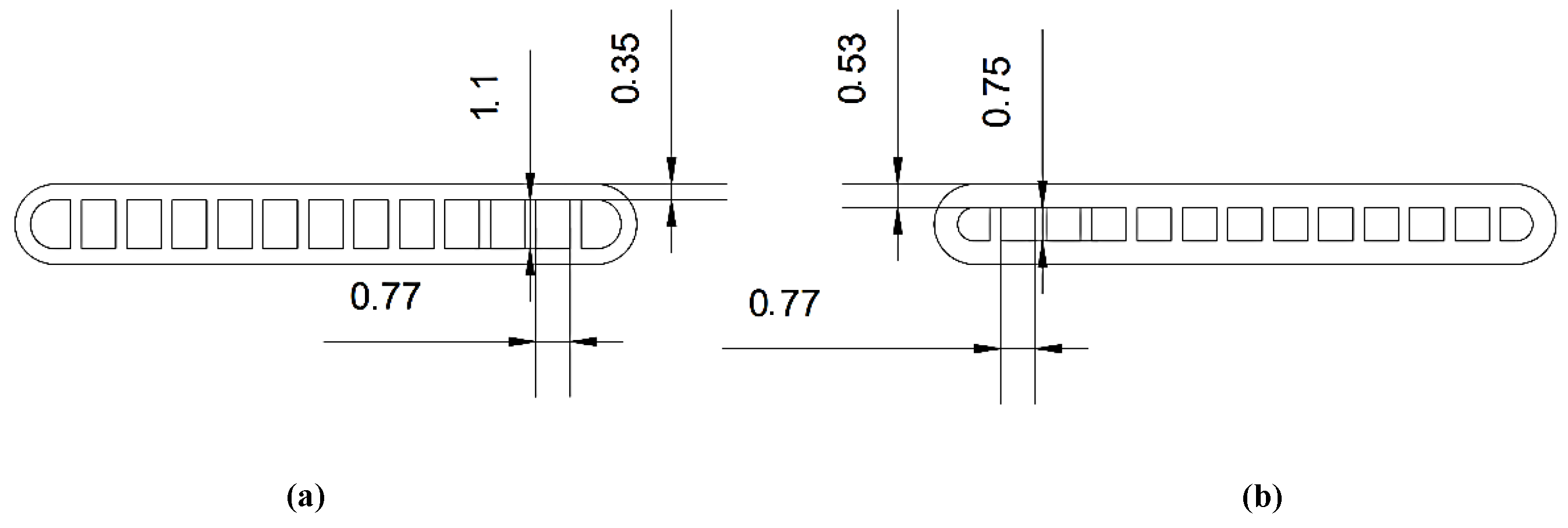
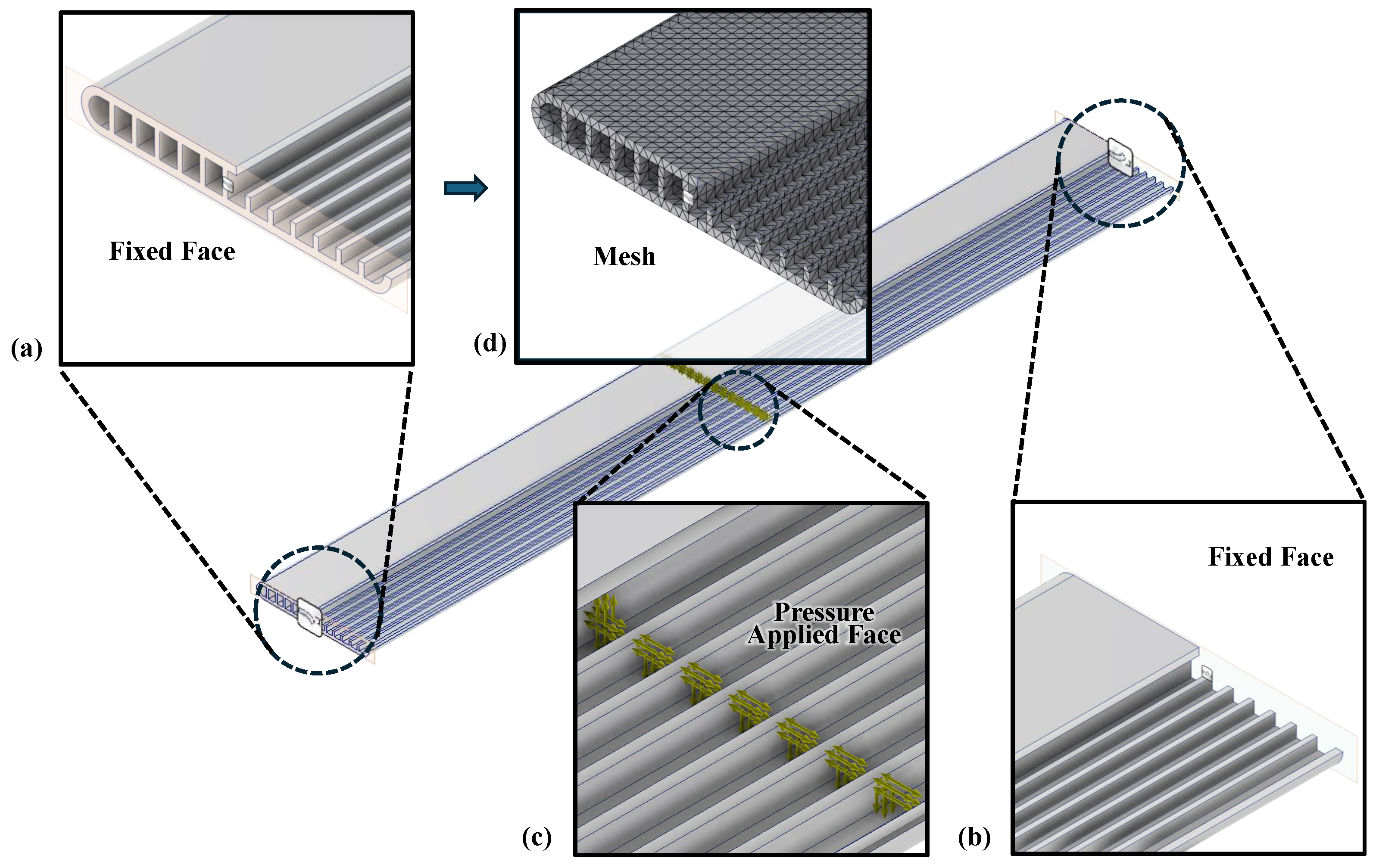
3.2. Simulation to Reflect the Pressure Capacity in Microchannel Tube
- Safe stress is the maximum allowable stress that ensures the component will perform reliably under expected loads.
- Yield strength is the stress at which the material begins to yield or deform elastically.
- Safety factor is a multiplier used to provide a margin of safety, typically greater than 1, to account for uncertainties and variability in the material and loading conditions.
- is the von Mises stress.
- , , and are the principal stresses
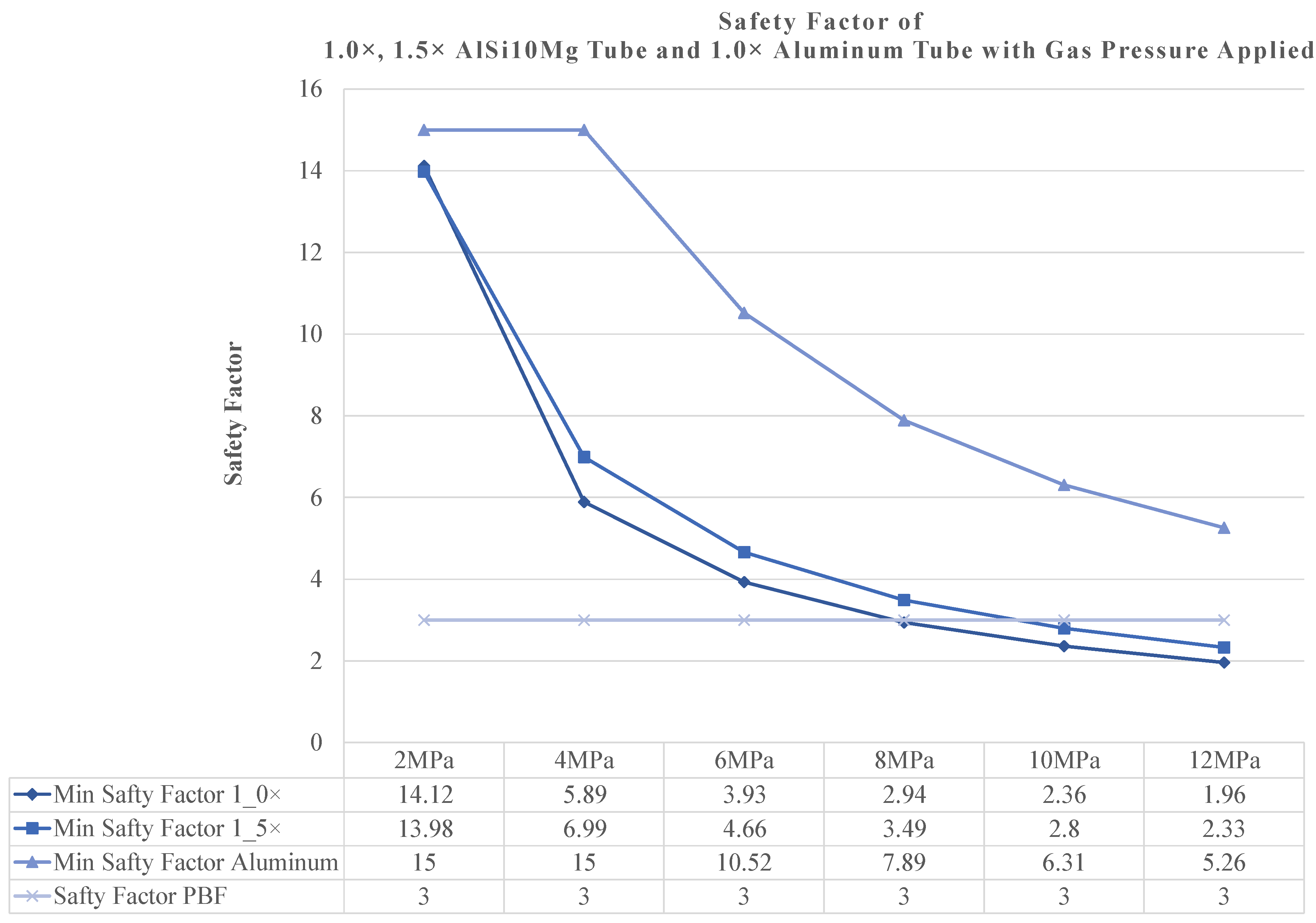
3.3. Simulation Result
4. Pressure Capacity Testing Experiment
4.1. Experiment Device Preparation
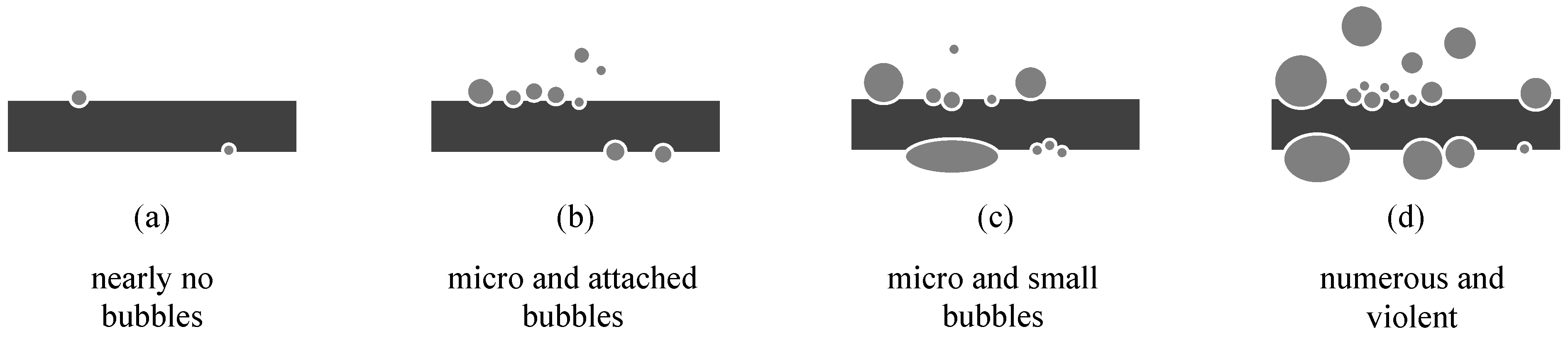
4.2. Experiment Method of Bubbles Phenomenon
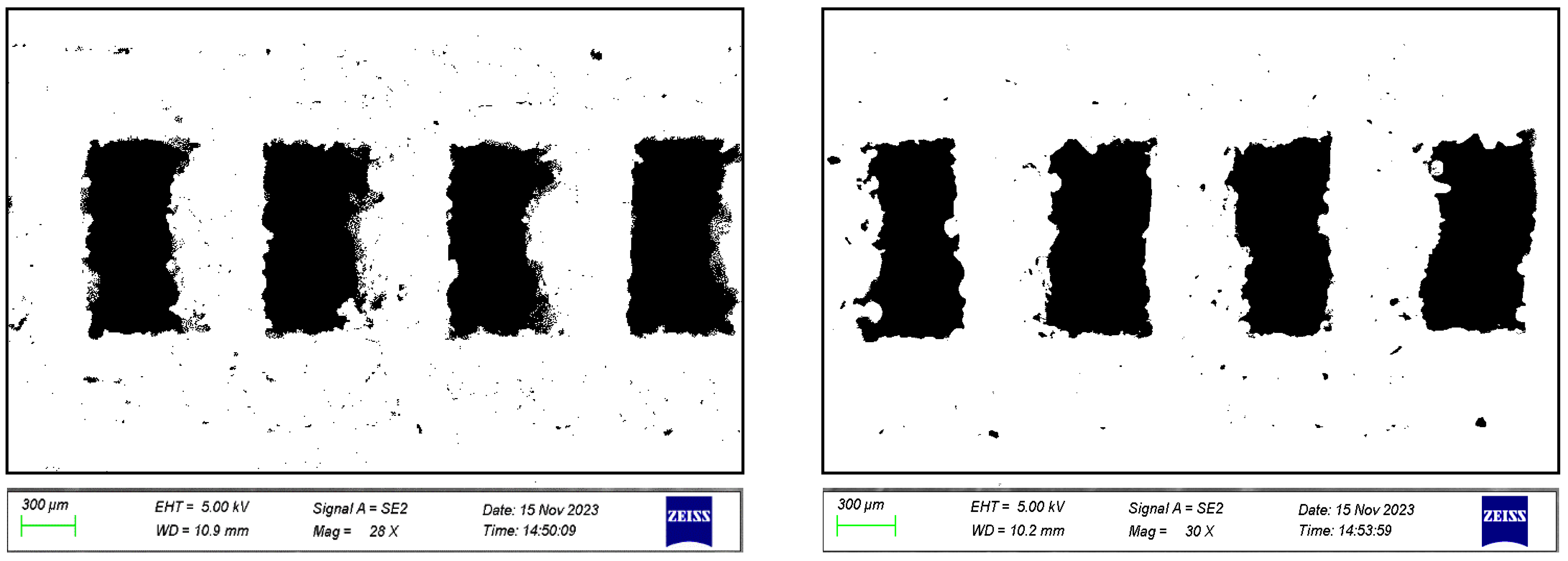
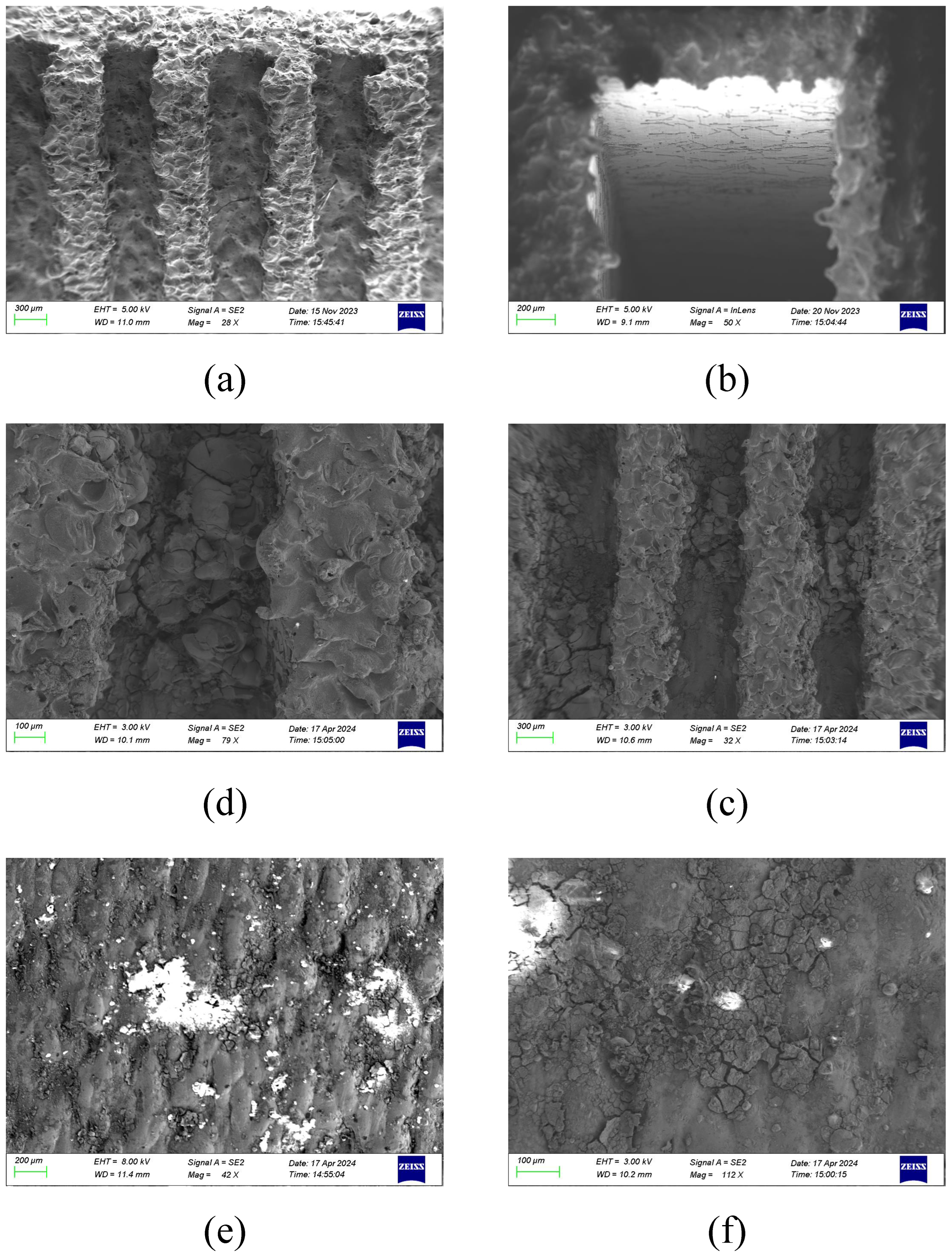
4.3. Tube Pressure Capacity Testing Experiment Result
4.4. Tube Pressure Capacity Testing Experiment Result Evaluation
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| L-PBF | laser powder bed fusion |
| MCHX | microchannel heat exchanger |
| PTD | pressure testing device |
| AT | acrylic tank |
| MPa | megapascal |
| SEM | scanning electron microscope |
Appendix A



Appendix B



References
- Hussain, A.R.J.; Alahyari, A.A.; Eastman, S.A.; Thibaud-Erkey, C.; Johnston, S.; Sobkowicz, M.J. Review of polymers for heat exchanger applications: Factors concerning thermal conductivity. Appl. Therm. Eng. 2017, 113, 1118–1127. [Google Scholar] [CrossRef]
- Zahir, M.Z.; Ahmed, A.; Akhtar, K.; Mehmood, A. Microchannel Heat Exchanger (MCHX) for Waste Heat Recovery Using Water and Organic Fluid. J. Appl. Emerg. Sci. 2023, 13, 67–75. [Google Scholar]
- Lei, H.; Li, C.; Meng, J.; Zhou, H.; Liu, Y.; Zhang, X.; Wang, P.; Fang, D. Evaluation of compressive properties of SLM-fabricated multi-layer lattice structures by experimental test and μ-CT-based finite element analysis. Mater. Des. 2019, 169, 107685. [Google Scholar] [CrossRef]
- Du Plessis, A. Porosity in laser powder bed fusion. In Fundamentals of Laser Powder Bed Fusion of Metals; Elsevier: Amsterdam, The Netherlands, 2021; pp. 155–178. [Google Scholar]
- Morano, C.; Pagnotta, L. Additive manufactured parts produced by selective laser sintering technology: Porosity formation mechanisms. J. Polym. Eng. 2023, 43, 537–555. [Google Scholar] [CrossRef]
- Stránský, O.; Beránek, L.; Pathak, S.; Kaufman, J.; Böhm, M.; Kopeček, J.; Holešovský, F.; Petrášek, Š.; Hlavňková, L.; Soukup, Z. Porosity and microstructure of L-PBF printed AlSi10Mg thin tubes in laser shock peening. J. Mater. Res. Technol. 2023, 27, 1683–1695. [Google Scholar] [CrossRef]
- Kleinschmit, N.N. A Shock Tube Technique for Blast Wave Simulation and Studies of Flow Structure Interactions in Shock Tube Blast Experiments. Master’s Thesis, University of Nebraska-Lincoln, Lincoln, NE, USA, 2011. [Google Scholar]
- Kumar, R.; Nedungadi, A. Using gas-driven shock tubes to produce blast wave signatures. Front. Neurol. 2020, 11, 90. [Google Scholar] [CrossRef] [PubMed]
- Zou, J.; Hirokawa, T.; An, J.; Huang, L.; Camm, J. Recent advances in the applications of machine learning methods for heat exchanger modeling—A review. Front. Energy Res. 2023, 11, 1294531. [Google Scholar] [CrossRef]
- Chmielewska, A.; Wysocki, B.; Kwaśniak, P.; Kruszewski, M.J.; Michalski, B.; Zielińska, A.; Adamczyk-Cieślak, B.; Krawczyńska, A.; Buhagiar, J.; Święszkowski, W. Heat treatment of NiTi alloys fabricated using laser powder bed fusion (LPBF) from elementally blended powders. Materials 2022, 15, 3304. [Google Scholar] [CrossRef] [PubMed]
- Khairallah, S.A.; Anderson, A.T.; Rubenchik, A.; King, W.E. Laser powder-bed fusion additive manufacturing: Physics of complex melt flow and formation mechanisms of pores, spatter, and denudation zones. Acta Mater. 2016, 108, 36–45. [Google Scholar] [CrossRef]
- Ceccanti, F.; Giorgetti, A.; Arcidiacono, G.; Citti, P. Laser powder bed fusion: A review on the design constraints. In Proceedings of the IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2021; Volume 1038, p. 012065. [Google Scholar]
- Singh, R.; Gupta, A.; Tripathi, O.; Srivastava, S.; Singh, B.; Awasthi, A.; Rajput, S.K.; Sonia, P.; Singhal, P.; Saxena, K.K. Powder bed fusion process in additive manufacturing: An overview. Mater. Today Proc. 2020, 26, 3058–3070. [Google Scholar] [CrossRef]
- Zhang, J.; Li, B. The Influence of Laser Powder Bed Fusion (L-PBF) Process Parameters on 3D-Printed Quality and Stress–Strain Behavior of High-Entropy Alloy (HEA) Rod-Lattices. Metals 2022, 12, 2109. [Google Scholar] [CrossRef]
- Kempen, K.; Thijs, L.; Van Humbeeck, J.; Kruth, J.-P. Mechanical properties of AlSi10Mg produced by selective laser melting. Phys. Procedia 2012, 39, 439–446. [Google Scholar] [CrossRef]
- Wu, Z.; Narra, S.P.; Rollett, A. Exploring the fabrication limits of thin-wall structures in a laser powder bed fusion process. Int. J. Adv. Manuf. Technol. 2020, 110, 191–207. [Google Scholar] [CrossRef]
- Rastan, H.; Abdi, A.; Hamawandi, B.; Ignatowicz, M.; Meyer, J.P.; Palm, B. Heat transfer study of enhanced additively manufactured minichannel heat exchangers. Int. J. Heat Mass Transf. 2020, 161, 120271. [Google Scholar] [CrossRef]
- Gong, H.; Rafi, K.; Gu, H.; Starr, T.; Stucker, B. Analysis of defect generation in Ti–6Al–4V parts made using powder bed fusion additive manufacturing processes. Addit. Manuf. 2014, 1, 87–98. [Google Scholar] [CrossRef]
- Du Plessis, A.; Yadroitsava, I.; Yadroitsev, I. Effects of defects on mechanical properties in metal additive manufacturing: A review focusing on X-ray tomography insights. Mater. Des. 2020, 187, 108385. [Google Scholar] [CrossRef]
- Rahman, M.E.; Weibel, J.A. Influence of convective heat transfer and wall thermal capacity on dynamic interactions between wall temperature and pressure drop oscillations during microchannel flow boiling. Int. J. Heat Mass Transf. 2024, 221, 125111. [Google Scholar] [CrossRef]
- Silva, E.C.; Candiango, J.A.; Rodrigues, S.J.; Sampaio, Á.M.; Pontes, A.J. Hybrid Manufacturing of Aluminium Parts Combining Additive and Conventional Technologies—Mechanical and Thermal Properties. J. Manuf. Mater. Process. 2022, 6, 40. [Google Scholar] [CrossRef]
- Lv, F.; Shen, L.; Liang, H.; Xie, D.; Wang, C.; Tian, Z. Mechanical properties of AlSi10Mg alloy fabricated by laser melting deposition and improvements via heat treatment. Optik 2019, 179, 8–18. [Google Scholar] [CrossRef]
- Jing, C.; Hou, W.; Wang, X.; Chu, S.; Yang, Z. Microstructure, porosity and mechanical properties of selective laser melted AlSi10Mg. Chin. J. Aeronaut. 2020, 33, 2043–2054. [Google Scholar]
- Lind, N.C. Consistent partial safety factors. J. Struct. Div. 1971, 97, 1651–1669. [Google Scholar] [CrossRef]
- Chai, L.; Xia, G.; Zhou, M.; Li, J.; Qi, J. Optimum thermal design of interrupted microchannel heat sink with rectangular ribs in the transverse microchambers. Appl. Therm. Eng. 2013, 51, 880–889. [Google Scholar] [CrossRef]
- Wang, Y.-Z.; Li, G.-Q.; Wang, Y.-B.; Lyu, Y.-F. Simplified method to identify full von Mises stress-strain curve of structural metals. J. Constr. Steel Res. 2021, 181, 106624. [Google Scholar] [CrossRef]
- Amstutz, S.; Novotny, A.A. Topological optimization of structures subject to von Mises stress constraints. Struct. Multidiscip. Optim. 2010, 41, 407–420. [Google Scholar] [CrossRef]
- Mischke, C.R. Fitting Weibull strength data and applying it to stochastic mechanical design. J. Mech. Des. 1992, 114, 29–34. [Google Scholar] [CrossRef]







| Parameter | Value |
|---|---|
| Orientation | 90° micro-rod (Parallel to the vertical direction) |
| Laser power | 190 W |
| Scanning speed | 900 mm/s |
| Displacement rate | 0.1 mm/min |
| Tensile strength | 509 MPa (approx.) |
| Test result | Elastic modulus in 73 GPa (50% of forged samples) |
| Tag | Value |
|---|---|
| Thermal Conductivity | |
| Specific heat | 0.730 J/g·°C |
| Thermal expansion coefficient | 21.800 µm/m·°C |
| Young’s modulus | 54.500 GPa |
| Poisson’s ratio | 0.25 |
| Shear modulus | 20.960 MPa |
| Density | 2.640 g/cm3 |
| Damping coefficient | 0.002(0) |
| Yield strength | 102.100 MPa |
| Tensile strength | 296.000 MPa |
| Stage | Pressure Gauge Value | Time Step | Important Time Step | Leakage Level | Bubbles Performance |
|---|---|---|---|---|---|
| Stage 1 | 1.0 MPa | 0 s | 0 min 34 s | medium | numerous and violent |
| Stage 2 | 1.2 MPa | 10 min | - | high | numerous and violent |
| Stage 3 | 1.4 MPa | 20 min | - | high | numerous and violent |
| Stage 4 | 1.6 MPa | 30 min | - | extreme | numerous and violent |
| Stage 5 | 1.8 MPa | 40 min | - | extreme | numerous and violent |
| Stage 6 | 2.0 MPa | 60 min | - | extreme | numerous and violent |
| Stage | Pressure Gauge Value | Time Step | Important Time Step | Leakage Level | Bubbles Performance |
|---|---|---|---|---|---|
| Stage 1 | 1.0 MPa | 0 s | - | No | Nearly-no-bubbles |
| Stage 2 | 1.2 MPa | 10 min | 10 min 23 s | slight | micro and attached bubbles |
| Stage 3 | 1.4 MPa | 20 min | 20 min 12 s | slight | micro and small bubbles |
| Stage 4 | 1.6 MPa | 30 min | - | low | micro and small bubbles |
| Stage 5 | 1.8 MPa | 40 min | - | low | micro and small bubbles |
| Stage 6 | 2.0 MPa | 60 min | - | low | micro and small bubbles |
| Stage | Pressure Gauge Value | Time Step | Important Time Step | Leakage Level | Bubbles Performance |
|---|---|---|---|---|---|
| Stage 1 | 1.0 MPa | 0 s | - | No | Nearly No Bubbles |
| Stage 2 | 1.2 MPa | 10 min | - | No | Nearly No Bubbles |
| Stage 3 | 1.4 MPa | 20 min | - | No | Nearly No Bubbles |
| Stage 4 | 1.6 MPa | 30 min | - | No | Nearly No Bubbles |
| Stage 5 | 1.8 MPa | 40 min | - | No | Nearly No Bubbles |
| Stage 6 | 2.0 MPa | 60 min | - | slight | micro and attached bubbles |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
An, J.; Guo, L.; Zou, J.; Zhang, K.; Zhong, Y.; Liu, T.; Huang, L.; Chen, Y. Pressure Capacity Assessment of L-PBF-Produced Microchannel Heat Exchangers. Inventions 2024, 9, 97. https://doi.org/10.3390/inventions9050097
An J, Guo L, Zou J, Zhang K, Zhong Y, Liu T, Huang L, Chen Y. Pressure Capacity Assessment of L-PBF-Produced Microchannel Heat Exchangers. Inventions. 2024; 9(5):97. https://doi.org/10.3390/inventions9050097
Chicago/Turabian StyleAn, Jiabao, Luyao Guo, Junjia Zou, Keliang Zhang, Yiheng Zhong, Taimingwang Liu, Long Huang, and Yi Chen. 2024. "Pressure Capacity Assessment of L-PBF-Produced Microchannel Heat Exchangers" Inventions 9, no. 5: 97. https://doi.org/10.3390/inventions9050097
APA StyleAn, J., Guo, L., Zou, J., Zhang, K., Zhong, Y., Liu, T., Huang, L., & Chen, Y. (2024). Pressure Capacity Assessment of L-PBF-Produced Microchannel Heat Exchangers. Inventions, 9(5), 97. https://doi.org/10.3390/inventions9050097







