Pavement Analysis with the Consideration of Unbound Granular Material Nonlinearity
Abstract
:1. Introduction
1.1. Overview
1.2. Background on the Granular Layers’ Performance
1.3. Study’s Objective
2. Resilient Modulus
- σd = σ1 − σ3, the deviator stress.
- εr, the recoverable strain.
- θ, the bulk stress in kPa (calculated as σ1 + 2σ3).
- pa, the atmospheric pressure in kPa.
- k1, k2, k3, regression constants.
- , the octahedral stress in kPa, calculated through the principal normal stresses as follows:
3. Methodology
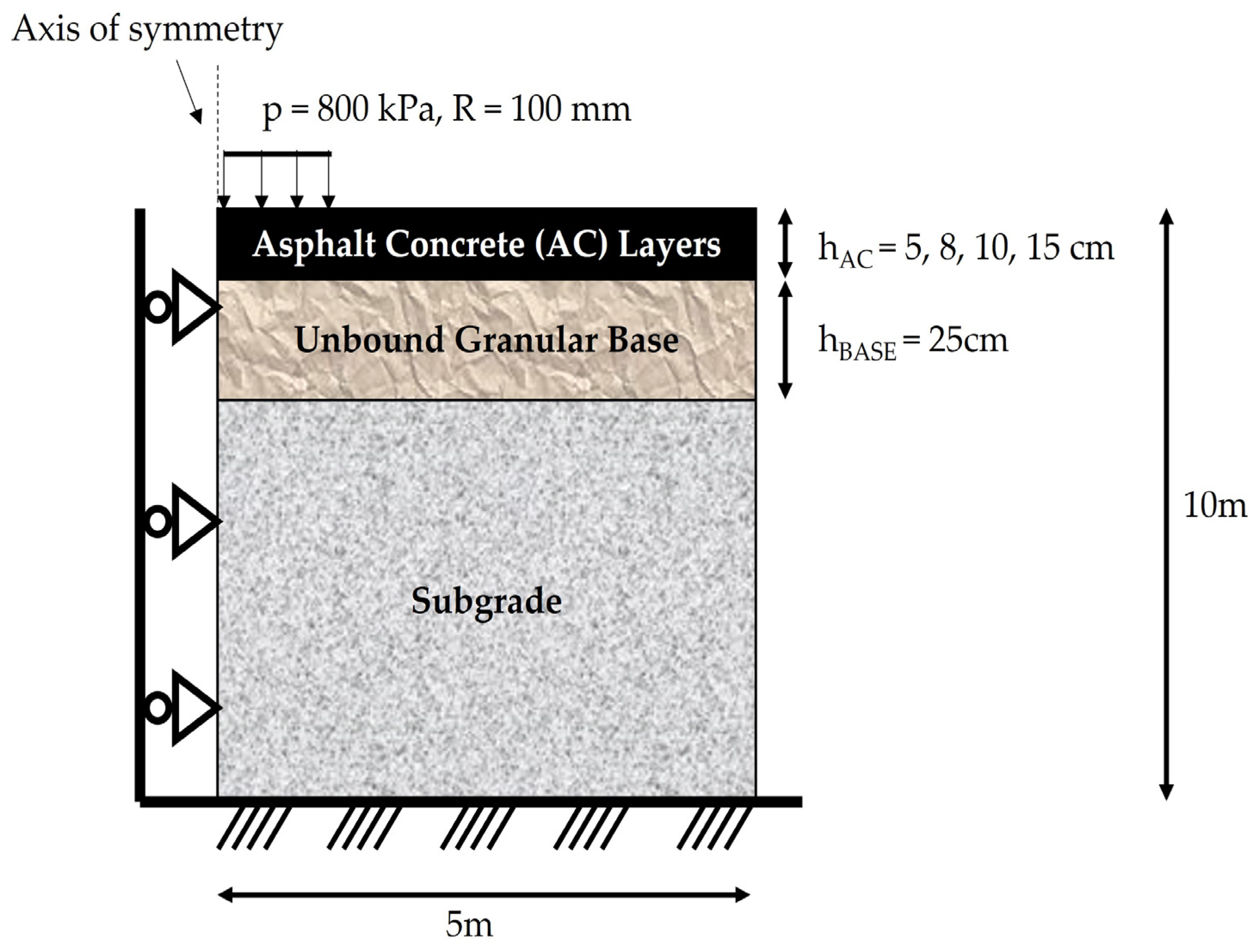
3.1. Description of the Developed Model
- (i)
- A unified AC layer with variable thicknesses to cover many cases from roads serving lower traffic volumes to more heavily trafficked pavements. The interest in low-volume roads (LVRs) is well-grounded based on other international studies linking the impact of material nonlinearity with this type of road, e.g., [18,32].
- (ii)
- A 25 cm thick unbound granular base (crushed stone material).
- (iii)
- A semi-infinite layer for the subgrade consisting of natural gravel.
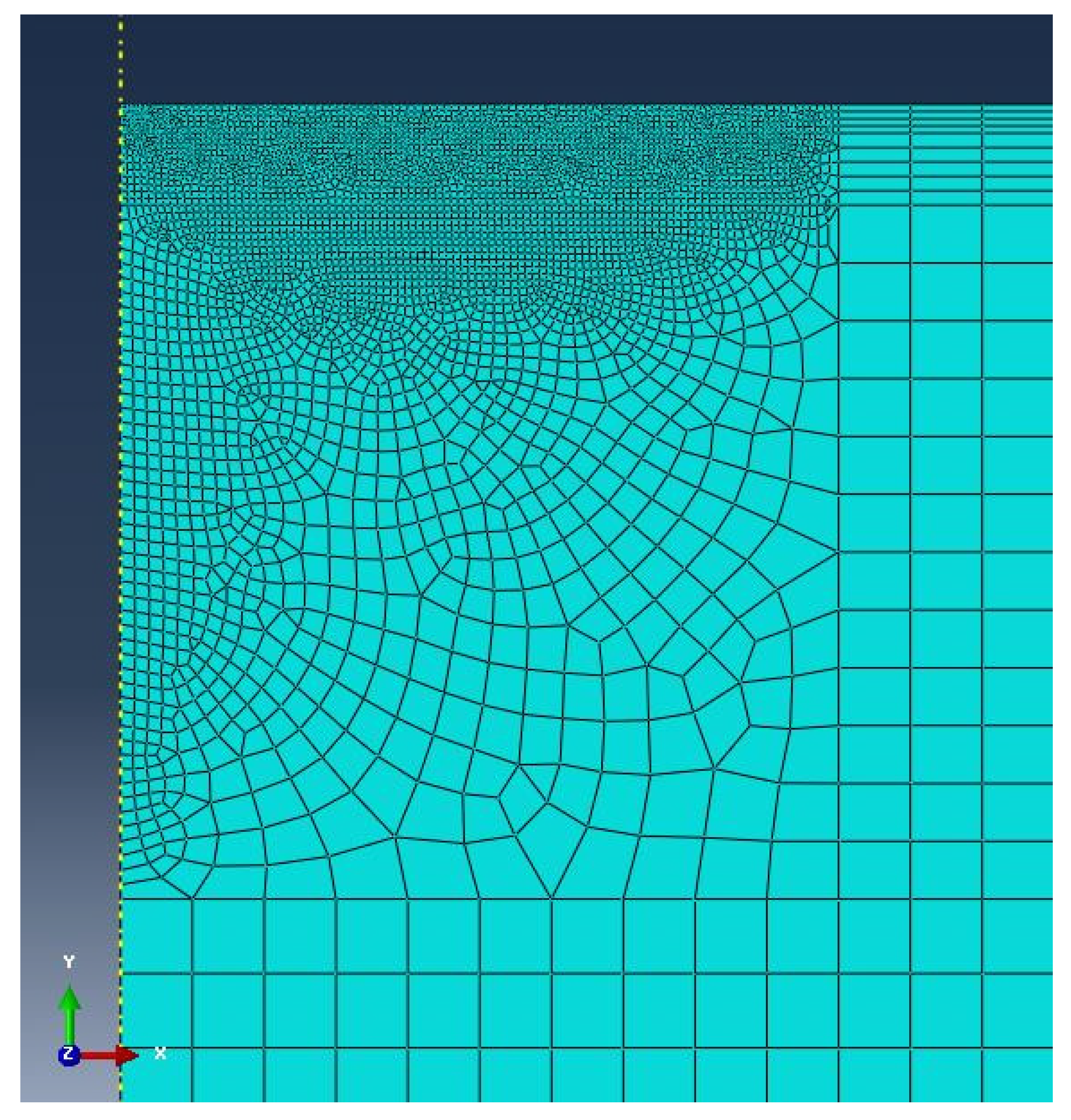
3.2. Response Calculations and Modeling Phases
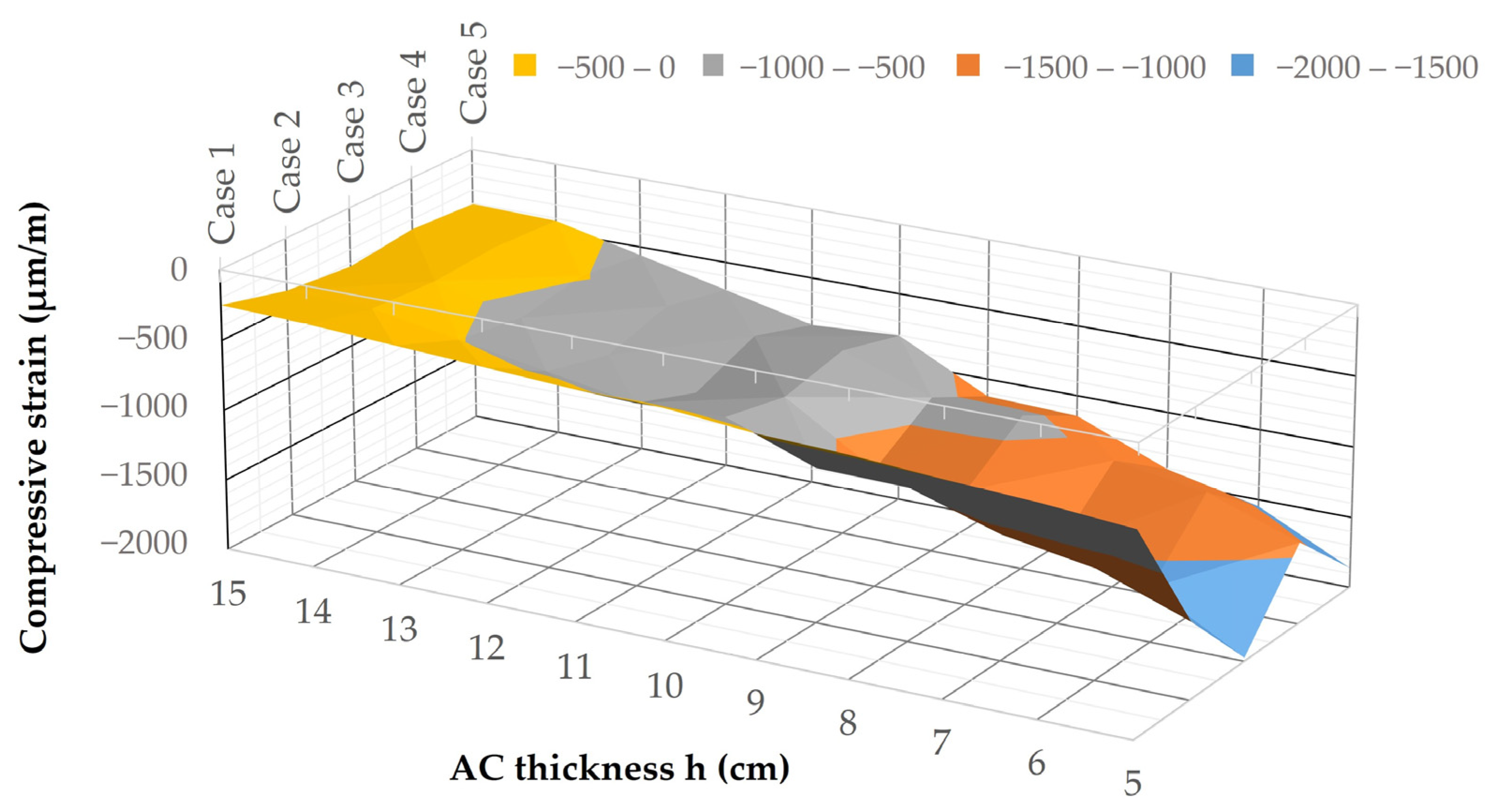
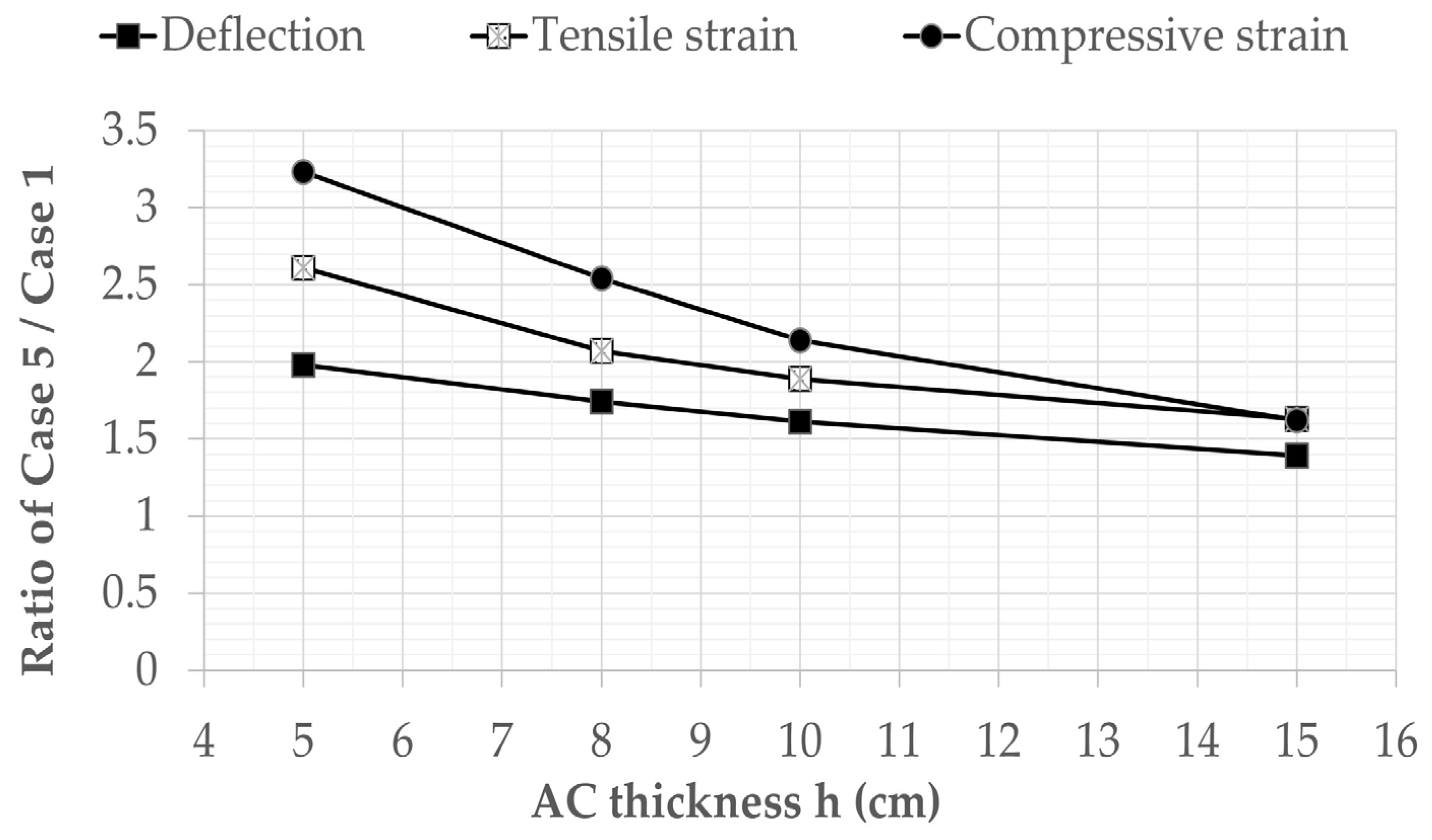
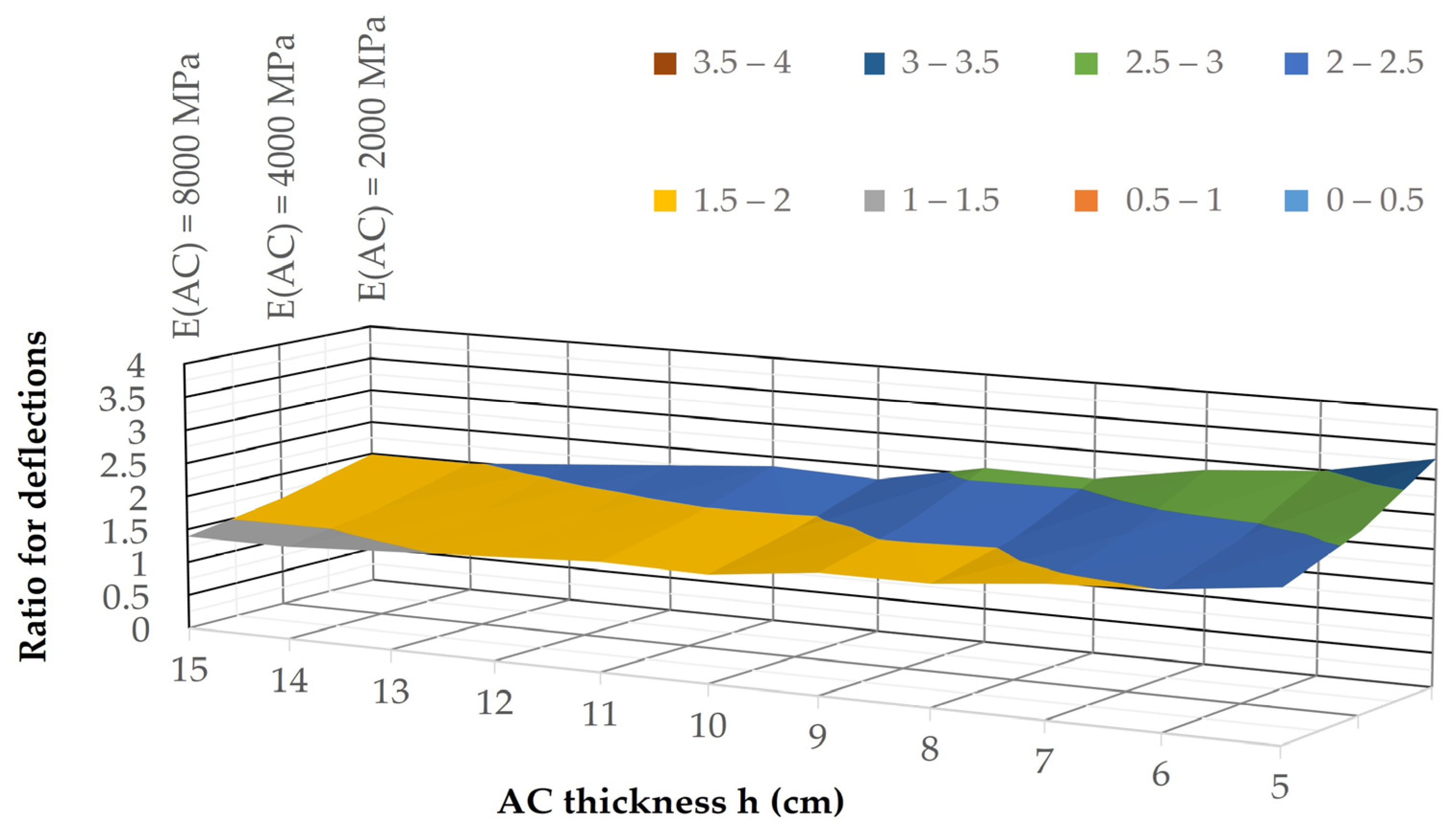
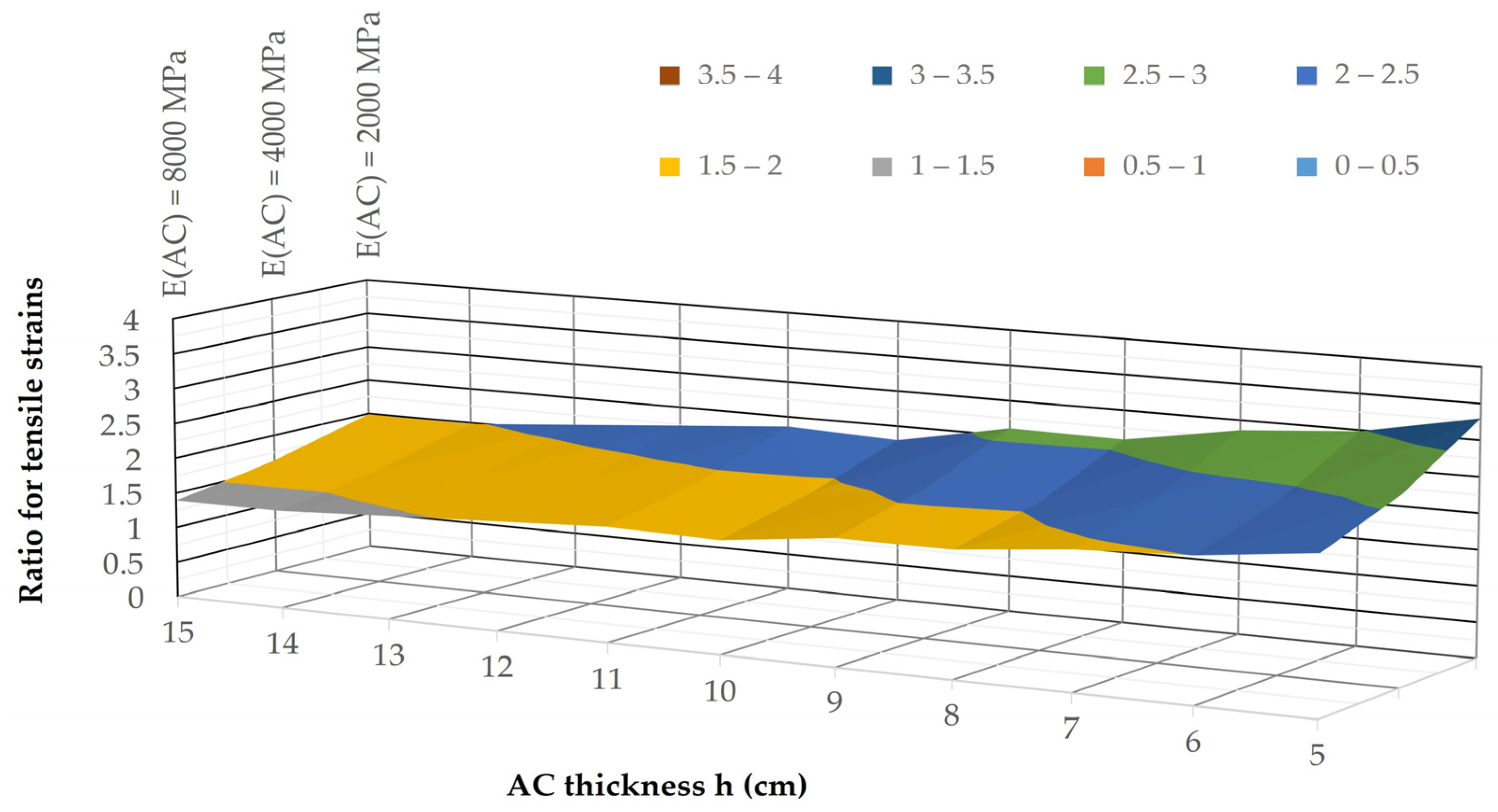
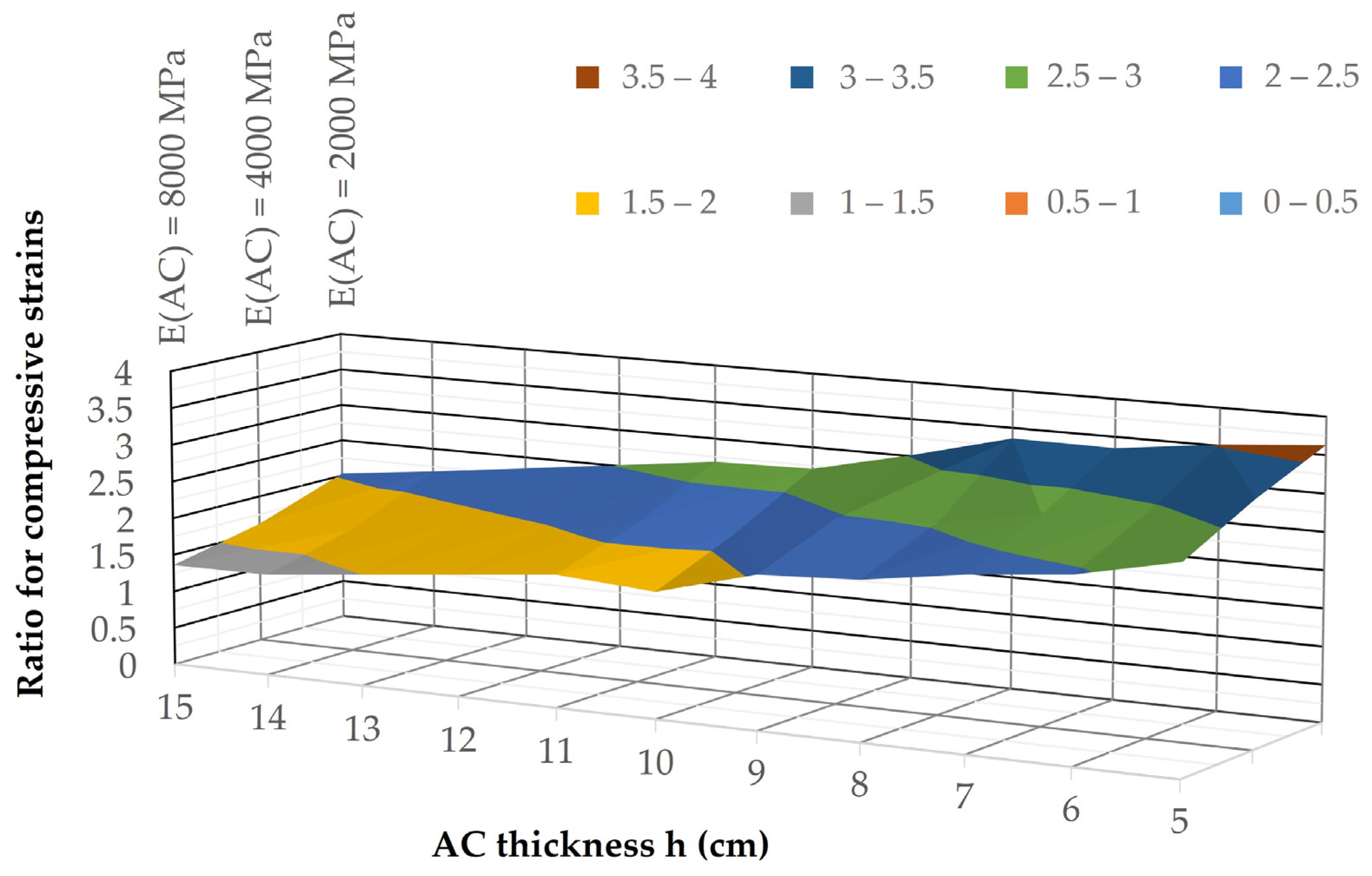
4. Results and Discussion
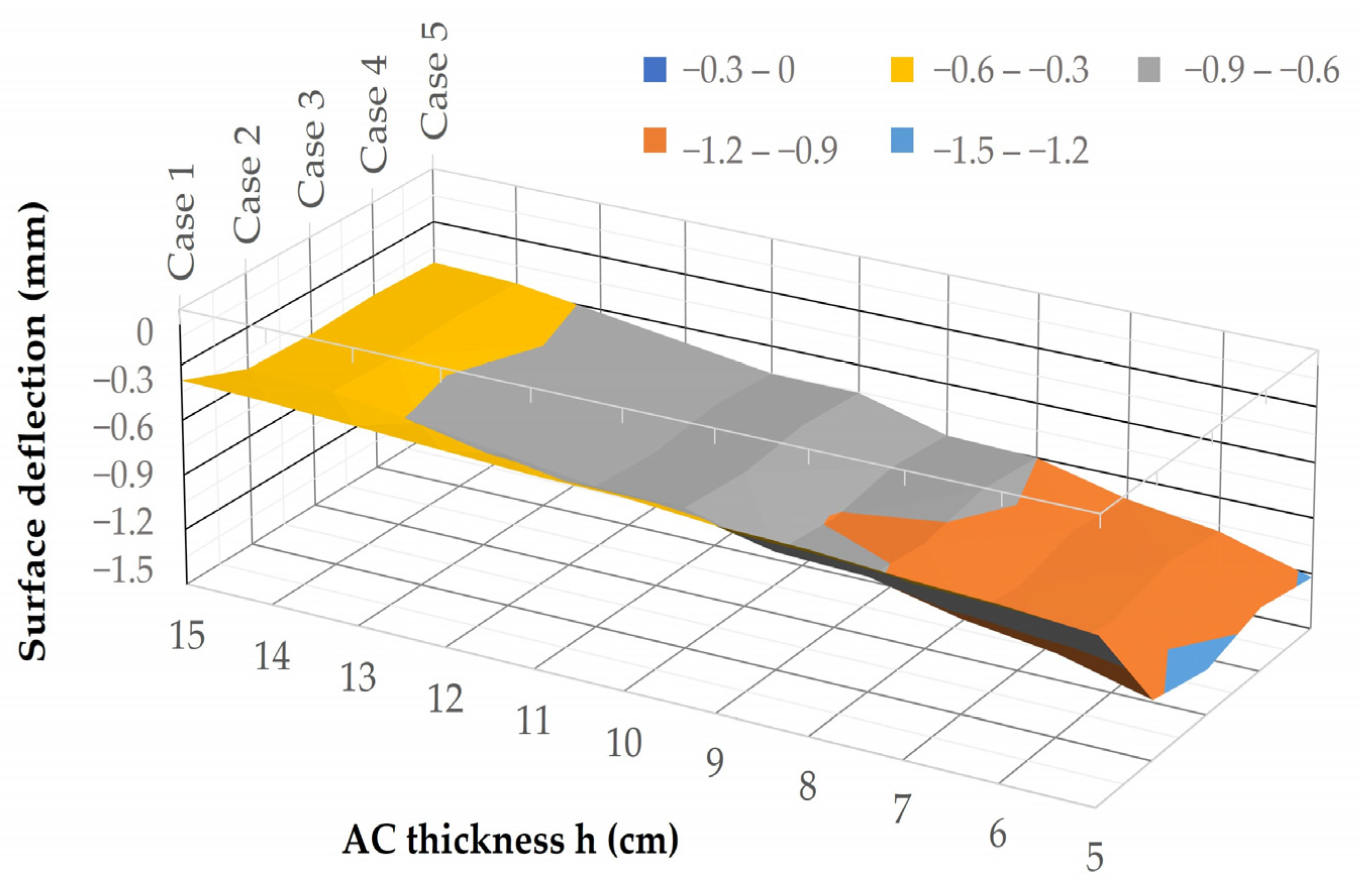
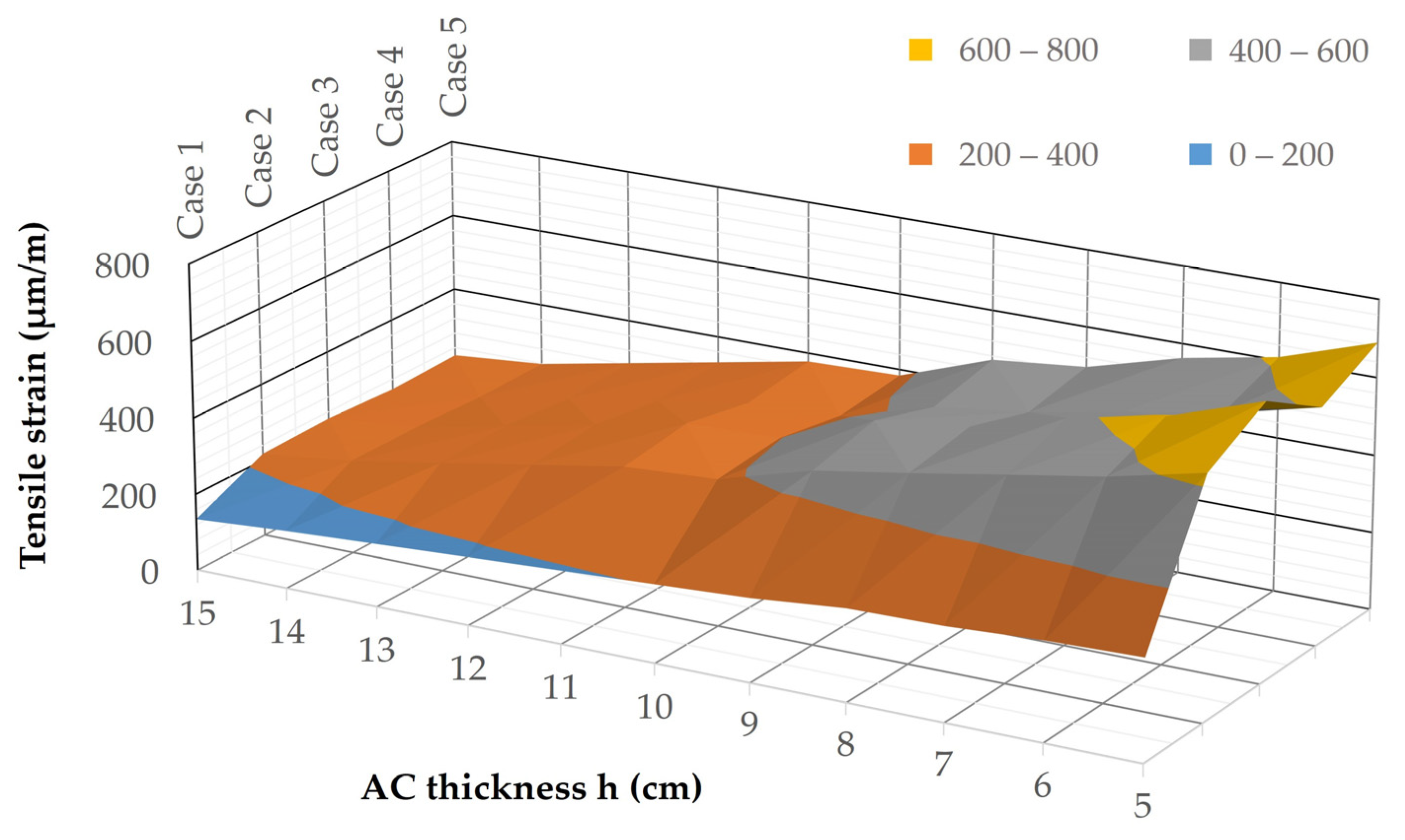
4.1. Analysis—Phase A
4.2. Analysis—Phase B
5. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yong-hong, Y.; Yuan-hao, J.; Xuan-cang, W. Pavement Performance Prediction Methods and Maintenance Cost Based on the Structure Load. Procedia Eng. 2016, 137, 41–48. [Google Scholar] [CrossRef]
- Ai, C.; He, H.; Zhang, X.; Meng, H.; Ren, D. Investigation of inter-layer bonding property in asphalt pavement based on 3D morphology of reconstructed interface. Constr. Build. Mater. 2022, 317, 125983. [Google Scholar] [CrossRef]
- White, G. Difference between Pavement Thickness Design and Pavement Life Prediction for Rigid Aircraft Pavements. Designs 2022, 6, 12. [Google Scholar] [CrossRef]
- Plati, C.; Gkyrtis, K.; Loizos, A. A Practice-Based Approach to Diagnose Pavement Roughness Problems. Int. J. Civ. Eng. 2023. [Google Scholar] [CrossRef]
- Svilar, M.; Peško, I.; Šešlija, M. Model for Estimating the Modulus of Elasticity of Asphalt Layers Using Machine Learning. Appl. Sci. 2022, 12, 10536. [Google Scholar] [CrossRef]
- Marecos, V.; Solla, M.; Fontul, S.; Antunes, V. Assessing the pavement subgrade by combining different non-destructive methods. Constr. Build. Mater. 2017, 135, 76–85. [Google Scholar] [CrossRef]
- Gkyrtis, K.; Plati, C.; Loizos, A. Mechanistic Analysis of Asphalt Pavements in Support of Pavement Preservation Decision-Making. Infrastructures 2022, 7, 61. [Google Scholar] [CrossRef]
- Chabot, A.; Chupin, O.; Deloffre, L.; Duhamel, D. ViscoRoute 2.0: A tool for the simulation of moving load effects on asphalt pavement. Road Mater. Pavement Des. 2010, 11, 227–250. [Google Scholar] [CrossRef]
- Georgouli, K.; Pomoni, M.; Cliatt, B.; Loizos, A. A simplified approach for the estimation of HMA dynamic modulus for in service pavements. In Proceedings of the 6th International Conference on Bituminous Mixtures and Pavements (ICONFBMP), Thessaloniki, Greece, 10–12 June 2015; Taylor and Francis: Abingdon, UK, 2015; pp. 661–670. [Google Scholar]
- Zhang, Q.; Leng, W.; Zhai, B.; Xu, F.; Dong, J.; Yang, Q. Evaluation of Critical Dynamic Stress and Accumulative Plastic Strain of an Unbound Granular Material Based on Cyclic Triaxial Tests. Materials 2021, 14, 5722. [Google Scholar] [CrossRef]
- Lekarp, F.; Isacsson, U.; Dawson, A. State of the Art I: Resilient Response of Unbound Aggregate. J. Transp. Eng. 2000, 126, 66–75. [Google Scholar] [CrossRef]
- Uzan, J. Resilient characterization of pavement material. Int. J. Numer. Anal. Methods Geomech. 1992, 16, 435–459. [Google Scholar] [CrossRef]
- Lin, M.; Hu, C.; Guan, H.; Easa, S.M.; Jiang, Z. Impacts of Material Orthotropy on Mechanical Behaviors of Asphalt Pavements. Appl. Sci. 2021, 11, 5481. [Google Scholar] [CrossRef]
- Tutumluer, E.; Thompson, M.R. Anisotropic modeling of granular bases in flexible pavements. Transp. Res. Rec. 1997, 1577, 18–26. [Google Scholar] [CrossRef]
- Masad, S.; Little, D.; Masad, E. Analysis of Flexible Pavement Response and Performance Using Isotropic and Anisotropic Material Properties. J. Transp. Eng. 2006, 132, 342–349. [Google Scholar] [CrossRef]
- Ciampa, D.; Cioffi, R.; Colangelo, F.; Diomedi, M.; Farina, I.; Olita, S. Use of Unbound Materials for Sustainable Road Infrastructures. Appl. Sci. 2020, 10, 3465. [Google Scholar] [CrossRef]
- Beriha, B.; Sahoo, U.C. Analysis of flexible pavement with cross-anisotropic material properties. Int. J. Pavement Res. Technol. 2020, 13, 411–416. [Google Scholar] [CrossRef]
- Al-Qadi, I.; Wang, H.; Tutumluer, E. Dynamic analysis of thin asphalt pavements by using cross-anisotropic stress-dependent properties for granular layer. Transp. Res. Rec. 2010, 2154, 156–163. [Google Scholar] [CrossRef]
- Cai, Y.; Sangghaleh, A.; Pan, E. Effect of anisotropic base/interlayer on the mechanistic responses of layered pavements. Comput. Geotech. 2015, 65, 250–257. [Google Scholar] [CrossRef]
- Gomes Correia, A.; Cunha, J. Analysis of nonlinear soil modelling in the subgrade and rail track responses under HST. Transp. Geotech. 2014, 1, 147–156. [Google Scholar] [CrossRef]
- Luan, Y.; Lu, W.; Fu, K. Research on Resilient Modulus Prediction Model and Equivalence Analysis for Polymer Reinforced Subgrade Soil under Dry–Wet Cycle. Polymers 2023, 15, 4187. [Google Scholar] [CrossRef]
- Jing, P.; Chazallon, C. Hydro-Mechanical Behaviour of an Unbound Granular Base Course Material Used in Low Traffic Pavements. Materials 2020, 13, 852. [Google Scholar] [CrossRef]
- Ranadive, M.S.; Tapase, A.B. Parameter sensitive analysis of flexible pavement. Int. J. Pavement Res. Technol. 2016, 9, 466–472. [Google Scholar] [CrossRef]
- Gu, F.; Luo, X.; Zhang, Y.; Lytton, R.; Sahin, H. Modeling of unsaturated granular materials in flexible pavements. In Proceedings of the 3rd European Conference on Unsaturated Soils—“E-UNSAT 2016”, Paris, France, 12–16 September 2016; Volume 9, p. 20002. [Google Scholar] [CrossRef]
- Jia, M.; Li, H.; Ma, G.; Zhang, X.; Yang, B.; Tian, Y.; Zhang, Y. Investigation on permanent deformation of unbound granular material base for permeable pavement: Laboratory and field study. Int. J. Transp. Sci. Technol. 2023, 12, 373–386. [Google Scholar] [CrossRef]
- Titi, H.H.; Matar, M.G. Estimating resilient modulus of base aggregates for mechanistic-empirical pavement design and performance evaluation. Transp. Geotech. 2018, 17, 141–153. [Google Scholar] [CrossRef]
- Pomoni, M.; Plati, C. Skid Resistance Performance of Asphalt Mixtures Containing Recycled Pavement Materials under Simulated Weather Conditions. Recycling 2022, 7, 47. [Google Scholar] [CrossRef]
- Xiao, Y.; Kong, K.; Aminu, U.F.; Li, Z.; Li, Q.; Zhu, H.; Cai, D. Characterizing and Predicting the Resilient Modulus of Recycled Aggregates from Building Demolition Waste with Breakage-Induced Gradation Variation. Materials 2022, 15, 2670. [Google Scholar] [CrossRef] [PubMed]
- Pomoni, M.; Plati, C.; Loizos, A. How Can Sustainable Materials in Road Construction Contribute to Vehicles’ Braking? Vehicles 2020, 2, 55–74. [Google Scholar] [CrossRef]
- Li, N.; Ma, B.; Wang, H. Strains Comparisons of Unbound Base/Subbase Layer Using Three Elasto-Plastic Models under Repeated Loads. Appl. Sci. 2021, 11, 9251. [Google Scholar] [CrossRef]
- Tarefder, R.; Ahdem, M.; Islam, M.; Rahman, M. Finite element model of pavement response under load considering cross-anisotropy in unbound layers. Adv. Civ. Eng. Mater. 2014, 3, 57–75. [Google Scholar] [CrossRef]
- Sahoo, U.C.; Reddy, K.S. Effect of Nonlinearity in Granular Layer on Critical Pavement Responses of Low Volume Roads. Int. J. Pavement Res. Technol. 2010, 3, 320–325. [Google Scholar]
- Loizos, A.; Spiliopoulos, K.; Cliatt, B.; Gkyrtis, K. Structural pavement responses using nonlinear finite element analysis of unbound materials. In Proceedings of the 10th International Conference on Bearing Capacity of Roads, Railways and Airfields (BCRRA), Athens, Greece, 28–30 June 2017; pp. 1343–1350. [Google Scholar]
- Araya, A.A.; Huurman, M.; Houben, L.J.M. Characterizing mechanical behavior of unbound granular materials for pavements. In Proceedings of the Transportation Research Board 90th Annual Meeting, Washington, DC, USA, 23–27 January 2011. [Google Scholar]
- Ullah, S.; Jamal, A.; Almoshaogeh, M.; Alharbi, F.; Hussain, J. Investigation of Resilience Characteristics of Unbound Granular Materials for Sustainable Pavements. Sustainability 2022, 14, 6874. [Google Scholar] [CrossRef]
- Stehlik, D.; Hyzl, P.; Dasek, O.; Malis, L.; Kaderka, R.; Komenda, R.; Sachr, J.; Vesely, P.; Spies, K.; Varaus, M. Comparison of Unbound Granular Materials’ Resilient Moduli Determined by Cyclic Triaxial Test and Innovative FWD Device. Appl. Sci. 2022, 12, 5673. [Google Scholar] [CrossRef]
- Loizos, A.; Gkyrtis, K.; Plati, C. Modelling Asphalt Pavement Responses Based on Field and Laboratory Data. In Accelerated Pavement Testing to Transport Infrastructure Innovation; Lecture Notes in Civil Engineering; Chabot, A., Hornych, P., Harvey, J., Loria-Salazar, L., Eds.; Springer: Cham, Switzerland; Volume 96, pp. 438–447.
- Ba, M.; Fall, M.; Samb, F.; Sarr, D.; Ndiaye, M. Resilient modulus of unbound aggregate base courses from Senegal (West Africa). Open J. Civ. Eng. 2011, 1, 1–6. [Google Scholar] [CrossRef]


| Layer | Modulus (MPa) | Poisson’s Ratio |
|---|---|---|
| AC | 2000, 4000, 8000 | 0.35 |
| Base | 400 | 0.40 |
| Subgrade | 40 | 0.45 |
| Case | Assumptions for the Unbound Materials |
|---|---|
| 1 | Linear analysis, Isotropic material |
| 2 | Nonlinear analysis, Isotropic material, No initial loading |
| 3 | Nonlinear analysis, Anisotropic material, No initial loading |
| 4 | Nonlinear analysis, Isotropic material, With initial loading |
| 5 | Nonlinear analysis, Anisotropic material, With initial loading |
| Case | hAC = 15 cm | hAC = 10 cm | hAC = 8 cm | hAC = 5 cm |
|---|---|---|---|---|
| 1 | −0.383 | −0.469 | −0.518 | −0.616 |
| 2 | −0.526 | −0.743 | −0.885 | −1.185 |
| 3 | −0.538 | −0.77 | −0.925 | −1.258 |
| 4 | −0.52 | −0.725 | −0.858 | −1.156 |
| 5 | −0.533 | −0.755 | −0.899 | −1.220 |
| Case | hAC = 15 cm | hAC = 10 cm | hAC = 8 cm | hAC = 5 cm |
|---|---|---|---|---|
| 1 | 135 | 204 | 240 | 264 |
| 2 | 217 | 380 | 488 | 627 |
| 3 | 222 | 397 | 517 | 716 |
| 4 | 213 | 366 | 465 | 612 |
| 5 | 219 | 384 | 495 | 690 |
| Case | hAC = 15 cm | hAC = 10 cm | hAC = 8 cm | hAC = 5 cm |
|---|---|---|---|---|
| 1 | −250 | −376 | −451 | −571 |
| 2 | −374 | −679 | −923 | −1462 |
| 3 | −424 | −775 | −1193 | −1975 |
| 4 | −373 | −678 | −902 | −1426 |
| 5 | −404 | −803 | −1145 | −1869 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gkyrtis, K. Pavement Analysis with the Consideration of Unbound Granular Material Nonlinearity. Designs 2023, 7, 142. https://doi.org/10.3390/designs7060142
Gkyrtis K. Pavement Analysis with the Consideration of Unbound Granular Material Nonlinearity. Designs. 2023; 7(6):142. https://doi.org/10.3390/designs7060142
Chicago/Turabian StyleGkyrtis, Konstantinos. 2023. "Pavement Analysis with the Consideration of Unbound Granular Material Nonlinearity" Designs 7, no. 6: 142. https://doi.org/10.3390/designs7060142
APA StyleGkyrtis, K. (2023). Pavement Analysis with the Consideration of Unbound Granular Material Nonlinearity. Designs, 7(6), 142. https://doi.org/10.3390/designs7060142







