Temperature-Driven Instabilities in High-Pressure Vessel Flat Plates: A Thermal Buckling Study
Abstract
1. Introduction
2. Mathematical Model of Circular Annular Plate
2.1. Kinetic and Kinematic Equations
2.2. Equations of Motion
3. Stability Analysis of Circular Annular Plate
3.1. Solving the Stability Equation
3.2. Case Study: A Real Heating Surface with Molten Salt
4. Design Parameters Analysis
4.1. Scalability Assessment
4.2. Effect of Thermal Expansion Coefficient ()
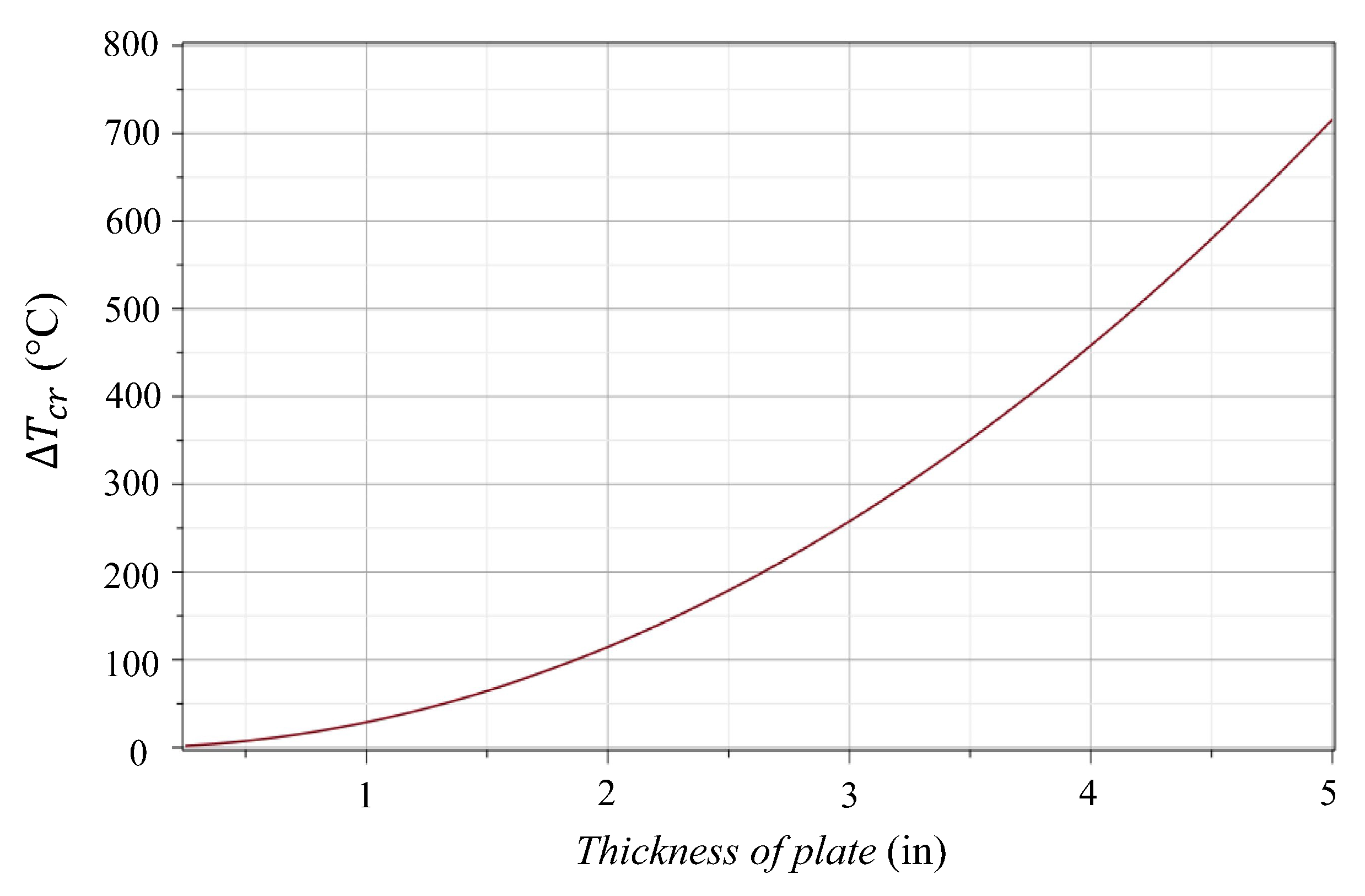
4.3. Effect of Thickness of Plate (h)
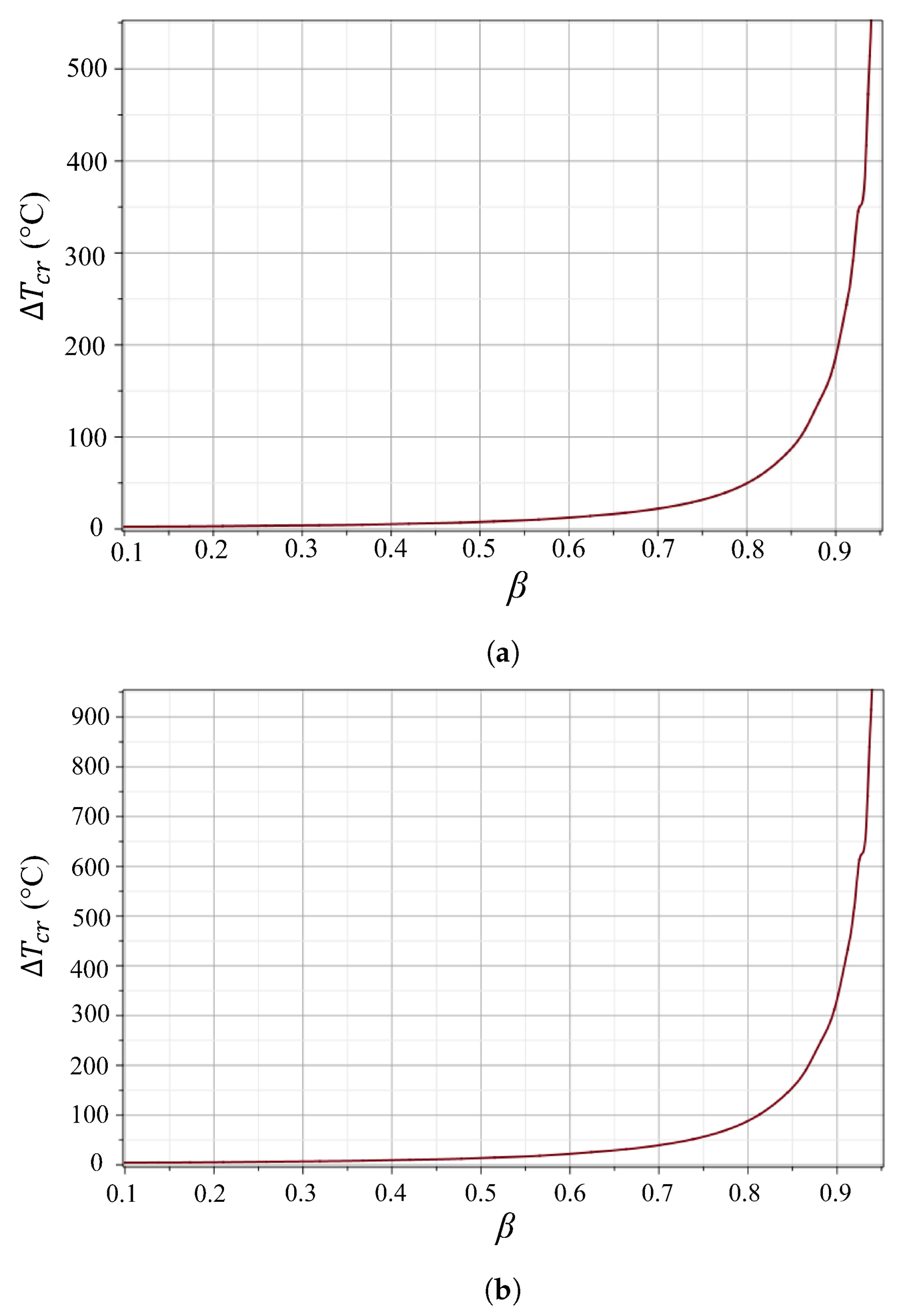
4.4. Effect of the Inner and Outer Diameter Ratio ()
5. Conclusions
- Edge Boundary Conditions: Clamped edges increase the critical buckling temperature, enhancing stability.
- Plate Thickness: Thicker plates show greater resistance to thermal buckling.
- Diameter Ratio: Increasing the inner diameter, while keeping the outer diameter constant, raises the critical buckling temperature.
- Material Selection: Materials with lower thermal expansion coefficients improve thermal buckling resistance.
- Elastic Foundation: Clamping the plates together, effectively creating an elastic foundation through additional pinning and fixing between the plates, increases the critical buckling temperature.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Singh, K.P.; Soler, A.I. Mechanical Design of Heat Exchangers: And Pressure Vessel Components; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar] [CrossRef]
- Moyya, S.; Thejasree, P.; Abraham, B.C.; Mangalathu, G.S. Design and analysis of single and multi-layer pressure vessel. Mater. Today Proc. 2023; in press. [Google Scholar] [CrossRef]
- Vignarooban, K.; Xu, X.; Wang, K.; Molina, E.; Li, P.; Gervasio, D.; Kannan, A.M. Vapor pressure and corrosivity of ternary metal-chloride molten-salt based heat transfer fluids for use in concentrating solar power systems. Appl. Energ. 2015, 159, 206–213. [Google Scholar] [CrossRef]
- Mahmoudinezhad, S.; Sadi, M.; Ghiasirad, H.; Arabkoohsar, A. A comprehensive review on the current technologies and recent developments in high-temperature heat exchangers. Renew. Sustain. Energy Rev. 2023, 183, 113467. [Google Scholar] [CrossRef]
- Mohiddin, M.N.B.; Tan, Y.H.; Seow, Y.X.; Kansedo, J.; Mubarak, N.; Abdullah, M.O.; San Chan, Y.; Khalid, M. Evaluation on feedstock, technologies, catalyst and reactor for sustainable biodiesel production: A review. J. Ind. Eng. Chem. 2021, 98, 60–81. [Google Scholar] [CrossRef]
- Thakur, A.; Kumar, R.; Kumar, S.; Kumar, P. Review of developments on flat plate solar collectors for heat transfer enhancements using phase change materials and reflectors. Mater. Today Proc. 2021, 45, 5449–5455. [Google Scholar] [CrossRef]
- Kruizenga, A.; Gill, D. Corrosion of iron stainless steels in molten nitrate salt. Energ. Proc. 2014, 49, 878–887. [Google Scholar] [CrossRef]
- Ali, M.; Ul-Hamid, A.; Alhems, L.M.; Saeed, A. Review of common failures in heat exchangers—Part I: Mechanical and elevated temperature failures. Eng. Fail. Anal. 2020, 109, 104396. [Google Scholar] [CrossRef]
- Toudehdehghan, A.; Hong, T.W. A critical review and analysis of pressure vessel structures. In Proceedings of the 1st International Postgraduate Conference on Mechanical Engineering (IPCME2018), Pekan, Malaysia, 31 October 2018; IOP Conference Series: Materials Science and Engineering. IOP Publishing: Bristol, UK, 2019; Volume 469, p. 012009. [Google Scholar] [CrossRef]
- ASME BPVC Section VIII-Rules for Construction of Pressure Vessels-Div. II—Alternative Rules; The American Society of Mechanical Engineers. 2023. Available online: https://www.asme.org/codes-standards/find-codes-standards/bpvc-viii-2-bpvc-section-viii-rules-construction-pressure-vessels-division-2-alternative-rules/2023/print-book (accessed on 1 July 2024).
- Khodamorad, S.; Alinezhad, N.; Fatmehsari, D.H.; Ghahtan, K. Stress corrosion cracking in Type. 316 plates of a heat exchanger. Case Stud. Eng. Fail. Anal. 2016, 5, 59–66. [Google Scholar] [CrossRef]
- Jabbari, M.; Nejad, M.Z. Mechanical and thermal stresses in radially functionally graded hollow cylinders with variable thickness due to symmetric loads. Aust. J. Mech. Eng. 2020, 18, S108–S121. [Google Scholar] [CrossRef]
- Boley, B.A.; Weiner, J.H. Theory of Thermal Stresses; Wiley: Hoboken, NJ, USA, 1960. [Google Scholar] [CrossRef]
- Sadeghi-Chahardeh, A.; Mollaabbasi, R.; Picard, D.; Taghavi, S.M.; Alamdari, H. Discrete element method modeling for the failure analysis of dry mono-size coke aggregates. Materials 2021, 14, 2174. [Google Scholar] [CrossRef]
- Sadeghi-Chahardeh, A.; Mollaabbasi, R.; Picard, D.; Taghavi, S.M.; Alamdari, H. Effect of particle size distributions and shapes on the failure behavior of dry coke aggregates. Materials 2021, 14, 5558. [Google Scholar] [CrossRef]
- Sarbanha, A.A.; Larachi, F.; Taghavi, S.M. Bubble and particle dynamics in a thin rectangular bubbling fluidized bed under emulated marine instabilities. Chem. Eng. Sci. 2023, 280, 119041. [Google Scholar] [CrossRef]
- Li, X.; Shlosman, I.; Pfenniger, D.; Heller, C. The origin of buckling instability in galactic bars: Searching for the scapegoat. Mon. Not. R. Astron. Soc. 2023, 520, 1243–1257. [Google Scholar] [CrossRef]
- Yang, Z.; Liu, A.; Lai, S.K.; Safaei, B.; Lv, J.; Huang, Y.; Fu, J. Thermally induced instability on asymmetric buckling analysis of pinned-fixed FG-GPLRC arches. Eng. Struct. 2022, 250, 113243. [Google Scholar] [CrossRef]
- Hajlaoui, A.; Chebbi, E.; Dammak, F. Three-dimensional thermal buckling analysis of functionally graded material structures using a modified FSDT-based solid-shell element. Int. J. Press. Vessel. Pip. 2021, 194, 104547. [Google Scholar] [CrossRef]
- Wang, C.M.; Wang, C.Y. Exact Solutions for Buckling of Structural Members; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar] [CrossRef]
- Zhang, J.; Zhao, Q.; Wang, X.; Ullah, S.; Zhao, D.; Civalek, Ö.; Xue, C.; Qi, W. New exact analytical thermal buckling solutions of composite thin plates with all edges rotationally-restrained. Mech. Adv. Mater. Struct. 2024, 31, 3518–3530. [Google Scholar] [CrossRef]
- Ghiasian, S.; Kiani, Y.; Sadighi, M.; Eslami, M. Thermal buckling of shear deformable temperature dependent circular/annular FGM plates. Int. J. Mech. Sci. 2014, 81, 137–148. [Google Scholar] [CrossRef]
- Weisz-Patrault, D.; Margerit, P.; Constantinescu, A. Residual stresses in thin walled-structures manufactured by directed energy deposition: In-situ measurements, fast thermo-mechanical simulation and buckling. Addit. Manuf. 2022, 56, 102903. [Google Scholar] [CrossRef]
- Reddy, J.N. Theory and Analysis of Elastic Plates and Shells; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar] [CrossRef]
- Kiani, Y.; Eslami, M. An exact solution for thermal buckling of annular FGM plates on an elastic medium. Compos. B Eng. 2013, 45, 101–110. [Google Scholar] [CrossRef]
- Eslami, M.R. Buckling and Post-buckling of Beams. In Buckling and Postbuckling of Beams, Plates, and Shells; Springer International Publishing: Cham, Switzerland, 2018; pp. 7–110. [Google Scholar] [CrossRef]
- Eslami, M.R. Thermal Stresses in Plates. In Thermal Stresses in Plates and Shells; Springer: Berlin/Heidelberg, Germany, 2024; pp. 43–143. [Google Scholar] [CrossRef]
- Javani, M.; Kiani, Y.; Eslami, M.R. Nonlinear dynamic response of a temperature-dependent FGM spherical shell under various boundary conditions and thermal shocks: Examination of dynamic snap-through. Thin-Walled Struct. 2024, 199, 111796. [Google Scholar] [CrossRef]
- Babaei, H.; Kiani, Y.; Reza Eslami, M. Perturbation method for thermal post-buckling analysis of shear deformable FG-CNTRC beams with different boundary conditions. Int. J. Struct. Stab. Dyn. 2021, 21, 2150175. [Google Scholar] [CrossRef]
- Qolipour, A.M.; Eipakchi, H.; Nasrekani, F.M. Asymmetric/Axisymmetric buckling of circular/annular plates under radial load using first-order shear deformation theory. Thin-Walled Struct. 2023, 182, 110244. [Google Scholar] [CrossRef]
- Bagheri, H.; Kiani, Y.; Eslami, M. Asymmetric thermal buckling of annular plates on a partial elastic foundation. J. Therm. Stress. 2017, 40, 1015–1029. [Google Scholar] [CrossRef]
- Kuchibhotla, A.; Banerjee, D.; Dhir, V. Forced convection heat transfer of molten Salts: A review. Nucl. Eng. Des. 2020, 362, 110591. [Google Scholar] [CrossRef]
- Fernández, A.; Galleguillos, H.; Fuentealba, E.; Pérez, F. Corrosion of stainless steels and low-Cr steel in molten Ca(NO3)2-NaNO3-KNO3 eutectic salt for direct energy storage in CSP plants. Sol. Energy Mater. Sol. Cells 2015, 141, 7–13. [Google Scholar] [CrossRef]
- Walczak, M.; Pineda, F.; Fernandez, A.G.; Mata-Torres, C.; Escobar, R.A. Materials corrosion for thermal energy storage systems in concentrated solar power plants. Renew. Sustain. Energy Rev. 2018, 86, 22–44. [Google Scholar] [CrossRef]
- Sheets, A.S.G.D. Atlas steels technical handbook of stainless steels. Stainl. Steel 2013, 630, 17–4PH. [Google Scholar] [CrossRef]
- Cverna, F. (Ed.) ASM Ready Reference: Thermal Properties of Metals; ASM International: Almere, The Netherlands, 2002. [Google Scholar]


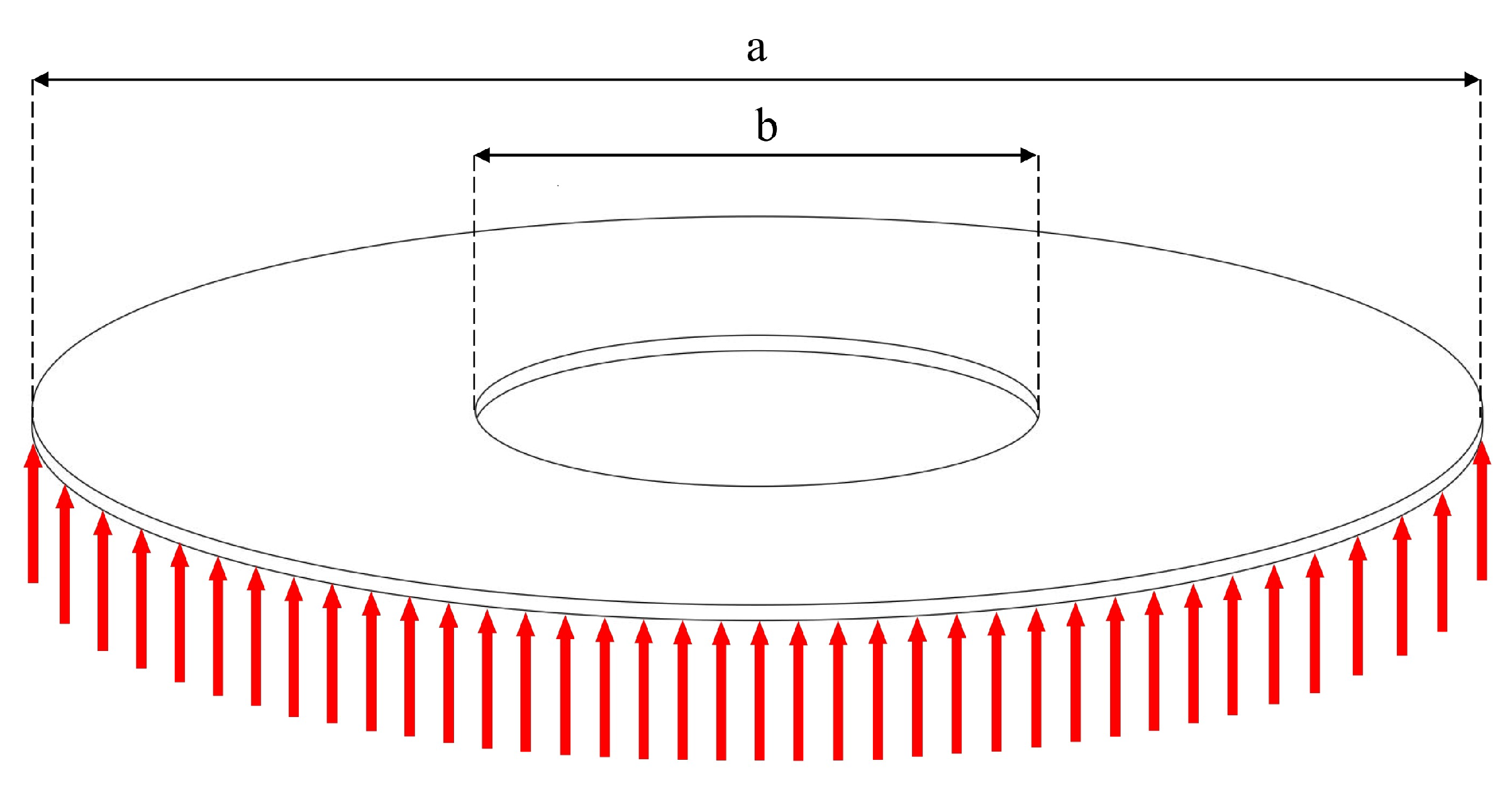

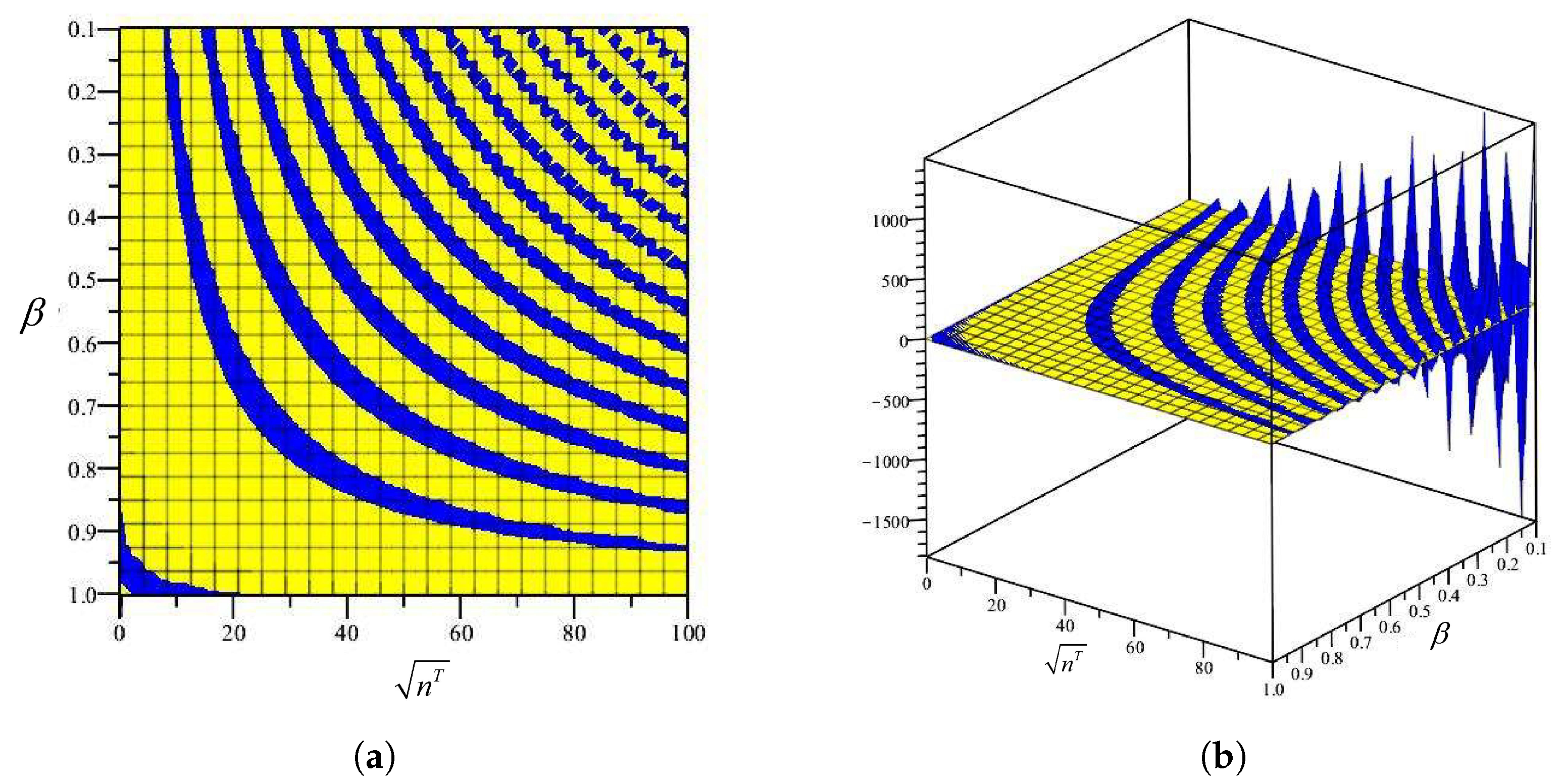
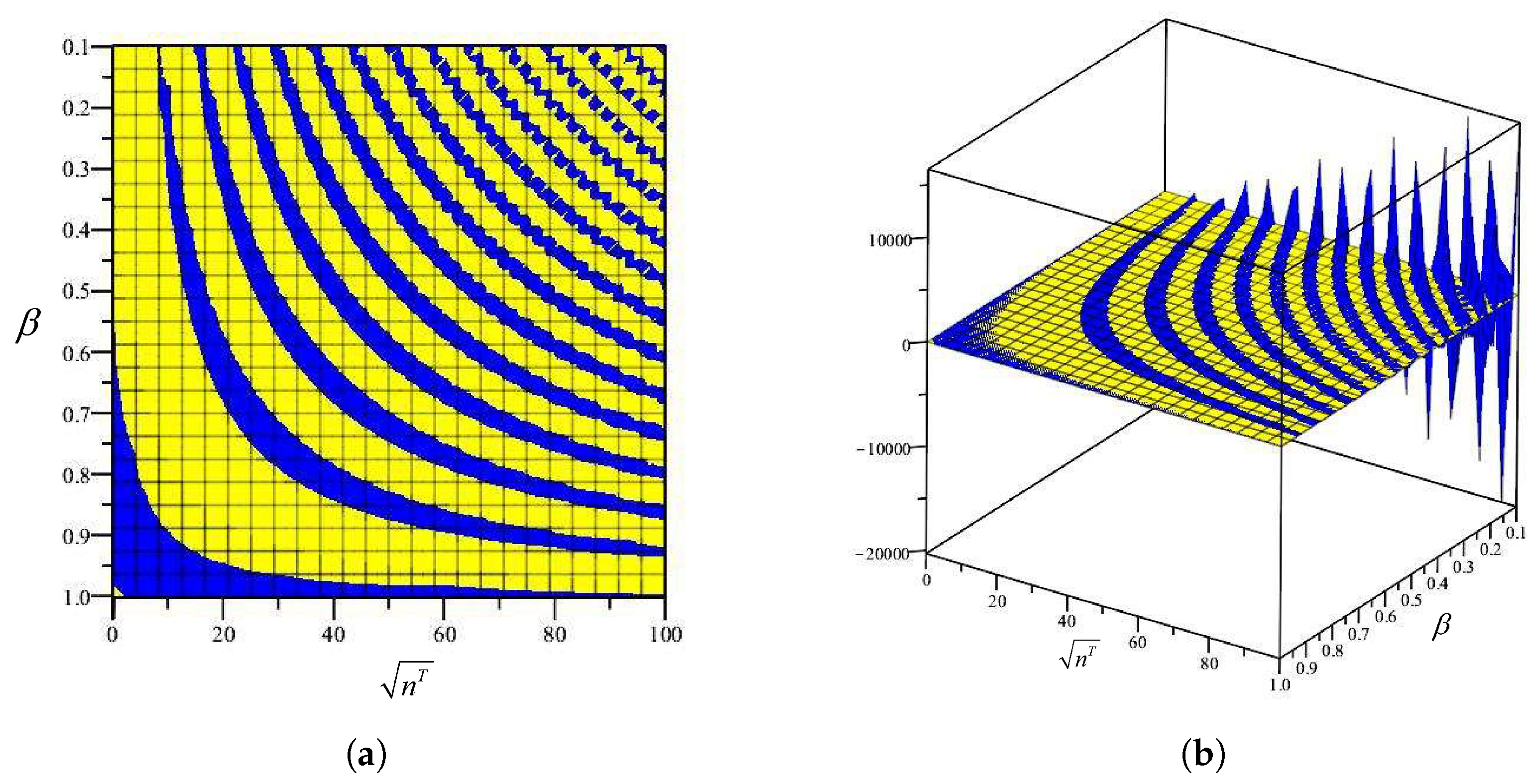
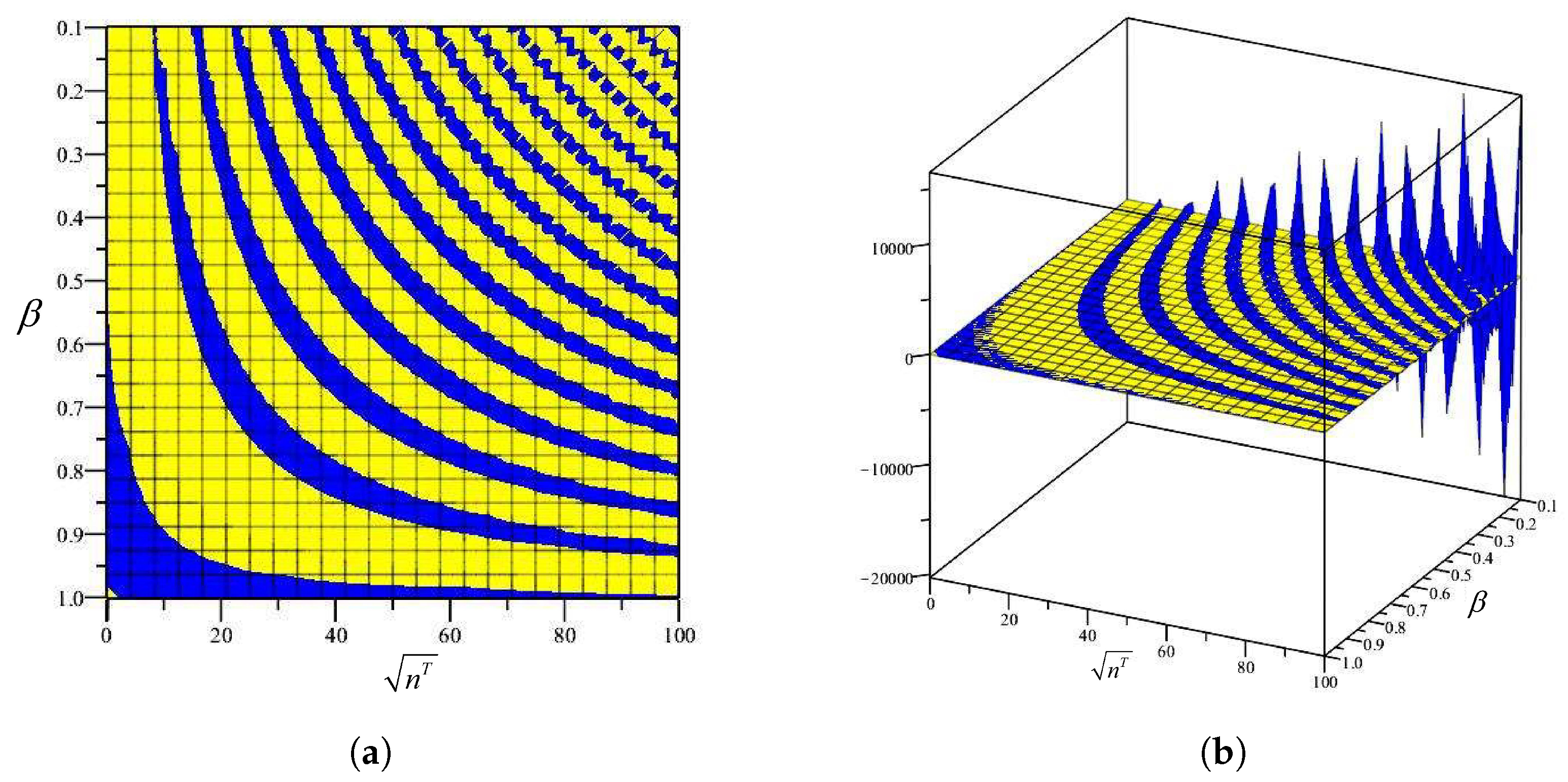
| Parameter | Notation |
|---|---|
| Outer Radius | a |
| Inner Radius | b |
| Modulus of Elasticity | E |
| Poisson’s Ratio | |
| Inside Radius Temperature | |
| Outside Radius Temperature | |
| Linear Expansion Coefficients |
| Number of Mode | Description of Buckling | (°C) | |
|---|---|---|---|
| 10.12 | Symmetric | 11.9 | |
| 11.25 | Asymmetric | 14.71 | |
| 12.55 | Asymmetric | 18.30 | |
| 13.08 | Asymmetric | 19.88 | |
| 14.98 | Asymmetric | 26.08 | |
| 15.53 | Asymmetric | 28.03 | |
| 16.05 | Asymmetric | 29.94 | |
| 17.51 | Asymmetric | 35.63 | |
| 19.13 | Asymmetric | 42.53 | |
| 20.28 | Asymmetric | 47.79 |
| Number of Mode | Description of Buckling | (°C) | |
|---|---|---|---|
| 8.83 | Symmetric | 4.03 | |
| 9.27 | Asymmetric | 4.44 | |
| 10.67 | Asymmetric | 5.89 | |
| 10.91 | Asymmetric | 6.15 | |
| 11.74 | Asymmetric | 7.12 | |
| 12.38 | Asymmetric | 7.92 | |
| 13.19 | Asymmetric | 8.98 | |
| 14.06 | Asymmetric | 10.2 | |
| 15.21 | Asymmetric | 11.95 | |
| 15.97 | Asymmetric | 13.17 |
| Number of Mode | Description of Buckling | (°C) | |
|---|---|---|---|
| 8.83 | Symmetric | 7.16 | |
| 9.27 | Asymmetric | 7.89 | |
| 10.67 | Asymmetric | 10.45 | |
| 10.91 | Asymmetric | 10.93 | |
| 11.74 | Asymmetric | 12.66 | |
| 12.38 | Asymmetric | 14.07 | |
| 13.19 | Asymmetric | 15.97 | |
| 14.06 | Asymmetric | 18.15 | |
| 15.21 | Asymmetric | 21.24 | |
| 15.97 | Asymmetric | 23.42 |
| Grade of Stainless Steel | () [36] | (°C) |
|---|---|---|
| SS304 | 17.3 | 4.02 |
| SS321 | 17.2 | 4.04 |
| SS317 | 16 | 4.35 |
| SS316 | 16 | 4.35 |
| SS309 | 14.9 | 4.67 |
| SS330 | 14.4 | 4.83 |
| SS310 | 14.4 | 4.83 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sadeghi-Chahardeh, A.; Abdollahi-Mamoudan, F. Temperature-Driven Instabilities in High-Pressure Vessel Flat Plates: A Thermal Buckling Study. Designs 2024, 8, 81. https://doi.org/10.3390/designs8040081
Sadeghi-Chahardeh A, Abdollahi-Mamoudan F. Temperature-Driven Instabilities in High-Pressure Vessel Flat Plates: A Thermal Buckling Study. Designs. 2024; 8(4):81. https://doi.org/10.3390/designs8040081
Chicago/Turabian StyleSadeghi-Chahardeh, Alireza, and Farima Abdollahi-Mamoudan. 2024. "Temperature-Driven Instabilities in High-Pressure Vessel Flat Plates: A Thermal Buckling Study" Designs 8, no. 4: 81. https://doi.org/10.3390/designs8040081
APA StyleSadeghi-Chahardeh, A., & Abdollahi-Mamoudan, F. (2024). Temperature-Driven Instabilities in High-Pressure Vessel Flat Plates: A Thermal Buckling Study. Designs, 8(4), 81. https://doi.org/10.3390/designs8040081







