Catastrophic Failure Analysis of a Wind Turbine Gearbox by the Finite Element Method and Fracture Analysis

Abstract

The wind turbine gearbox, used as a multiplier, is one of the main components directly related to a wind turbine’s efficiency and lifespan. Therefore, strict control of the gearbox and its manufacturing processes and even minor improvements in this component strongly and positively impact energy production/generation over time. Since only some papers in the literature analyze the mechanical aspect of wind turbines, focusing on some parts in depth, this paper fills the gap by offering an analysis of the gearbox component under the highest amount of stress, namely relating to the sun shaft, as well as a more holistic analysis of the main gear drives, its components, and the lubrification system. Thus, this work diagnoses the fracture mechanics of a 1600 kW gearbox to identify the main reason for the fracture and how the chain of events took place, leading to catastrophic failure. The diagnoses involved numerical simulation (finite element analysis—FEA) and further analysis of the lubrication system, bearings, planetary stage gears, helical stage gears, and the high-speed shaft. In conclusion, although the numerical simulation showed high contact stresses on the sun shaft teeth, the region with the unexpectedly nucleated crack was the tip of the tooth. The most likely factors that led to premature failure were the missed lubrication for the planetary bearings, a lack of cleanliness in regard to the raw materials of the gears (voids found), and problems with the sun shaft heat treatment. With the sun gear’s shaft, planet bearings, and planet gears broken into pieces, those small and large pieces dropped into the oil, between the gears, and into the tooth ring, causing the premature and catastrophic gearbox failure.

1. Introduction

Renewable energy production has grown significantly in recent decades [1,2]. One of the pieces of equipment that has been crucial to this renewable trend is the wind turbine [3,4]. Kinetic energy is harvested from the wind by turning the rotor blades of a wind turbine, which are connected to a gearbox, working as a multiplier, which increases the speed and reduces the torque proportionally. This kinetic energy reaches a generator that produces electrical energy [5,6]. When it comes to the fracture of the main component, namely the gearbox, many fracture analyses in the literature focus on bearing failure; for example, Sankar et al. [7], Bruce [8], and Evans [9] studied gearbox bearings, concluding that the bearings failed due to overloading and fatigue. Dilip et al. [10] worked on improving the lubrication system of gearboxes from 2 to 4000 kW and with regard to oil purification. The same author revealed that the main bearings affected by lubrication are the high-speed bearings in the high-speed shaft (HSS) and the helical gear bearings in the intermediate shaft (IMS). Rajinikanth et al. [11] focused on another area, studying the failure mode of planet gears, concluding that the failure was initiated by micropitting, which aggravated subsurface microcracks and led to spalling, which, afterward, generated a complete fracture. The same research approach was followed by Wang et al. [12], who suggested that the gearbox in their study fractured due to crack-initiation sites related to a fatigue fracture situated at the bottom of pits left by particles broken from the tooth flank. In addition, they assessed the fracture using finite element analysis and concluded that the presence of an uneven load applied to the gears contributed to the failure. In addition, Bai et al. [13] studied the fracture of gears in a wind turbine and proposed improvements, including raising the gear pressure angle, inducing compressive residual stress, combining high surface hardness with low core hardness, and ensuring a sizeable and adequate case depth to inhibit crack nucleation and avoid fatigue phenomena. Although D. Chen et al. [14] studied wind turbine gearbox failure, the authors focused on a specific planet gear, concluding that there was a higher nitrogen content present than recommended, plus an improper heat treatment had been applied, causing material brittleness. Cheng Wang [15] focused on the dynamics and design of a gear train in order to optimize it. The author obtained a high power density by optimizing the gear train volume and transmission efficiency. Zizhen Qiao et al. [16] dealt with crack propagation using fracture mechanics theory and created a new dynamic simulation model for a wind turbine’s two-stage cracked gear drive. The authors analyzed the change in the vibration frequencies to determine whether a crack was present in the gears, and the gear acceleration amplitudes to determine the presence of a single or multiple cracks by comparing the relevant values.

In theory, and based on the studies by Paik, C., Chung, Y., and Kim, Y.J. [17], the power of a wind turbine and, consequently, a gearbox, has a linear correlation with the speed of the wind blowing toward the wind turbine. For a 1.600 kW wind turbine, linearity occurs at intervals from 7 m/s to 11.5 m/s. This means that the torque in the gearbox is constant during this interval, with no cycling loads. Regarding the gearbox analyzed, a few additional data inputs are that the gearbox ran for six years and had a lower Nacelle due to significant vibrations and blockages. The operational conditions of the equipment were not shared. As is known by the scientific community that studies fractures, most of the time, the analysis of a machine or equipment fracture is not easy since it involves collecting data, which are sometimes unavailable. On the other hand, the visual analysis of the equipment components, the usage of the macro-morphology of fractured surfaces of fractured parts, combined with numerical simulation, and references from the literature, are worthy of determining the most probable root causes that led to the fracture. No papers in the literature describe a case study dealing with a combination of numerical simulation of the sun shaft and analysis of the whole fractured components of a wind turbine gearbox. This is the only research paper of this kind; therefore, it offers a highly technical contribution to the work by the scientific community, which can be used as a reference for the creation of new generations of wind turbine gearboxes (product-wise) and additional controls with regard to the manufacturing processes and operation of wind turbines. With that said, this paper’s main objective is to provide an overview and conduct an analysis of the components of a 1600 kW gearbox, beginning with a numerical simulation (finite element analysis—FEA) to determine the regions of high stresses on the highest-stressed component, the sun shaft, followed by verifying the hydraulic system, bearings, sun shaft, planet carrier, planet gears, and gear tooth. In the end, the root cause of the failure is determined based on FEA and the analysis of the main gearbox components.

2. Materials and Methods

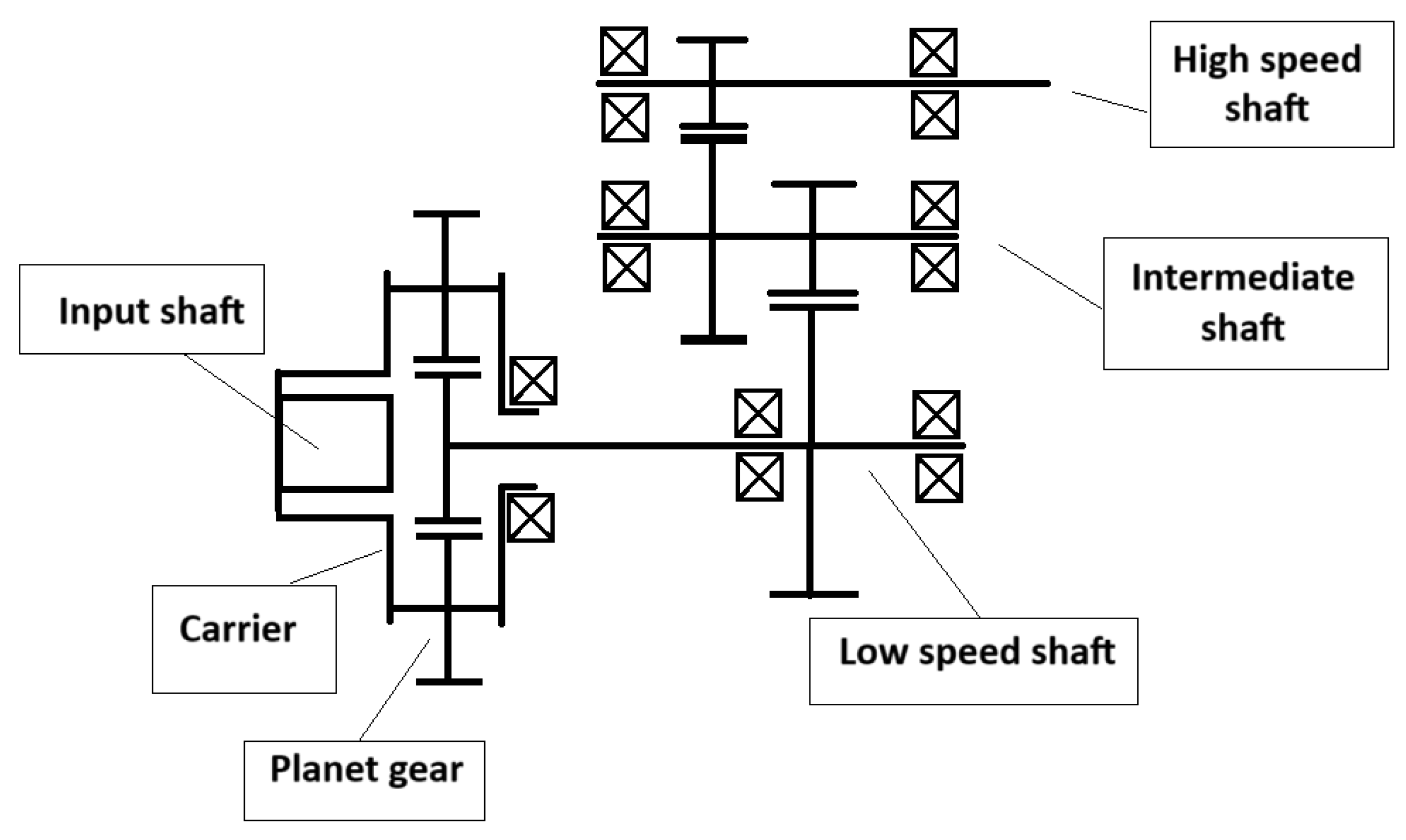
2.1. Gearbox Anatomy

The gearbox analyzed (powered to 1600-kW) and its main dimensions are shown in Figure 1. Figure 2 schematically demonstrates the gearbox internal configuration: the planet stage receives the movement from the rotor by the main shaft and has the lowest speed; then, the movement is transferred to the intermediate stage and ends in the high-speed stage (output) to the generator. Although the gearbox belongs to the company DESCH North America in Canada, the end user cannot be revealed due to a non-disclosure agreement. The company DESCH reverse-engineered the gearbox and generated drawings, which cannot be detailed due to a non-disclosure agreement. The analysis of the gearbox‘s failure mode started with a peripherical verification with an initial inspection by borescope to check the lubrication system and filtering, pressure into the cooler, functionality of heaters, and sensors. Afterward, a more detailed evaluation was performed by checking the conditions of gears, shafts, and bearings.

2.2. Power and Analysis of the Torque at the Planet Stage

The methods utilized for the analysis of the peripherical components were as follows. Heaters and sensors were checked by measuring their continuity and resistance, and the manifold and the pump–coupling components were disassembled and checked. The shafts were visually inspected for marks, dents, pitting, corrosion, and other significant damages, while the gears and sun gear were visually checked, seeking marks, dents, and craters. The gears were also visually analyzed using a Borescope View Tech model VJ-3 with a 6 and 3 mm diameter. Figure 2 also helped navigate the gearbox torques/forces evaluation per stage. Another critical piece of information is analyzing the gearbox functionality. The Equations (1) and (2) were utilized for this purpose:

$$P={\displaystyle \frac{1}{2}}·A·\rho · v^3·C_p·{10}^{-3}$$

(1)

$$T=9550·{\displaystyle \frac{P}{n}}$$

(2)

where P is power in kW, ρ is the density of the air (kg/m³), A is the area of the rotor (m²), $v$ is the velocity of the air (m/s), and $C_p$ is dimensionless and constant 0.45—Jerson R.P. Vaz et al., Shigley, J. E. et al., and Tazi, N. et al. [18,19,20] for a wind turbine with three blades. T equals torque (N·m), 9550 is a constant, and n is the rotation of the blades in min⁻¹.

A few equations are necessary to determine the speed and torque of each gearbox state. For the planet stage with the tooth ring fixed, the planet carrier’s input, and the sun shaft’s output, Equation (3) is utilized, while for regular gear engagement, Equation (4) is used, Provenza, F. [21].

$${\phi }_p=1+{\displaystyle \frac{Z_3}{Z_1}}$$

(3)

$${\phi }_R={\displaystyle \frac{Z_o}{Z_i}}$$

(4)

where ${\phi }_p$ is the ratio of a planet stage gearbox, $Z_3$ is the number of teeth of the tooth ring, $Z_1$ is the number of teeth of the sun shaft, ${\phi }_R$ is the ratio of a regular stage gearbox, $Z_i$ is the number of teeth of the input gear, and $Z_0$ is the number of teeth of the output gearbox (dimensionless).

After analyzing the Equations (1)–(3), few initial statements are possible, which makes the fracture evaluation more robust. The first is that the input side from the equipment rotor and main shaft drives the planet carrier of the gearbox, which, despite having three planets, carries the maximum load on the planet gears, planet gears’ bearings, and sun shaft. Therefore, this stage is under the highest torque, vector forces, and lowest speed (Provenza, F.) [21]. On the output side, the gearbox output to the generator presents the lowest torque. Therefore, the main concern is now directed toward failures at the output gear teeth (high-speed shaft) due to high speed.

2.3. Numerical Simulation (Finite Element Method) and Hertz Contact

A numerical simulation using the finite element analysis (FEA) was utilized to obtain a map of stresses on the gear teeth and compare it with the fractured zone morphology. The calculation used a linear elastic for structural simulation, as the software is for. The software used for the calculation was the module ANSYS [22] for the linear-elastic numerical calculation present at Autodesk F-360 [23]. Before the calculation, the deformed area on the tooth was obtained by utilizing the Hertz equation for the contact between two cylinders, which was determined geometrically by studying the teeth’s geometries and material of the gears (Equations (5)–(7)—Vinod K Sarin) [24].

$$C=2{\displaystyle \frac{1-{\nu }^2}{E}}$$

(5)

$$b=\sqrt{{\displaystyle \frac{2}{\pi }}}·\sqrt{{\displaystyle \frac{P}{L}}·{\displaystyle \frac{C}{\frac{1}{d_1}+\frac{1}{d_2}}}}$$

(6)

$$p_{max}={\displaystyle \frac{4}{\pi }}·{\displaystyle \frac{P}{2·b·L}}$$

(7)

where ν is the coefficient of Poison, E is the module of Young (MPa), C is the coefficient for material properties, P is the force applied per gear tooth (N), L is the length of the gear, d1 and d2 are the diameter of the circles traced on the gear tooth in contact, and b is half of the contact area (mm).

The material considered for the simulation was AISI 4340 350C QT, which has a yield strength of 1.178 MPa and an ultimate tensile strength of 1240 MPa. As usual, a case hardening with a tensile strength of 2100 MPa is considered for the sun shaft teeth.

A parabolic element was used for the mesh’s construction on the sun shaft, with an average element size of 10%—the model-based size—a maximum turn angle on the curve of 60°, and a 20% maximum element size—the percentage of average size. The maximum number of refinements was considered to be 6, with a 5% convergence tolerance and a portion of 40% of the elements to refine. The number of elements was 92,373, and the number of nodes was 141,255. The element’s maximum size was conditioned to 1.5 mm (refined) at the contact area. The constraint of the sun shaft was localized at the opposite end of the gear teeth, with a larger diameter where a condition of zero degrees of freedom was imposed.

2.4. Torque at the Planetary Stage

In a planet gearbox stage (Figure 2), the sun shaft receives the highest torque from three planet carrier gears, making it more susceptible to failure (Figure 3). This was the reason for analyzing only this part via FEA. With the pieces of evidence collected in this work and based on the design (torque-wise), there is no need to explore more components.

Equations (1) and (2) were utilized to better understand the tangential force at the planetary stage and the pressures on the teeth. For the calculation, the wind turbine was rated to 1600-kW, the air density was 1 kg/m³, the diameter of the rotor was 100 m, the velocity of the air was 9.7 m/s, and the Betz efficiency was 0.45 [14]. The rotation of the rotor was considered to be 16.2 min⁻¹.

Module 14.5; number of Teeth, 18; contact angle, 25°; helix, 8.6°.

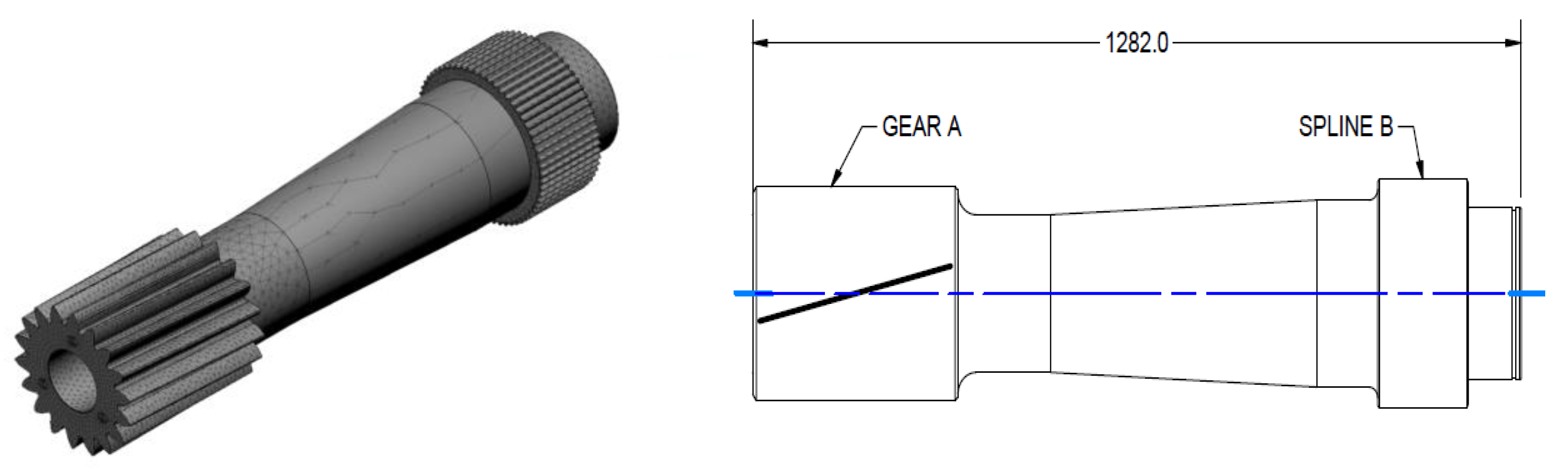
2.5. Investigation of the Main Components of the Gearbox

All components were verified to obtain a broader picture and determine the failure’s root cause(s). It started with the peripherical components and moved to the shafts, gears, and bearings. The diagnosis was vital to tracing the failed parts and creating a broader view of the failure characteristic. The purpose was to gather data to determine the most likely causes of the gearbox failure.

It is not uncommon to use a macro-analysis of the fractured surfaces of fracture parts as an evaluation method. Thus, fatigue phenomena can sometimes be evidenced visually (naked eye) (macro morphology) and do not require a deep analysis using a microscope.

3. Results and Remarks

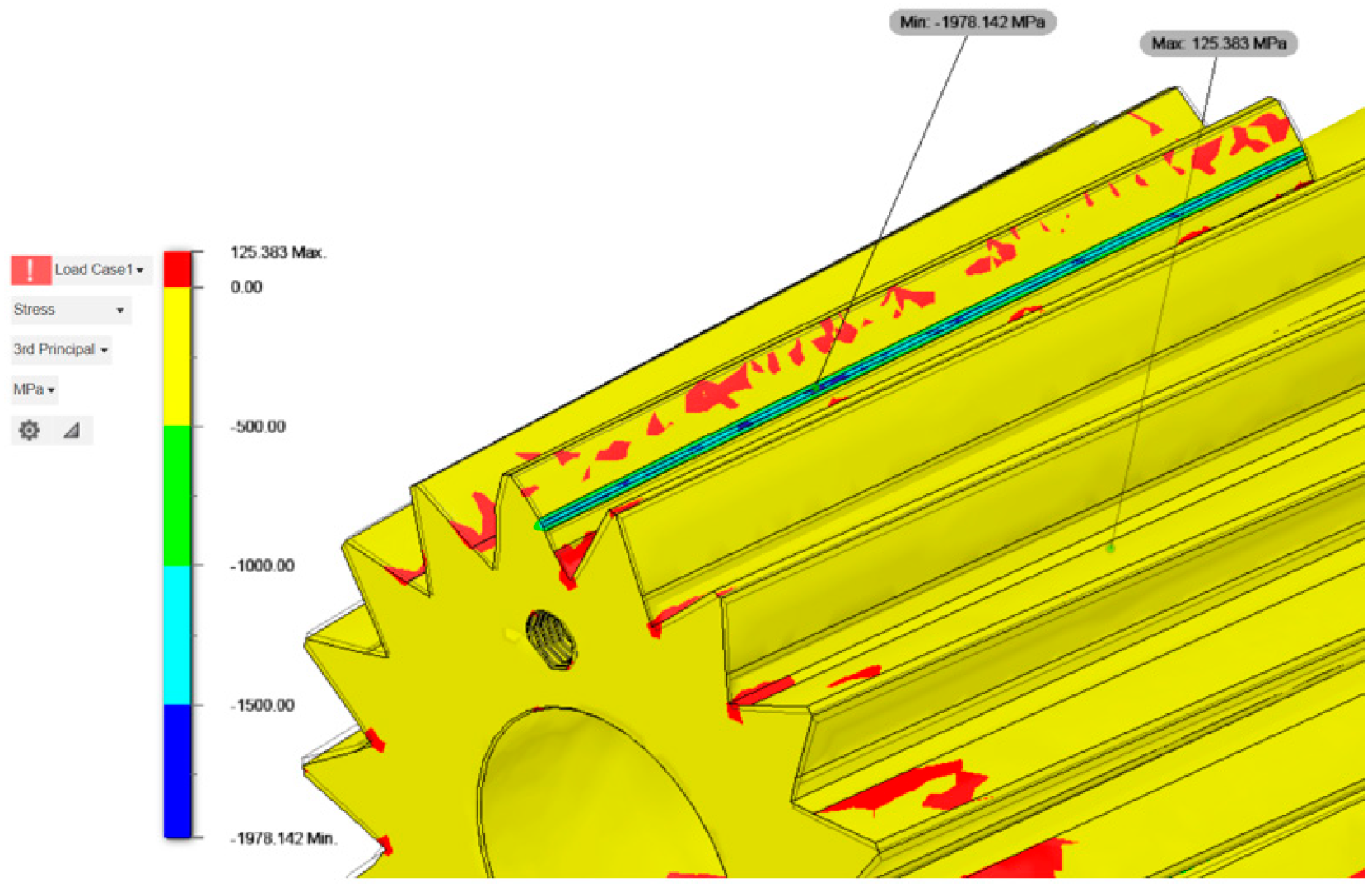
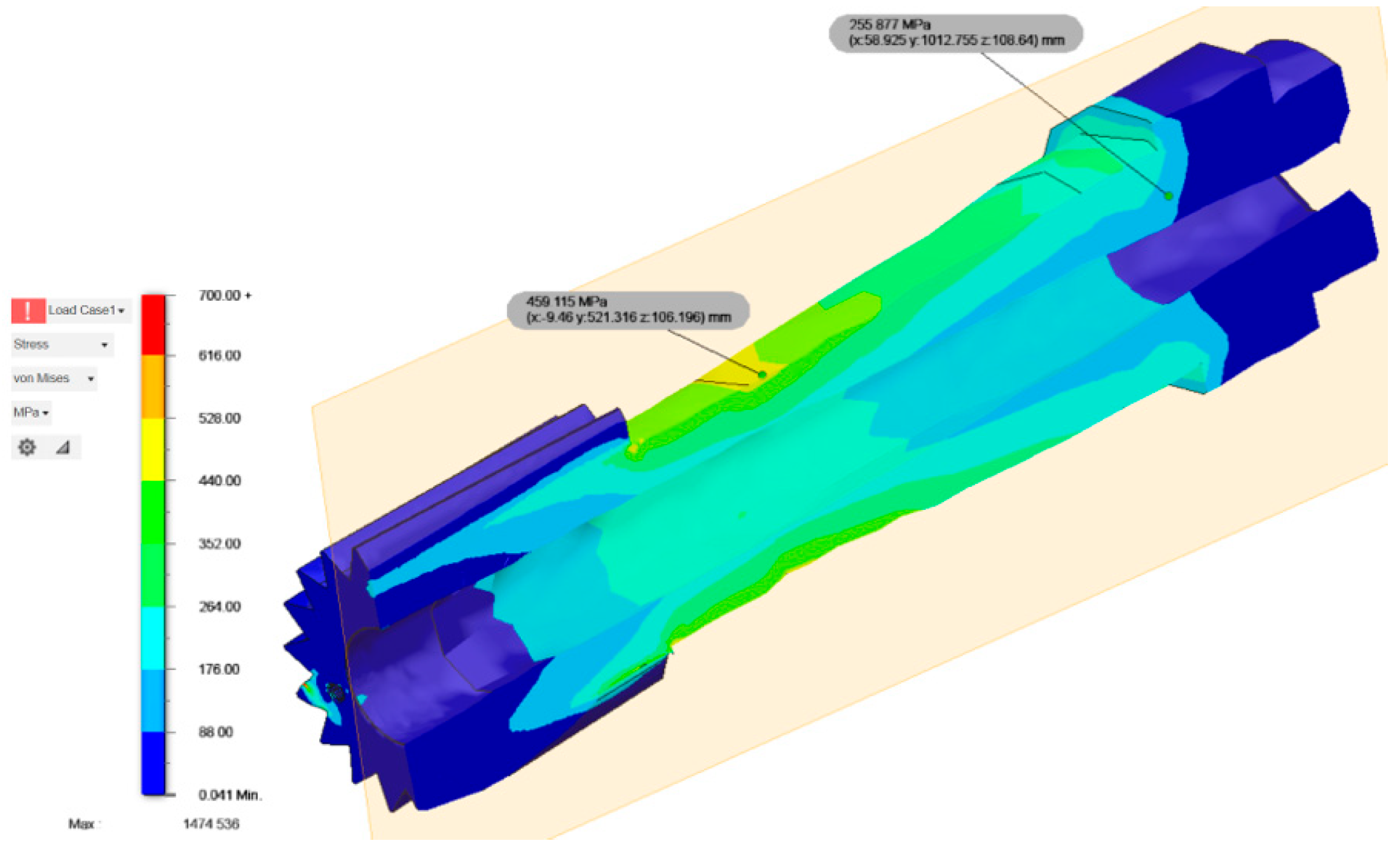
3.1. Torque at the Planetary Stage and Numerical Simulation (FEA)

The total torque calculated using the power from Equations (1) and (2) was 943,209 nm, which resulted in a tangential force of 1,190,916 N per tooth. Considering the area of contact (2b) according to Hertz Equations (5)–(7), the pressure per pair of teeth resulted in 2170 MPa. It was based on the equipment’s operating conditions and the design of the gears (parts).

Figure 4 and Figure 5 show the von Mises stresses throughout the sun shaft. The highest stress was found in the contact area of the teeth, reaching a maximum of 1474 MPa.

Figure 6 shows the morphology of the von Mises stress. The region of the highest contact and the gradient of stresses are more evident, with the tooth fillet revealed as the second region of high stress. The top land of the tooth does not present high stress.

Principal stress σ₁ (Figure 7) shows the maximum stress at the region of the tooth fillet as the minimum stress. The maximum value is 732 MPa, and the minimum is −868 MPa. Figure 8 demonstrates stress σ₃ with a maximum compressive stress of −1978 MPa and a tensile stress of 125 MPa.

Figure 9 shows a longitudinal cross-section of the sun shaft. There is a von Mises stress of 755 MPa close to the spline, where the shaft has a fixed constraint, and a stress of 459 MPa close to the gear at the external diameter of the shaft.

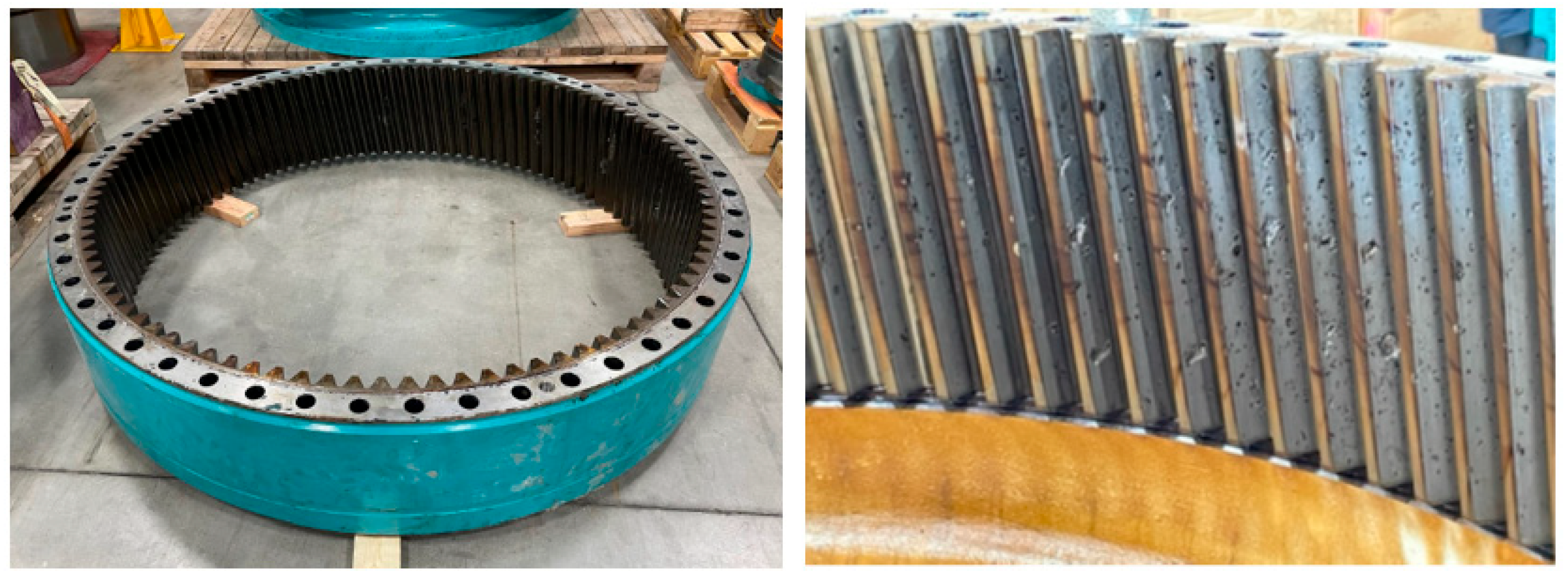
3.2. Peripherical Components

The analyses with pictures that follow demonstrate the significant changes in the original condition or design of the gearbox and its peripherals. The first hint obtained from the condition of the lubrication system was the glass window, which showed a significant presence of air in the oil, creating a thick foam (Figure 10—left). Also, with the hydraulic system (Figure 10—mid) disassembled, it was verified that the coupling had the main spider slatted (Figure 10—right), which created a temporary lack of lubrication and mechanical/hydraulic impacts on the system.

The other components of the peripherical system were not compromised, such as the filter, which still had a mid-life; the pressure in the cooler, which remained constant with no drop when tested under 150-kPa; and all heaters and sensors, which presented electrical continuity and were in good condition.

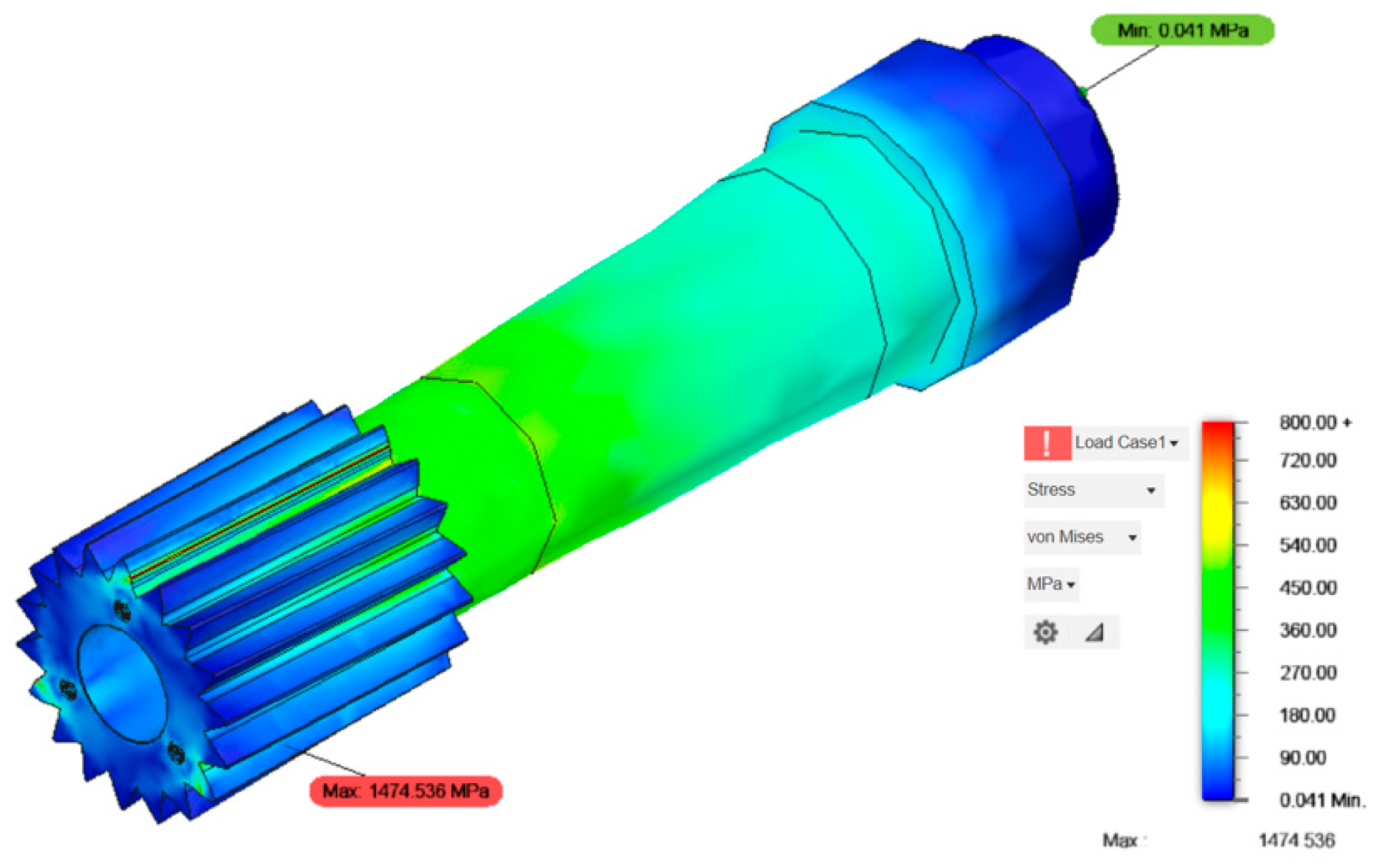
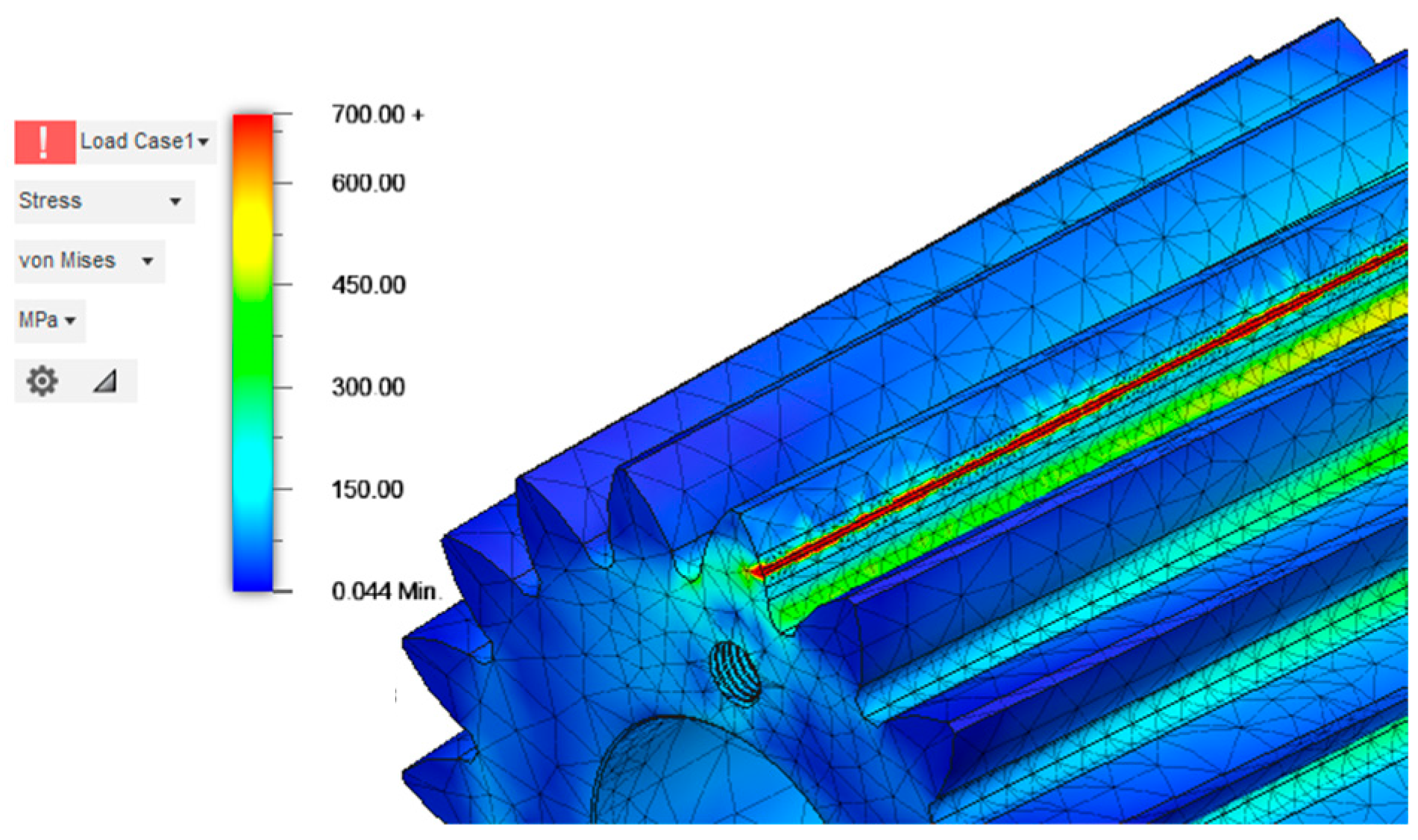
3.3. Shafts, Gears, and Bearings

When looking closer at the high-speed shaft, noticeable adhesion wear marks can be seen on the teeth’ faces and close to the flank. Adhesion wear marks were also localized on the teeth’ faces (Figure 11—right) and close to the flank (Figure 11—left).

The low-speed gear presented dents on the teeth, most likely due to material between the teeth contacting the intermediate shaft gear. When measured, the dents presented a surface roughness of around 4.8 μm (Rz) (Figure 12). Other than that, the low-speed gear teeth were in good surface finish condition.

When looking at the connection between the high-speed shaft and the intermediate gear, a few particles were found stuck on the teeth’ roots (Figure 13), most likely from the gearbox’s contaminated oil.

Figure 14 of the intermediate shaft presents a visual analysis that reveals that both sides of the gear did not show damage like dents, risks, or deep scratches; there were only a few fretting (risks) at the bottom of the teeth, but they were minor and not significant for the gearbox’s operation.

The sun shaft and the bearings were damaged. Figure 15 shows the conditions of the planet’s bearings. It exhibits damage on the rollers, which had the black coating peeled off and pieces of material removed, and the inner part of the outer race, which had the black coating detached from it as well.

Among the gears analyzed, as expected, the sun shaft was the most damaged. It presented craters on the dedendum, flank, and tooth face. All defects were highly detrimental to the part and efficiently contributed to the final fracture (Figure 16). The teeth showed significant crack initiation and beach marks. The crack nucleation started at the tip of the tooth and moved toward the flank (see arrows). Beach marks reveal the direction of the propagation of cracks throughout the flank of the sun shaft tooth.

Moreover, on the top of the tooth, there was a crack where the material separation occurred, with a crack flowing throughout the tooth length, called Case/Core separation (Figure 17). It usually happens when compressive residual stresses in the case exceed the material’s tensile strength in the core near the tooth tip due to excessive case depth at the tip. Internal cracks can propagate, causing corners, edges, or entire teeth tips to separate. Errichello, R; Milburn and AGMA [25,26,27]. Internal cracks propagated, causing corners, edges, or the entire teeth’s top land to separate. Cracks can appear immediately after the heat treatment, during handling or storage, or after time in service.

A lack of material is noticeable at the bottom of the tooth, which indicates a void generated by inclusion (Figure 17—bottom left). On the other side of the tooth, the flank had material severely removed and deformed plastically (smashed), which indicates that there were also heavy impacts on the gearbox (Figure 18).

A visual inspection of the planet gears revealed severe damage with chunks of teeth missing. This occurred most likely due to pieces of the bearing rollers falling between the sun shaft and planet gears and at the bottom of the tooth ring (Figure 19). Once the tooth ring has particles within the teeth, the contact with the planet gear generates intense pressure/damage (profound craters, dents, and marks).

The next step was verifying the ring gear’s integrity, as shown in Figure 20, Figure 21 and Figure 22. These figures show how severely the metallic particles in the oil damaged the tooth ring.

Based on the diagnosis, it is possible to make the following statements. The coupling rubber spider was shattered. The oil in the gearbox was aired, and a thick foam resulted from coupling failure. The high-speed shaft presented slight fretting, which was expected due to high speed and poor lubrication. The intermediate gear also had slight fretting. The sun shaft had large dents and craters, and a void was identified, too; these were due to a lack of raw material cleanliness.

Chunks of material peeled off the planet gears, sun shaft, and roller bearings and fell into the tooth ring’s oil. When in motion (rotation), such pieces caused significant damage to all the gearbox components.

The finite element analysis (FEA) revealed that the gear tooth’s top surface was under a substantially low von Mises stress level. This reinforced the problem related to the heat treatment and material (voids) (Errichello, R; Milburn) [25], but was not product design-related.

4. Conclusions

It was observed in the literature that only a few papers cover the mechanics of wind turbine main gearbox and, if so, are restricted to narrow analysis of some parts. With that being said, this paper aims to fill the gap and diagnose a 1600-kW gearbox’s fracture, identify the main reason(s) for the failure, and explain how the chain reaction led to the catastrophic failure. It is essential to highlight that the numerical simulation was performed only on the sun shaft due to the characteristics of the component as having the highest torque (stress] in the gearbox. A numerical finite element analysis (FEA) simulation of the sun shaft revealed the regions with the highest stresses. As expected, the contact of the teeth presented high stresses, followed by the tooth fillets. However, in contrast to the calculations, the regions that had crack nucleated were close to the top land of the sun gear teeth, regions with lowstress levels, as per numerical calculation (FEA).

With that said, the failure of the gearbox occurred because of a premature fracture of the sun shaft since it suffered predominantly a fatigue phenomenon (evidenced by beach marks on the tooth), even though a few signs of impact were observed. As mentioned previously, there were multiple points where the teeth’s surface had cracks initiated at the top land, a region of low stress. In addition, a void was observed underneath the teeth due to a clear void in the sun shaft tooth, which revealed inclusion into the material. The fracture morphology suggests a problem with the heat treatment of the sun shaft, according to the literature—Errichello, R; Milburn and Parrish, G. [25,26]. The gearbox was also compromised due to oil contamination by metallic particles peeling off planet gears’ bearing coating. The cause of black coating removal was pitting corrosion.

Although the lubrication pipes inspected were clean, allowing a smooth flow of oil throughout the lubrication system, another reason for the gearbox failure was the temporary poor lubrication on the planet bearings. This is attributed to the lubrication pump that falsely stepped on due to the coupling spider breakage, resulting in a temporary miss lubrication or even a pulsating lubrication on the planet bearing. The formation of oxides at pitting sites is enhanced with aeration in the system. The foaming oil in the oil level and inside the gearbox demonstrates that the lubrication line experienced high aeration.

To sum up, based on the data and analyses performed, the failure root causes of the gearbox were a combination of events such as problems with the case depth of the sun gear from heat treatment (tensile residual stress) and voids into the raw material, which triggered the phenomenon of fatigue and impacted the gearbox during operation. Last but not least, the missed lubrication of the planet’s bearings was also an issue. All these significant problems were confirmed by the fracture diagnosis.

Another very detrimental problem was related to the falling of small and large particles from the roller bearings, sun shaft teeth, and planet gears into the tooth ring’s oil, contaminating the oil and damaging most of the gears.

The next step in gearbox improvements is to obtain more data from the failed gearbox in the field to check whether it was an isolated cause of fracture (special cause) or a common failure mode. In addition, SEM will be applied to analyze a few components.

5. Recommendations

This study reinforces the need for strict controls on heat treatment in terms of case depth thickness and hardness; control of raw material cleanliness; regular visual inspections, mainly by borescope, at the planet stage and high-speed shaft; vibration monitoring at the same stages; oil contamination monitoring; and regular checking of the lubrication system, manifold, filter cartridge, and coupling.