Double-Exposure Method with Synchrotron White X-ray for Stress Evaluation of Coarse-Grain Materials
Abstract
:1. Introduction
2. Methods
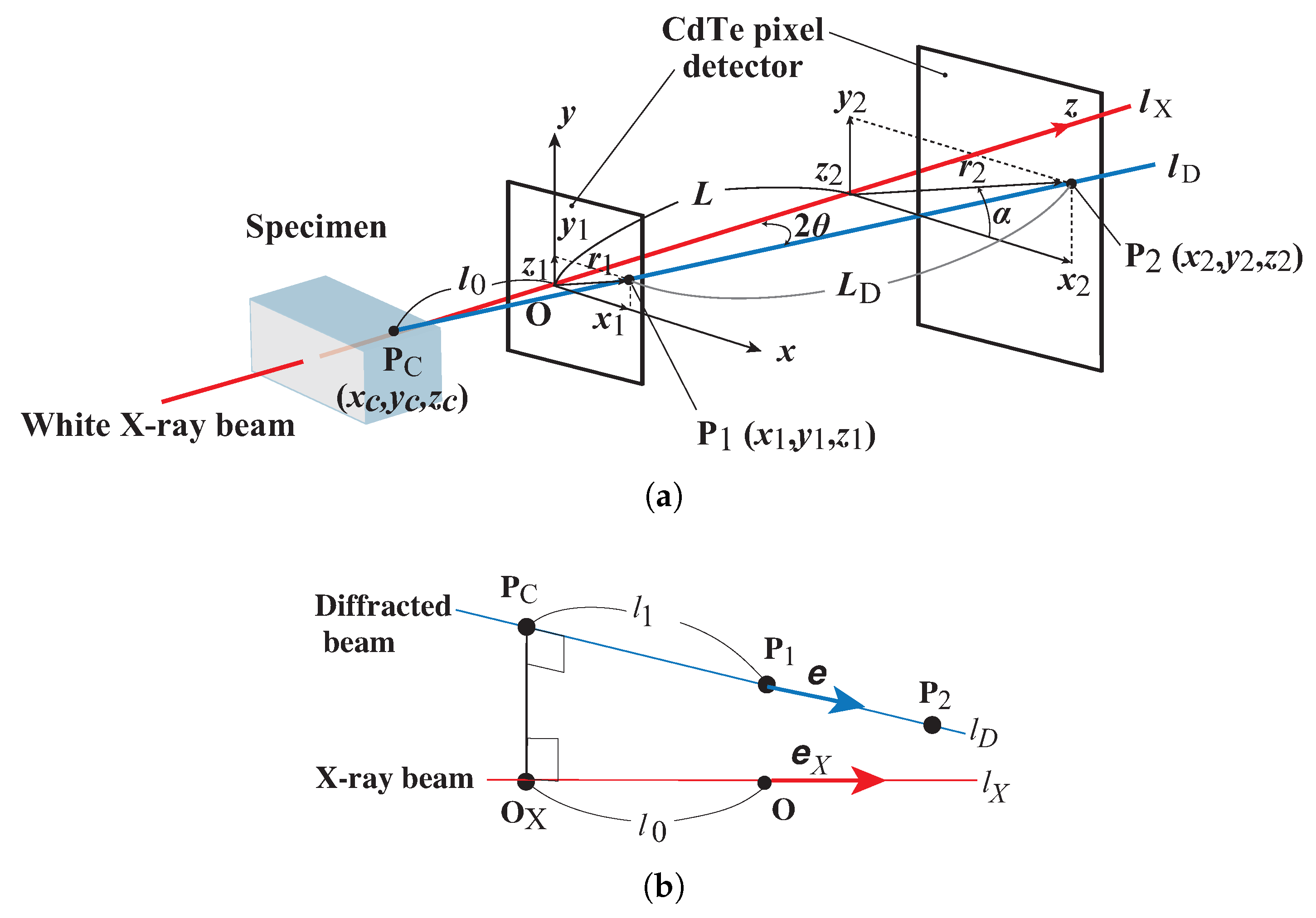
2.1. Double-Exposure Method
2.2. CdTe Pixel Detector
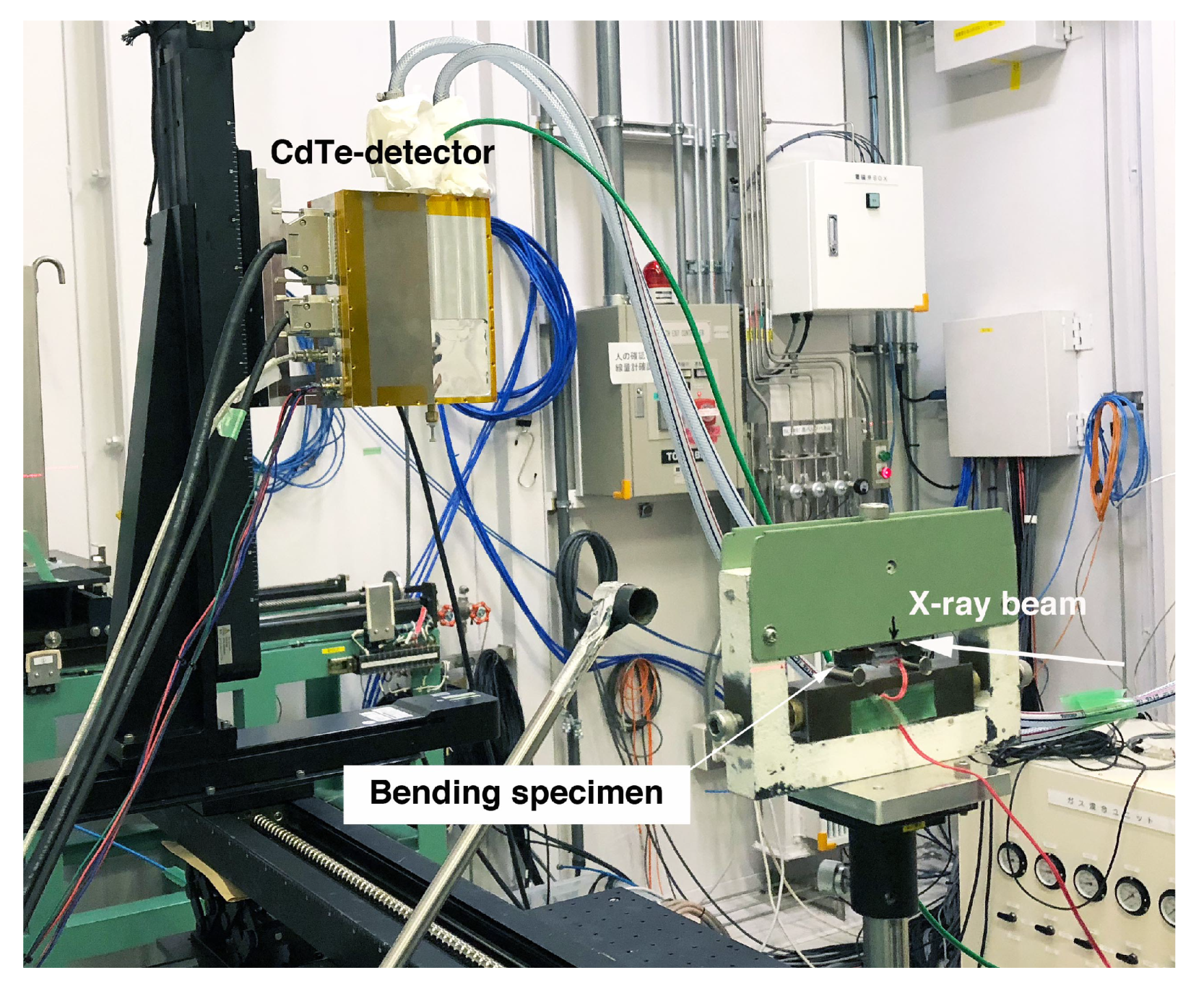
2.3. Experiments with Synchrotron White X-rays
3. Results and Discussion
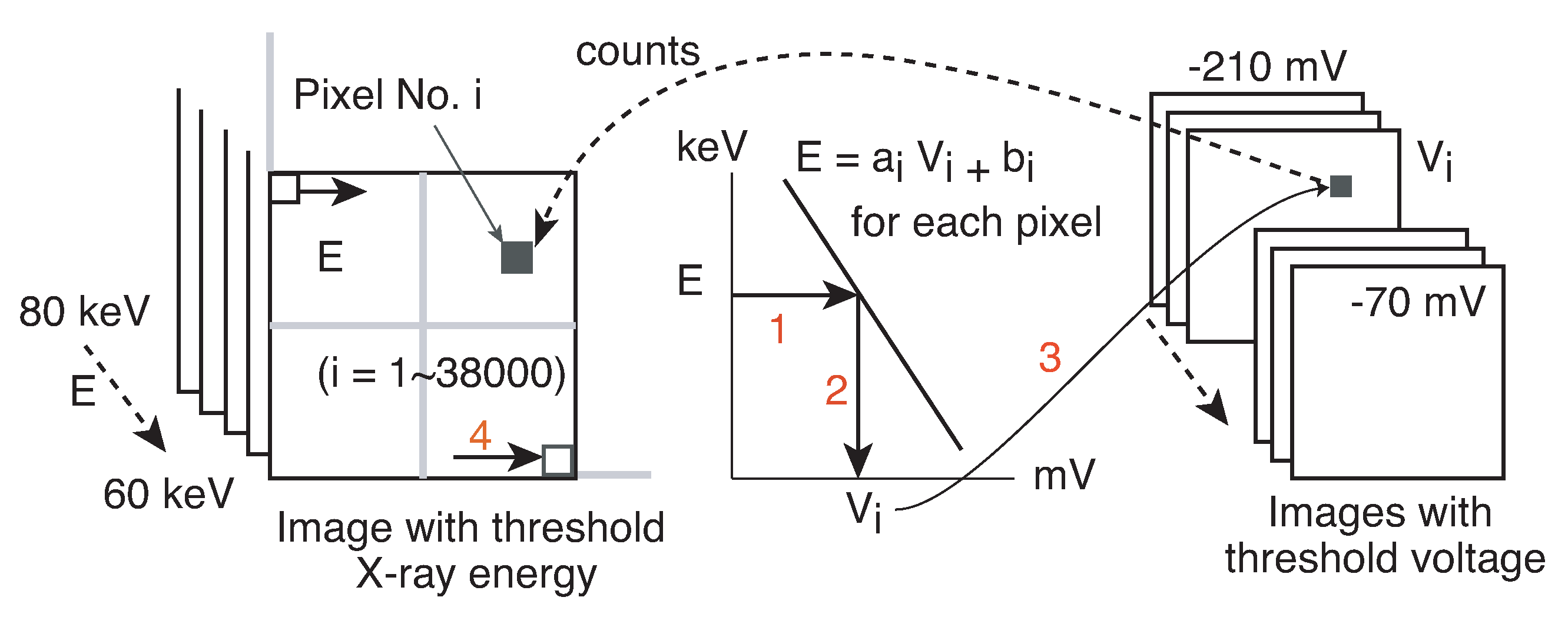
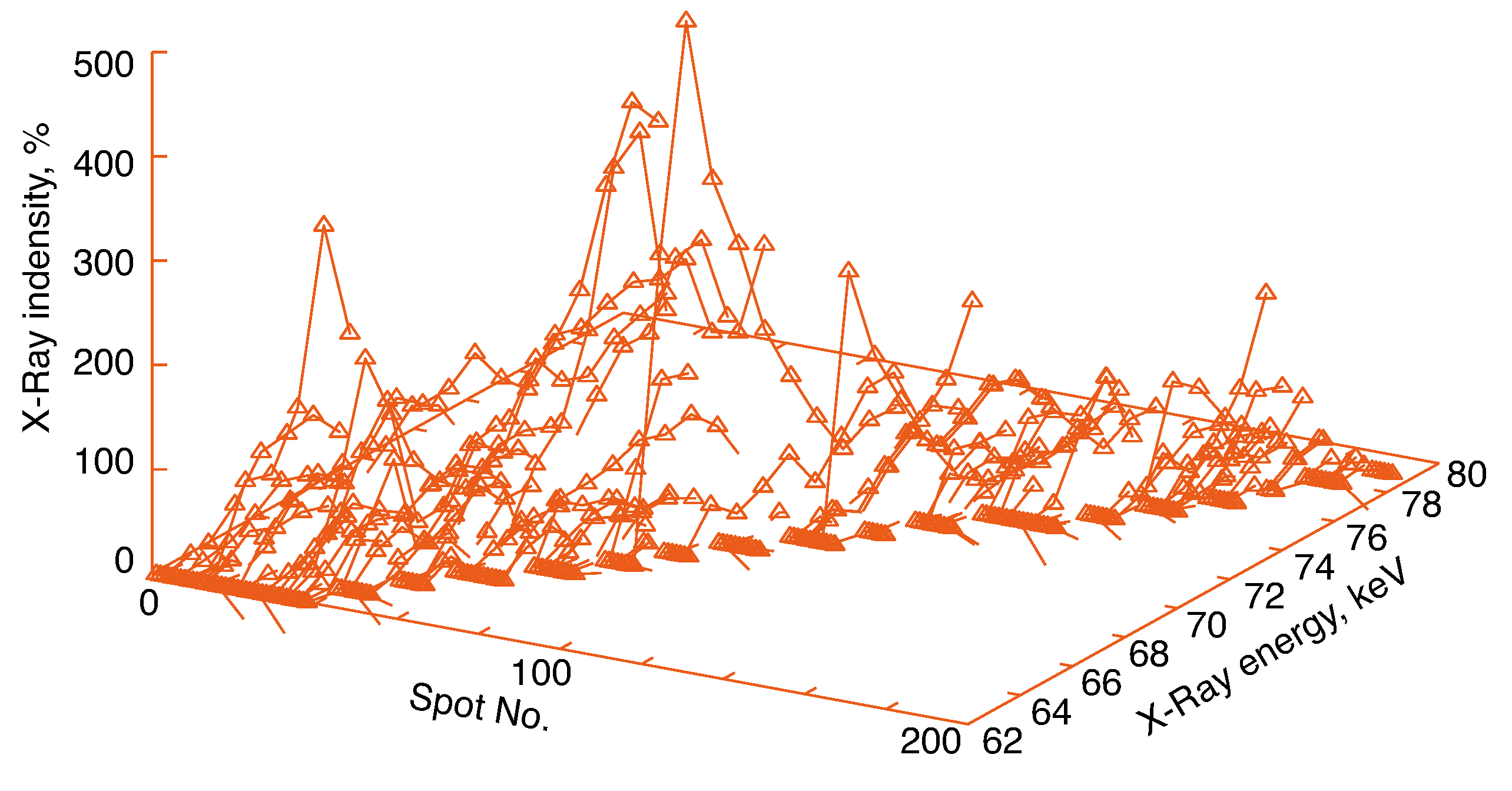
3.1. Energy Calibration of CdTe Pixel Detector
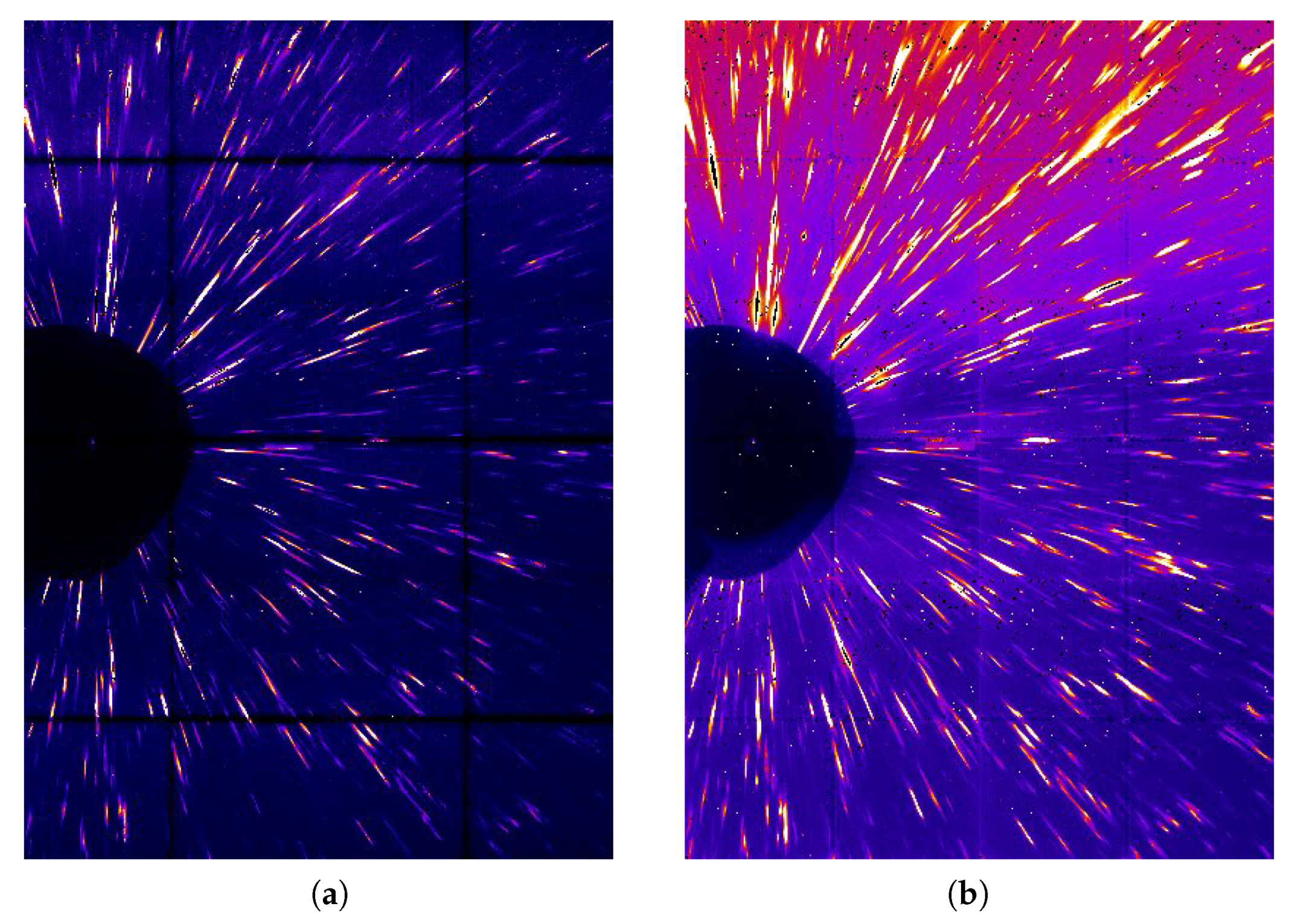
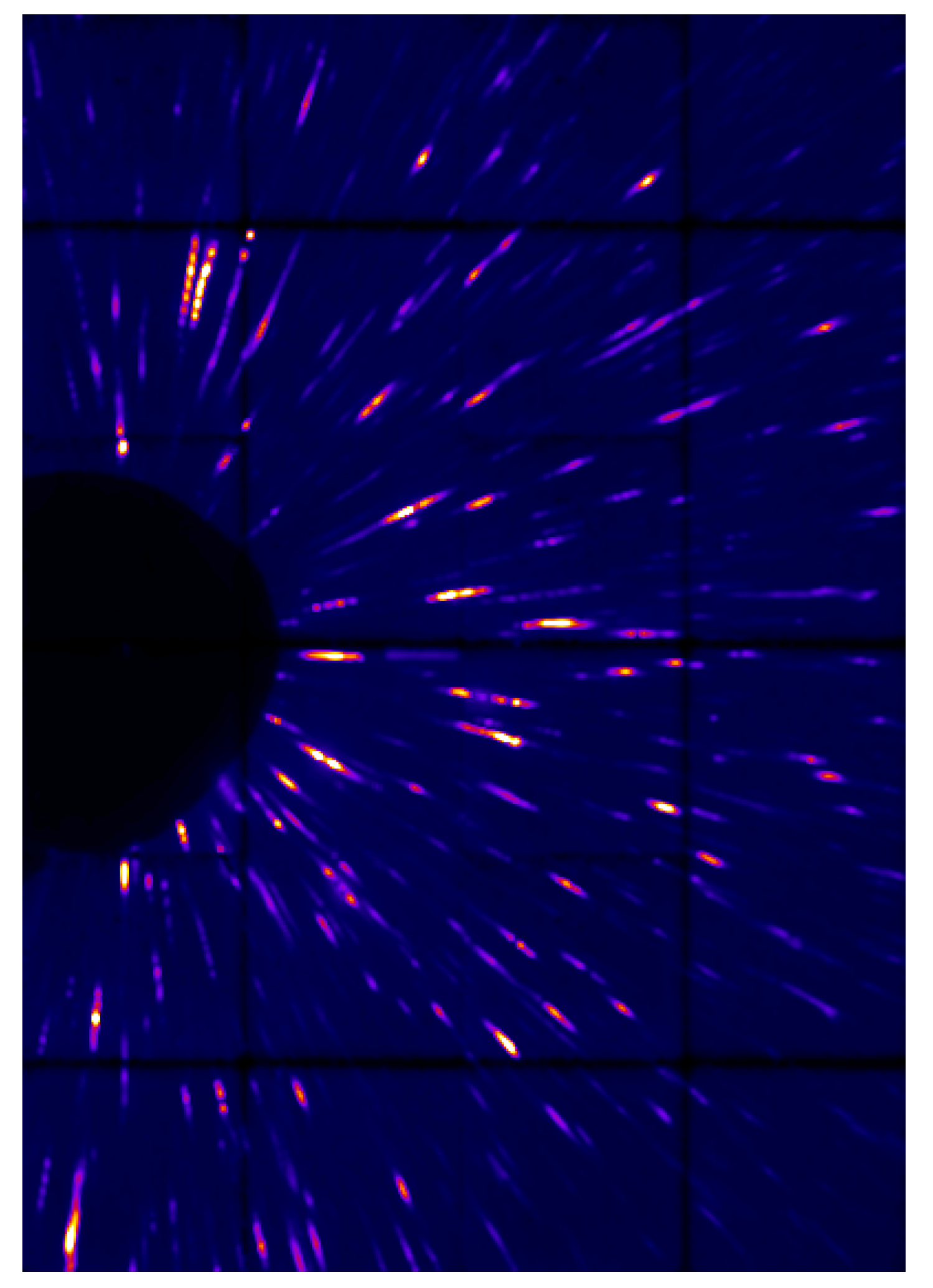
3.2. Constructing Images by X-ray Energy
- A value of the threshold X-ray energy E is determined.
- The threshold voltage is determined using E, , and .
- The X-ray intensity of the pixel is read out from the image measured with the threshold voltage .
- The above-mentioned operation is executed for all pixels.
- This procedure is applied for every X-ray energy.
- The intersection distance is within 5 mm, i.e., .
- The intersection position is within to mm.
- The obtained lattice spacing d has the consistency with the a lattice plane for a face-centered cubic system.
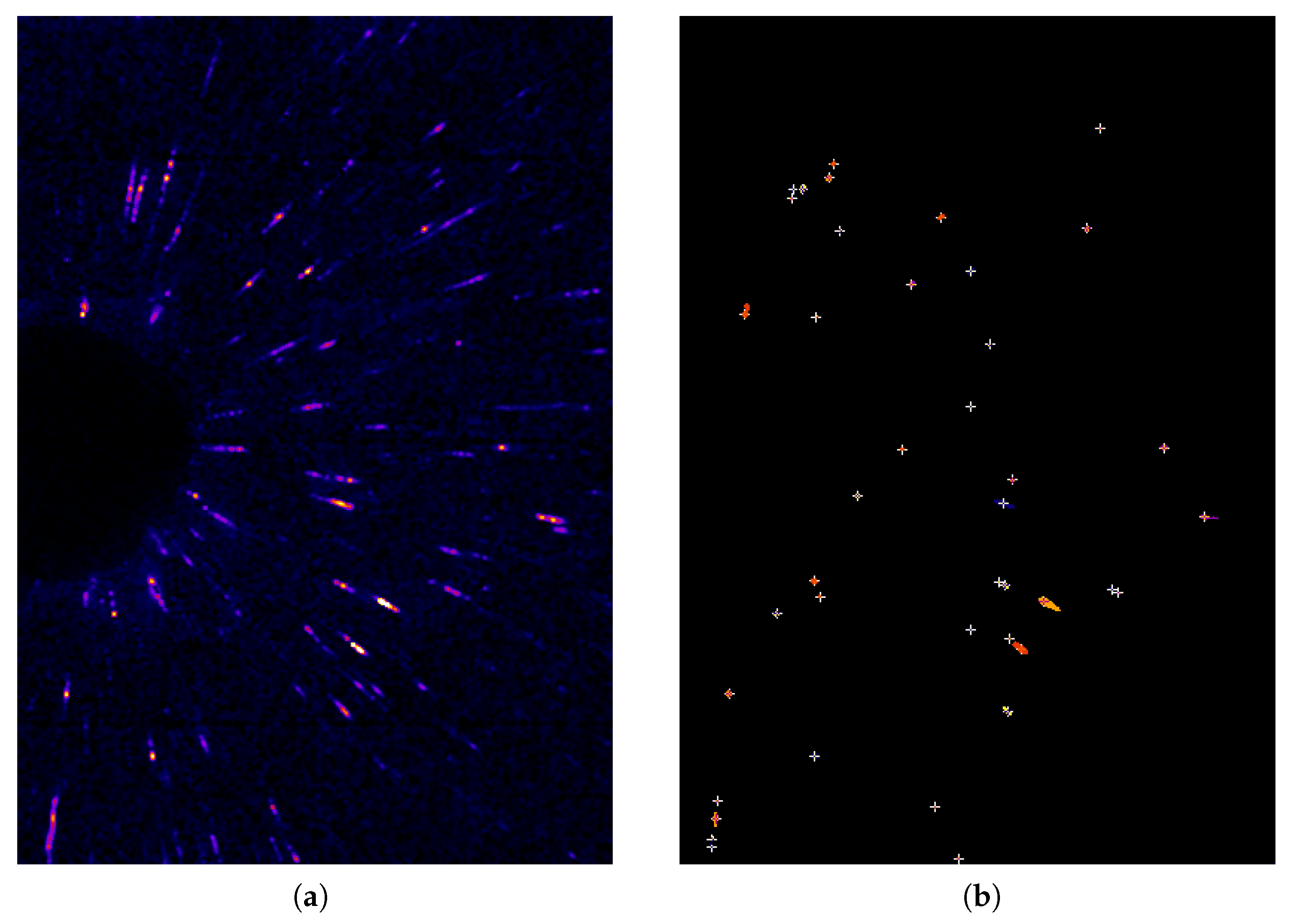
3.3. Measurement of Bending Strains
4. Conclusions
- (1)
- We proposed a new X-ray stress measurement method, a double-exposure method with a CdTe pixel detector, and synchrotron white X-rays. This method is useful for coarse-grained materials and large sample sizes.
- (2)
- The procedure and system for processing the image data measured with the CdTe pixel detector were discussed. As a result, we found that an X-ray energy image can be obtained from the difference between the energy threshold images.
- (3)
- The double-exposure method was applied to the diffraction spot image obtained from the above procedure. The distribution of the bending strain measured by our proposed method corresponded to the applied bending strain.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Liss, K.D.; Bartels, A.; Schreyer, A.; Clemens, H. High-Energy X-rays: A tool for advanced bulk investigations in materials science and physics. Textures Microstruct. 2003, 35, 219–252. [Google Scholar] [CrossRef]
- Withers, P.J. Use of synchrotron X-ray radiation for stress measurement. In Analysis of Residual Stress by Diffraction Using Neutron and Synchrotron Radiation; Fitzpatrick, M.E., Lodini, A., Eds.; Taylor & Francis: Abingdon, UK, 2003; pp. 170–189. [Google Scholar]
- Nielsen, S.F.; Wolf, A.; Poulsen, H.F.; Ohler, M.; Lienert, U.; Owen, R.A. A conical slit for three-dimensional XRD mapping. J. Synchrotron Rad. 2000, 7, 103–109. [Google Scholar] [CrossRef]
- Martins, R.V.; Honkimäki, V. Depth resolved strain and phase mapping of dissimilar friction stir welds using high energy synchrotron radiation. Textures Microstruct. 2003, 35, 145–152. [Google Scholar] [CrossRef] [Green Version]
- Martins, R.V. Residual stress analysis by monochromatic high-energy X-rays. In Neutrons and Synchrotron Radiation in Engineering Materials Science; Reimers, W., Pyzalla, A.R., Schreyer, A., Clemens, H., Eds.; Wiley-VHC: Weinheim, Germany, 2008; pp. 177–194. [Google Scholar]
- Martins, R.V.; Ohms, C.; Decroos, K. Full 3D spatially resolved mapping of residual strain in a 316L austenitic stainless steel weld specimen. Mater. Sci. Eng. A 2010, 527, 4779–4787. [Google Scholar] [CrossRef]
- Suzuki, K.; Shobu, T.; Shiro, A.; Toyokawa, H. Evaluation of internal stresses using rotating-slit and 2D detector. Mater. Sci. Forum 2014, 772, 15–19. [Google Scholar] [CrossRef]
- Suzuki, K.; Shobu, T.; Shiro, A.; Zhang, S. Internal stress measurement of weld part using diffraction spot trace method. Mater. Sci. Forum 2014, 777, 155–160. [Google Scholar] [CrossRef]
- Suzuki, K.; Shobu, T.; Shiro, A. Stress measurements of coarse grain materials using double exposure method with hard synchrotron X-rays. Mater. Res. Proc. 2018, 6, 69–74. [Google Scholar]
- Broennimann, C.; Eikenberry, E.F.; Henrich, B.; Horisberger, R.; Huelsen, G.; Pohl, E.; Schmitt, B.; Schulze-Briese, C.; Suzuki, M.; Tomisaki, T.; et al. The PILATUS 1M detector. J. Synchrotron Radiat. 2006, 13, 120–130. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Poulsen, H.F. Three-Dimensional X-ray Diffraction Microscopy; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Tamura, N. A versatile tool for analyzing synchrotron X-ray microdiffraction data. In Strain and Dislocation Gradients from Diffraction: Spatially-Resolved Local Structure and Defects; Barabash, R., Ice, G., Eds.; Imperial College Press: London, UK, 2014; pp. 156–204. [Google Scholar]
- Laue Tools: Laue X-Ray Microdiffraction Analysis Software. Available online: https://gitlab.esrf.fr/micha/lauetools/ (accessed on 9 June 2020).
- Robach, O.; Micha, J.S.; Ulrich, O.; Gergaud, P. Full local elastic strain tensor from Laue microdiffraction: Simultaneous Laue pattern and spot energy measurement. J. Appl. Cryst. 2011, 44, 688–696. [Google Scholar] [CrossRef]
- Ibrahim, M.; Castelier, É.; Palancher, H.; Bornert, M.; Caré, S.; Micha, J.S. Laue pattern analysis for two-dimensional strain mapping in light-ion-implanted polycrystals. J. Appl. Cryst. 2015, 48, 990–999. [Google Scholar] [CrossRef]
- Altinkurt, G.; Févre, M.; Geandier, G.; Robach, O.; Guernaoui, S.; Dehmas, M. Effect of thermal and mechanical loadings on the residual stress field in a nickel based superalloy using X-ray Laue microdiffraction. In Residual Stresses 2016; Holden, T.M., Muránsky, O., Edwards, L., Eds.; Materials Research Forum: Millersville, PA, USA, 2017; pp. 527–532. [Google Scholar]
- Toyokawa, H.; Saji, C.; Kawase, M.; Wu, S.; Furukawa, Y.; Kajiwara, K.; Sata, M.; Hirono, T.; Shiro, A.; Shobu, T.; et al. Development of CdTe pixel detectors combined with an aluminum Schottky diode sensor and photon-counting ASICs. J. Instrum. 2017, 12, C01044. [Google Scholar] [CrossRef]
- Toyokawa, H.; Saji, C.; Kawase, M.; Ohara, K.; Shiro, A.; Yasuda, R.; Shobu, T.; Suenaga, A.; Ikeda, H. Development of CdTe pixel detectors for energy-resolved X-ray diffractions. In Proceedings of the Second International Symposium on Radiation Detectors and Their Uses (ISRD2018), Tsukuba, Japan, 23–26 January 2019; Volume 24, p. 011015. [Google Scholar]
- Kröner, E. Berechnung der elastischen Konstanten des Vierkristalls aus den Konstanten des Einkristalls. Z. Physik 1958, 151, 504–518. [Google Scholar] [CrossRef]
- Suzuki, K. X-ray and Mechanical Elastic Constants for Cubic System By Kröner Model. Available online: http://www.rigaku.co.jp/app/kroner/kroner_c.html (accessed on 15 April 2020).






© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suzuki, K.; Shiro, A.; Toyokawa, H.; Saji, C.; Shobu, T. Double-Exposure Method with Synchrotron White X-ray for Stress Evaluation of Coarse-Grain Materials. Quantum Beam Sci. 2020, 4, 25. https://doi.org/10.3390/qubs4030025
Suzuki K, Shiro A, Toyokawa H, Saji C, Shobu T. Double-Exposure Method with Synchrotron White X-ray for Stress Evaluation of Coarse-Grain Materials. Quantum Beam Science. 2020; 4(3):25. https://doi.org/10.3390/qubs4030025
Chicago/Turabian StyleSuzuki, Kenji, Ayumi Shiro, Hidenori Toyokawa, Choji Saji, and Takahisa Shobu. 2020. "Double-Exposure Method with Synchrotron White X-ray for Stress Evaluation of Coarse-Grain Materials" Quantum Beam Science 4, no. 3: 25. https://doi.org/10.3390/qubs4030025
APA StyleSuzuki, K., Shiro, A., Toyokawa, H., Saji, C., & Shobu, T. (2020). Double-Exposure Method with Synchrotron White X-ray for Stress Evaluation of Coarse-Grain Materials. Quantum Beam Science, 4(3), 25. https://doi.org/10.3390/qubs4030025





