The Large-Area Detector for Small-Angle Neutron Scattering on iMATERIA at J-PARC
Abstract
:1. Introduction
2. Instrumentation
2.1. 3He PSD Tube
2.2. 3He PSD Tube Arrangement
2.3. Readout Module and Data Processing Software
3. Data Processing
3.1. Position Sensitive Detection by Charge Division Method
3.2. Event-Mode Data Histograming
3.3. Calculation of Geometric Arrays
y(i, j) = β(j)y1(i) + (1 − β(j))y2(i),
z(i, j) = β(j)z1(i) + (1 − β(j))z2(i).
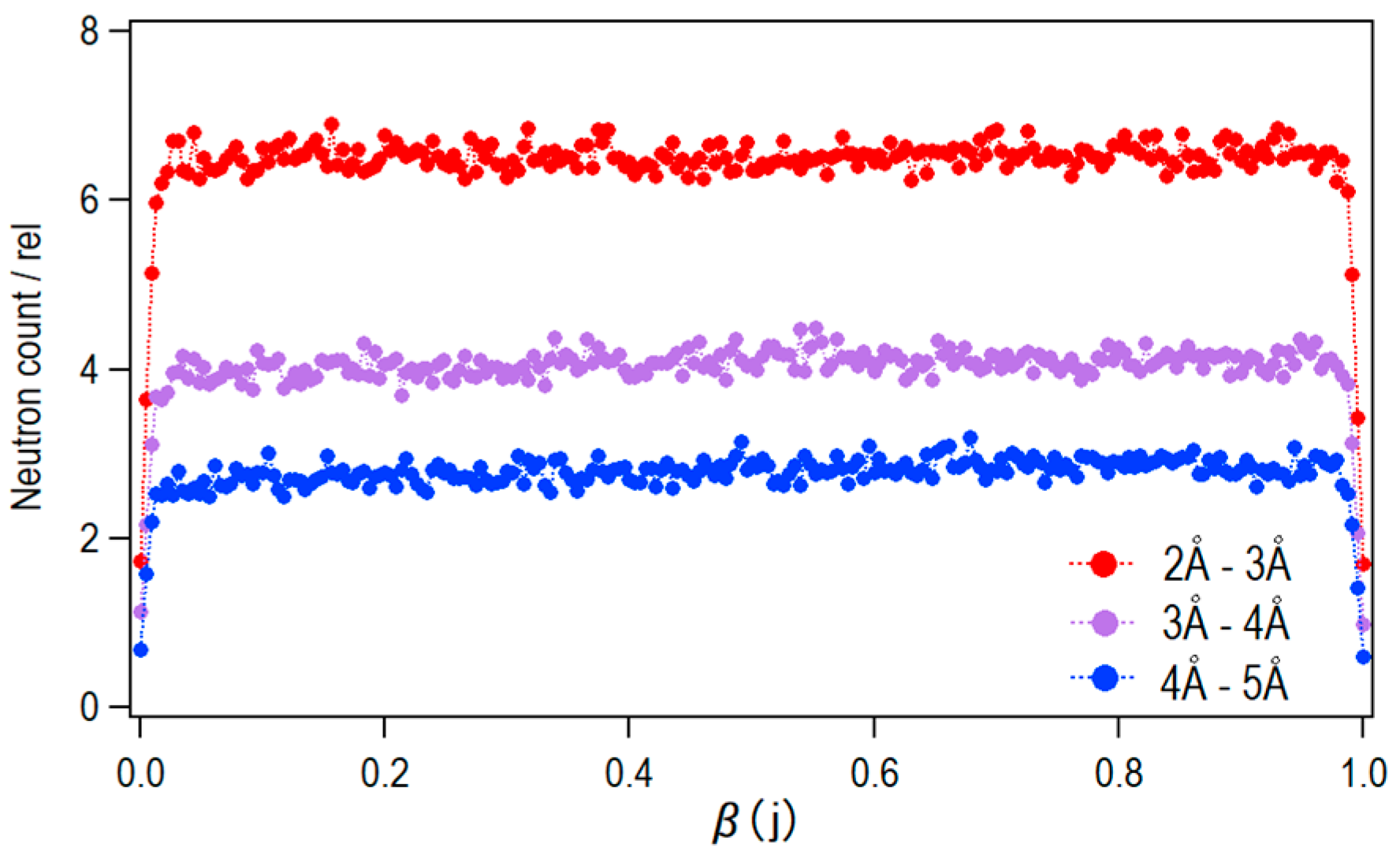
3.4. One-dimensional Profile Calculation
4. Results and Discussion
4.1. Calibration of Position Detection
4.2. Efficiency of Each PSD, ηPSD(i)
4.3. Neutron Wavelength Dependence of Detection Efficiency, ηλ(λ)
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Heller, W.T.; Cuneo, M.J.; DeBeer-Schmitt, L.M.; Do, C.; He, L.; Heroux, L.; Littrell, K.; Pingali, S.V.; Qian, S.; Stanley, C.B.; et al. The suite of small-angle neutron scattering instruments at Oak Ridge National Laboratory. J. Appl. Crystallogr. 2018, 51, 242–248. [Google Scholar] [CrossRef]
- NIST Center for Neutron Research, Neutron Instruments. Available online: https://www.nist.gov/ncnr/neutron-instruments (accessed on 22 September 2020).
- Lindner, P.; Schweins, R. The D11 Small-Angle Scattering Instrument: A New Benchmark for SANS. Neutron News 2010, 21, 15–18. [Google Scholar] [CrossRef]
- Dewhurst, C.; Grillo, I.; Honecker, D.; Bonnaud, M.; Jacques, M.; Amrouni, C.; Perillo-Marcone, A.; Manzin, G.; Cubitt, R. The small-angle neutron scattering instrument D33 at the Institut Laue–Langevin. J. Appl. Crystallogr. 2016, 49, 1–14. [Google Scholar] [CrossRef]
- Feoktystov, A.; Frielinghaus, H.; Di, Z.; Jaksch, S.; Pipich, V.; Appavou, M.-S.; Babcock, E.; Hanslik, R.; Engels, R.; Kemmerling, G.; et al. KWS-1 high-resolution small-angle neutron scattering instrument at JCNS: Current state. J. Appl. Crystallogr. 2015, 48, 61–70. [Google Scholar] [CrossRef]
- Houston, J.E.; Brandl, G.; Drochner, M.; Kemmerling, G.; Engels, R.; Papagiannopoulos, A.; Sarter, M.; Stadler, A.; Radulescu, A. The high-intensity option of the SANS diffractometer KWS-2 at JCNS – characterization and performance of the new multi-megahertz detection system. J. Appl. Cryst. 2018, 51, 323–336. [Google Scholar] [CrossRef]
- Mühlbauer, S.; Heinemann, A.; Wilhelm, A.; Karge, L.; Ostermann, A.; Defendi, I.; Schreyer, A.; Petry, W.; Gilles, R. The new small-angle neutron scattering instrument SANS-1 at MLZ—Characterization and first results. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2016, 832, 297–305. [Google Scholar] [CrossRef] [Green Version]
- Wood, K.; Jeffries, C.M.; Knott, R.B.; Sokolova, A.V.; Jacques, D.; Duff, A.P. Exploring the structure of biological macromolecules in solution using Quokka, the small angle neutron scattering instrument, at ANSTO. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2015, 798, 44–51. [Google Scholar] [CrossRef]
- Wood, K.; Mata, J.; Garvey, C.J.; Wu, C.-M.; Hamilton, W.A.; Abbeywick, P.; Bartlett, D.; Bartsch, F.; Baxter, P.; Booth, N.; et al. QUOKKA, the pinhole small-angle neutron scattering instrument at the OPAL Research Reactor, Australia: Design, performance, operation and scientific highlights. J. Appl. Crystallogr. 2018, 51, 294–314. [Google Scholar] [CrossRef]
- Sokolova, A.V.; Whitten, A.E.; De Campo, L.; Christoforidis, J.; Eltobaji, A.; Barnes, J.; Darmann, F.; Berry, A. Performance and characteristics of the BILBY time-of-flight small-angle neutron scattering instrument. J. Appl. Crystallogr. 2019, 52, 1–12. [Google Scholar] [CrossRef]
- Han, Y.-S.; Choi, S.-M.; Kim, T.-H.; Lee, C.-H.; Cho, S.-J.; Seong, B.-S. A new 40m small angle neutron scattering instrument at HANARO, Korea. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2013, 721, 17–20. [Google Scholar] [CrossRef]
- Koizumi, S.; Iwase, H.; Suzuki, J.-I.; Oku, T.; Motokawa, R.; Sasao, H.; Tanaka, H.; Yamaguchi, D.; Shimizu, H.M.; Hashimoto, T. Focusing and polarized neutron small-angle scattering spectrometer (SANS-J-II). The challenge of observation over length scales from an ångström to a micrometre. J. Appl. Crystallogr. 2007, 40, s474–s479. [Google Scholar] [CrossRef]
- Noda, Y.; Koizumi, S.; Yamaguchi, D. Multi-tube area detector developed for reactor small-angle neutron scattering spectrometer SANS-J-II. J. Appl. Crystallogr. 2016, 49, 128–138. [Google Scholar] [CrossRef]
- Okabe, S.; Karino, T.; Nagao, M.; Watanabe, S.; Shibayama, M. Current status of the 32m small-angle neutron scattering instrument, SANS-U. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2007, 572, 853–858. [Google Scholar] [CrossRef]
- Zhao, J.; Gao, C.Y.; Liu, D. The extendedQ-range small-angle neutron scattering diffractometer at the SNS. J. Appl. Crystallogr. 2010, 43, 1068–1077. [Google Scholar] [CrossRef] [Green Version]
- Zhao, J. Data processing for the SNS EQ-SANS diffractometer. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2011, 647, 107–111. [Google Scholar] [CrossRef]
- Duxbury, D.; Heenan, R.; McPhail, D.; Raspino, D.; Rhodes, N.; Rogers, S.; Schooneveld, E.; Spill, E.; Terry, A. Performance characteristics of the new detector array for the SANS2d instrument on the ISIS spallation neutron source. J. Instrum. 2014, 9, C12051. [Google Scholar] [CrossRef]
- Takata, S.-I.; Suzuki, J.-I.; Shinohara, T.; Oku, T.; Tominaga, T.; Ohishi, K.; Iwase, H.; Nakatani, T.; Inamura, Y.; Ito, T.; et al. The Design and q Resolution of the Small and Wide Angle Neutron Scattering Instrument (TAIKAN) in J-PARC. In Proceedings of the 2nd International Symposium on Science at J-PARC—Unlocking the Mysteries of Life, Matter and the Universe—; Physical Society of Japan: Tokyo, Japan, 2015; Volume 8, p. 036020. [Google Scholar]
- Iwase, H.; Takata, S.-I.; Morikawa, T.; Katagiri, M.; Birumachi, A.; Suzuki, J.-I. Installation of a high-resolution position-sensitive scintillation detector in the small and wide angle neutron scattering instrument (TAIKAN), MLF, J-PARC. Phys. B Condens. Matter 2018, 551, 501–505. [Google Scholar] [CrossRef]
- Andersen, K.; Argyriou, D.; Jackson, A.; Houston, J.; Henry, P.; Deen, P.; Toft-Petersen, R.; Beran, P.; Strobl, M.; Arnold, T.; et al. The instrument suite of the European Spallation Source. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2020, 957, 163402. [Google Scholar] [CrossRef]
- Seeger, P.A.; Jnr, R.P.H. Small-angle neutron scattering at pulsed spallation sources. J. Appl. Crystallogr. 1991, 24, 467–478. [Google Scholar] [CrossRef] [Green Version]
- Thiyagarajan, P.; Epperson, J.E.; Crawford, R.K.; Carpenter, J.M.; Klippert, T.E.; Wozniak, D.G. The Time-of-Flight Small-Angle Neutron Diffractometer (SAD) at IPNS, Argonne National Laboratory. J. Appl. Crystallogr. 1997, 30, 280–293. [Google Scholar] [CrossRef]
- Heenan, R.K.; Penfold, J.; King, S.M. SANS at pulsed spallation sources: Present and future prospect. J. Appl. Cryst. 1997, 30, 1140–1147. [Google Scholar] [CrossRef]
- Ishigaki, T.; Hoshikawa, A.; Yonemura, M.; Morishima, T.; Kamiyama, T.; Oishi-Tomiyasu, R.; Aizawa, K.; Sakuma, T.; Tomota, Y.; Arai, M.; et al. IBARAKI materials design diffractometer (iMATERIA)—Versatile neutron diffractometer at J-PARC. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2009, 600, 189–191. [Google Scholar] [CrossRef]
- Nakajima, K.; Kawakita, Y.; Itoh, S.; Abe, J.; Aizawa, K.; Aoki, H.; Endo, H.; Fujita, M.; Funakoshi, K.-I.; Gong, W.; et al. Materials and Life Science Experimental Facility (MLF) at the Japan Proton Accelerator Research Complex II: Neutron Scattering Instruments. Quantum Beam Sci. 2017, 1, 9. [Google Scholar] [CrossRef] [Green Version]
- Sakasai, K.; Satoh, S.; Seya, T.; Nakamura, T.; Toh, K.; Yamagishi, H.; Soyama, K.; Yamazaki, D.; Maruyama, R.; Oku, T.; et al. Materials and Life Science Experimental Facility at the Japan Proton Accelerator Research Complex III: Neutron Devices and Computational and Sample Environments. Quantum Beam Sci. 2017, 1, 10. [Google Scholar] [CrossRef] [Green Version]
- Takada, H.; Haga, K.; Teshigawara, M.; Aso, T.; Meigo, S.-I.; Kogawa, H.; Naoe, T.; Wakui, T.; Ooi, M.; Harada, M.; et al. Materials and Life Science Experimental Facility at the Japan Proton Accelerator Research Complex I: Pulsed Spallation Neutron Source. Quantum Beam Sci. 2017, 1, 8. [Google Scholar] [CrossRef] [Green Version]
- Satoh, S.; Muto, S.; Kaneko, N.; Uchida, T.; Tanaka, M.; Yasu, Y.; Nakayoshi, K.; Inoue, E.; Sendai, H.; Nakatani, T.; et al. Development of a readout system employing high-speed network for J-PARC. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers, Detect. Assoc. Equip. 2009, 600, 103–106. [Google Scholar] [CrossRef]
- Nakayoshi, K.; Yasu, Y.; Inoue, E.; Sendai, H.; Tanaka, M.; Satoh, S.; Muto, S.; Kaneko, N.; Otomo, T.; Nakatani, T.; et al. Development of a data acquisition sub-system using DAQ-Middleware. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2009, 600, 173–175. [Google Scholar] [CrossRef]
- He, L.; Do, C.; Qian, S.; Wignall, G.; Heller, W.T.; Littrell, K.; Smith, G.S. Corrections for the geometric distortion of the tube detectors on SANS instruments at ORNL. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2015, 775, 63–70. [Google Scholar] [CrossRef] [Green Version]
- Brulet, A.; Lairez, D.; Lapp, A.; Cotton, J.-P. Improvement of data treatment in small-angle neutron scattering. J. Appl. Crystallogr. 2007, 40, 165–177. [Google Scholar] [CrossRef]
- Grillo, I. Small-Angle Neutron Scattering and Applications in Soft Condensed Matter. In Soft Matter Characterization; Springer Science and Business Media LLC: Berlin, Germany, 2008; pp. 723–782. [Google Scholar]
- Zhang, F.; Ilavsky, J.; Long, G.G.; Quintana, J.P.G.; Allen, A.J.; Jemian, P.R. Glassy Carbon as an Absolute Intensity Calibration Standard for Small-Angle Scattering. Met. Mater. Trans. A 2009, 41, 1151–1158. [Google Scholar] [CrossRef] [Green Version]
- Holderer, O.; Carmo, M.; Shviro, M.; Lehnert, W.; Noda, Y.; Koizumi, S.; Appavou, M.-S.; Appel, M.; Frielinghaus, H. Fuel Cell Electrode Characterization Using Neutron Scattering. Materials 2020, 13, 1474. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhao, Y.; Yoshimura, K.; Mahmoud, A.M.A.; Yu, H.-C.; Okushima, S.; Hiroki, A.; Kishiyama, Y.; Shishitani, H.; Yamaguchi, S.; Tanaka, H.; et al. A long side chain imidazolium-based graft-type anion-exchange membrane: Novel electrolyte and alkaline-durable properties and structural elucidation using SANS contrast variation. Soft Matter 2020. [Google Scholar] [CrossRef] [PubMed]
- Koizumi, S.; Noda, Y.; Maeda, T.; Inada, T.; Ueda, S.; Fujisawa, T.; Izunome, H.; Robinson, R.A.; Frielingshaus, H. Advanced Small-angle scattering Instrument Available in Tokyo area II. Time-of-flight Small-Angle Neutron Scattering Developed on iMATERIA Spectrometer at High Intensity Pulsed Neutron Source J-PARC. Quantum Beam Sci. 2020. submitted. [Google Scholar]

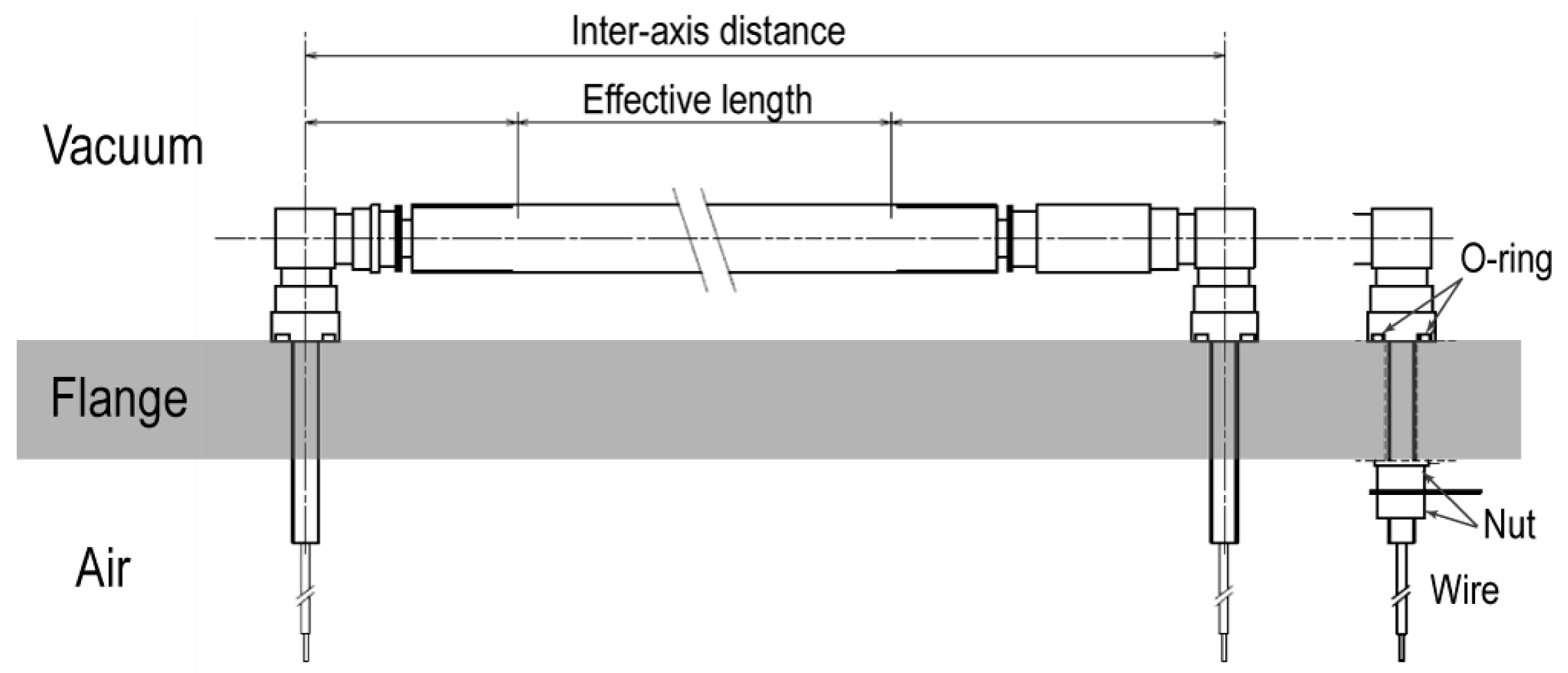

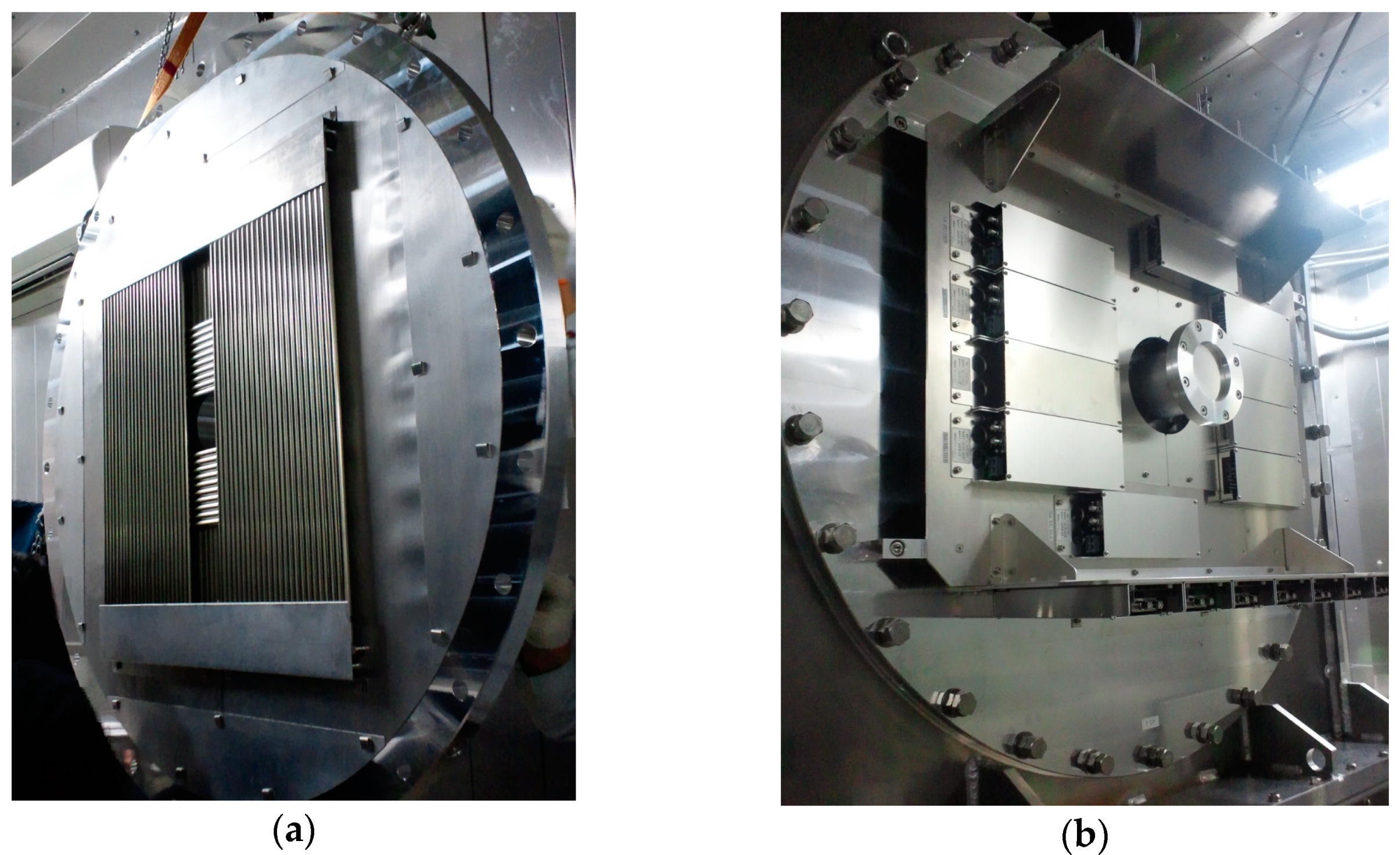
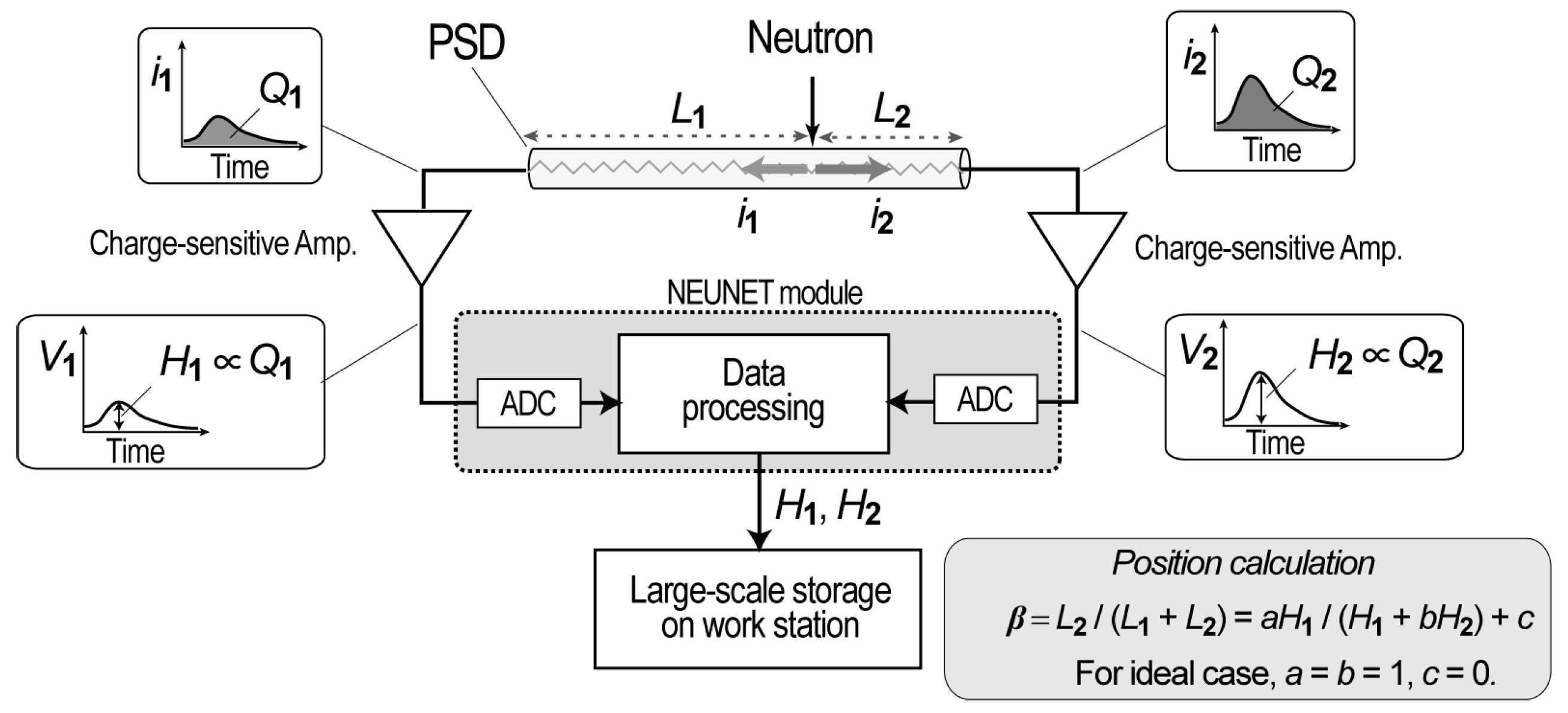

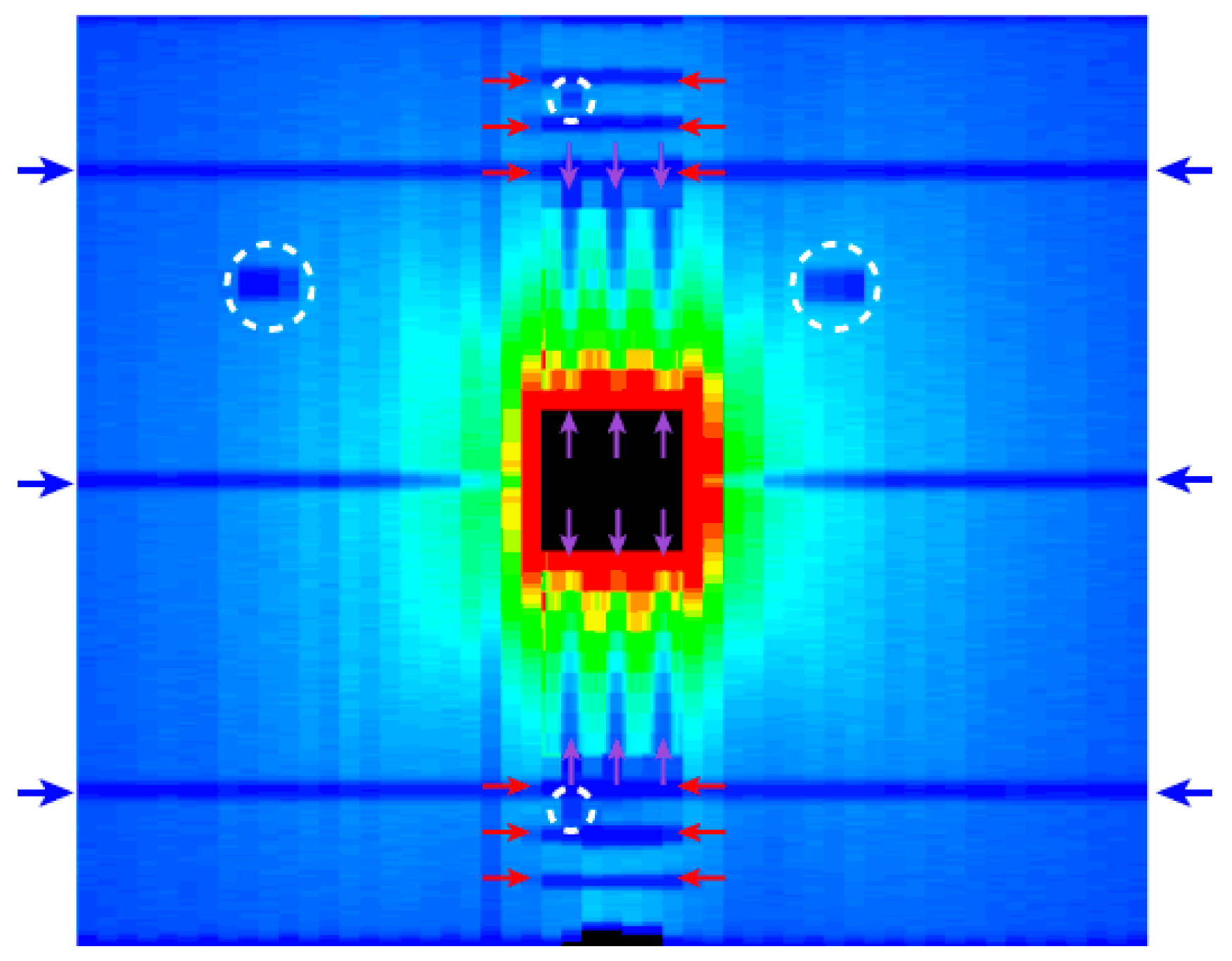

| Product Code | Effective Length [mm] | Inter-Axis Distance [mm] |
|---|---|---|
| E6867-600 | 600 | 749 |
| E6867-127 | 127 | 230 |
| E6867-100 | 100 | 203 |
| Detector Area (SA bank) | 689 mm × 600 mm 91 mm × 91 mm (Central hole) |
| Number of PSD tubes | 46 (E6867-600) 14 (E6867-127) 20 (E6867-100) |
| Scattering angle, 2θ | 0.6°–5.5° (SA bank) 12°–40° (LA bank) |
| Moderator-to-sample distance | 26.500 m |
| Sample-to-detector distance | 4.684 m (first layer) 4.6695 m (second layer) 4.655 m (third layer) |
| Neutron wavelength range | 1–5 Å (single frame mode) 1–10 Å (double frame mode) |
| Standard collimation | 20 × 20 mm (2.17 m away from sample) 10 × 10 mm (0.22 m away from sample) |
| Total q range (double frame mode) | 0.007–0.60 Å−1 (SA bank) 0.13–4.3 Å−1 (LA bank) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Noda, Y.; Izunome, H.; Maeda, T.; Inada, T.; Ueda, S.; Koizumi, S. The Large-Area Detector for Small-Angle Neutron Scattering on iMATERIA at J-PARC. Quantum Beam Sci. 2020, 4, 32. https://doi.org/10.3390/qubs4040032
Noda Y, Izunome H, Maeda T, Inada T, Ueda S, Koizumi S. The Large-Area Detector for Small-Angle Neutron Scattering on iMATERIA at J-PARC. Quantum Beam Science. 2020; 4(4):32. https://doi.org/10.3390/qubs4040032
Chicago/Turabian StyleNoda, Yohei, Hideki Izunome, Tomoki Maeda, Takumi Inada, Satoru Ueda, and Satoshi Koizumi. 2020. "The Large-Area Detector for Small-Angle Neutron Scattering on iMATERIA at J-PARC" Quantum Beam Science 4, no. 4: 32. https://doi.org/10.3390/qubs4040032
APA StyleNoda, Y., Izunome, H., Maeda, T., Inada, T., Ueda, S., & Koizumi, S. (2020). The Large-Area Detector for Small-Angle Neutron Scattering on iMATERIA at J-PARC. Quantum Beam Science, 4(4), 32. https://doi.org/10.3390/qubs4040032





