Twisted Fibers Can Have an Adjustable Thermal Expansion †
Abstract
:1. Introduction
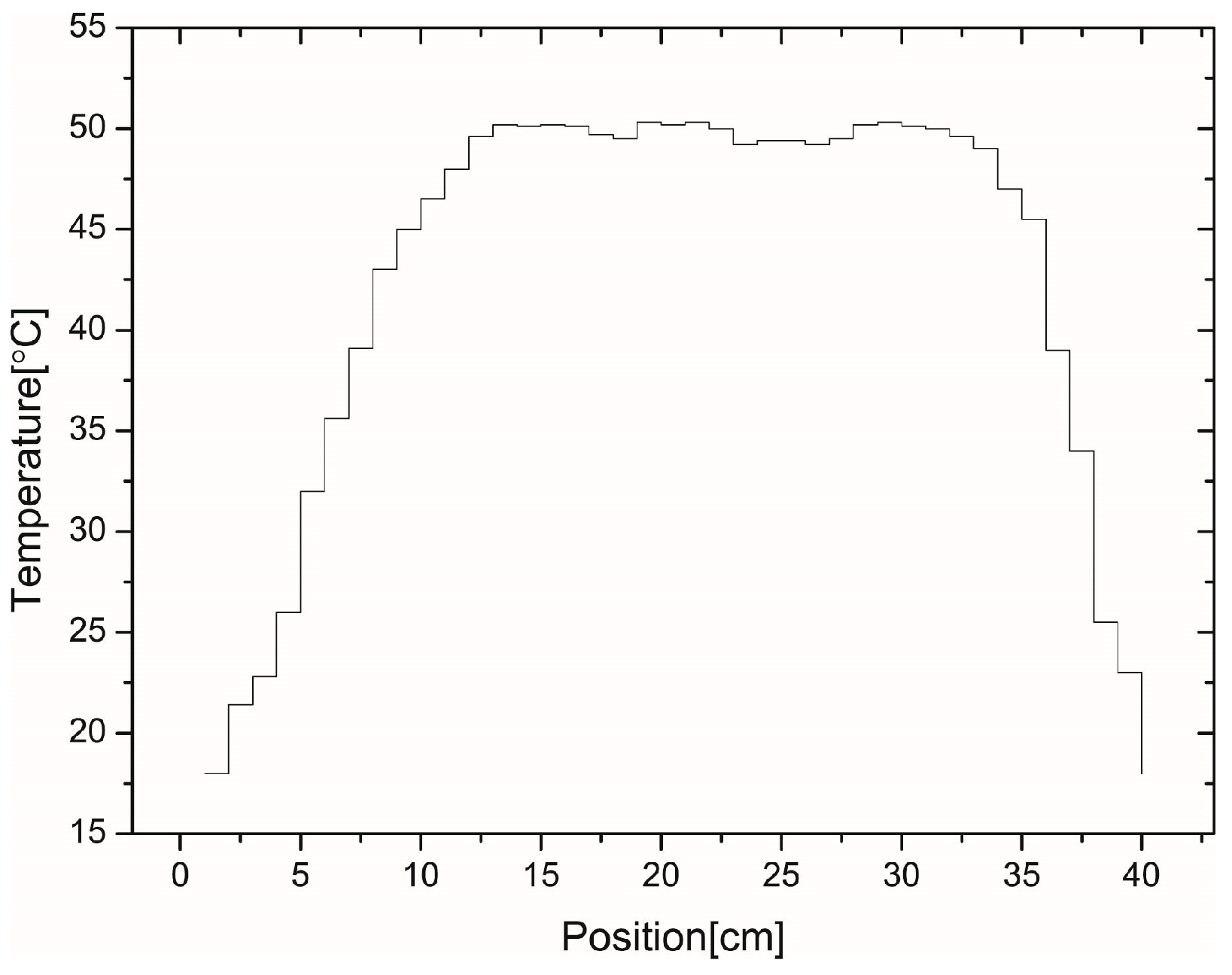
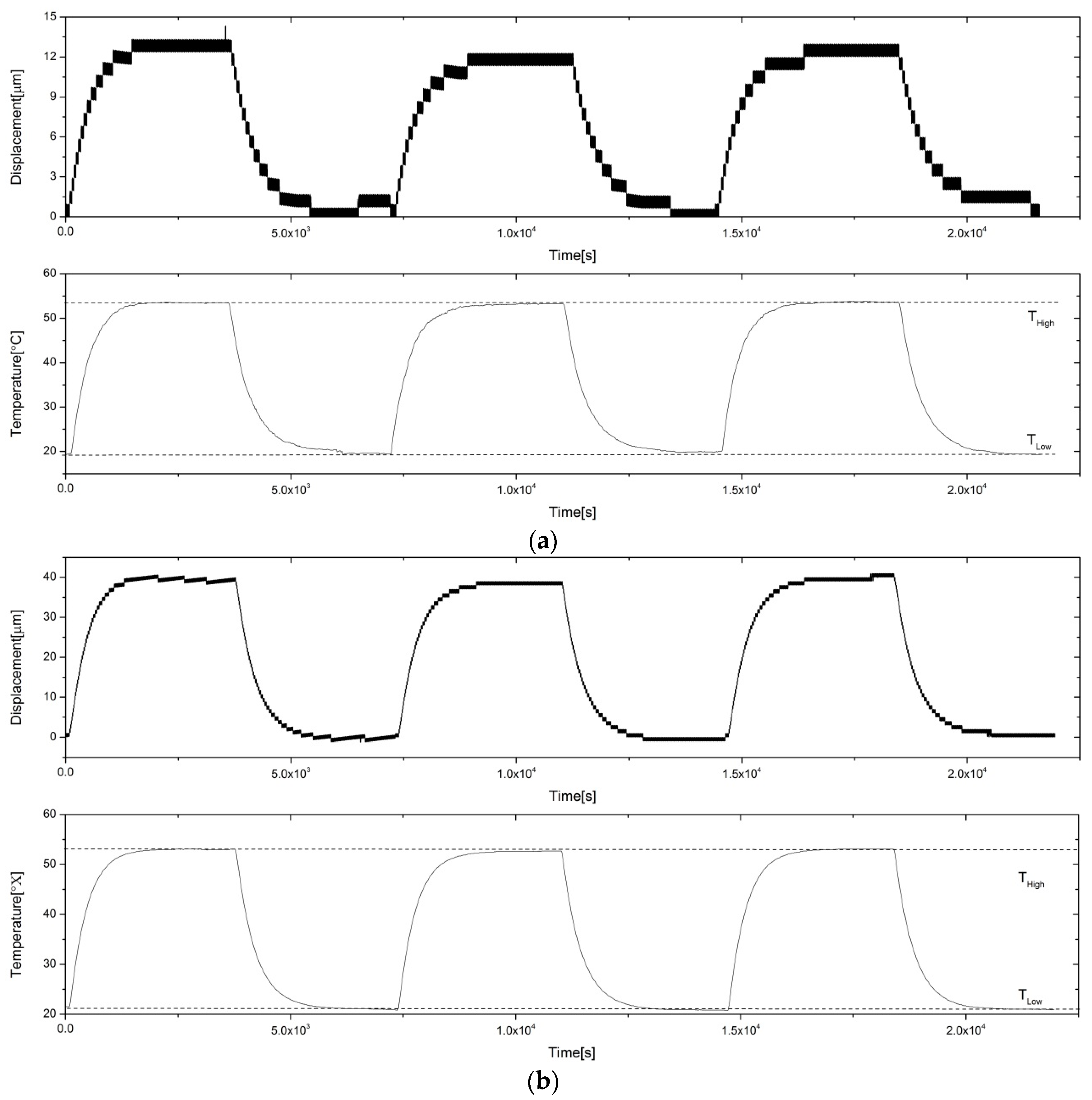
2. Experiment
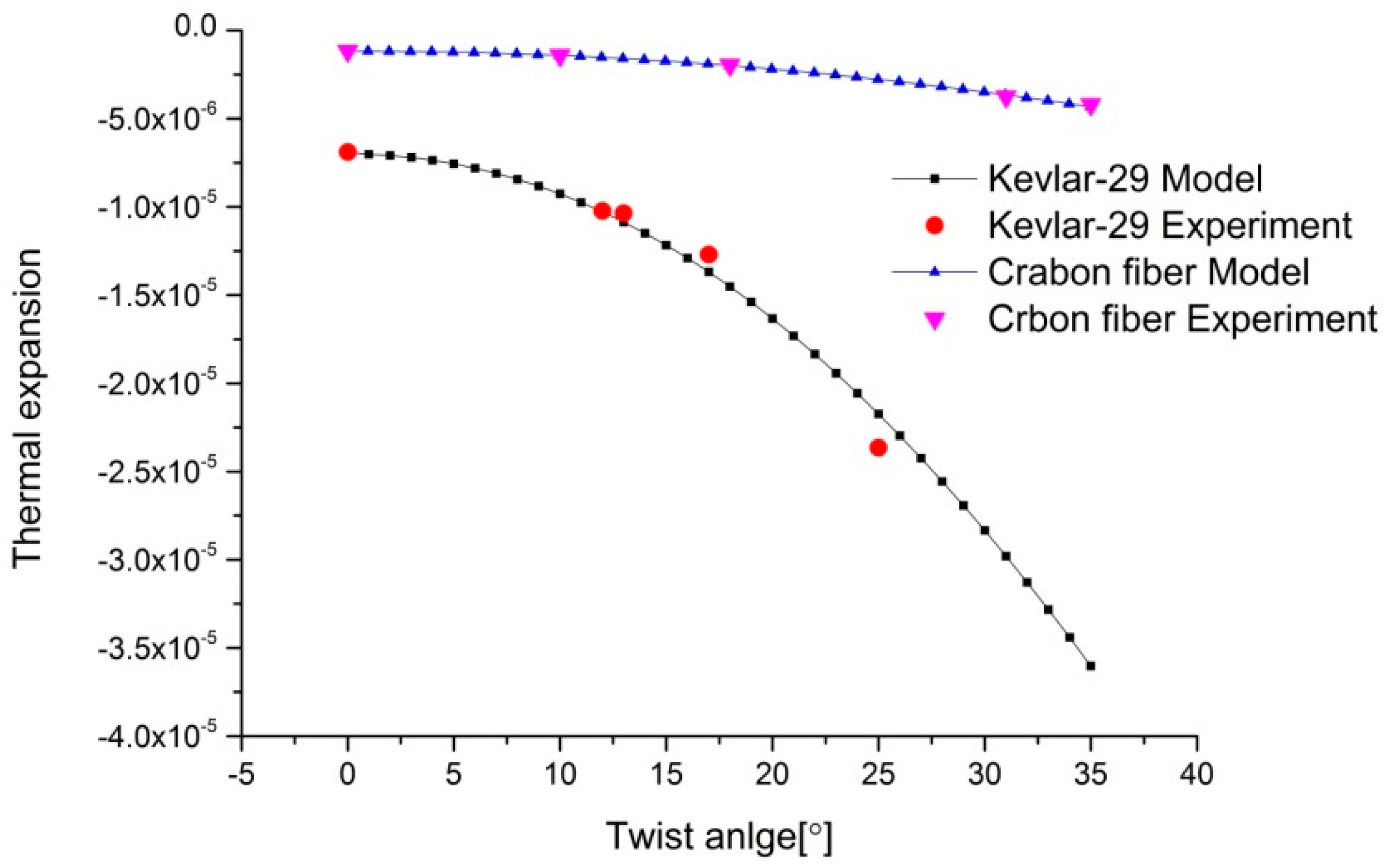
3. Results and Discussion
4. Conclusion
Acknowledgments
References
- Bell, C.J.; Reid, S.; Faller, J.; Hammond, G.D.; Hough, J.; Martin, I.W.; Rowan, S.; Tokmakov, K.V. Experimental results for nulling the effective thermal expansion coefficient of fused silica fibres under a static stress. Class. Quantum Grav. 2014, 31, 065010. [Google Scholar] [CrossRef]
- Lima, M.D.; Li, N.; De Andrade, M.J.; Fang, S.; Oh, J.; Spinks, G.M.; Kozlov, M.E.; Haines, C.S.; Suh, D.; Foroughi, J.; et al. Electrically, chemically, and photonically powered torsional and tensile actuation of hybrid carbon nanotube yarn muscles. Science 2012, 338, 928–932. [Google Scholar] [CrossRef] [PubMed]
- Bohr, J.O.K. The ancient art of laying rope. EPL Europhys. Lett. 2011, 93, 60004. [Google Scholar] [CrossRef]
- Costello, G.A. Theory of Wire Rope; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1997. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yue, D.; Wei, L. Twisted Fibers Can Have an Adjustable Thermal Expansion. Proceedings 2018, 2, 456. https://doi.org/10.3390/ICEM18-05341
Yue D, Wei L. Twisted Fibers Can Have an Adjustable Thermal Expansion. Proceedings. 2018; 2(8):456. https://doi.org/10.3390/ICEM18-05341
Chicago/Turabian StyleYue, Donghua, and Liming Wei. 2018. "Twisted Fibers Can Have an Adjustable Thermal Expansion" Proceedings 2, no. 8: 456. https://doi.org/10.3390/ICEM18-05341
APA StyleYue, D., & Wei, L. (2018). Twisted Fibers Can Have an Adjustable Thermal Expansion. Proceedings, 2(8), 456. https://doi.org/10.3390/ICEM18-05341



