Uncertainty Control Method for Non-Uniform Wear of the Driving Mechanism of Flapping Wing Aircraft
Abstract
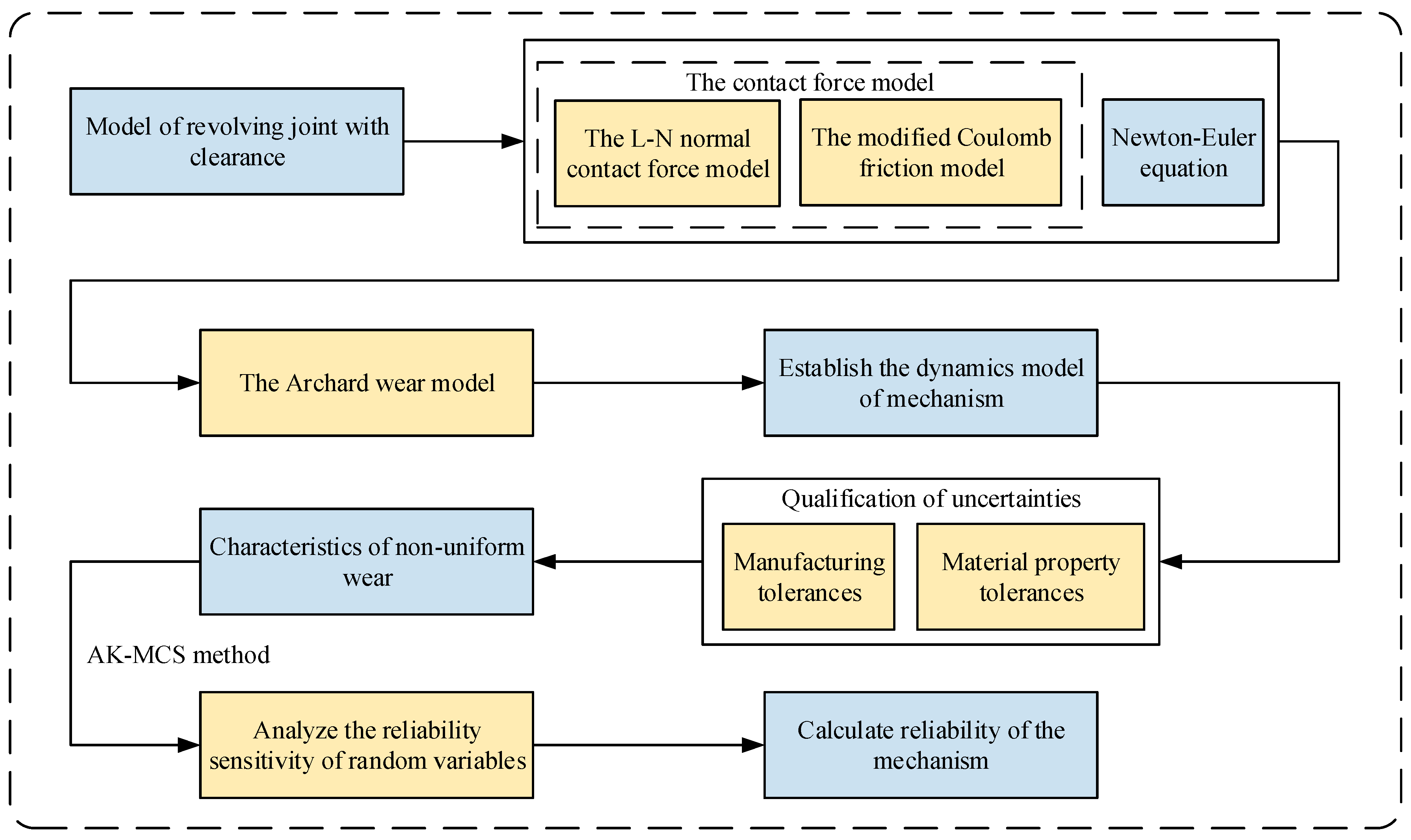
1. Introduction
2. Multibody Dynamics Analysis of Joint with Clearance
2.1. Kinematics Equations for Ideal Joint Multibody System
2.2. Vector Modeling of Clearance for Revolving Joint
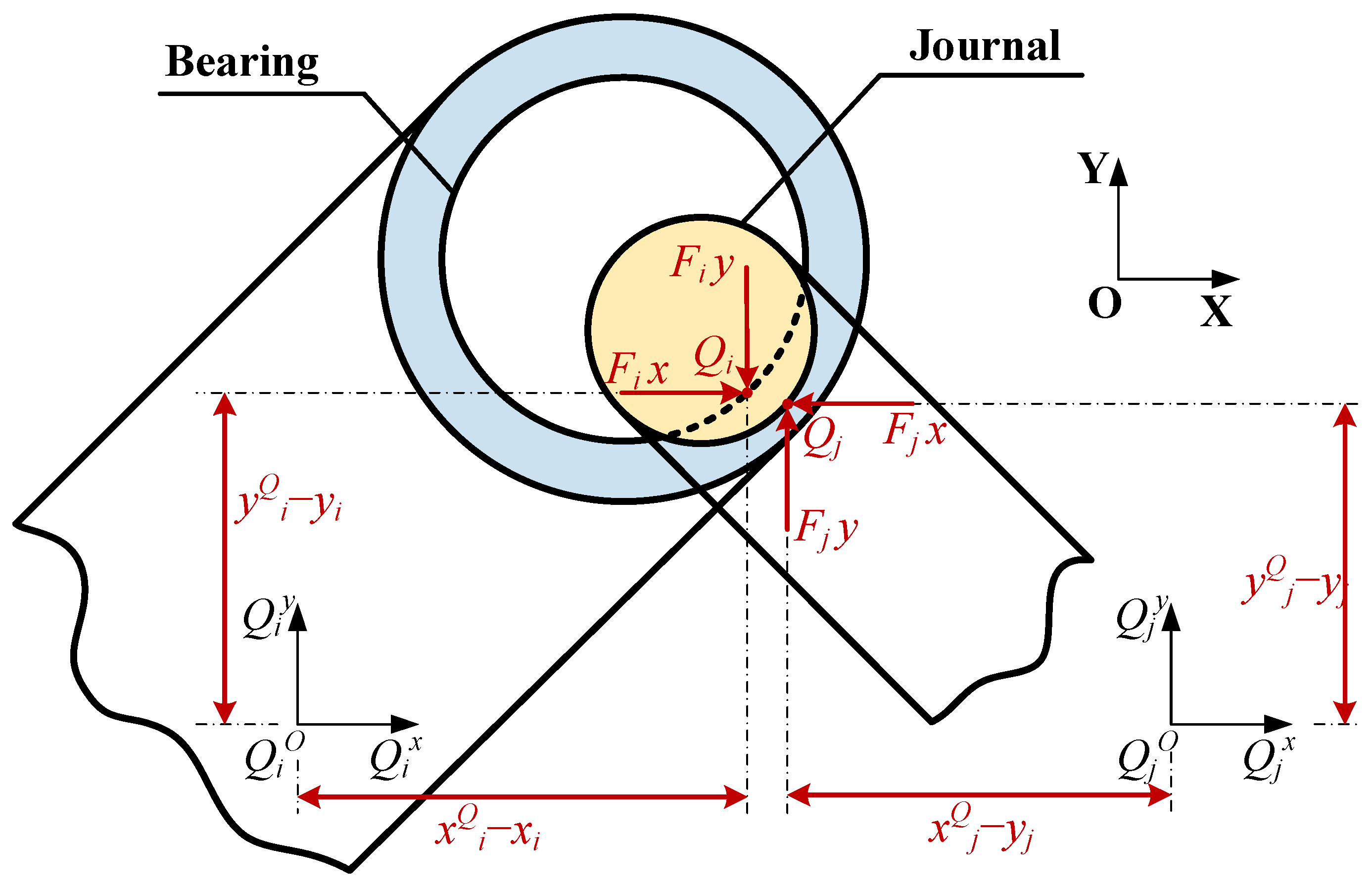
2.3. Mathematical Modeling of Revolving Joint with Clearance
2.4. Contact Force Modeling of Revolving Joint with Clearance
2.4.1. Normal Contact Force Model
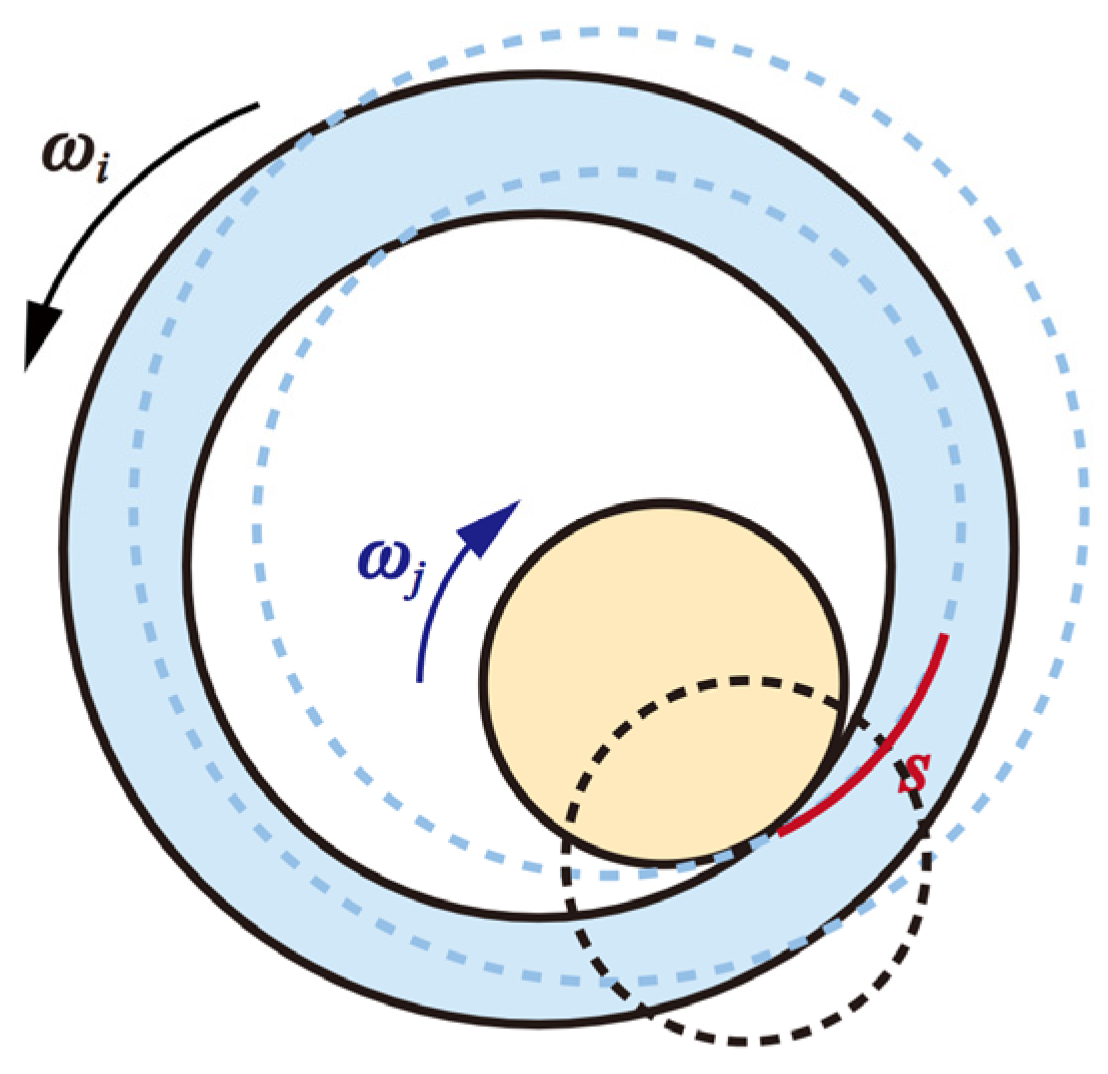
2.4.2. Tangential Contact Force Model
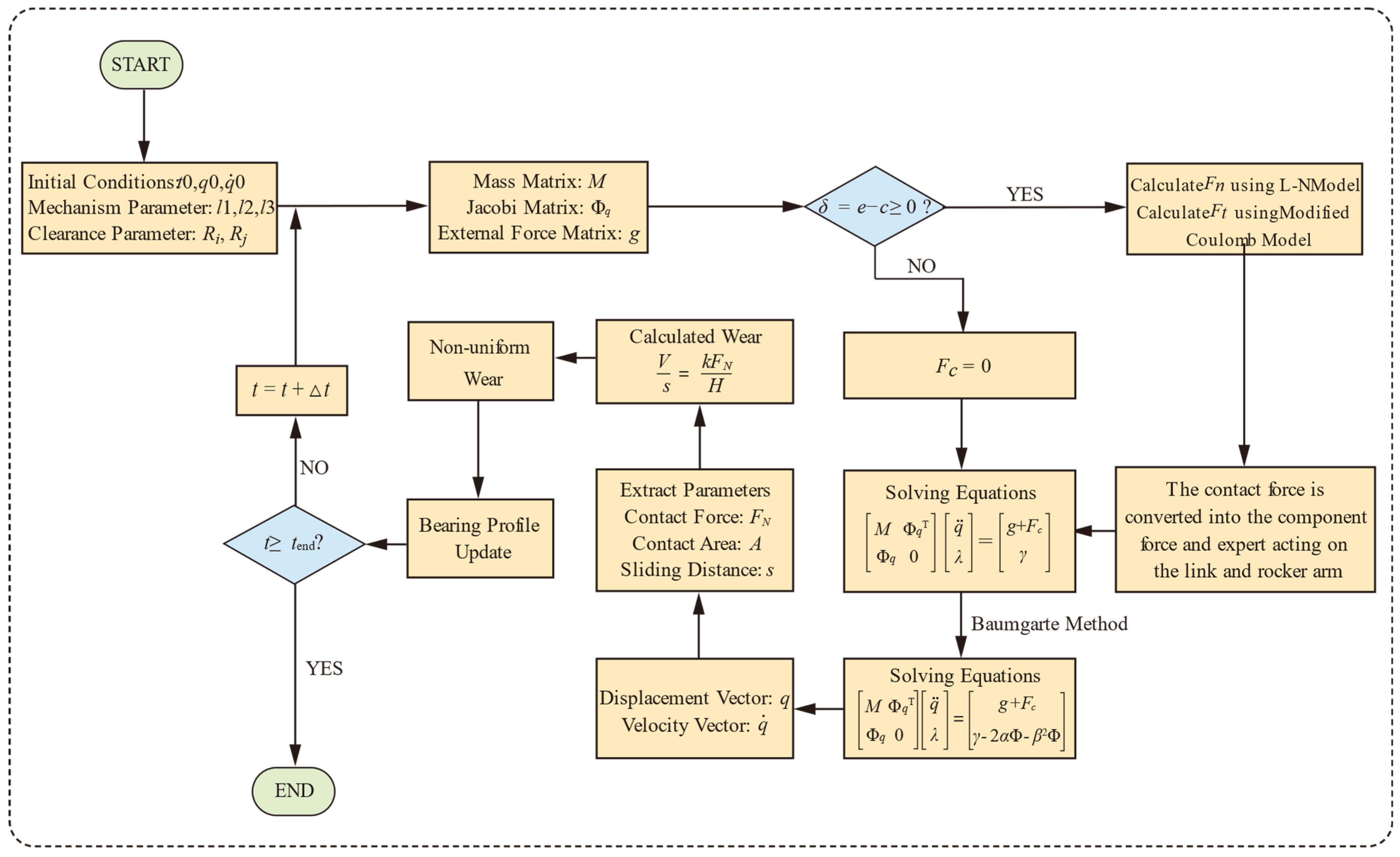
3. Wear Calculation for Revolving Joint with Clearance
- Step 1:
- Set the initial parameters of the multi-body mechanism, including the dimensions of the mechanism, the displacement vector, the velocity vector, and the initial clearance, etc. and establish the dynamics equations of the ideal joint multi-body mechanism;
- Step 2:
- Establish a mathematical model of the clearance joint, and judge whether or not contact occurs between the bearing and journal. If contact occurs, calculate the normal contact force and tangential contact force, and the contact force can be converted into the component force and moment applied to the connecting rods and form a matrix of contact force. Otherwise, the matrix of contact force is a zero matrix;
- Step 3:
- Combine the dynamics equations of the ideal joint mechanism and the contact force matrix, and then apply the Baumgarte stabilization method to obtain the dynamic equations of the multi-body mechanism with the clearance joint and solve the dynamics response;
- Step 4:
- According to the results of the dynamics solution, combine with the Archard model to calculate the wear depth of the joint, and finally obtain the wear profile of the bearing and journal;
- Step 5:
- Update the wear profile of the joint in the multi-body model, perform the dynamics solution and wear calculation for the next cycle, and repeat Step 2-Step 5 until the desired number of cycles is achieved.
4. Analysis and Quantification of Uncertainty
4.1. Manufacturing Tolerances
4.2. Material Properties Variations
5. Wear Reliability Model
5.1. Reliability Function with Uncertainty
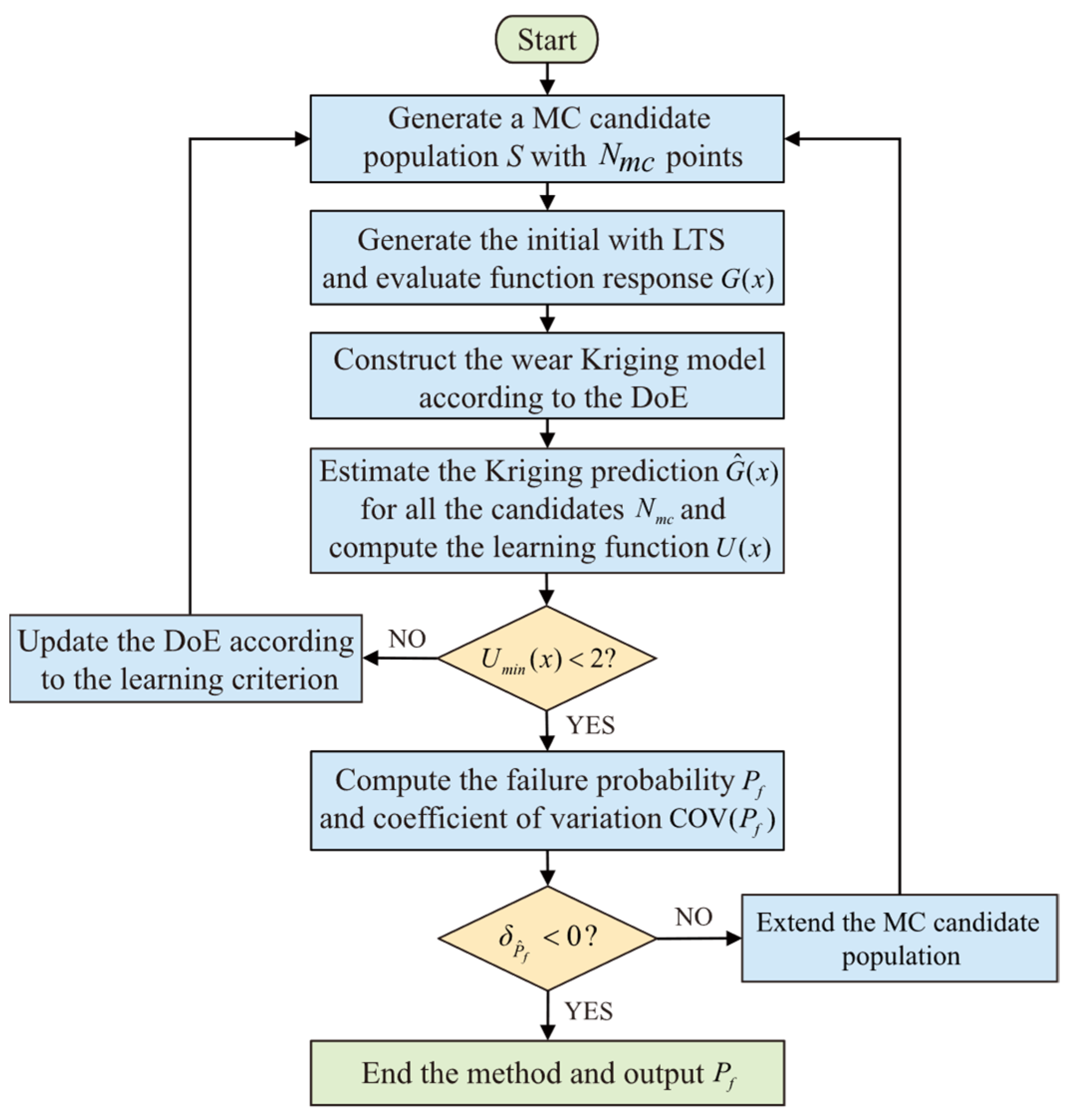
5.2. AK-MCS Method
- Step 1:
- Generate a candidate population of sample points based on the probability distribution of the random variables;
- Step 2:
- Generate initial sample design points by random sampling in the sample space according to the Latin Hypercubic Sampling Method, and calculate the corresponding function values , which are used as the experimental initial design of the Kriging model;
- Step 3:
- Use the DACE toolbox of MATLAB (R2024b) to build the experimental design Kriging model, with the Gaussian model selected for the correlation model and a constant for the regression model;
- Step 4:
- Estimate the Kriging response and variance of all candidate samples . Calculate the learning function for all candidate sample points ;
- Step 5:
- If , find the optimized training point, that is, the sample point with the smallest value of the function, and update the Kriging model and rerun Step 3. If , continue to Step 6;
- Step 6:
- Calculate the failure rate and coefficient of variation according to Equations (47) and (48). If the coefficient of variation , the candidate samples should be increased and Step 1 should be restarted;
- Step 7:
- End the AK-MCS process and output the failure probability of the mechanism .
6. Results and Discussion
6.1. Wear Characteristics of Flapping Driving Mechanism
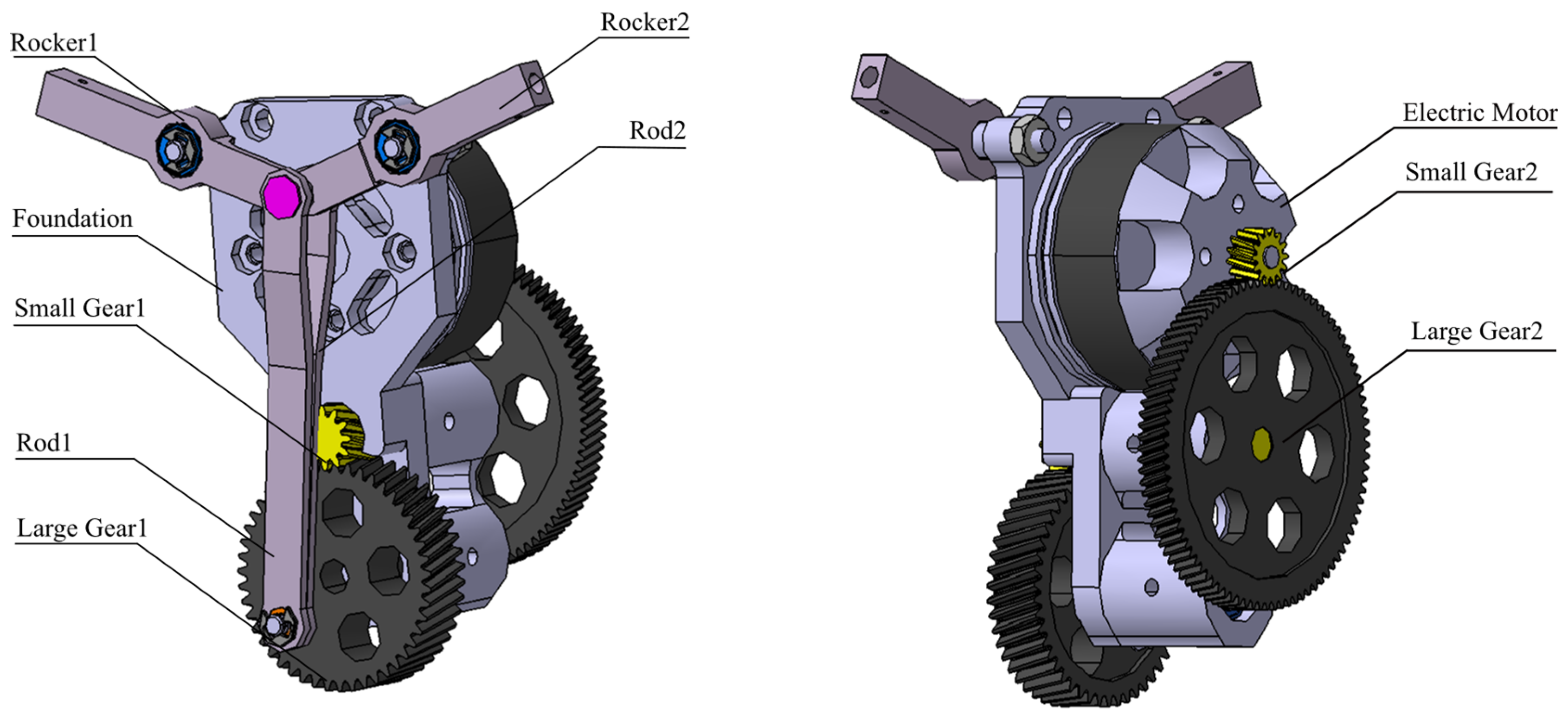
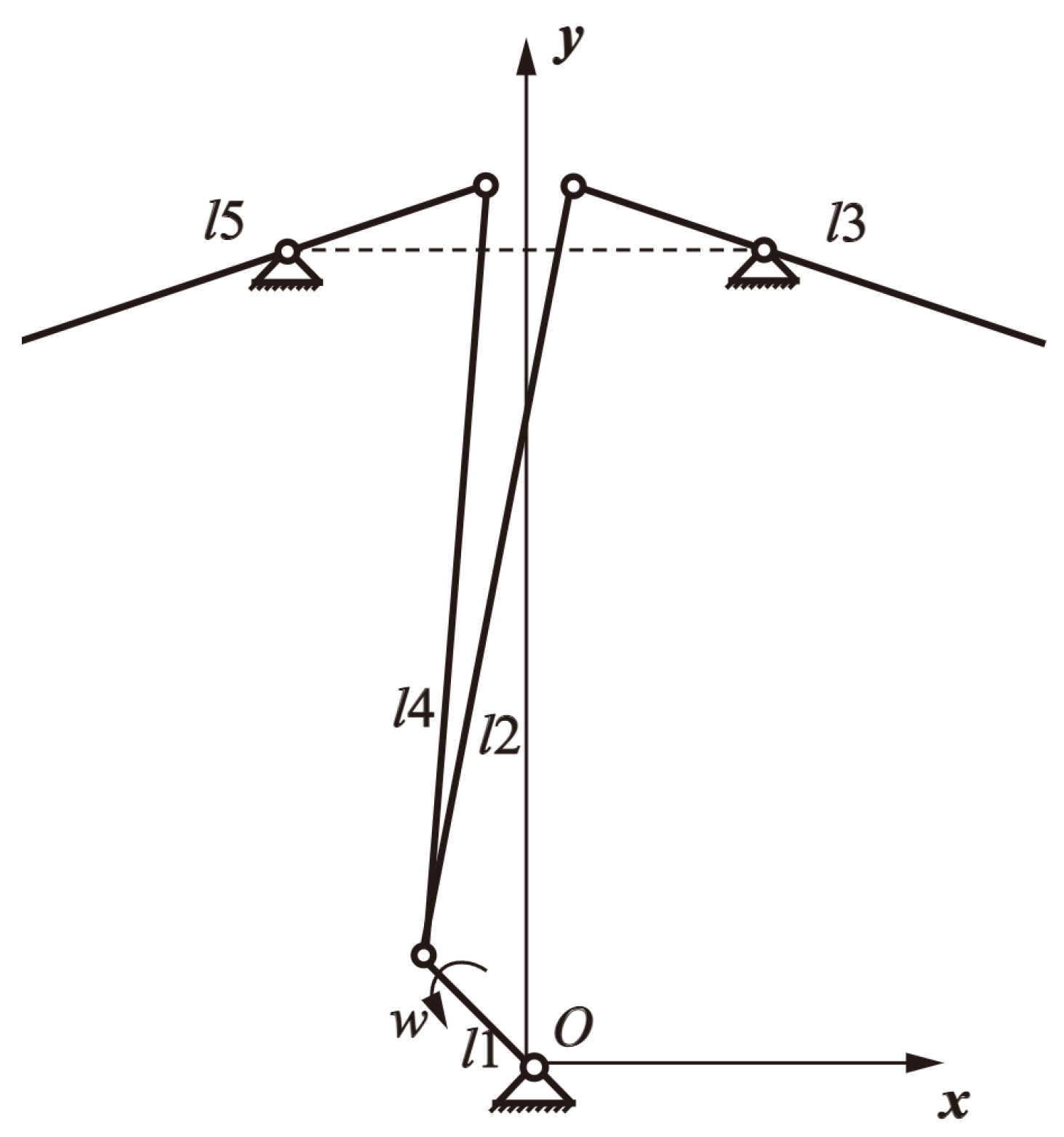
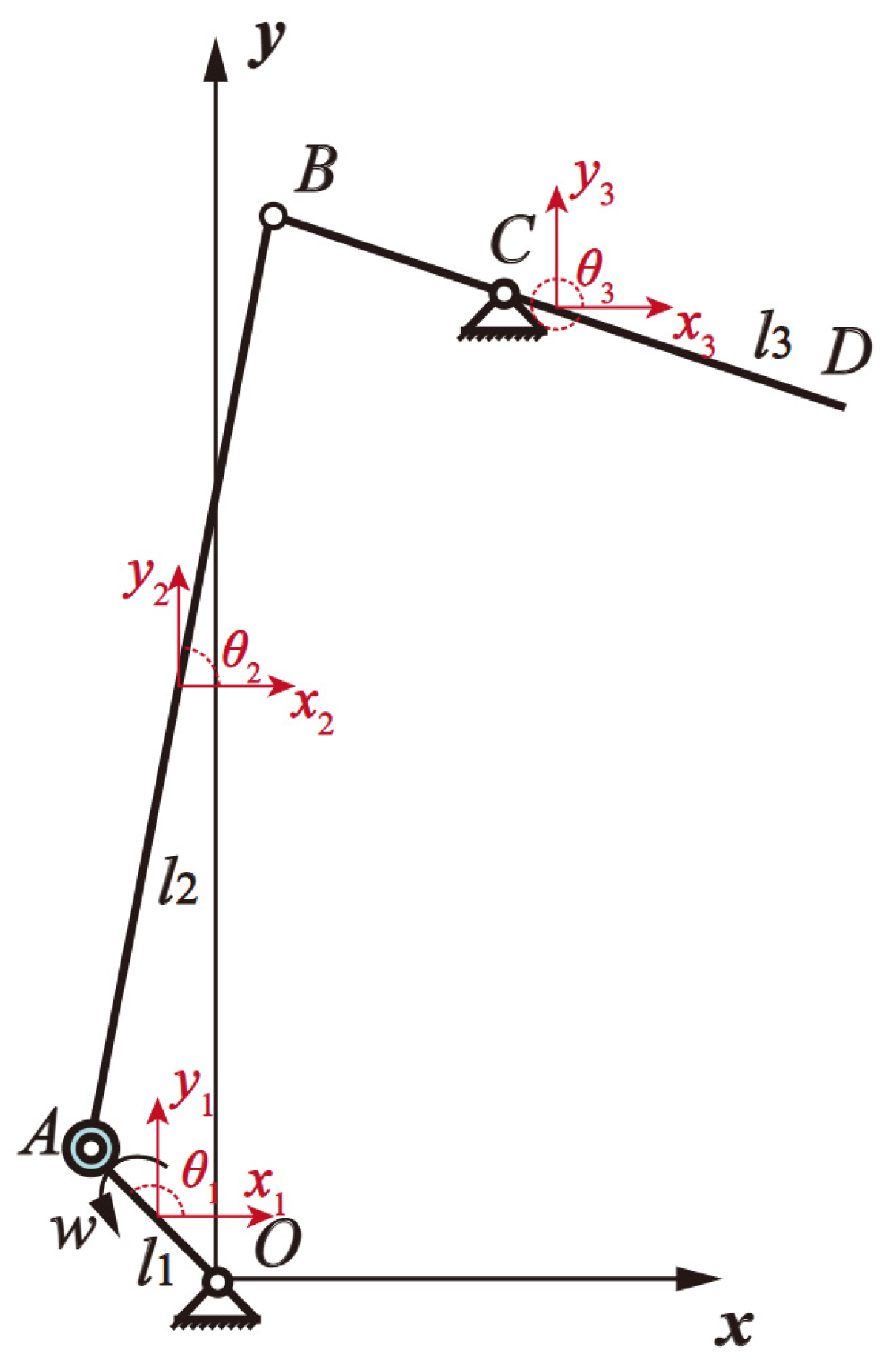
6.1.1. Dynamics Modeling
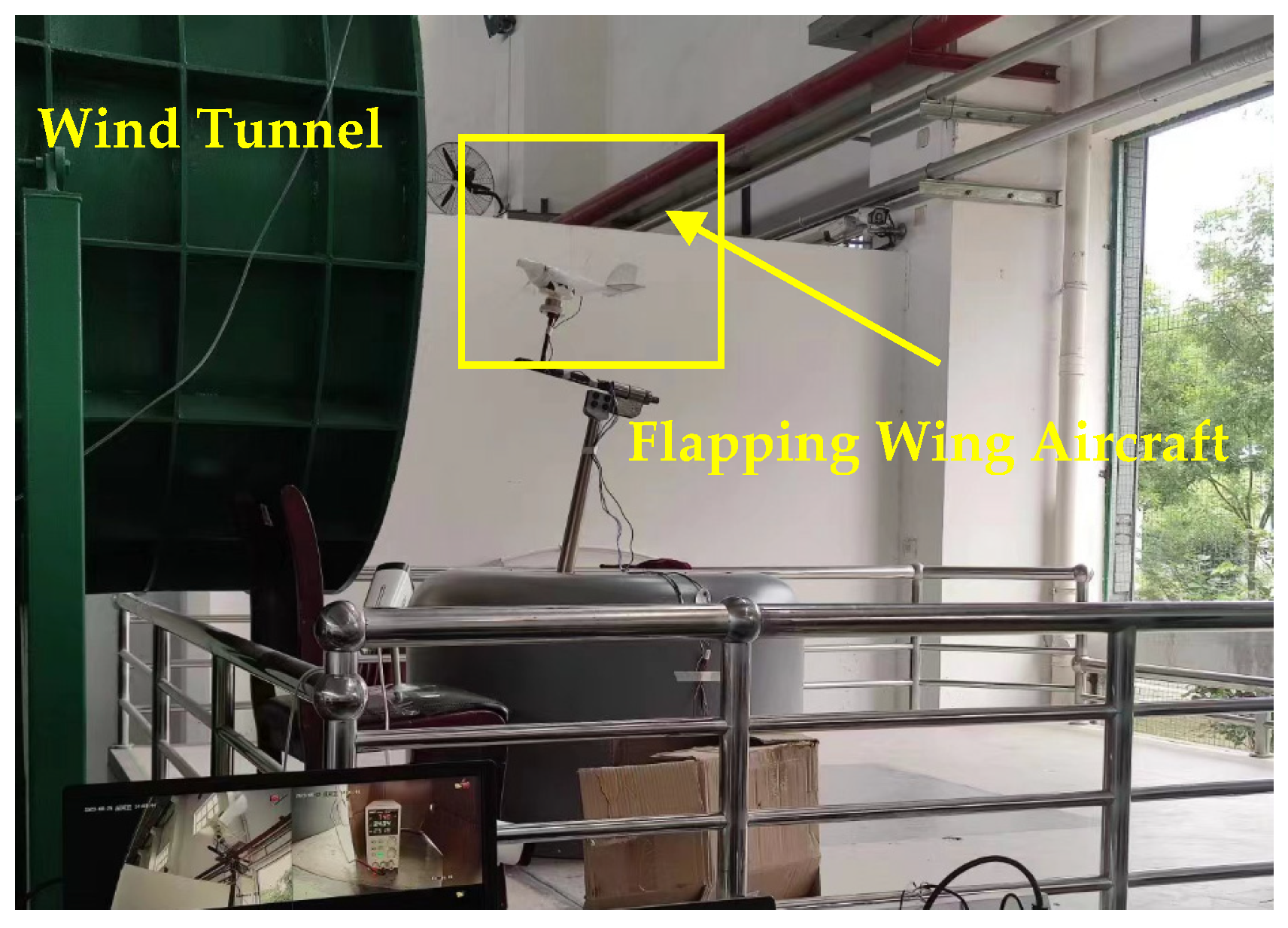
6.1.2. Practical Experiment
- Fix the flapping wing driving mechanism together with its specialized hull on the specialized wind tunnel experimental platform, adjusting the angle of attack to 10° and setting the incoming speed of the wind tunnel to 10 m/s;
- Wait for the wind speed in the wind tunnel to stabilize, and then activate the flapping wing aircraft. Adopt a DC voltage to supply power to the flapping wing aircraft, and operate it stably at the speed of 480 r/min in the experiment;
- Use high-definition cameras to monitor the operation of the mechanism in real-time;
- Use a magnifying glass and microscope at intervals to observe the wear of the mechanism joint until the mechanism fails.
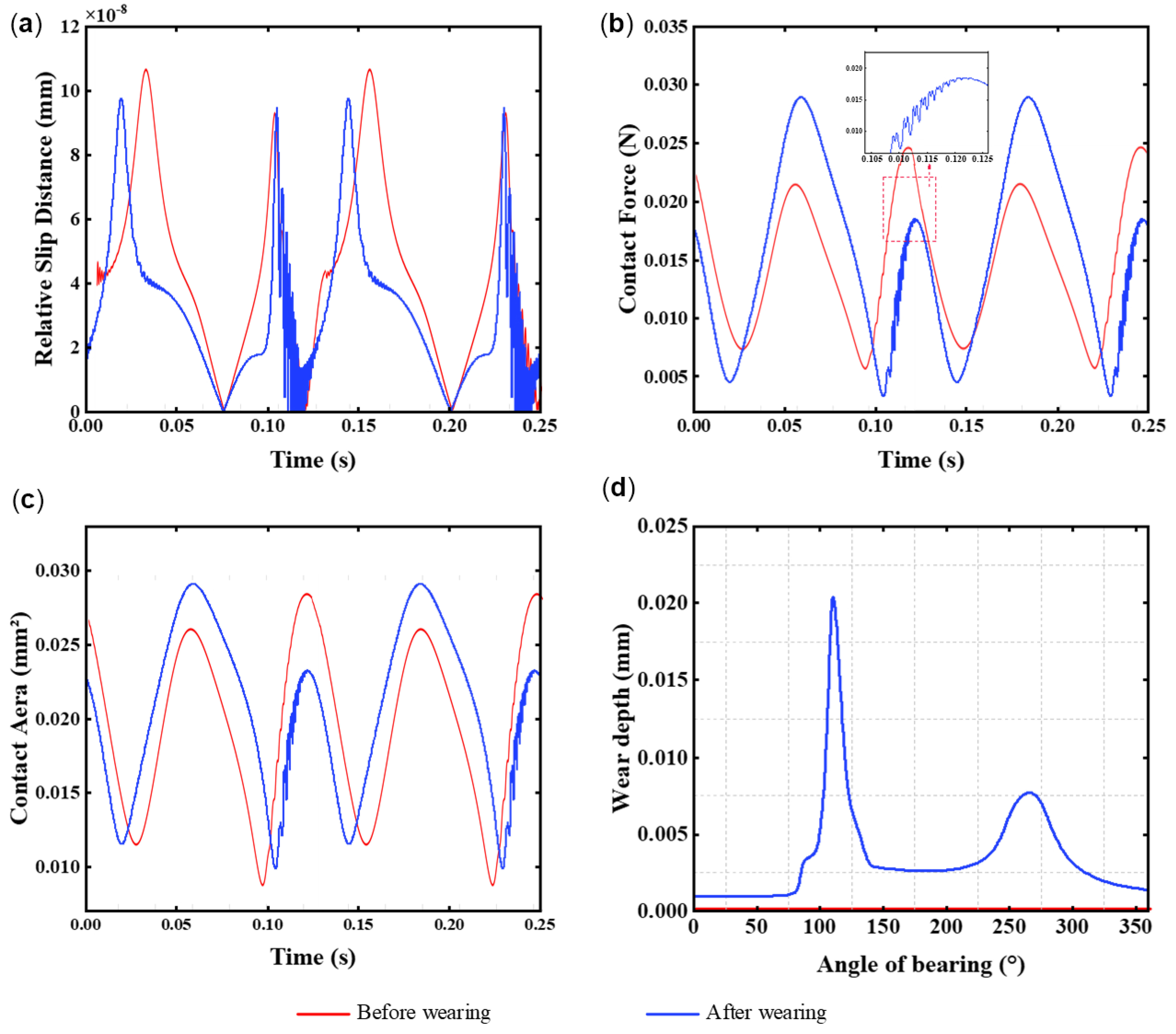
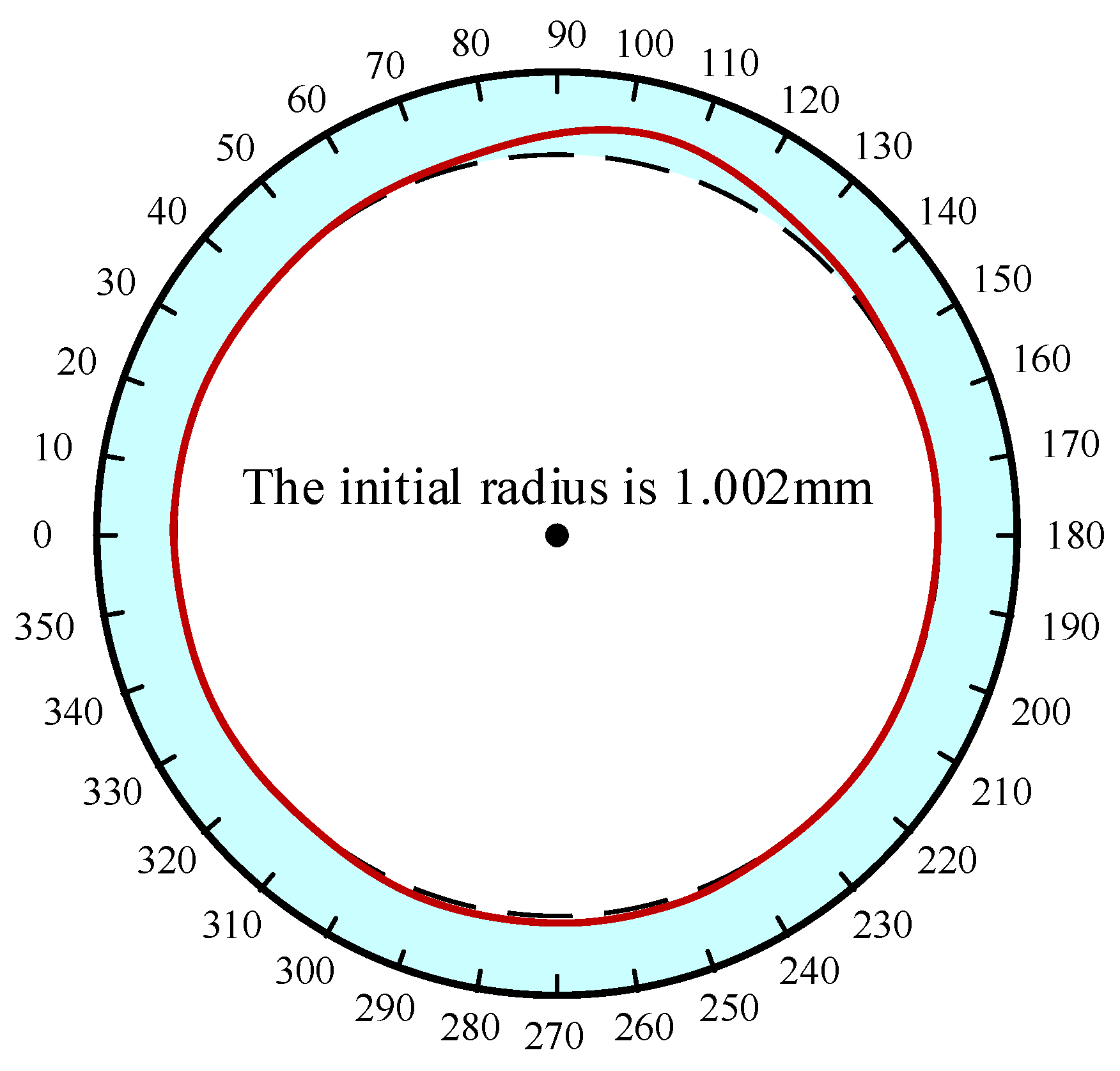
6.1.3. Dynamic Wear Characteristics
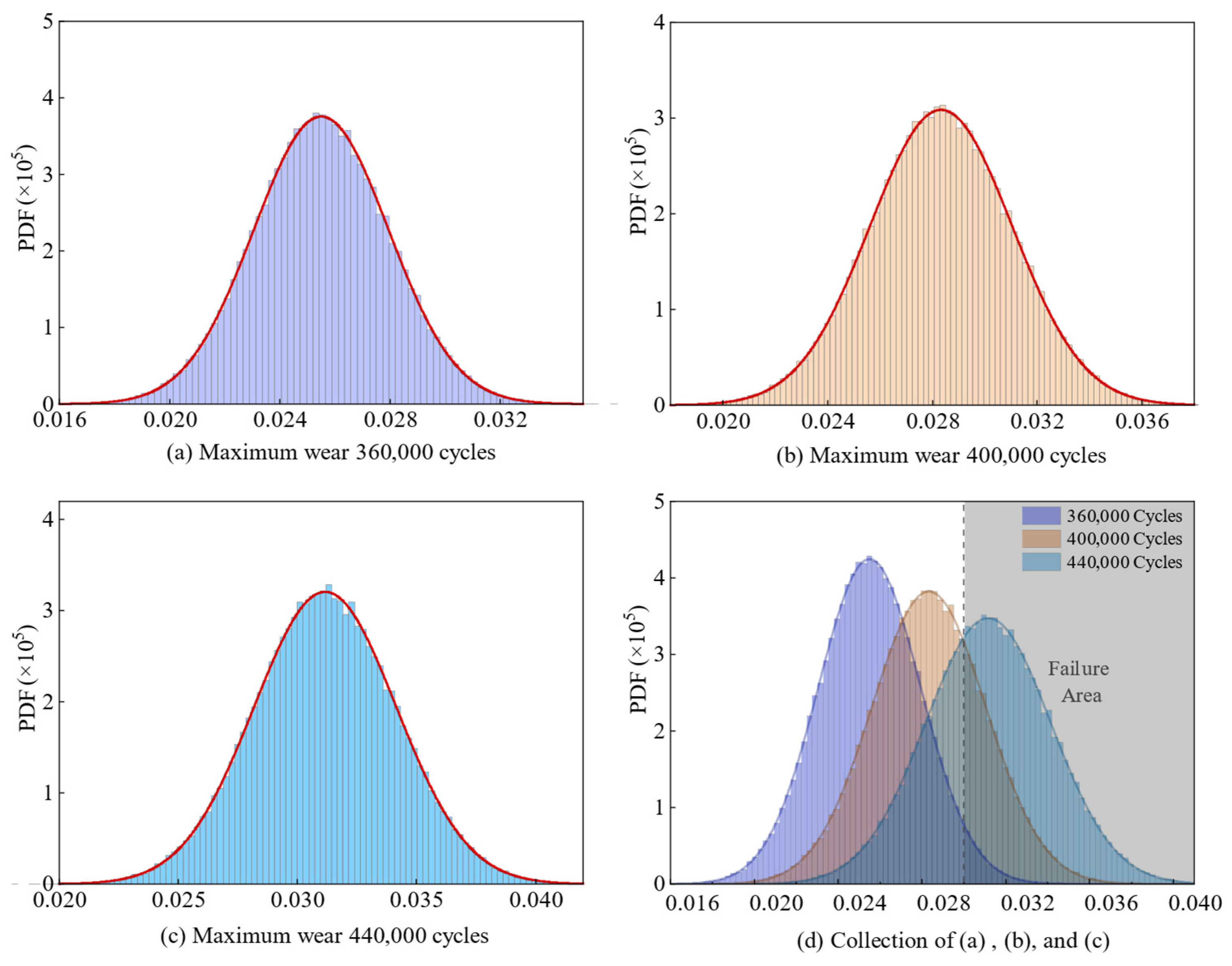
6.2. Define the Allowable Wear of the Joint
6.2.1. Define the Random Variables
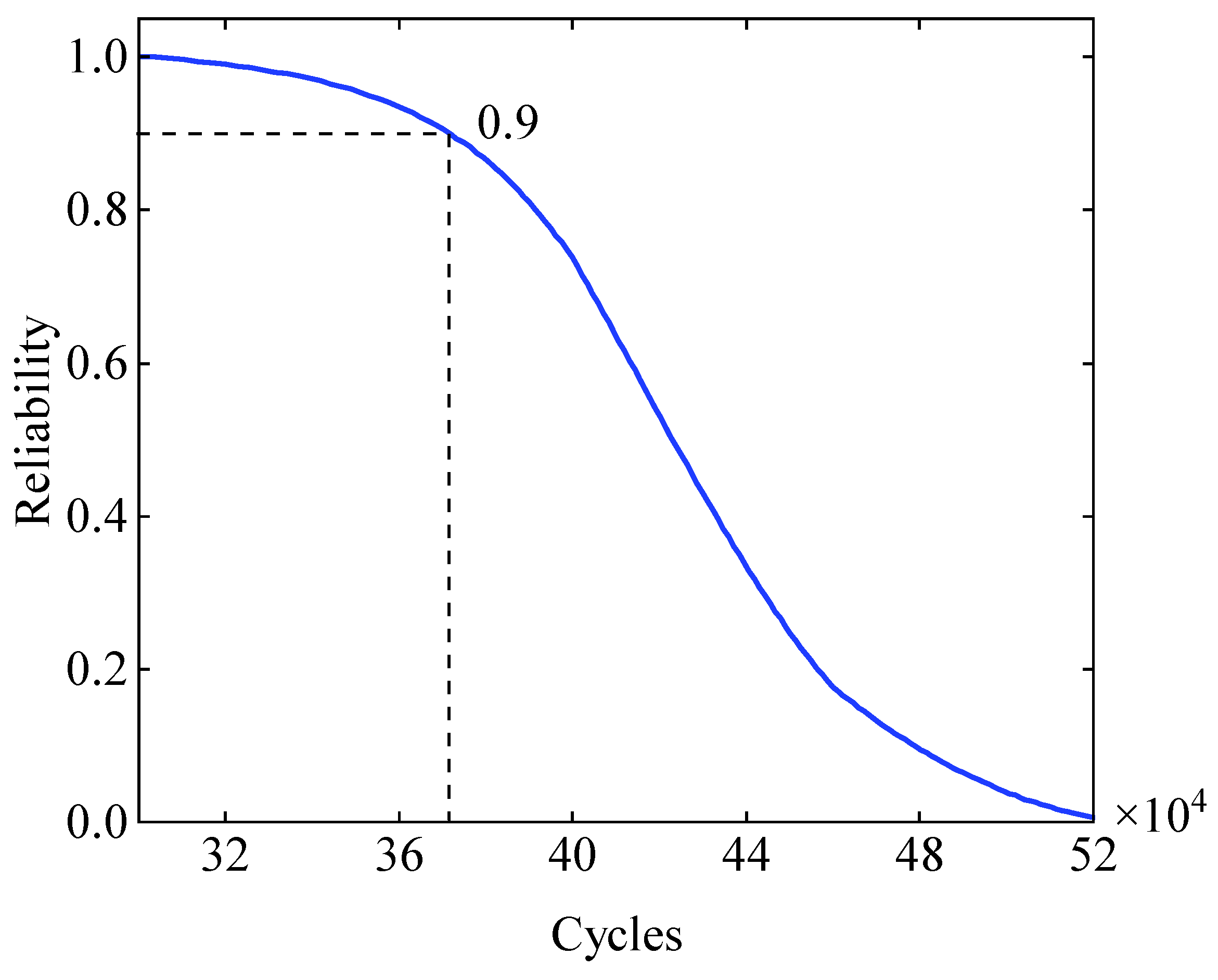
6.2.2. Reliability Analysis
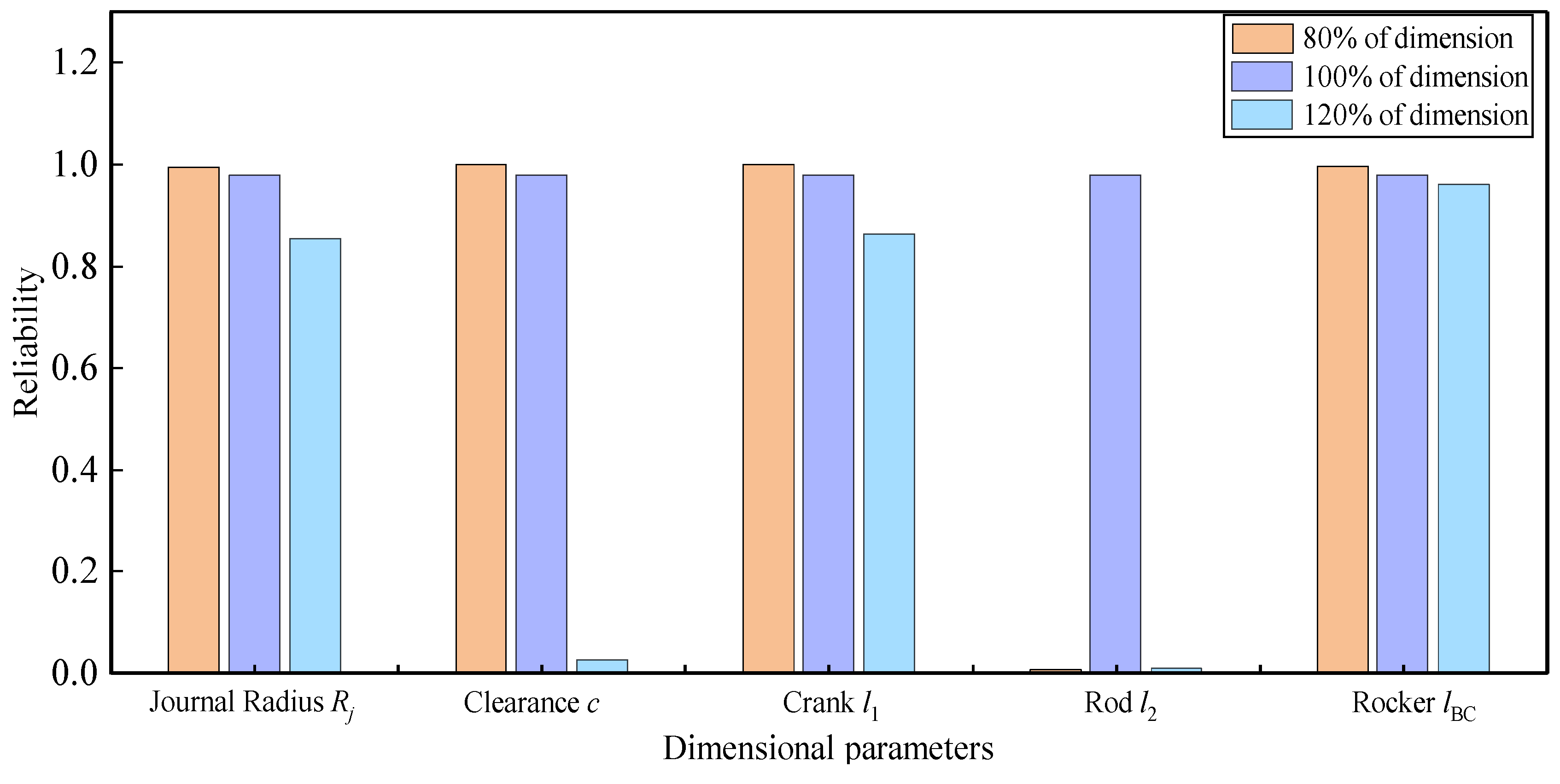
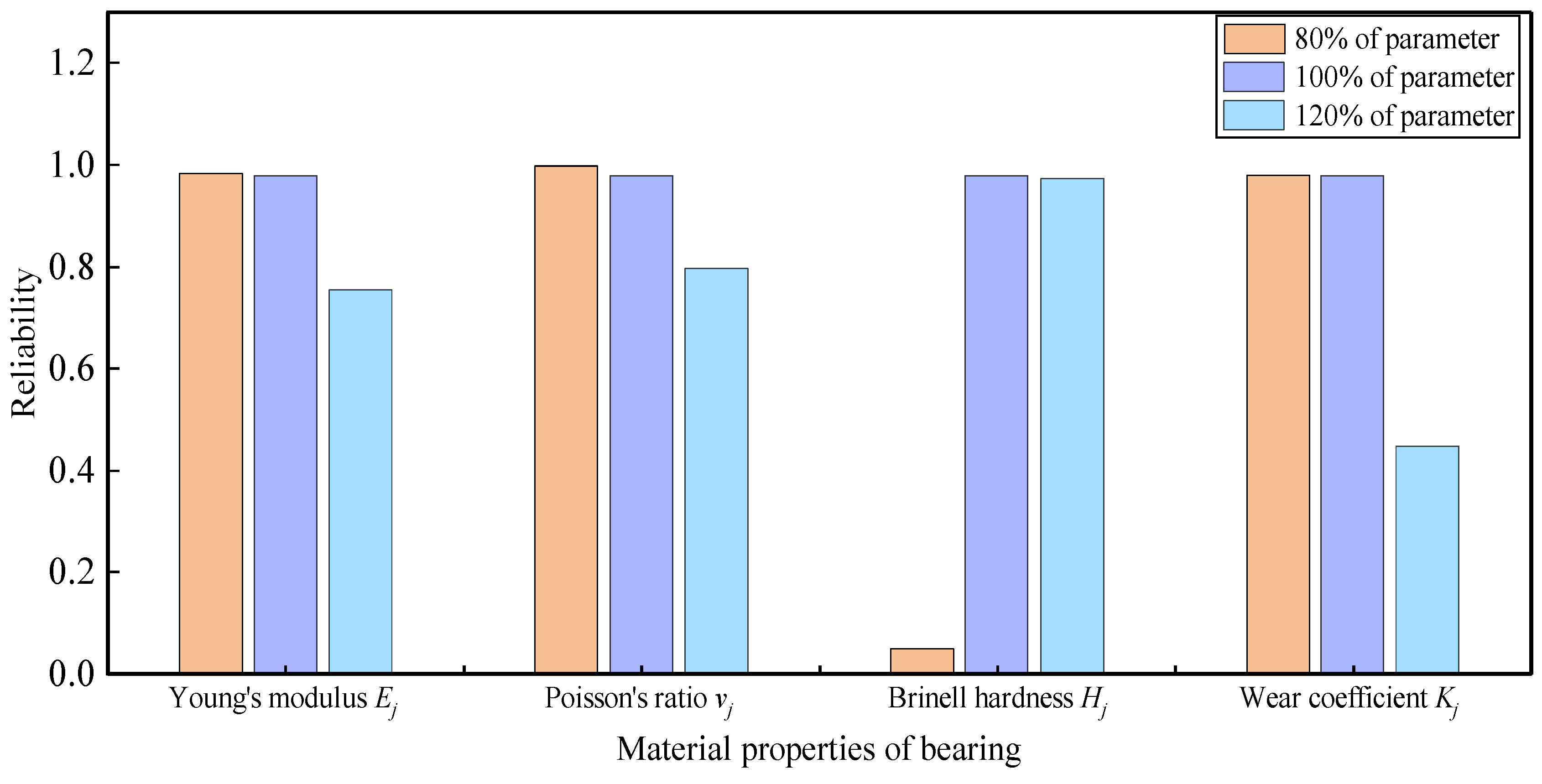
6.2.3. Reliability Sensitivity Analysis
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, H.; Song, B.; Sun, Z.; Wang, L. Driving mechanism of flapping wing aircraft: Review and prospect. Acta Aeronaut. Astronaut. Sin. 2021, 42, 74–95. [Google Scholar]
- Flores, P.; Ambrósio, J. Revolute joints with clearance in multibody systems. Comput. Struct. 2004, 82, 1359–1369. [Google Scholar] [CrossRef]
- López-Lombardero, M.; Cuadrado, J.; Cabello, M.; Martinez, F.; Dopico, D.; Varela, A.L. Simulating fatigue damage on planar mechanisms considering wear in revolute joints with clearance. Mech. Mach. Theory 2024, 203, 105805. [Google Scholar] [CrossRef]
- López-Lombardero, M.; Cuadrado, J.; Cabello, M.; Martinez, F.; Dopico, D.; López-Varela, A. A multibody-dynamics based method for the estimation of wear evolution in the revolute joints of mechanisms that considers link flexibility. Mech. Mach. Theory 2024, 194, 105583. [Google Scholar] [CrossRef]
- Li, H.; Xie, J.; Wei, W. Numerical and Dynamic Errors Analysis of Planar Multibody Mechanical Systems With Adjustable Clearance Joints Based on Lagrange Equations and Experiment. J. Comput. Nonlinear Dyn. 2020, 15, 081001. [Google Scholar] [CrossRef]
- Lin, Y.P.; Wang, J.C.; Zhang, J.L.; An, M.X.; Jiang, S. Research on the Dynamic and Reliability of Planar Multi-Link Mechanisms with Multiple Clearances. Mech. Solids 2024, 59, 1537–1558. [Google Scholar] [CrossRef]
- Wei, Z.; Wang, Z.; Li, D.; Liang, D. Rigid-flexible coupling dynamic modelling and dynamic accuracy analysis of planar cam four-bar mechanism with multiple clearance joints. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2024, 238, 421–444. [Google Scholar] [CrossRef]
- Zhu, X.; Zhao, F.; Yang, X.; Xu, Y.; Jia, P.; Zhou, B.; Xue, S. Study on Collision Dynamics Model and Multi-Body Contact Forces of Ball Cage Flexible Joint Considering Clearance. Machines 2023, 11, 466. [Google Scholar] [CrossRef]
- Roupa, I.; Gonçalves, S.B.; da Silva, M.T. Kinematics and dynamics of planar multibody systems with fully Cartesian coordinates and a generic rigid body. Mech. Mach. Theory 2023, 180, 105134. [Google Scholar] [CrossRef]
- Zhuang, X. Time-dependent kinematic reliability of a dual-axis driving mechanism for satellite antenna considering non-uniform planar revolute joint clearance. Acta Astronaut. 2022, 197, 91–106. [Google Scholar] [CrossRef]
- Wang, H.; Wang, J. Dynamics and control of spacecraft solar array deployment considering physical contacts between locking mechanisms. Acta Astronaut. 2022, 195, 481–492. [Google Scholar] [CrossRef]
- Zhou, Z.; Zheng, X.; Chen, Z.; Wang, X.; Liang, B.; Wang, Q. Dynamics modeling and analysis of cable-driven segmented manipulator considering friction effects. Mech. Mach. Theory 2022, 169, 104633. [Google Scholar] [CrossRef]
- Wang, J.; Su, Y.; Subramaniam, N.A.; Pang, J.H.L. Archard model guided feature engineering improved support vector regression for rail wear analysis. Eng. Fail. Anal. 2022, 137, 106248. [Google Scholar] [CrossRef]
- Jia, J.; Cui, W.; Zhang, Y.; Shen, L.; Wu, H. Analysis of Uneven Wear in Driving Mechanism of Flapping-Wing UAV. In Proceedings of the 2020 IEEE International Conference on Mechatronics and Automation (ICMA), Beijing, China, 13–16 October 2020. [Google Scholar] [CrossRef]
- Sun, Y.; Li, Y.; Zhang, Q.; Qin, X.; Chen, K. Wear analysis and simulation of small module gear based on Archard model. Eng. Fail. Anal. 2023, 144, 106990. [Google Scholar] [CrossRef]
- Zhuang, X.; Yu, T.; Liu, J.; Song, B. Kinematic reliability evaluation of high-precision planar mechanisms experiencing non-uniform wear in revolute joints. Mech. Syst. Signal Process. 2022, 169, 108748. [Google Scholar] [CrossRef]
- Li, Y.; Yang, Y.; Li, M.; Liu, Y.; Huang, Y. Dynamics analysis and wear prediction of rigid-flexible coupling deployable solar array system with clearance joints considering solid lubrication. Mech. Syst. Signal Process. 2022, 162, 108059. [Google Scholar] [CrossRef]
- Jiang, S.; Wang, T.; Xiao, L. Experiment research and dynamic behavior analysis of multi-link mechanism with wearing clearance joint. Nonlinear Dyn. 2022, 109, 1325–1340. [Google Scholar] [CrossRef]
- Li, X.-Y.; Chen, W.-B.; Kang, R. Performance margin-based reliability analysis for aircraft lock mechanism considering multi-source uncertainties and wear. Reliab. Eng. Syst. Saf. 2021, 205, 107234. [Google Scholar] [CrossRef]
- Jiang, D.; Han, Y.; Wang, K.; Jiang, S.; Cui, W.; Song, B. Functional degradation reliability analysis for non-uniform wear of multi-rotating joints of mechanical structures. Eng. Fail. Anal. 2024, 157, 107934. [Google Scholar] [CrossRef]
- Afshari, S.S.; Enayatollahi, F.; Xu, X.; Liang, X. Machine learning-based methods in structural reliability analysis: A review. Reliab. Eng. Syst. Saf. 2022, 219, 108223. [Google Scholar] [CrossRef]
- Wang, W.; Shen, G.; Zhang, Y.; Zhu, Z.; Li, C.; Lu, H. Dynamic reliability analysis of mechanical system with wear and vibration failure modes. Mech. Mach. Theory 2021, 163, 104385. [Google Scholar] [CrossRef]
- Wang, T.; Chen, Z.; Li, G.; He, J.; Shi, R.; Liu, C. Adaptive Kriging-based probabilistic subset simulation method for structural reliability problems with small failure probabilities. Structures 2024, 70, 107726. [Google Scholar] [CrossRef]
- Prentzas, I.; Fragiadakis, M. Quantified active learning Kriging model for structural reliability analysis. Probabilistic Eng. Mech. 2024, 78, 103699. [Google Scholar] [CrossRef]
- Shi, Y.; Lu, Z.; He, R.; Zhou, Y.; Chen, S. A novel learning function based on Kriging for reliability analysis. Reliab. Eng. Syst. Saf. 2020, 198, 106857. [Google Scholar] [CrossRef]
- Ouyang, L.; Huang, L.; Han, M. An active learning reliability analysis algorithm: Based on the perspective of Kriging prediction variance. Xitong Gongcheng Lilum Yu Shijian 2023, 43, 2154–2165. [Google Scholar] [CrossRef]
- Zhang, W.; Guan, Y.; Wang, Z.; Xu, H. A novel active learning Kriging based on improved Metropolis-Hastings and importance sampling for small failure probabilities. Comput. Methods Appl. Mech. Eng. 2025, 435, 117658. [Google Scholar] [CrossRef]
- Yuan, Y.; Au, F.T.; Yang, D.; Zhang, J. An active learning Kriging-based Bayesian framework for probabilistic structural model exploration. J. Sound Vib. 2025, 596, 118730. [Google Scholar] [CrossRef]
- Gong, Y.; Zhang, J.; Xu, D.; Huang, Y. Quantile-based optimization under uncertainties for complex engineering structures using an active learning basis-adaptive PC-Kriging model. Chin. J. Aeronaut. 2025, 38, 103197. [Google Scholar] [CrossRef]
- Zhang, S.; Gao, Y.; Yang, J. Dynamic modeling and analysis of vehicle scissor door mechanism with mixed clearance based on a hybrid contact force model. Multibody Syst. Dyn. 2024, 61, 509–538. [Google Scholar] [CrossRef]
- Ding, S.; Jian, B.; Zhang, Y.; Xia, R.; Hu, G. A normal contact force model for viscoelastic bodies and its finite element modeling verification. Mech. Mach. Theory 2023, 181, 105202. [Google Scholar] [CrossRef]
- Dong, Z.; Liang, X.; Hou, Y.; Zhang, Z. 6σ uncertainty optimisation strategy for USV navigation performance based on reliability analysis. Ocean Eng. 2022, 249, 110915. [Google Scholar] [CrossRef]
- Dong, X.; Sun, Y.; Wu, X.; Wang, R. Dynamic modeling and performance analysis of toggle-linkage presses considering mixed clearances and flexibility. Int. J. Non-linear Mech. 2022, 147, 104243. [Google Scholar] [CrossRef]
- Chen, X.; Jiang, S.; Wang, T. Dynamic modeling and analysis of multi-link mechanism considering lubrication clearance and flexible components. Nonlinear Dyn. 2022, 107, 3365–3383. [Google Scholar] [CrossRef]
- Chen, X.; Wang, T. Dynamic behavior analysis of rigid-flexible coupling planar mechanism considering dry clearances and lubrication clearances. J. Mech. Sci. Technol. 2023, 37, 1585–1600. [Google Scholar] [CrossRef]
- Zhuang, X.; Yu, T.; Sun, Z.; Song, K. Wear prediction of a mechanism with multiple joints based on ANFIS. Eng. Fail. Anal. 2021, 119, 104958. [Google Scholar] [CrossRef]
- Zhang, L.; Fang, Y.; Bai, G.; Tao, J. Dynamic Investigations on the Wear Behavior of a 3D Revolute Joint Considering Time-Varying Contact Stiffness: Simulation and Experiment. Chin. J. Mech. Eng. 2023, 36, 137. [Google Scholar] [CrossRef]
- Wang, Z.; Jin, G.; Wei, Z.; Liang, D.; Chang, B.; Zhou, Y. Research on irregular wear mechanism of planar multi-body mechanical system with multi-clearance joints. Adv. Mech. Eng. 2022, 14, 16878132221125043. [Google Scholar] [CrossRef]
- Hou, Y.-L.; Deng, Y.-J.; Zeng, D.-X. Dynamic modelling and properties analysis of 3RSR parallel mechanism considering spherical joint clearance and wear. J. Central South Univ. 2021, 28, 712–727. [Google Scholar] [CrossRef]
- Li, B.; Wang, M.S.; Gantes, C.J.; Tan, U.-X. Modeling and simulation for wear prediction in planar mechanical systems with multiple clearance joints. Nonlinear Dyn. 2022, 108, 887–910. [Google Scholar] [CrossRef]
- Wang, K.; Jiang, D.; Jiang, S.; Gao, Z.; Zhuang, X.; Cui, W. A Method for Predicting Non-Uniform Wear of Flapping Driving Mechanism Joint Considering Uncertainty, 1 April 2024, PREPRINT (Version 1) Available at Research Square. Available online: https://doi.org/10.21203/rs.3.rs-4176114/v1 (accessed on 10 December 2024).
- Flores, P.; Ambrósio, J.; Claro, J.P.; Lankarani, H.M. Kinematics and Dynamics of Multibody Systems with Imperfect Joints: Models and Case Studies; Springer Science & Business Media: Berlin, Germany, 2008. [Google Scholar]
- Bhalerao, K.; Issac, K.K. Simulation of impact, based on an approach to detect interference. Adv. Eng. Softw. 2006, 37, 805–813. [Google Scholar] [CrossRef]
- Brezeanu, L.C. Contact Stresses between Two Cylindrical Bodies—Cylinder and Cylindrical Cavity with Parallel Axes—Part II: 2D and 3D FEA Comparative Study. Procedia Technol. 2015, 19, 177–184. [Google Scholar] [CrossRef][Green Version]
- Wang, J.; Zhang, J.; Du, X. Hybrid dimension reduction for mechanism reliability analysis with random joint clearances. Mech. Mach. Theory 2011, 46, 1396–1410. [Google Scholar] [CrossRef]
- ISO.286-2; Geometrical Product Specifications (GPS)-ISO Code System for Tolerance on Linear Sizes, Part 2: Tables of Standard Tolerance Classes and Limit Deviations for Holes and Shafts. ISO: Geneva, Switzerland, 2010.
- Ashby, M.F.J.K. Materials and Design: The Art and Science of Material Selection in Product Design; Butterworth-Heinemann: Oxford, UK, 2013. [Google Scholar]
- Bond, A.H.A. Decoding Eurocode 7; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Zhuang, B.Z.L.Y.D.; Zhang, Y.Q. Random Vibration of Structures; National Defense Industry Press: Arlington, VA, USA, 1995. [Google Scholar]




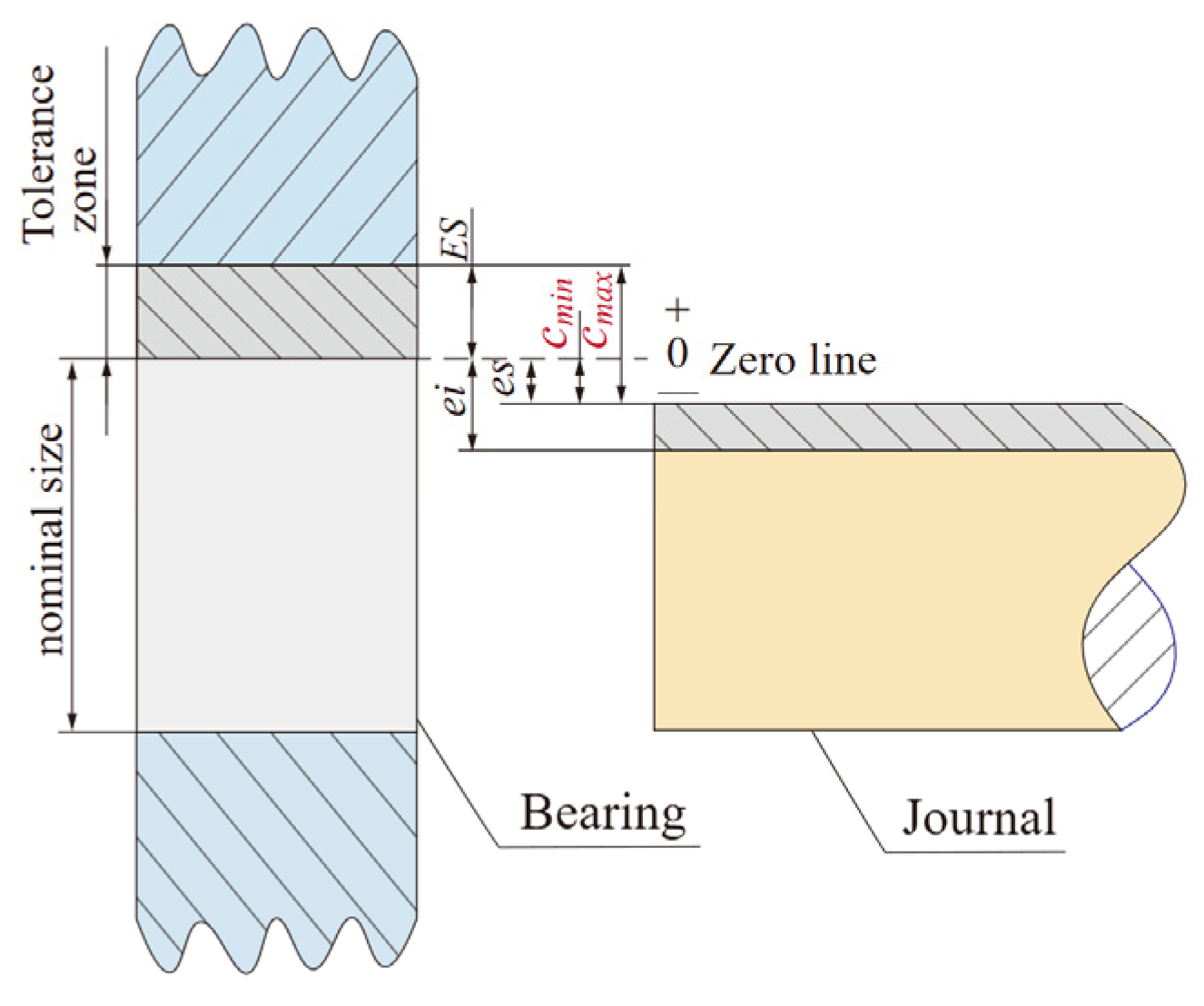




| Parameters | Distributions | Parameters | Distributions |
|---|---|---|---|
| Elements | Journal | Bearing |
|---|---|---|
| Radius (mm) | 1 | inner: 1.002/outer:1.5 |
| Material | 40 Cr | Titanium |
| Young’s modulus (N/m2) | 2.11 × 1011 | 1.2 × 1011 |
| Poisson’s ratio | 0.277 | 0.32 |
| Material hardness (HB) | 207 | 350 |
| Wear coefficient (mm3/(Nm)) | 2.1 × 10−3 | 1.2 × 10−3 |
| Elements | Length (m) | Mass (kg) | Moment of Inertia (kgm2) |
|---|---|---|---|
| Crank | 0.006 | 6.77 × 10−4 | 3.17 × 10−7 |
| Rod | 0.038 | 8.71 × 10−4 | 1.44 × 10−7 |
| Rocker | 0.027 | 9.38 × 10−4 | 5.72 × 10−8 |
| Parameters | Values |
|---|---|
| Rocker length lBC | 0.012 (m) |
| Coordinates of point C in Figure 14 | (0.0115, 0.038) (m) |
| Restitution coefficient Cr | 0.9 |
| Journal width L | 0.001 (m) |
| Crank speed ω | 480 (r/min) |
| Parameter | Distributions |
|---|---|
| Parameter | Distributions | Parameter | Distributions |
|---|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, Y.; Chen, X.; Wang, K.; Jiang, D.; Liu, J.; Pang, H. Uncertainty Control Method for Non-Uniform Wear of the Driving Mechanism of Flapping Wing Aircraft. Drones 2025, 9, 282. https://doi.org/10.3390/drones9040282
Jin Y, Chen X, Wang K, Jiang D, Liu J, Pang H. Uncertainty Control Method for Non-Uniform Wear of the Driving Mechanism of Flapping Wing Aircraft. Drones. 2025; 9(4):282. https://doi.org/10.3390/drones9040282
Chicago/Turabian StyleJin, Yujia, Xingyu Chen, Keke Wang, Deyin Jiang, Jingyi Liu, and Huan Pang. 2025. "Uncertainty Control Method for Non-Uniform Wear of the Driving Mechanism of Flapping Wing Aircraft" Drones 9, no. 4: 282. https://doi.org/10.3390/drones9040282
APA StyleJin, Y., Chen, X., Wang, K., Jiang, D., Liu, J., & Pang, H. (2025). Uncertainty Control Method for Non-Uniform Wear of the Driving Mechanism of Flapping Wing Aircraft. Drones, 9(4), 282. https://doi.org/10.3390/drones9040282






