Evaluation of Optimization Parameters of Semi-Solid Metal 6063 Aluminum Alloy from Friction Stir Welding Process Using Factorial Design Analysis
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
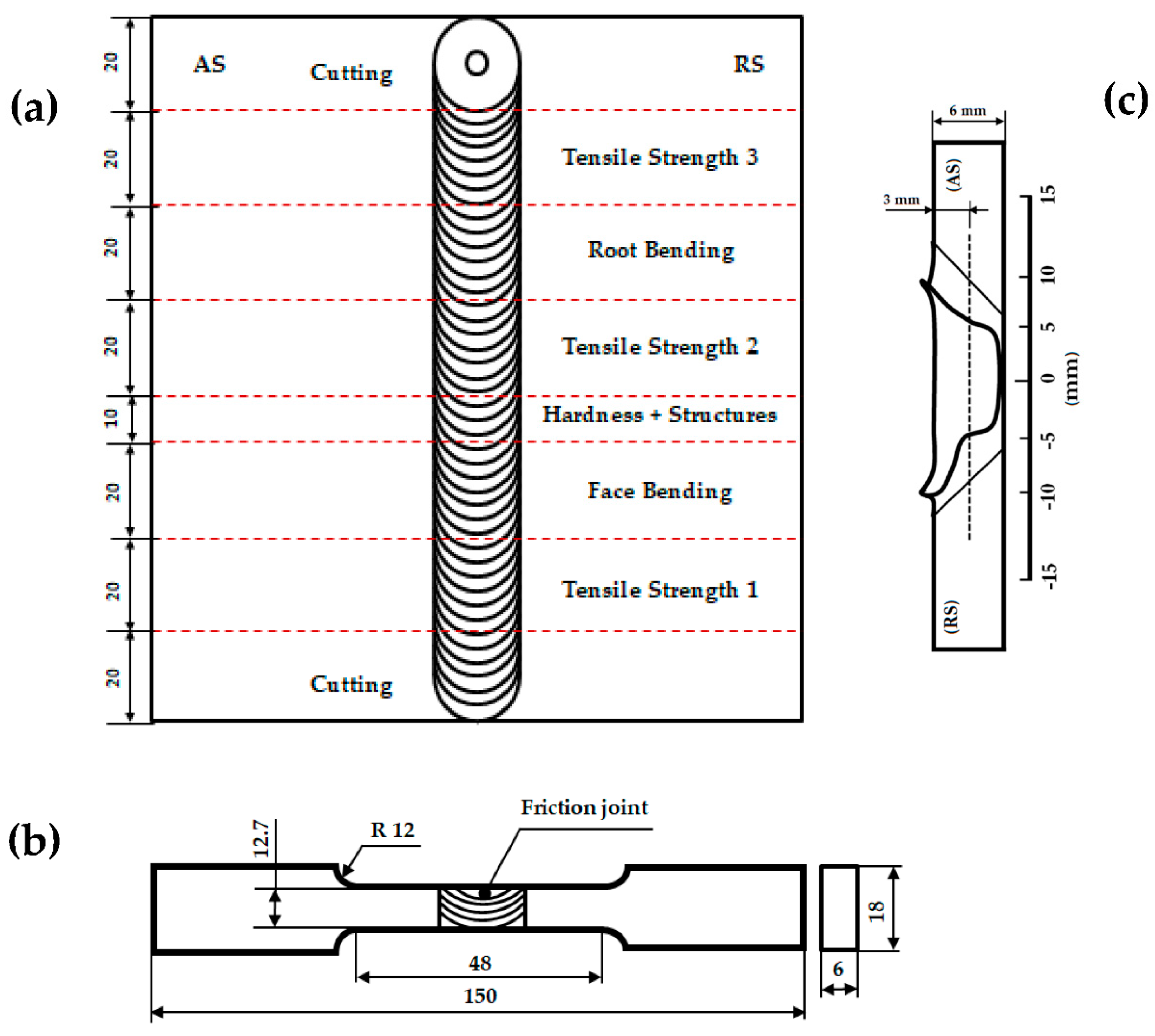
2.2. Experimental Procedures
2.3. Development of Regression Model
2.4. Mechanical Testing and Metallurgy
3. Results and Discussion
3.1. Optimization Parameters with General Full Factorial Design Analysis
3.2. Tensile Strength in the Joint Analysis
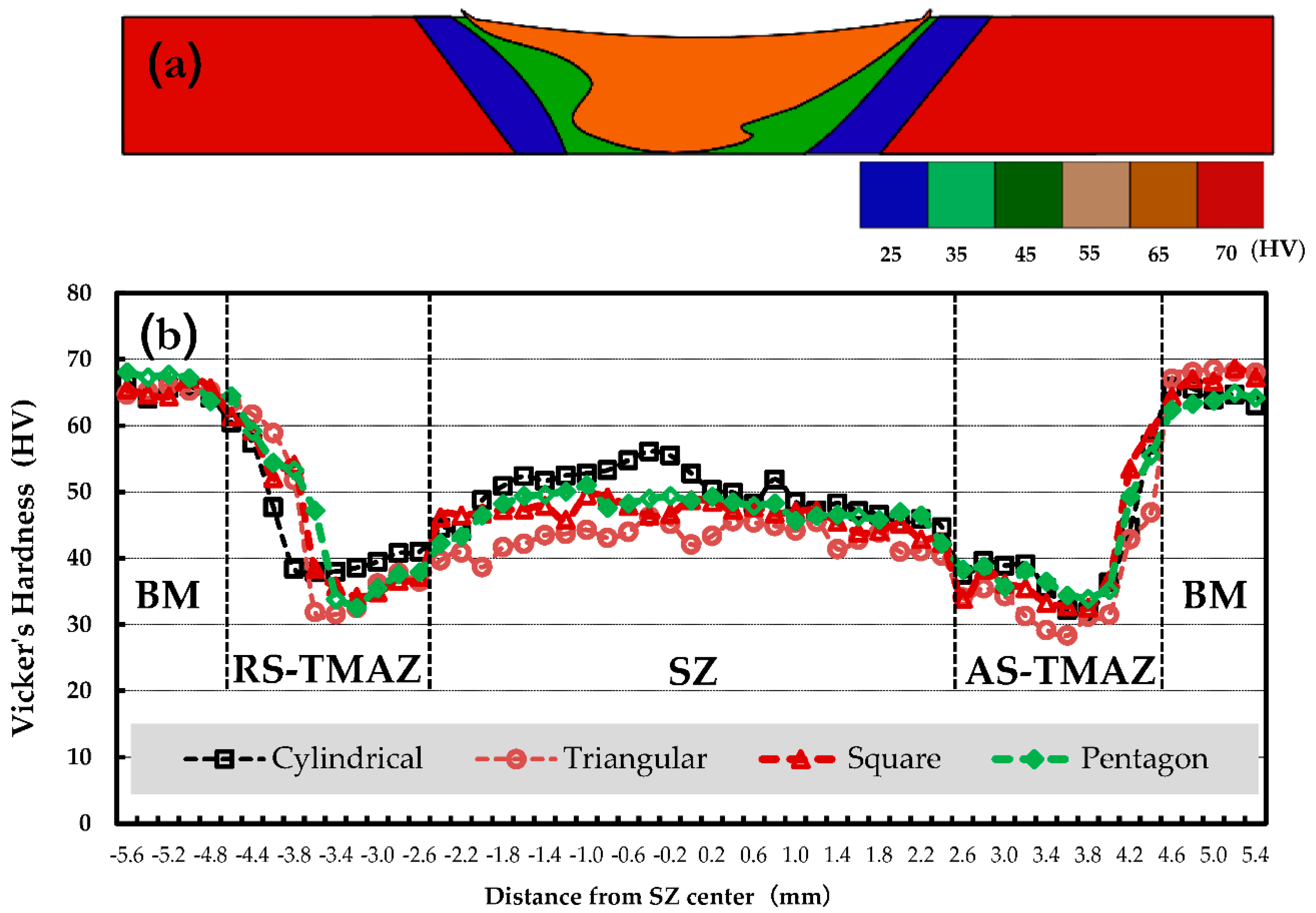
3.3. Vickers Hardness Analysis
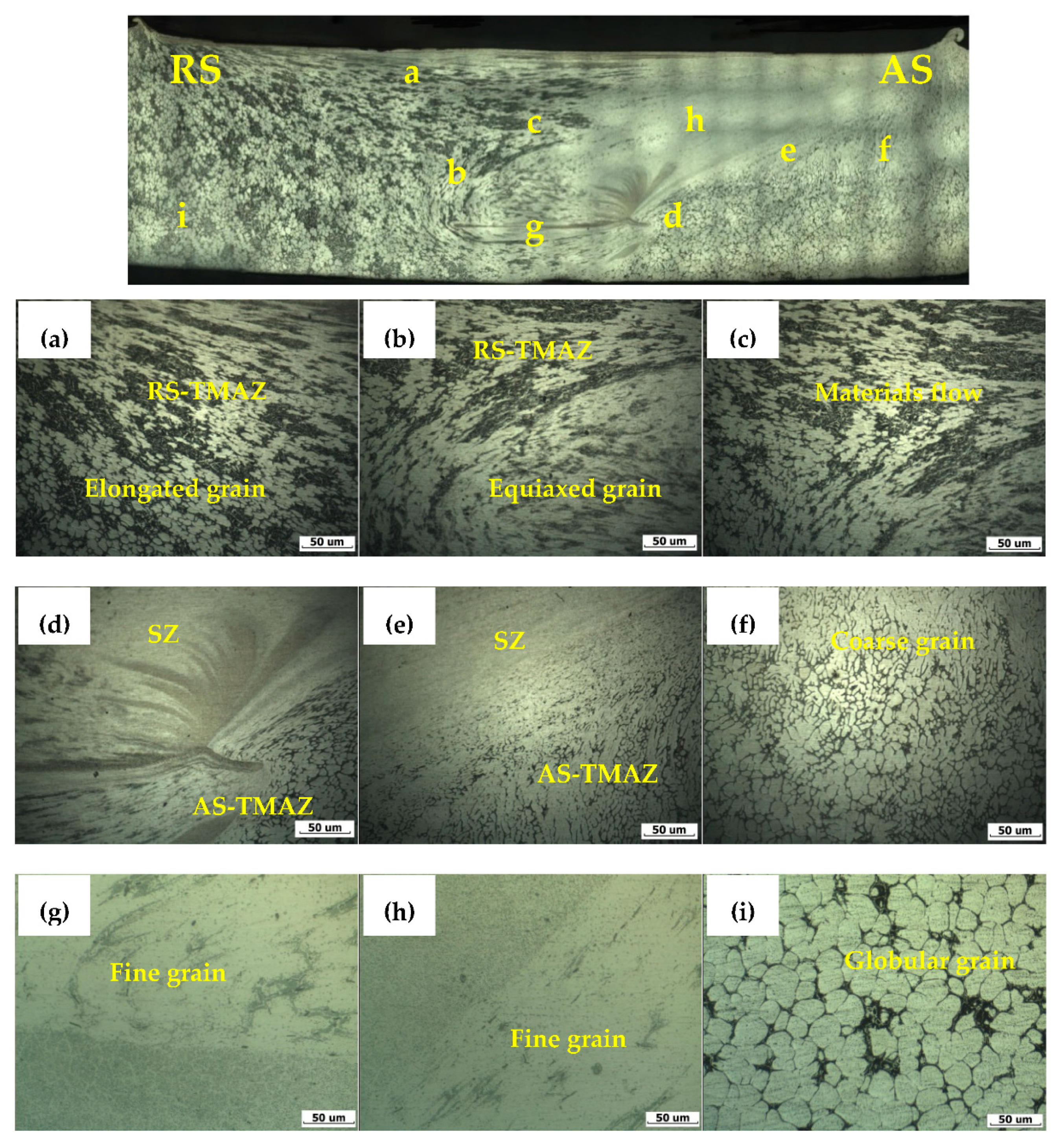
3.4. Microstructure in Joint Analysis
4. Conclusions
- For the optimization of tensile strength prediction from the regression equation, the mathematical relationship of factors found that the response optimizer was 86.17 to 138.41 MPa from cylindrical tool, rotation speed was 1300 to 2100 rpm, and welding speed was 75 mm/min with the coefficient of determination R2 at 95.09% when verifying tensile strength was 120.7 MPa with confident α = 0.05 and demonstrating the accuracy and precision.
- The maximum tensile strength was at 123.59 MPa, and maximum elongation was at 23 mm from the rotation speed at 1320 rpm and welding speed at 60 mm/min with the cylindrical tool. The joint efficiency was at 82.95%, compared to the tensile strength at 149.00 MPa of base SSM 6063 aluminum alloy. On the other hand, the lowest tensile strength (47.93 MPa) was from the rotation speed at 1110 rpm and welding speed at 120 mm/min; joint efficiency stayed consistent at 32.17%, in all, over AS-TMAZ.
- The hardness profiles in the welded joint are similar to “W” shape. It was also found that heat, friction force, and microstructure changes are the causes of different hardness in each area, and TMAZ shows the lowest hardness in comparison to other areas.
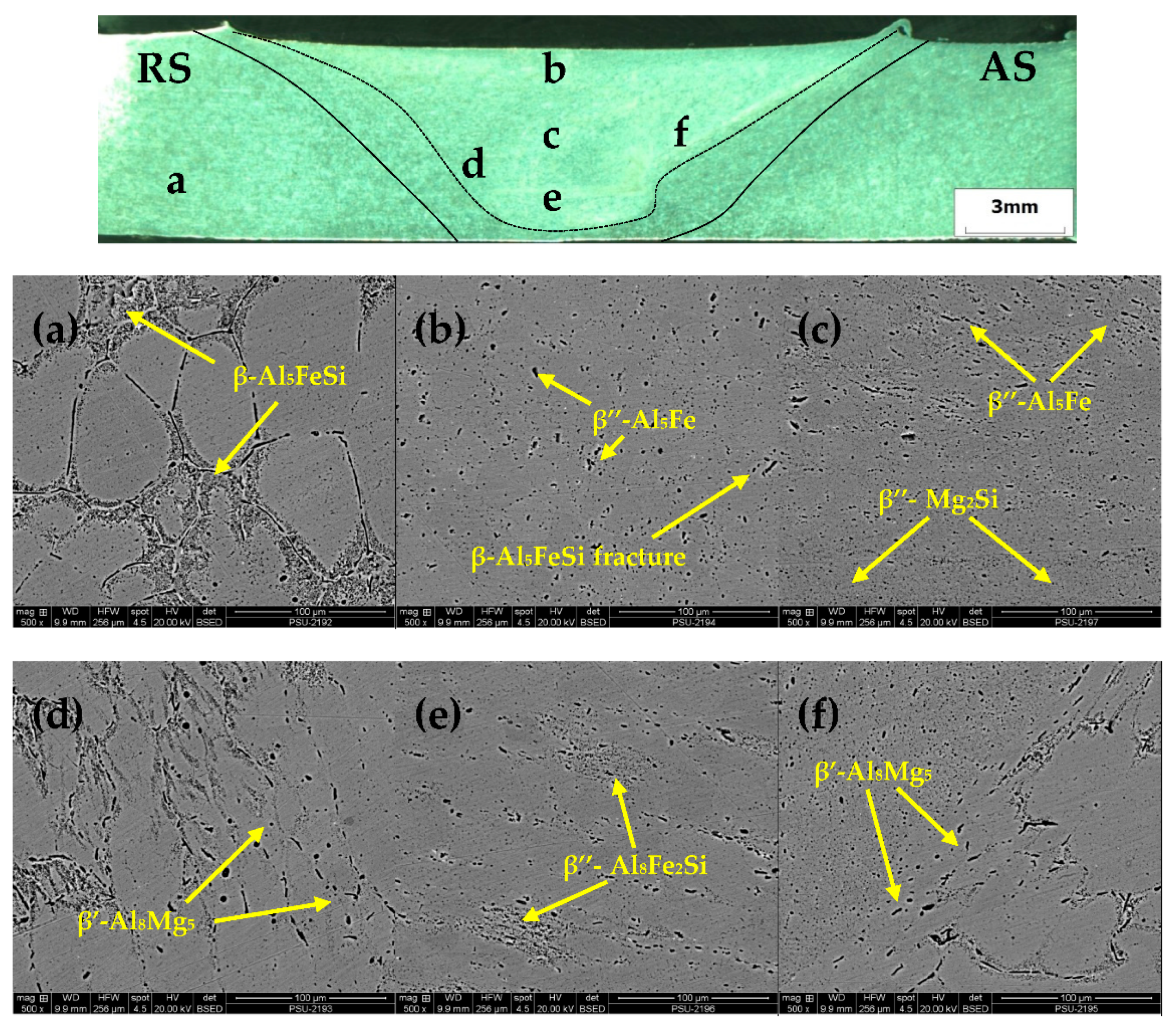
- The globular microstructure of base SSM 6063 aluminum alloy transformation phase was formed as new recrystallization of the microstructure, which can be grouped into three types of structural changes: (1) fine-grain areas in SZ, (2) coarse-grain areas in AS-TMAZ, and (3) equiaxed-grain areas in RS-TMAZ. Moreover, the average size of the intermetallic compound β-Al5FeSi phase was around 79–114 µm. Its structure changes from a needle-like structure of base particle precipitation to β″-Al5Fe phase, which was a small particle size with an average of 8–13 µm in SZ from rod-type and elements formula of Mg2Si and Al8Fe2Si phase observed.
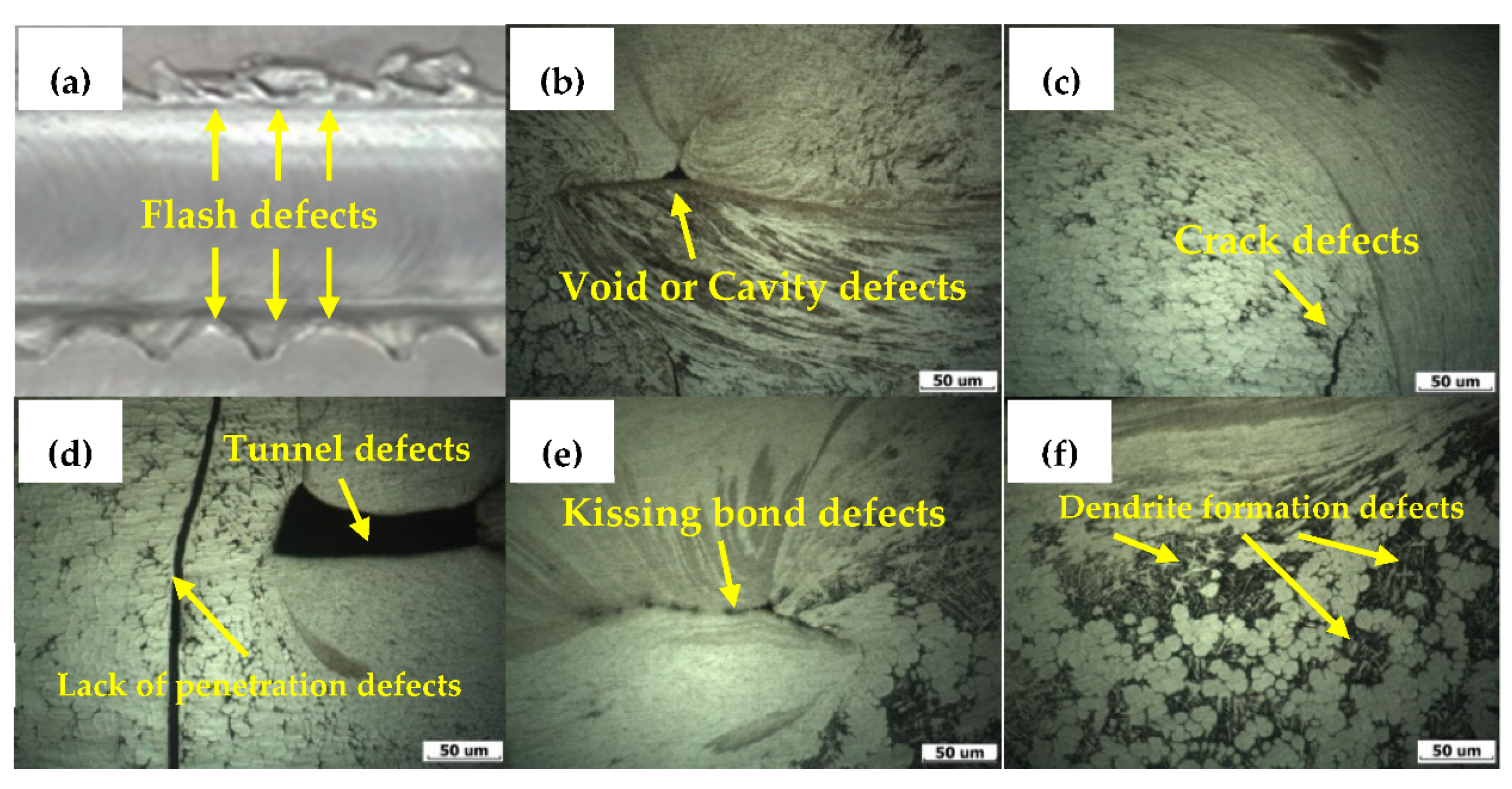
- Six types of defects after welding found in SZ and TMAZ come from the differences in welding parameters. There are flash defects, void or cavity defects, crack defects, lack of penetration defects, tunnel defects, kissing bond defects, and dendrite formation defects. These can affect the ability of the joint efficiency.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| SSM | semi-solid-metal |
| GISSM | gas-induced semi-solid-metal |
| FSWp | friction stir welding process |
| ANOVA | analysis of variance |
| GFFD | General Full Factorial Design |
| SEM | Scanning Electron Microscopy |
| EDX | energy-dispersive X-ray spectroscopy |
| OM | optical microscopy |
| BM | base metal |
| SZ | stir zone |
| TMAZ | thermal mechanical affect zone |
| AS-TMAZ | advancing-side thermal mechanical affect zone |
| RS-TMAZ | retracting-side thermal mechanical affect zone |
| ASTM | American Society for Testing and Materials |
References
- Alessio, G.; Paolo, M.; Fabio, D. Review of Aluminum-To-Steel Welding Technologies for Car-Body Applications. Metals 2019, 9, 315. [Google Scholar]
- Wannasin, J.; Janudom, S.; Rattanochaikul, T.; Canyook, R.; Burapa, R.; Chucheep, T.; Thanabumrungkul, S. Research and development of gas induced semi-solid process for industrial applications. Trans. Nonferrous Met. Soc. China 2010, 20, s1010–s1015. [Google Scholar] [CrossRef]
- Richter-Trummer, V.; Tavares, S.M.A.; Moreira, P.M.G.P.; Castro, P.M.S.T. Friction stir welding of aluminium alloys and damage tolerance of integral monolithic structures. Mechanics 2008, 73, 18–22. [Google Scholar]
- Ma, Z.Y.; Feng, A.H.; Chen, D.L.; Shen, J. Recent advance in friction stir welding/processing in aluminum alloys: Microstructure evaluation and mechanical properties. Crit. Rev. Solid State Mater. Sci. 2018, 43, 269–333. [Google Scholar] [CrossRef]
- Mishra, R.S.; Ma, Z.Y. Friction stir welding and processing. Mater Sci. Eng. R 2005, 50, 78. [Google Scholar] [CrossRef]
- Anand, R.; Sridhar, V.G. Studies on process parameters and tool geometry selecting aspects of friction stir welding. Mater. Today Proc. 2020, 27, 576–583. [Google Scholar] [CrossRef]
- Virendra, P.S.; Surendra, K.P.; Alok, R.; Basil, K. Recent research progress in solid state friction-stir welding of aluminum–magnesium alloys: A critical review. J. Mater. Res. Technol. 2020, 9, 6217–6256. [Google Scholar]
- Morteza, S.; Abbas, M.; Jalal, K.; Jerzy, S.A. Characterization and mechanical behavior of AISI316L/Incoloy825 dissimilar welds processed by friction stir welding. J. Manuf. Process. 2020, 55, 66–77. [Google Scholar]
- Meengam, C.; Chainarong, S.; Muangjunburee, P. Friction Welding of Semi-Solid Metal 7075 Aluminum Alloy. Mater. Today Proc. 2017, 4, 1303–1311. [Google Scholar] [CrossRef]
- Hua, J.; Yunlai, D.; Hongyong, X.; Sen, L.; Wenquan, W.; Honggang, D. The mechanism of rotational and non-rotational shoulder affecting the microstructure and mechanical properties of Al-Mg-Si alloy friction stir welded joint. Mater. Des. 2020, 192, 108729. [Google Scholar]
- Mir, M.H.; Hassan, B.T.; Nima, J. Thermal optimization of friction stir welding with simultaneous cooling using inverse approach. Appl. Therm. Eng. 2016, 108, 751–763. [Google Scholar]
- Moustafa, B.; Nadhir, L.; Amina, M.; Abdelhakim, S.; Mouloud, A. Friction stir welding process improvement through coupling an optimization procedure and three-dimensional transient heat transfer numerical analysis. J. Manuf. Process. 2018, 34, 566–578. [Google Scholar]
- Li, G.H.; Zhou, L.; Luo, S.F.; Dong, F.B.; Guo, N. Quality Improvement of Bobbin Tool Friction Stir Welds in Mg-Zn-Zr Alloy by Adjusting tool geometry. J. Mater. Process. Technol. 2020, 282, 14. [Google Scholar] [CrossRef]
- Pankul, G.; Arshad, N.S.; Noor, Z.K.; Mohd, A.H.; Zahid, A.K.; Mustufa, H.A.; Abdulrahman, A.A. Investigation on the Effect of Tool Pin Profiles on Mechanical and Microstructural Properties of Friction Stir Butt and Scarf Welded Aluminum Alloy 6063. Metals 2018, 8, 74. [Google Scholar]
- Joaquín, M.P.; Hernán, G.S. Tool geometry optimization in friction stir spot welding of Al-steel joints. J. Manuf. Process. 2017, 26, 142–154. [Google Scholar]
- Donatus, U.; da Silva, R.M.P.; de Sousa Araujo, J.V.; Milagre, M.X.; de Abreu, C.P.; Machado, C.D.S.C.; Costa, I. Macro and microgalvanic interactions in friction stir weldment of AA2198-T851 alloy. J. Mater. Res. Technol. 2019, 8, 6209–6222. [Google Scholar] [CrossRef]
- Anumat, S.; Vedavyas, T.; Indrajit, C.; Rajiv, S.M. Microstructure, mechanical properties and strengthening mechanisms of friction stir welded Kanthal APMT™ steel. J. Nucl. Mater. 2018, 509, 435–444. [Google Scholar]
- Kittima, S.; Yoshiharu, M.; Yukio, M.; Nobushiro, S. Fatigue Strength Estimation Based on Local Mechanical Properties for Aluminum Alloy FSW Joints. Metals 2017, 10, 186. [Google Scholar]
- Bayazid, S.M.; Farhangi, H.; Ghahramani, A. Investigation of friction stir welding parameters of 6063-7075 Aluminum alloys by Taguchi method. Mater. Today Proc. 2015, 11, 6–11. [Google Scholar] [CrossRef]
- Ahsok, K.; Murukan, N. Optimization of Friction Stir Welding Process Parameters to Maximize Tensile Strength of Stir Cast AA6061-T6/AINp Composite. Mater. Des. 2014, 57, 383–393. [Google Scholar]
- Palanivel, R.; Laubscher, R.F.; Vigneshwaran, S. Prediction and optimization of the mechanical properties of dissimilar friction stir welding of aluminum alloys using design of experiments. Proc. Inst. Mech. Eng. B. J. Eng. Manuf. 2016, 232, 1384–1394. [Google Scholar] [CrossRef]
- Wannasin, J.; Canyook, R.; Wisutmethangoon, S.; Flemings, M.C. Grain refinement behavior of an aluminum alloy by inoculation and dynamic nucleation. Acta Mater. 2013, 61, 3897–3903. [Google Scholar] [CrossRef]
- Kumar, N.; Mishra, R.S.; Huskamp, C.S.; Sankaran, K.K. Microstructure and mechanical behavior of friction stir processed ultrafine grained Al–Mg–Sc alloy. Mater. Sci. Eng. A 2011, 258, 5883–5887. [Google Scholar] [CrossRef]
- Noor, Z.K.; Zahid, A.K.; Arshad, N.S. Effect of shoulder diameter to pin diameter (D/d) ratio on tensile strength of friction stir welded 6063 aluminum alloy. Mater. Today Proc. 2015, 2, 1450–1457. [Google Scholar]
- Wang, D.; Xiao, B.L.; Wang, Q.Z.; Ma, Z.Y. Evolution of the Microstructure and strength in the nugget zone of friction stir welded SiCp/Al-Cu-Mg composite. J. Mater. Sci. Technol. 2014, 30, 54–60. [Google Scholar] [CrossRef]
- Dinaharan, I.; Murukan, N.; Parameswaran, S. Development and Empirical Relationship to Predict the influence of Process Parameters on Tensile Strength of Friction Stir Welded AA6061/0-10 wt% ZrB2 in Situ Composite. Trans. Indian Inst. Met. 2012, 65, 70–159. [Google Scholar] [CrossRef]
- Montgomery, D.C. Designing and Analysis of Experiments, 8th ed.; John Wiley & Sons Inc.: New York, NY, USA, 2000; pp. 24–146. [Google Scholar]
- Seetharaman, R.; Seeman, M.; Kanagarajan, D.; Sivaraj, P.; Saravanan, I. A statistical evaluation of the corrosion behaviour of friction stir welded AA2024 aluminum alloy. Mater. Today Proc. 2020, 22, 673–680. [Google Scholar] [CrossRef]
- Box, G.E.P.; Draper, N.R. Empirical Model-Building and Response Surface, 1st ed.; John Wiley & Sons Inc.: New York, NY, USA, 1987; pp. 52–189. [Google Scholar]
- Ryan, T.P. Modern Engineering Statistics, 1st ed.; John Wiley & Sons Inc.: New York, NY, USA, 2007; pp. 140–189. [Google Scholar]
- Salih, O.S.; Ou, H.; Wei, X.G.; Sun, W. Microstructure and mechanical properties of friction stir welded AA6092/SiC metal matrix composite. Mater. Sci. Eng. A 2019, 742, 78–88. [Google Scholar] [CrossRef]
- Zhanga, C.; Cui, L.; Liua, Y.; Liua, C.; Li, H. Microstructures and mechanical properties of friction stir welds on 9% Cr reduced activation ferritic/martensitic steel. J. Mater. Sci. Technol. 2018, 34, 756–766. [Google Scholar] [CrossRef]
- Yutaka, S.S.; Hiroyuki, K.; Masatoshi, E.; Shigetoshi, J. Microstructural Evolution of 6063 Aluminum during Friction Stir Welding. Metall. Mater. Trans. A 1999, 30A, 1999–2431. [Google Scholar]
- Zhang, X.X.; Wang, D.; Xiao, B.L. Enhanced multiscale modeling of macroscopic and microscopic residual stresses evolution during multi-thermo-mechanical processes. Mater. Des. 2017, 115, 364–378. [Google Scholar] [CrossRef]
- Moradi, M.M.; Jamshidi, A.H.; Jamaati, R.; Amirkhanlou, S.; Ji, S. Effect of SiC nanoparticles on the microstructure and texture of friction stir welded AA2024/AA6061. Mater. Charact. 2019, 152, 169–179. [Google Scholar] [CrossRef]
- Parikh, V.K.; Badgujar, A.D.; Ghetiya, N.D. Joining of metal matrix composites using friction stir welding: A review. Mater. Manuf. Process. 2018, 34, 123–146. [Google Scholar] [CrossRef]
- Zhou, L.; Li, G.H.; Zha, G.D.; Shu, F.Y.; Liu, H.J.; Feng, J.C. Effect of rotation speed on microstructure and mechanical properties of bobbin tool friction stir welded AZ61 magnesium alloy. Sci. Technol. Weld Join. 2018, 23, 596–605. [Google Scholar] [CrossRef]
- Heidarzadeh, A.; Saeid, T.; Klemm, V.; Chabok, A.; Pei, Y. Effect of stacking fault energy on the restoration mechanisms and mechanical properties of friction stir welded copper alloys. Mater. Des. 2019, 162, 185–197. [Google Scholar] [CrossRef]
- Javadi, M.; Tajdari, M. Experimental investigation of the friction coefficient between aluminum and steel. Mater. Sci.-Poland 2006, 24, 305–310. [Google Scholar]
- Karen, J.Q.; Jose, L.L.S. Mechanistic models and experimental analysis for the torque in FSW considering the tool geometry and the process velocities. J. Manuf. Process. 2017, 30, 406–417. [Google Scholar]
- Firouzdor, V.; Kou, S. Formation of liquid and intermetallic in Al-to-Mg friction stir welding. Metall. Mater. Trans. A 2010, 20, s619–s623. [Google Scholar] [CrossRef]
- Pourahmad, P.; Abbasi, M. Materials flow and phase transformation in friction stir welding of Al 6013/Mg. Trans. Nonferrous Met. Soc. China 2013, 23, 1253–1261. [Google Scholar] [CrossRef]
- Hi, H.; Chen, K.; Liang, Z.; Dong, F.; Yu, T.; Dong, X. Intermetallic compounds in the banded structure and their effect on mechanical properties of Al/Mg dissimilar friction stir welding joints. J. Mater. Sci. Technol. 2017, 33, 359–366. [Google Scholar]
- Rao, H.M.; Jordon, J.B.; Ghaffari, B.; Su, X.; Khosrovaneh, A.K.; Barkey, M.E. Fatigue and fracture of friction stir linear welded dissimilar aluminum to magnesium alloys. Int. J. Fatigue 2016, 82, 737–747. [Google Scholar] [CrossRef]
- Sharma, N.; Khan, Z.A.; Siddiquee, A.N. Friction stir welding of aluminum to copper—An overview. Trans. Nonferrous Met. Soc. China. 2017, 27, 2113–2136. [Google Scholar] [CrossRef]
- Bozkurt, Y.; Kentli, A.; Uzun, H.; Salman, S. Experimental investigation and prediction of mechanical properties of friction stir welded aluminum metal matrix composite plates. Mater. Sci. Medzg. 2012, 18, 336–340. [Google Scholar]
- Liu, H.; Hu, Y.Y.; Zhao, Y.Q.; Fujii, H. Improving the particle distribution and mechanical properties of friction-stir-welded composites by using a smooth pin tool. Mech. Compos. Mater. 2017, 53, 515–524. [Google Scholar] [CrossRef]
- Yutaka, S.S.; Hiroyuki, K.; Masatoshi, E.; Shigetoshi, J.; Takenori, H. Precipitation Sequence in Friction Stir Weld of 6063 Aluminum during Aging. Metall. Mater. Trans. A 1999, 30A, 1999–3125. [Google Scholar]
- Kumar, L.; Yazar, K.U.; Pramanik, S. Effect of fusion and friction stir welding techniques on the microstructure, crystallographic texture and mechanical properties of mild steel. Mater. Sci. Eng. A 2019, 754, 400–410. [Google Scholar] [CrossRef]
- Luo, C.; Li, X.; Song, D.; Zhou, N.; Li, Y.; Qi, W. Microstructure evolution and mechanical properties of friction stir welded dissimilar joints of Mg–Zn–Gd and Mg–Al–Zn alloys. Mater. Sci. Eng. A 2016, 664, 103–113. [Google Scholar] [CrossRef]

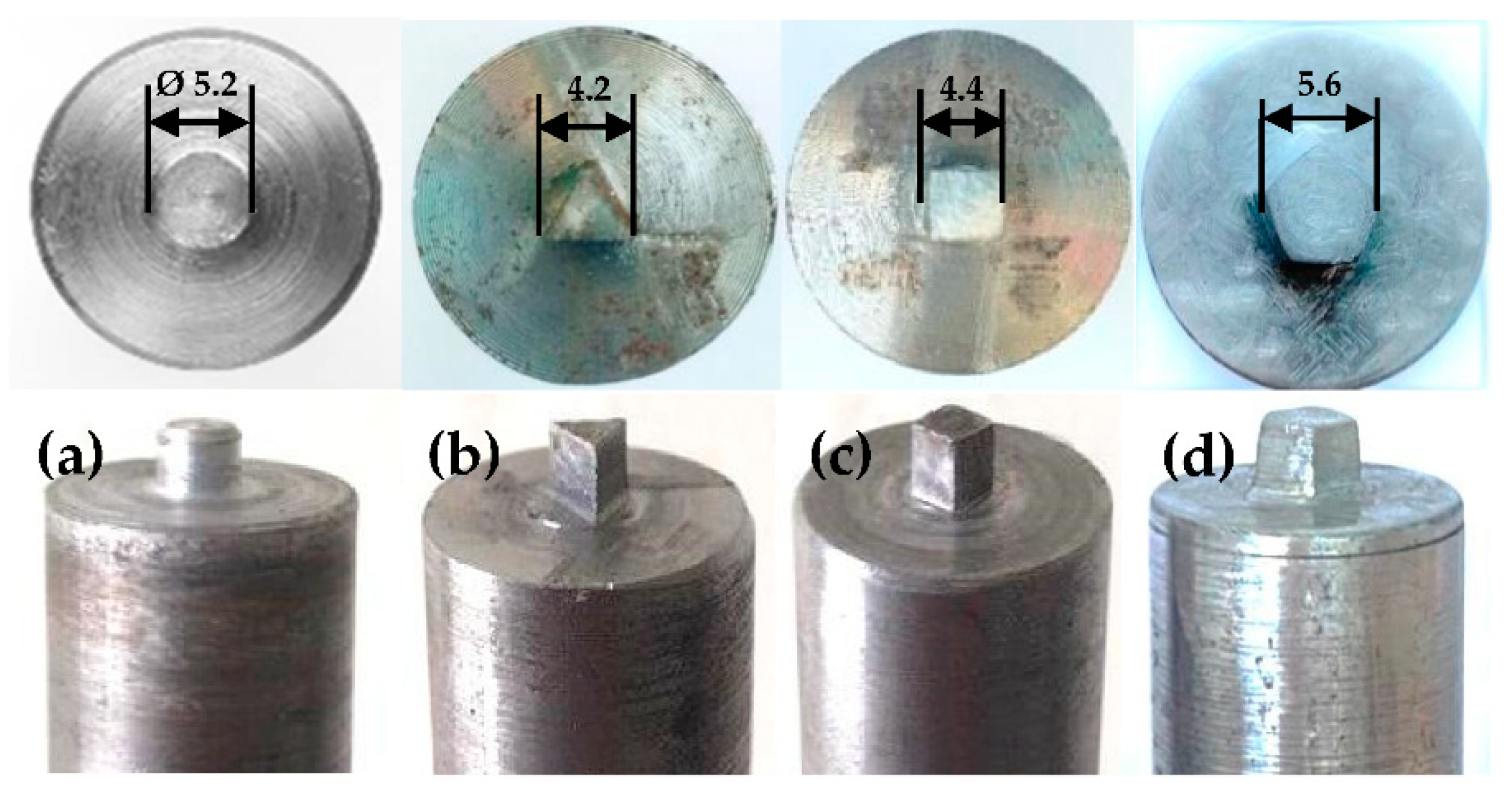




| Element | Mg | Si | Fe | Others | Al |
|---|---|---|---|---|---|
| SSM 6063 | 0.45–0.90 | 0.20–0.60 | 0.35 | 0.15 | Balance |
| Ultimate Tensile Strength in MPa | Elongation in mm | Yield Strength in MPa | Hardness in HV |
|---|---|---|---|
| 149 | 27–42 | 112 | 67 |
| Experiment No. | Parameters | Notation | Unit | Levels | |||
|---|---|---|---|---|---|---|---|
| −1 | −2 | −3 | −4 | ||||
| 1 | Rotation speed | R | Rpm | 1100 | 1320 | 1750 | 2220 |
| 2 | Welding speed | W | mm/min | 30 | 60 | 90 | 120 |
| 3 | Tool geometry | T | --- | Cylindrical | Triangular | Square | Pentagon |
| Experiment No. | Design Matrix Process | Tensile Strength (MPa) | Joint Efficiency (%) | Experiment No. | Design Matrix Process | Tensile Strength (MPa) | Joint Efficiency (%) | ||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| R | W | T | R | W | T | ||||||
| 1 | −4 | −4 | −1 | 101.52 | 68.13 | 33 | −4 | −3 | −4 | 108.45 | 72.79 |
| 2 | −3 | −2 | −4 | 105.22 | 70.62 | 34 | −2 | −3 | −2 | 98.41 | 66.05 |
| 3 | −2 | −4 | −4 | 96.00 | 64.43 | 35 | −4 | −1 | −3 | 83.51 | 56.05 |
| 4 | −4 | −4 | −3 | 79.87 | 53.60 | 36 | −2 | −4 | −1 | 83.35 | 55.94 |
| 5 | −4 | −2 | −3 | 84.64 | 56.81 | 37 | −3 | −4 | −3 | 81.02 | 54.38 |
| 6 | −1 | −4 | −3 | 74.09 | 49.72 | 38 | −3 | −1 | −1 | 120.20 | 80.67 |
| 7 | −3 | −4 | −4 | 111.11 | 74.57 | 39 | −3 | −1 | −2 | 107.00 | 71.81 |
| 8 | −3 | −2 | −2 | 78.44 | 52.64 | 40 | −3 | −3 | −1 | 107.27 | 71.99 |
| 9 | −1 | −2 | −3 | 108.25 | 72.65 | 41 | −4 | −4 | −4 | 88.51 | 59.40 |
| 10 | −2 | −1 | −2 | 104.79 | 70.33 | 42 | −2 | −1 | −3 | 97.23 | 65.26 |
| 11 | −2 | −1 | −4 | 108.99 | 73.15 | 43 | −4 | −2 | −2 | 100.29 | 67.31 |
| 12 | −1 | −3 | −2 | 63.37 | 42.53 | 44 | −4 | −4 | −2 | 76.62 | 51.42 |
| 13 | −1 | −1 | −3 | 111.55 | 74.87 | 45 | −4 | −3 | −1 | 97.08 | 65.15 |
| 14 | −1 | −2 | −2 | 101.42 | 68.07 | 46 | −1 | −1 | −2 | 103.39 | 69.39 |
| 15 | −4 | −1 | −1 | 113.96 | 76.48 | 47 | −2 | −3 | −1 | 113.18 | 75.96 |
| 16 | −3 | −3 | −2 | 89.09 | 59.79 | 48 | −1 | −3 | −4 | 107.98 | 72.47 |
| 17 | −1 | −1 | −4 | 112.74 | 75.66 | 49 | −1 | −2 | −1 | 101.85 | 68.36 |
| 18 | −1 | −2 | −4 | 114.76 | 77.02 | 50 | −3 | −2 | −3 | 115.53 | 77.54 |
| 19 | −4 | −3 | −3 | 111.58 | 74.89 | 51 | −2 | −3 | −3 | 93.00 | 62.42 |
| 20 | −1 | −3 | −1 | 90.07 | 60.45 | 52 | −1 | −4 | −1 | 88.79 | 59.59 |
| 21 | −1 | −4 | −4 | 80.99 | 54.36 | 53 | −4 | −1 | −4 | 101.08 | 67.84 |
| 22 | −3 | −1 | −4 | 108.35 | 72.72 | 54 | −4 | −1 | −2 | 96.25 | 64.60 |
| 23 | −4 | −2 | −1 | 111.11 | 74.57 | 55 | −2 | −2 | −2 | 102.66 | 68.90 |
| 24 | −3 | −2 | −1 | 115.43 | 77.47 | 56 | −2 | −4 | −2 | 65.15 | 43.72 |
| 25 | −2 | −2 | −3 | 96.03 | 64.45 | 57 | −3 | −4 | −2 | 82.07 | 55.08 |
| 26 | −2 | −3 | −4 | 95.09 | 63.82 | 58 | −3 | −3 | −4 | 91.06 | 61.11 |
| 27 | −1 | −4 | −2 | 47.93 | 32.17 | 59 | −2 | −4 | −3 | 83.36 | 55.95 |
| 28 | −2 | −1 | −1 | 114.98 | 77.17 | 60 | −3 | −1 | −3 | 101.90 | 68.39 |
| 29 | −2 | −2 | −1 | 123.59 | 82.95 | 61 | −1 | −3 | −3 | 105.70 | 70.94 |
| 30 | −2 | −2 | −4 | 118.76 | 79.70 | 62 | −4 | −2 | −4 | 112.70 | 75.64 |
| 31 | −3 | −4 | −1 | 106.53 | 71.50 | 63 | −3 | −3 | −3 | 92.70 | 62.21 |
| 32 | −1 | −1 | −1 | 115.50 | 77.52 | 64 | −4 | −3 | −2 | 83.39 | 55.97 |
| Source | DF | Adj SS | Adj MS | F-Value | p-Value | |
|---|---|---|---|---|---|---|
| Regression Model | 63 | 43,840.4 | 695.88 | 39.33 | 0.000 | Significant |
| Linear | 9 | 27,307.9 | 3034.21 | 171.50 | 0.000 | |
| R | 3 | 890.3 | 296.76 | 16.77 | 0.000 | |
| W | 3 | 15,497.5 | 5165.82 | 291.99 | 0.000 | |
| T | 3 | 10,920.2 | 3640.05 | 205.75 | 0.000 | |
| 2-Way Interactions | 27 | 9341.8 | 345.99 | 19.56 | 0.000 | |
| R × W | 9 | 4264.1 | 373.79 | 26.78 | 0.000 | |
| R × T | 9 | 2320.7 | 257.89 | 14.57 | 0.000 | |
| W × T | 9 | 2757.0 | 306.33 | 17.31 | 0.000 | |
| 3-Way Interactions | 27 | 7190.7 | 266.32 | 15.05 | ||
| R × W × T | 27 | 7190.7 | 266.32 | 15.05 | ||
| Pure Error | 128 | 2264.6 | 17.69 | |||
| Total | 191 | 46,105.0 | ||||
| S = 4.20619 | R2 = 95.09% Adjusted R2 = 92.67% | |||||
| Prediction for Shear Strength | ||||
|---|---|---|---|---|
| Multiple response prediction | ||||
| Variables | Setting | |||
| Rotation speed (rpm) | 2220 | |||
| Welding speed (mm/min) | 30 | |||
| Tool geometry | 1 | |||
| Response | Fit | SE Fit | 95% CI | 95% PI |
| Tensile strength | 112.29 | 2.43 | 107.49, 117.09 | 86.17, 138.41 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meengam, C.; Sillapasa, K. Evaluation of Optimization Parameters of Semi-Solid Metal 6063 Aluminum Alloy from Friction Stir Welding Process Using Factorial Design Analysis. J. Manuf. Mater. Process. 2020, 4, 123. https://doi.org/10.3390/jmmp4040123
Meengam C, Sillapasa K. Evaluation of Optimization Parameters of Semi-Solid Metal 6063 Aluminum Alloy from Friction Stir Welding Process Using Factorial Design Analysis. Journal of Manufacturing and Materials Processing. 2020; 4(4):123. https://doi.org/10.3390/jmmp4040123
Chicago/Turabian StyleMeengam, Chaiyoot, and Kittima Sillapasa. 2020. "Evaluation of Optimization Parameters of Semi-Solid Metal 6063 Aluminum Alloy from Friction Stir Welding Process Using Factorial Design Analysis" Journal of Manufacturing and Materials Processing 4, no. 4: 123. https://doi.org/10.3390/jmmp4040123
APA StyleMeengam, C., & Sillapasa, K. (2020). Evaluation of Optimization Parameters of Semi-Solid Metal 6063 Aluminum Alloy from Friction Stir Welding Process Using Factorial Design Analysis. Journal of Manufacturing and Materials Processing, 4(4), 123. https://doi.org/10.3390/jmmp4040123






