Fracture Characterisation and Modelling of AHSS Using Acoustic Emission Analysis for Deep Drawing †
Abstract
:1. Introduction
1.1. Failure Modelling for Finite Element Simulation
1.2. Material Characterisation Using Acoustic Emission Analysis
2. Materials and Methods
2.1. Material Characterisation
2.2. Material Modelling
2.3. Deep Drawing Simulations and Experiments
3. Results and Discussion
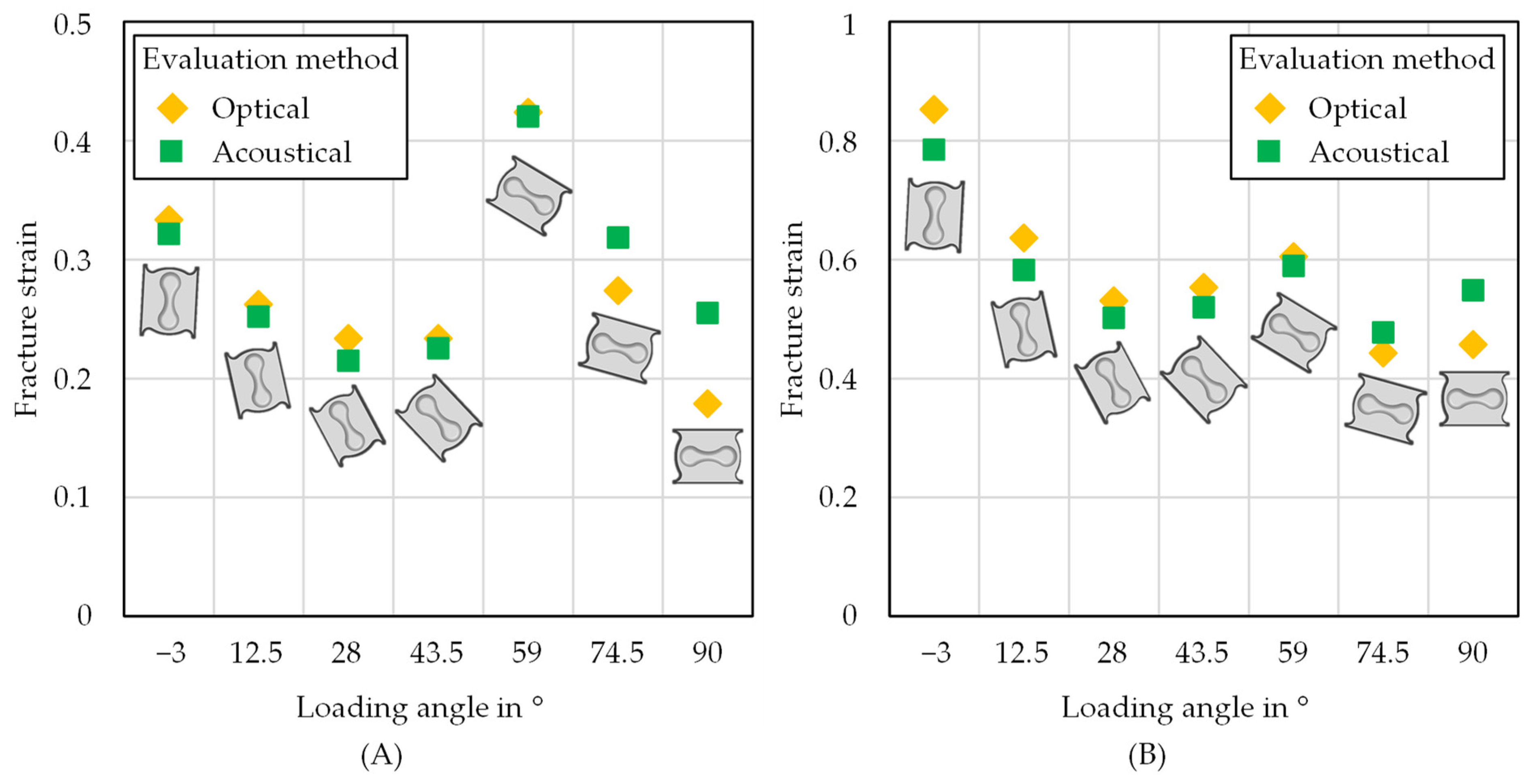
3.1. Comparison of the Fracture Evaluation Methods
3.2. Impact of the Fracture Modelling
4. Summary and Conclusions
- No significant difference could be observed regarding the displacement at fracture from the optical and acoustical evaluation method for the materials under investigation.
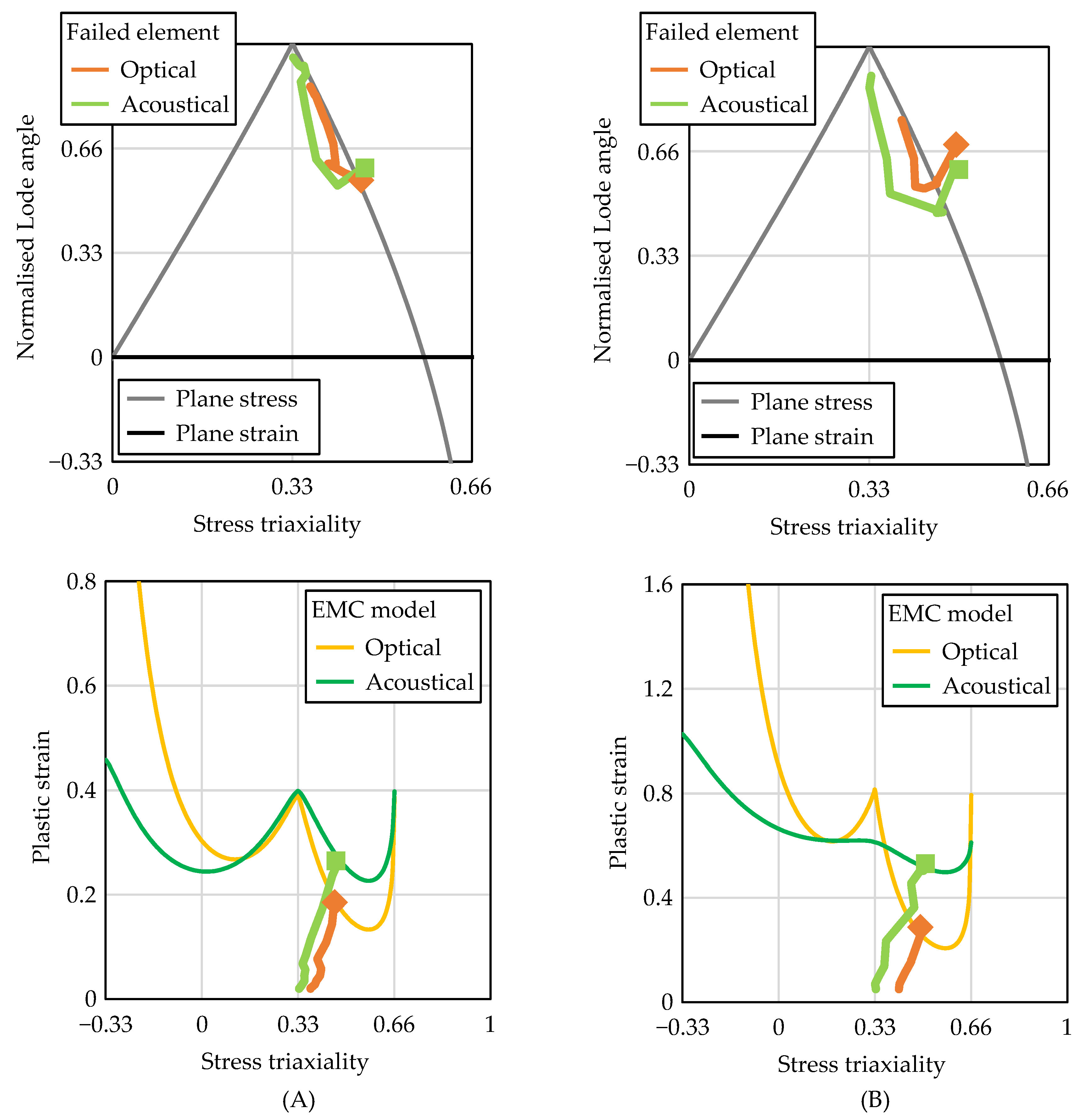
- For higher loading angles, the characteristic stress states from the acoustical evaluation method are shifted to increased stress triaxialities compared to the optical method.
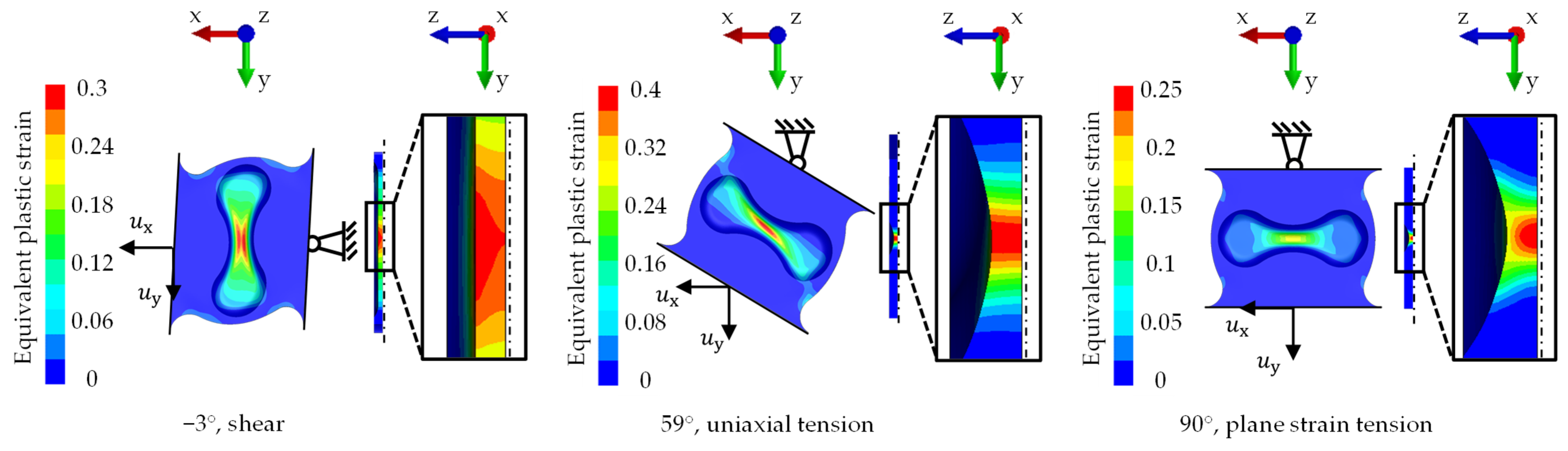
- The equivalent plastic strain at the fracture shows a gradient over the sheet thickness for higher loading angles, which leads to increased values for the acoustical method.
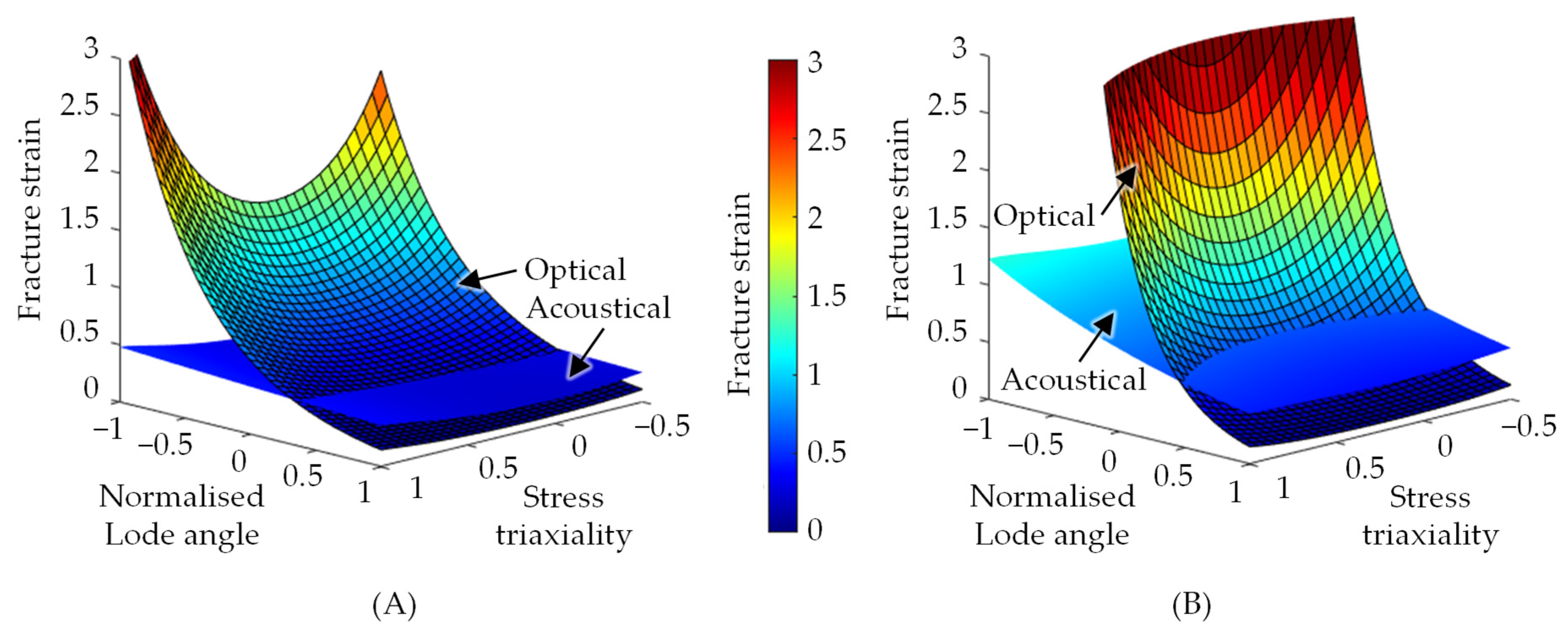
- The differences in the characteristic stress state and the fracture strain lead to a steeper development of the optical EMC fracture model than the acoustical model.
- In a process simulation, the optical EMC fracture model predicted the material fracture too early compared to experimental investigations.
- Overall, the area where fracture initiation is analysed can have a high impact on fracture modelling depending on the material used and the specimen’s geometry.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fonstein, N. Dual-phase steels. In Automotive Steels—Design, Metallurgy, Processing and Applications; Elsevier: Amsterdam, The Netherlands, 2017; pp. 169–216. [Google Scholar] [CrossRef]
- Behrens, B.-A.; Rosenbusch, D.; Wester, H.; Stockburger, E. Material Characterization and Modeling for Finite Element Simulation of Press Hardening with AISI 420C. J. Mater. Eng. Perform. 2022, 31, 825–832. [Google Scholar] [CrossRef]
- Behrens, B.-A.; Dröder, K.; Hürkamp, A.; Droß, M.; Wester, H.; Stockburger, E. Finite Element and Finite Volume Modelling of Friction Drilling HSLA Steel under Experimental Comparison. Materials 2021, 14, 5997. [Google Scholar] [CrossRef] [PubMed]
- Mohr, D.; Marcadet, S.J. Micromechanically-motivated phenomenological Hosford–Coulomb model for predicting ductile fracture initiation at low stress triaxialities. Int. J. Sol. Struc. 2015, 67–68, 40–55. [Google Scholar] [CrossRef]
- Bai, Y.; Wierzbicki, T. Application of extended Mohr–Coulomb criterion to ductile fracture. Int. J. Fract. 2010, 161, 1–20. [Google Scholar] [CrossRef]
- Martinez-Gonzalez, E.; Picas, I.; Casellas, D.; Romeu, J. Detection of crack nucleation and growth in tool steels using fracture tests and acoustic emission. Meccanica 2015, 50, 1155–1166. [Google Scholar] [CrossRef]
- Behrens, B.-A.; Hübner, S.; Wölki, K. Acoustic Emission—A promising and challenging technique for process monitoring in sheet metal forming. J. Manu Proc. 2017, 29, 281–288. [Google Scholar] [CrossRef]
- Shen, G.; Li, L.; Zhang, Z.; Wu, Z. Acoustic Emission Behavior of Titanium During Tensile Deformation. In Advances in Acoustic Emission Technology; Springer: New York, NY, USA, 2015; pp. 235–243. [Google Scholar] [CrossRef]
- Murav’ev, T.V.; Zuev, L.B. Acoustic emission during the development of a Lüders band in a low-carbon steel. Tech. Phys. 2008, 53, 1094–1098. [Google Scholar] [CrossRef]
- Chuluunbat, T.; Lu, C.; Kostryzhev, A.; Tieu, K. Investigation of X70 line pipe steel fracture during single edge-notched tensile testing using acoustic emission monitoring. Mater. Sci. Eng. A 2015, 640, 471–479. [Google Scholar] [CrossRef]
- Panasiuk, K.; Kyziol, L.; Dudzik, K.; Hajdukiewicz, G. Application of the Acoustic Emission Method and Kolmogorov-Sinai Metric Entropy in Determining the Yield Point in Aluminium Alloy. Materials 2020, 13, 1386. [Google Scholar] [CrossRef] [Green Version]
- Piñal-Moctezuma, F.; Delgado-Prieto, M.; Romeral-Martínez, L. An acoustic emission activity detection method based on short-term waveform features: Application to metallic components under uniaxial tensile test. Mech. Syst. Signal Process. 2020, 142, 106753. [Google Scholar] [CrossRef] [Green Version]
- Petit, J.; Montay, G.; François, M. Strain Localization in Mild (Low Carbon) Steel Observed by Acoustic Emission—ESPI Coupling during Tensile Test. Exp. Mech. 2018, 58, 743–758. [Google Scholar] [CrossRef]
- Khamedi, R.; Fallahi, A.; Refahi Oskouei, A. Effect of martensite phase volume fraction on acoustic emission signals using wavelet packet analysis during tensile loading of dual phase steels. Mat. Des. 2010, 31, 2752–2759. [Google Scholar] [CrossRef]
- Pathak, N.; Adrien, J.; Butcher, C.; Maire, E.; Worswick, M. Experimental stress state-dependent void nucleation behavior for advanced high strength steels. Int. J. Mech. Sci. 2020, 179, 105661. [Google Scholar] [CrossRef]
- DP1000 Voestalpine Steel Division. Data Sheet Dual-Phase Steels. June 2022. Available online: https://www.voestalpine.com/stahl/en/content/download/4523/file/voestalpine_datasheet_dual-phase_steels_EN_20170531.pdf (accessed on 2 May 2023).
- CP8000 Voestalpine Steel Division. Data Sheet Complex Phase Steels. October 2022. Available online: https://www.voestalpine.com/stahl/en/content/download/4522/file/voestalpine_datasheet_complexphase_steels_EN_20180711.pdf (accessed on 2 May 2023).
- DIN EN ISO 10275:2020; Metallic Materials—Sheet and Strip—Determination of Tensile Strain Hardening Exponent. Beuth: Berlin, Germany, 2020. [CrossRef]
- Pelleg, J. Mechanical Testing of Materials. In Mechanical Properties of Materials; Springer: Berlin/Heidelberg, Germany, 2013; pp. 1–84. [Google Scholar] [CrossRef]
- DIN EN ISO 10113:2020; Metallic Materials—Sheet and Strip—Determination of Plastic Strain Ratio. Beuth: Berlin, Germany, 2021. [CrossRef]
- DIN EN ISO 16808:2022-08; Metallic Materials—Sheet and Strip—Determination of Biaxial Stress-Strain Curve by Means of Bulge Test with Optical Measuring Systems. Beuth: Berlin, Germany, 2022.
- Sigvant, M.; Mattiasson, K.; Vegter, H.; Thilderkvist, H. A viscous pressure bulge test for the determination of a plastic hardening curve and equibiaxial material data. Int. J. Mater. Form. 2009, 2, 235–242. [Google Scholar] [CrossRef]
- Peshekhodov, I.; Jiang, S.; Vucetic, M.; Bouguecha, A.; Behrens, B.-A. Experimental-numerical evaluation of a new butterfly specimen for fracture characterisation of AHSS in a wide range of stress states. IOP Conf. Ser. Mater. Sci. Eng. 2016, 159, 012015. [Google Scholar] [CrossRef]
- Stockburger, E.; Vogt, H.; Wester, H.; Hübner, S.; Behrens, B.-A. Evaluating Material Failure of AHSS Using Acoustic Emission Analysis. Mat. Res. Pro. 2023, 25, 379–386. [Google Scholar] [CrossRef]
- Swift, H.W. Plastic instability under plane stress. J. Mech. Phys. Solids 1952, 1, 1–18. [Google Scholar] [CrossRef]
- Barlat, F.; Brem, J.C.; Yoon, J.W.; Chung, K.; Dick, R.E.; Lege, D.J.; Pourboghrat, F.; Choi, S.H.; Chu, E. Plane Stress Yield Function for Aluminum Alloy Sheets—Part 1: Theory. Int. J. Plast. 2003, 19, 1297–1319. [Google Scholar] [CrossRef]
- Grüner, M.; Merklein, M. Determination of friction coefficients in deep drawing by modification of Siebel’s formula for calculation of ideal drawing force. Prod. Eng. Res. Devel. 2014, 8, 577–584. [Google Scholar] [CrossRef]
- Neukamm, F.; Feucht, M.; Haufe, A.; Roll, K. On closing the constitutive gap between forming and crash simulation. In Proceedings of the 10th International LS-DYNA Users Conference, Dearborn, MI, USA, 8–10 June 2008. [Google Scholar]
- Heibel, S.; Dettinger, T.; Nester, W.; Clausmeyer, T.; Tekkaya, A.E. Damage Mechanisms and Mechanical Properties of High-Strength Multiphase Steels. Materials 2018, 11, 761. [Google Scholar] [CrossRef] [Green Version]
- Pathak, N.; Butcher, C.; Adrien, J.; Maire, E.; Worswick, M. Micromechanical modelling of edge failure in 800 MPa advanced high strength steels. J. Mech. Phys. Solids 2020, 137, 103855. [Google Scholar] [CrossRef]
- Bao, Y.; Wierzbicki, T. On fracture locus in the equivalent strain and stress triaxiality space. Int. J. Mech. Sci. 2004, 46, 81–98. [Google Scholar] [CrossRef]




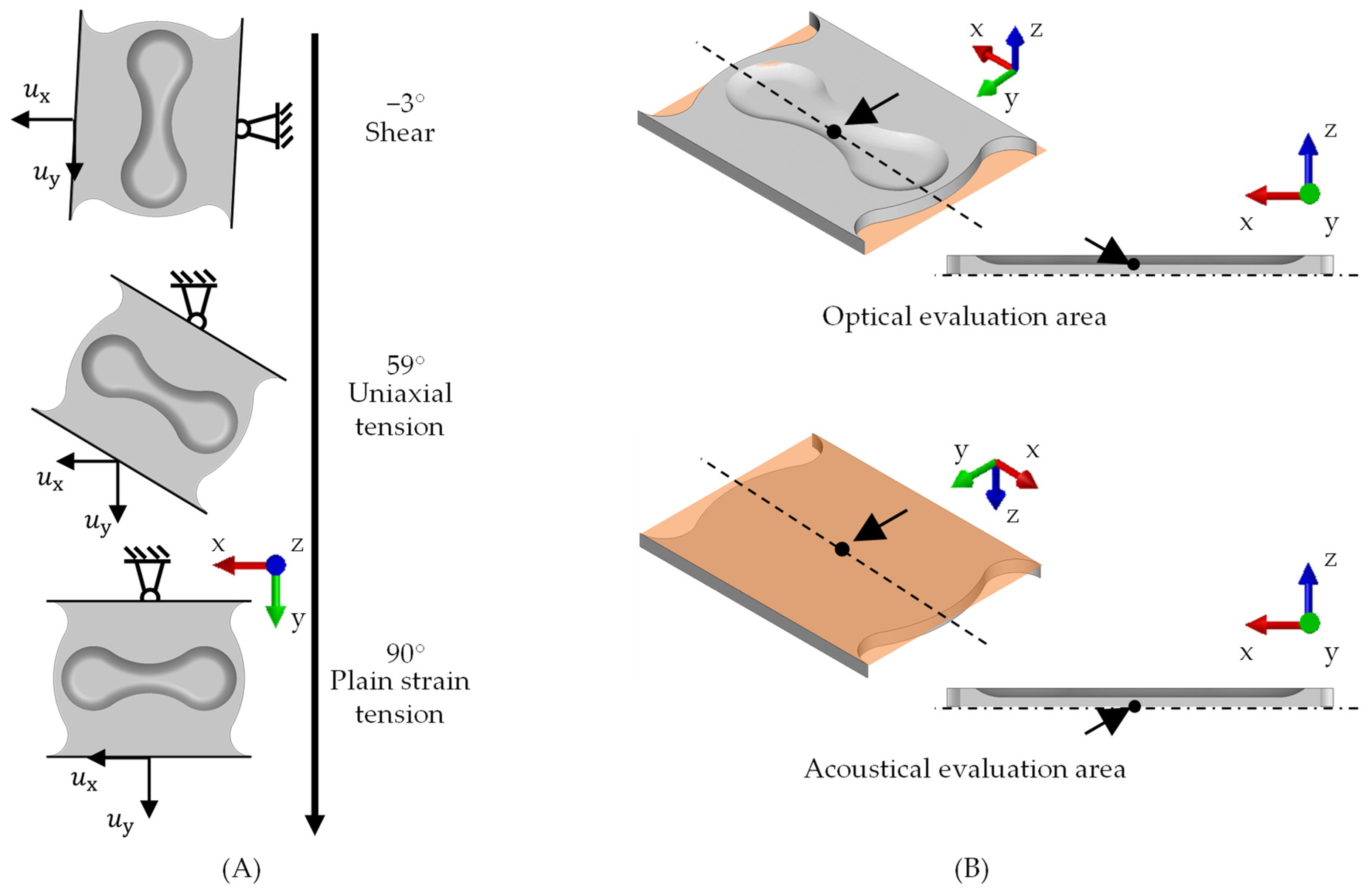
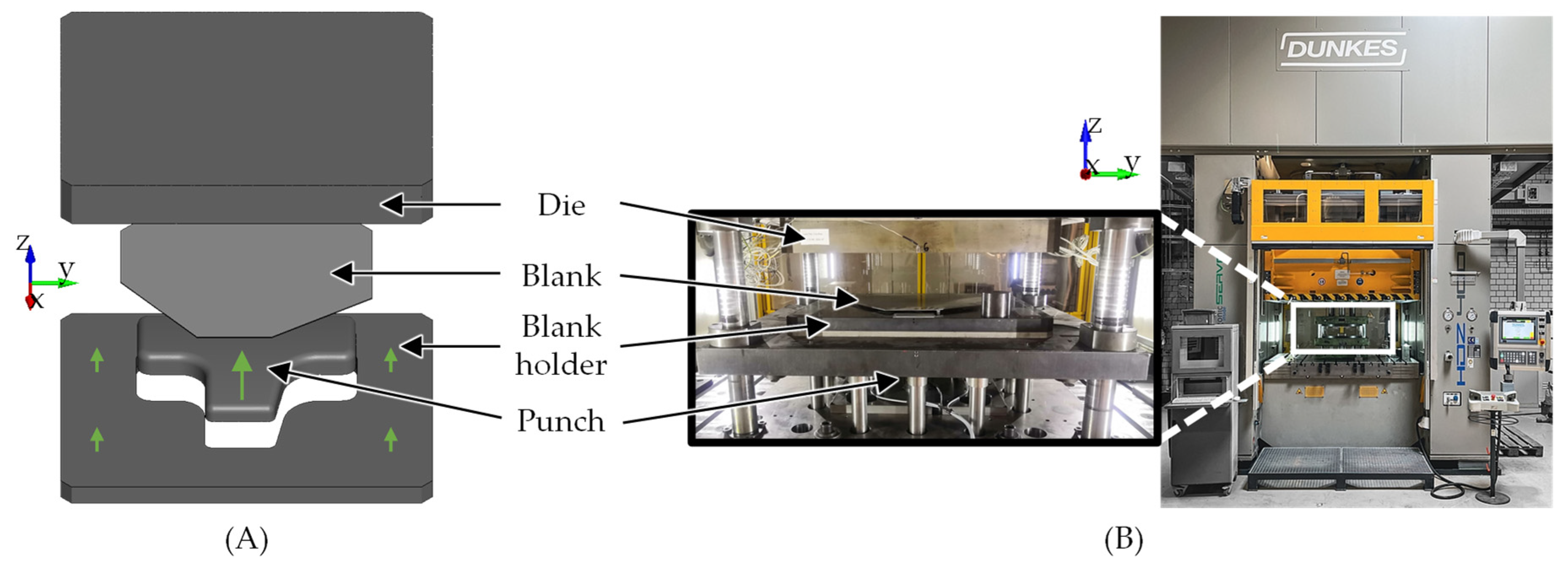

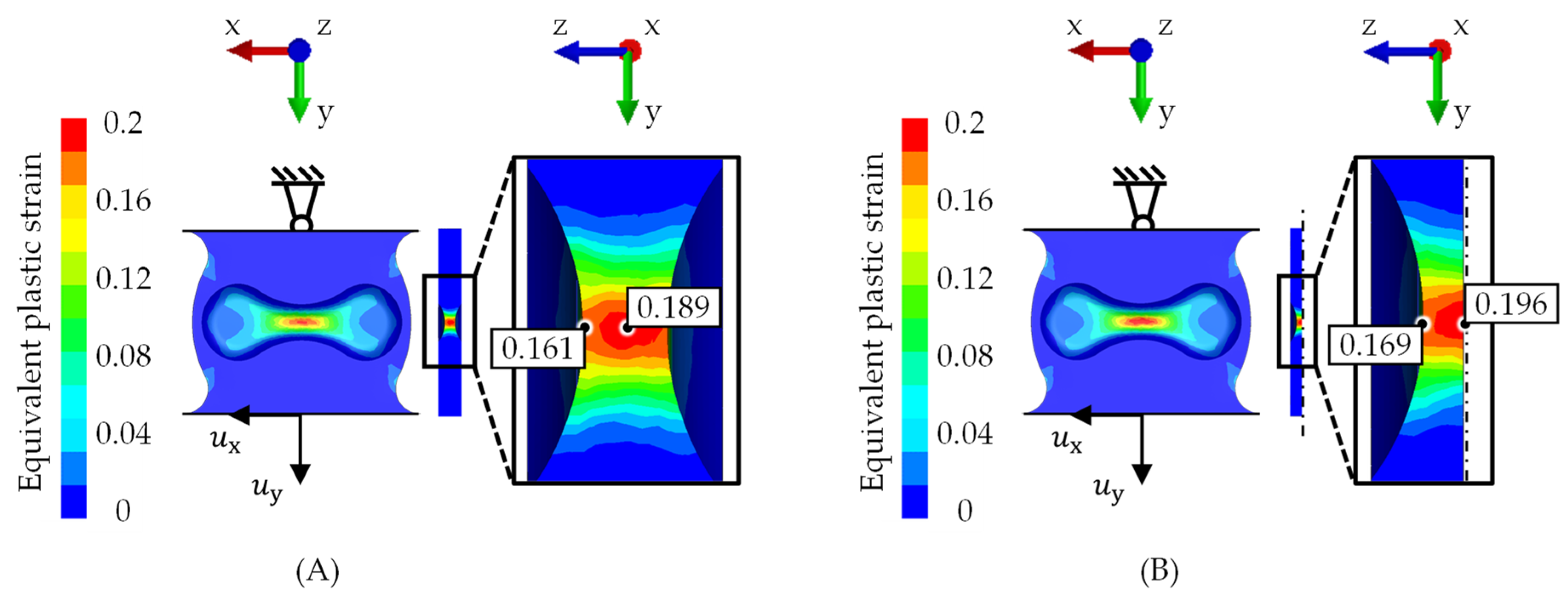

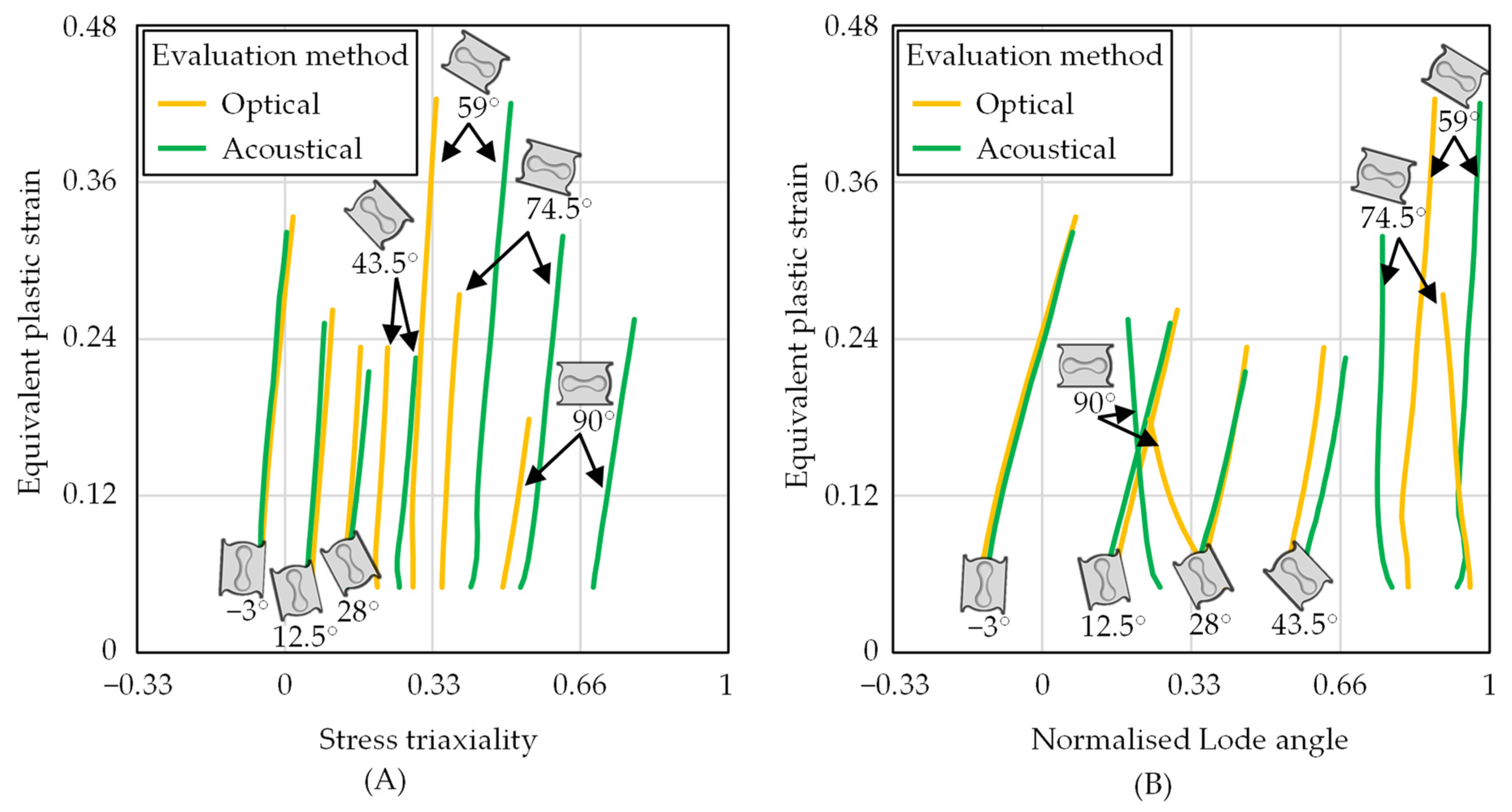


| Element | C | Si | Mn | P | S | Al | Cr + Mo | Ti + Nb | B | V | Fe |
|---|---|---|---|---|---|---|---|---|---|---|---|
| HCT980X | 0.139 | 0.186 | 1.562 | 0.021 | 0.003 | 0.241 | 0.844 | 0.053 | 0.0005 | 0.02 | Bal. |
| HCT780C | 0.102 | 0.206 | 1.847 | 0.022 | 0.002 | 0.197 | 0.784 | 0.0695 | 0.0004 | 0.08 | Bal. |
| Parameter | |||||||
| HCT980X | 747.3 | 1052.4 | 7.43 | 11.87 | 0.904 | 1.041 | 1.068 |
| HCT780C | 629.2 | 809.7 | 9.53 | 16.58 | 0.884 | 1.094 | 0.949 |
| Parameter | in MPa | in MPa | in MPa | in MPa | |||
| HCT980X | 757.3 | 742.2 | 743.6 | 1379 | 7.16 × 10−4 | 7.97 × 10−2 | |
| HCT780C | 631.4 | 622.7 | 605.8 | 1123 | 21.65 × 10−4 | 10.57 × 10−2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stockburger, E.; Wester, H.; Behrens, B.-A. Fracture Characterisation and Modelling of AHSS Using Acoustic Emission Analysis for Deep Drawing. J. Manuf. Mater. Process. 2023, 7, 127. https://doi.org/10.3390/jmmp7040127
Stockburger E, Wester H, Behrens B-A. Fracture Characterisation and Modelling of AHSS Using Acoustic Emission Analysis for Deep Drawing. Journal of Manufacturing and Materials Processing. 2023; 7(4):127. https://doi.org/10.3390/jmmp7040127
Chicago/Turabian StyleStockburger, Eugen, Hendrik Wester, and Bernd-Arno Behrens. 2023. "Fracture Characterisation and Modelling of AHSS Using Acoustic Emission Analysis for Deep Drawing" Journal of Manufacturing and Materials Processing 7, no. 4: 127. https://doi.org/10.3390/jmmp7040127
APA StyleStockburger, E., Wester, H., & Behrens, B.-A. (2023). Fracture Characterisation and Modelling of AHSS Using Acoustic Emission Analysis for Deep Drawing. Journal of Manufacturing and Materials Processing, 7(4), 127. https://doi.org/10.3390/jmmp7040127






