Fatigue Life and Residual Stress of Flat Stainless Steel Specimens Laser-Cladded with a Cobalt-Based Alloy and Postprocessed with Laser Shock Peening
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Sample Preparation
2.3. Laser Shock Peening
2.4. Residual Stress Estimation
2.5. Fatigue Crack Initiation Tests
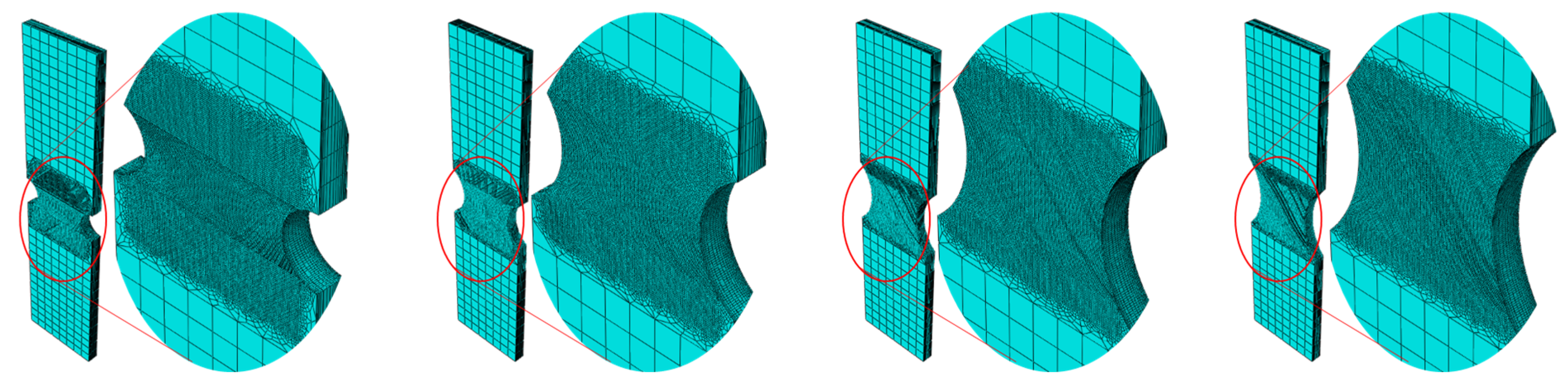
2.6. LSP Simulation
2.7. Fatigue Life Prediction
3. Results and Discussion
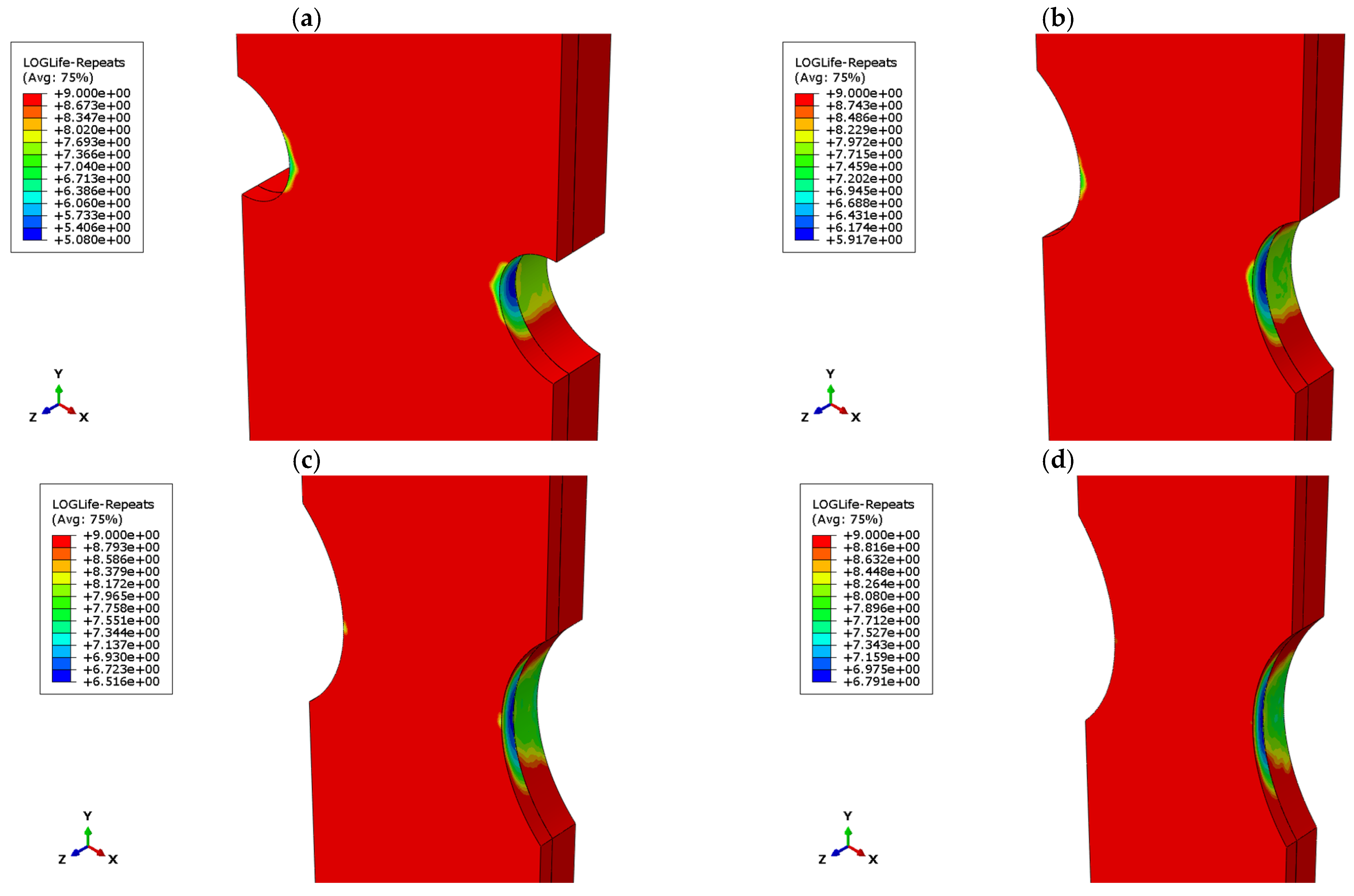
3.1. Fatigue Life
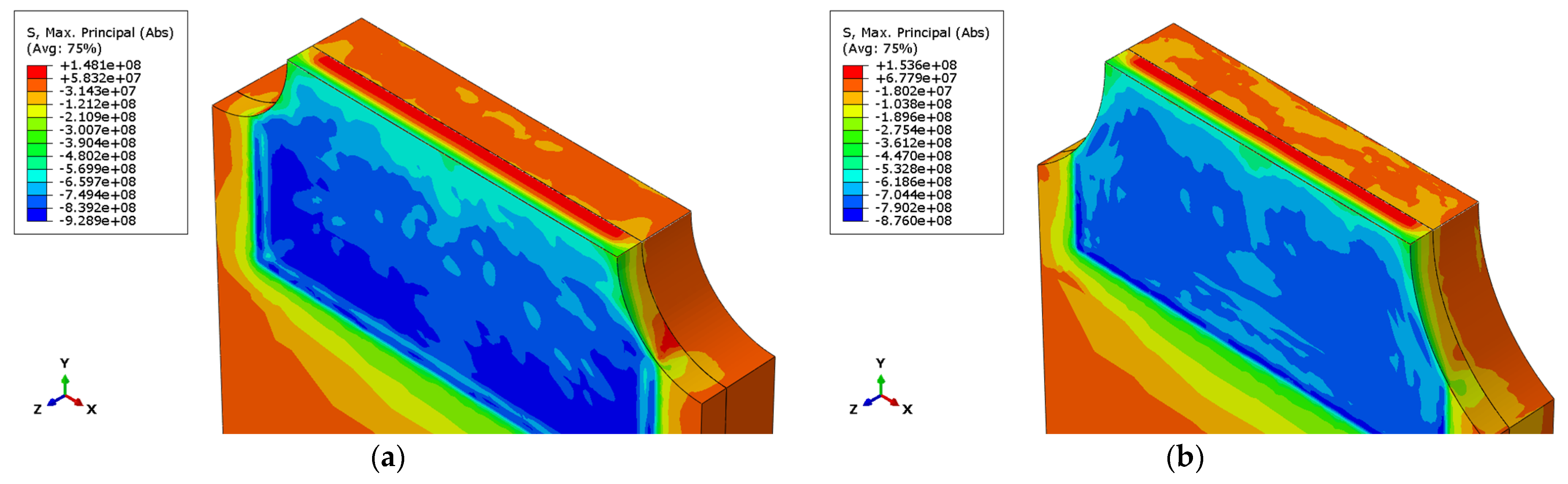
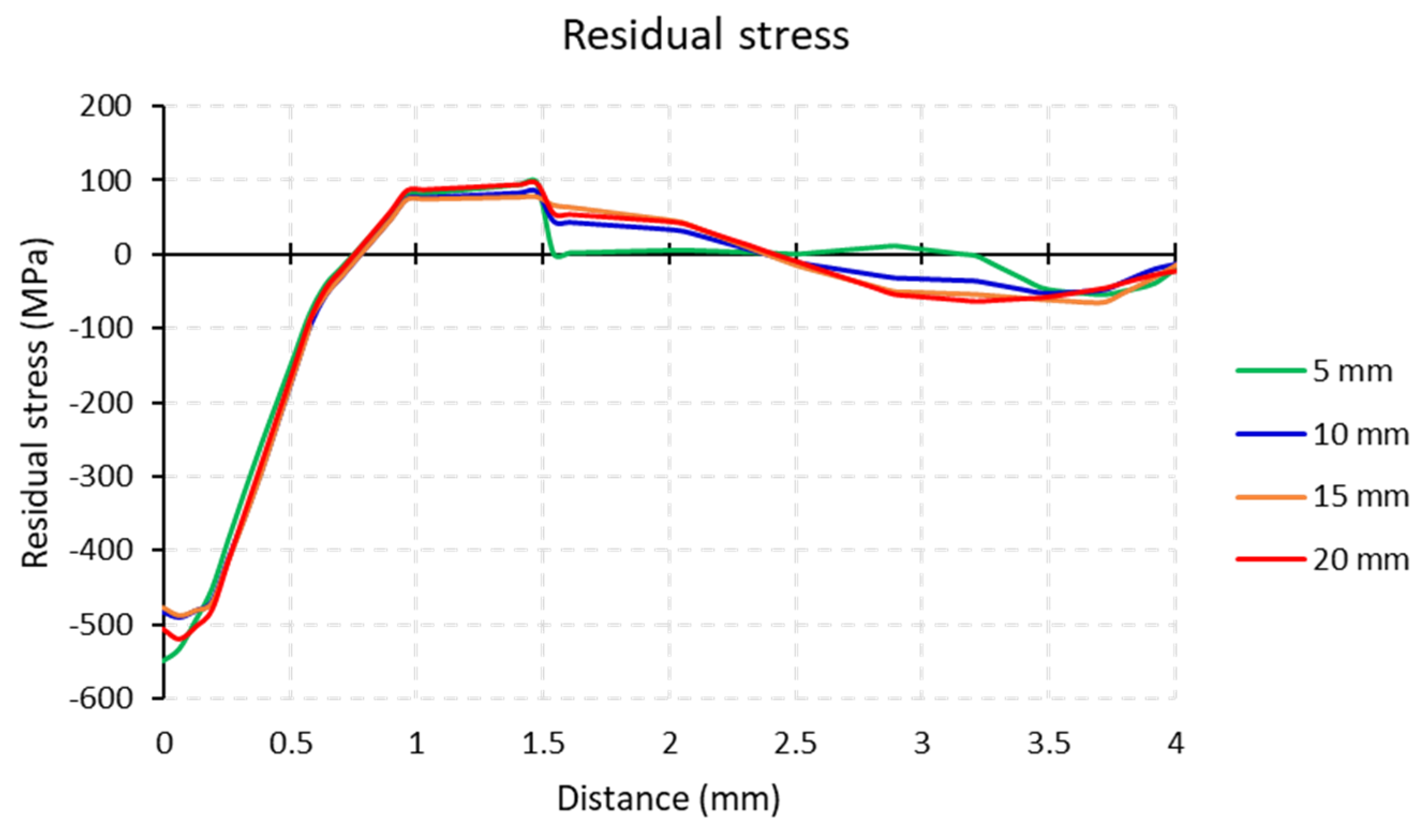
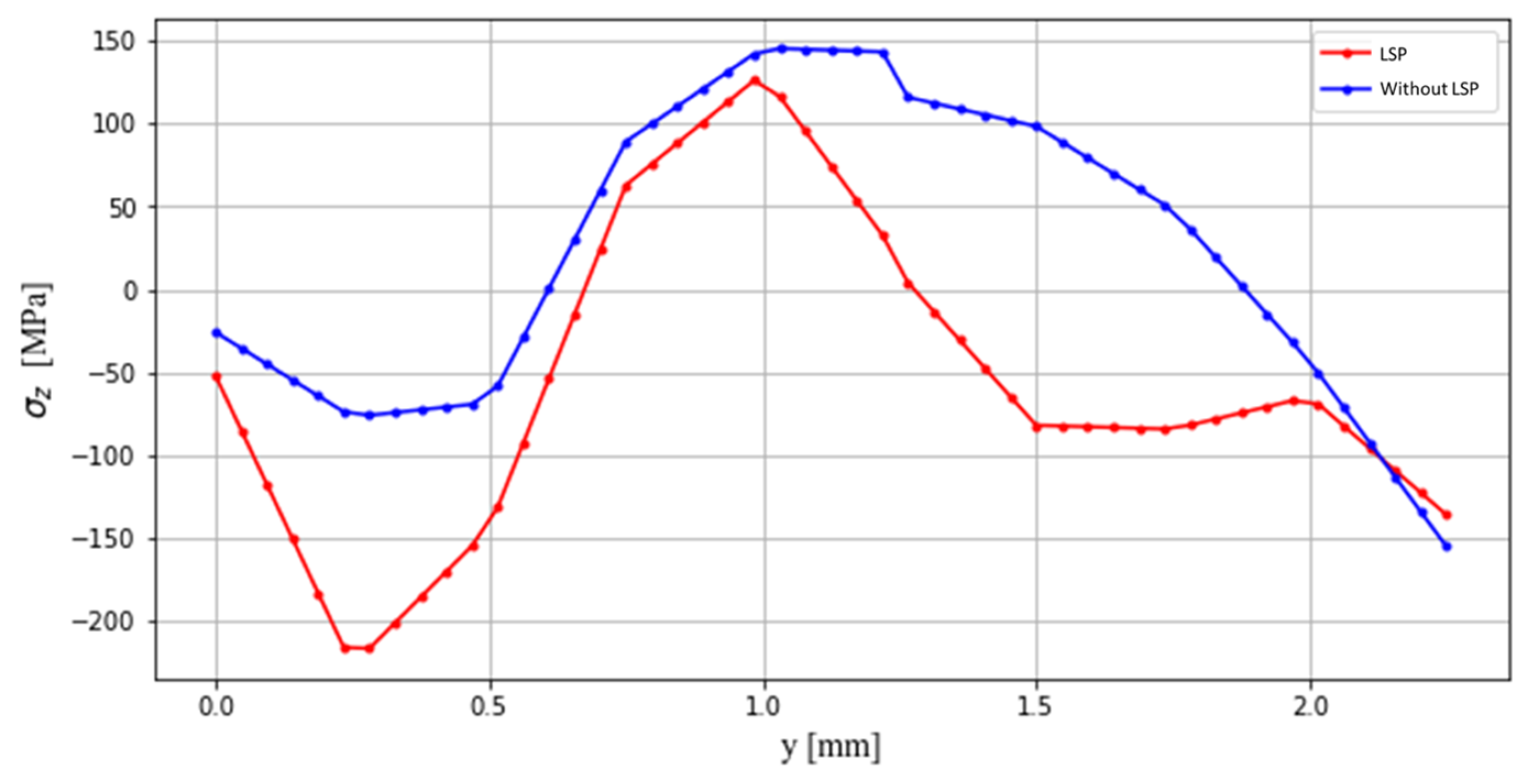
3.2. Residual Stresses Using the Contour Method
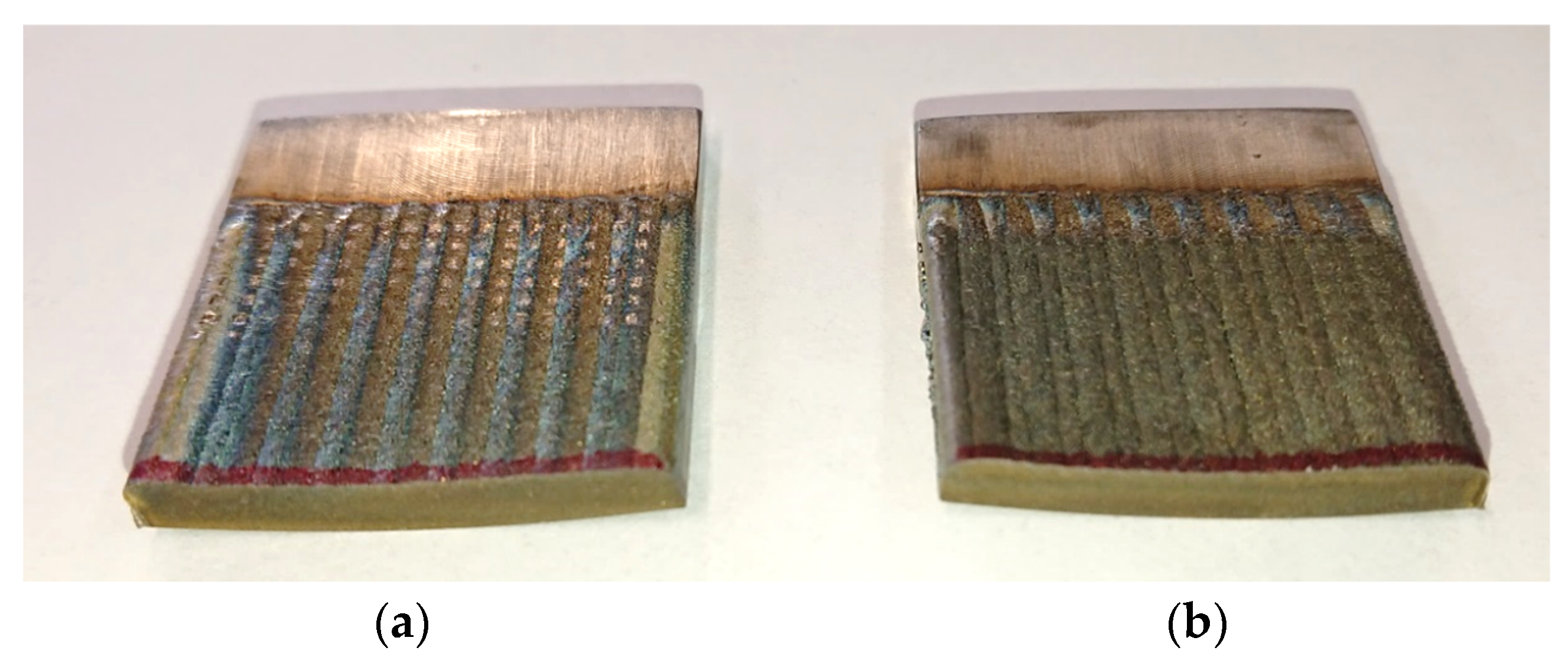
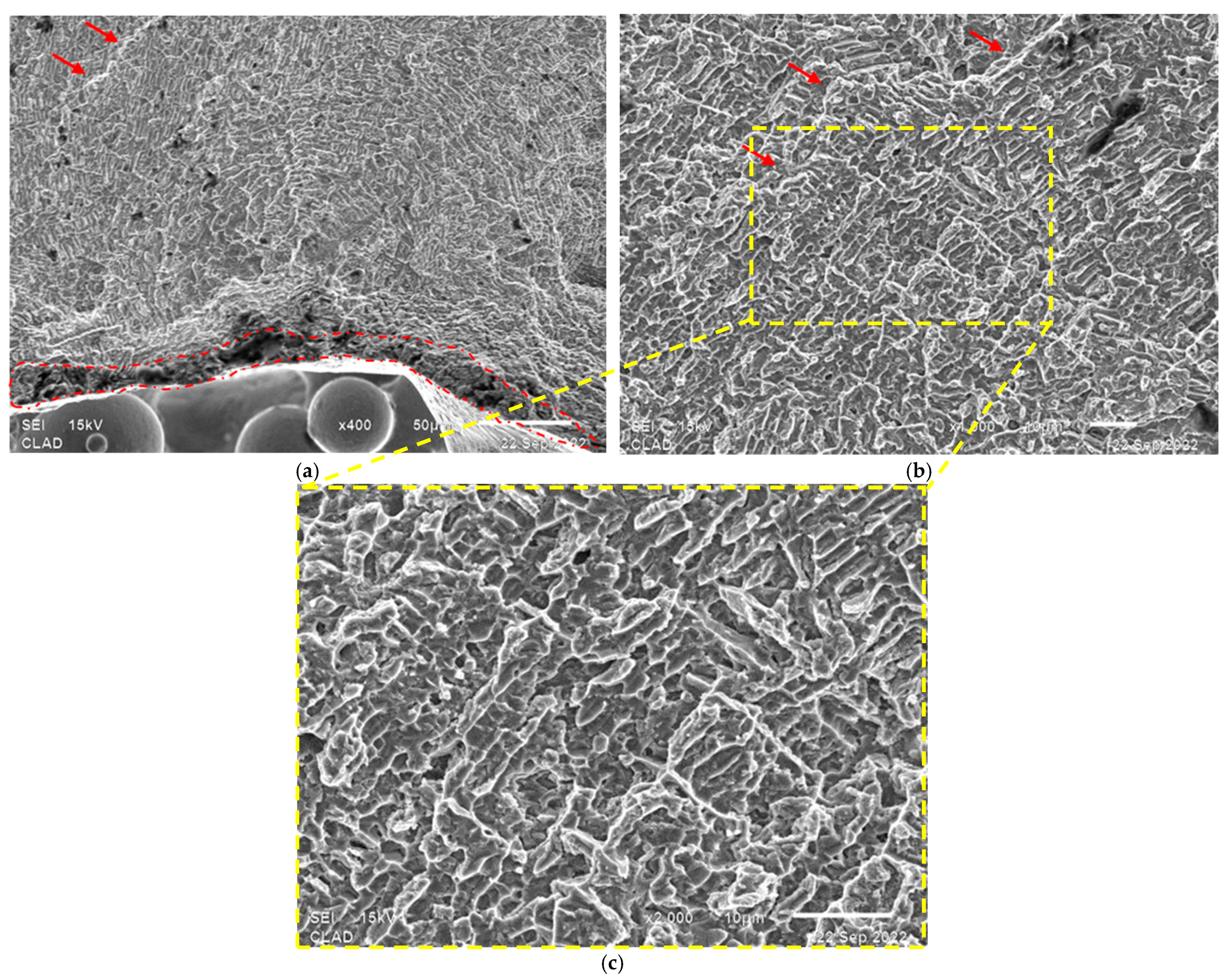
3.3. Fracture Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ding, K.; Ye, L. Laser Shock Peening: Performance and Process Simulation; Woodhead Publishing: Sawston, UK, 2006. [Google Scholar]
- Zhang, Y.; Luo, K.; Lu, J. Laser Shock Processing of FCC Metals; Springer: Berlin, Germany, 2013. [Google Scholar]
- Yadav, M.; Jinoop, A.; Danduk, C.; Subbu, S. Laser Shock Processing: Process Physics, Parameters, and Applications. Mater. Today Proc. 2017, 4, 7921–7930. [Google Scholar] [CrossRef]
- Correa, C.; Peral, D.; Porro, J.A.; Díaz, M.; de Lara, L.R.; García-Beltrán, A.; Ocaña, J.L. Random-type scanning patterns in laser shock peening without absorbing coating in 2024-T351 Al alloy: A solution to reduce residual stress anisotropy. Opt. Laser Technol. 2015, 73, 179–187. [Google Scholar] [CrossRef]
- Silvello, A.; Perrone, A. Laser Cladding of Metals; Cavaliere, P., Ed.; Springer: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Mazumder, J. Laser-aided direct metal deposition of metals and alloys. In Laser Additive Manufacturing; Woodhead Publishing: Sawston, UK, 2017; pp. 21–53. [Google Scholar]
- Lawrence, J.R.; Waugh, D. (Eds.) Laser Surface Engineering: Processes and Applications; Elsevier: Amsterdam, The Netherlands, 2014. [Google Scholar]
- Durejko, T.; Łazińska, M. Characterization of Cobalt-Based Stellite 6 Alloy Coating Fabricated by Laser-Engineered Net Shaping (LENS). Materials 2021, 14, 7442. [Google Scholar] [CrossRef] [PubMed]
- Peyre, P.; Fabbro, R.; Merrien, P.; Lieurade, H. Laser shock processing of aluminium alloys. Application to high cycle fatigue behaviour. Mater. Sci. Eng. A 1996, 210, 102–113. [Google Scholar]
- Xu, P.; Lin, C.; Zhou, C.; Yi, X. Wear and corrosion resistance of laser cladding AISI 304 stainless steel/Al2O3 composite coatings. Surf. Coat. Technol. 2014, 238, 9–14. [Google Scholar] [CrossRef]
- Hatamleh, O. Effects of peening on mechanical properties in friction stir welded 2195 aluminum alloy joints. Mater. Sci. Eng. A 2008, 492, 168–176. [Google Scholar] [CrossRef]
- Rubio-González, C.; Felix-Martinez, C.; Gomez-Rosas, G.; Ocaña, J.L.; Morales, M.; Porro, J.A. Effect of laser shock processing on fatigue crack growth of duplex stainless steel. Mater. Sci. Eng. A 2011, 528, 914–919. [Google Scholar] [CrossRef]
- Spadaro, L.; Gomez-Rosas, G.; Rubio-González, C.; Bolmaro, R.; Chavez-Chavez, A.; Hereñú, S. Fatigue behavior of superferritic stainless steel laser shock treated without protective coating. Opt. Laser Technol. 2017, 93, 208–215. [Google Scholar] [CrossRef]
- Granados-Alejo, V.; Rubio-González, C.; Vázquez-Jiménez, C.A.; Banderas, J.A.; Gómez-Rosas, G. Influence of specimen thickness on the fatigue behavior of notched steel plates subjected to laser shock peening. Opt. Laser Technol. 2018, 101, 531–544. [Google Scholar] [CrossRef]
- Vasu, A.; Grandhi, R.V. Effects of curved geometry on residual stress in laser peening. Surf. Coat. Technol. 2013, 218, 71–79. [Google Scholar] [CrossRef]
- Xu, G.; Lu, H.; Luo, K.; Dai, F.; Lu, J. Effects of surface curvature on residual stress field of 316L stainless steel subjected to laser shock peening. Opt. Laser Technol. 2021, 144, 107420. [Google Scholar] [CrossRef]
- Luo, S.; He, W.; Chen, K.; Nie, X.; Zhou, L.; Li, Y. Regain the fatigue strength of laser additive manufactured Ti alloy via laser shock peening. J. Alloys Compd. 2018, 750, 626–635. [Google Scholar] [CrossRef]
- Tong, Z.; Liu, H.; Jiao, J.; Zhou, W.; Yang, Y.; Ren, X. Improving the strength and ductility of laser directed energy deposited CrMnFeCoNi high-entropy alloy by laser shock peening. Addit. Manuf. 2020, 35, 101417. [Google Scholar] [CrossRef]
- Ge, M.Z.; Tang, Y.; Zhang, Y.K.; Wang, Y. Enhancement in fatigue property of Ti-6Al-4V alloy remanufactured by combined laser cladding and laser shock peening processes. Surf. Coat. Technol. 2022, 444, 128671. [Google Scholar] [CrossRef]
- Lu, H.; Wu, L.; Wei, H.; Cai, J.; Luo, K.; Xu, X.; Lu, J. Microstructural evolution and tensile property enhancement of remanufactured Ti6Al4V using hybrid manufacturing of laser directed energy deposition with laser shock peening. Addit. Manuf. 2022, 55, 102877. [Google Scholar] [CrossRef]
- Lu, H.F.; Xue, K.N.; Xu, X.; Luo, K.Y.; Xing, F.; Yao, J.H.; Lu, J.Z. Effects of laser shock peening on microstructural evolution and wear property of laser hybrid remanufactured Ni25/Fe104 coating on H13 tool steel. J. Mater. Process. Technol. 2021, 291, 117016. [Google Scholar] [CrossRef]
- Wang, Q.; Shi, J.; Zhang, L.; Tsutsumi, S.; Feng, J.; Ma, N. Impacts of laser cladding residual stress and material properties of functionally graded layers on titanium alloy sheet. Addit. Manuf. 2020, 35, 101303. [Google Scholar] [CrossRef]
- Nguyen, T.P.; Tanabe-Yamagishi, R.; Ito, Y. Impact of liquid layer thickness on the dynamics of nano-to sub-microsecond phenomena of nanosecond pulsed laser ablation in liquid. Appl. Surf. Sci. 2019, 470, 250–258. [Google Scholar] [CrossRef]
- Rubio-González, C.; Gomez-Rosas, G.; Ocaña, J.L.; Molpeceres, C.; Banderas, A.; Porro, J.; Morales, M. Effect of an absorbent overlay on the residual stress field induced by laser shock processing on aluminum samples. Appl. Surf. Sci. 2006, 252, 6201–6205. [Google Scholar] [CrossRef]
- Prime, M.B. Cross-sectional mapping of residual stresses by measuring the surface contour after a cut. J. Eng. Mater. Technol. 2001, 123, 162–168. [Google Scholar] [CrossRef]
- Kim, R.; Suh, J.; Shin, D.; Lee, K.-H.; Bae, S.-H.; Cho, D.-W.; Yi, W.-G. FE Analysis of Laser Shock Peening on STS304 and the Effect of Static Damping on the Solution. Metals 2021, 11, 1516. [Google Scholar] [CrossRef]
- Jalali, A.M.; Tolouei-Rad, M. Evaluation of residual stress in HVOF stellite-6 coatings using non-contact drilling. Mater. Res. Express 2019, 6, 066577. [Google Scholar] [CrossRef]
- Ivetic, G.; Meneghin, I.; Troiani, E.; Molinari, G.; Ocaña, J.L.; Morales, M.; Porro, J. Fatigue in laser shock peened open-hole thin aluminium specimens. Mater. Sci. Eng. A 2012, 534, 573–579. [Google Scholar] [CrossRef]
- Ren, C.X.; Wang, Q.; Zhang, Z.J.; Yang, H.J.; Zhang, Z.F. Enhanced tensile and bending yield strengths of 304 stainless steel and H62 brass by surface spinning strengthening. Mater. Sci. Eng. A 2019, 754, 593–601. [Google Scholar] [CrossRef]
- Roy, T.; Abrahams, R.; Paradowska, A.; Lai, Q.; Mutton, P.; Soodi, M.; Fasihi, P.; Yan, W. Evaluation of the mechanical properties of laser cladded hypereutectoid steel rails. Wear 2019, 432, 202930. [Google Scholar] [CrossRef]
- Peyre, P.; Chaieb, I.; Braham, C. FEM calculation of residual stresses induced by laser shock processing in stainless steels. Model. Simul. Mater. Sci. Eng. 2007, 15, 205. [Google Scholar]
- Granados-Alejo, V.; Rubio-González, C.; Banderas, J.A.; Flores, S. The Laser Shock Peening Effect on Fatigue Performance of Curved Components with and Without Stress Concentrators. J. Mater. Eng. Perform. 2022, 31, 1046–1057. [Google Scholar] [CrossRef]
- Fatigue Theory Reference Manual, Fe-safe Documentation. 2019. Available online: https://help.3ds.com/2019/English/DSSIMULIA_Established/FesafeFatigueTheoryPdf/FesafeFatigueTheory.pdf (accessed on 1 January 2024).
- Marquis, G.B.; Socie, D.F. Multiaxial Fatigue; SAE International: Valendale, PA, USA, 2003; pp. 221–252. [Google Scholar]
- Kim, K.S.; Chen, X.; Han, C.; Lee, H.W. Estimation methods for fatigue properties of steels under axial and torsional loading. Int. J. Fatigue 2002, 24, 783–793. [Google Scholar] [CrossRef]
- Troshchenko, V.T.; Khamaza, L.A. Strain–life curves of steels and methods for determining the curve parameters. Part 1. Conventional methods. Strength Mater. 2010, 42, 647–659. [Google Scholar] [CrossRef]
- Lai, Y.; Yue, X.; Yue, W. A Study on the Residual Stress of the Co-Based Alloy Plasma Cladding Layer. Materials 2022, 15, 5143. [Google Scholar] [CrossRef] [PubMed]
- Vázquez Jiménez, C.A.; Granados Alejo, V.; Rubio-González, C.; Gomez Rosas, G.; Llamas Zamorano, S. Fatigue life behavior of laser shock peened duplex stainless steel with different samples geometry. Adv. Mater. Sci. Eng. 2019, 2019, 8053248. [Google Scholar] [CrossRef]
- Barr, C.; Rashid, R.A.R.; Sun, S.D.; Easton, M.; Palanisamy, S.; Orchowski, N.; Matthews, N.; Walker, K.; Brandt, M. Role of deposition strategy and fill depth on the tensile and fatigue performance of 300 M repaired through laser directed energy deposition. Int. J. Fatigue 2021, 146, 106135. [Google Scholar] [CrossRef]







| Element | Cr | W | Ni | Si | C | Mo | Co |
|---|---|---|---|---|---|---|---|
| (wt%) | 28 | 4 | 3 | 1.5 | 1 | 1 | balance |
| Material | A (MPa) | B (MPa) | C | n | m | |
|---|---|---|---|---|---|---|
| SS 304 | 336 | 1000 | 0.07 | 0.65 | 1 | 1 |
| Stellite 6 | 925 | 1800 | 0.01 | 0.57 | 0.1 | 1 |
| Time (ns) | 0 | 3 | 5 | 7 | 9 | 12 | 15 | 19 | 26 | 33 | 59 | 80 | 120 | 178 | 200 |
| Pressure (GPa) | 0 | 0.63 | 1.19 | 2.46 | 4.06 | 4.38 | 4.30 | 3.58 | 2.31 | 1.75 | 1.11 | 0.87 | 0.47 | 0.15 | 0 |
| Parameter | Uniform Material Law: Stellite 6 | Uniform Material Law: SS 304 |
|---|---|---|
| 1953 | 1116 | |
| 0.36254 | 0.52246 | |
| b | −0.087 | −0.087 |
| c | −0.58 | −0.58 |
| Notch Radius (mm) | Without LSP | With LSP | Improvement (%) |
|---|---|---|---|
| 5 | 82,212 | 119,428 | 45.2 |
| 10 | 551,829 | 820,470 | 48.6 |
| 15 | 2,218,371 | 3,254,561 | 46.7 |
| 20 | 4,389,208 | 6,100,181 | 38.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Flores-García, S.; Martínez-Pérez, C.E.; Rubio-González, C.; Banderas-Hernández, J.A.; Félix-Martínez, C.; Jiménez, S.M.A. Fatigue Life and Residual Stress of Flat Stainless Steel Specimens Laser-Cladded with a Cobalt-Based Alloy and Postprocessed with Laser Shock Peening. J. Manuf. Mater. Process. 2024, 8, 45. https://doi.org/10.3390/jmmp8020045
Flores-García S, Martínez-Pérez CE, Rubio-González C, Banderas-Hernández JA, Félix-Martínez C, Jiménez SMA. Fatigue Life and Residual Stress of Flat Stainless Steel Specimens Laser-Cladded with a Cobalt-Based Alloy and Postprocessed with Laser Shock Peening. Journal of Manufacturing and Materials Processing. 2024; 8(2):45. https://doi.org/10.3390/jmmp8020045
Chicago/Turabian StyleFlores-García, Santiago, Carlos E. Martínez-Pérez, Carlos Rubio-González, J. Antonio Banderas-Hernández, Christian Félix-Martínez, and Salomón M. A. Jiménez. 2024. "Fatigue Life and Residual Stress of Flat Stainless Steel Specimens Laser-Cladded with a Cobalt-Based Alloy and Postprocessed with Laser Shock Peening" Journal of Manufacturing and Materials Processing 8, no. 2: 45. https://doi.org/10.3390/jmmp8020045
APA StyleFlores-García, S., Martínez-Pérez, C. E., Rubio-González, C., Banderas-Hernández, J. A., Félix-Martínez, C., & Jiménez, S. M. A. (2024). Fatigue Life and Residual Stress of Flat Stainless Steel Specimens Laser-Cladded with a Cobalt-Based Alloy and Postprocessed with Laser Shock Peening. Journal of Manufacturing and Materials Processing, 8(2), 45. https://doi.org/10.3390/jmmp8020045






