FRP Stay-in-Place Formworks for High Performance of Concrete Slabs
Abstract
1. Introduction
2. Materials and Methods
2.1. Test Specimens and Parameters
2.2. Materials
2.3. Preparation of Specimens
2.4. Experimental Setup
3. Results
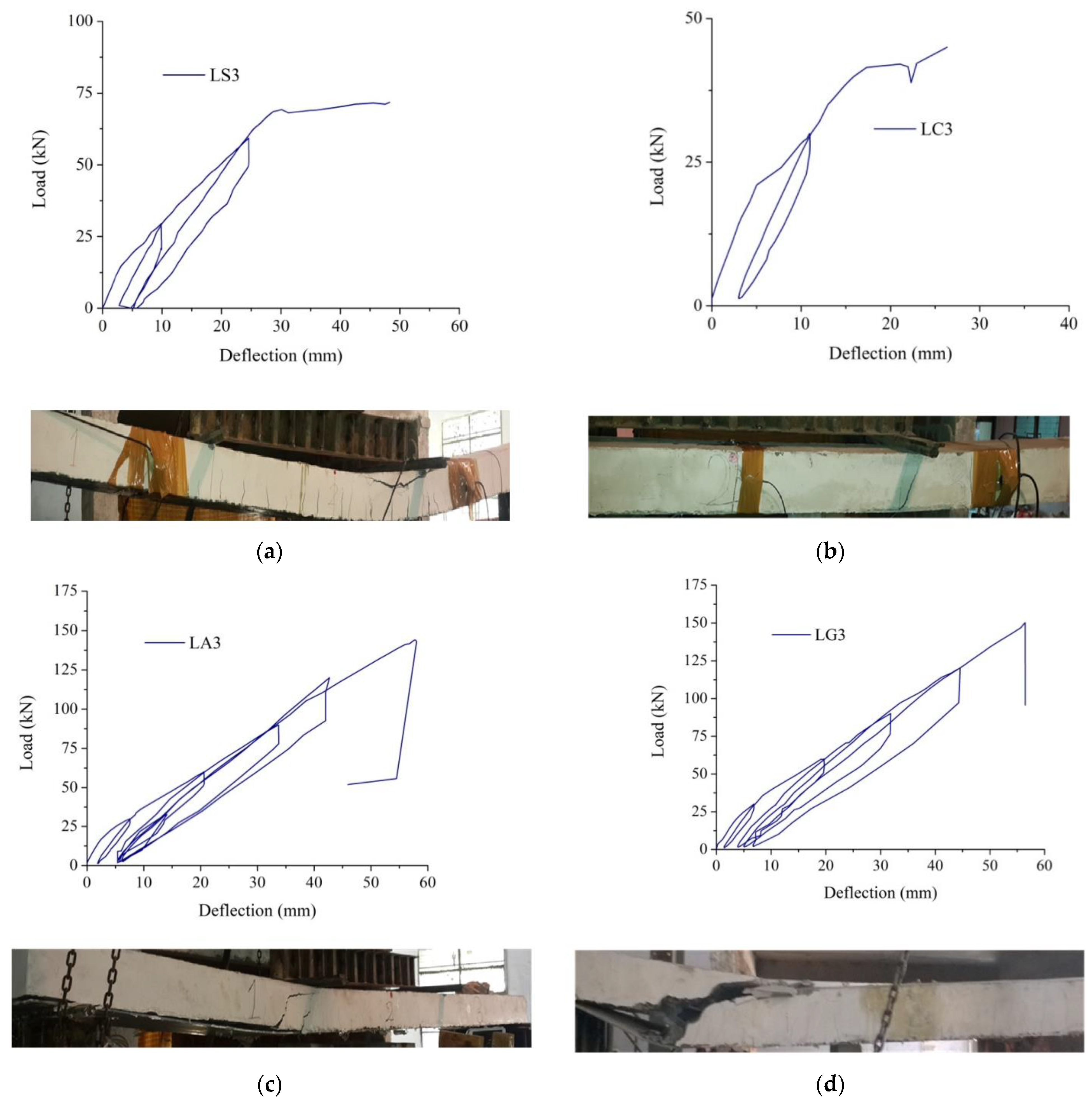
3.1. Load-Deflection Plot
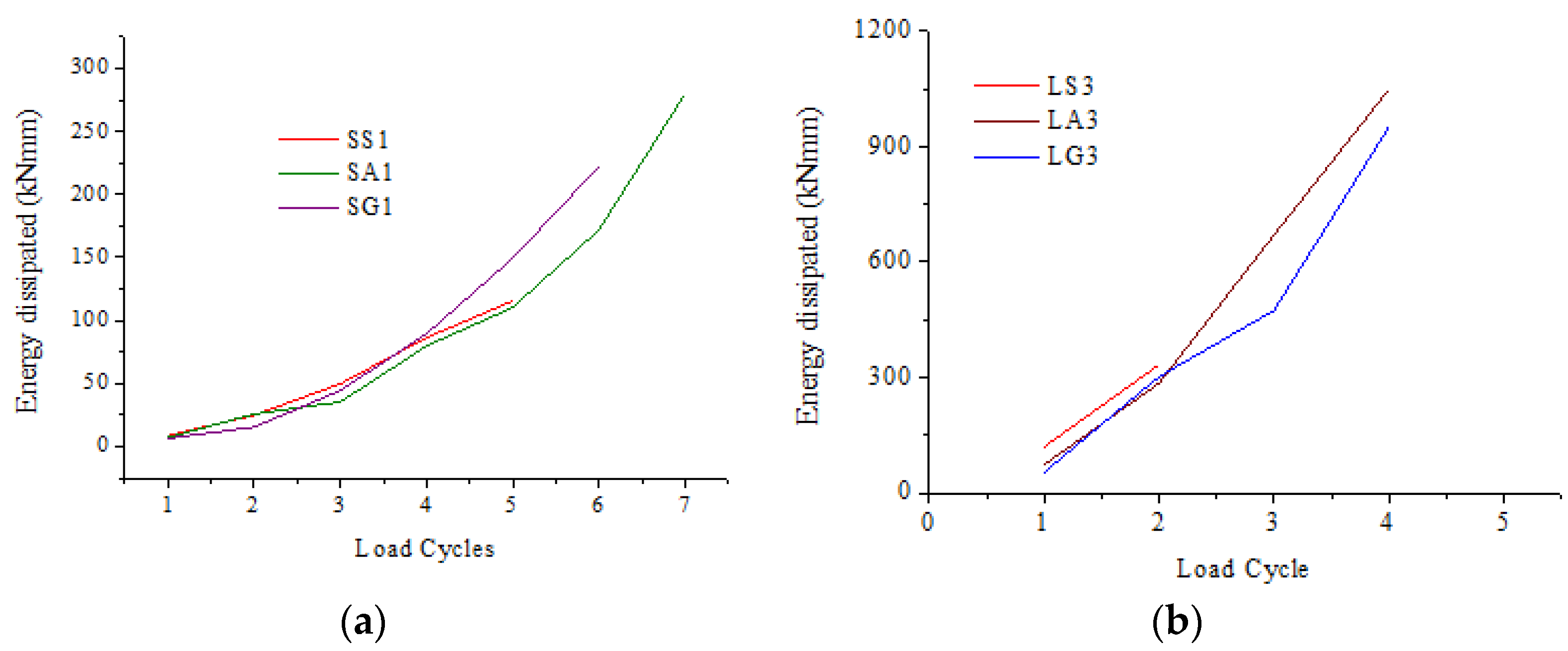
3.2. Hysteric Properties
- Ei = Energy absorbed in the ith cycle of loading
- di1,2,3 = Displacements at the beginning, peak load, and end of ith cycle
- F = Applied force
4. Design Methodology
5. Concluding Remarks
- The present FRP-SiP formwork could serve its dual intended purpose of formwork and tensile reinforcement. The FRP-SiP considerably reduced the number of work steps and eased the construction of the slabs.
- Bond treatment at the FRP–concrete interface is a critical factor in this type of construction. No gain compared to the standard steel bar reinforced slabs was observed in the case when the interface was left untreated. However, bond-treated FRP-SiP surpassed the performance of the standard slab by a considerable margin.
- The performances of the adhesive bonding and aggregate bonding were close to one another. From the point of view of ease of construction, wet adhesive bonding is convenient. However, the application of the adhesive on the FRP must take place within the pot life of the adhesive.
- All of the short slabs (S series) exhibited the typical shear failure. A diagonal crack was observed within one of the shear spans leading to failure. However, in the case of FRP-SiP, the direction of the crack diverted to the concrete–FRP interface, and the FRP ripped off from the concrete. FRP-SiP had 40% more load capacity than the conventional steel reinforced slab.
- In the case of the long slabs (L series), the steel reinforced slab failed by yielding steel reinforcements, followed by concrete crushing. The failure mode in the bond-treated FRP-SiP remained the same for the S and the L series, with diagonal cracking followed by the ripping off of the FRP from concrete. FRP-SiP had a 107% greater load capacity than the conventional steel reinforced slab.
- A thin layer of concrete was attached to the FRP along the failure plane. This indicates that the failure was in the concrete.
- The hysteretic properties of the slabs were estimated from the cyclic load tests. Bond-treated FRP-SiP showed superior energy dissipation capacity than even the steel bar-reinforced specimens.
- The specimens were analysed using both the ACI Committee 440 recommended flexure and shear design provisions. As ACI 440 considers the shear capacity of the cracked concrete only, the shear capacity was also calculated based on ACI 318 that including the contribution from the FRP. The load capacities obtained experimentally were compared with those from the codes. It was observed that the experimental capacities were considerably higher than those of the codes.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bakis, C.; Bank, L.; Brown, V.; Cosenza, E.; Davalos, J.; Lesko, J.; Machida, A.; Rizkalla, S.; Triantafillou, T. Fiber-Reinforced Polymer Composites for Construction—State-of-the-Art Review. J. Compos. Constr. 2002, 6, 73–87. [Google Scholar] [CrossRef]
- Djeddi, F.; Ghernouti, Y.; Abdelaziz, Y.; Alex, L. Strengthening in flexure–shear of RC beams with hybrid FRP systems: Experiments and numerical modeling. J. Reinf. Plast. Compos. 2016, 35, 1642–1660. [Google Scholar] [CrossRef]
- Zaman, A.; Gutub, S.A.; Wafa, M.A. A review on FRP composites applications and durability concerns in the construction sector. J. Reinf. Plast. Compos. 2013, 32, 1966–1988. [Google Scholar] [CrossRef]
- Hall, J.; Mottram, J. Combined FRP Reinforcement and Permanent Formwork for Concrete Members. J. Compos. Constr. 1998, 2, 78–86. [Google Scholar] [CrossRef]
- Alagusundaramoorthy, P.; Harik, I.E.; Choo, C.C. Structural Behavior of FRP Composite Bridge Deck Panels. J. Bridge Eng. 2006, 11, 384–393. [Google Scholar] [CrossRef]
- Honickman, H.; Fam, A. Investigating a Structural Form System for Concrete Girders Using Commercially Available GFRP Sheet-Pile Sections. J. Compos. Constr. 2009, 13, 455–465. [Google Scholar] [CrossRef]
- Fam, A.; Boles, R.; Robert, M. Durability in a Salt Solution of Pultruded Composite Materials Used in Structural Sections for Bridge Deck Applications. J. Bridge Eng. 2015, 21, 04015032. [Google Scholar] [CrossRef]
- Boles, R.; Nelson, M.; Fam, A. Durability of Bridge Deck with FRP Stay-in-Place Structural Forms under Freeze-Thaw Cycles. J. Compos. Constr. 2014, 19, 04014070. [Google Scholar] [CrossRef]
- Nelson, M.; Fam, A.; Busel, J.; Bakis, C.; Nanni, A.; Bank, L.; Henderson, M.; Hanus, J. FRP Stay-In-Place Structural Forms for Concrete Bridge Decks: A State-Of-The-Art Review. ACI Struct. J. 2014, 111, 1069–1080. [Google Scholar] [CrossRef]
- Cheng, L.; Zhao, L.; Karbhari, V.; Hegemier, G.; Seible, F. Assessment of a Steel-Free Fiber Reinforced Polymer-Composite Modular Bridge System. J. Struct. Eng. 2005, 131, 498–506. [Google Scholar] [CrossRef]
- Bank, L.; Oliva, M.; Bae, H.-U.; Barker, J.; Yoo, S.-W. Pultruded FRP Plank as Formwork and Reinforcement for Concrete Members. Adv. Struct. Eng. 2007, 10, 525–535. [Google Scholar] [CrossRef]
- Keller, T.; Schaumann, E.; Vallée, T. Flexural behavior of a Hybrid FRP and Lightweight Concrete Sandwich Bridge Deck. Compos. Part A Appl. Sci. Manuf. 2007, 38, 879–889. [Google Scholar] [CrossRef]
- Nelson, M.; Fam, A. Structural GFRP Permanent Forms with T-Shape Ribs for Bridge Decks Supported by Precast Concrete Girders. J. Bridge Eng. 2013, 18, 813–826. [Google Scholar] [CrossRef]
- Dieter, D.; Dietsche, J.; Bank, L.; Oliva, M.; Russell, J. Concrete Bridge Decks Constructed with Fiber-Reinforced Polymer Stay-in-Place Forms and Grid Reinforcing. Transp. Res. Rec. J. Transp. Res. Board 2002, 1814, 219–226. [Google Scholar] [CrossRef]
- Bank, L.C.; Oliva, M.G.; Bae, H.-U.; Bindrich, B.V. Hybrid Concrete and Pultruded-Plank Slabs for Highway and Pedestrian Bridges. Constr. Build. Mater. 2010, 24, 552–558. [Google Scholar] [CrossRef]
- Ringelstetter, T.; Bank, L.; Oliva, M.; Russell, J.; Matta, F.; Nanni, A. Cost-Effective, Structural Stay-in-Place Formwork System of Fiber—Reinforced Polymer for Accelerated and Durable Bridge Deck Construction. Transp. Res. Rec. J. Transp. Res. Board 2006, 1976, 183–189. [Google Scholar] [CrossRef]
- Matta, F.; Nanni, A.; Ringelstetter, T.E.; Bank, L. Rapid construction of concrete bridge deck using prefabricated FRP reinforcement. In Proceedings of the CICE 2006, Third International Conference on FRP Composites in Civil Engineering, Miami, FL, USA, 13–15 December 2006; pp. 151–154. Available online: https://www.scinapse.io/papers/80772422 (accessed on 4 September 2022).
- Fam, A.; Nelson, M. New Bridge Deck Cast onto Corrugated GFRP Stay-in-Place Structural Forms with Interlocking Connections. J. Compos. Constr. 2012, 16, 110–117. [Google Scholar] [CrossRef]
- Nelson, M.; Eldridge, A.; Fam, A. The effects of splices and bond on performance of bridge deck with FRP stay-in-place forms at various boundary conditions. Eng. Struct. 2013, 56, 509–516. [Google Scholar] [CrossRef]
- Nelson, M.; Beriker, E.; Fam, A. Splices of FRP Stay-in-Place Structural Forms in Concrete Bridge Decks. J. Compos. Constr. 2014, 18, 04014001–04014009. [Google Scholar] [CrossRef]
- Jawdhari, A.; Fam, A. Numerical Study on Mechanical and Adhesive Splices for Ribbed GFRP Plates used in Concrete Beams. Eng. Struct. 2018, 174, 478–494. [Google Scholar] [CrossRef]
- He, J.; Liu, Y.; Chen, A.; Dai, L. Experimental Investigation of Movable Hybrid GFRP and Concrete Bridge Deck. Constr. Build. Mater. 2012, 26, 49–64. [Google Scholar] [CrossRef]
- Cho, J.R.; Cho, K.; Park, S.Y.; Kim, S.T.; Kim, B.-S. Bond Characteristics of Coarse Sand Coated Interface between Stay-in-Place Fibre-Reinforced Polymer Formwork and Concrete based on Shear and Tension Tests. Can. J. Civ. Eng. 2010, 37, 706–718. [Google Scholar] [CrossRef]
- Goyal, R.; Mukherjee, A.; Goyal, S. An Investigation on Bond between FRP Stay-in-Place Formwork and Concrete. Constr. Build. Mater. 2016, 113, 741–751. [Google Scholar] [CrossRef]
- Goyal, R.; Goyal, S.; Mukherjee, A. Pultruded Fibre Reinforced Polymer Planks as Stay-in-Place Formwork for Concrete Structures. Curr. Sci. 2017, 113, 245–252. Available online: https://www.currentscience.ac.in/Volumes/113/02/0245.pdf (accessed on 4 September 2022). [CrossRef]
- Goyal, R.; Mukherjee, A.; Goyal, S. Bond between FRP Formwork and Concrete- Effect of Surface Treatment and Adhesives. Steel Compos. Struct.-Int. J. 2016, 20, 671–692. [Google Scholar] [CrossRef]
- Gai, X.; Darby, A.; Ibell, T.; Evernden, M. Experimental Investigation into a Ductile FRP Stay-in-Place Formwork System for Concrete Slabs. Constr. Build. Mater. 2013, 49, 1013–1023. [Google Scholar] [CrossRef]
- Cheng, L. Flexural Fatigue Analysis of a CFRP Form Reinforced Concrete Bridge Deck. Compos. Struct. 2011, 93, 2895–2902. [Google Scholar] [CrossRef]
- Richardson, P.; Nelson, M.; Fam, A. Fatigue behavior of concrete bridge decks cast on GFRP stay-in-place structural forms. J. Compos. Constr. 2013, 18, A4013010. [Google Scholar] [CrossRef]
- Nelson, M.; Fam, A. Modeling of Flexural Behavior and Punching Shear of Concrete Bridge Decks with FRP Stay-in-Place Forms Using the Theory of Plates. J. Eng. Mech. 2014, 140, 04014095. [Google Scholar] [CrossRef]
- Noël, M.; Fam, A. Design Equations for Concrete Bridge Decks with FRP Stay-in-Place Structural Forms. J. Compos. Constr. 2016, 20, 875–890. [Google Scholar] [CrossRef]
- ACI Committee 440. Guide for the Design and Construction of Structural Concrete Reinforced with Fiber-Reinforced Polymer (FRP) Bars, ACI 440. 1R-15; American Concrete Institute: Farmington Hills, MI, USA, 2015; Available online: https://www.concrete.org/Portals/0/Files/PDF/Previews/440_1R_15.pdf (accessed on 4 September 2022).
- Hanus, J.P.; Bank, L.C.; Oliva, M.G. Combined loading of a bridge deck reinforced with a structural FRP stay-in-place form. Constr. Build. Mater. 2009, 23, 1605–1619. [Google Scholar] [CrossRef]
- Hanus, J.; Bank, L.; Oliva, M. Investigation of a Structural FRP Stay-in-Place Form for a Prototype Military Bridge System. Spec. Publ. 2008, 257, 53–70. Available online: https://www.concrete.org/publications/internationalconcreteabstractsportal/m/details/id/20240 (accessed on 4 September 2022).
- Tureyen, A.K.; Robert, J.F. Shear Tests of FRP-Reinforced Concrete Beams without Stirrups. ACI Struct. J. 2002, 99, 427–434. [Google Scholar] [CrossRef]
- Mwafy, A.; Elnashai, A. Importance of shear assessment of concrete structures detailed to different capacity design requirements. Eng. Struct. 2008, 30, 1590–1604. [Google Scholar] [CrossRef]
- Kara, I.F. Prediction of Shear Strength of FRP-Reinforced Concrete Beams without Stirrups based on Genetic Programming. Adv. Eng. Softw. 2011, 42, 295–304. [Google Scholar] [CrossRef]
- Matta, F.; Mazzoleni, P.; Zappa, E.; Sutton, M.A.; ElBatanouny, M.; Larosche, A.K.; Paul, H.Z. Shear Strength of FRP Reinforced Concrete Beams without Stirrups: Verification of Fracture Mechanics Formulation. In ACI Special Publication Spring Convention; American Concrete Institute: Farmington Hills, MI, USA, 2012. [Google Scholar] [CrossRef]
- Matta, F.; El-Sayed, A.K.; Nanni, A.; Brahim, B. Size Effect on Concrete Shear Strength in Beams Reinforced with Fiber-Reinforced Polymer Bars. ACI Struct. J. 2013, 110, 616–628. [Google Scholar] [CrossRef]
- Nasrollahzadeh, K.; Basiri, M.M. Prediction of shear strength of FRP reinforced concrete beams using fuzzy inference system. Expert Syst. Appl. 2014, 41, 1006–1020. [Google Scholar] [CrossRef]
- Said, M.; Adam, M.A.; Mahmoud, A.A.; Shanour, A.S. Experimental and analytical shear evaluation of concrete beams reinforced with glass fiber reinforced polymers bars. Constr. Build. Mater. 2016, 102 Pt 1, 574–591. [Google Scholar] [CrossRef]
- ACI Committee 318. Building Code Requirements for Structural Concrete (ACI 318-14) and Commentary; American Concrete Institute: Farmington Hills, MI, USA, 2014; Available online: https://www.concrete.org/Portals/0/Files/PDF/Previews/318-14_preview1.pdf (accessed on 4 September 2022).
- Ringelstetter, T.E. Investigation of Modular FRP Grid Reinforcing Systems with Integral Stay-In-Place Form for Concrete Bridge Decks. Master’s Thesis, University of Wisconsin-Madison, Madison, WI, USA, 2006. [Google Scholar]

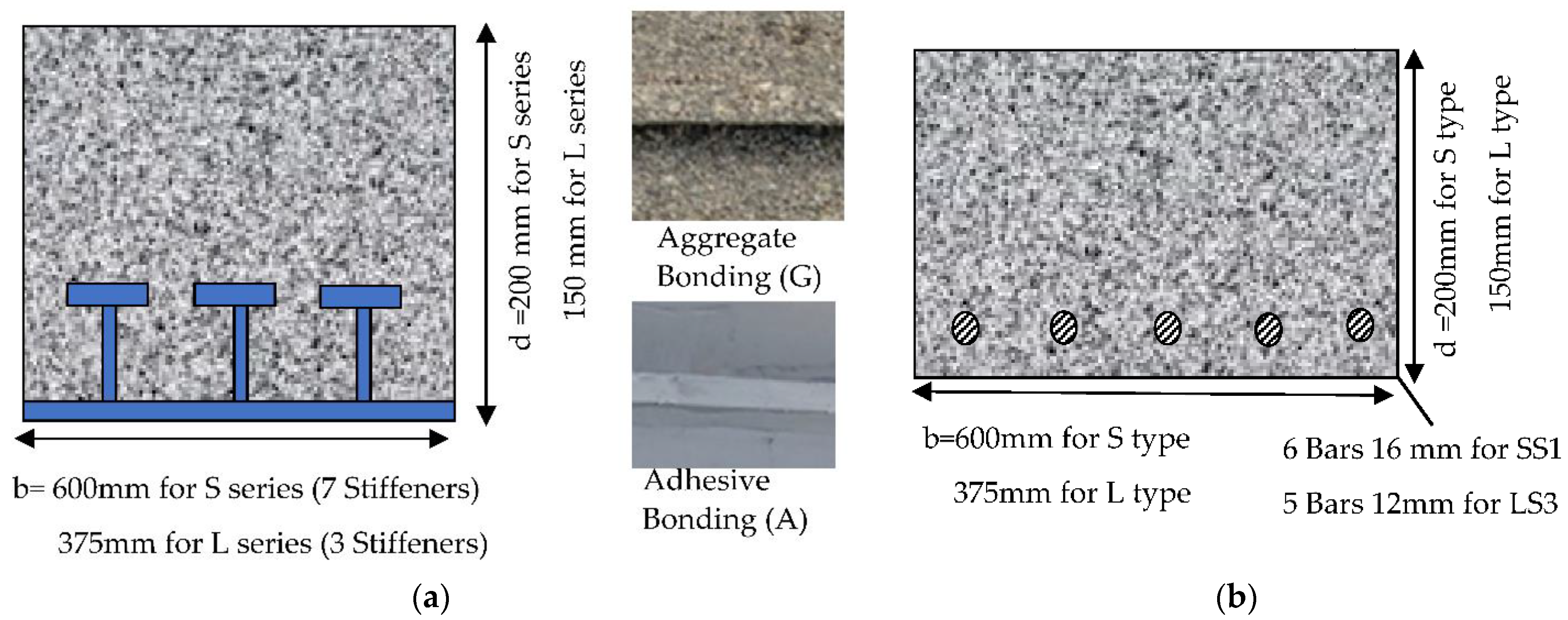





| Specimen | Span (m) | Depth (m) | Width (m) | Tensile Reinforcement |
|---|---|---|---|---|
| SS1 | 1 | 0.20 | 0.60 | Steel bars (6–16 mm) |
| SC1 | 1 | 0.20 | 0.60 | FRP plank (without any bond treatment) |
| SA1 | 1 | 0.20 | 0.60 | Adhesively bonded FRP plank |
| SG1 | 1 | 0.20 | 0.60 | Aggregate bonded FRP plank |
| LS3 | 3 | 0.15 | 0.375 | Steel bars (5–12 mm) |
| LC3 | 3 | 0.15 | 0.375 | FRP plank (without any bond treatment) |
| LA3 | 3 | 0.15 | 0.375 | Adhesively bonded FRP plank |
| LG3 | 3 | 0.15 | 0.375 | Aggregate bonded FRP plank |
| Thickness (mm) | Young’s Modulus (GPa) | Tensile Strength (MPa) | Volume Fraction | |||
|---|---|---|---|---|---|---|
| Avg.*1 | SD *2 | Avg. | SD | |||
| Plate | 4.5 | 27.9 | 2.55 | 375.5 | 5.86 | 0.35 |
| Ribs | 4 | 23.8 | 2.2 | 352.3 | 5.45 | 0.30 |
| Properties | Values |
|---|---|
| Epoxy content | Two-part epoxy |
| Pot life | 45 min at 25 °C |
| Viscosity | Viscous Thixotropic |
| Elastic modulus | 5 GPa |
| Elongation at break | 0.4% |
| Tensile strength | 15 MPa |
| Flexural strength | 30 MPa |
| Bond strength | 8–10 MPa |
| Specimen | Failure Load (kN) | Deflection at Failure (mm) | Failure Mode |
|---|---|---|---|
| SS1 | 297.77 | 3.11 | Concrete Shear |
| SC1 | 176.32 | 2.65 | Interfacial bond |
| SA1 | 453.51 | 4.27 | Concrete bond |
| SG1 | 397.00 | 3.49 | Concrete bond |
| LS3 | 69.30 | 30.10 | Flexure |
| LC3 | 42.03 | 17.32 | Interfacial bond |
| LA3 | 143.74 | 57.91 | Concrete bond |
| LG3 | 150.24 | 56.43 | Concrete bond |
| Parameter | S Series Slabs | L Series Slabs |
|---|---|---|
| Effective depth d (mm) | 187.73 | 139.97 |
| FRP effective stiffness Ef (GPa) | 26.22 | 26.58 |
| Reinforcement ratio ρ | 0.040 | 0.048 |
| Balanced reinforcement ratio ρb | 0.0141 | 0.0142 |
| Elastic neutral axis depth ce (mm) | 41.28 | 33.59 |
| Ultimate neutral axis depth cover (mm) | 50.88 | 41.96 |
| Type of Slab | Experimental | Estimated Capacity (kN) | |||
|---|---|---|---|---|---|
| Capacity (kN) | Flexure | Shear | Limit | ||
| ACI440 | ACI440 | ACI318 | |||
| S | 226.5 | 948 | 72.38 | 133.9 | 134 |
| L | 75 | 116 | 36.5 | 62 | 62 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Goyal, R.; Majhi, S.; Mukherjee, A.; Goyal, S. FRP Stay-in-Place Formworks for High Performance of Concrete Slabs. J. Compos. Sci. 2022, 6, 313. https://doi.org/10.3390/jcs6100313
Goyal R, Majhi S, Mukherjee A, Goyal S. FRP Stay-in-Place Formworks for High Performance of Concrete Slabs. Journal of Composites Science. 2022; 6(10):313. https://doi.org/10.3390/jcs6100313
Chicago/Turabian StyleGoyal, Reema, Subhra Majhi, Abhijit Mukherjee, and Shweta Goyal. 2022. "FRP Stay-in-Place Formworks for High Performance of Concrete Slabs" Journal of Composites Science 6, no. 10: 313. https://doi.org/10.3390/jcs6100313
APA StyleGoyal, R., Majhi, S., Mukherjee, A., & Goyal, S. (2022). FRP Stay-in-Place Formworks for High Performance of Concrete Slabs. Journal of Composites Science, 6(10), 313. https://doi.org/10.3390/jcs6100313







