Investigating the Effect of Screw Size on the Stress Level in MERO Joint for Space Frame Structures
Abstract
:1. Introduction
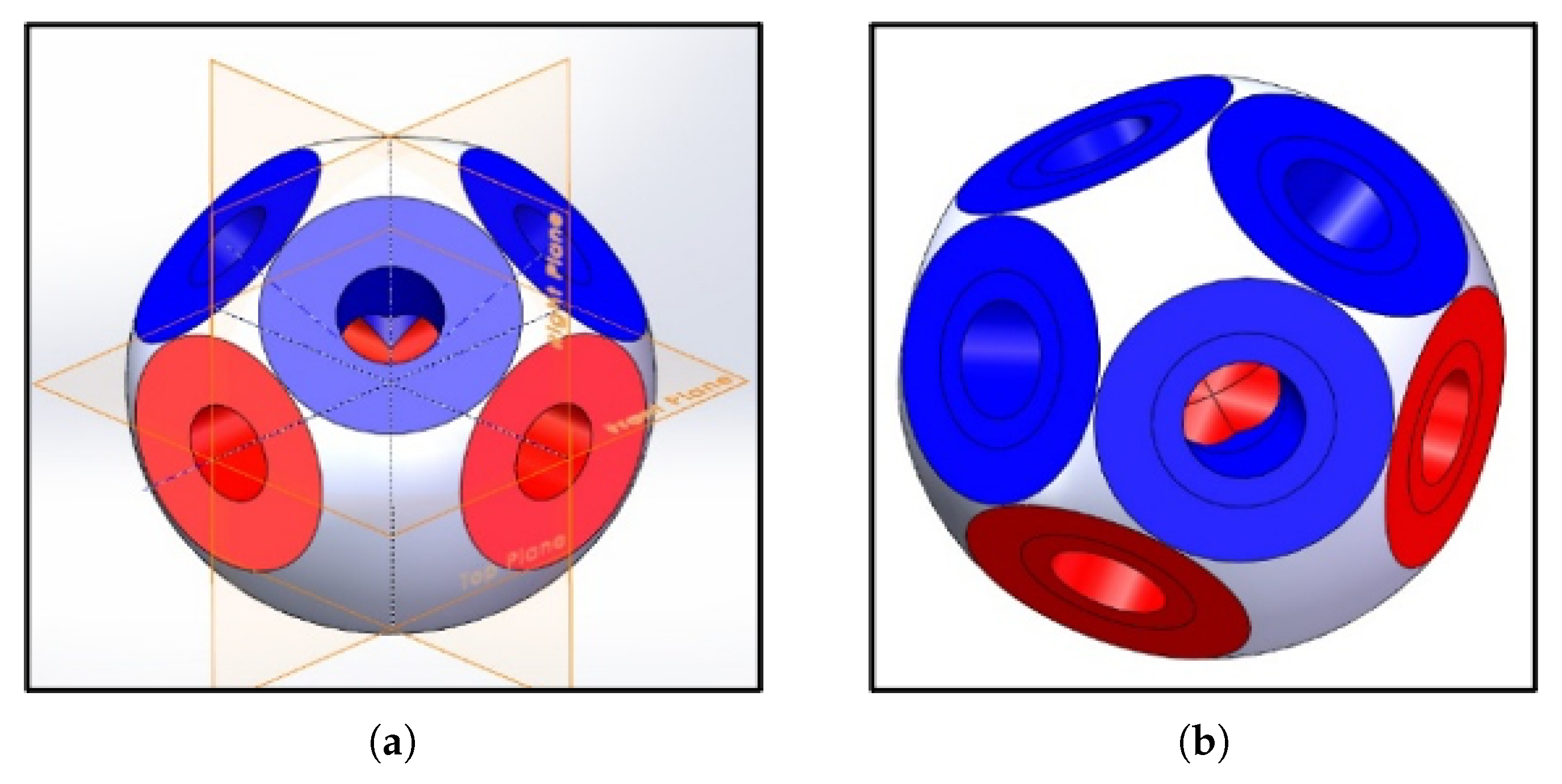
2. Geometry and Dimensions of MERO Double-Layer Joint
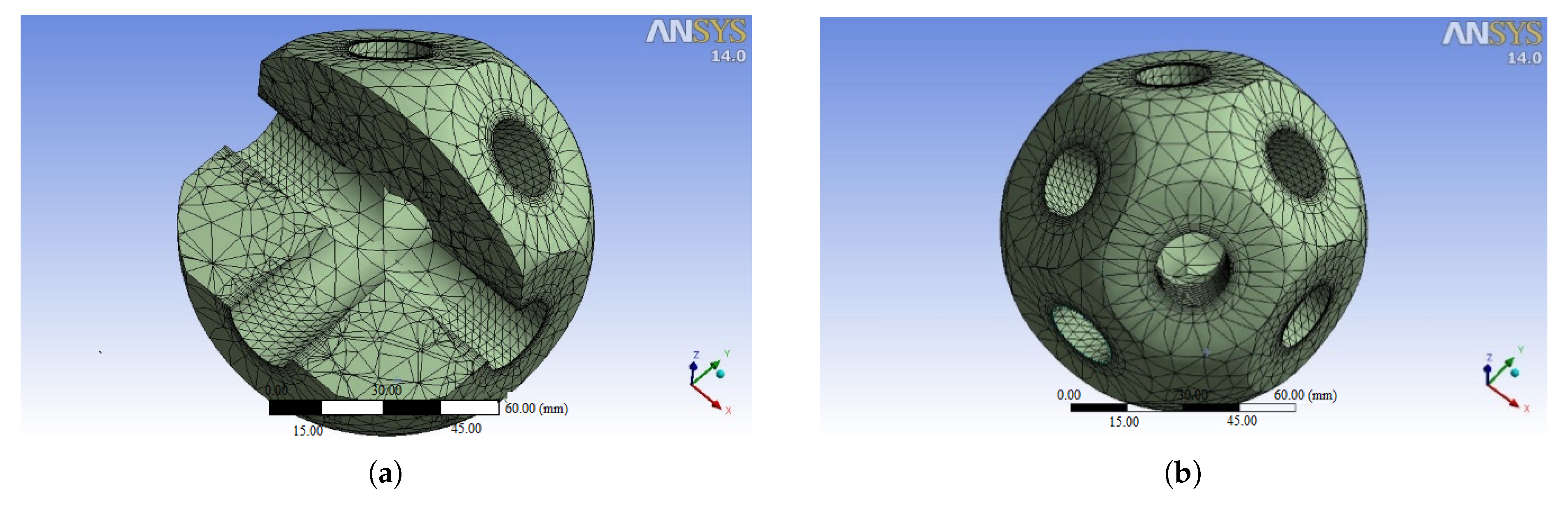
2.1. Grid Generation and Materials
2.2. Loading and Analysis
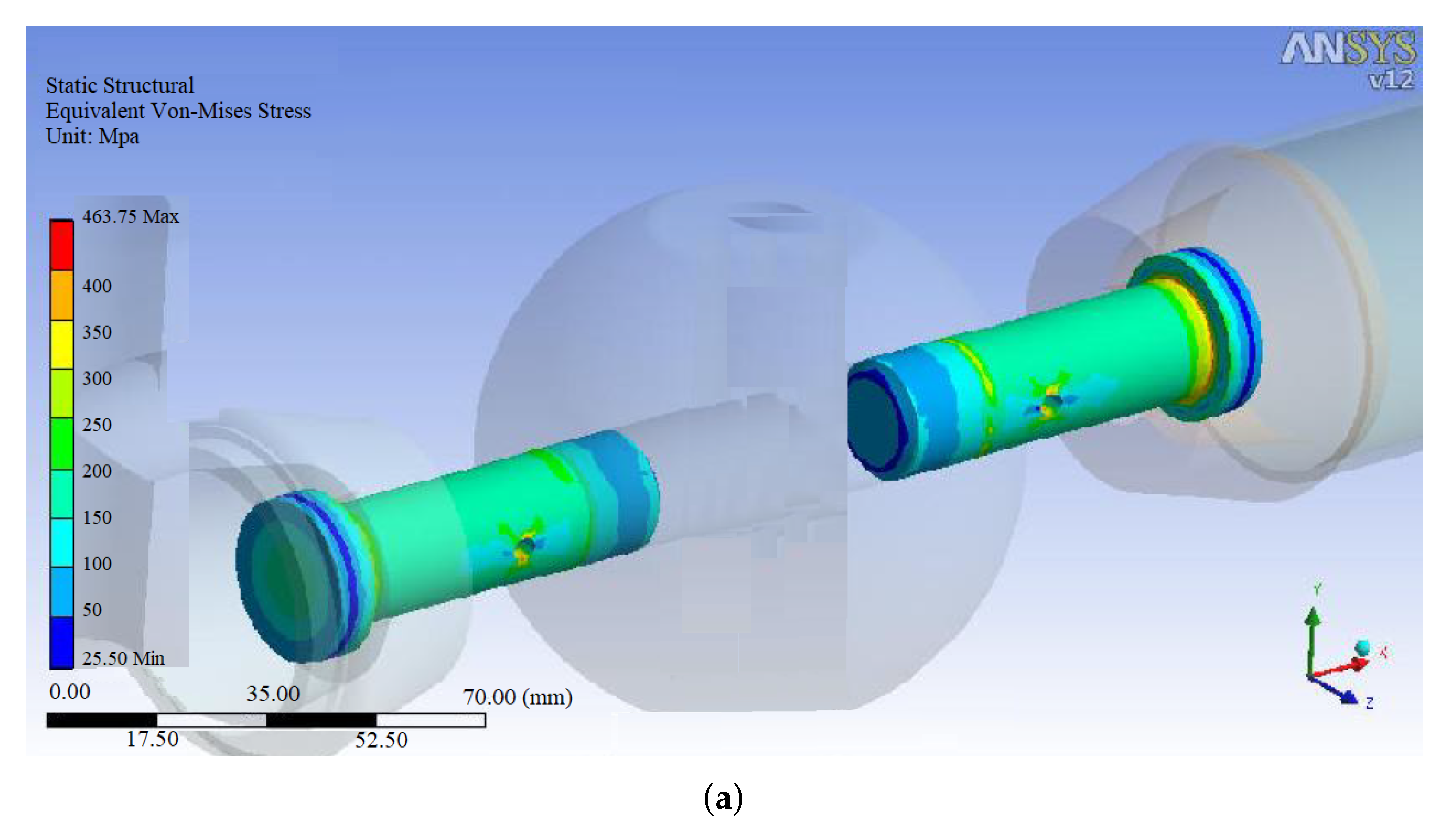
3. Results and Discussion
3.1. Effect of Changing the Screw Size on The Maximum Stress Level in the MERO Joint
3.2. Comparing the Effect of the Ball Joint Size on the Stress Levels for Constant Screw Sizes
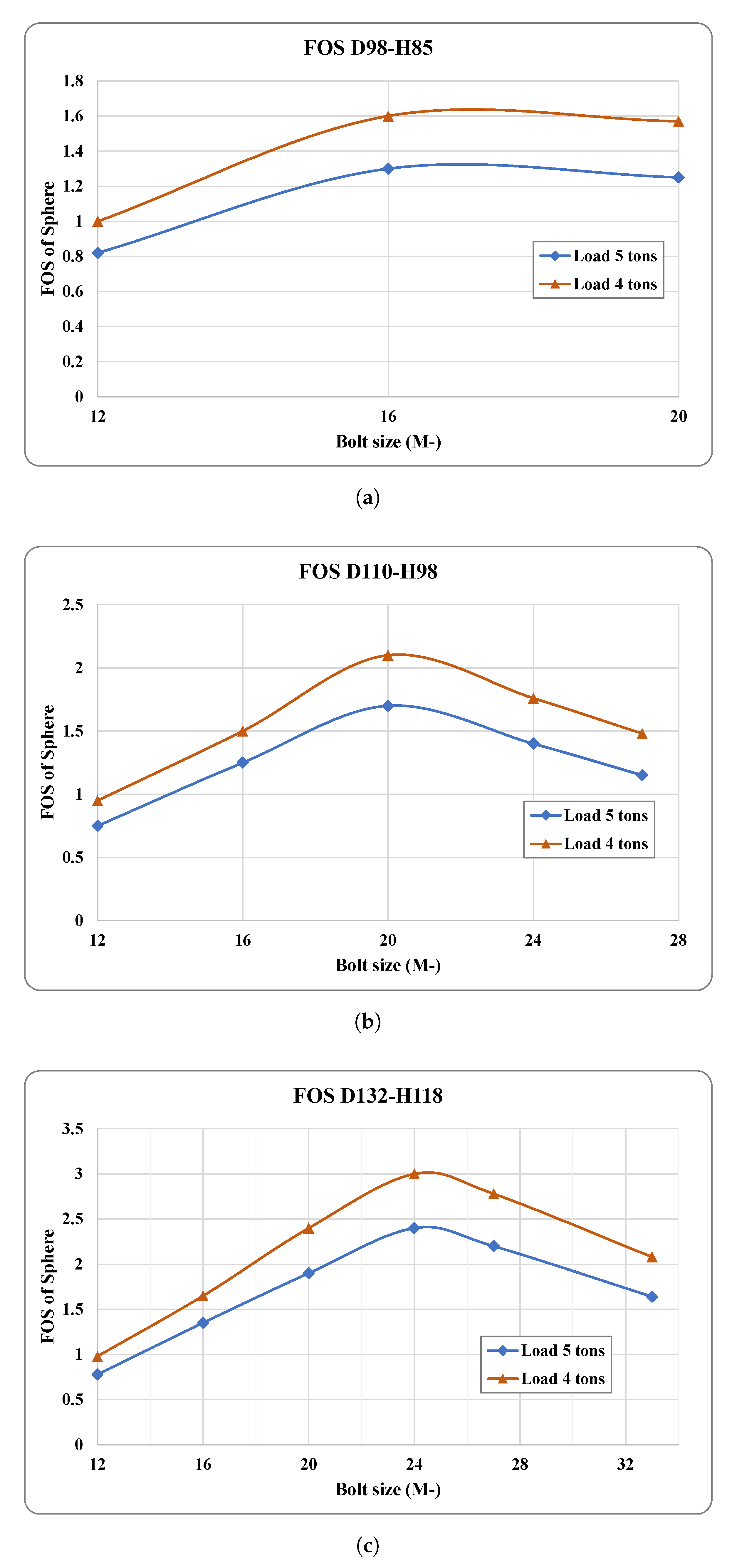
3.3. Comparing the Factor of Safety (FOS)
4. Conclusions
- As far as it is possible, it is recommended to avoid embedding a hole in the body of the screw or another mechanism is utilized for rotation and bolt tightening, like press-fitting of the pin in the hole, to prohibit stress concentration in the screw.
- A fillet is created under the screw head to decrease the stress concentration in this location.
- In case of a fillet generation under the screw head as mentioned above, in the corresponding location over the internal surface of conical piece hole, an appropriate bevel is created to assure a complete contact between the lower surface of the screw head and the internal surface of the conical piece hole and stress concentration is decreased, simultaneously.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Golabchi, M.; Golabchi, M. Fundamentals of Designing Space Frame Structures (Persian Language), 1st ed.; Pars University Press: Tehran, Iran, 2016. (In Persian) [Google Scholar]
- Ali, M.I.; Fan, F.; Khakina, P.N.; Ma, H. Cost-effective design of space structures joints: A review. Int. J. Struct. Constr. Eng. 2013, 7, 21–25. [Google Scholar]
- Subramanian, N. Principles of Space Frame Structures; Wheeler Publishing: Allahabad, India, 1983. [Google Scholar]
- Abdolvand, R. Dynamic Analysis and Modeling of Space Frame Structures with a Semi-Rigid System of MERO Ball Joint. 2001. Available online: https://www.tpbin.com/thesis-details/167 (accessed on 18 October 2021). (In Persian).
- Chilton, J. Space Grid Structures; Routledge: London, UK, 1999. [Google Scholar]
- Code of Practice for Skeletal Steel Space Structures Office of Deputy for Strategic Supervision Bureau of Technical Execution Systemstructure Code No. 400.2010. Available online: http://tec.mporg.ir (accessed on 18 October 2021).
- Arekar, V.A.; Bhavsar, B. Analytical study of MERO connector in double layer grid structure. IJLTET 2013, 2, 35–42. [Google Scholar]
- Ma, H.; Fan, F.; Cao, Z.; Cui, M.; Shen, S. Numerical simulation of semi-rigid joints in single-layer dome structures. J. Int. Assoc. Shell Spat. Struct. 2011, 52, 3–18. [Google Scholar]
- Subramanian, N. Space Structures: Principles and Practice; Multi-Science Publishing Co.: Essex, UK, 2007; Volume 2, p. 820. ISBN 0906522420. [Google Scholar]
- Jaspart, J.P. General report: Session on connections. J. Constr. Steel Res. 2000, 55, 69–89. [Google Scholar] [CrossRef]
- Nethercot, D. Frame structures: Global performance, static and stability behaviour: General report. J. Constr. Steel Res. 2000, 55, 109–124. [Google Scholar] [CrossRef]
- Dai, L. Finite-element model updating of the traditional beam–column joint in Tibetan heritage buildings using uniform design. Adv. Struct. Eng. 2020, 23, 1890–1901. [Google Scholar] [CrossRef]
- Abd-Elhady, A.; Abu-Sinna, A.; Atta, M.; Sallam, H.E.D.M. Identification of damage stages in bolted metallic joints for different joint geometries and tightening torques using statistical analysis. Adv. Struct. Eng. 2020, 23, 911–923. [Google Scholar] [CrossRef]
- Wong, C.; Mak, W.; Ko, J. System and parametric identification of flexible connections in steel framed structures. Eng. Struct. 1995, 17, 581–595. [Google Scholar] [CrossRef]
- Hadianfard, M.; Razani, R. Effects of semi-rigid behavior of connections in the reliability of steel frames. Struct. Saf. 2003, 25, 123–138. [Google Scholar] [CrossRef]
- Ihaddoudène, A.; Saidani, M.; Chemrouk, M. Mechanical model for the analysis of steel frames with semi rigid joints. J. Constr. Steel Res. 2009, 65, 631–640. [Google Scholar] [CrossRef]
- Ma, H.; Shan, Z.; Fan, F. Dynamic behaviour and seismic design method of a single-layer reticulated shell with semi-rigid joints. Thin-Walled Struct. 2017, 119, 544–557. [Google Scholar] [CrossRef]
- Ma, H.; Ma, Y.; Yu, Z.; Fan, F. Experimental and numerical research on gear-bolt joint for free-form grid spatial structures. Eng. Struct. 2017, 148, 522–540. [Google Scholar] [CrossRef]
- Feng, R.q.; Wang, X.; Chen, Y.; Cai, Q. Static performance of double-ring joints for freeform single-layer grid shells subjected to a bending moment and shear force. Thin-Walled Struct. 2018, 131, 135–150. [Google Scholar] [CrossRef]
- Li, H.; Taniguchi, Y. Load-carrying capacity of semi-rigid double-layer grid structures with initial crookedness of member. Eng. Struct. 2019, 184, 421–433. [Google Scholar] [CrossRef]
- Tsavdaridis, K.D.; Feng, R.; Liu, F. Shape optimization of assembled single-layer grid structure with semi-rigid joints. Procedia Manuf. 2020, 44, 12–19. [Google Scholar] [CrossRef]
- Liu, F.; Feng, R.; Tsavdaridis, K.D. Form finding of assembled lattice structure considering the effect of joint stiffness. In Structures; Elsevier: Amsterdam, The Netherlands, 2021; Volume 31, pp. 1096–1105. [Google Scholar]
- Kato, S.; Mutoh, I.; Shomura, M. Collapse of semi-rigidly jointed reticulated domes with initial geometric imperfections. J. Constr. Steel Res. 1998, 48, 145–168. [Google Scholar] [CrossRef]
- López, A.; Puente, I.; Serna, M.A. Direct evaluation of the buckling loads of semi-rigidly jointed single-layer latticed domes under symmetric loading. Eng. Struct. 2007, 29, 101–109. [Google Scholar] [CrossRef]
- López, A.; Puente, I.; Serna, M.A. Numerical model and experimental tests on single-layer latticed domes with semi-rigid joints. Comput. Struct. 2007, 85, 360–374. [Google Scholar] [CrossRef]
- Ma, H.; Fan, F.; Shen, S. Numerical parametric investigation of single-layer latticed domes with semi-rigid joints. J. Int. Assoc. Shell Spat. Struct. 2008, 49, 99–110. [Google Scholar]
- Fan, F.; Ma, H.h.; Cao, Z.G.; Shen, S.Z. Direct estimation of critical load for single-layer reticulated domes with semi-rigid joints. Int. J. Space Struct. 2010, 25, 15–24. [Google Scholar] [CrossRef]
- Hou, R.; Beck, J.L.; Zhou, X.; Xia, Y. Structural damage detection of space frame structures with semi-rigid connections. Eng. Struct. 2021, 235, 112029. [Google Scholar] [CrossRef]
- Amiri, J.V.; Davoodi, M. Modeling the semi-rigid behaviour of the MERO jointing system. Space Struct. 5 2002, 1, 309–316. [Google Scholar] [CrossRef]
- Davoodi, M.; Ebadi Jamkhaneh, M. Presenting a numerical model and investigating of MERO joint system components under combined axial and bending moment loading. In Proceedings of the 4th National Spatial Structures Conference, Tehran University, Tehran, Iran, 25–26 May 2014. (In Persian). [Google Scholar]
- Ebadi Jamkhaneh, M.; Davoodi, M.R.; Ebadi Jamkhaneh, J.A. Assessment of the ball joint behavior under combine loading. Modares Civ. Eng. J. 2017, 17. [Google Scholar]
- DIN EN 10083-2. Steels for Quenching and Tempering—Part 2: Technical Delivery Conditions for Non Alloy Steels, English Version of DIN EN 10083-2:2006-10; European Committee for Standardization: Brussels, Belgium, 2006.














| D (mm) | Yield Stress-Normalized (MPa) |
|---|---|
| 16–100 | 305 |
| 100–250 | 275 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Doaei, Y.; Aghakouchaki Hosseini, S.E.; Momenzadeh, A.; Harirchian, E. Investigating the Effect of Screw Size on the Stress Level in MERO Joint for Space Frame Structures. Appl. Syst. Innov. 2021, 4, 84. https://doi.org/10.3390/asi4040084
Doaei Y, Aghakouchaki Hosseini SE, Momenzadeh A, Harirchian E. Investigating the Effect of Screw Size on the Stress Level in MERO Joint for Space Frame Structures. Applied System Innovation. 2021; 4(4):84. https://doi.org/10.3390/asi4040084
Chicago/Turabian StyleDoaei, Yaser, Seyed Ehsan Aghakouchaki Hosseini, Amir Momenzadeh, and Ehsan Harirchian. 2021. "Investigating the Effect of Screw Size on the Stress Level in MERO Joint for Space Frame Structures" Applied System Innovation 4, no. 4: 84. https://doi.org/10.3390/asi4040084
APA StyleDoaei, Y., Aghakouchaki Hosseini, S. E., Momenzadeh, A., & Harirchian, E. (2021). Investigating the Effect of Screw Size on the Stress Level in MERO Joint for Space Frame Structures. Applied System Innovation, 4(4), 84. https://doi.org/10.3390/asi4040084








