Stripping Model for Short GRBs: The Impact of Nuclear Data
Abstract
:1. Introduction
2. The Influence of the NS EoS on the Stripping Time
2.1. The NS Inspiral Stage
2.2. The Stable Mass Transfer Stage
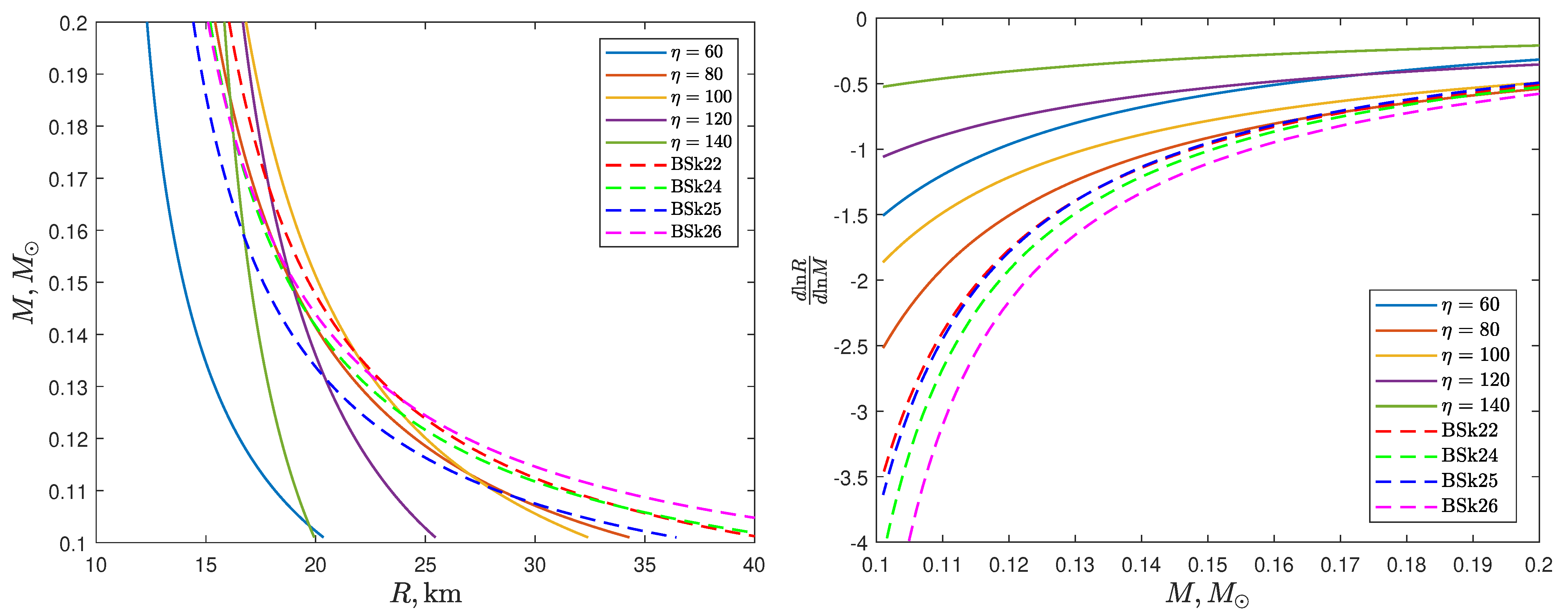
2.3. The NS EoS in the Low-Mass Region
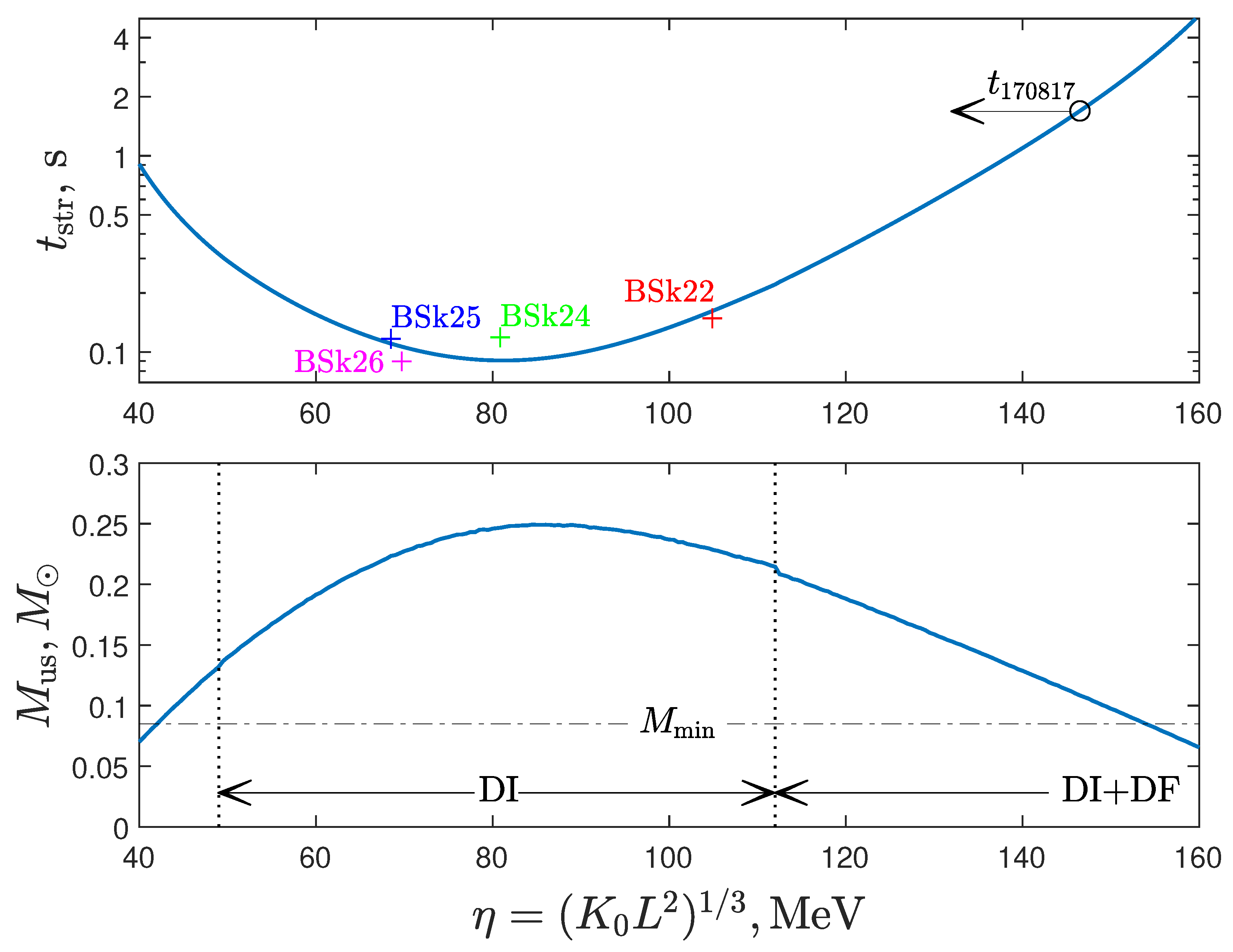
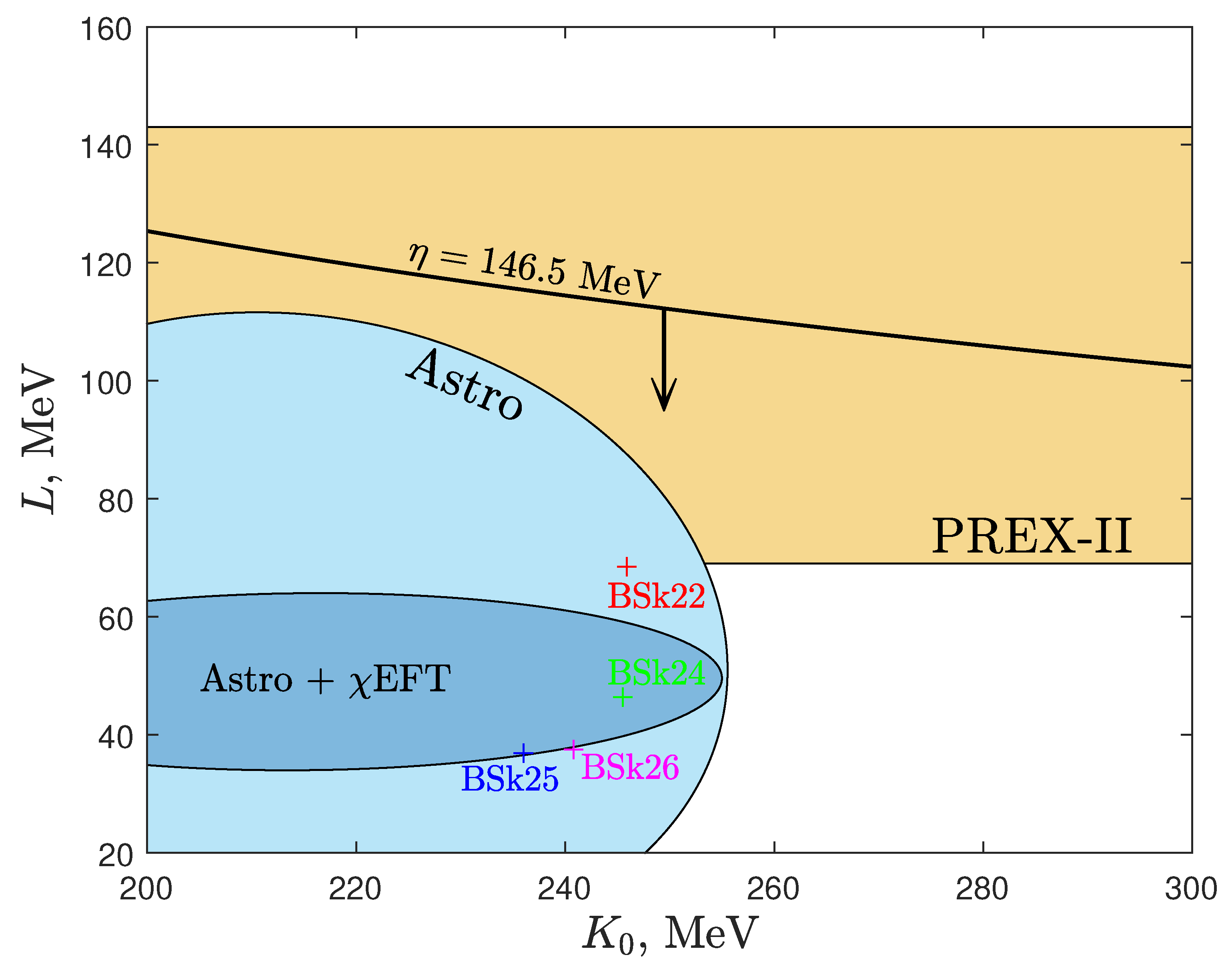
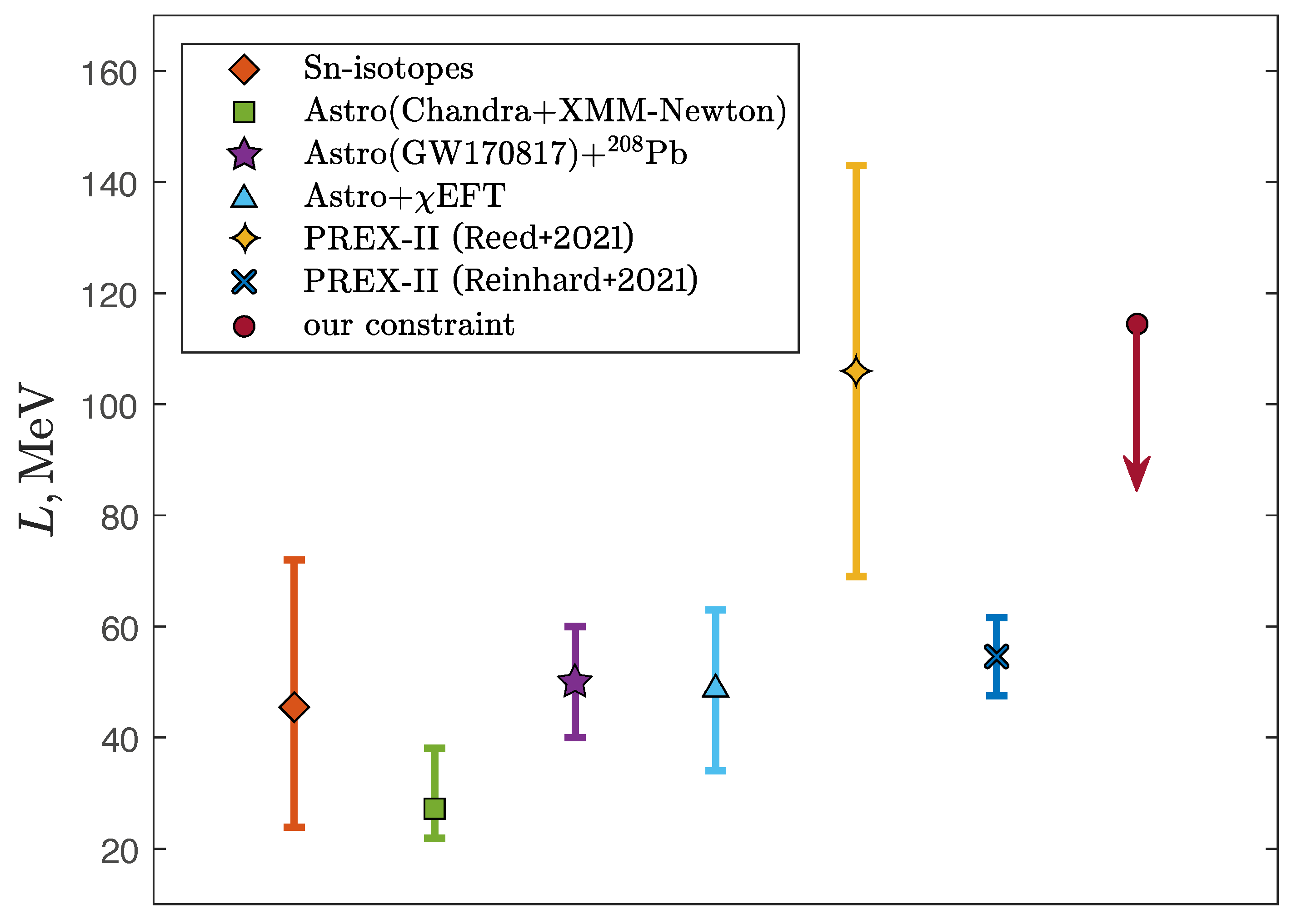
2.4. The Nuclear Parameters and the Stripping Time
3. r-Process during the LMNS Explosion
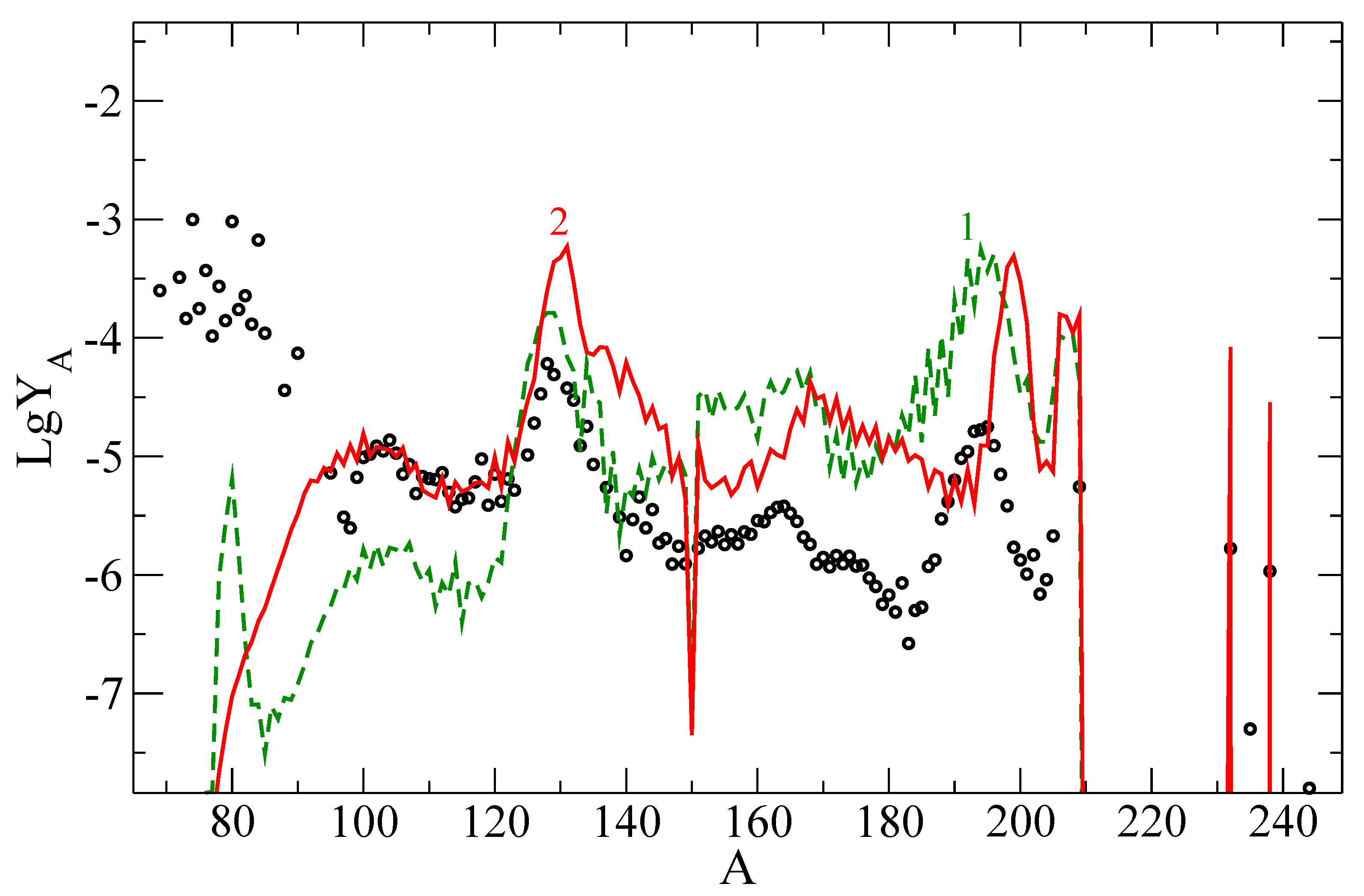
3.1. On the Influence of the EoS on the Results of Nucleosynthesis
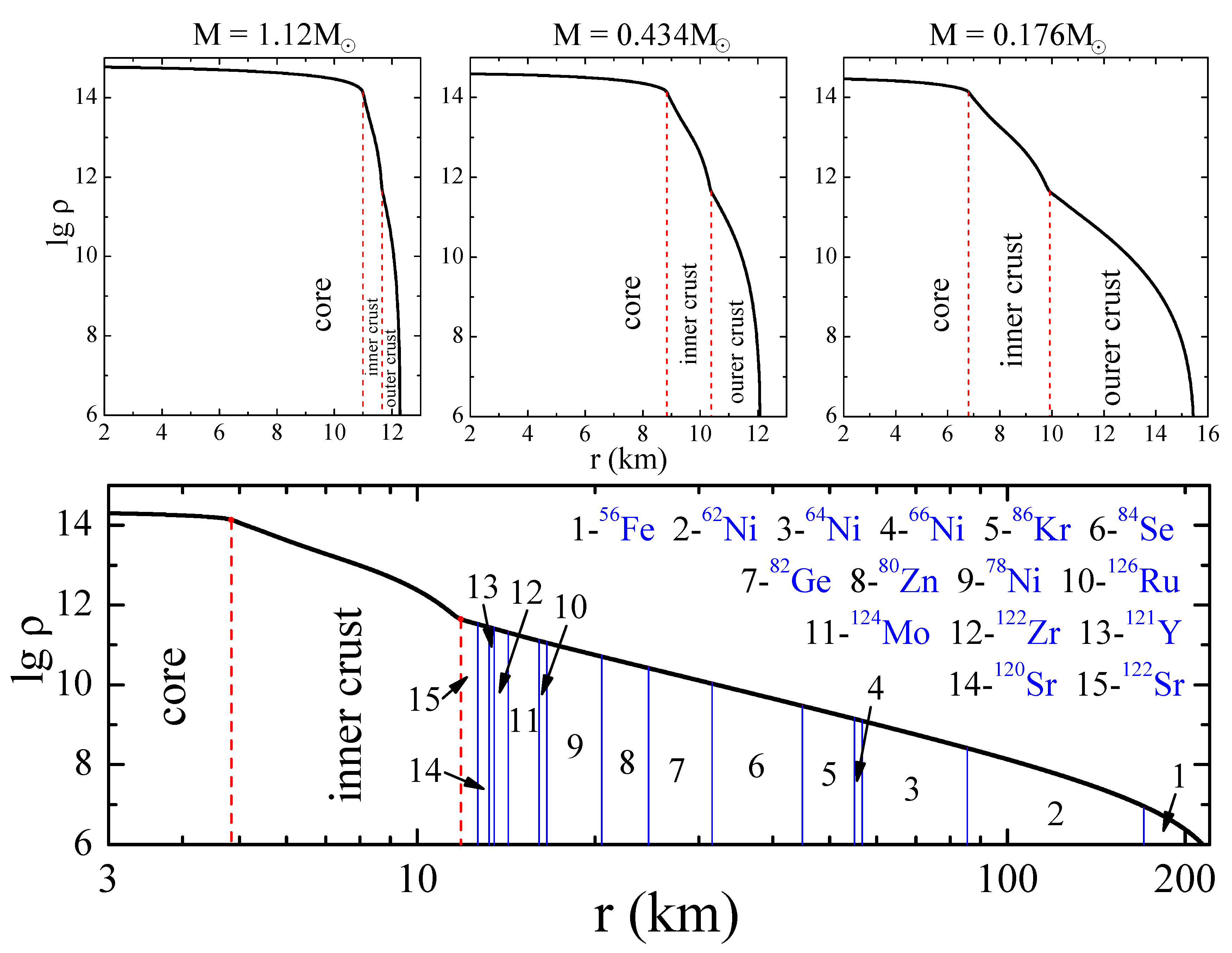
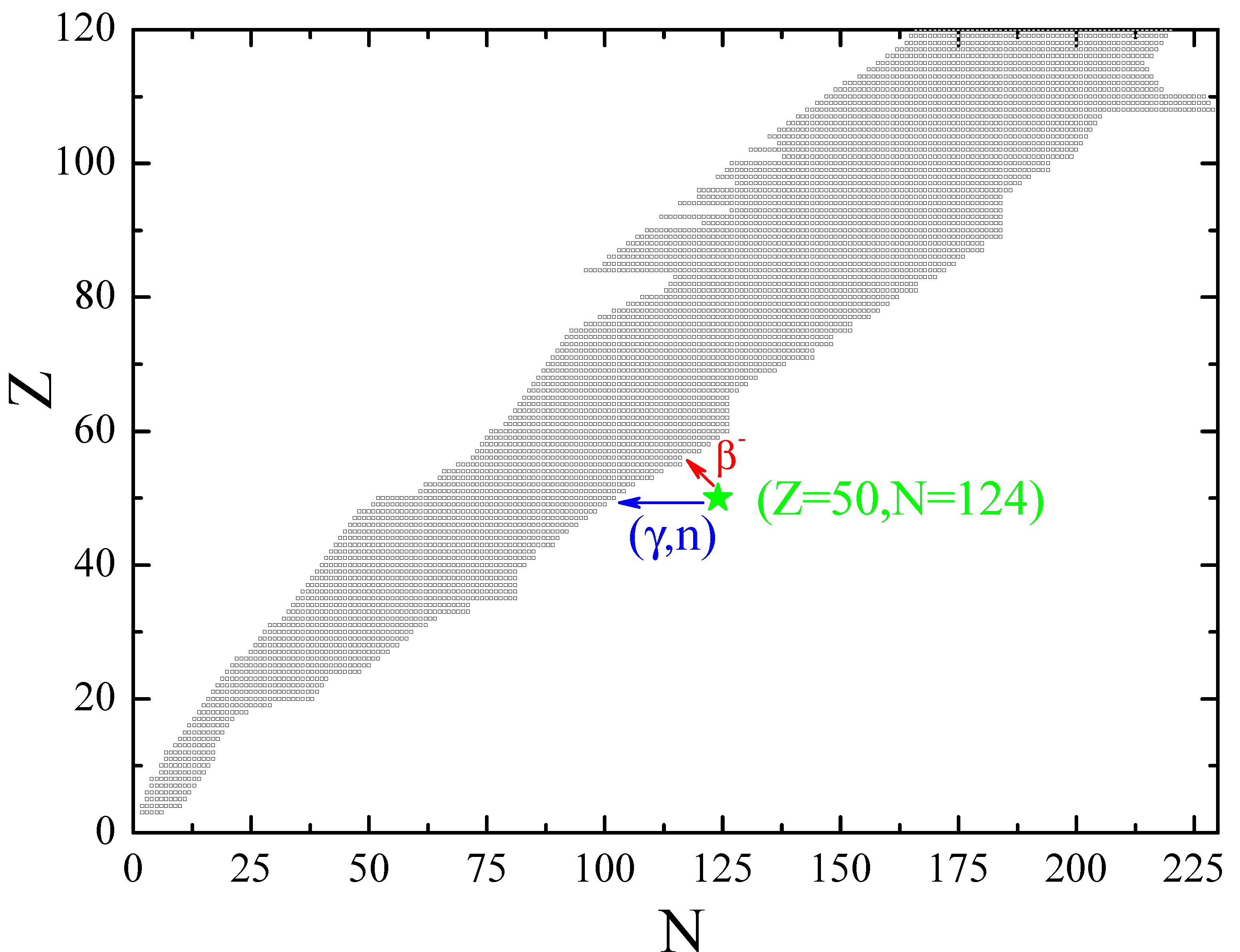
3.2. Nucleosynthesis in the Inner Crust
3.3. The Influence of Beta Decay Rates
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| GRB | Gamma-ray burst |
| GW | Gravitational wave |
| NS | Neutron star |
| LMNS | Low-mass NS |
| BH | Black hole |
| EoS | Equation of state |
References
- Clark, J.P.A.; Eardley, D.M. Evolution of close neutron star binaries. Astrophys. J. 1977, 215, 311–322. [Google Scholar] [CrossRef]
- Blinnikov, S.I.; Novikov, I.D.; Perevodchikova, T.V.; Polnarev, A.G. Exploding Neutron Stars in Close Binaries. Sov. Astron. Lett. 1984, 10, 177–179. [Google Scholar]
- Eichler, D.; Livio, M.; Piran, T.; Schramm, D.N. Nucleosynthesis, neutrino bursts and γ-rays from coalescing neutron stars. Nature 1989, 340, 126–128. [Google Scholar] [CrossRef]
- Narayan, R.; Paczynski, B.; Piran, T. Gamma-Ray Bursts as the Death Throes of Massive Binary Stars. Astrophys. J. Lett. 1992, 395, L83. [Google Scholar] [CrossRef]
- Li, L.X.; Paczyński, B. Transient Events from Neutron Star Mergers. Astrophys. J. 1998, 507, L59–L62. [Google Scholar] [CrossRef]
- Metzger, B.D.; Martínez-Pinedo, G.; Darbha, S.; Quataert, E.; Arcones, A.; Kasen, D.; Thomas, R.; Nugent, P.; Panov, I.V.; Zinner, N.T. Electromagnetic counterparts of compact object mergers powered by the radioactive decay of r-process nuclei. Mon. Not. R. Astron. Soc. 2010, 406, 2650–2662. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. Multi-messenger Observations of a Binary Neutron Star Merger. Astrophys. J. Lett. 2017, 848, L12. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. Gravitational Waves and Gamma-Rays from a Binary Neutron Star Merger: GW170817 and GRB 170817A. Astrophys. J. Lett. 2017, 848, L13. [Google Scholar] [CrossRef]
- Villar, V.A.; Guillochon, J.; Berger, E.; Metzger, B.D.; Cowperthwaite, P.S.; Nicholl, M.; Alexander, K.D.; Blanchard, P.K.; Chornock, R.; Eftekhari, T.; et al. The Combined Ultraviolet, Optical, and Near-infrared Light Curves of the Kilonova Associated with the Binary Neutron Star Merger GW170817: Unified Data Set, Analytic Models, and Physical Implications. Astrophys. J. Lett. 2017, 851, L21. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. Properties of the Binary Neutron Star Merger GW170817. Phys. Rev. X 2019, 9, 011001. [Google Scholar] [CrossRef]
- Faber, J.A.; Rasio, F.A. Binary Neutron Star Mergers. Living Rev. Relativ. 2012, 15, 8. [Google Scholar] [CrossRef] [PubMed]
- Baiotti, L.; Rezzolla, L. Binary neutron star mergers: A review of Einstein’s richest laboratory. Rep. Prog. Phys. 2017, 80, 096901. [Google Scholar] [CrossRef] [PubMed]
- Metzger, B.D. Kilonovae. Living Rev. Relativ. 2019, 23, 1. [Google Scholar] [CrossRef] [PubMed]
- Nakar, E. Short-hard gamma-ray bursts. Phys. Rep. 2007, 442, 166–236. [Google Scholar] [CrossRef]
- Lee, W.H.; Ramirez-Ruiz, E. The progenitors of short gamma-ray bursts. New J. Phys. 2007, 9, 17. [Google Scholar] [CrossRef]
- Murguia-Berthier, A.; Montes, G.; Ramirez-Ruiz, E.; De Colle, F.; Lee, W.H. Necessary Conditions for Short Gamma-Ray Burst Production in Binary Neutron Star Mergers. Astrophys. J. Lett. 2014, 788, L8. [Google Scholar] [CrossRef]
- Haensel, P.; Potekhin, A.Y.; Yakovlev, D.G. Neutron Stars 1: Equation of State and Structure; Springer: Berlin/Heidelberg, Germany, 2007; Volume 326. [Google Scholar]
- Blinnikov, S.I.; Imshennik, V.S.; Nadezhin, D.K.; Novikov, I.D.; Perevodchikova, T.V. Explosion of a Low-Mass Neutron Star. Sov. Astron. 1990, 34, 595. [Google Scholar]
- Panov, I.V.; Yudin, A.V. Production of Heavy Elements during the Explosion of a Low-Mass Neutron Star in a Close Binary. Astron. Lett. 2020, 46, 518–527. [Google Scholar] [CrossRef]
- Yip, C.M.; Chu, M.C.; Leung, S.C.; Lin, L.M. R-process nucleosynthesis of low-mass neutron star explosions. arXiv 2022, arXiv:2211.14023. [Google Scholar] [CrossRef]
- Blinnikov, S.I.; Nadyozhin, D.K.; Kramarev, N.I.; Yudin, A.V. Neutron Star Mergers and Gamma-Ray Bursts: Stripping Model. Astron. Rep. 2021, 65, 385–391. [Google Scholar] [CrossRef]
- Blinnikov, S.; Yudin, A.; Kramarev, N.; Potashov, M. Stripping Model for Short Gamma-Ray Bursts in Neutron Star Mergers. Particles 2022, 5, 198–209. [Google Scholar] [CrossRef]
- Kramarev, N.; Yudin, A. Accretion spin-up of the massive component in the neutron star stripping model for short gamma-ray bursts. Mon. Not. R. Astron. Soc. 2023. [Google Scholar] [CrossRef]
- Farrow, N.; Zhu, X.J.; Thrane, E. The Mass Distribution of Galactic Double Neutron Stars. Astrophys. J. 2019, 876, 18. [Google Scholar] [CrossRef]
- Doroshenko, V.; Suleimanov, V.; Pühlhofer, G.; Santangelo, A. A strangely light neutron star within a supernova remnant. Nat. Astron. 2022, 6, 1444–1451. [Google Scholar] [CrossRef]
- Pearson, J.M.; Chamel, N.; Potekhin, A.Y.; Fantina, A.F.; Ducoin, C.; Dutta, A.K.; Goriely, S. Unified equations of state for cold non-accreting neutron stars with Brussels-Montreal functionals—I. Role of symmetry energy. Mon. Not. R. Astron. Soc. 2018, 481, 2994–3026. [Google Scholar] [CrossRef]
- Yudin, A.V. Explosion of a Minimum-Mass Neutron Star within Relativistic Hydrodynamics. Astron. Lett. 2022, 48, 311–320. [Google Scholar] [CrossRef]
- Bauswein, A.; Blaschke, D.B.; Fischer, T. Effects of a Strong Phase Transition on Supernova Explosions, Compact Stars and Their Mergers. In Astrophysics in the XXI Century with Compact Stars; Vasconcellos, C.A.Z., Ed.; World Scientific: Singapore, 2022; pp. 283–320. ISBN 978-981-12-2094-4. [Google Scholar] [CrossRef]
- Kowalska, I.; Bulik, T.; Belczynski, K.; Dominik, M.; Gondek-Rosinska, D. The eccentricity distribution of compact binaries. Astron. Astrophys. 2011, 527, A70. [Google Scholar] [CrossRef]
- Lenon, A.K.; Nitz, A.H.; Brown, D.A. Measuring the eccentricity of GW170817 and GW190425. Mon. Not. R. Astron. Soc. 2020, 497, 1966–1971. [Google Scholar] [CrossRef]
- Paczyński, B. Gravitational Waves and the Evolution of Close Binaries. Acta Astron. 1967, 17, 287. [Google Scholar]
- Zahn, J.P. Tidal friction in close binary systems. Astron. Astrophys. 1977, 57, 383–394. [Google Scholar]
- Eggleton, P.P. Aproximations to the radii of Roche lobes. Astrophys. J. 1983, 268, 368–369. [Google Scholar] [CrossRef]
- Kramarev, N.; Yudin, A. Dynamics of direct impact accretion in degenerate binary systems. Mon. Not. R. Astron. Soc. 2023, 522, 626–634. [Google Scholar] [CrossRef]
- Lubow, S.H.; Shu, F.H. Gas dynamics of semidetached binaries. Astrophys. J. 1975, 198, 383–405. [Google Scholar] [CrossRef]
- Sotani, H.; Iida, K.; Oyamatsu, K.; Ohnishi, A. Mass and radius formulas for low-mass neutron stars. Prog. Theor. Exp. Phys. 2014, 2014, 051E01. [Google Scholar] [CrossRef]
- Lattimer, J.M. The Nuclear Equation of State and Neutron Star Masses. Annu. Rev. Nucl. Part. Sci. 2012, 62, 485–515. [Google Scholar] [CrossRef]
- Lattimer, J.M. Constraints on Nuclear Symmetry Energy Parameters. Particles 2023, 6, 30–56. [Google Scholar] [CrossRef]
- Reed, B.T.; Fattoyev, F.J.; Horowitz, C.J.; Piekarewicz, J. Implications of PREX-2 on the Equation of State of Neutron-Rich Matter. Phys. Rev. Lett. 2021, 126, 172503. [Google Scholar] [CrossRef]
- Horowitz, C.J.; Piekarewicz, J. Neutron Star Structure and the Neutron Radius of 208Pb. Phys. Rev. Lett. 2001, 86, 5647–5650. [Google Scholar] [CrossRef]
- Essick, R.; Landry, P.; Schwenk, A.; Tews, I. Detailed examination of astrophysical constraints on the symmetry energy and the neutron skin of 208Pb with minimal modeling assumptions. Phys. Rev. C 2021, 104, 065804. [Google Scholar] [CrossRef]
- Machleidt, R.; Entem, D.R. Chiral effective field theory and nuclear forces. Phys. Rep. 2011, 503, 1–75. [Google Scholar] [CrossRef]
- Stone, J.R.; Stone, N.J.; Moszkowski, S.A. Incompressibility in finite nuclei and nuclear matter. Phys. Rev. C 2014, 89, 044316. [Google Scholar] [CrossRef]
- Xu, J.; Xie, W.J.; Li, B.A. Bayesian inference of nuclear symmetry energy from measured and imagined neutron skin thickness in 116,118,120,122,124,130,132Sn, 208Pb, and 48Ca. Phys. Rev. C 2020, 102, 044316. [Google Scholar] [CrossRef]
- Baillot d’Etivaux, N.; Guillot, S.; Margueron, J.; Webb, N.; Catelan, M.; Reisenegger, A. New Constraints on the Nuclear Equation of State from the Thermal Emission of Neutron Stars in Quiescent Low-mass X-Ray Binaries. Astrophys. J. 2019, 887, 48. [Google Scholar] [CrossRef]
- Lim, Y.; Holt, J.W. Bayesian modeling of the nuclear equation of state for neutron star tidal deformabilities and GW170817. Eur. Phys. J. A 2019, 55, 209. [Google Scholar] [CrossRef]
- Reinhard, P.G.; Roca-Maza, X.; Nazarewicz, W. Information Content of the Parity-Violating Asymmetry in 208Pb. Phys. Rev. Lett. 2021, 127, 232501. [Google Scholar] [CrossRef]
- Panov, I.V.; Yudin, A.V. Synthesis of “Light” Heavy Elements upon the Explosion of a Low-Mass Neutron Star. Phys. At. Nucl. 2023, 86, 1–8. [Google Scholar] [CrossRef]
- Goriely, S.; Chamel, N.; Pearson, J.M. Further explorations of Skyrme-Hartree-Fock-Bogoliubov mass formulas. XIII. The 2012 atomic mass evaluation and the symmetry coefficient. Phys. Rev. C 2013, 88, 024308. [Google Scholar] [CrossRef]
- Wang, M.; Audi, G.; Wapstra, A.H.; Kondev, F.G.; MacCormick, M.; Xu, X.; Pfeiffer, B. The Ame2012 atomic mass evaluation. Chin. Phys. C 2012, 36, 1603. [Google Scholar] [CrossRef]
- Radice, D.; Perego, A.; Hotokezaka, K.; Fromm, S.A.; Bernuzzi, S.; Roberts, L.F. Binary Neutron Star Mergers: Mass Ejection, Electromagnetic Counterparts, and Nucleosynthesis. Astrophys. J. 2018, 869, 130. [Google Scholar] [CrossRef]
- Panov, I.V.; Kolbe, E.; Pfeiffer, B.; Rauscher, T.; Kratz, K.L.; Thielemann, F.K. Calculations of fission rates for r-process nucleosynthesis. Nucl. Phys. A 2005, 747, 633–654. [Google Scholar] [CrossRef]
- Panov, I.V. What Path the r-Process Takes: Extreme Cases and Comparison with Observations. Astron. Lett. 2003, 29, 163–169. [Google Scholar] [CrossRef]
- Panov, I.V.; Lutostansky, Y.S. Nucleosynthesis-Rate Dependence of Abundances of Nuclei Produced in the r-Process. Phys. At. Nucl. 2020, 83, 613–620. [Google Scholar] [CrossRef]
- Marketin, T.; Huther, L.; Martínez-Pinedo, G. Large-scale evaluation of β-decay rates of r-process nuclei with the inclusion of first-forbidden transitions. Phys. Rev. C 2016, 93, 025805. [Google Scholar] [CrossRef]
- Ney, E.M.; Engel, J.; Li, T.; Schunck, N. Global description of β− decay with the axially deformed Skyrme finite-amplitude method: Extension to odd-mass and odd-odd nuclei. Phys. Rev. C 2020, 102, 034326. [Google Scholar] [CrossRef]
- Borzov, I.N. Beta-decay rates. Nucl. Phys. A 2006, 777, 645–675. [Google Scholar] [CrossRef]
- Borzov, I.N. Global Calculations of Beta-Decay Properties Based on the Fayans Functional. Phys. At. Nucl. 2020, 83, 700–713. [Google Scholar] [CrossRef]
- Möller, P.; Pfeiffer, B.; Kratz, K.L. New calculations of gross β-decay properties for astrophysical applications: Speeding-up the classical r process. Phys. Rev. C 2003, 67, 055802. [Google Scholar] [CrossRef]
- Panov, I.V. Use of Global Predictions for Beta-Decay Rates in Astrophysical Models. Phys. At. Nucl. 2023, 86, 173–180. [Google Scholar] [CrossRef]
- Eichler, M.; Arcones, A.; Kelic, A.; Korobkin, O.; Langanke, K.; Marketin, T.; Martinez-Pinedo, G.; Panov, I.; Rauscher, T.; Rosswog, S.; et al. The Role of Fission in Neutron Star Mergers and Its Impact on the r-Process Peaks. Astrophys. J. 2015, 808, 30. [Google Scholar] [CrossRef]
- Cowan, J.J.; Sneden, C.; Lawler, J.E.; Aprahamian, A.; Wiescher, M.; Langanke, K.; Martínez-Pinedo, G.; Thielemann, F.K. Origin of the heaviest elements: The rapid neutron-capture process. Rev. Mod. Phys. 2021, 93, 015002. [Google Scholar] [CrossRef]



| № | Composition | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| BSk22 | BSk25 | BSk22 | BSk25 | BSk22 | BSk25 | BSk22 | BSk25 | BSk22 | BSk25 | BSk22 | BSk25 | |
| 18 | Sr | - | 0.98 | - | 11.64 | - | 0.297 | - | 0.88 | - | 0.88 | - |
| 17 | Sr | - | 1.19 | - | 11.60 | - | 0.302 | - | 1.25 | - | 2.13 | - |
| 16 | Sr | - | 1.39 | - | 11.56 | - | 0.306 | - | 0.80 | - | 2.93 | - |
| 15 | Sr | Sr | 1.62 | 8.63 | 11.49 | 11.59 | 0.311 | 0.311 | 3.60 | 2.64 | 6.53 | 2.64 |
| 14 | Y | Sr | 1.96 | 10.01 | 11.38 | 11.52 | 0.322 | 0.317 | 1.60 | 1.90 | 8.13 | 4.54 |
| 13 | Zr | Y | 2.17 | - | 11.31 | - | 0.328 | 0.322 | 1.48 | 1.10 | 9.61 | 5.64 |
| 12 | Mo | Zr | 2.59 | 10.57 | 11.20 | 11.39 | 0.339 | 0.328 | 3.87 | 2.20 | 13.48 | 7.84 |
| 11 | Ni | Mo | 3.19 | 11.44 | 11.01 | 11.24 | 0.350 | 0.339 | 4.95 | 4.10 | 18.43 | 11.94 |
| 10 | Ni | Ru | 3.80 | - | 10.84 | - | 0.359 | 0.349 | 1.74 | 1.05 | 20.17 | 12.99 |
| 9 | Ni | Ni | 4.27 | 13.76 | 10.74 | 10.88 | 0.368 | 0.359 | 4.92 | 7.00 | 25.09 | 19.99 |
| 8 | - | Zn | - | 14.74 | - | 10.58 | - | 0.375 | - | 5.10 | - | 25.09 |
| Model | Before Decompression | After Decompression | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Evaporated n | |||||||||
| 50 | 174 | 186 | 22 | 50 | 152 | 0.00278 | 0.578 | 0.139 | |
| 50 | 174 | 186 | 0 | 54 | 174 | 0.00278 | 0.517 | 0.150 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yudin, A.; Kramarev, N.; Panov, I.; Ignatovskiy, A. Stripping Model for Short GRBs: The Impact of Nuclear Data. Particles 2023, 6, 784-800. https://doi.org/10.3390/particles6030050
Yudin A, Kramarev N, Panov I, Ignatovskiy A. Stripping Model for Short GRBs: The Impact of Nuclear Data. Particles. 2023; 6(3):784-800. https://doi.org/10.3390/particles6030050
Chicago/Turabian StyleYudin, Andrey, Nikita Kramarev, Igor Panov, and Anton Ignatovskiy. 2023. "Stripping Model for Short GRBs: The Impact of Nuclear Data" Particles 6, no. 3: 784-800. https://doi.org/10.3390/particles6030050
APA StyleYudin, A., Kramarev, N., Panov, I., & Ignatovskiy, A. (2023). Stripping Model for Short GRBs: The Impact of Nuclear Data. Particles, 6(3), 784-800. https://doi.org/10.3390/particles6030050








