Modeling the Impact of the Viscoelastic Layer Thickness and the Frictional Strength to the Lithosphere Deformation in a Strike-Slip Fault: Insight to the Seismicity Pattern along the Great Sumatran Fault
Abstract
:1. Introduction
2. Methods
2.1. Model Building
2.2. Deformation Simulation
3. Results
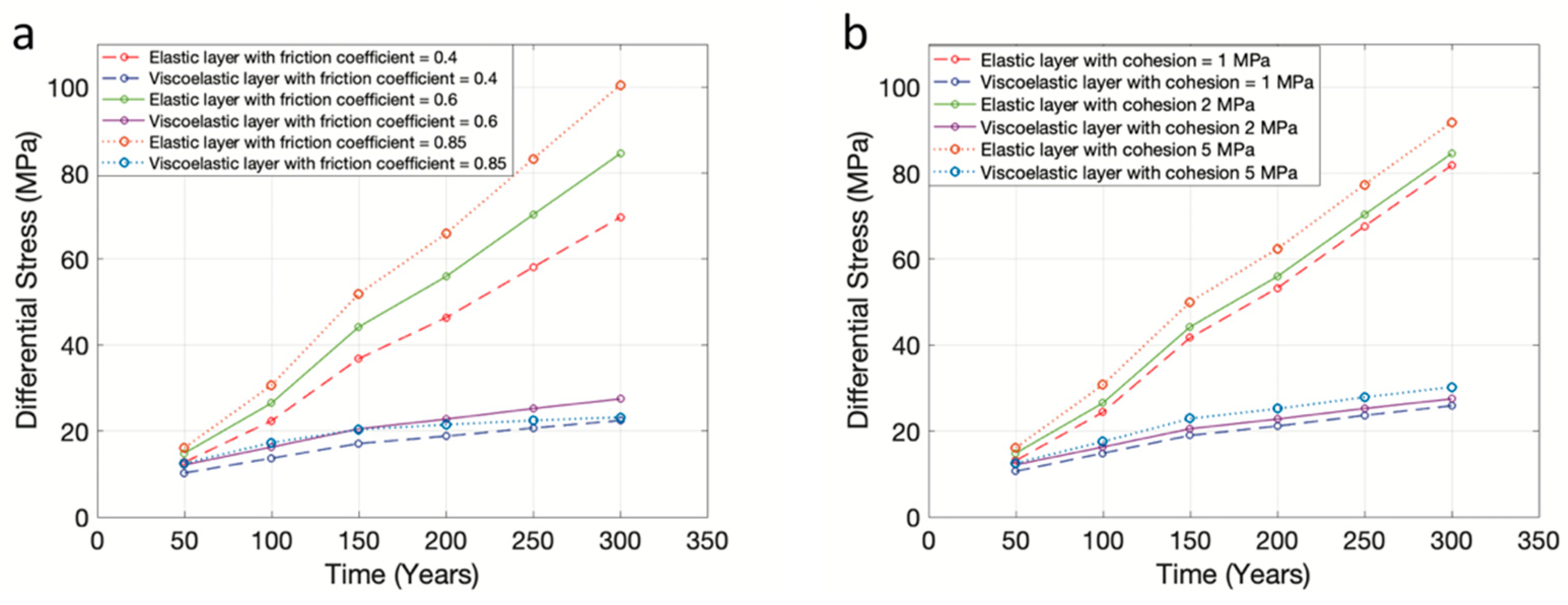
3.1. Strain Build-Up Model
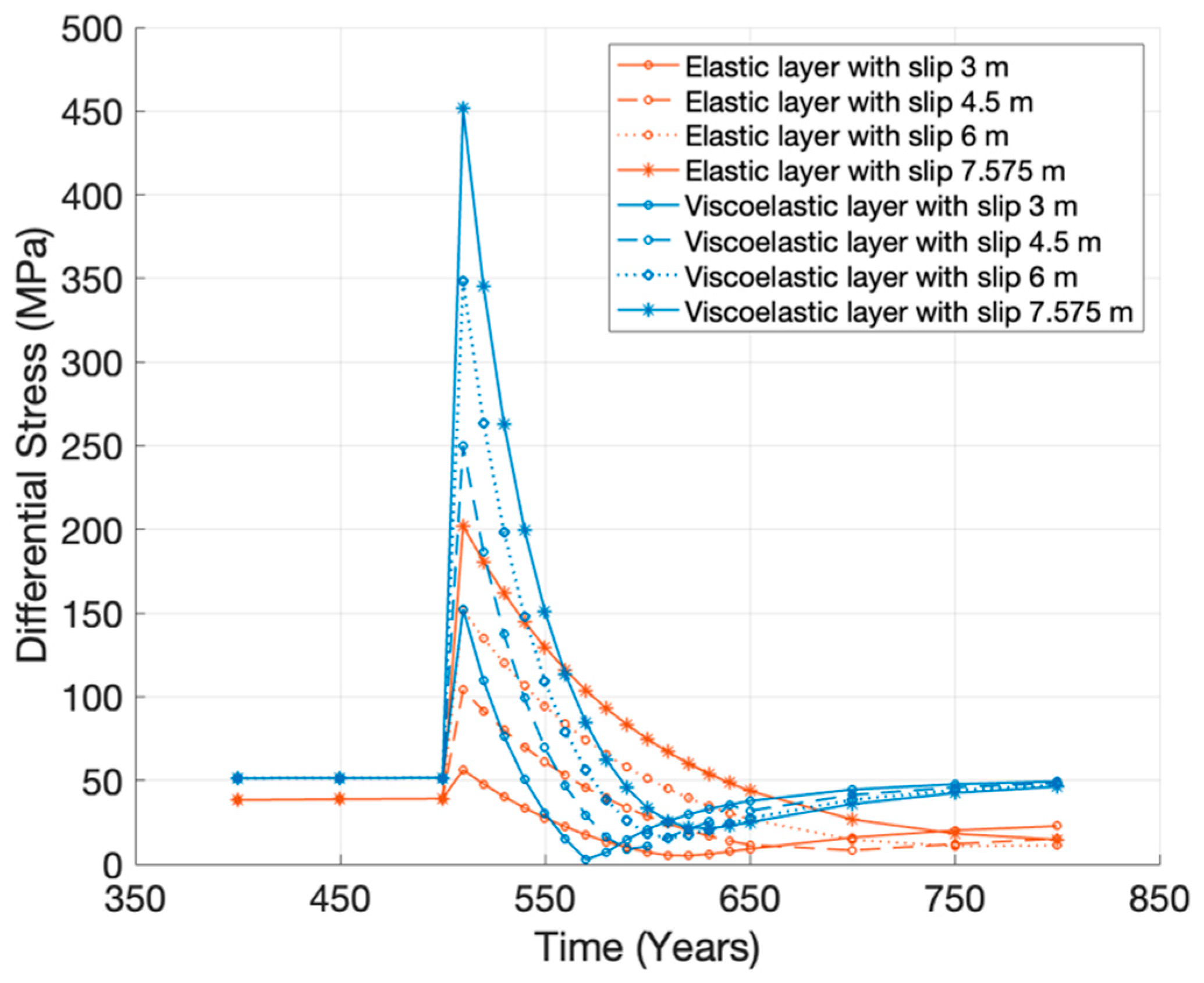
3.2. Strain Release Model
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Scholz, C.H. The Mechanics of Earthquakes and Faulting; Cambridge University Press: Cambridge, UK, 2019. [Google Scholar]
- Reid, H.F. The Mechanics of the Earthquake. The California Earthquake of April 18, 1906. Report of the State Earthquake Investigation Commission 1910. Available online: http://activetectonics.asu.edu/ActiveFaultingSeminar/Papers/Reid_1910.pdf (accessed on 8 July 2022).
- Thatcher, W. Strain accumulation and release mechanism of the 1906 San Francisco Earthquake. J. Geophys. Res. Solid Earth 1975, 80, 4862–4872. [Google Scholar] [CrossRef]
- Koseluk, R.A.; Bischke, R.E. An elastic rebound model for normal fault earthquakes. J. Geophys. Res. Solid Earth 1981, 86, 1081–1090. [Google Scholar] [CrossRef]
- Ziv, A.; Schmittbuhl, J. The seismic cycle and the difference between foreshocks and aftershocks in a mechanical fault model. Geophys. Res. Lett. 2003, 30. [Google Scholar] [CrossRef] [Green Version]
- Wright, T.J. The earthquake deformation cycle. Astron. Geophys. 2016, 57, 4.20–4.26. [Google Scholar] [CrossRef]
- Thatcher, W.; Rundle, J.B. A viscoelastic coupling model for the cyclic deformation due to periodically repeated Earthquakes at subduction zones. J. Geophys. Res. Solid Earth 1984, 89, 7631–7640. [Google Scholar] [CrossRef]
- Nur, A.; Mavko, G. Postseismic viscoelastic rebound. Science 1974, 183, 204–206. [Google Scholar] [CrossRef]
- Savage, J.C.; Prescott, W.H. Asthenosphere readjustment and the earthquake cycle. J. Geophys. Res. Solid Earth 1978, 83, 3369–3376. [Google Scholar] [CrossRef]
- Cohen, S.C. A multilayer model of time dependent deformation following an earthquake on a strike slip fault. J. Geophys. Res. Solid Earth 1982, 87, 5409–5421. [Google Scholar] [CrossRef]
- Li, V.C.; Rice, J.R. Crustal deformation in Great California earthquake cycles. J. Geophys. Res. Solid Earth 1987, 92, 11533–11551. [Google Scholar] [CrossRef] [Green Version]
- Kato, N. Seismic cycle on a strike-slip fault with rate- and state-dependent strength in an elastic layer overlying a viscoelastic half-space. Earth Planets Space 2002, 54, 1077–1083. [Google Scholar] [CrossRef]
- Fitch, T.J. Plate convergence, transcurrent faults, and internal deformation adjacent to Southeast Asia and the western Pacific. J. Geophys. Res. 1972, 77, 4432–4460. [Google Scholar] [CrossRef]
- Jarrard, R.D. Terrane motion by strike-slip faulting of forearc slivers. Geology 1986, 14, 780–783. [Google Scholar] [CrossRef]
- Bradley, K.E.; Feng, L.; Hill, E.M.; Natawidjaja, D.H.; Sieh, K. Implications of the diffuse deformation of the Indian Ocean lithosphere for slip partitioning of oblique plate convergence in Sumatra. J. Geophys. Res. Solid Earth 2017, 122, 572–591. [Google Scholar] [CrossRef]
- Prawirodirdjo, L.; Bock, Y.; Genrich, J.F.; Puntodewo, S.S.O.; Rais, J.; Subarya, C.; Sutisna, S. One century of tectonic deformation along the Sumatran fault from triangulation and Global Positioning System surveys. J. Geophys. Res. Solid Earth 2000, 105, 28343–28361. [Google Scholar] [CrossRef]
- Natawidjaja, D.H.; Triyoso, W. The Sumatran fault zone—From source to hazard. J. Earthq. Tsunami 2007, 1, 21–47. [Google Scholar] [CrossRef]
- McCaffrey, R. The Tectonic Framework of the Sumatran Subduction Zone. Annu. Rev. Earth Planet. Sci. 2009, 37, 345–366. [Google Scholar] [CrossRef] [Green Version]
- Bellier, O.; Sébrier, M.; Pramumijoyo, S.; Beaudouin, T.; Harjono, H.; Bahar, I.; Forni, O. Paleoseismicity and seismic hazard along the Great Sumatran Fault (Indonesia). J. Geodyn. 1997, 24, 169–183. [Google Scholar] [CrossRef]
- Hurukawa, N.; Wulandari, B.R.; Kasahara, M. Earthquake history of the Sumatran fault, Indonesia, since 1892, derived from relocation of large earthquakes. Bull. Seismol. Soc. Am. 2014, 104, 1750–1762. [Google Scholar] [CrossRef]
- Natawidjaja, D.H. Updating active fault maps and sliprates along the Sumatran Fault Zone, Indonesia. IOP Conf. Series: Earth Environ. Sci. 2018, 118, 012001. [Google Scholar] [CrossRef] [Green Version]
- Ito, T.; Gunawan, E.; Kimata, F.; Tabei, T.; Simons, M.; Meilano, I.; Agustan; Ohta, Y.; Nurdin, I.; Sugiyanto, D. Isolating along-strike variations in the depth extent of shallow creep and fault locking on the northern Great Sumatran Fault. J. Geophys. Res. Solid Earth 2012, 117. [Google Scholar] [CrossRef]
- Natawidjaja, D.H.; Bradley, K.; Daryono, M.R.; Aribowo, S.; Herrin, J. Late Quaternary Eruption of the Ranau Caldera and New Geological Slip Rates of the Sumatran Fault Zone in Southern Sumatra, Indonesia. Geosci. Lett. 2017, 4, 21. [Google Scholar] [CrossRef] [Green Version]
- National Center for Earthquake Studies of Indonesia (PUSGEN). Indonesia’s Earthquake Source and Hazard Map (In Bahasa Indonesia); Research and Development Agency of Ministry of Public Work and Housing: Jakarta, Indonesia, 2017; ISBN 978-602-5489-01-3. [Google Scholar]
- Wang, K.; He, J.; Hu, Y. Elastic and Viscoelastic models of Crustal Deformation in Great Earthquake Cycles. AGU Fall Meet. Abstr. 2003, 2003, G22E-02. [Google Scholar]
- Li, Q.; Liu, M.; Zhang, H. A 3-D viscoelastoplastic model for simulating long-term slip on non-planar faults. Geophys. J. Int. 2009, 176, 293–306. [Google Scholar] [CrossRef] [Green Version]
- Aagaard, B.T.; Knepley, M.G.; Williams, C.A. A domain decomposition approach to implementing fault slip in finite-element models of quasi-static and dynamic crustal deformation. J. Geophys. Res. Solid Earth 2013, 118, 3059–3079. [Google Scholar] [CrossRef] [Green Version]
- Aagard, B.T.; Knepley, M.; Williams, C. PyLith User Manual, Version 2.2.0.; Computational Infrastructure for Geodynamics: Davis, CA, USA, 2017.
- Lynch, J.C.; Richards, M.A. Finite-element Models of Stress Orientations in Well-developed Strike-slip Fault Zones: Implications for the Distribution of Lower Crustal Strain. J. Geophys. Res. Solid Earth 2001, 106, 26707–26729. [Google Scholar] [CrossRef] [Green Version]
- Burton, P.W.; Hall, T.R. Segmentation of the Sumatran fault. Geophys. Res. Lett. 2014, 41, 4149–4158. [Google Scholar] [CrossRef] [Green Version]
- Indrastuti, N.; Nugraha, A.D.; McCausland, W.A.; Hendrasto, M.; Gunawan, H.; Kusnandar, R.; Kasbani, K. 3-D Seismic Tomographic study of Sinabung Volcano, Northern Sumatra, Indonesia, during the inter-eruptive period October 2010–July 2013. J. Volcanol. Geotherm.Res. 2019, 382, 197–209. [Google Scholar] [CrossRef]
- Yegorova, T.P.; Pavlenkova, G.A. Velocity-density models of the Earth’s crust and upper mantle from the quartz, Craton, and Kimberlite superlong seismic profile. Izv. Phys. Solid Earth 2015, 51, 250–267. [Google Scholar] [CrossRef]
- Turcotte, D.L.; Schubert, G. Geodynamics; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Billen, M.I.; Gurnis, M. A low viscosity wedge in subduction zones. Earth Planet. Sci. Lett. 2001, 193, 227–236. [Google Scholar] [CrossRef]
- Johnson, K.M.; Hilley, G.E.; Burgmann, R. Influence of lithosphere viscosity structure on estimates of fault slip rate in the Mojave region of the San Andreas fault system. J. Geophys. Res. Solid Earth 2007, 112. [Google Scholar] [CrossRef] [Green Version]
- Byerlee, J.; Wyss, M. Rock Friction and Earthquake Prediction; Birkhäuser Verlag: Basel, Switzerland, 1978; Volume 116. [Google Scholar]
- Andrews, D.J. Rupture dynamics with energy loss outside the slip zone. J. Geophys. Res. 2005, 110, B01307. [Google Scholar] [CrossRef]
- Sieh, K.; Natawidjaja, D.H. Neotectonics of the Sumatran fault, Indonesia. J. Geophys. Res. Solid Earth 2000, 105, 28295–28326. [Google Scholar] [CrossRef]
- Laske, G.; Masters, G.; Ma, Z.; Pasyanos, M. Update on CRUST1.0—A 1-degree Global Model of Earth’s Crust. Geophys. Res. Abstr. 2013, 15, 2658. [Google Scholar]
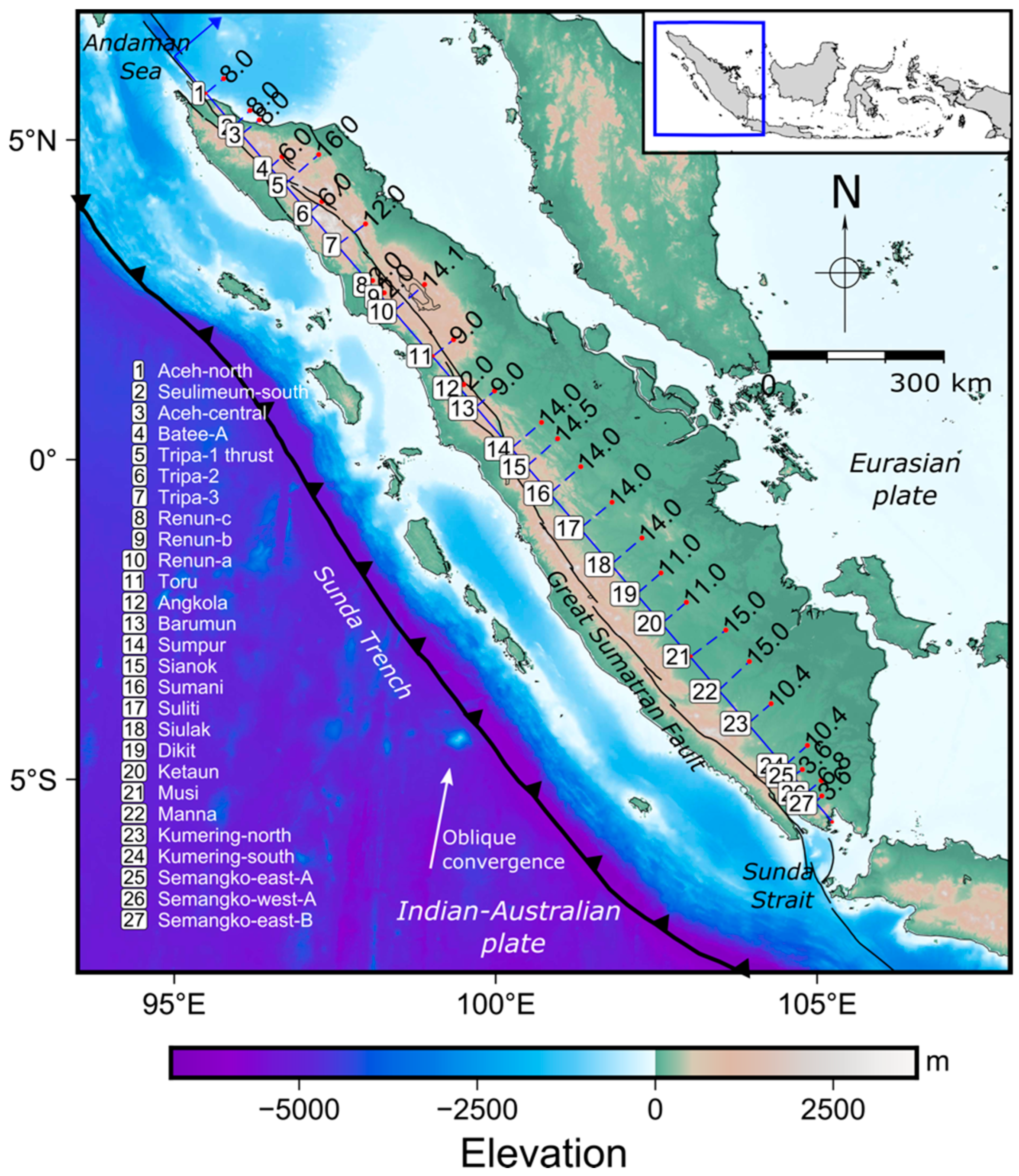
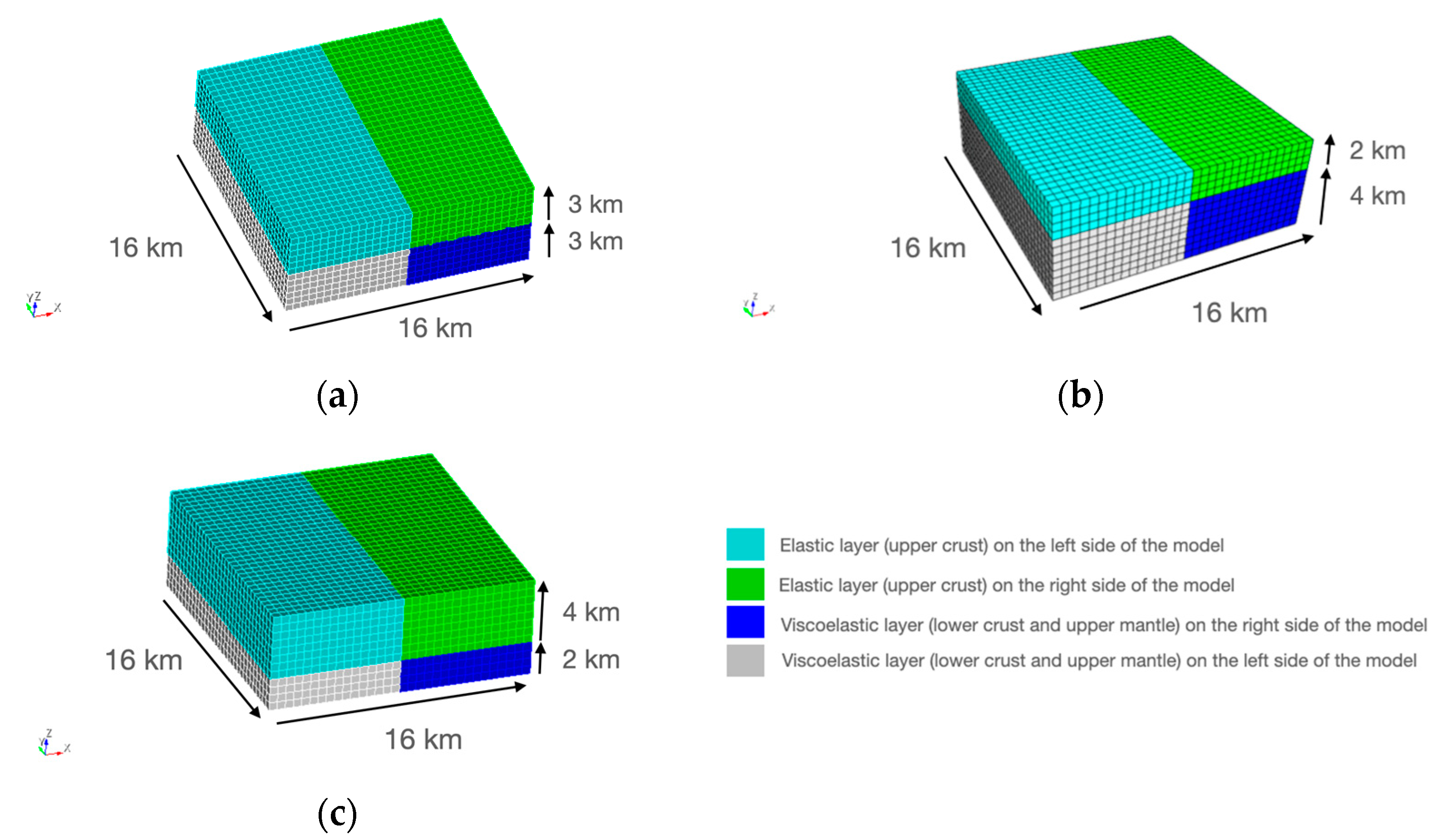


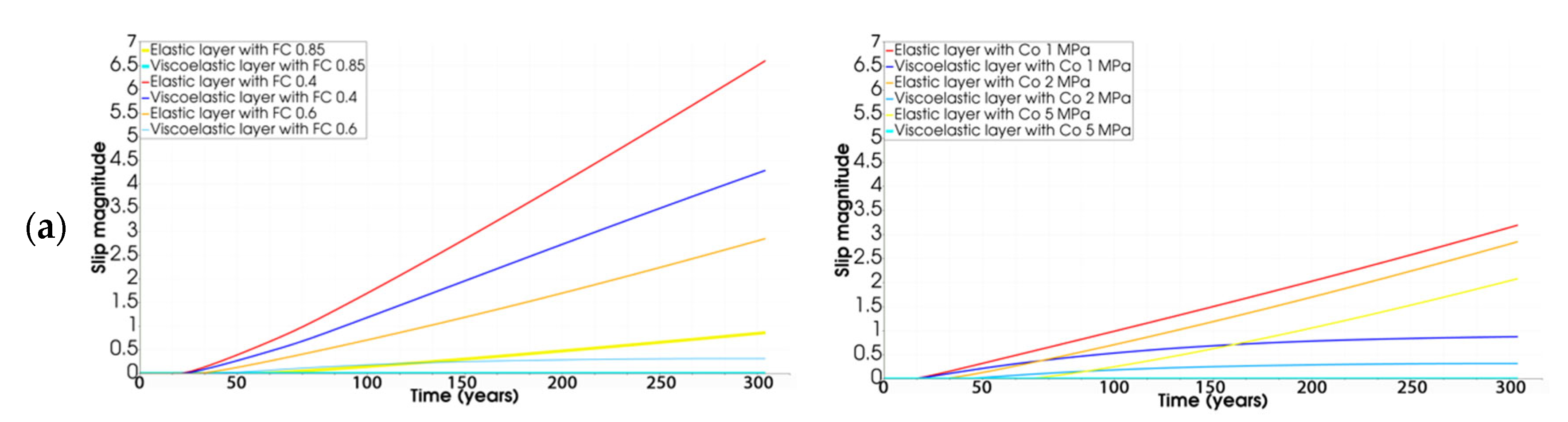
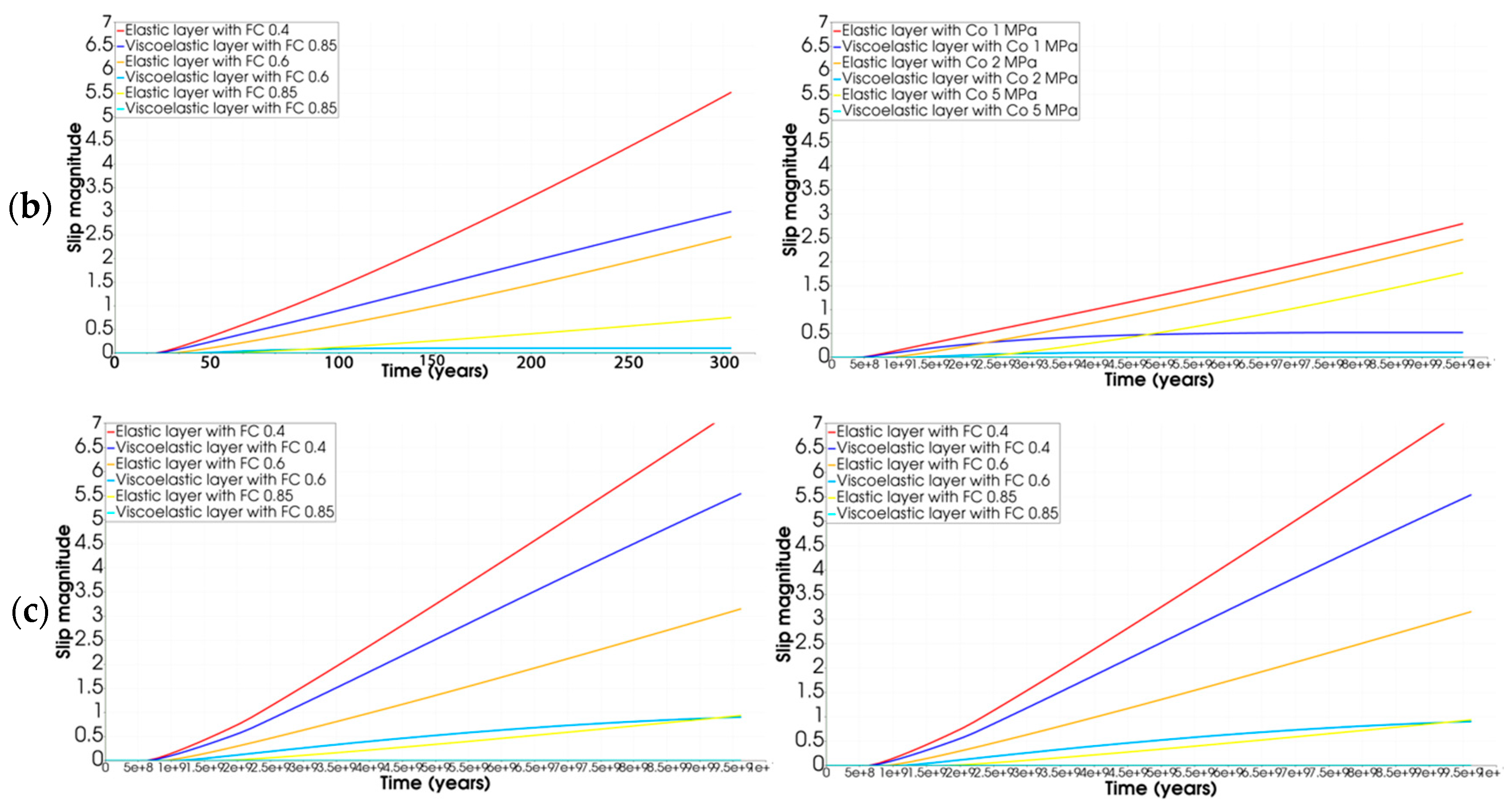



| Material Block | P-Wave Velocity (m/s) | S-Wave Velocity (m/s) | Density (kg/m3) | Viscosity (Pa s) |
|---|---|---|---|---|
| Upper crust (elastic) | 6625 | 4064 | 2700 | 0 |
| Lower crust & upper mantle (viscoelastic Maxwell) | 7230 | 4470 | 2900 | 7.10046 × 1019 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bening, M.A.; Sahara, D.P.; Triyoso, W.; Kusumawati, D. Modeling the Impact of the Viscoelastic Layer Thickness and the Frictional Strength to the Lithosphere Deformation in a Strike-Slip Fault: Insight to the Seismicity Pattern along the Great Sumatran Fault. GeoHazards 2022, 3, 452-464. https://doi.org/10.3390/geohazards3040023
Bening MA, Sahara DP, Triyoso W, Kusumawati D. Modeling the Impact of the Viscoelastic Layer Thickness and the Frictional Strength to the Lithosphere Deformation in a Strike-Slip Fault: Insight to the Seismicity Pattern along the Great Sumatran Fault. GeoHazards. 2022; 3(4):452-464. https://doi.org/10.3390/geohazards3040023
Chicago/Turabian StyleBening, Maulidia A., David P. Sahara, Wahyu Triyoso, and Dian Kusumawati. 2022. "Modeling the Impact of the Viscoelastic Layer Thickness and the Frictional Strength to the Lithosphere Deformation in a Strike-Slip Fault: Insight to the Seismicity Pattern along the Great Sumatran Fault" GeoHazards 3, no. 4: 452-464. https://doi.org/10.3390/geohazards3040023
APA StyleBening, M. A., Sahara, D. P., Triyoso, W., & Kusumawati, D. (2022). Modeling the Impact of the Viscoelastic Layer Thickness and the Frictional Strength to the Lithosphere Deformation in a Strike-Slip Fault: Insight to the Seismicity Pattern along the Great Sumatran Fault. GeoHazards, 3(4), 452-464. https://doi.org/10.3390/geohazards3040023







