Next Article in Journal
Constructing a Triangle Ensemble of Pt Clusters for Enhanced Direct-Pathway Electrocatalysis of Formic Acid Oxidation
Next Article in Special Issue
Rare Nuclearities and Unprecedented Structural Motifs in Manganese Cluster Chemistry from the Combined Use of Di-2-Pyridyl Ketone with Selected Diols
Previous Article in Journal
Low-Temperature Properties of the Sodium-Ion Electrolytes Based on EC-DEC, EC-DMC, and EC-DME Binary Solvents
Previous Article in Special Issue
Synthesis of the Bipyridine-Type Ligand 3-(2-Pyridyl)-5,6-diphenyl-1,2,4-triazine and Structural Elucidation of Its Cu(I) and Ag(I) Complexes
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Chemistry
Volume 5
Issue 3
10.3390/chemistry5030110
chemistry-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Structural and Magnetic Analysis of a Family of Structurally Related Iron(III)-Oxo Clusters of Metal Nuclearity Fe8, Fe12Ca4, and Fe12La4 †

by
Alok P. Singh
1,
ChristiAnna L. Brantley
1,
Kenneth Hong Kit Lee
1,
Khalil A. Abboud
1,
Juan E. Peralta
2 and
George Christou
1,*
1
Department of Chemistry, University of Florida, Gainesville, FL 32611, USA
2
Department of Physics and Science of Advanced Materials, Central Michigan University, Mount Pleasant, MI 48859, USA
*
Author to whom correspondence should be addressed.
†
Dedicated to Prof. Spyros P. Perlepes on the occasion of his 70th birthday: A wonderful friend, excellent researcher, great teacher, and true lover of inorganic chemistry and football!
Chemistry 2023, 5(3), 1599-1620; https://doi.org/10.3390/chemistry5030110
Submission received: 1 July 2023 / Revised: 18 July 2023 / Accepted: 19 July 2023 / Published: 24 July 2023
(This article belongs to the Special Issue Coordination Chemistry: Current Developments and Future Perspectives — A Themed Issue in Honor of Professor Spyros P. Perlepes on the Occasion of His 70th Birthday)
Browse Figures
Versions Notes

Abstract

:
The synthesis, crystal structure, and magnetic characterization are reported for three new structurally related iron(III) compounds (NHEt3)[Fe8O5(OH)5(O2PPh2)10] (1), [Fe12 Ca4O10(O2CPh)10(hmp)4] (2), and [Fe12La4O10(OH)4(tbb)24] (3), where hmpH is 2-(hydroxymethyl)pyridine and tbbH is 4-tBu-benzoic acid. 1 was obtained from the reaction of Fe(NO3)3·9H2O, diphenylphosphinic acid (Ph2PO2H), and NEt3 in a 1:4:16 molar ratio in MeCN at 50 °C; 2 was obtained from the reaction of [Fe3O(O2CPh)6(H2O)3](NO3), Ca(NO3)2, and NEt3 in a 1:1:4:2 ratio at 130 °C; and 3 was obtained from the reaction of Fe(NO3)3·9H2O, La(NO3)3·6H2O, 4-tBu-benzoic acid, and NEt3 in a 1:1:4:4 ratio in PhCN at 140 °C. The core of 1 consists of two {Fe4(µ3-O)2}8+ butterfly units stacked on top of each other and bridged by O2− and HO− ions. The cores of 2 and 3 also contain two stacked butterfly units, plus four additional Fe atoms, two at each end, and four M atoms (M = Ca2+ (2); La3+ (3)) on the sides. Variable-temperature (T) and solid-state dc and ac magnetization (M) data collected in the 1.8–300 K range revealed that 1 has an S = 0 ground state, 2 has a χMT value at low T consistent with the central Fe8 in a local S = 0 ground state and the two Fe3+ ions in each end-pair to be non-interacting, whereas 3 has a χMT value at low T consistent with these end-pairs each being ferromagnetically coupled with S = 5 ground states, plus intermolecular ferromagnetic interactions. These conclusions were reached from complementing the experimental studies with the calculation of the various Fe2 pairwise Jij exchange couplings by DFT computations and by using a magnetostructural correlation (MSC) for polynuclear Fe3+/O complexes, as well as a structural analysis of the intermolecular contacts in the crystal packing of 3.

1. Introduction

The chemistry of Fe3+/oxo complexes attracts considerable attention owing to its relevance to a wide range of areas including molecular magnetism [1], bioinorganic chemistry [2], catalysis [3,4], and materials science. Many Fe3+/oxo/carboxylate complexes spanning various nuclearities have been synthesized over the years from Fe2 [5,6,7,8,9,10,11] up to hexameric [Fe28]6 nanocages [12,13]. Dinuclear Fe3+ complexes serve as model systems to understand magnetic exchange couplings via magnetostructural correlations (MSCs) and as synthetic analogues of di-iron biomolecules such as ribonucleotide reductase [14,15,16], methane monooxygenase [14,15,17,18,19,20], hemerythrin [21,22,23], and others [24,25,26]. Higher nuclearity Fe3+/oxo clusters are highly desired and very useful for studies of interesting magnetic effects such as spin frustration, and even as models of intermediates in the growth of nanoscale Fe3+/O/OH units within the ferritin Fe storage protein [27,28,29,30,31,32,33,34,35]. The high charge and Lewis acidity of Fe3+ strongly favor the formation of oxide bridges from water molecules and thus higher-nuclearity clusters [27,36,37], and these have been of particular interest within the field of molecular magnetism since spin frustration often leads to a significant ground state spin value [28,38,39] and even single-molecule magnetism. Thus, there is continuing interest in Fe3+/oxo cluster chemistry.
Our work in this area has concentrated on carboxylates, either alone or in conjunction with chelating/bridging groups, and has led to clusters such as, e.g., [Fe18(pd)12(pdH)12(O2CPh)6(NO3)6]6+ (pdH2 = propane-1,3-diol) [40], which is the largest single-stranded homometallic iron wheel and [Fe22O14(OH)3(O2CMe)21(mda)6]2+ (mdaH = N-(methyl)diethanolamine) salts. [27] We have also extended our work to various ‘pseudo-carboxylates’, anionic groups that can bridge metals in a manner analogous to that of carboxylates but with differing electronic and/or steric properties. Their general formula is [RxYOy]z−, where Y = P, As, S, or Se, x = 1 or 2, y = 2 or 3, and z = 1 or 2, and examples include diphenylphosphinate (Ph2PO2–), benzenesulfonate (PhSO3–), benzeneseleninate (PhSeO2–), and dimethylarsinate (Me2AsO2–) groups. In previous work, we have explored such ligands extensively in Mn/O cluster chemistry [41,42,43,44,45,46,47], but related application in Fe/O chemistry has been limited to date [48,49,50,51,52]. Therefore, we have employed diphenylphosphinic acid (Ph2PO2H) [53] in the present work.
In addition to the ligand type, we have also explored some reactions that contain a heterometal salt and have chosen diamagnetic La3+ and Ca2+ for preliminary study for the following reasons: (i) The number of known Fe-lanthanide (Ln) clusters is currently limited and includes Fe12Ln4, Fe14Gd12, Fe13La6, Fe22La6, Fe29M16, and Fe33M12 (M = Y, Gd) [54,55,56,57,58]; and (ii) given our past interest in the Mn4Ca/oxo cluster that is part of the oxygen-evolving complex (OEC) in the photosynthetic apparatus of green plants and cyanobacteria [59,60,61], we have found it interesting that the alkaline phosphatase from P. fluorescens, PhoX, consists of an Fe2Ca/oxo cluster with two additional Ca2+ ions nearby [62,63,64,65]. There are only a few Fe/Ca/oxo clusters in the literature, including moderate nuclearity examples: Fe2Ca, and two Fe3Ca clusters with differing oxidation states [64,66,67], and higher-nuclearity Fe14Ca12 [68] and Fe9Ca2 [69].
A variety of reactions were explored involving different permutations of the above ligand types and metal compositions, as well as metal:ligand and Fe:La(Ca) ratios, reaction temperature, and the additional presence of a chelate such as 2-(hydroxymethyl)pyridine (hmpH). Among the products that could be isolated in pure form and structurally characterized, we noted that three of them are structurally related, in that they all contain the same {Fe8(oxo)10} core unit either alone or as a fragment of a larger core unit, consisting of two butterfly units [28] stacked on top of each other and linked by six additional O2−/HO− ions. These clusters were (NHEt3)[Fe8O5(OH)5(O2PPh2)10] (1), [Fe12Ca4O10(O2CPh)10(hmp)4] (2), and [Fe12La4O10(OH)4(tbb)24] (3), where tbbH is 4-tBu-benzoic acid. We herein describe the syntheses and structures of 1–3, together with a detailed analysis of their magnetic properties using experimental magnetic susceptibility studies, density functional theory (DFT), and magnetostructural correlation (MSC) methods.

2. Materials and Methods

2.1. Synthesis

All manipulations were performed under aerobic conditions using chemicals as received. [Fe3O(O2CPh)6(H2O)3](NO3) was prepared as described elsewhere [70]. Abbreviations: hmpH = 2-(hydroxymethyl)pyridine; tbbH = 4-tBu-benzoic acid.

2.1.1. (NHEt3)[Fe8O5(OH)5(O2PPh2)10] (1)

To a stirred solution of NEt3 (1.11 mL, 8.00 mmol) and Ph2PO2H (0.436 g, 2.00 mmol) in warm (~50 °C), MeCN (20 mL) was added, Fe(NO3)·9H2O (0.20 g, 0.50 mmol), resulting in an orange suspension. After stirring for 2 h, the reaction was filtered, the resulting orange solid was discarded, and the filtrate was capped and maintained undisturbed at ambient temperature. After 1 week, the closed cap was replaced with a slow evaporation cap. Well-formed X-ray quality orange crystals of 1·7MeCN grew over 12 days. These were collected by filtration, washed with Et2O, and dried under vacuum; the yield was ~9% based on Fe. Selected IR data (KBr pellet, cm−1): 3439(w), 1592(w), 1484(m), 1437(m), 1400(m), 1385(m), 1311(w), 1127(s) 1044(s), 1022(s), 996(s), 925(w), 754(s), 727(s), 693(s), 558(s), 532(s), 471(m), 413(m). Elemental analysis: Calc (Found) for 1·½MeCN (C127H122.5N1.5Fe8P10O30): C 52.49 (51.95), H 4.25 (4.32), N 0.72 (0.85)%.

2.1.2. [Fe12Ca4O10(O2CPh)20(hmp)4] (2)

To a stirred solution of Ca(NO3)2·4H2O (0.030 g, 0.125 mmol), hmpH (0.055 g, 0.50 mmol) and NEt3 (0.350 mL, 0.25 mmol) in MeCN/MeOH (11 mL; 10:1 v/v) was added as a solid [Fe3O(O2CPh)6(H2O)3](NO3) (0.13 g, 0.125 mmol), resulting in a brown slurry. The mixture was heated in a microwave reactor for 20 min at 130 °C, and the resulting dark red solution was filtered, and the filtrate was left undisturbed at ambient temperature. After 3–5 days, X-ray quality red block crystals of 2·(solv) had formed. These were collected by filtration, washed with MeCN and Et2O, and dried under vacuum; the yield was ~10% based on Fe. Selected IR data (KBr, cm−1): 3422(br), 2934(w), 1601(s), 1545(s), 1405(vs), 1069(m), 1047(m), 764(w), 718(s), 678(m), 578(w), 464(m). Elemental analysis: Calc (Found) for 2·2H2O (C164H128N4Ca4Fe12O56): C 50.75 (50.47), H 3.32 (3.37), N 1.44 (1.49)%.

2.1.3. [Fe12La4O10(OH)4(tbb)24] (3)

Method A. To a stirred colourless solution of (tbbH) (0.71 g, 4.0 mmol) in benzonitrile (PhCN) (10 mL) in a microwave reaction vial was added NEt3 (0.56 mL, 4.0 mmol) followed by Fe(NO3)3·9H2O (0.40, 1.0 mmol) and La(NO3)3·6H2O (0.43, 1.0 mmol) was added, resulting in a brown solution. This was stirred for a further 5 min at room temperature and then the vial was sealed and heated at 140 °C in a microwave reactor for 1 h. After cooling to room temperature, the vial was removed from the microwave reactor, and the obtained near-black solution was mixed with CH2Cl2 (5 mL) and then filtered to remove any undissolved solids. The filtrate was layered with MeCN and left undisturbed in a sealed vial at ambient temperature for 3 days, during which time orange-red crystals of 3·5PhCN had formed. These were collected by filtration, washed with Me2CO, and dried under vacuum; the yield was ~15% based on Fe. Selected IR data (KBr, cm−1): 3422(br), 2362(m), 2336(m), 1611(w), 1592(m), 1534(m), 1412(br), 784(m), 711(m), 590(m), 543(m), 468(m), 427(m). Elemental analysis: Calc. (Found) for 3·5PhCN·2H2O (C299H345N5Fe12La4O64): C, 57.38 (57.13); H, 5.56 (5.37); N, 1.12 (0.91)%.
Method B. The above procedure was repeated in MeCN (15 mL) as a solvent instead of PhCN. After cooling the microwave reaction vial to ambient temperature, a yellow-orange precipitate was collected by filtration and washed with MeCN. It was dissolved in CH2Cl2 (10 mL) and layered with an equal volume of EtOH. After two days, X-ray quality orange-red crystals had grown, and these were collected by filtration, washed with Me2CO, and dried under vacuum. The product was confirmed to be 3 by infrared spectral comparison with the product from Method A. The yield was ~45% based on Fe. Elemental analysis: Calc. (Found) for 3·4H2O (C264H324O66Fe12La4): C, 54.87 (54.95); H, 5.65 (5.73); N, 0.00 (0.0)%.

2.2. X-ray Crystallography

Single-crystal X-ray data were collected at 100 K on a Bruker Dual micro source D8 Venture diffractometer and PHOTON III detector running APEX4 software package of programs and using MoKα radiation (λ = 0.71073 Å). The data frames were integrated, multi-scan scaling was applied, and the intrinsic phasing structure solution provided all the non-H atoms. The structures were refined using full-matrix least-squares cycles [71]. Non-H atoms were refined with anisotropic displacement parameters, and all H atoms were placed in calculated, idealized positions and refined riding on their parent atoms. The refinements were carried out on F2 by minimizing the wR2 function; R1 is calculated to provide a reference to the conventional R value but its function was not minimized (Table 1).
For 1·7MeCN, the asymmetric unit consists of a complete Fe8 cluster anion, one NHEt3+ cation, and seven MeCN solvent molecules disordered over 9 positions. The cluster has one disordered phenyl ring and was refined in two parts; partial H2O solvent molecules accompany the disorder. The solvent molecules were too disordered to be properly refined, and thus the program SQUEEZE/PLATON [72,73] was applied to remove the solvent contribution to the total diffraction intensity of 5728 Å3 and 1312 electrons per cell. Five hydroxyl protons were obtained from a Difference Fourier map and refined freely, H5, H6, H7, H8 and H111. In the final cycle of refinement, 50,378 reflections (of which 35,688 are observed with I > 2σ(I)) were used to refine 1646 parameters, and the resulting R1, wR2, and S (goodness of fit) were 4.49%, 9.90%, and 1.015, respectively.
For 2·(solv), the asymmetric unit consists of a half Fe12Ca4 cluster located on an inversion center and a mixture of disordered MeCN and MeOH solvent molecules accounting for the removal of 254 electrons per cell and a total void of 1256 Å3. The cluster exhibits a disorder over three iron centers where part one has two coordinated two 2-hydroxymethylpyridine and benzoate ligands and partial methanol and acetonitrile solvent molecules. In the final cycle of refinement, 21,402 reflections (of which 17,520 are observed with I > 2σ(I)) were used to refine 868 parameters, and the resulting R1, wR2, and S (goodness of fit) were 5.89%, 15.00%, and 1.107, respectively.
For 3·5PhCN, the asymmetric unit consists of a half Fe12La4 cluster lying on an inversion center and three PhCN molecules. Most of the cluster ligands and two of the PhCN molecules are disordered to various degrees, and each was refined in two positions. The third PhCN was present at only 50% occupancy, giving a total of 5 PhCN per cluster. In the final cycle of refinement, 28,232 reflections (of which 23,130 are observed with I > 2σ(I)) were used to refine 1825 parameters and the resulting R1, wR2, and S (goodness of fit) were 4.72%, 11.52%, and 1.090, respectively.

2.3. Physical Measurements

Infrared spectra in the 400–4000 cm−1 range were recorded in the solid state (KBr pellets) using a Nicolet iS5 FTIR spectrometer. Elemental analyses (C, H, and N) were performed by Atlantic Microlabs in Norcross, GA, USA. Metal oxidation states were determined from bond valence sum (BVS) calculations [74,75]. Variable temperature dc and ac magnetic susceptibility data were collected on vacuum-dried samples using a Quantum Design MPMS-XL superconducting quantum interference device (SQUID) magnetometer, capable of operating with applied dc fields up to 7 T. Microcrystalline samples were restrained in solid eicosane to prevent torquing. Dc magnetic susceptibility data were collected under a constant 0.1 T applied field in the 5.0–300 K temperature range. Ac magnetic susceptibility studies were performed using a 3.5 G applied ac field in frequencies up to 1000 Hz and in the 1.8–15 K range. Pascal’s constants were used to estimate the diamagnetic correction, and eicosane and gel capsule contributions were measured as a blank. These values were subtracted from the experimental susceptibility to provide the molar paramagnetic susceptibility (χM) [76].

2.4. Theoretical Calculations

DFT calculations on Fe12La4 complex 3 were performed using the crystal structure coordinates. A total of 24 distinct Jij nearest-neighbour exchange couplings were determined from DFT calculations by mapping broken-symmetry solutions to Ising-type spin configurations, S . The employed configurations were one high spin (all spins parallel), all 12 possible single-spin inversions, and all 24 nearest-neighbor two-spin inversions, giving a total of 37 broken-symmetry solutions. The energies of these configurations are expressed in terms of a sum over spin interactions (Equation (1)), where ⟨ i j ⟩ stands for all neighbouring ij pairs, Sk = ±5/2 for Fe3+, and E0 is a constant introduced to match the spin model with the DFT energies.
E S =   E 0 − 2 ∑ ⟨ i j ⟩ J i j S i · S j
The energies of all configurations S resulting from the broken spin-symmetry DFT calculations were used as the l.h.s. of Equation (1) to perform a linear fit and determine all the exchange couplings, Jij. This same approach has been successfully used in the literature to determine exchange couplings in multicenter transition metal complexes [77,78,79,80,81]. In our case, the R2 coefficient of the linear regression differs from 1 by less than 10−6, indicating that the magnetization is well localized at the magnetic centers, thus the broken spin-symmetry DFT solutions are reliable representations of the Ising-type model spin configurations. For all cases, the atomic spin populations of the DFT calculations are consistent with the expected broken spin-symmetry configurations.
In all DFT calculations, the hybrid Perdew–Burke–Ernzerhof (PBEh) density functional approximation, an admixture of exactly 25% (Hartree-Fock-type) exchange and 75% PBE exchange, is known to perform well for magnetic exchange couplings [82], and thus was employed. An RMS error of approximately 10%, was determined for the particular case of oxo-bridged Fe2 couplings, as shown for a set of eleven dinuclear Fe3+ complexes [83]. Pople’s all-electron 6-311+G** basis was used for Fe atoms, 6-31G** for lighter elements [84,85,86], and the segmented all-electron relativistically contracted SARC-DKH2 basis for La atoms [87]. In all calculations, scalar relativistic effects were included through the second-order Douglass–Kroll–Hess approximation [88,89,90]. An in-house version of the Gaussian 16 program [91] was used for all broken-symmetry DFT energies obtained, which allowed for spin inversions of the individual magnetic centers to produce a suitable initial guess for self-consistent broken spin-symmetry calculations. No point group symmetry was assumed at any point in the model or the DFT calculations. Self-consistency convergence thresholds of 10−6 Ha = 0.2 cm−1 in the energy and 10−8 in the RMS changes in the density matrix were used in all calculations.

3. Results

3.1. Synthesis

As stated in the introduction, many reactions were explored involving different permutations of metal sources, ligands, and other reaction parameters. Complexes 1–3 were obtained from overall similar reaction systems that nevertheless had some distinct differences: the FeIII source was either Fe(NO3)3 or the preformed trinuclear [Fe3O(O2CPh)6(H2O)3]+ cluster; the peripheral ligands were either carboxylates or pseudo-carboxylate Ph2PO2− groups; the reactions were homo- or heterometallic; the chelate hmpH was either included or not; the solvents were MeCN, MeCN/MeOH, or PhCN; and the reactions were carried out in the 50–140 °C range under thermal or microwave heating. The overall unifying theme is that 1–3 all contain the same central Fe8 unit. The yields were generally low (~10%), but through crystallographic identification of 3 we were able to then devise a rational synthesis that greatly increased the yield to ~45%.

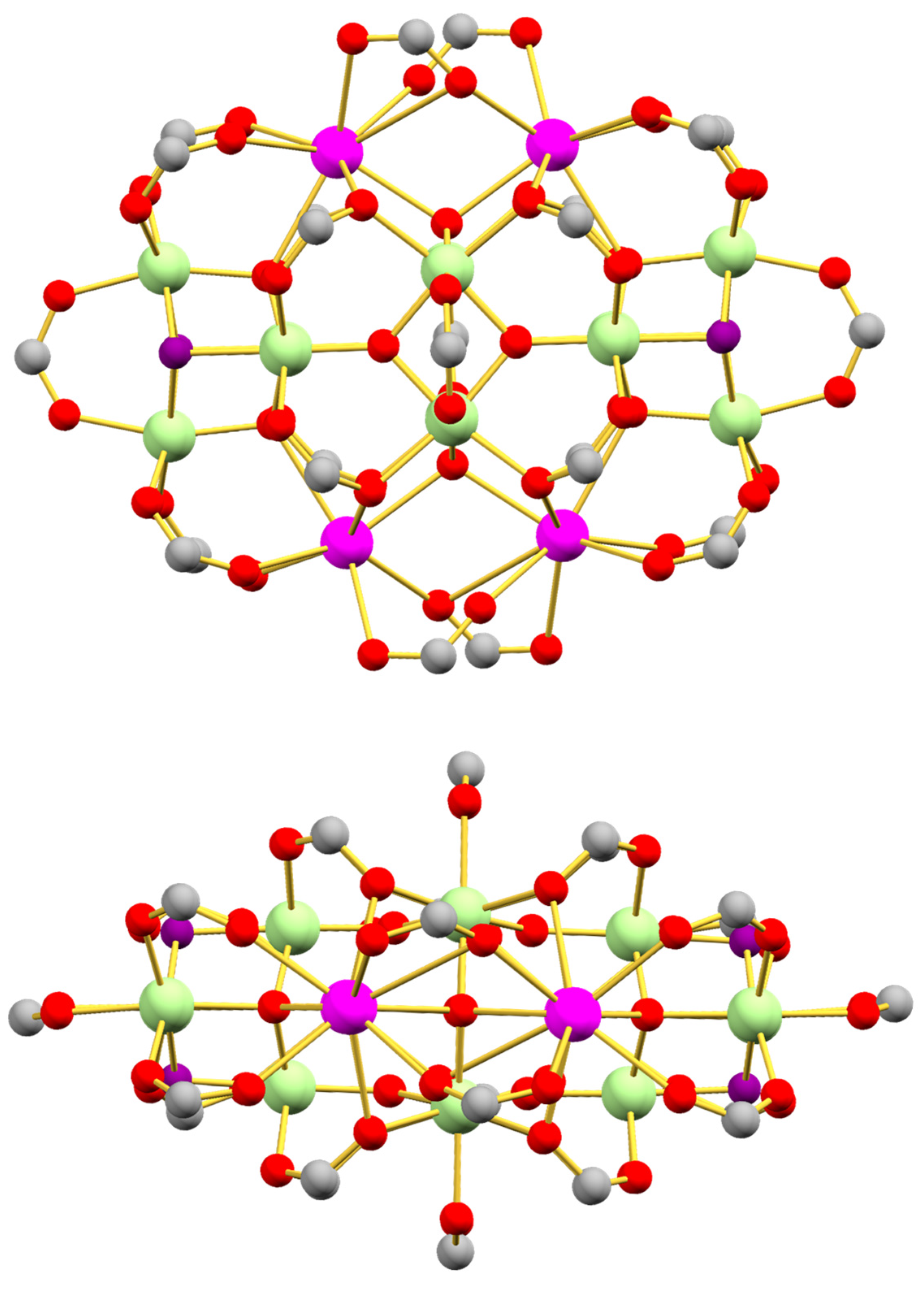
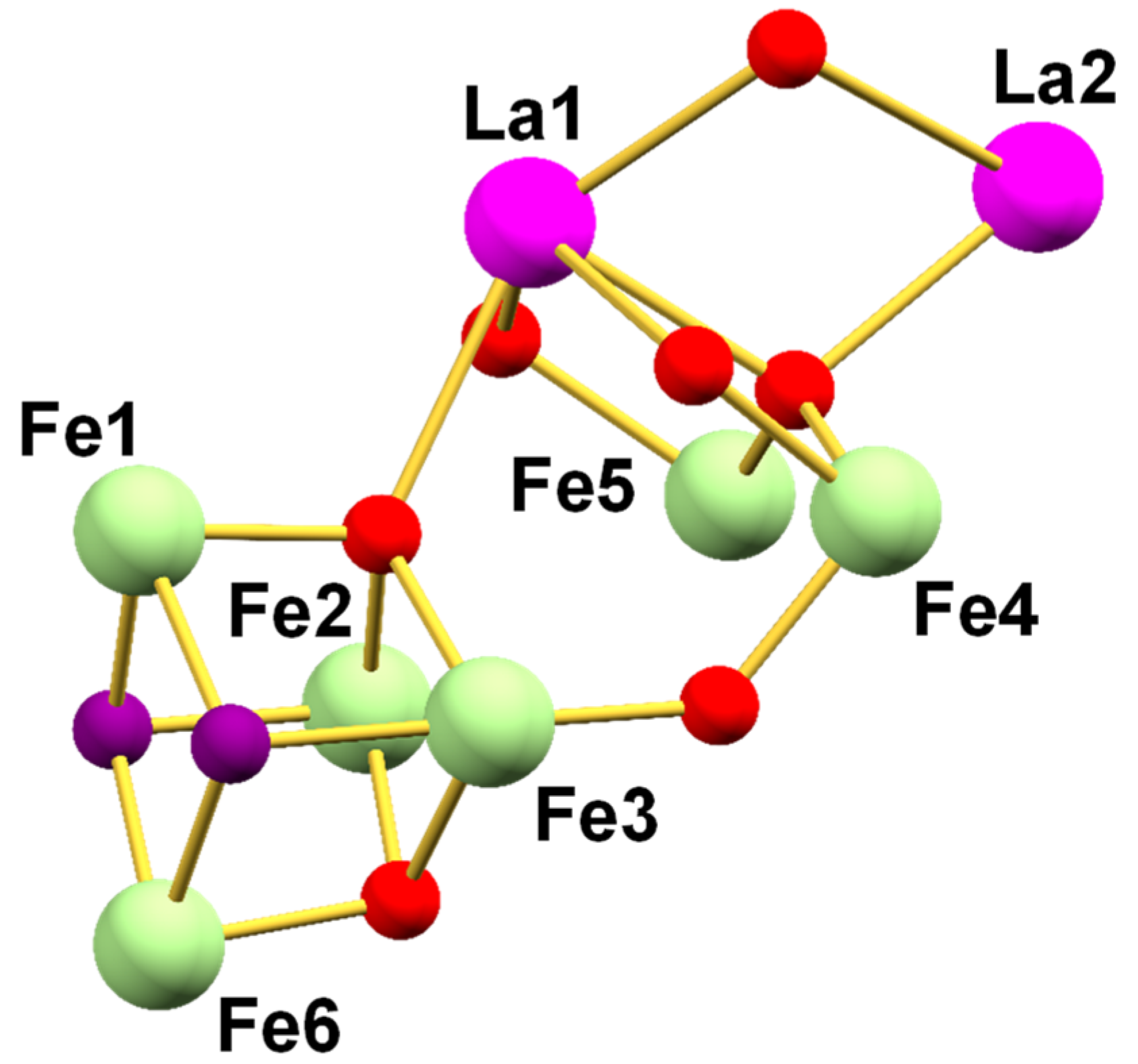
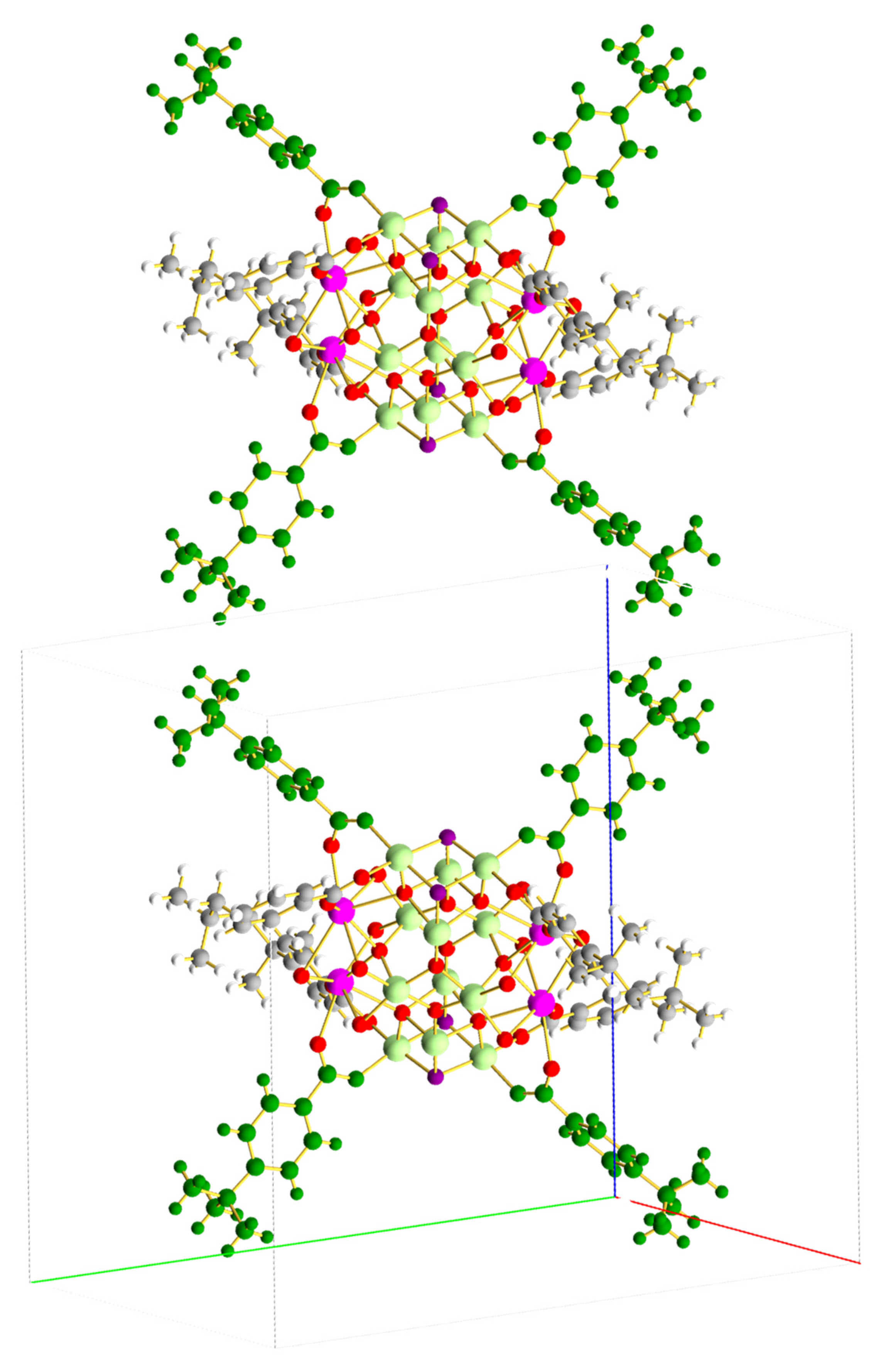
3.2. Description of Structures

Complex 1 crystallizes in the orthorhombic space group Pbca with the asymmetric unit containing the complete Fe8 anion. The structure of the latter and its labeled core are shown in Figure 1; a stereopair is provided in Figure S1 (Supplementary Materials). The core consists of two {Fe4(µ3-O)2}8+ butterfly units, common structural units in Fe4 cluster chemistry [28,92,93,94,95,96,97,98,99,100], stacked on top of each other and bridged by one O2− and five HO− ions. The octahedral FeIII oxidation states (Table S1, Supplementary Materials) and the protonation level of core O atoms (Table 2) were confirmed by Fe and O bond valence sum (BVS) calculations, respectively [74,75]; BVS values for all core and ligand O atoms are listed in Table S2. The BVS of O2− ion O112 is 1.55, lower than expected because it is involved in a hydrogen-bond with the NHEt3+ cation (O112···H-N = 2.807(3)Å), akin to a ‘partial-protonation’. Peripheral ligation about the {Fe8O5(OH)5}9+ core is provided by ten η1:η1:μ2-PhPO2− groups, and the complete cation has virtual D2h symmetry, ignoring the disorder and rotation positions of the Ph rings.
Complex 2 crystallizes in the monoclinic space group P21/c with the asymmetric unit containing half the Fe12Ca4 cluster. The structure (without aromatic rings for clarity) from two viewpoints and the partially labeled core are shown in Figure 2; a stereopair of the complete molecule is provided in Figure S2. Additionally, 2 contains the same core of two stacked butterfly units as seen in the anion of 1, but now all its inter-butterfly bridging ions are O2− (i.e., {Fe8O10}4+) because they are attached to additional metal ions: (i) on each end is an attached {Fe2(μ3-OR)2} unit forming an {Fe4O2(μ3-OR)2} cubane, where RO− is the alkoxide arm of an hmp− N,O chelate; and (ii) on each side two seven-coordinate pentagonal bipyramidal Ca2+ ions are attached, each of them connecting to a cubane μ3-O2− ion, making them μ4, and to one of the central μ2-O2− ions bridging two butterfly units, making it a μ4-O2− that bridges two Ca2+ ions. Fe and O BVS calculations were again used to confirm FeIII oxidation states and non-protonated core O2− ions (Table S3). Peripheral ligation is by 4 η1:η3:μ4-hmp−, 12 η1:η1:μ2-PhCO2−, and 8 η1:η2:μ3-PhCO2− groups, the latter providing further linkages between the central {Fe8O10}4+ unit and the Ca2+ ions. Four of the η1:η2:μ3-PhCO2− groups bridge FeCa pairs, two bridge the butterfly ‘body’ Fe2 pairs, and two bridge Ca2 pairs.
Complex 3 crystallizes in the triclinic space group P 1 ¯ with the asymmetric unit containing half the Fe12La4 cluster. The structure (without 4-tBu-Ph groups for clarity) from two viewpoints and the partially labeled core is shown in Figure 3; a stereopair of the complete molecule is provided in Figure S2. Furthermore, 3 contains the same core of two stacked butterfly units as seen in 2 and the anion of 1, and its overall structure is similar to that of 2 except for the following: (i) four nine-coordinate tricapped trigonal prismatic La3+ ions have replaced the four Ca2+; and (ii) the cubanes at each end are now {Fe4O2(μ3-OH)2} with an η1:η1:μ2-RCO2−, instead of the two chelating/bridging hmp− groups. FeIII oxidation states and protonation levels of core O2−/HO− ions were again confirmed by BVS calculations (Table 2 and Table S4). Peripheral ligation is by 14 η1:η1:μ2-tBuPhO2−, 8 η1:η2:μ3-tBuPhO2−, and 2 η1:η2:μ2-tBuPhO2− groups, which are disposed as for 2, except that owing to the higher coordination number of La3+ vs. Ca2+, the La2 pairs on each side are now each bridged by two carboxylates, one η1:η1:μ2-tBuPhO2− and the other η1:η2:μ3-tBuPhO2−.
The degree of similarity between the Fe8 core of 1 and those within the cores of 2 and 3 was assessed by carrying out root-mean-square-difference (RMSD) calculations for the cores of 1 vs. 2 and 1 vs. 3. The results are listed in Tables S5 and S6, respectively, and shown pictorially in Figure 4. The RMSD values are only 0.096 and 0.109 Å, respectively, and the overall conclusion is therefore that the Fe8 units within the three compounds are essentially superimposable.

3.3. SQUID Magnetometry

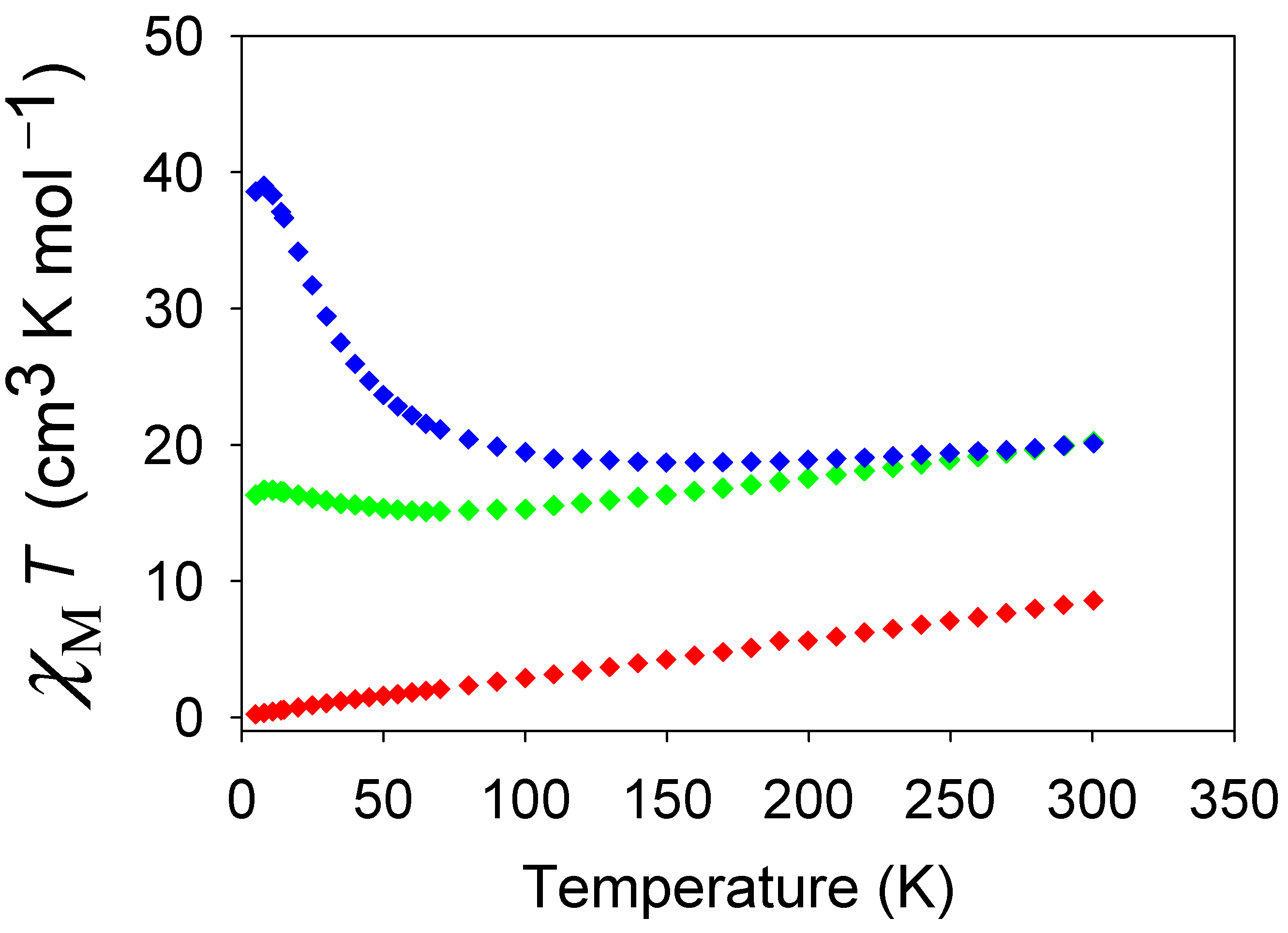
3.3.1. Dc Magnetic Susceptibility Studies

Solid-state, variable-temperature dc magnetic susceptibility (χM) data were collected on vacuum-dried microcrystalline samples of 1·½MeCN, 2·2H2O and 3·4H2O, restrained in eicosane to prevent torquing, in a 1.0 kG (0.10 T) magnetic field and a 5.0 to 300 K temperature range. The data are plotted as χMT vs. T in Figure 5.
For 1·½MeCN, χMT decreases monotonically and near-linearly from 8.57 cm3 K mol−1 at 300 K to 0.22 cm3 K mol−1 at 5.0 K. The 300 K value is much lower than the spin-only (g = 2.0) value of 35.0 cm3 K mol−1 for eight non-interacting Fe3+ ions (S = 5/2) indicates strong antiferromagnetic (AF) interactions within the cluster, and the 5.0 K value and plot profile indicate an S = 0 ground state.
For 2·2H2O, χMT decreases from 20.23 cm3 K mol−1 at 300 K to a minimum of 15.09 cm3 K mol−1 at 65 K and then increases to a maximum of 16.68 cm3 K mol−1 at 8.0 K before a final slight decrease to 16.31 cm3 K mol−1 at 5.0 K (Figure 5). The 300 K value is again much smaller than the spin-only value for twelve non-interacting Fe3+ ions of 52.50 cm3 K mol−1 indicating strong AF interactions. Given the structural similarity between the three clusters, it is reasonable to propose that 2 consists of a strongly AF central Fe8 unit with an S = 0 local ground state, as seen for the anion of 1, and a Fe2 pair at each end that is responsible for the observed χMT at the lowest temperatures. Entertaining this possibility further, the 16.68 cm3 K mol−1 at 8.0 K would be consistent with four non-interacting Fe3+ ions (spin-only χMT = 17.5 cm3 K mol−1), suggesting little or no interaction within each Fe2 pair. This possibility will be assessed further below (vide infra).
For 3·4H2O, χMT decreases from 20.11 cm3 K mol−1 at 300 K to a minimum of 18.71 cm3 K mol−1 at 150 K and then increases to a maximum of 39.01 cm3 K mol−1 at 8.0 K before a final drop to 38.59 cm3 K mol−1 at 5.0 K (Figure 5). The 300 K value is similar to that for 2·2H2O, and indeed the χMT vs. T profiles of the two complexes are somewhat similar except that χMT for 3·4H2O increases to much higher values at the lowest T. Based on the proposed explanation for the χMT vs. T profile for 2·2H2O, we suggest that the coupling within the Fe2 pairs at each end is now ferromagnetic (F), leading to each Fe2 having an S = 5 ground state. However, the spin-only χMT for two independent S = 5 units is 30.0 cm3 K mol−1, significantly below the 8.0 K value. The latter is more consistent with two S = 6 units (spin-only χMT = 42.0 cm3 K mol−1) but this seemed very unlikely, and it was clear that additional studies were necessary to resolve this problem (vide infra).

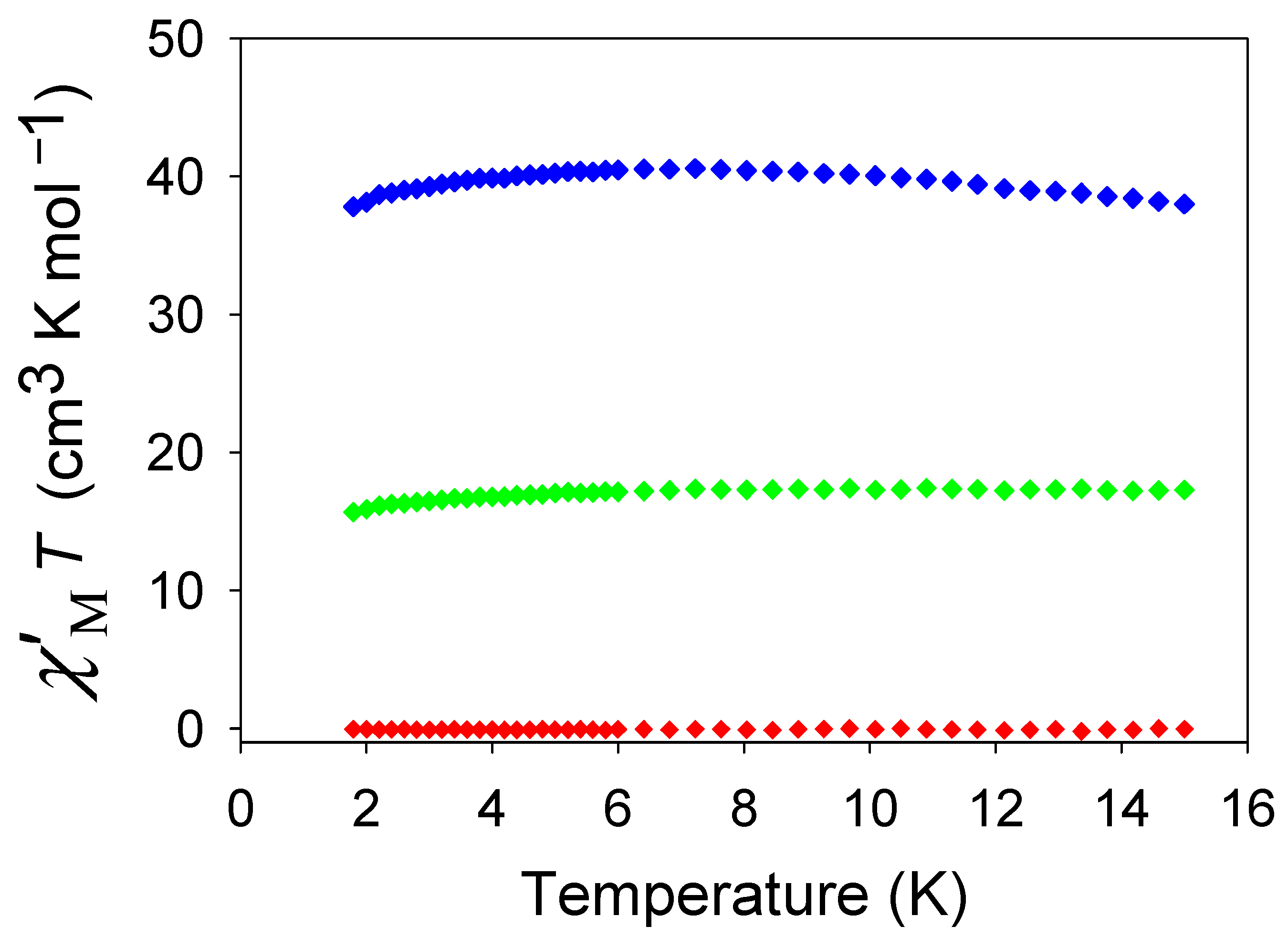
3.3.2. Ac Magnetic Susceptibility Studies

To remove the possibility of any complicating effect of the dc field on the lowest T data, especially when studying complexes with some very weak couplings, alternating current (ac) magnetic susceptibility studies were carried out in the 1.8–15.0 K range using a 3.5 G ac field at a 1000 Hz oscillation frequency, and with no applied dc field. The obtained ac in-phase (χ′M) susceptibility of the three complexes is plotted as χ′MT vs. T in Figure 6. For 1·½MeCN, χ′MT is essentially zero below 15.0 K, confirming a well-isolated S = 0 ground state spin as deduced from the dc data. For 2·H2O, χ′MT is essentially constant below 15.0 K at ~17.0 cm3 K mol−1, in agreement with the dc data suggesting four non-interacting Fe3+ ions. For 3·4H2O, we were very interested to see that χ′MT agreed with the dc χMT data, with a plateau value at 6–10 K of ~40.5 cm3 K mol−1, confirming that the surprisingly high value is not an artifact of the dc field.

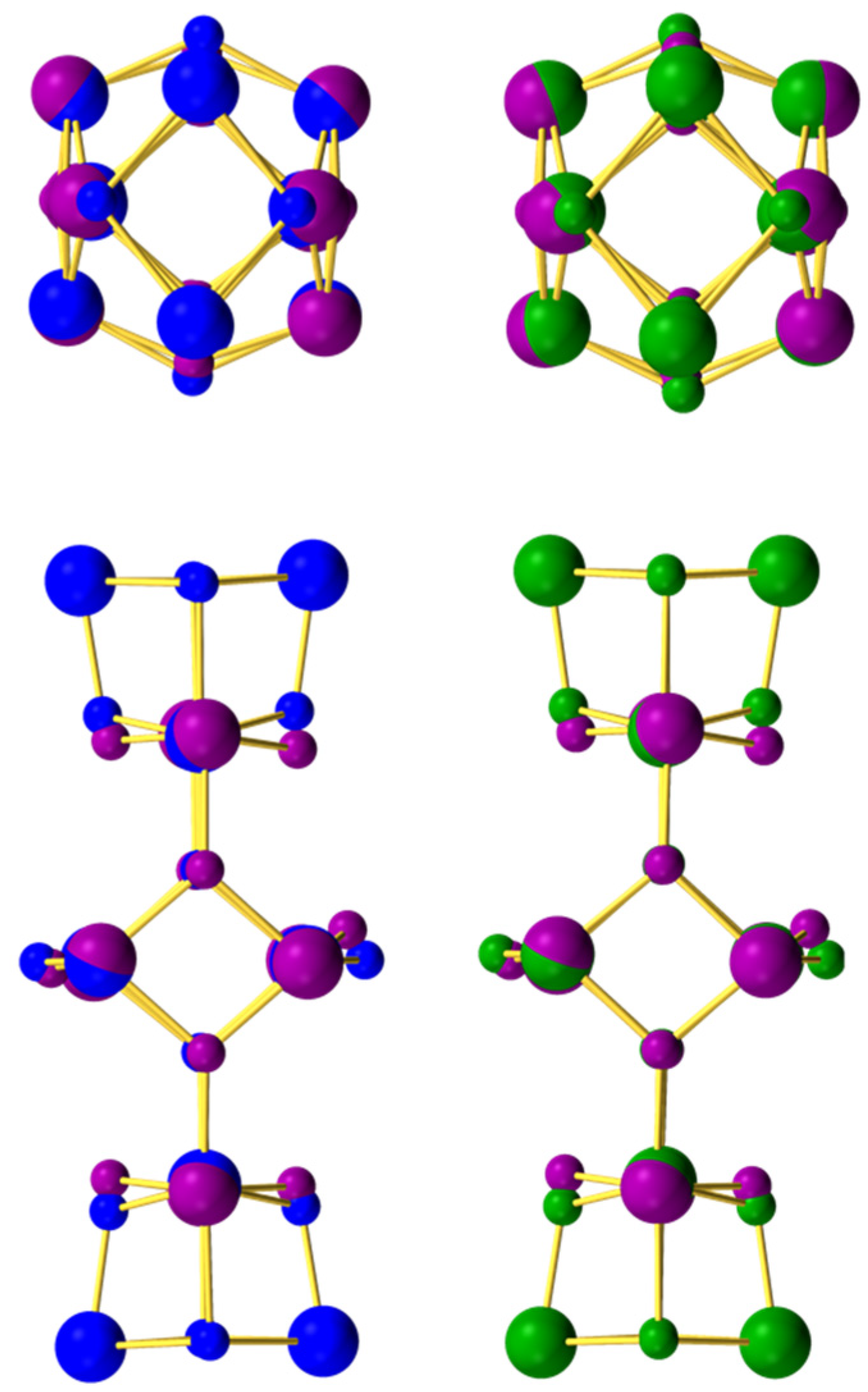
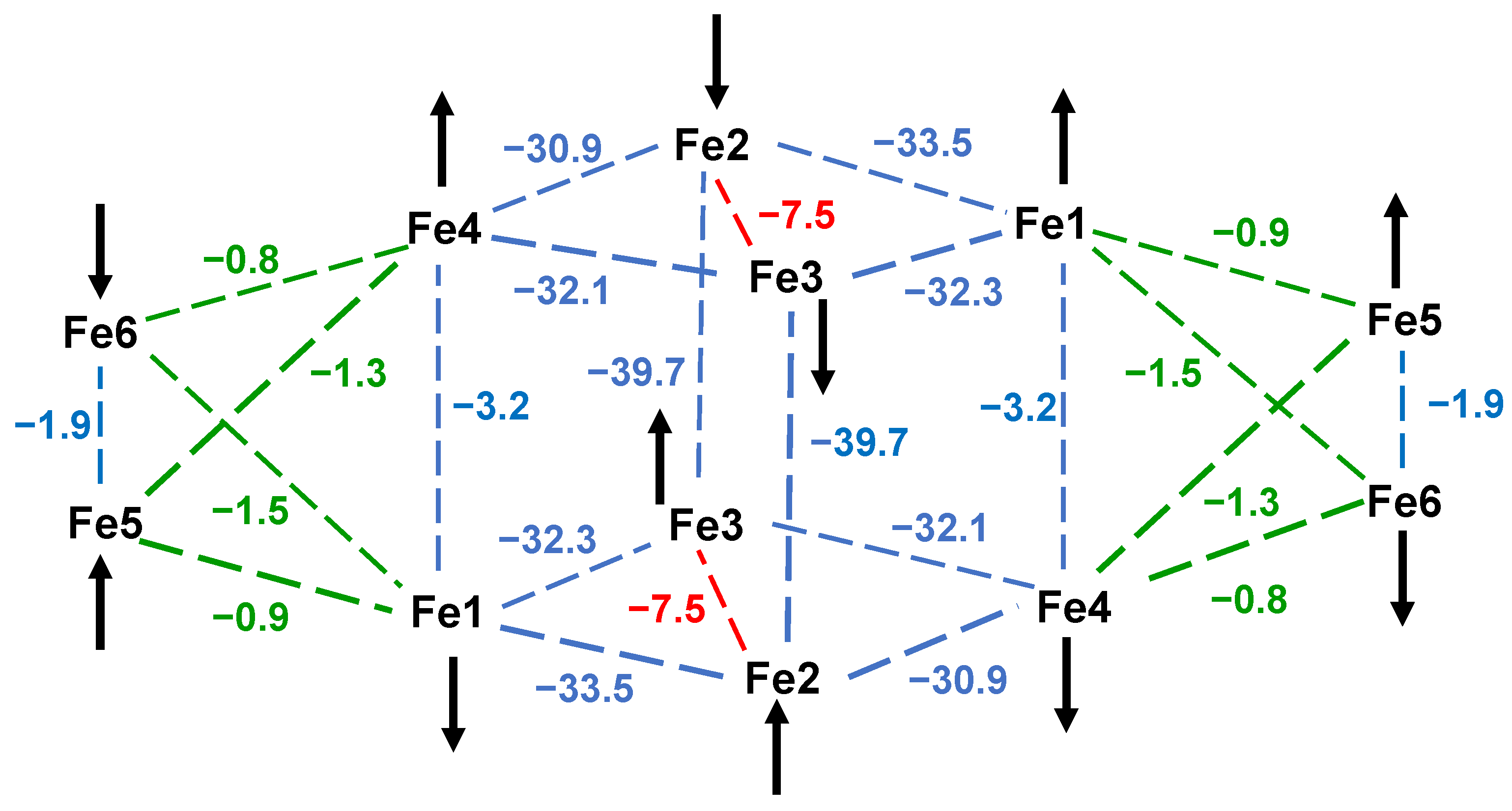
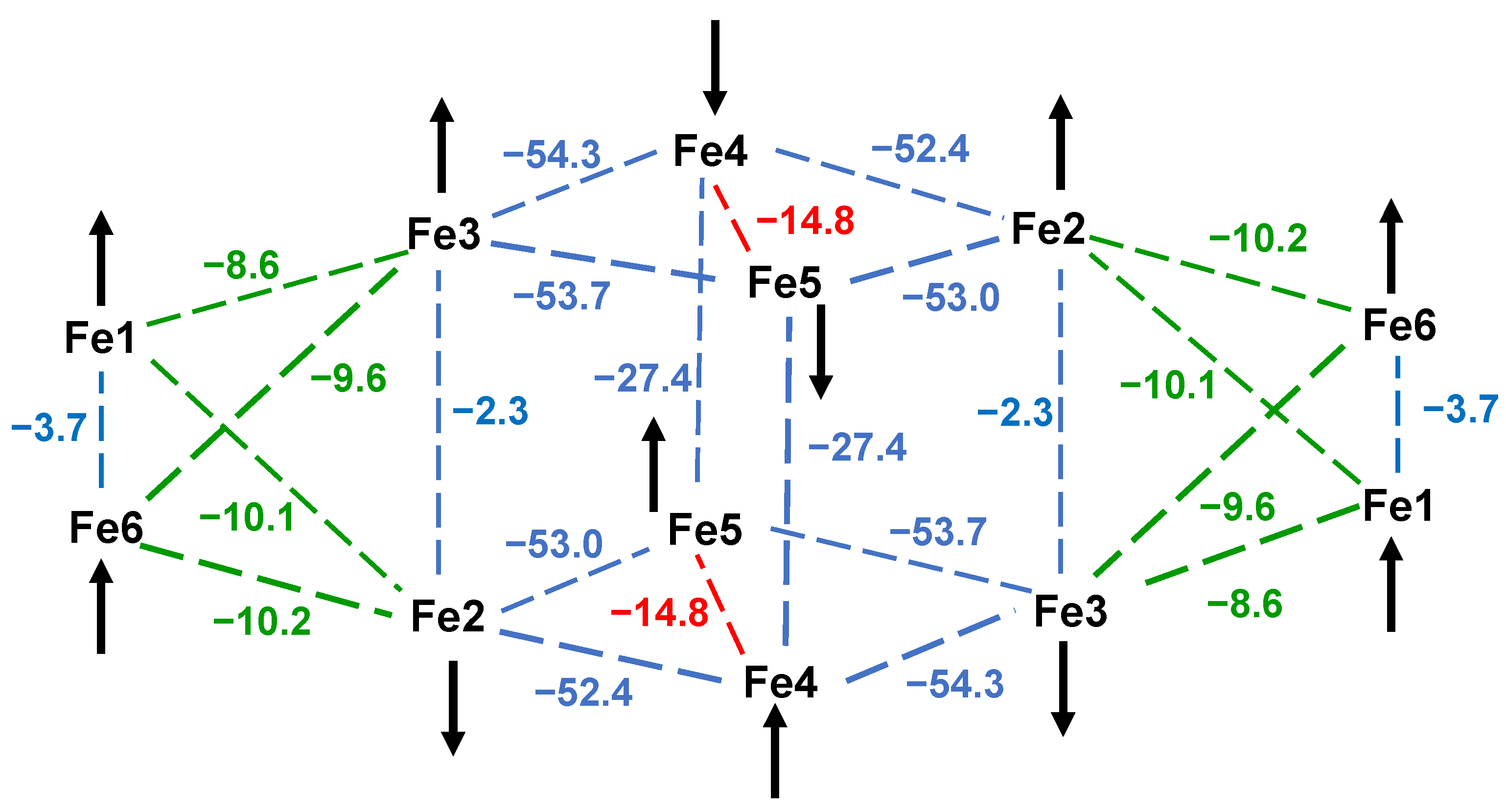
3.3.3. Ground State Spin Rationalization Using a Magnetostructural Correlation (MSC)

Generally, a fit of magnetic susceptibility data is used to assess coupling constants between magnetic ions in cluster chemistry; however, high nuclearity clusters are difficult to simulate and the experimental data is difficult to fit. Therefore, a more quantitative rationalization of the magnetic data requires attainment of the constituent pairwise Fe2 exchange interactions, Jij, within the three clusters. However, given their high nuclearity, low symmetry, and many symmetry-inequivalent Jij even for the anion of 1, we could not obtain them from fits of experimental data. We thus employed the magnetostructural correlation (MSC) that we developed specifically for high nuclearity FeIII/oxo clusters, which yields estimates of the Jij couplings from Fe-O-Fe angles (ϕ) and average Fe-O bond lengths (r) for each Fe2 pair [101]. The MSC (Equation (2)) is based on the angular overlap model and the H = −2JijŜi·Ŝj convention.
J = ( 1.23 × 109 ) ( − 0.12 + 1.57 c o s ϕ + c o s 2 ϕ ) e x p ( − 8.99 r )
The Fe-O and Fe-O-Fe values for each Fe2 pair were used to generate the JMSC values for 1–3, and these are listed in Table 3. For comparison, we also carried out DFT calculations on representative 3 using the broken-symmetry approach, and the resulting JDFT are provided in Table 3. Because of the very similar Fe8 units in 1–3, we did not carry out DFT calculations on 1 and 2.
Elucidating the magnetic properties of the Fe8 anion of 1 is also important in allowing interpretation of the magnetic properties of the larger Fe12 cores of 2 and 3 that contain an Fe8 sub-unit. The JMSC for the anion of 1 separates into three groups: weak, strong, and very strong. Within each Fe4 butterfly, the body-body (Jbb) interactions (Fe2Fe4 and Fe6Fe8) are weak (−8.8 and −9.9 cm−1, respectively), as expected for bis-monoatomically bridged Fe2 pairs with their smaller Fe-O-Fe angles (<100°) [13,39,51,102]. In contrast, the wingtip-body (Jwb) interactions within each butterfly are strong (−20.9 to −29.4 cm−1), reflecting their single monoatomic bridge and consequently larger angles (127–132°). Since each butterfly unit comprises two edge-fused Fe3 triangles and all the intra-butterfly interactions are AF, there will be spin frustration effects operating (competing exchange interactions). However, within each Fe3 triangle, the one weak Jbb is competing with two strong Jwb so the former is completely frustrated and the Jwb are satisfied, i.e., the spin vector alignments are determined only by the Jwb (Figure 7). There are four inter-butterfly interactions, two of which (Fe1Fe5 and Fe3Fe7) are again weak (−5.4 and −2.3 cm−1, respectively) due to being bis-monoatomically bridged. The third is Fe2(μ2-OH)Fe6 and is strong (−24.7 cm−1), whereas the fourth is Fe4(μ2-O)Fe8 and is very strong (−51.7 cm−1), the difference assignable to the latter’s shorter Fe-O bonds (av. 1.855 Å) compared with the former’s Fe-OH bonds (av. 1.936 Å) since the Fe-O-Fe angles are similar (138.63 vs. 134.75°, respectively). The inter-butterfly interactions are not competing with each other nor the intra-butterfly ones, and they are therefore all satisfied, even the weakest ones. This provides the overall spin vector alignments shown in Figure 7, rationalizing the experimentally observed S = 0 ground state.
The JMSC of the central Fe8 subunit of 2 shows that the Jbb (Fe2Fe3) are again weak (−7.5 cm−1) and the Jwb are again strong (−28.1 to −33.5 cm−1), slightly stronger than those for 1. The latter is assigned to the extra Fe3+ and Ca2+ ions affecting the Fe-O bond lengths in 2; for example, the average wingtip Fe-μ3-O2− lengths decrease from 1.924 Å in 1 to 1.853 Å in 2, giving stronger Jwb in 2. The central Fe8 of 2 should thus have an S = 0 local ground state (Figure 8), analogous to 1, and the overall ground state is thus determined by the intra-Fe2 coupling within the Fe2 pairs at each end. If each intra-Fe2 coupling were AF, as shown arbitrarily in Figure 8, then 2 would have an overall S = 0 ground state, which it clearly does not; both the dc and ac data indicate four essentially non-interacting Fe3+ ions. In fact, this is consistent with the very weak JMSC value J56 = −1.9 cm−1 (Table 3), which is within experimental error of zero. Note also that the whole molecule behaves, at low T, as two Fe2 pairs separated by a diamagnetic Fe8 ‘bridge’, so the JMSC couplings between Fe2 pairs and Fe8 ions are moot (Figure 8).
The JMSC and JDFT for 3 are overall in satisfying agreement (Table 3) and thus provide independent support for each other. For both, the Jbb are stronger than those for 2, and we assign this to an even bigger effect of the La3+ on the Fe8 structural parameters than the Ca2+. For example, the average wingtip Fe-μ3-O2− lengths are 1.924 Å, 1.853 Å, and 1.845 Å in 1–3, respectively, and although those for 2 and 3 are similar, their average body Fe-μ3-O2− lengths are very different at 1.958 Å and 1.908 Å, respectively, rationalizing the stronger couplings in 3. The central Fe8 of 3 should again have an S = 0 local ground state (Figure 9), whereas as for 2, the overall ground state is again determined by the intra-Fe2 coupling within Fe2 pairs (Fe1Fe6) at each end, for which JMSC and JDFT values are very weakly AF (−3.7 and −0.1 cm−1). However, both the dc and ac data clearly indicate their coupling to be F, resulting in S = 5 ground states for both pairs, and showing that their AF JMSC and JDFT values must be artifacts of the very small numbers involved and their inherent uncertainties.
There is still one unexpected experimental observation that needs to be resolved. At low T, 3 can be described as two S = 5 Fe2 pairs separated by a large diamagnetic Fe8 unit, and the inter-Fe2 interaction within each molecule of 3 should therefore be zero and the χMT should be ~30.0 cm3 K mol−1, the spin-only value for two independent S = 5 units. As stated earlier, however, it is instead 39.01 cm3 K mol−1 at 8.0 K, significantly greater than expected. After close examination of the crystal packing, we assign this to inter-Fe2 interactions between adjacent molecules of 3, i.e., intermolecular interactions.
The packing shows that 4-tBu-benzoate groups on one molecule of 3 lie essentially perpendicular to those on the adjacent molecule, and this is true for all the nearest-neighbours of a particular molecule. One such pair of molecules showing two of the near-perpendicular pairs of ligands is shown in Figure 10. Since it is well known that significant π-spin density will delocalize from metal dπ orbitals to the para-position of an aromatic ligand, such as benzoate through a π-spin-delocalization mechanism, and then onto any para-substituent with available π-symmetry atomic or molecular orbitals, such as CH3, CR3, Cl, F, etc, then the fact that the two π-systems on the different molecules are near-perpendicular should lead to them being orthogonal and thus provide a resulting F interaction. Its magnitude is expected to be very weak, but since there is a 3D network of such interactions, it should lead to an overall significant contribution to χMT at low T, and this would rationalize the unexpectedly high observed value. Crucially, there are no π-π-stacking interactions between phenyl groups, common in unsubstituted benzoate complexes, that would be expected to provide AF interactions, the bulky para-tBu groups preventing close approach of the aromatic rings in 3. Support for the above rationalization includes the intermolecular F interactions seen for a Mn4 complex with 4-tert-butyl-salicylidene-2-ethanolamine ligation, whose tBu-substituted aromatic ligands are also near-perpendicular [103]. Previously reported compounds containing Fe12Ln4 with aromatic ligands also have exhibited unusually high values of χMT at low temperatures, consistent with the observation of intermolecular F interactions for such compounds [54,55].

4. Conclusions

The attainment of a family of three structurally related complexes 1–3 has allowed comparisons and contrasts of their observed magnetic properties and yielded important insights into their origin, including those that at first glance appear surprising, and the effect of the attachment of heterometals Ca2+ and La3+. The presence of spin frustration and its importance in determining the ground states of polynuclear complexes is yet again emphasized, as is the usefulness of a multi-pronged approach to their analysis using experimental data in coordination with estimates of the constituent Jij exchange couplings, using DFT computations and a magnetostructural correlation derived specifically for FeIII-oxo clusters.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/chemistry5030110/s1, Figures S1–S3: Stereopair of the complete anion of 1, complex 2 and complex 3, respectively; Figure S4: Expanded version of Figure 10 showing the near-perpendicular alignment of 4-tBu-benzoate ligands for four molecules of 3. The a axis is the red line, b axis is the lime green line, and the c axis is the blue line; Figures S5–S7: Infrared spectrum of 1, 2, and 3, respectively; Table S1: Bond valence sums and assignments for the Fe atoms in the asymmetric unit of 1; Table S2: Bond valence sums and assignments for O atoms in the cation of 1; Table S3: Bond valence sums and assignments for the Fe and O atoms in the asymmetric unit of 2; Table S4: Bond valence sums and assignments for the Fe and O atoms in the asymmetric unit of 3; Table S5: RMSD calculations for 1 with 2; Table S6: RMSD calculations for 1 with 3. CCDC 2276564 contains supplementary crystallographic data for 1. CCDC 2276685 contains supplementary crystallographic data for 2. CCDC 2276660 contains supplementary crystallographic data for 3.

Author Contributions

Conceptualization, G.C.; methodology, A.P.S., C.L.B. and K.H.K.L.; software, A.P.S., C.L.B., K.H.K.L. and J.E.P.; validation, G.C.; formal analysis, A.P.S., C.L.B., K.H.K.L. and K.A.A.; investigation, C.L.B., A.P.S. and K.H.K.L.; resources, G.C. and J.E.P.; data curation, J.E.P., A.P.S., C.L.B., K.A.A. and K.H.K.L.; writing—original draft preparation, A.P.S., C.L.B. and K.H.K.L.; writing—review and editing, G.C., A.P.S., C.L.B. and K.H.K.L.; visualization, K.A.A., C.L.B. and K.H.K.L.; supervision, G.C.; project administration, G.C.; funding acquisition, G.C. All authors have read and agreed to the published version of the manuscript.

Funding

This work was supported by the U.S. National Science Foundation (Grant CHE-1900321), and by the Department of Energy, Office of Science, Office of Basic Energy Sciences, as part of the Computational Chemical Sciences Program under Award #DE-SC0018331. We thank the U.S. National Science Foundation for funding of the X-ray diffractometer at the University of Florida through grant CHE-1828064.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

The crystallographic data can be obtained free of charge via http://www.ccdc.cam.ac.uk/conts/retrieving.html (accessed on 30 June 2023), or from the Cambridge Crystallographic Data Centre, 12 Union Road, Cambridge CB2 1EZ, UK; fax: (+44) 1223-336-033; or e-mail: [email protected].

Conflicts of Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

References

  1. Bagai, R.; Christou, G. The Drosophila of single-molecule magnetism: Mn12O12(O2CR)16(H2O)4. Chem. Soc. Rev. 2009, 38, 1011–1026. [Google Scholar] [CrossRef] [PubMed]
  2. Brimblecombe, R.; Swiegers, G.F.; Dismukes, G.C.; Spiccia, L. Sustained water oxidation photocatalysis by a bioinspired manganese cluster. Angew. Chem. Int. Ed. Engl. 2008, 47, 7335–7338. [Google Scholar] [CrossRef]
  3. Maayan, G.; Gluz, N.; Christou, G. A bioinspired soluble manganese cluster as a water oxidation electrocatalyst with low overpotential. Nat. Cat. 2018, 1, 48–54. [Google Scholar] [CrossRef]
  4. Chakraborty, R.; Das Kumar, B. Cobalt(III)-oxo cubane clusters as catalysts for oxidation of organic substrates. J. Chem. Sci. 2011, 123, 163–173. [Google Scholar]
  5. Vincent, J.B.; Huffman, J.C.; Christou, G.; Li, Q.; Nanny, M.A.; Hendrickson, D.N.; Fong, R.H.; Fish, R.H. Modeling the dinuclear sites of iron biomolecules: Synthesis and properties of Fe2O(OAc)2Cl2(bipy)2 and its use as an alkane activation catalyst. J. Am. Chem. Soc. 1988, 110, 6898–6900. [Google Scholar] [CrossRef]
  6. Seddon, E.J.; Yoo, J.; Folting, K.; Huffman, J.C.; Hendrickson, D.N.; Christou, G. Dinuclear and hexanuclear iron(III) carboxylate clusters with a bis(bipyridine) ligand: Supramolecular aggregation of [Fe2O2] units to give a [Fe6O6] ladder structure. J. Chem. Soc. Dalton Trans. 2000, 3640–3648. [Google Scholar] [CrossRef]
  7. Grant, C.M.; Knapp, M.J.; Streib, W.E.; Huffman, J.C.; Hendrickson, D.N.; Christou, G. Dinuclear and Hexanuclear Iron(III) Oxide Complexes with a Bis(bipyridine) Ligand:  A New [Fe6(μ3-O)4]10+ Core. Inorg. Chem. 1998, 37, 6065–6070. [Google Scholar] [CrossRef]
  8. Zheng, H.; Yoo, S.J.; Münck, E.; Que, L. The Flexible Fe2(μ-O)2 Diamond Core:  A Terminal Iron(IV)−Oxo Species Generated from the Oxidation of a Bis(μ-oxo)diiron(III) Complex. J. Am. Chem. Soc. 2000, 122, 3789–3790. [Google Scholar] [CrossRef]
  9. Zang, Y.; Dong, Y.; Que, L.; Kauffmann, K.; Muenck, E. The First Bis(.mu.-oxo)diiron(III) Complex. Structure and Magnetic Properties of [Fe2(μ-O)2(6TLA)2](ClO4). J. Am. Chem. Soc. 1995, 117, 1169–1170. [Google Scholar] [CrossRef]
  10. Boudalis, A.K.; Raptopoulou, C.P.; Terzis, A.; Perlepes, S.P. 2,2′-Bipyrimidine (bpym) in iron(III) carboxylate chemistry: Preparation and characterization of [Fe2O(O2CMe)2 (bpym)2(H2O)2](ClO4)2, and the influence of weak interactions in the formation of metal clusters. Polyhedron 2004, 23, 1271–1277. [Google Scholar] [CrossRef]
  11. Zhang, H.-T.; Su, X.-J.; Xie, F.; Liao, R.-Z.; Zhang, M.-T. Iron-Catalyzed Water Oxidation: O–O Bond Formation via Intramolecular Oxo–Oxo Interaction. Angew. Chem. Int. Ed. 2021, 60, 12467–12474. [Google Scholar] [CrossRef] [PubMed]
  12. Zhang, Z.-M.; Yao, S.; Li, Y.-G.; Clérac, R.; Lu, Y.; Su, Z.-M.; Wang, E.-B. Protein-Sized Chiral Fe168 Cages with NbO-Type Topology. J. Am. Chem. Soc. 2009, 131, 14600–14601. [Google Scholar] [CrossRef] [PubMed]
  13. Hale, A.R.; Lott, M.E.; Peralta, J.E.; Foguet-Albiol, D.; Abboud, K.A.; Christou, G. Magnetic Properties of High-Nuclearity Fex-oxo (x = 7, 22, 24) Clusters Analyzed by a Multipronged Experimental, Computational, and Magnetostructural Correlation Approach. Inorg. Chem. 2022, 61, 11261–11276. [Google Scholar] [CrossRef]
  14. Herold, S.; Lippard, S.J. Carboxylate-bridged diiron(II) complexes: Synthesis characterization, and O2-reactivity of models for the reduced diiron centers in methane monooxygenase and ribonucleotide reductase. J. Am. Chem. Soc. 1997, 119, 145–156. [Google Scholar] [CrossRef]
  15. Lee, D.; Lippard, S.J. Structural and functional models of the dioxygen-activating centers of non-heme diiron enzymes ribonucleotide reductase and soluble methane monooxygenase. J. Am. Chem. Soc. 1998, 120, 12153–12154. [Google Scholar] [CrossRef]
  16. Menage, S.; Zang, Y.; Hendrich, M.P.; Que, L. Structure and reactivity of a bis(.mu.-acetato-O,O′)diiron(II) complex, [Fe2(O2CCH3)2(TPA)2](BPh4)2. A model for the diferrous core of ribonucleotide reductase. J. Am. Chem. Soc. 1992, 114, 7786–7792. [Google Scholar] [CrossRef]
  17. Kodera, M.; Itoh, M.; Kano, K.; Funabiki, T.; Reglier, M. A Diiron Center Stabilized by a Bis-TPA Ligand as a Model of Soluble Methane Monooxygenase: Predominant Alkene Epoxidation with H2O2. Angew. Chem. Int. Ed. 2005, 44, 7104–7106. [Google Scholar] [CrossRef]
  18. Xue, G.; Wang, D.; De Hont, R.; Fiedler, A.T.; Shan, X.; Münck, E.; Que, L. A synthetic precedent for the [FeIV2(μ-O)2] diamond core proposed for methane monooxygenase intermediate Q. Proc. Nat. Acad. Sci. USA 2007, 104, 20713–20718. [Google Scholar] [CrossRef]
  19. Costas, M.; Rohde, J.U.; Stubna, A.; Ho, R.Y.N.; Quaroni, L.; Münck, E.; Que, L., Jr. A synthetic model for the putative FeIV2O2 diamond core of methane monooxygenase intermediate Q. J. Am. Chem. Soc. 2001, 123, 12931–12932. [Google Scholar] [CrossRef]
  20. Sankaralingam, M.; Palaniandavar, M. Diiron(III) complexes of tridentate 3N ligands as functional models for methane monooxygenases: Effect of the capping ligand on hydroxylation of alkanes. Polyhedron 2014, 67, 171–180. [Google Scholar] [CrossRef]
  21. Arii, H.; Nagatomo, S.; Kitagawa, T.; Miwa, T.; Jitsukawa, K.; Einaga, H.; Masuda, H. A novel diiron complex as a functional model for hemerythrin. J. Inorg. Biochem. 2000, 82, 153–162. [Google Scholar] [CrossRef]
  22. Mizoguchi, T.J.; Kuzelka, J.; Spingler, B.; DuBois, J.L.; Davydov, R.M.; Hedman, B.; Hodgson, K.O.; Lippard, S.J. Synthesis and spectroscopic studies of non-heme diiron(III) species with a terminal hydroperoxide ligand: Models for hemerythrin. Inorg. Chem. 2001, 40, 4662–4673. [Google Scholar] [CrossRef] [PubMed]
  23. He, C.; Mishina, Y. Modeling non-heme iron proteins. Curr. Opin. Chem. Biol. 2004, 8, 201–208. [Google Scholar] [CrossRef] [PubMed]
  24. Tshuva, E.Y.; Lippard, S.J. Synthetic Models for Non-Heme Carboxylate-Bridged Diiron Metalloproteins: Strategies and Tactics. Chem. Rev. 2004, 104, 987–1012. [Google Scholar] [CrossRef] [PubMed]
  25. Que, L. The Road to Non-Heme Oxoferryls and Beyond. Acc. Chem. Res. 2007, 40, 493–500. [Google Scholar] [CrossRef]
  26. Zhang, X.; Furutachi, H.; Fujinami, S.; Nagatomo, S.; Maeda, Y.; Watanabe, Y.; Kitagawa, T.; Suzuki, M. Structural and Spectroscopic Characterization of (μ-Hydroxo or μ-Oxo)(μ-peroxo)diiron(III) Complexes:  Models for Peroxo Intermediates of Non-Heme Diiron Proteins. J. Am. Chem. Soc. 2005, 127, 826–827. [Google Scholar] [CrossRef] [PubMed]
  27. Foguet-Albiol, D.; Abboud, K.A.; Christou, G. High-nuclearity homometallic iron and nickel clusters: Fe22 and Ni24 complexes from the use of N-methyldiethanolamine. Chem. Commun. 2005, 4282–4284. [Google Scholar] [CrossRef]
  28. McCusker, J.K.; Vincent, J.B.; Schmitt, E.A.; Mino, M.L.; Shin, K.; Coggin, D.K.; Hagen, P.M.; Huffman, J.C.; Christou, G.; Hendrickson, D.N. Molecular spin frustration in the [Fe4O2]8+ core: Synthesis, structure, and magnetochemistry of tetranuclear iron-oxo complex [Fe4O2(O2CR)7(bpy)2](C1O4) (R = Me, Ph). J. Am. Chem. Soc. 1991, 113, 3012–3021. [Google Scholar] [CrossRef]
  29. Kahn, O. Competing spin interactions and degenerate frustration for discrete molecular species. Chem. Phys. Lett. 1997, 265, 109–114. [Google Scholar] [CrossRef]
  30. Gatteschi, D.; Caneschi, A.; Sessoli, R.; Cornia, A. Magnetism of large iron-oxo clusters. Chem. Soc. Rev. 1996, 25, 101–109. [Google Scholar] [CrossRef]
  31. Murch, B.P.; Boyle, P.D.; Que, L. Structures of binuclear and tetranuclear iron(III) complexes as models for ferritin core formation. J. Am. Chem. Soc. 1985, 107, 6728–6729. [Google Scholar] [CrossRef]
  32. Mansour, A.N.; Thompson, C.; Theil, E.C.; Chasteen, N.D.; Sayers, D.E. Fe(III).ATP complexes. Models for ferritin and other polynuclear iron complexes with phosphate. J. Bio. Chem. 1985, 260, 7975–7979. [Google Scholar] [CrossRef]
  33. Islam, Q.T.; Sayers, D.E.; Theil, E.C.; Gorun, S.M. A comparison of an undecairon(III) complex with the ferritin iron core. J. Inorg. Biochem. 1989, 36, 51–62. [Google Scholar] [CrossRef] [PubMed]
  34. Taft, K.L.; Papaefthymiou, G.C.; Lippard, S.J. Synthesis, Structure, and Electronic Properties of a Mixed-Valent Dodecairon Oxo Complex, a Model for the Biomineralization of Ferritin. Inorg. Chem. 1994, 33, 1510–1520. [Google Scholar] [CrossRef]
  35. Lachicotte, R.J.; Hagen, K.S. Synthesis and structures of trinuclear and μ-hydroxo pentanuclear iron(II) carboxylates as models of reduced ferritin and white rust. Inorg. Chimi. Acta 1997, 263, 407–414. [Google Scholar] [CrossRef]
  36. Taguchi, T.; Thompson, M.S.; Abboud, K.A.; Christou, G. Unusual Fe9 and Fe18 structural types from the use of 2,6-pyridinedimethanol in FeIII cluster chemistry. Dalton Trans. 2010, 39, 9131–9139. [Google Scholar] [CrossRef]
  37. Gass, I.A.; Milios, C.J.; Evangelisti, M.; Heath, S.L.; Collison, D.; Parsons, S.; Brechin, E.K. Synthesis and magnetic properties of heptadecametallic Fe(III) clusters. Polyhedron 2007, 26, 1835–1837. [Google Scholar] [CrossRef]
  38. Canada-Vilalta, C.; O’Brien, T.A.; Brechin, E.K.; Pink, M.; Davidson, E.R.; Christou, G. Large spin differences in structurally related Fe6 molecular clusters and their magnetostructural explanation. Inorg. Chem. 2004, 43, 5505–5521. [Google Scholar] [CrossRef]
  39. Singh, A.P.; Joshi, R.P.; Abboud, K.A.; Peralta, J.E.; Christou, G. Molecular spin frustration in mixed-chelate Fe5 and Fe6 oxo clusters with high ground state spin values. Polyhedron 2020, 176, 114182. [Google Scholar] [CrossRef]
  40. King, P.; Stamatatos, T.C.; Abboud, K.A.; Christou, G. Reversible Size Modification of Iron and Gallium Molecular Wheels: A Ga10 “Gallic Wheel” and Large Ga18 and Fe18 Wheels. Angew. Chem. Int. Ed. 2006, 45, 7379–7383. [Google Scholar] [CrossRef]
  41. Artus, P.; Boskovic, C.; Yoo, J.; Streib, W.E.; Brunel, L.C.; Hendrickson, D.N.; Christou, G. Single-molecule magnets: Site-specific ligand abstraction from [Mn12O12(O2CR)16(H2O)4] and the preparation and properties of [Mn12O12(NO3)4(O2CCH2Bu(t))12(H2O)4]. Inorg. Chem. 2001, 40, 4199–4210. [Google Scholar] [CrossRef] [PubMed]
  42. Boskovic, C.; Pink, M.; Huffman, J.C.; Hendrickson, D.N.; Christou, G. Single-molecule magnets: Ligand-induced core distortion and multiple Jahn-Teller isomerism in Mn12O12(O2CMe)8(O2PPh2)8(H2O)4. J. Am. Chem. Soc. 2001, 123, 9914–9915. [Google Scholar] [CrossRef] [Green Version]
  43. Brockman, J.T.; Abboud, K.A.; Hendrickson, D.N.; Christou, G. A new family of Mn12 single-molecule magnets: Replacement of carboxylate ligands with diphenylphosphinates. Polyhedron 2003, 22, 1765–1769. [Google Scholar] [CrossRef]
  44. Chakov, N.E.; Abboud, K.A.; Zakharov, L.N.; Rheingold, A.L.; Hendrickson, D.N.; Christou, G. Reaction of Mn12O12(O2CR)16(H2O)4 single-molecule magnets with non-carboxylate ligands. Polyhedron 2003, 22, 1759–1763. [Google Scholar] [CrossRef]
  45. Chakov, N.E.; Wernsdorfer, W.; Abboud, K.A.; Hendrickson, D.N.; Christou, G. Single-molecule magnets. A Mn12 complex with mixed carboxylate-sulfonate ligation: Mn12O12(O2CMe)8(O3SPh)8(H2O)4. Dalt. Trans. 2003, 2243–2248. [Google Scholar] [CrossRef]
  46. Chakov, N.E.; Wernsdorfer, W.; Abboud, K.A.; Christou, G. Mixed-valence (MnMnIV)-Mn-III clusters Mn7O8(O2SePh)8(O2CMe)(H2O) and Mn7O8(O2SePh)9(H2O): Single-chain magnets exhibiting quantum tunneling of magnetization. Inorg. Chem. 2004, 43, 5919–5930. [Google Scholar] [CrossRef]
  47. Chakov, N.E.; Thuijs, A.E.; Wernsdorfer, W.; Rheingold, A.L.; Abboud, K.A.; Christou, G. Unusual Mn(III/IV)4 Cubane and MnIII16M4 (M = Ca, Sr) Looplike Clusters from the Use of Dimethylarsinic Acid. Inorg. Chem. 2016, 55, 8468–8477. [Google Scholar] [CrossRef]
  48. Konar, S.; Clearfield, A. Synthesis and Characterization of High Nuclearity Iron(III) Phosphonate Molecular Clusters. Inorg. Chem. 2008, 47, 5573–5579. [Google Scholar] [CrossRef]
  49. Yao, H.-C.; Li, Y.-Z.; Zheng, L.-M.; Xin, X.-Q. Syntheses, structures and magnetic properties of tetra- and heptanuclear iron(III) clusters incorporating phosphonate ligands. Inorg. Chim. Acta 2005, 358, 2523–2529. [Google Scholar] [CrossRef]
  50. Tolis, E.I.; Helliwell, M.; Langley, S.; Raftery, J.; Winpenny, R.E.P. Synthesis and Characterization of Iron(III) Phosphonate Cage Complexes. Angew. Chem. Int. Ed. 2003, 42, 3804–3808. [Google Scholar] [CrossRef]
  51. Lee, K.H.K.; Peralta, J.E.; Abboud, K.A.; Christou, G. Iron(III)–Oxo Cluster Chemistry with Dimethylarsinate Ligands: Structures, Magnetic Properties, and Computational Studies. Inorg. Chem. 2020, 59, 18090–18101. [Google Scholar] [CrossRef] [PubMed]
  52. Lee, K.H.K.; Aebersold, L.; Peralta, J.E.; Abboud, K.A.; Christou, G. Synthesis, Structure, and Magnetic Properties of an Fe36 Dimethylarsinate Cluster: The Largest “Ferric Wheel”. Inorg. Chem. 2022, 61, 17256–17267. [Google Scholar] [CrossRef]
  53. Carson, I.; Healy, M.R.; Doidge, E.D.; Love, J.B.; Morrison, C.A.; Tasker, P.A. Metal-binding motifs of alkyl and aryl phosphinates; versatile mono and polynucleating ligands. Coord. Chem. Rev. 2017, 335, 150–171. [Google Scholar] [CrossRef] [Green Version]
  54. Zeng, Y.F.; Xu, G.C.; Hu, X.; Chen, Z.; Bu, X.H.; Gao, S.; Sanudo, E.C. Single-Molecule-Magnet Behavior in a Fe12Sm4 Cluster. Inorg. Chem. 2010, 49, 9734–9736. [Google Scholar] [CrossRef]
  55. Liu, S.J.; Zeng, Y.F.; Xue, L.; Han, S.D.; Jia, J.M.; Hu, T.L.; Bu, X.H. Tuning the magnetic behaviors in FeIII12LnIII4 clusters with aromatic carboxylate ligands. Inorg. Chem. Front. 2014, 1, 200–206. [Google Scholar] [CrossRef]
  56. Zheng, X.Y.; Zhang, H.; Wang, Z.X.; Liu, P.X.; Du, M.H.; Han, Y.Z.; Wei, R.J.; Ouyang, Z.W.; Kong, X.J.; Zhuang, G.L.; et al. Insights into Magnetic Interactions in a Monodisperse Gd12Fe14 Metal Cluster. Angew. Chem.-Int. Ed. 2017, 56, 11475–11479. [Google Scholar] [CrossRef]
  57. Zheng, X.Y.; Du, M.H.; Amiri, M.; Nyman, M.; Liu, Q.; Liu, T.; Kong, X.J.; Long, L.S.; Zheng, L.S. Atomically Precise Lanthanide-Iron-Oxo Clusters Featuring the epsilon-Keggin Ion. Chem. Eur. J. 2020, 26, 1388–1395. [Google Scholar] [CrossRef] [PubMed]
  58. Zheng, X.Y.; Chen, M.T.; Du, M.H.; Wei, R.J.; Kong, X.J.; Long, L.S.; Zheng, L.S. Capturing Lacunary Iron-Oxo Keggin Clusters and Insight Into the Keggin-Fe-13 Cluster Rotational Isomerization. Chem. Eur. J. 2020, 26, 11985–11988. [Google Scholar] [CrossRef]
  59. Koumousi, E.S.; Mukherjee, S.; Beavers, C.M.; Teat, S.J.; Christou, G.; Stamatatos, T.C. Towards models of the oxygen-evolving complex (OEC) of photosystem II: A Mn4Ca cluster of relevance to low oxidation states of the OEC. Chem. Comm. 2011, 47, 11128–11130. [Google Scholar] [CrossRef]
  60. Alaimo, A.A.; Takahashi, D.; Cunha-Silva, L.; Christou, G.; Stamatatos, T.C. Emissive {MnIII4Ca} Clusters with Square Pyramidal Topologies: Syntheses and Structural, Spectroscopic, and Physicochemical Characterization. Inorg. Chem. 2015, 54, 2137–2151. [Google Scholar] [CrossRef]
  61. Mukherjee, S.; Stull, J.A.; Yano, J.; Stamatatos, T.C.; Pringouri, K.; Stich, T.A.; Abboud, K.A.; Britt, R.D.; Yachandra, V.K.; Christou, G. Synthetic model of the asymmetric [Mn3CaO4] cubane core of the oxygen-evolving complex of photosystem II. Proc. Nat. Acad. Sci. USA 2012, 109, 2257–2262. [Google Scholar] [CrossRef] [PubMed]
  62. Coleman, J.E. Structure and mechanism of alkaline phosphatase. Annu. Rev. Biophys. Biomol. Struct. 1992, 21, 441–483. [Google Scholar] [CrossRef] [PubMed]
  63. Lassila, J.K.; Zalatan, J.G.; Herschlag, D. Biological phosphoryl-transfer reactions: Understanding mechanism and catalysis. Annu. Rev. Biochem. 2011, 80, 669–702. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  64. Kamerlin, S.C.; Sharma, P.K.; Prasad, R.B.; Warshel, A. Why nature really chose phosphate. Q. Rev. Biophys. 2013, 46, 1–132. [Google Scholar] [CrossRef]
  65. Yong, S.C.; Roversi, P.; Lillington, J.; Rodriguez, F.; Krehenbrink, M.; Zeldin, O.B.; Garman, E.F.; Lea, S.M.; Berks, B.C. A complex iron-calcium cofactor catalyzing phosphotransfer chemistry. Science 2014, 345, 1170–1173. [Google Scholar] [CrossRef] [Green Version]
  66. Herbert, D.E.; Lionetti, D.; Rittle, J.; Agapie, T. Heterometallic Triiron-Oxo/Hydroxo Clusters: Effect of Redox-Inactive Metals. J. Am. Chem. Soc. 2013, 135, 19075–19078. [Google Scholar] [CrossRef] [Green Version]
  67. Prodius, D.; Turta, C.; Mereacre, V.; Shova, S.; Gdaniec, M.; Simonov, Y.; Lipkowski, J.; Kuncser, V.; Filoti, G.; Caneschi, A. Synthesis, structure and properties of heterotrinuclear carboxylate complexes [Fe2M(Ca, Sr, Ba)O(CCl3COO)6(THF)n]. Polyhedron 2006, 25, 2175–2182. [Google Scholar] [CrossRef]
  68. Burger, J.; Klüfers, P. Stabilization of Iron Clusters by Polyolato Ligands and Calcium Ions: An Fe14 Oxocluster from Aqueous Alkaline Solution. Angew. Chem. Int. Ed. 1997, 36, 776–779. [Google Scholar] [CrossRef]
  69. Prakash, R.; Saalfrank, R.W.; Maid, H.; Scheurer, A.; Heinemann, F.W.; Trautwein, A.X.; Böttger, L.H. Synthesis and Redox Properties of Mixed-Valent Octanuclear Iron Defective Hexacubanes and a (CaCl)-Capped Body-Centered Six-Sided Iron(III) Polyhedron. Angew. Chem. Int. Ed. 2006, 45, 5885–5889. [Google Scholar] [CrossRef]
  70. Earnshaw, A.; Figgis, B.N.; Lewis, J. Chemistry of polynuclear compounds. Part VI. Magnetic properties of trimeric chromium and iron carboxylates. J. Chem. Soc. A 1966, 1656–1663. [Google Scholar] [CrossRef]
  71. Sheldrick, G. Crystal structure refinement with SHELXL. Acta Crystallogr. Sect. C 2015, 71, 3–8. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  72. Spek, A. PLATON SQUEEZE: A tool for the calculation of the disordered solvent contribution to the calculated structure factors. Acta Cryst. Sec. C 2015, 71, 9–18. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  73. Spek, A. Structure validation in chemical crystallography. Acta Cryst. Sec. D 2009, 65, 148–155. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  74. Gagne, O.C.; Hawthorne, F.C. Comprehensive derivation of bond-valence parameters for ion pairs involving oxygen. Acta Cryst. Sec. B Struc. Sci. Cryst. Eng. Mat. 2015, 71, 562–578. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  75. Brown, I.D.; Altermatt, D. Bond-Valence Parameters Obtained from a Systematic Analysis of the Inorganic Crystal-Structure Database. Acta Cryst. Sec. B Struc. Sci. 1985, 41, 244–247. [Google Scholar] [CrossRef] [Green Version]
  76. Bain, G.A.; Berry, J.F. Diamagnetic corrections and Pascal’s constants. J. Chem. Edu. 2008, 85, 532–536. [Google Scholar] [CrossRef]
  77. Van Wullen, C. Broken Symmetry Approach to Density Functional Calculation of Magnetic Anisotropy or Zero Field Splittings for Multinuclear Complexes with Antiferromagnetic Coupling. J. Phys. Chem. A 2009, 113, 11535–11540. [Google Scholar] [CrossRef]
  78. Bencini, A.; Totti, F.; Daul, C.A.; Doclo, K.; Fantucci, P.; Barone, V. Density functional calculations of magnetic exchange interactions in polynuclear transition metal complexes. Inorg. Chem. 1997, 36, 5022–5030. [Google Scholar] [CrossRef]
  79. Ruiz, E.; Rodriguez-Fortea, A.; Cano, J.; Alvarez, S.; Alemany, P. About the calculation of exchange coupling constants in polynuclear transition metal complexes. J. Comp. Chem. 2003, 24, 982–989. [Google Scholar] [CrossRef]
  80. Valero, R.; Costa, R.; Moreira, I.; Truhlar, D.G.; Illas, F. Performance of the M06 family of exchange-correlation functionals for predicting magnetic coupling in organic and inorganic molecules. J. Chem. Phys. 2008, 128, 114103. [Google Scholar] [CrossRef] [Green Version]
  81. Comba, P.; Hausberg, S.; Martin, B. Calculation of Exchange Coupling Constants of Transition Metal Complexes with DFT. J. Phys. Chem. 2009, 113, 6751–6755. [Google Scholar] [CrossRef] [PubMed]
  82. Phillips, J.J.; Peralta, J.E. Magnetic Exchange Couplings from Semilocal Functionals Evaluated Nonself-Consistently on Hybrid Densities: Insights on Relative Importance of Exchange, Correlation, and Delocalization. J. Chem. Theory Comp. 2012, 8, 3147–3158. [Google Scholar] [CrossRef]
  83. Joshi, R.P.; Phillips, J.J.; Mitchell, K.J.; Christou, G.; Jackson, K.A.; Peralta, J.E. Accuracy of density functional theory methods for the calculation of magnetic exchange couplings in binuclear iron(III) complexes. Polyhedron 2020, 176, 114194. [Google Scholar] [CrossRef]
  84. Hehre, W.J.; Ditchfield, R.; Pople, J.A. Self-consistent molecular-orbital methods.12. further extensions of gaussian-type basis sets for use in molecular-orbital studies of organic-molecules. J. Chem. Phys. 1972, 56, 2257. [Google Scholar] [CrossRef]
  85. Krishnan, R.; Binkley, J.S.; Seeger, R.; Pople, J.A. Self-consistent molecular-orbital methods.20. basis set for correlated wave-functions. J. Chem. Phys. 1980, 72, 650–654. [Google Scholar] [CrossRef]
  86. Blaudeau, J.P.; McGrath, M.P.; Curtiss, L.A.; Radom, L. Extension of Gaussian-2 (G2) theory to molecules containing third-row atoms K and Ca. J. Chem. Phys. 1997, 107, 5016–5021. [Google Scholar] [CrossRef]
  87. Pantazis, D.A.; Neese, F. All-Electron Scalar Relativistic Basis Sets for the Lanthanides. J. Chem. Theory Comp. 2009, 5, 2229–2238. [Google Scholar] [CrossRef]
  88. Douglas, M.; Kroll, N.M. Quantum electrodynamical corrections to the fine structure of helium. Ann. Phys. 1974, 82, 89–155. [Google Scholar] [CrossRef]
  89. Hess, B.A. Applicability of the no-pair equation with free-particle projection operators to atomic and molecular-structure calculations. Phys. Rev. 1985, 32, 756–763. [Google Scholar] [CrossRef]
  90. Hess, B.A. Relativistic electronic-structure calculations employing a two-component no-pair formalism with external-field projection operators. Phys. Rev. 1986, 33, 3742–3748. [Google Scholar] [CrossRef] [Green Version]
  91. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision B.01, Gaussian, Inc.: Wallingford, CT, USA, 2016.
  92. Armstrong, W.H.; Roth, M.E.; Lippard, S.J. Tetranuclear iron-oxo complexes. Synthesis, structure, and properties of species containing the nonplanar {Fe4O2}8+ core and seven bridging carboxylate ligands. J. Am. Chem. Soc. 1987, 109, 6318–6326. [Google Scholar] [CrossRef]
  93. Boudalis, A.K.; Lalioti, N.; Spyroulias, G.A.; Raptopoulou, C.P.; Terzis, A.; Bousseksou, A.; Tangoulis, V.; Tuchagues, J.-P.; Perlepes, S.P. Novel Rectangular [Fe4(μ4-OHO)(μ-OH)2]7+ versus “Butterfly” [Fe4(μ3-O)2]8+ Core Topology in the FeIII/RCO2−/phen Reaction Systems (R = Me, Ph; phen = 1,10-Phenanthroline):  Preparation and Properties of [Fe4(OHO)(OH)2(O2CMe)4(phen)4](ClO4)3, [Fe4O2(O2CPh)7(phen)2](ClO4), and [Fe4O2(O2CPh)8(phen)]. Inorg. Chem. 2002, 41, 6474–6487. [Google Scholar] [CrossRef] [PubMed]
  94. Arizaga, L.; Gancheff, J.S.; Faccio, R.; Cañón-Mancisidor, W.; González, R.; Kremer, C.; Chiozzone, R. Synthesis, crystal structure and magnetic properties of a novel tetranuclear oxo-bridged iron(III) butterfly. J. Mol. Struct. 2014, 1058, 149–154. [Google Scholar] [CrossRef]
  95. Bagai, R.; Abboud, K.A.; Christou, G. A New N,N,O Chelate for Transition Metal Chemistry:  Fe5 and Fe6 Clusters from the Use of 6-Hydroxymethyl-2,2‘-bipyridine (hmbpH). Inorg. Chem. 2007, 46, 5567–5575. [Google Scholar] [CrossRef]
  96. Bagai, R.; Datta, S.; Betancur-Rodriguez, A.; Abboud, K.A.; Hill, S.; Christou, G. Diversity of New Structural Types in Polynuclear Iron Chemistry with a Tridentate N,N,O Ligand. Inorg. Chem. 2007, 46, 4535–4547. [Google Scholar] [CrossRef]
  97. Chaudhuri, P.; Winter, M.; Fleischhauer, P.; Haase, W.; Flörke, U.; Haupt, H.-J. Synthesis, structure and magnetism of a tetranuclear Fe(III) complex containing an [Fe4(μ3-O)2]8+ core. Inorg. Chim. Acta 1993, 212, 241–249. [Google Scholar] [CrossRef]
  98. Ammala, P.; Cashion, J.D.; Kepert, C.M.; Moubaraki, B.; Murray, K.S.; Spiccia, L.; West, B.O. An Octanuclear FeIII Compound Featuring a New Type of Double Butterfly Iron–Oxo Core. Angew. Chem. Int. Ed. 2000, 39, 1688–1690. [Google Scholar] [CrossRef]
  99. Cauchy, T.; Ruiz, E.; Alvarez, S. Magnetostructural Correlations in Polynuclear Complexes:  The Fe4 Butterflies. J. Am. Chem. Soc. 2006, 128, 15722–15727. [Google Scholar] [CrossRef]
  100. Overgaard, J.; Hibbs, D.E.; Rentschler, E.; Timco, G.A.; Larsen, F.K. Experimental and Theoretical Electron Density Distribution and Magnetic Properties of the Butterfly-like Complex [Fe4O2(O2CCMe3)8(NC5H4Me)2]·2CH3CN. Inorg. Chem. 2003, 42, 7593–7601. [Google Scholar] [CrossRef]
  101. Mitchell, K.J.; Abboud, K.A.; Christou, G. Magnetostructural Correlation for High-Nuclearity Iron(III)/Oxo Complexes and Application to Fe5, Fe6, and Fe8 Clusters. Inorg. Chem. 2016, 55, 6597–6608. [Google Scholar] [CrossRef]
  102. Hale, A.R.; Aebersold, L.E.; Peralta, J.E.; Foguet-Albiol, D.; Abboud, K.A.; Christou, G. Analysis of spin frustration in an FeIII7 cluster using a combination of computational, experimental, and magnetostructural correlation methods. Polyhedron 2022, 225, 116045. [Google Scholar] [CrossRef]
  103. Boskovic, C.; Bircher, R.; Tregenna-Piggott, P.L.W.; Gudel, H.U.; Paulsen, C.; Wernsdorfer, W.; Barra, A.L.; Khatsko, E.; Neels, A.; Stoeckli-Evans, H. Ferromagnetic and antiferromagnetic intermolecular interactions in a new family of Mn4 complexes with an energy barrier to magnetization reversal. J. Am. Chem. Soc. 2003, 125, 14046–14058. [Google Scholar] [CrossRef] [PubMed]
Chemistry 05 00110 g001 550
Figure 1. (top) Complete structure of the anion of 1 from a viewpoint parallel to the stacking axis of the two Fe4 butterfly units. H atoms are omitted for clarity. (bottom) Labeled {Fe8O5(OH)5}8+ core from a viewpoint nearly perpendicular to the stacking axis. Colour code: Fe3+ light green, P orange, O red, HO− purple, C grey.
Figure 1. (top) Complete structure of the anion of 1 from a viewpoint parallel to the stacking axis of the two Fe4 butterfly units. H atoms are omitted for clarity. (bottom) Labeled {Fe8O5(OH)5}8+ core from a viewpoint nearly perpendicular to the stacking axis. Colour code: Fe3+ light green, P orange, O red, HO− purple, C grey.
Chemistry 05 00110 g001
Chemistry 05 00110 g002 550
Figure 2. Partial structure of 2 from viewpoints parallel (top) and perpendicular (middle) to the stacking axis. H atoms and aromatic rings are omitted for clarity. (bottom) Partially labeled half of the core emphasizing the means of attachment of the end Fe2 unit and Ca2+ ions. Color code: Fe3+ light green, Ca2+ yellow, O red, N blue, and C grey.
Figure 2. Partial structure of 2 from viewpoints parallel (top) and perpendicular (middle) to the stacking axis. H atoms and aromatic rings are omitted for clarity. (bottom) Partially labeled half of the core emphasizing the means of attachment of the end Fe2 unit and Ca2+ ions. Color code: Fe3+ light green, Ca2+ yellow, O red, N blue, and C grey.
Chemistry 05 00110 g002
Chemistry 05 00110 g003a 550Chemistry 05 00110 g003b 550
Figure 3. Partial structure of 3 from viewpoints parallel (top) and perpendicular (middle) to the stacking axis. H atoms and aromatic rings are omitted for clarity. (bottom) Partially labeled half of the core emphasizing the means of attachment of the end Fe2 unit and La3+ ions. Color code: Fe3+ light green, La3+ magenta, O red, HO− purple, and C grey.
Figure 3. Partial structure of 3 from viewpoints parallel (top) and perpendicular (middle) to the stacking axis. H atoms and aromatic rings are omitted for clarity. (bottom) Partially labeled half of the core emphasizing the means of attachment of the end Fe2 unit and La3+ ions. Color code: Fe3+ light green, La3+ magenta, O red, HO− purple, and C grey.
Chemistry 05 00110 g003aChemistry 05 00110 g003b
Chemistry 05 00110 g004 550
Figure 4. RMSD overlay of the core of the anion of 1 on the cores of 2 (left) and 3 (right) from two perpendicular viewpoints each. Color code: 1 purple, 2 blue, 3 green.
Figure 4. RMSD overlay of the core of the anion of 1 on the cores of 2 (left) and 3 (right) from two perpendicular viewpoints each. Color code: 1 purple, 2 blue, 3 green.
Chemistry 05 00110 g004
Chemistry 05 00110 g005 550
Figure 5. Plots of dc χMT vs. T in the 5.0–300 K range and a 0.1 T dc field for vacuum-dried 1·½MeCN (♦), 2·2H2O (♦), and 3·4H2O (♦).
Figure 5. Plots of dc χMT vs. T in the 5.0–300 K range and a 0.1 T dc field for vacuum-dried 1·½MeCN (♦), 2·2H2O (♦), and 3·4H2O (♦).
Chemistry 05 00110 g005
Chemistry 05 00110 g006 550
Figure 6. Plots of ac in-phase χ′MT vs. T in the 1.8–15.0 K range and a 0.35 G ac field at a 1000 Hz frequency for vacuum-dried 1·½MeCN (♦), 2·2H2O (♦), and 3·4H2O (♦).
Figure 6. Plots of ac in-phase χ′MT vs. T in the 1.8–15.0 K range and a 0.35 G ac field at a 1000 Hz frequency for vacuum-dried 1·½MeCN (♦), 2·2H2O (♦), and 3·4H2O (♦).
Chemistry 05 00110 g006
Chemistry 05 00110 g007a 550Chemistry 05 00110 g007b 550
Figure 7. (top) Calculated JMSC from Table 3 and the predicted spin vector alignments for the anion of 1. Frustrated and satisfied JMSC are shown in red and blue, respectively. (bottom) Spin vector alignments on the core to emphasize the bridging oxo positions.
Figure 7. (top) Calculated JMSC from Table 3 and the predicted spin vector alignments for the anion of 1. Frustrated and satisfied JMSC are shown in red and blue, respectively. (bottom) Spin vector alignments on the core to emphasize the bridging oxo positions.
Chemistry 05 00110 g007aChemistry 05 00110 g007b
Chemistry 05 00110 g008a 550Chemistry 05 00110 g008b 550
Figure 8. (top) Calculated JMSC from Table 3 and the predicted spin vector alignments for 2. Frustrated and satisfied JMSC are shown in red and blue, respectively; green interactions are moot at low T due to the central Fe8 being in its local S = 0 ground state (bottom) Spin vector alignments shown on the core to emphasize the bridging oxo positions. In both figures, the relative spin vector alignments at Fe5 and Fe6 are arbitrary.
Figure 8. (top) Calculated JMSC from Table 3 and the predicted spin vector alignments for 2. Frustrated and satisfied JMSC are shown in red and blue, respectively; green interactions are moot at low T due to the central Fe8 being in its local S = 0 ground state (bottom) Spin vector alignments shown on the core to emphasize the bridging oxo positions. In both figures, the relative spin vector alignments at Fe5 and Fe6 are arbitrary.
Chemistry 05 00110 g008aChemistry 05 00110 g008b
Chemistry 05 00110 g009a 550Chemistry 05 00110 g009b 550
Figure 9. (top) Calculated JMSC from Table 3 and the predicted spin vector alignments for 3. Frustrated and satisfied JMSC are shown in red and blue, respectively; green interactions are moot at low T due to the central Fe8 being in its local S = 0 ground state. (bottom) Spin vector alignments shown on the core to emphasize the bridging oxo positions.
Figure 9. (top) Calculated JMSC from Table 3 and the predicted spin vector alignments for 3. Frustrated and satisfied JMSC are shown in red and blue, respectively; green interactions are moot at low T due to the central Fe8 being in its local S = 0 ground state. (bottom) Spin vector alignments shown on the core to emphasize the bridging oxo positions.
Chemistry 05 00110 g009aChemistry 05 00110 g009b
Chemistry 05 00110 g010 550
Figure 10. Two adjacent molecules of 3 in the crystal showing two pairs of near-perpendicular 4-tBu-benzoate ligands (all green) between them. Other colours: Fe3+ light green, La3+ magneta, O red, OH− purple, C grey, and H white. Some C and H atoms were omitted for clarity. Ligands in dark green are to highlight the interaction pathway.
Figure 10. Two adjacent molecules of 3 in the crystal showing two pairs of near-perpendicular 4-tBu-benzoate ligands (all green) between them. Other colours: Fe3+ light green, La3+ magneta, O red, OH− purple, C grey, and H white. Some C and H atoms were omitted for clarity. Ligands in dark green are to highlight the interaction pathway.
Chemistry 05 00110 g010
Table
Table 1. Crystal data and structural refinement parameters for 1–3.
Table 1. Crystal data and structural refinement parameters for 1–3.
123
Formula aC126H115Fe8NO30.50P10C163.6H122.4Fe12Ca4N3.6O55.6C161.76H177.25Fe6La2N4.25O31
Fw, g/mol2887.683858.763289.74
Crystal

system		OrthorhombicMonoclinicTriclinic
Space groupPbcaP21/cP 1 ¯
a, Å25.7321(8)18.4877(16)19.6897(9)
b, Å29.8328(9)23.1092(19)21.5274(10)
c, Å37.9740(12)23.7055(19)24.0099(11)
α, °909097.3530(10)
β, °90112.917(2)111.5360(10)
γ, °9090115.5490(10)
Volume, Å329,151.1(16)9328.4(13)8028.5(6)
Z822
T, K100(2)100(2)100(2)
λ, Å a0.710730.710730.71073
ρcalc, Mg/m31.3161.3741.361
R1 b, d4.495.894.72
wR2 c, e9.9015.0011.52
a solvent molecules not included. b Graphite monochromator. c I > 2σ(I). d R1 = Σ(||Fo| − |Fc||)/Σ|Fo|. e wR2 = [Σ[w(Fo2 − Fc2)2]/Σ[w(Fo2)2]]1/2 where w = 1/[σ2(Fo2) + (m × p)2 + n × p], p = [max(Fo2,0)+ 2 × Fc2]/3, and m & n are constants.
Table
Table 2. BVS values and assignments for core O atoms of the anion of 1 and 3.
Table 2. BVS values and assignments for core O atoms of the anion of 1 and 3.
ComplexAtomBVSAssignment a
1O11.86O2−
O21.86O2−
O31.87O2−
O41.91O2−
O50.98OH−
O61.00OH−
O71.02OH−
O80.81OH−
O1111.24OH−
O1121.55 bO2− b
3O11.72O2−
O21.73O2−
O31.83O2−
O42.07O2−
O51.14OH−
O62.01O2−
O71.15OH−
a Non-, singly, and doubly protonated O atoms have typical BVS values of ~1.8 to 2.0, ~0.9 to 1.2, and ~0.2 to 0.4, although H-bonding can affect the ranges. b Decreased from a typical O2− value due to hydrogen-bonding with the NHEt3+ cation.
Table
Table 3. Exchange interactions Jij for Fe2 pairs in 1–3.
Table 3. Exchange interactions Jij for Fe2 pairs in 1–3.
PairJMSC 1 aPairJMSC 2 aPairJMSC 3 aJDFT 3 a
Fe1–Fe2−26.9 Fe1–Fe2−33.5Fe2–Fe4−52.4−44.9
Fe1–Fe4−20.9Fe1–Fe3−32.3Fe2–Fe5−53.0−44.3
Fe2–Fe3−26.2Fe2–Fe4−28.1Fe3–Fe4−54.3−46.3
Fe3–Fe4−25.4Fe3–Fe4−29.3Fe3–Fe5−53.7−44.2
Fe2–Fe4−8.8 bFe2–Fe3−7.6 bFe4–Fe5−14.8+0.8 b
Fe5–Fe6−29.4Fe1–Fe4−3.2Fe4–Fe5′−27.4−27.4
Fe5–Fe8−20.5Fe1–Fe5−0.9Fe1–Fe6−3.7−0.1 d
Fe6–Fe7−25.7Fe1–Fe6−1.5Fe3–Fe6−9.6−7.5
Fe7–Fe8−29.4Fe2–Fe3′−36.3Fe2–Fe6−10.2−7.8
Fe6–Fe8−9.9 bFe4–Fe5−1.3Fe2–Fe3−2.3+2.0
Fe1–Fe5−5.4Fe4–Fe6−0.8Fe1–Fe2−10.1−8.0
Fe3–Fe7−2.3Fe5–Fe6−1.9 dFe1–Fe3−8.6−5.1
Fe2–Fe6−24.7
Fe4–Fe8−51.7 c
a cm−1. b Body-body pairs within the Fe4 butterfly units. c This is the Fe4-O112-F8 unit, the only Fe2 pair with a μ2-O2− bridge, rationalizing a much stronger JMSC even though O112 is involved in hydrogen-bonding with the NHEt3+ cation. d Fe2 pairs attached to each end of the central Fe8 unit giving the cubanes.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Singh, A.P.; Brantley, C.L.; Lee, K.H.K.; Abboud, K.A.; Peralta, J.E.; Christou, G. Structural and Magnetic Analysis of a Family of Structurally Related Iron(III)-Oxo Clusters of Metal Nuclearity Fe8, Fe12Ca4, and Fe12La4. Chemistry 2023, 5, 1599-1620. https://doi.org/10.3390/chemistry5030110

AMA Style

Singh AP, Brantley CL, Lee KHK, Abboud KA, Peralta JE, Christou G. Structural and Magnetic Analysis of a Family of Structurally Related Iron(III)-Oxo Clusters of Metal Nuclearity Fe8, Fe12Ca4, and Fe12La4. Chemistry. 2023; 5(3):1599-1620. https://doi.org/10.3390/chemistry5030110

Chicago/Turabian Style

Singh, Alok P., ChristiAnna L. Brantley, Kenneth Hong Kit Lee, Khalil A. Abboud, Juan E. Peralta, and George Christou. 2023. "Structural and Magnetic Analysis of a Family of Structurally Related Iron(III)-Oxo Clusters of Metal Nuclearity Fe8, Fe12Ca4, and Fe12La4" Chemistry 5, no. 3: 1599-1620. https://doi.org/10.3390/chemistry5030110

APA Style

Singh, A. P., Brantley, C. L., Lee, K. H. K., Abboud, K. A., Peralta, J. E., & Christou, G. (2023). Structural and Magnetic Analysis of a Family of Structurally Related Iron(III)-Oxo Clusters of Metal Nuclearity Fe8, Fe12Ca4, and Fe12La4. Chemistry, 5(3), 1599-1620. https://doi.org/10.3390/chemistry5030110

Article Metrics

Back to TopTop