Fisher Information for a System Composed of a Combination of Similar Potential Models
Abstract
:1. Introduction
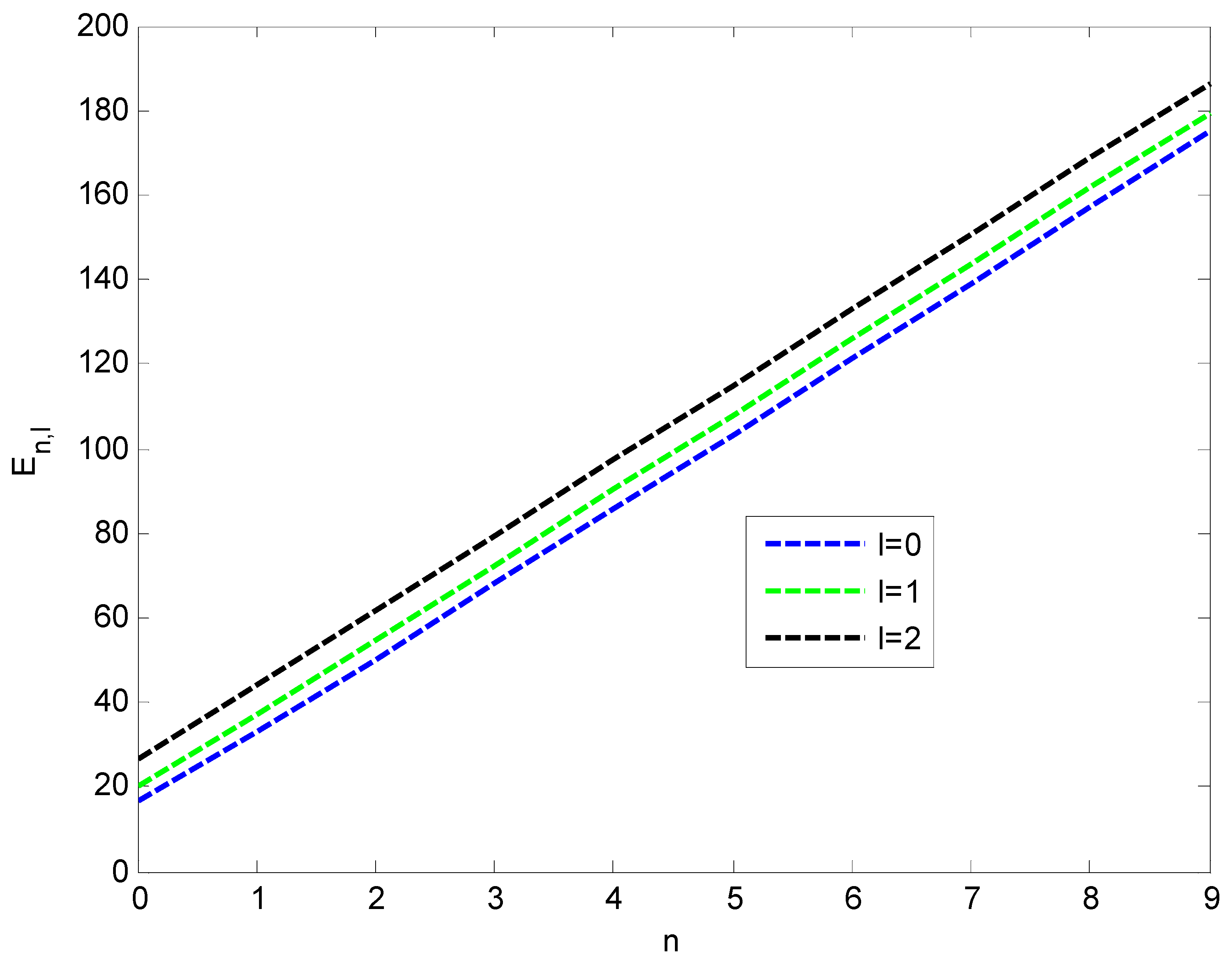
2. Bound State Solutions
3. Calculation of Expectation Values
4. Fisher Information
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jia, C.-S.; Diao, Y.-F.; Liu, X.-J.; Wang, P.-Q.; Liu, J.-Y.; Zhang, G.-D. Equivalence of the Wei potential model and Tietz potential model for diatomic molecules. J. Chem. Phys. 2012, 137, 014101. [Google Scholar] [CrossRef]
- Qiang, W.-C.; Dong, S.-H. Analytical approximations to the solutions of the Manning—Rosen potential with centrifugal term. Phys. Lett. A 2007, 368, 13–17. [Google Scholar] [CrossRef]
- Tang, H.-M.; Liang, G.-C.; Zhang, L.-H.; Zhao, F.; Jia, C.-S. Molecular energies of the improved Tietz potential energy model. Can. J. Chem. 2014, 92, 201–205. [Google Scholar] [CrossRef]
- Gu, X.-Y.; Dong, S.-H.; Ma, Z.-Q. Energy spectra for modified Rosen-Morse potential solved by the exact quantization rule. J. Phys. A Math. Theor. 2009, 42, 035303. [Google Scholar] [CrossRef]
- Hamzavi, M.; Thylwe, K.A.; Rajabi, A.A. Approximate Bound States Solution of the Hellmann Potential. Commun. Theor. Phys. 2013, 60, 1–8. [Google Scholar] [CrossRef]
- Barakat, T. The asymptotic iteration method for the eigenenergies of the anharmonic oscillator potential. Phys. Lett. A 2005, 344, 411–417. [Google Scholar] [CrossRef]
- Pratiwi, B.N.; Suparmi, A.; Cari, C.; Anwar, F. Asymptotic iteration method for the eigenfunctions and eigenvalue analysis in Schrodinger equation with modified anisotropic nonquadratic potential. J. Phys. Conf. Ser. 2016, 776, 012090. [Google Scholar] [CrossRef]
- Barakat, T. The asymptotic iteration method for the iegenenergies of the Schrödinger equation with potential V (r) = −Z/r + gr + λ r2. J. Phys. A Math. Gen 2006, 39, 823–831. [Google Scholar] [CrossRef]
- Bayrak, O.; Boztosun, I. Bound state solutions of the Hulthén potential by using the asymptotic iteration method. Phys. Scr. 2007, 76, 92–96. [Google Scholar] [CrossRef]
- Ozfidan, A. Approximate Bound State Solutions of the Hellmann Plus Kratzer Potential in N-dimensional Space. Gazi Univ. J. Sci. 2020, 33, 791–804. [Google Scholar] [CrossRef]
- Dong, S.-H.; Cruz-Irisson, M. Energy spectrum for a modified Rosen-Morse potential solved by proper quantization rule and its thermodynamic properties. J. Math. Chem. 2012, 50, 881–892. [Google Scholar] [CrossRef]
- Hajigeorgiou, P.G. Exact analytical expressions for diatomic rotational and centrifugal distortion constants for a Kratzer–Fues oscillator. J. Mol. Spectrosc. 2006, 235, 111–116. [Google Scholar] [CrossRef]
- Zhang, L.-H.; Li, X.-P.; Jia, C.-S. Approximate Solutions of the Schrodinger Equation with the Generalized Morse potential model including the centrifugal term. Int. J. Quant. Chem. 2011, 111, 1870–1878. [Google Scholar] [CrossRef]
- Onate, C.A.; Okon, I.B.; Jude, G.O.; Onyeaju, M.O.; Antia, A.D. Uncertainty Relation and the Thermal Properties of an Isotropic Harmonic Oscillator (IHO) with the Inverse Quadratic (IQ) Potentials and the Pseudo-Harmonic (PH) with the Inverse Quadratic (IQ) Potentials. Quantum Rep. 2023, 5, 38–51. [Google Scholar] [CrossRef]
- Jia, C.-S.; Zhang, L.-H.; Peng, X.-L.; Luo, J.-X.; Zhao, Y.-L.; Liu, J.-Y.; Guo, J.-J.; Tang, L.-D. Prediction of entropy and Gibbs free energy for nitrogen. Chem. Eng. Sci. 2019, 202, 70–74. [Google Scholar] [CrossRef]
- Jiang, R.; Jia, C.-S.; Wang, Y.-Q.; Peng, X.-L.; Zhang, L.-H. Prediction of Gibbs free energy for the gases Cl2, Br2, and HCl. Chem. Phys. Lett. 2019, 726, 83–86. [Google Scholar] [CrossRef]
- Okorie, U.S.; Edet, C.O.; Ikot, A.N.; Rampho, G.J.; Sever, R. Thermodynamic functions for diatomic molecules with modified Kratzer plus screened Coulomb potential. Indian J. Phys. 2021, 95, 411–421. [Google Scholar] [CrossRef]
- Njoku, I.J.; Onyenegecha, C.P.; Okereke, C.J.; Opara, A.I.; Ukewuihe, U.M.; Nwaneho, F.U. Approximate solutions of Schrodinger equation and thermodynamic properties with Hua potential. Results Phys. 2021, 24, 104208. [Google Scholar] [CrossRef]
- Yaşuk, F.; Berkdemir, C.; Berkdemir, A. Exact solutions of the Schrödinger equation with non-central potential by the Nikiforov–Uvarov method. J. Phys. A Math. Gen. 2005, 38, 6579. [Google Scholar] [CrossRef]
- Okon, I.B.; Popoola, O.; Ituen, E.E. Bound state solution to Schrodinger equation with Hulthen plus exponential Coulombic potential with centrifugal potential barrier using parametric Nikiforov-Uvarov method. Int. J. Recent. Advan. Phys. 2016, 5, 1–15. [Google Scholar] [CrossRef]
- Yahya, W.A.; Issa, K. Approximate Analytical Solutions of the Improved Tietz and Improved Rosen-Morse Potential Models. Chin. J. Phys. 2015, 53, 060401-1–060401-9. [Google Scholar]
- Davidson, P. Proceedings of the Royal Society of London. Series A, Containing Papers of a Mathematical and Physical Character. R. Soc. 1932, 135, 459–472. [Google Scholar]
- Oyewumi, K.J.; Sen, K.D. Exact solutions of the Schrödinger equation for the pseudoharmonic potential: An application to some diatomic molecules. J. Math. Chem. 2012, 50, 1039–1059. [Google Scholar] [CrossRef]
- Oyewumi, K.J.; Akinpelu, F.O.; Agboola, A.D. Exactly Complete Solutions of the Pseudoharmonic Potential in N-Dimensions. Int. J. Theor. Phys. 2008, 47, 1039–1057. [Google Scholar] [CrossRef]
- Sever, R.; Tezcan, C.; Yeșiltaș, O. Exact solution of Schrödinger equation for Pseudoharmonic potential. J. Math. Chem. 2008, 43, 845–851. [Google Scholar] [CrossRef]
- Ikhdair, S.; Sever, R. Exact polynomial eigensolutions of the Schrödinger equation for the pseudoharmonic potential. J. Mol. Struct. Theochem. 2007, 806, 155–158. [Google Scholar] [CrossRef]
- Das, T.; Arda, A. Exact Analytical Solution of the N-Dimensional Radial Schrödinger Equation with Pseudoharmonic Potential via Laplace Transform Approach. Advan. High. Energy Phys. 2015, 2015, 137038. [Google Scholar] [CrossRef]
- Okorie, U.S.; Ikot, A.N.; Okon, I.B.; Obagboye, L.F.; Horchani, R.; Abdullah, H.Y.; Qadir, K.W.; Abdel-Aty, A.-H. Exact solutions of k-dependent Schrödinger equation with quantum pseudo-harmonic oscillator and its applications for the thermodynamic properties in normal and superstatistics. Sci. Rep. 2023, 13, 2108. [Google Scholar] [CrossRef] [PubMed]
- Bayrak, O.; Boztosun, I.; Ciftci, H. Exact solutions of κ-dependent Schrödinger equation with quantum pseudo-harmonic oscillator and its applications for the thermodynamic properties in normal and superstatistics. Int. J. Quant. Chem. 2007, 107, 540–544. [Google Scholar] [CrossRef]
- Oyewumi, K.J. Analytical solutions of the Kratzer-Fues potential in arbitrary number of dimensions, Found. Phys. Lett. 2005, 18, 75–84. [Google Scholar]
- Witten, E. Dynamical breaking of supersymmetry. Nuclear Phys. B 1981, 188, 513–554. [Google Scholar] [CrossRef]
- Gendenshtein, L. Derivation of exact spectra of the schrodinger equation by means of supersymmetry. JETP Lett. 1983, 38, 356–359. [Google Scholar]
- Cooper, F.; Khare, A.; Sukhatme, U. Supersymmetry and quantum mechanics. Phys. Rep. 1995, 251, 267–385. [Google Scholar] [CrossRef]
- Feynman, R.P. Forces in molecules. Phys. Rev. 1939, 56, 340–342. [Google Scholar] [CrossRef]
- Hassanabadi, H.; Yazarloo, H.B.; Lu, L.-L. Approximate analytical solutions to the generalized Poschl-Teller potential in D dimensions. Chin. Phys. Lett. 2012, 29, 020303. [Google Scholar]
- Falaye, B.J.; Oyewumi, K.J.; Ikhdair, S.M.; Hamzavi, M. Eigensolution techniques, their applications and Fisher’s information entropy of the Tietz–Wei diatomic molecular model. Phys. Scr. 2014, 89, 115204. [Google Scholar] [CrossRef]
- Onate, C.A.; Okon, I.B.; Omugbe, E.; Onyeaju, M.C.; Owolabi, J.A.; Emeje, K.O.; Eyube, E.S.; Obasuyi, A.R.; William, E.S. Eigensolutions, virial theorem and molecular study of nonrelativistic Krazer-Fues potential. Int. J. Quantum Chem. 2023, 124, 27286. [Google Scholar] [CrossRef]
- Gibilisco, P.; Isola, T. Uncertainty principle and quantum Fisher information. Am. Int. Sch. Med. 2007, 59, 147–159. [Google Scholar] [CrossRef]
- Luo, S.; Zhang, Z. An informational characterization of Schrödinger’s uncertainty relations. J. Stat. Phys. 2004, 11, 1557–1576. [Google Scholar] [CrossRef]
- Gibilisco, P.; Imparato, D.; Isola, T. Uncertainty principle and quantum Fisher information II. J. Math. Phys. 2007, 48, 072109. [Google Scholar] [CrossRef]
- Martin, M.T.; Pennini, F.; Plastino, A. Fisher’s information and the analysis of complex signals. Phys. Lett. A 1999, 256, 173–176. [Google Scholar] [CrossRef]
- Boumali, A.; Labidi, M. Shannon entropy and Fisher information of the one-dimensional Klein-Godon oscillator with energy-dependent potential. Mod. Phys. Lett. A 2018, 33, 1850033. [Google Scholar] [CrossRef]
- Romera, E.; Sảnchez-Moreno, P.; Dehesa, J.S. The Fisher information of single-particle systems with a central potential. Chem. Phys. Lett. 2005, 414, 468–472. [Google Scholar] [CrossRef]
- Dehesa, J.S.; Gonzảlez-Fẻrez, R.; Sảnchez-Moreno, P. Fisher-information-based uncertainty relation, Cramer-Rao inequality and kinetic energy for D-dimensional central problem. J. Phys. A Math. Theor. 2007, 40, 1845–1856. [Google Scholar] [CrossRef]
- Onate, C.A.; Oyewumi, K.J.; Falaye, B.J.; Okon, I.B.; Omugbe, E.; Chen Wen-Li, C. Fisher information of a modified trigonometric inversely quadratic potential. Chin. J. Phys. 2022, 80, 1–11. [Google Scholar] [CrossRef]
- Dehesa, J.S.; Lόpez-Rosa, S.; Olmos, B. Fisher information of D-dimensional hydrogenic systems in position and momentum Spaces. J. Math. Phys. 2006, 47, 052104. [Google Scholar] [CrossRef]


| 0 | 0 | 19.1915543 | 30.2367130 | 29.9096370 | 50.2267069 |
| 1 | 26.8693976 | 38.6168018 | 38.0538010 | 58.8074820 | |
| 2 | 37.5880690 | 51.8488919 | 50.2771052 | 73.2878939 | |
| 3 | 49.3042587 | 67.2542617 | 64.1033929 | 90.9334004 | |
| 1 | 0 | 43.8601841 | 64.5835661 | 59.8627152 | 91.8404299 |
| 1 | 51.8866789 | 73.5761120 | 68.5040080 | 101.195400 | |
| 2 | 62.7622893 | 87.2096164 | 81.0061110 | 116.302626 | |
| 3 | 74.5398554 | 102.806150 | 94.9538017 | 134.292068 | |
| 2 | 0 | 68.9627325 | 99.8304090 | 90.4917350 | 134.751205 |
| 1 | 77.0734494 | 109.000745 | 99.2664182 | 144.358222 | |
| 2 | 88.0004415 | 122.777346 | 111.864086 | 159.703767 | |
| 3 | 99.8033288 | 138.455898 | 125.862895 | 177.845801 | |
| 3 | 0 | 94.1756967 | 135.354381 | 121.312779 | 178.109873 |
| 1 | 102.316428 | 144.594507 | 130.137638 | 187.822519 | |
| 2 | 113.265083 | 158.434548 | 142.776689 | 203.277995 | |
| 3 | 125.080290 | 174.154043 | 156.800703 | 221.497925 | |
| 0 | 0.5 | 12.4202881 | 14.5803237 | 21.9178843 | 24.0760055 |
| 1.0 | 9.39252710 | 9.91579930 | 18.2993918 | 18.8139539 | |
| 1.5 | 8.44361600 | 8.66857060 | 17.1549376 | 17.3764753 | |
| 2.0 | 7.98103440 | 8.10491030 | 36.3343188 | 16.7170015 | |
| 1 | 0.5 | 24.3346767 | 26.7141787 | 25.2162768 | 38.7409248 |
| 1.0 | 15.1059713 | 15.6968954 | 21.6715263 | 25.7961769 | |
| 1.5 | 12.1681436 | 12.4202881 | 19.9405623 | 21.9178843 | |
| 2.0 | 10.7362083 | 10.8734364 | 16.5947227 | 20.0746906 | |
| 2 | 0.5 | 36.6586629 | 39.1157627 | 51.3010558 | 53.8062866 |
| 1.0 | 21.0620459 | 21.6877923 | 32.4241376 | 33.0409857 | |
| 1.5 | 16.0437357 | 16.3126127 | 26.3605159 | 26.6233344 | |
| 2.0 | 13.5932412 | 13.7395803 | 23.3994205 | 23.5421470 | |
| 3 | 0.5 | 49.1359643 | 51.6272352 | 66.4978821 | 69.0498216 |
| 1.0 | 27.1474431 | 27.7929105 | 39.8011807 | 40.4404751 | |
| 1.5 | 20.0143131 | 20.2940459 | 31.1657135 | 31.4398758 | |
| 2.0 | 16.5207835 | 16.6735512 | 26.9411554 | 27.0902716 | |
| 4 | 0.5 | 61.6833974 | 64.1920084 | 81.8076698 | 84.3845319 |
| 1.0 | 33.3080324 | 33.9654942 | 47.2832585 | 47.9369683 | |
| 1.5 | 24.0475954 | 24.3346767 | 36.0520860 | 36.3343188 | |
| 2.0 | 19.4986801 | 19.6561126 | 30.5450424 | 30.6990056 | |
| 5 | 0.5 | 74.2674019 | 76.7857329 | 97.1793420 | 99.7706981 |
| 1.0 | 39.5153037 | 40.1804755 | 54.8339773 | 55.4973648 | |
| 1.5 | 28.1239350 | 28.4161668 | 40.9968719 | 41.2850021 | |
| 2.0 | 22.5134945 | 22.6743939 | 34.1964520 | 34.3541585 | |
| 1 | 0 | 4.18168780 | 6.0217369 | 9.72050810 | 11.6301374 |
| 1 | 8.50000000 | 10.5510171 | 16.2222222 | 18.4038820 | |
| 2 | 12.5793525 | 14.7015198 | 21.9412282 | 24.2349788 | |
| 3 | 16.5379816 | 18.6878788 | 27.2631497 | 29.6126984 | |
| 4 | 20.4202881 | 22.5803237 | 32.3346767 | 34.7141787 | |
| 5 | 24.2487113 | 26.4107479 | 37.2301178 | 39.6259364 | |
| 2 | 0 | 6.50000000 | 8.55101710 | 14.2222222 | 16.4038820 |
| 1 | 14.5379816 | 16.6878788 | 25.2631497 | 27.6126984 | |
| 2 | 22.2487113 | 24.4107479 | 35.2301178 | 37.6259364 | |
| 3 | 29.7921099 | 31.9479971 | 44.6521671 | 47.0601538 | |
| 4 | 37.2291330 | 39.3742236 | 53.7304216 | 56.1377674 | |
| 5 | 44.5908154 | 46.7242693 | 62.5667333 | 64.9679851 | |
| 3 | 0 | 8.57935250 | 10.7015198 | 17.9412282 | 20.2349788 |
| 1 | 20.2487113 | 22.4107479 | 33.2301178 | 35.6259364 | |
| 2 | 31.5215665 | 33.6722999 | 47.2265378 | 49.6351901 | |
| 3 | 42.5908154 | 44.7242693 | 60.5667333 | 62.9679851 | |
| 4 | 53.5303880 | 55.6472139 | 73.4925650 | 75.8806382 | |
| 5 | 64.3780215 | 66.4800000 | 86.1265502 | 88.5000000 | |
| 4 | 0 | 10.5379816 | 12.6878788 | 21.2631497 | 23.6126984 |
| 1 | 25.7921099 | 27.9479971 | 40.6521671 | 43.0601538 | |
| 2 | 40.5908154 | 42.7242693 | 58.5667333 | 60.9679851 | |
| 3 | 55.1551611 | 57.2668299 | 75.7320533 | 78.1153066 | |
| 4 | 69.5701085 | 71.6631972 | 92.4238854 | 94.7875816 | |
| 5 | 83.8790804 | 85.9565223 | 108.781987 | 111.126964 | |
| 5 | 0 | 12.4202881 | 14.5803237 | 24.3346767 | 26.7141787 |
| 1 | 31.2291330 | 33.3742236 | 47.7304216 | 50.1377674 | |
| 2 | 49.5303880 | 51.6472139 | 69.4925650 | 71.8806382 | |
| 3 | 67.5701085 | 69.6631972 | 90.4238854 | 92.7875816 | |
| 4 | 85.4430187 | 87.5169397 | 110.829779 | 113.170292 | |
| 5 | 103.197567 | 105.255816 | 130.865107 | 133.184707 | |
| 0 | 12.9965839 | 8.5737778 | 111.4298232 | 21.5583462 | 15.7382452 | 339.2905400 |
| 1 | 11.8185475 | 15.2579808 | 180.3271707 | 19.6777166 | 23.7495543 | 467.3369980 |
| 2 | 11.2615469 | 22.1905981 | 249.9004614 | 18.7115540 | 32.1266502 | 601.1395518 |
| 3 | 10.9565616 | 29.2240214 | 320.1947901 | 18.1551929 | 40.6676667 | 738.3293339 |
| 4 | 10.7720175 | 36.3056214 | 391.0847898 | 17.8069278 | 49.2919216 | 877.7376907 |
| 5 | 10.6520394 | 43.4130476 | 462.4374938 | 17.5749107 | 57.9626756 | 1018.688851 |
| 6 | 10.5697147 | 50.5355353 | 534.1461897 | 17.4127458 | 66.6613706 | 1160.757499 |
| 7 | 10.5108053 | 57.6673891 | 606.1306983 | 17.2950190 | 75.3778408 | 1303.661192 |
| 8 | 10.4672122 | 64.8053694 | 678.3315540 | 17.2068853 | 84.1061482 | 1447.204844 |
| 8 | 10.4340553 | 71.9475241 | 750.7044447 | 17.1392093 | 92.8426388 | 1591.249420 |
| 10 | 10.4082527 | 79.0926206 | 823.2159806 | 17.0861231 | 101.584965 | 1735.693225 |
| 11 | 10.3877810 | 86.2398494 | 895.8406674 | 17.0437192 | 110.331565 | 1880.460203 |
| 12 | 10.3712673 | 93.3886615 | 968.5587745 | 17.0093145 | 119.081364 | 2025.492369 |
| 13 | 10.3577538 | 100.538674 | 1041.354832 | 16.9810180 | 127.833606 | 2170.744768 |
| 14 | 10.3465556 | 107.689612 | 1114.216560 | 16.9574657 | 136.587746 | 2316.182020 |
| 15 | 10.3371727 | 114.841276 | 1187.134100 | 16.9376539 | 145.343382 | 2461.775909 |
| 1.0 | 6.3749475 | 6.7680001 | 43.1456451 | 13.9837226 | 13.7463577 | 192.2252527 |
| 1.5 | 12.0910640 | 6.2814977 | 75.9499903 | 20.5118296 | 13.1590415 | 269.9160160 |
| 2.0 | 14.6231981 | 6.0423780 | 88.3588904 | 23.4211406 | 12.8653865 | 301.3220276 |
| 2.5 | 16.0247585 | 5.8983477 | 94.5195968 | 25.0442629 | 12.6881230 | 317.7646874 |
| 3.0 | 16.9078176 | 5.8018537 | 98.0966833 | 26.0739294 | 12.5694080 | 327.7338557 |
| 3.5 | 17.5129218 | 5.7326651 | 100.395716 | 26.7834340 | 12.4843480 | 334.3737107 |
| 4.0 | 17.9525752 | 5.6806283 | 101.981906 | 27.3012806 | 12.4204164 | 339.0932721 |
| 4.5 | 18.2860804 | 5.6400719 | 103.134809 | 27.6955632 | 12.3706163 | 342.6111838 |
| 5.0 | 18.5475457 | 5.6075771 | 104.006792 | 28.0056349 | 12.3307320 | 345.3299783 |
| 5.5 | 18.7579355 | 5.5809594 | 104.687276 | 28.2557876 | 12.2980723 | 347.4917179 |
| 6.0 | 18.9308264 | 5.5587579 | 105.231880 | 28.4618122 | 12.2708384 | 349.2502995 |
| 6.5 | 19.0753897 | 5.5399585 | 105.676868 | 28.6344110 | 12.2477829 | 350.7080500 |
| 7.0 | 19.1980391 | 5.5238356 | 106.046812 | 28.7810910 | 12.2280132 | 351.9355594 |
| 7.5 | 19.3033929 | 5.5098559 | 106.358913 | 28.9072717 | 12.2108738 | 352.9830473 |
| 8.0 | 19.3948598 | 5.4976189 | 106.625548 | 29.0169624 | 12.1958730 | 353.8871873 |
| 8.5 | 19.4750097 | 5.4868181 | 106.855836 | 29.1131925 | 12.1826340 | 354.6753683 |
| 9.0 | 19.5458162 | 5.4772149 | 107.056635 | 29.1982930 | 12.1708638 | 355.3684483 |
| 0 | 2.0753109 | 4.2441101 | 8.8078482 | 3.9027949 | 6.5411490 | 25.5287631 |
| 1 | 2.1434445 | 3.2491460 | 6.9643639 | 3.9345613 | 5.3895866 | 21.2056588 |
| 2 | 2.4796239 | 2.3486134 | 5.8236779 | 4.2100767 | 4.1263248 | 17.3721438 |
| 3 | 3.0345051 | 1.7624127 | 5.3480505 | 4.7530480 | 3.1895560 | 15.1601125 |
| 4 | 3.7149293 | 1.3858907 | 5.1484858 | 5.4798280 | 2.5445494 | 13.9436929 |
| 5 | 4.4660484 | 1.1325692 | 5.0581087 | 6.3206480 | 2.0942879 | 13.2372565 |
| 6 | 5.2591091 | 0.9534294 | 5.0141894 | 7.2328358 | 1.7693011 | 12.7970647 |
| 7 | 6.0785638 | 0.8212049 | 4.9917465 | 8.1913537 | 1.5265679 | 12.5046576 |
| 8 | 6.9155189 | 0.7201144 | 4.9799647 | 9.1811818 | 1.3396688 | 12.2997429 |
| 9 | 7.7645982 | 0.6405721 | 4.9737848 | 10.192968 | 1.1919700 | 12.1497115 |
| 10 | 8.6223893 | 0.5764852 | 4.9706794 | 11.220659 | 1.0726518 | 12.0358600 |
| 11 | 9.4866342 | 0.5238228 | 4.9693156 | 12.260199 | 0.9744445 | 11.9468833 |
| 12 | 10.355785 | 0.4798244 | 4.9689582 | 13.308778 | 0.8923155 | 11.8756286 |
| 13 | 11.228746 | 0.4425416 | 4.9691872 | 14.364399 | 0.8226858 | 11.8173877 |
| 14 | 12.104725 | 0.4105633 | 4.9697553 | 15.425605 | 0.7629492 | 11.7689537 |
| 15 | 12.983131 | 0.3828440 | 4.9705140 | 16.491312 | 0.7111670 | 11.7280766 |
| 0 | 8.30124380 | 16.976441 | 140.925571 | 15.6111797 | 26.1645959 | 408.4602096 |
| 1 | 8.57377780 | 12.996584 | 111.429823 | 15.7382452 | 21.5583462 | 339.2905400 |
| 2 | 9.91849550 | 9.3944536 | 93.1788457 | 16.8403066 | 16.5052993 | 277.9543004 |
| 3 | 12.1380204 | 7.0496510 | 85.5688076 | 19.0121919 | 12.7582239 | 242.5618001 |
| 4 | 14.8597170 | 5.5435626 | 82.3757720 | 21.9193120 | 10.1781975 | 223.0990871 |
| 5 | 17.8641936 | 4.5302766 | 80.9297386 | 25.2825921 | 8.37715150 | 211.7961041 |
| 6 | 21.0364363 | 3.8137178 | 80.2270307 | 28.9313433 | 7.07720460 | 204.7530351 |
| 7 | 24.3142550 | 3.2848197 | 79.8679439 | 32.7654146 | 6.10627160 | 200.0745216 |
| 8 | 27.6620757 | 2.8804576 | 79.6794347 | 36.7247270 | 5.35867530 | 196.7958870 |
| 9 | 31.0583928 | 2.5622883 | 79.5805565 | 40.7718702 | 4.76788000 | 194.3953836 |
| 10 | 34.4895572 | 2.3059406 | 79.5308712 | 44.8826362 | 4.29060710 | 192.5737593 |
| 11 | 37.9465368 | 2.0952913 | 79.5090499 | 49.0407939 | 3.89777810 | 191.1501323 |
| 12 | 41.4231385 | 1.9192976 | 79.5033319 | 53.2351111 | 3.56926200 | 190.0100570 |
| 13 | 44.9149846 | 1.7701664 | 79.5069946 | 57.4575959 | 3.29074340 | 189.0782028 |
| 14 | 48.4188988 | 1.6422531 | 79.5160843 | 61.7024212 | 3.05179690 | 188.3032599 |
| 15 | 51.9325232 | 1.5313761 | 79.5282236 | 65.9652465 | 2.84466800 | 187.6492262 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Onate, C.A.; Okon, I.B.; Eyube, E.S.; Omugbe, E.; Emeje, K.O.; Onyeaju, M.C.; Ajani, O.O.; Akinpelu, J.A. Fisher Information for a System Composed of a Combination of Similar Potential Models. Quantum Rep. 2024, 6, 184-199. https://doi.org/10.3390/quantum6020015
Onate CA, Okon IB, Eyube ES, Omugbe E, Emeje KO, Onyeaju MC, Ajani OO, Akinpelu JA. Fisher Information for a System Composed of a Combination of Similar Potential Models. Quantum Reports. 2024; 6(2):184-199. https://doi.org/10.3390/quantum6020015
Chicago/Turabian StyleOnate, Clement Atachegbe, Ituen B. Okon, Edwin Samson Eyube, Ekwevugbe Omugbe, Kizito O. Emeje, Michael C. Onyeaju, Olumide O. Ajani, and Jacob A. Akinpelu. 2024. "Fisher Information for a System Composed of a Combination of Similar Potential Models" Quantum Reports 6, no. 2: 184-199. https://doi.org/10.3390/quantum6020015
APA StyleOnate, C. A., Okon, I. B., Eyube, E. S., Omugbe, E., Emeje, K. O., Onyeaju, M. C., Ajani, O. O., & Akinpelu, J. A. (2024). Fisher Information for a System Composed of a Combination of Similar Potential Models. Quantum Reports, 6(2), 184-199. https://doi.org/10.3390/quantum6020015







