Quantum Theory of Lee–Naughton–Lebed’s Angular Effect in Strong Electric Fields
Abstract
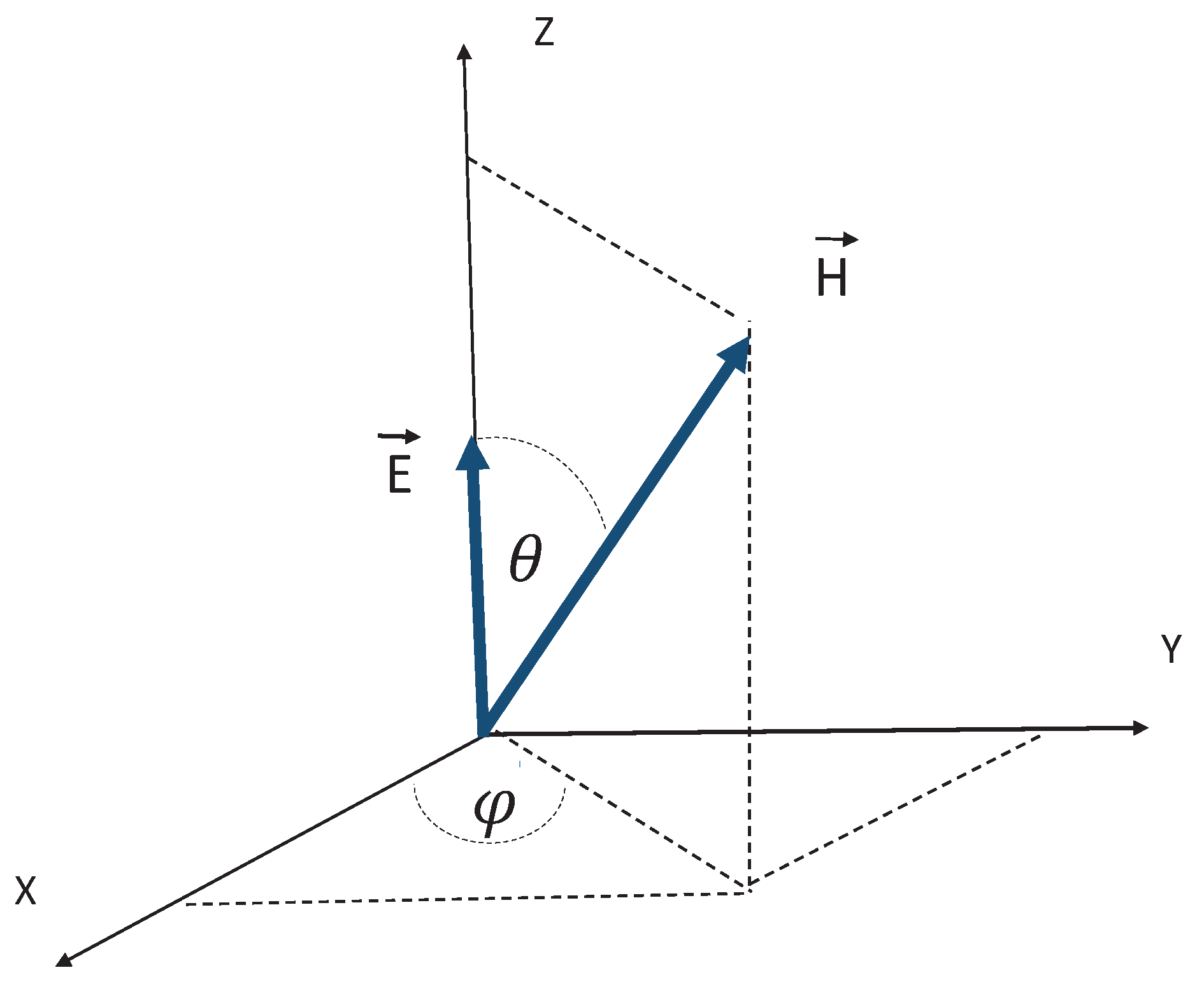
:1. Introduction
2. Materials and Methods
3. Results
4. Discussion
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lebed, A.G. (Ed.) The Physics of Organic Superconductors and Conductors; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Ishiguro, T.; Yamaji, K.; Saito, G. Organic Superconductors, 2nd ed.Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Gor’kov, L.P.; Lebed, A.G. On the stability of the quasi-one-dimensional metallic phase in magnetic fields against the spin density wave formation. J. Phys. Lett. 1984, 45, L-433. [Google Scholar]
- Heritier, M.; Montambaux, G.; Lederer, P. Stability of the spin density wave phases in (TMTSF) 2ClO4: Quantized nesting effect. J. Phys. Lett. 1984, 45, 943–952. [Google Scholar] [CrossRef]
- Chaikin, P.M. Magnetic-field-induced transition in quasi-two-dimensional systems. Phys. Rev. 1985, B31, 4770. [Google Scholar] [CrossRef] [PubMed]
- Chaikin, P.M.; Choi, M.-Y.; Kwak, J.F.; Brooks, J.S.; Martin, K.P.; Naughton, M.J.; Engler, E.M.; Greene, R.L. Tetramethyltetraselenafulvalenium Perchlorate, (TMTSF)2ClO4, in High Magnetic Fields. Phys. Rev. Lett. 1983, 51, 2333. [Google Scholar] [CrossRef]
- Ribault, M.; Jerome, D.; Tuchendler, J.; Weyl, C.; Bechgaard, K. Low-field and anomalous high-field Hall effect in (TMTSF)2ClO4. J. Phys. Lett. 1983, 44, 953–961. [Google Scholar] [CrossRef]
- Lebed, A.G. Field-induced spin-density-wave phases in quasi-one-dimensional conductors: Theory versus experiments. Phys. Rev. Lett. 2002, 88, 177001. [Google Scholar] [CrossRef]
- Zanchi, D.; Bjelis, A.; Montambaux, G. Phase diagram for charge-density waves in a magnetic field. Phys. Rev. 1996, B53, 1240. [Google Scholar] [CrossRef] [PubMed]
- Qualls, J.S.; Balicas, L.; Brooks, J.S.; Harrison, N.; Montgomery, L.K.; Tokumoto, M. Competition between Pauli and orbital effects in a charge-density-wave system. Phys. Rev. 2000, B62, 10008. [Google Scholar] [CrossRef]
- Andres, D.; Kartsovnik, M.V.; Biberacher, W.; Weiss, H.; Balthes, E.; Muller, H.; Kushch, N. Orbital effect of a magnetic field on the low-temperature state in the organic metal α–(BEDT–TTF) 2 KHg (SCN) 4. Phys. Rev. 2001, B64, 161104(R). [Google Scholar] [CrossRef]
- Andres, D.; Kartsovnik, M.V.; Grigoriev, P.D.; Biberacher, W.; Muller, H. Orbital quantization in the high-magnetic-field state of a charge-density-wave system. Phys. Rev. 2003, B68, 201101. [Google Scholar] [CrossRef]
- Lebed, A.G. Theory of magnetic field-induced charge-density-wave phases. JETP Lett. 2003, 78, 138–142. [Google Scholar] [CrossRef]
- Hannahs, S.T.; Brooks, J.S.; Kang, W.; Chiang, L.Y.; Chaikin, P.M. Quantum Hall effect in a bulk crystal. Phys. Rev. Lett. 1989, 63, 1988. [Google Scholar] [CrossRef]
- Cooper, J.R.; Kang, W.; Auban, P.; Montambaux, G.; Jerome, D.; Bechgaard, K. Quantized Hall effect and a new field-induced phase transition in the organic superconductor (TMTSF)2PF6. Phys. Rev. Lett. 1989, 63, 1984. [Google Scholar] [CrossRef]
- Yakovenko, V.M. Quantum Hall effect in quasi-one-dimensional conductors. Phys. Rev. 1991, B43, 11353. [Google Scholar] [CrossRef]
- Lebed, A.G.; Bak, P. Theory of unusual anisotropy of magnetoresistance in organic superconductors. Phys. Rev. Lett. 1989, 63, 1315. [Google Scholar] [CrossRef]
- Naughton, M.J.; Chung, O.H.; Chiang, L.Y.; Brooks, J.S. MRS-Symposia Proceedings. Mater. Res. Soc. Symp. Proc. 1990, 173, 257. [Google Scholar] [CrossRef]
- Osada, T.; Kawasumi, A.; Kagoshima, S.; Miura, N.; Saito, G. Commensurability effect of magnetoresistance anisotropy in the quasi-one-dimensional conductor tetramethyltetraselenafulvalenium perchlorate, (TMTSF)2ClO4. Phys. Rev. Lett. 1991, 66, 1525. [Google Scholar] [CrossRef]
- Boebinger, G.S.; Montambaux, G.; Kaplan, M.L.; Haddon, R.C.; Chichester, S.V.; Chiang, L.Y. Anomalous magnetoresistance anisotropy in metallic and spin-density-wave phases of the quasi-one-dimensional organic conductor (TMTSF)2ClO4. Phys. Rev. Lett. 1990, 64, 591. [Google Scholar] [CrossRef]
- Naughton, M.J.; Chung, O.H.; Chaparala, M.; Bu, X.; Coppens, P. Commensurate fine structure in angular-dependent studies of (TMTSF)2ClO4. Phys. Rev. Lett. 1991, 67, 3712. [Google Scholar] [CrossRef]
- Kang, W.; Hannahs, S.T.; Chaikin, P.M. Lebed’s magic angle effects in (TMTSF)2PF6. Phys. Rev. Lett. 1992, 69, 2827. [Google Scholar] [CrossRef]
- Kartsovnik, M.V.; Kovalev, A.E.; Laukhin, V.N.; Pesotskii, S.E. Giant angular magnetoresistance oscillations in (BEDT-TTF) 2 TlHg (SCN) 4: The warped plane Fermi surface effect. J. Phys. I 1992, 2, 223–228. [Google Scholar] [CrossRef]
- Kartsovnik, M.V.; Kovalev, A.E.; Kushch, N.D. Magnetotransport investigation of the low-temperature state of transition (BEDT-TTF) 2TIHg (SCN) 4: Evidence for a Peierls-type transition. J. Phys. I 1993, 3, 1187–1199. [Google Scholar] [CrossRef]
- Benhia, K.; Ribault, M.; Lenior, C. Lebed resonance effects in the metallic and spin-density-wave phases of (TMTSF) 2PF6. Europhys. Lett. 1994, 25, 285. [Google Scholar]
- Kartsovnik, M.V.; Kovalev, A.E.; Laukhin, V.N.; Ito, H.; Ishiguro, T.; Kushch, N.D.; Anzai, H.; Saito, G. Agnetoresistance anisotropy in the organic superconductor κ-(BEDT-TTF) 2Cu (NCS)2. Synth. Met. 1995, 70, 819–820. [Google Scholar] [CrossRef]
- Chashechkina, E.I.; Chaikin, P.M. Magic Angles and the Ground States in (TMTSF)2PF6. Phys. Rev. Lett. 1998, 80, 2181. [Google Scholar] [CrossRef]
- Osada, T.; Nose, H.; Kuraguchi, M. Angular dependent phenomena in low-dimensional conductors under high magnetic fields. Phys. B Condens. Matter 2001, 294–295, 402–407. [Google Scholar] [CrossRef]
- Chashechkina, E.I.; Chaikin, P.M. Simple fit for magic-angle magnetoresistance in (TMTSF)2PF6. Phys. Rev. 2002, B65, 012405. [Google Scholar] [CrossRef]
- Kang, H.; Jo, Y.J.; Uji, S.; Kang, W. Evidence for coherent interchain electron transport in quasi-one-dimensional molecular conductors. Phys. Rev. 2003, B68, 132508. [Google Scholar] [CrossRef]
- Kang, H.; Jo, Y.J.; Kang, W. Pressure dependence of the angular magnetoresistance of (TMTSF)2PF6. Phys. Rev. 2004, B69, 033103. [Google Scholar] [CrossRef]
- Ito, H.; Suzuki, D.; Yokochi, Y.; Kuroda, S.; Umemiya, M.; Miyasaka, H.; Sugiura, K.-I.; Yamashita, M.; Tajima, H. Quasi-one-dimensional electronic structure of (DMET)2CuCl2. Phys. Rev. 2005, B71, 212503. [Google Scholar] [CrossRef]
- Kartsovnik, M.V.; Andres, D.; Simonov, S.V.; Biberacher, W.; Sheikin, I.; Kushch, N.D.; Miller, H. Angle-Dependent Magnetoresistance in the Weakly Incoherent Interlayer Transport Regime in a Layered Organic Conductor. Phys. Rev. Lett. 2006, 96, 166601. [Google Scholar] [CrossRef] [PubMed]
- Takahashi, S.; Betancur-Rodiguez, A.; Hill, S.; Takasaki, S.; Yamada, J.; Anzai, H. Are lebed’s magic angles truly magic? J. Low Temp. Phys. 2007, 142, 311–314. [Google Scholar] [CrossRef]
- Kang, W.; Osada, T.; Jo, Y.J.; Kang, H. Interlayer magnetoresistance of quasi-one-dimensional layered organic conductors. Phys. Rev. Lett. 2007, 99, 017002. [Google Scholar] [CrossRef] [PubMed]
- Kang, W. Absence of magic-angle effects in the intralayer resistance Rxx of the quasi-one-dimensional organic conductor (TMTSF)2ClO4. Phys. Rev. 2007, B76, 193103. [Google Scholar] [CrossRef]
- Bangura, A.F.; Goddard, P.A.; Singleton, J.; Tozer, S.W.; Coldea, A.I.; Ardavan, A.; McDonald, R.D.; Blundell, S.J.; Schlueter, J.A. Angle-dependent magnetoresistance oscillations due to magnetic breakdown orbits. Phys. Rev. 2007, B76, 0525010. [Google Scholar] [CrossRef]
- Kang, W.; Chung, O.-H. Quasi-one-dimensional Fermi surface of (TMTSF)2NO3. Phys. Rev. 2009, B79, 045115. [Google Scholar] [CrossRef]
- Graf, D.; Brooks, J.S.; Choi, E.S.; Almeida, M.; Henriques, R.T.; Dias, J.C.; Uji, S. Geometrical and orbital effects in a quasi-one-dimensional conductor. Phys. Rev. 2009, B80, 155104. [Google Scholar] [CrossRef]
- Kang, W.; Jo, Y.J.; Noh, D.Y.; Son, K.Y.; Chung, O.-H. Stereoscopic study of angle-dependent interlayer magnetoresistance in the organic conductor κ-(BEDT-TTF)2Cu(NCS)2. Phys. Rev. 2009, B80, 155102. [Google Scholar] [CrossRef]
- Naughton, M.J.; Lee, I.J.; Chaikin, P.M.; Danner, G.M. Critical fields and magnetoresistance in the molecular superconductors (TMTSF) 2X. Synth. Metals 1997, 85, 1481–1485. [Google Scholar] [CrossRef]
- Yoshino, H.; Saito, K.; Nishikawa, H.; Kikuchi, K.; Kobayashi, K.; Ikemoto, I. Fine structure of in-plane angular effect of magnetoresistance of (DMET) 2I3. J. Phys. Soc. Jpn. 1997, 66, 2248–2251. [Google Scholar] [CrossRef]
- Lee, I.J.; Naughton, M.J. Effective electrons and angular oscillations in quasi-one-dimensional conductors. Phys. Rev. 1998, B57, 7423. [Google Scholar] [CrossRef]
- Lee, I.J.; Naughton, M.J. Metallic state in (TMTSF)2PF6 at low pressure. Phys. Rev. 1998, B58, R13343. [Google Scholar] [CrossRef]
- Lebed, A.G.; Ha, H.-I.; Naughton, M.J. Angular magnetoresistance oscillations in organic conductors. Phys. Rev. 2005, B71, 132504. [Google Scholar] [CrossRef]
- Ha, H.I.; Lebed, A.G.; Naughton, M.J. Interference effects due to commensurate electron trajectories and topological crossovers in (TMTSF)2ClO4. Phys. Rev. 2006, B73, 033107. [Google Scholar] [CrossRef]
- Wu, S.; Lebed, A.G. Unification theory of angular magnetoresistance oscillations in quasi-one-dimensional conductors. Phys. Rev. 2010, B82, 075123. [Google Scholar] [CrossRef]
- McKenzie, R.H.; Moses, P. Periodic orbit resonances in layered metals in tilted magnetic fields. Phys. Rev. 1999, B60, R11241. [Google Scholar] [CrossRef]
- Lebed, A.G.; Naughton, M.J. Fermi surface interference effects and angular magnetic oscillations in Q1D conductors. J. Phys. IV 2002, 12, 369–372. [Google Scholar] [CrossRef]
- Osada, T. Resonant tunneling tuned by magnetic field orientations in anisotropic multilayer systems. Phys. E Low-Dimens. Syst. Nanostructures 2002, E12, 272–275. [Google Scholar] [CrossRef]
- Lebed, A.G.; Naughton, M.J. Interference commensurate oscillations in quasi-one-dimensional conductors. Phys. Rev. Lett. 2003, 91, 187003. [Google Scholar] [CrossRef]
- Osada, T.; Kuraguchi, M. General quantum picture for magnetoresistance angular effects in quasi-one-dimensional conductors. Synth. Met. 2003, 133–134, 75–77. [Google Scholar] [CrossRef]
- Banerjee, A.; Yakovenko, V.M. Angular magnetoresistance oscillations in quasi-one-dimensional organic conductors in the presence of a crystal superstructure. Phys. Rev. 2008, B78, 125404. [Google Scholar] [CrossRef]
- Cooper, B.K.; Yakovenko, V.M. Interlayer Aharonov-Bohm Interference in Tilted Magnetic Fields in Quasi-One-Dimensional Organic Conductors. Phys. Rev. Lett. 2006, 96, 037001. [Google Scholar] [CrossRef] [PubMed]
- Kobayashi, K.; Saito, M.; Omichi, E.; Osada, T. Electric-Field Effect on the Angle-Dependent Magnetotransport Properties of Quasi-One-Dimensional Conductors. Phys. Rev. Lett. 2006, 96, 126601. [Google Scholar] [CrossRef] [PubMed]
- Abrikosov, A.A. Fundamentals of Theory of Metals; Elsevier Science: Amsterdam, The Netherland, 1988. [Google Scholar]
- LIfshits, I.M.; Azbel, M.Y.; Kaganov, M.I. Electron Theory of Metals; Consultants Bureau: New York, NY, USA, 1973. [Google Scholar]
- Grosso, G.; Parravichini, G.P. Solid State Physics; Academic Press: New York, USA, 2000. [Google Scholar]
- Gradshteyn, L.S.; Ryzhik, I.M. Tables of Integrals, Series, and Products, 5th ed.; Academic Press, Inc.: London, UK, 1994. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lebed, A.G. Quantum Theory of Lee–Naughton–Lebed’s Angular Effect in Strong Electric Fields. Quantum Rep. 2024, 6, 359-365. https://doi.org/10.3390/quantum6030023
Lebed AG. Quantum Theory of Lee–Naughton–Lebed’s Angular Effect in Strong Electric Fields. Quantum Reports. 2024; 6(3):359-365. https://doi.org/10.3390/quantum6030023
Chicago/Turabian StyleLebed, Andrei G. 2024. "Quantum Theory of Lee–Naughton–Lebed’s Angular Effect in Strong Electric Fields" Quantum Reports 6, no. 3: 359-365. https://doi.org/10.3390/quantum6030023




