Adherent Moving of Polymers in Spherical Confined Binary Semiflexible Ring Polymer Mixtures
Abstract
:1. Introduction
2. Model and Method
3. Results and Discussion
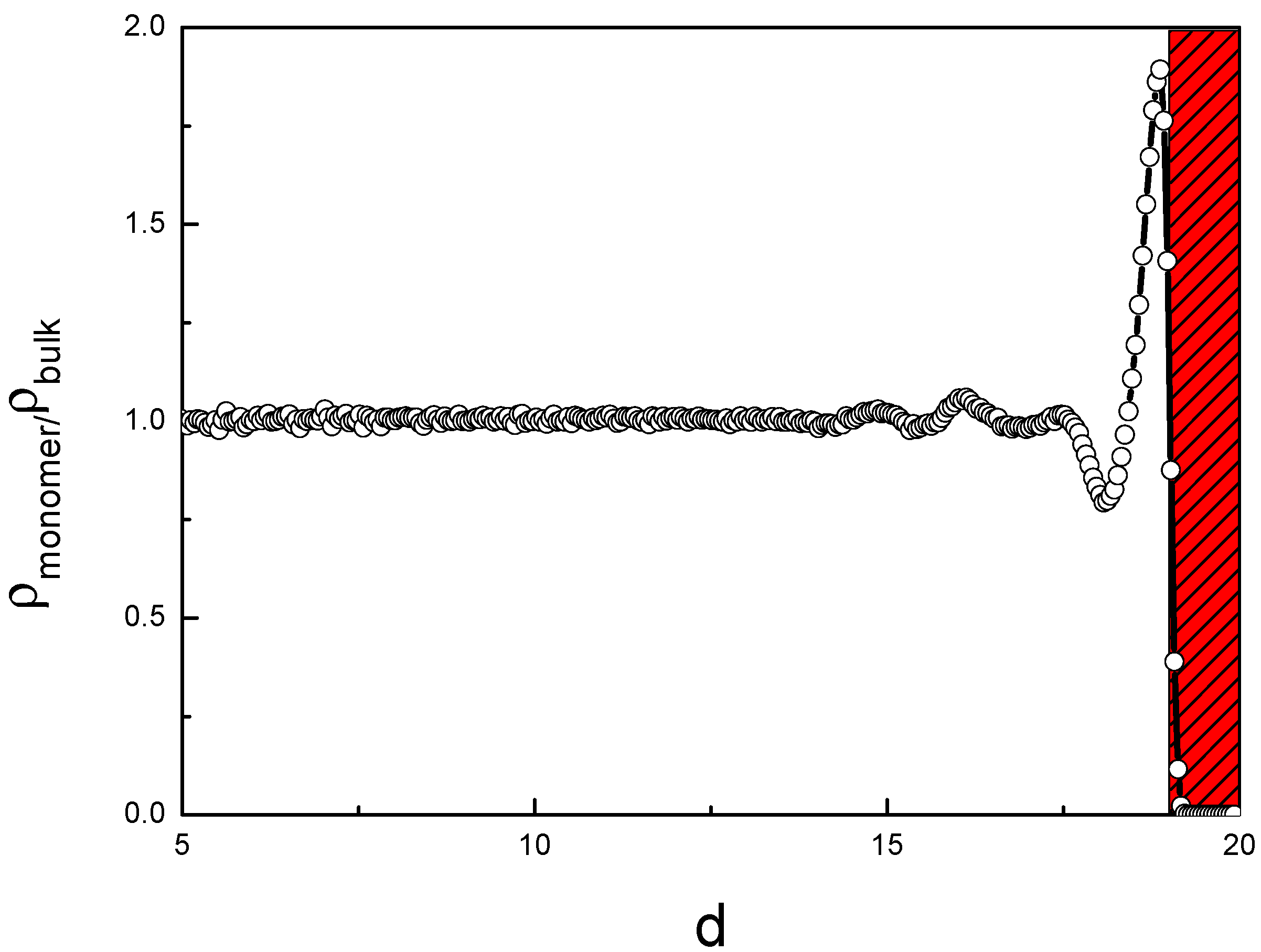
3.1. Distribution of the Binary Mixture
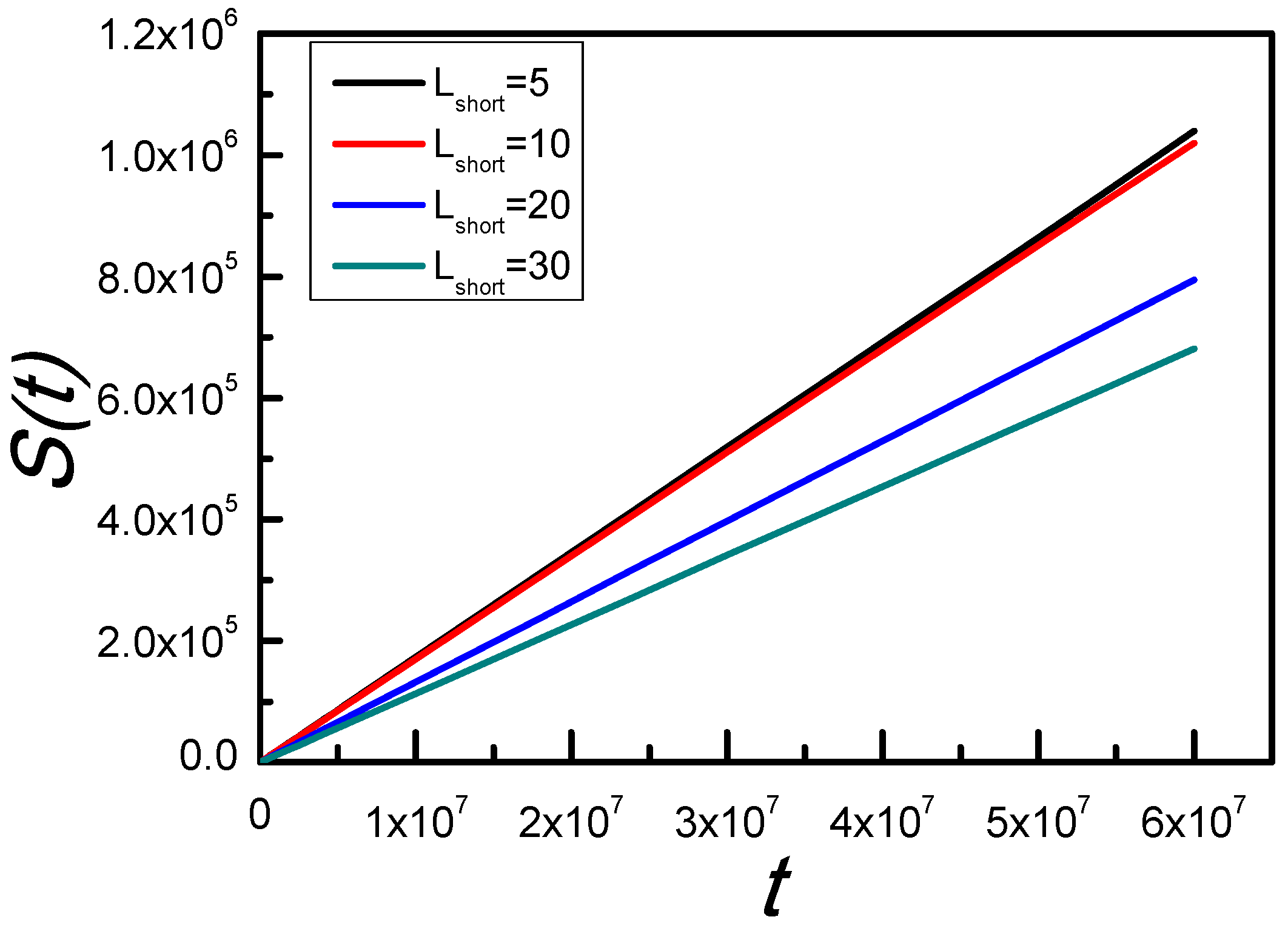
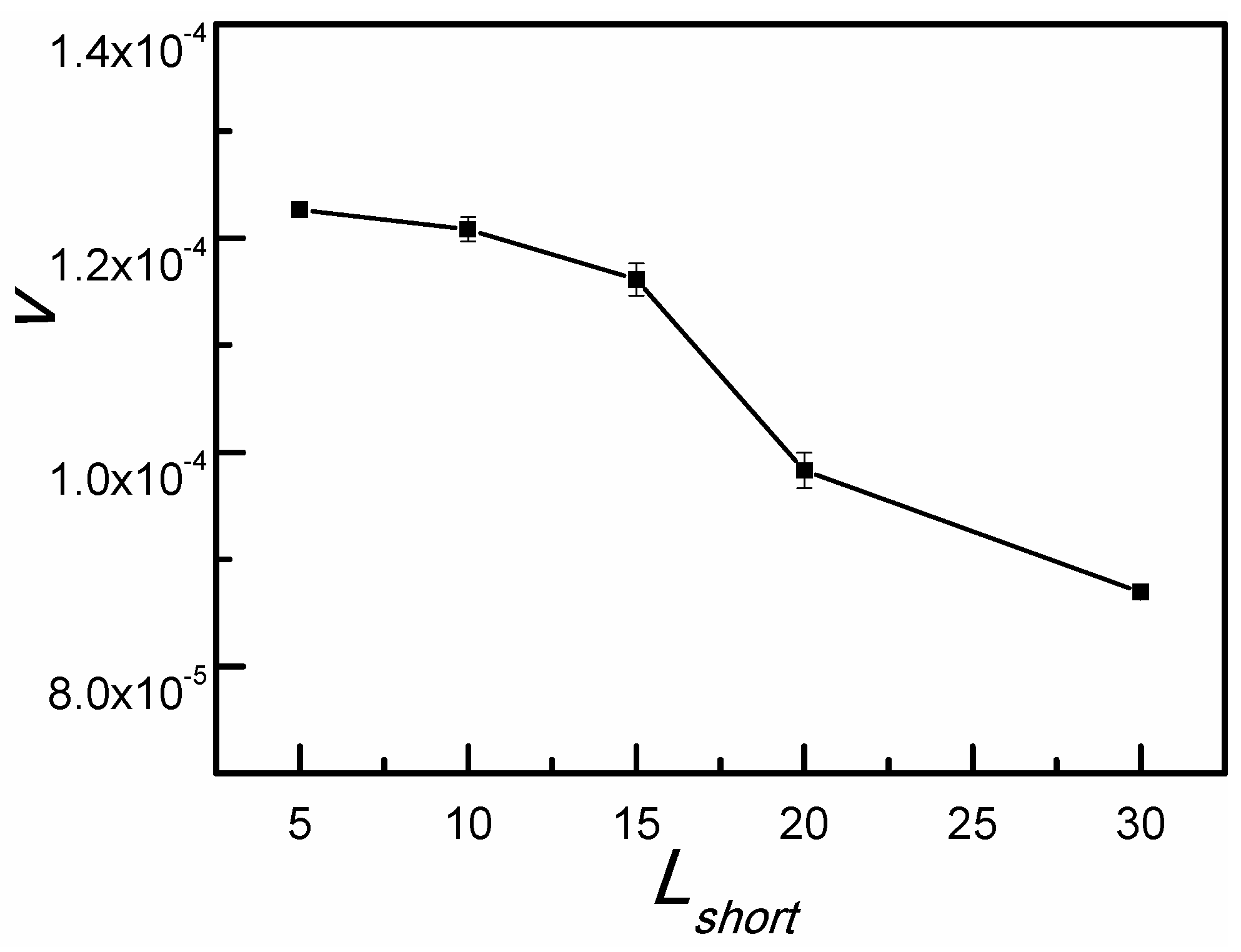
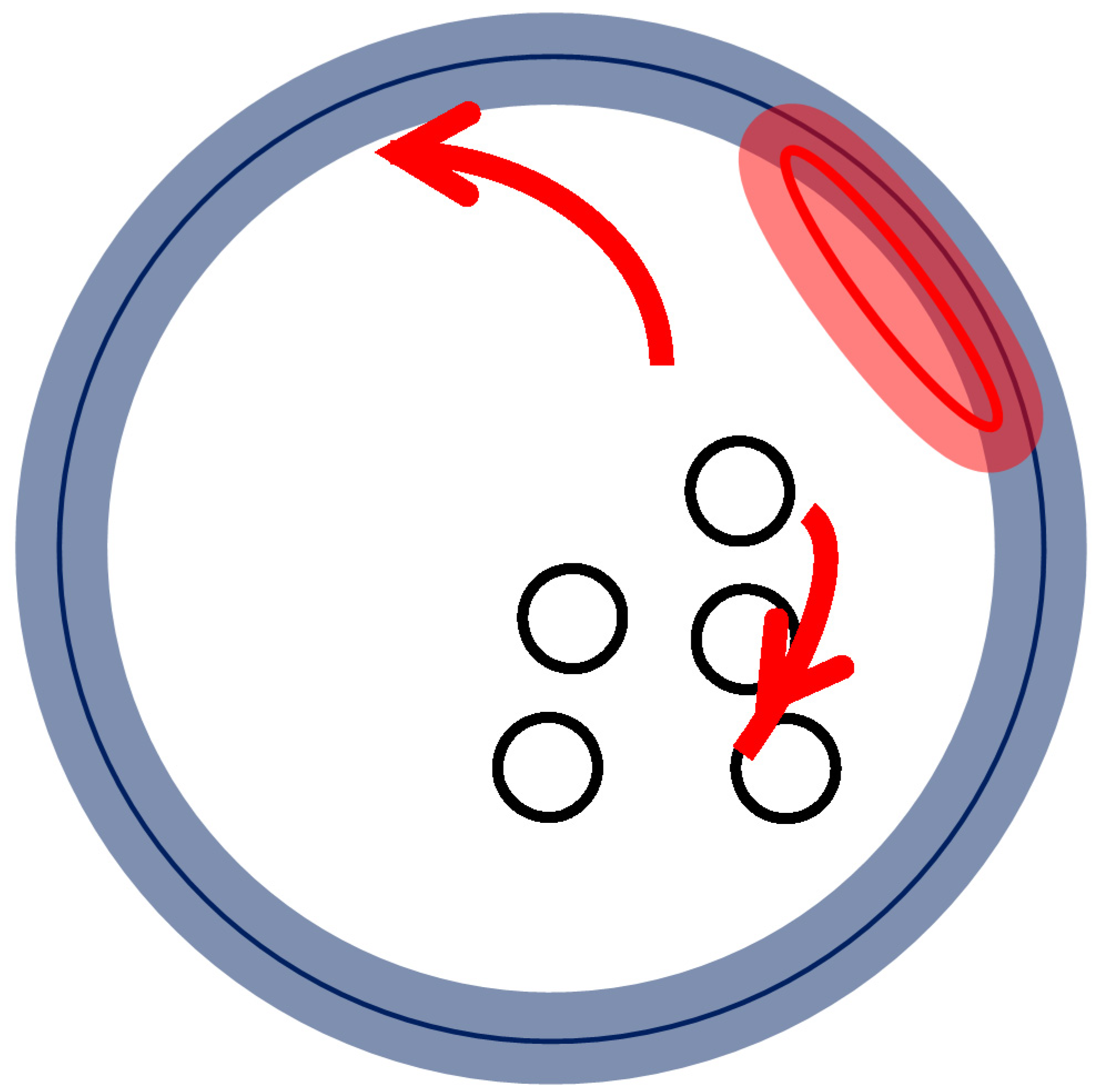
3.2. Adherent Moving of the Long SRP
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wu, F.B.; Japaridze, A.; Zheng, X.; Wiktor, J.; Kerssemakers, J.W.J.; Dekker, C. Direct imaging of the circular chromosome in a live bacterium. Nat. Commun. 2019, 10, 2194. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shao, Y.Y.; Lu, N.; Cai, C.; Zhou, F.; Wang, S.S.; Zhao, Z.H.; Zhao, G.P.; Zhou, J.Q.; Xue, X.L.; Qin, Z.J. A single circular chromosome yeast. Cell Res. 2019, 29, 87–89. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jacob, F.; Wollman, E.L. Genetic and physical determinations of chromosomal segments in Escherichia coli. Sym. Soc. Exp. Biol. 1958, 12, 75–92. [Google Scholar]
- Freifelder, D.; Kleinschmidt, A.K.; Sinsheimer, R.L. Electron microscopy of single-stranded DNA: Circularity of DNA of bacteriophage ϕX174. Science 1964, 146, 254–255. [Google Scholar] [CrossRef] [PubMed]
- Wasserman, S.A.; Cozzarelli, N.R. Biochemical topology: Applications to DNA recombination and replication. Science 1986, 232, 951–960. [Google Scholar] [CrossRef] [PubMed]
- Kundukad, B.; Yan, J.; Doyle, P.S. Efect of YOYO-1 on the mechanical properties of DNA. Soft Matter 2014, 10, 9721–9728. [Google Scholar] [CrossRef]
- Ireland, D.C.; Clark, R.J.; Daly, N.L.; Craik, D.J. Isolation, sequencing, and structure–activity relationships of cyclotides. J. Nat. Prod. 2010, 73, 1610–1622. [Google Scholar] [CrossRef] [PubMed]
- Parisi, D.; Truzzolillo, D.; Deepak, V.D. Transition from confined to bulk dynamics in symmetric star–linear polymer mixtures. Macromolecules 2019, 52, 5872–5883. [Google Scholar] [CrossRef] [Green Version]
- Li, S.J.; Ding, M.M.; Shi, T.F. Spatial distribution of entanglements and dynamics in polymer films confined by smooth walls. Polymer 2019, 172, 365–371. [Google Scholar] [CrossRef]
- Song, J.; Kahraman, R.; Collinson, D.W. Temperature effects on the nanoindentation characterization of stiffness gradients in confined polymers. Soft Matter 2019, 15, 359–370. [Google Scholar] [CrossRef]
- Raffaele, P.; Alessio, D.; Mose, C. Influence of wall heterogeneity on nanoscopically confined polymers. Phys. Chem. Chem. Phys. 2019, 21, 772–779. [Google Scholar]
- Zuo, B.; Zhou, H.; Davis, M.J.B. Effect of local chain conformation in adsorbed nanolayers on confined polymer molecular mobility. Phys. Rev. Lett. 2019, 122, 217801. [Google Scholar] [CrossRef] [PubMed]
- Jun, S.; Mulder, B. Entropy-driven spatial organization of highly confined polymers: Lessons for the bacterial chromosome. Proc. Natl. Acad. Sci. USA 2006, 103, 12388–12393. [Google Scholar] [CrossRef] [Green Version]
- e Silva, M.S.; Alvarado, J.; Nguyen, J.; Georgoulia, N.; Mulder, B.; Koenderink, G. Self-organized patterns of actin filaments in cell-sized confinement. Soft Matter 2011, 7, 10631–10641. [Google Scholar] [CrossRef]
- Qi, W.; Yan, X.; Fei, J.; Wang, A.; Cui, Y.; Li, J. Triggered release of insulin from glucose-sensitive enzyme multilayer shells. Biomaterials 2009, 30, 2799–2806. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Wang, L.; Li, B.; Cheng, Y.; Zhou, D.; Chen, X.; Jing, X.; Huang, Y. Compact vesicles self-assembled from binary graft copolymers with high hydrophilic fraction for potential drug/protein delivery. ACS Macro Lett. 2017, 6, 1186–1190. [Google Scholar] [CrossRef] [PubMed]
- Falk, M.; Feodorova, Y.; Naumova, N.; Imakaev, M.; Lajoie, B.; Leonhardt, H.; Joffe, B.; Dekker, J.; Fudenberg, G.; Solovei, I.; et al. Heterochromatin drives compartmentalization of inverted and conventional nuclei. Nature 2019, 510, 395. [Google Scholar] [CrossRef] [PubMed]
- Halverson, J.D.; Smrek, J.; Kremer, K.; Grosberg, A.Y. From a melt of rings to chromosome territories: The role of topological constraints in genome folding. Rep. Prog. Phys. 2014, 77, 022601. [Google Scholar] [CrossRef] [Green Version]
- Marenduzzo, D.; Micheletti, C.; Orlandini, E. Biopolymer organization upon confinement. J. Phys. Condens. Matter 2010, 22, 283102. [Google Scholar] [CrossRef] [PubMed]
- Bolzer, A.; Kreth, G.; Solovei, I.; Koehler, D.; Saracoglu, K.; Fauth, C.; Eils, S.R.; Cremer, C.; Speicher, M.R.; Cremer, T. Three-dimensional maps of all chromosomes in human male fibroblast nuclei and prometaphase rosettes. PLoS Biol. 2005, 3, 157. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Brangwynne, C.P.; Eckmann, C.R.; Courson, D.S.; Rybarska, A.; Hoege, C.; Gharakhani, J.; Julicher, F.; Hyman, A.A. Germline P Granules Are Liquid Droplets That Localize by Controlled Dissolution/Condensation. Science 2009, 324, 1729–1732. [Google Scholar] [CrossRef]
- Joyeux, M. A segregative phase separation scenario of the formation of the bacterial nucleoid. Soft Matter 2018, 14, 7368–7381. [Google Scholar] [CrossRef] [PubMed]
- Bernabei, M.; Bacova, P.; Moreno, A.J. Fluids of semiflexible ring polymers: Effective potentials and clustering. Soft Matter 2013, 9, 1287–1300. [Google Scholar] [CrossRef] [Green Version]
- Poier, P.; Bacova, P.; Moreno, A.J.; Likos, C.N.; Blaak, R. Anisotropic effective interactions and stack formation in mixtures of semiflexible ring polymers. Soft Matter 2016, 12, 4805–4820. [Google Scholar] [CrossRef] [PubMed]
- Ostermeir, K.; Alim, K.; Frey, E. Buckling of stiff polymer rings in weak spherical confinement. Phys. Rev. E 2010, 81, 061802. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ostermeir, K.; Alim, K.; Frey, E. Confinement induces conformational transition of semiflexible polymer rings to figure eight form. Soft Matter 2010, 6, 3467–3471. [Google Scholar] [CrossRef] [Green Version]
- Jeong, S.; Cho, S.; Kim, J.M.; Baig, C. Interfacial Molecular structure and dynamics of confined ring polymer melts under shear Flow. Macromolecules 2018, 51, 4670–4677. [Google Scholar] [CrossRef]
- Fritsche, M.; Heermann, D.W. Confinement driven spatial organization of semiflexible ring polymers: Implications for biopolymer packaging. Soft Matter 2011, 7, 6906–6913. [Google Scholar] [CrossRef]
- Tubiana, L.; Orlandini, E.; Micheletti, C. Multiscale Entanglement in ring polymers under spherical confinement. Phys. Rev. Lett. 2011, 107, 188302. [Google Scholar] [CrossRef] [PubMed]
- Micheletti, C.; Marenduzzo, D.; Orlandini, E.; Sumners, D.W. Knotting of random ring polymers in confined spaces. J. Chem. Phys. 2006, 124, 064903. [Google Scholar] [CrossRef] [Green Version]
- Ding, M.M.; Duan, X.Z.; Lu, Y.Y.; Shi, T.F. Flow-induced ring polymer translocation through nanopores. Macromolecules 2015, 48, 6002–6007. [Google Scholar] [CrossRef]
- Gutjahr, P.; Lipowsky, R.; Kierfeld, J. Semiflflexible polymer rings on topographically and chemically structured Surfaces. Soft Matter 2010, 6, 5461–5475. [Google Scholar] [CrossRef]
- Polson, J.M.; Kerry, D.R.M. Segregation of polymers under cylindrical confinement: Effects of polymer topology and crowding. Soft Matter 2018, 14, 6360–6373. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Minton, A.P. The influence of macromolecular crowding and macromolecular confinement on biochemical reactions in physiological media. J. Biol. Chem. 2001, 276, 10577–10580. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gaitho, F.M.; Tsige, M.; Mola, G.T.; Pellicane, G. Surface segregation of cyclic chains in binary melts of thin polymer films: The Influence of Constituent Concentration. Polymers 2018, 10, 324. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gómez, L.R.; García, N.A.; Pöschel, T. Packing structure of semiflexible rings. Proc. Natl. Acad. Sci. USA 2020, 117, 3382–3387. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhou, Y.C.; Hsiao, K.W.; Regan, K.E.; Kong, D.J.; McKenna, G.B.; Robertson-Anderson, R.M.; Schroeder, C.M. Effect of molecular architecture on ring polymer dynamics in semidilute linear polymer solutions. Nat. Commun. 2019, 10, 1753. [Google Scholar] [CrossRef] [Green Version]
- Zhou, X.L.; Li, K.; Guo, F.C.; Zhang, L.X. Ordered aggregation structures of semiflexible ring polymers in ring-linear blends. Polymer 2019, 175, 129–136. [Google Scholar] [CrossRef]
- Witz, G.; Rechendorff, K.; Adamcik, J.; Dietler, G. Conformation of ring polymers in 2D constrained environments. Phys. Rev. Lett. 2011, 106, 248301. [Google Scholar] [CrossRef] [Green Version]
- Zhou, X.L.; Liu, L.; Chen, J.M.; Zhang, L.X. Unusual conformations of semiflexible ring polymers confined in two parallel surfaces. Polymer 2018, 157, 180–189. [Google Scholar] [CrossRef]
- Grest, G.S.; Kremer, K. Molecular dynamics simulation for polymers in the presence of a heat bath. Phys. Rev. A 1986, 33, 3628–3631. [Google Scholar] [CrossRef] [PubMed]
- Cifra, P. Channel confinement of flexible and semiflexible macromolecules. J. Chem. Phys. 2009, 131, 224903. [Google Scholar] [CrossRef] [PubMed]
- Narros, A.; Moreno, A.J.; Likos, C.N. Influence of topology on effective potentials: Coarse-graining ring polymers. Soft Matter 2010, 6, 2435–2441. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Dinsmore, A.D.; Yodh, A.G. Phase diagrams of nearly-hard-sphere binary colloids. Phys. Rev. E 1995, 52, 4045–4057. [Google Scholar] [CrossRef]
- Dinsmore, A.D.; Wong, D.T.; Nelson, P.; Yodh, A.G. Hard spheres in vesicles: Curvature-induced forces and particle-induced curvature. Phys. Rev. Lett. 1998, 80, 409–412. [Google Scholar] [CrossRef] [Green Version]
- Asakura, S.; Oosawa, F. Surface tension of high-polymer solutions. J. Chem. Phys. 1954, 22, 1255. [Google Scholar] [CrossRef]
- Pais, A.A.C.C.; Miguel, M.G.; Linse, P.; Lindman, B. Polyelectrolytes confined to spherical cavities. J. Chem. Phys. 2002, 117, 1385. [Google Scholar] [CrossRef]
- Milchev, A.; Egorov, S.A.; Nikoubashman, A.; Binder, K. Conformations and orientational ordering of semiflexible polymers in spherical confinement. J. Chem. Phys. 2017, 146, 194907. [Google Scholar] [CrossRef] [Green Version]
- Sarabadani, J.; Milchev, A.; Vilgis, T.A. Structure and dynamics of polymer melt confined between two solid surfaces: A molecular dynamics study. J. Chem. Phys. 2014, 141, 044907. [Google Scholar] [CrossRef]
- Milchev, A.; Egorov, S.A.; Nikoubashman, A.; Binder, K. Adsorption and structure formation of semiflflexible polymers on spherical surfaces. Polymer 2018, 145, 463–472. [Google Scholar] [CrossRef]
- Zhou, Y.X.; Milner, S.T. Short-time dynamics reveals Tg suppression in simulated polystyrene thin films. Macromolecules 2017, 50, 5599–5610. [Google Scholar] [CrossRef]
- Milchev, A.; Egorov, S.A.; Vega, D.A.; Binder, K.; Nikoubashman, A. Densely Packed semiflflexible macromolecules in a rigid spherical capsule. Macromolecules 2018, 51, 2002–2016. [Google Scholar] [CrossRef]
- Nikoubashman, A.; Vega, D.A.; Binder, K.; Milchev, A. Semiflexible polymers in spherical confinement: Bipolar orientational order versus tennis ball states. Phys. Rev. Lett. 2017, 118, 217803. [Google Scholar] [CrossRef]
- Vacatello, M. Monte Carlo Simulations of the interface between polymer melts and solids. effects of chain stiffness. Macromol. Theory Simul. 2001, 10, 187–195. [Google Scholar] [CrossRef]
- Lee, E.; Jung, Y.J. Segregated structures of ring polymer melts near the surface: A molecular dynamics simulation study. Soft Matter 2015, 11, 6018–6028. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- DeYoung, A.D.; Park, S.W.; Dhumal, N.R.; Shim, Y.; Jung, Y.; Kim, H.J. Graphene oxide supercapacitors: A computer simulation study. J. Phys. Chem. C 2014, 118, 18472–18480. [Google Scholar] [CrossRef]
- Morrison, G.; Thirumalai, D. Semiflexible chains in confined spaces. Phys. Rev. E 2009, 79, 011924. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jones, R.L.; Kumar, S.K.; Ho, D.L.; Briber, R.M.; Russell, T.P. Chain conformation in ultrathin polymer films. Nature 1999, 400, 146. [Google Scholar] [CrossRef]
- Riggleman, R.A.; Yoshimoto, K.; Douglas, J.F.; de Pablo, J.J. Influence of confinement on the fragility of antiplasticized and pure polymer films. Phys. Rev. Lett. 2006, 97, 045502. [Google Scholar] [CrossRef]
- Micheletti, C.; Marenduzzo, D.; Orlandini, E. Polymers with spatial or topological constraints: Theoretical and computational results. Phys. Rep. 2011, 504, 1–73. [Google Scholar] [CrossRef] [Green Version]
- Kato, A.; Shindo, E.; Sakaue, T.; Tsuji, A.; Yoshikawa, K. Conformational transition of giant DNA in a confined space surrounded by a phospholipid membrane. Biophys. J. 2009, 97, 1678. [Google Scholar] [CrossRef] [PubMed]
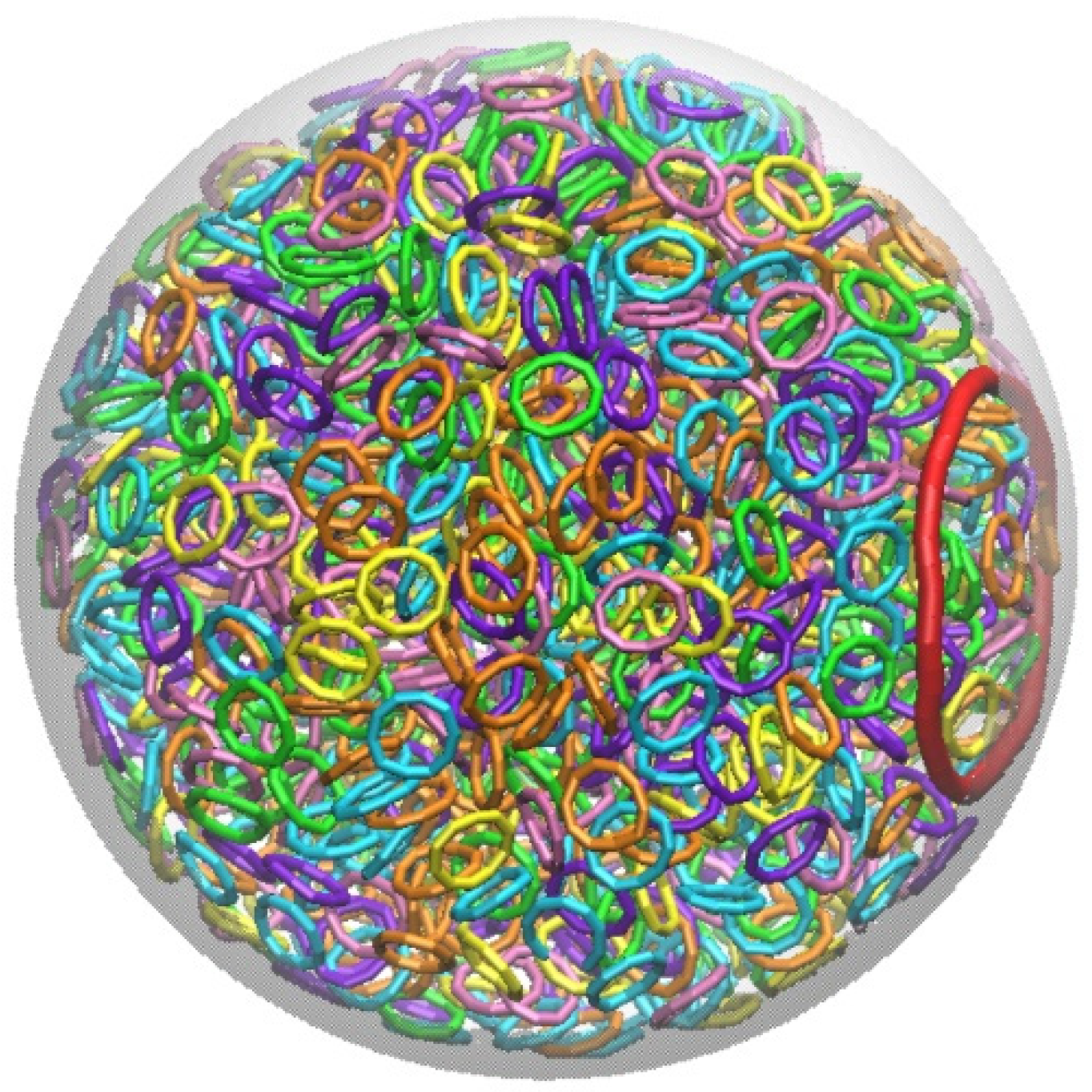
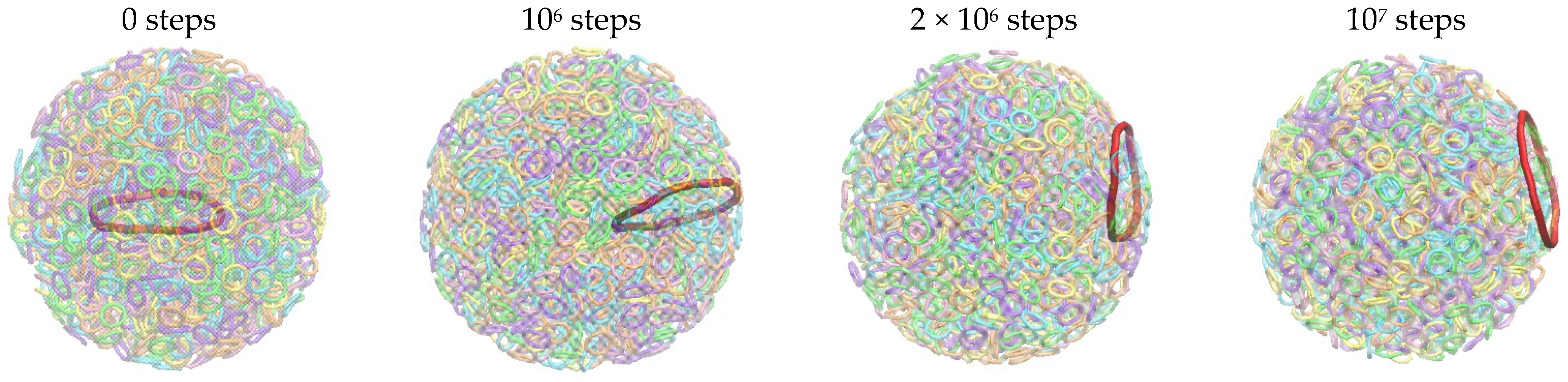
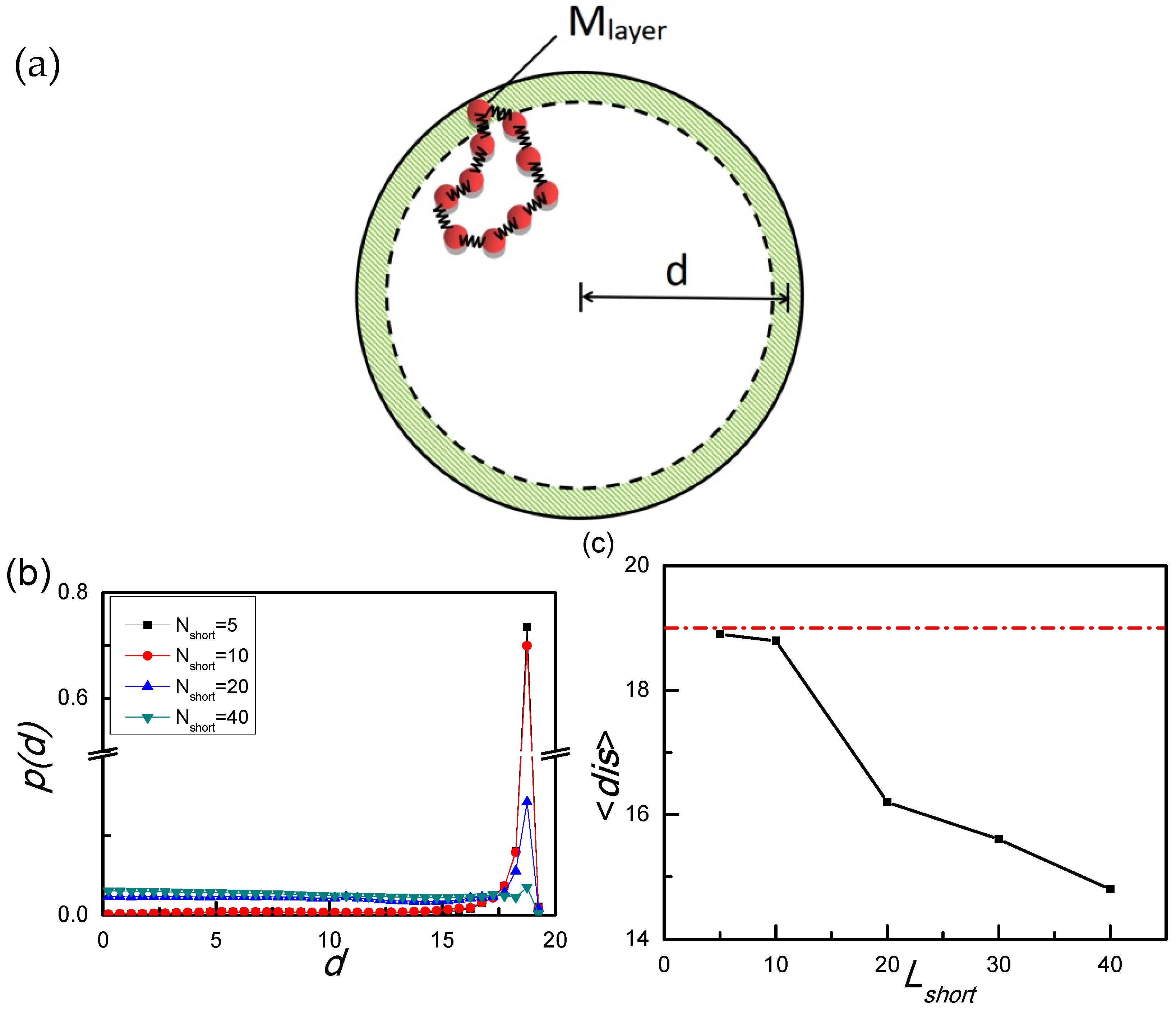
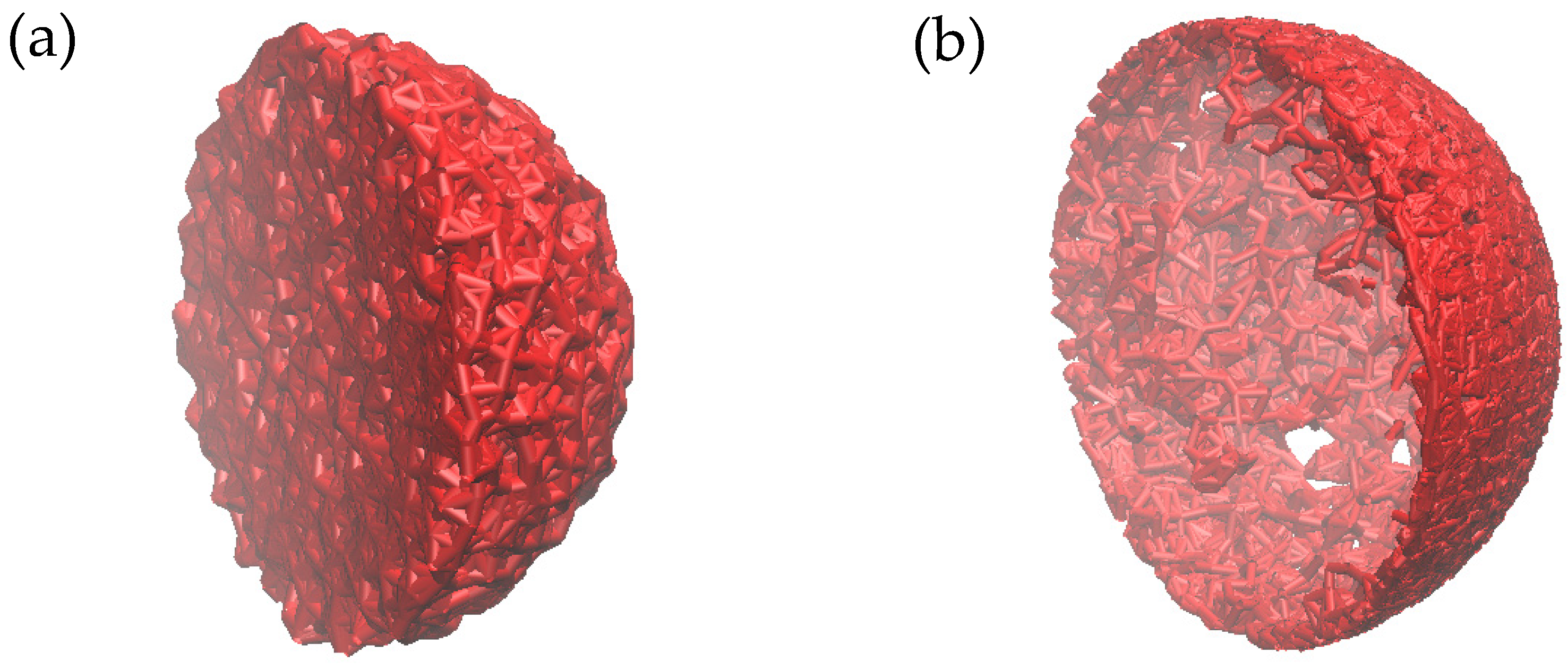
| Radius of Sphere | Llong | Nlong | Lshrot | Nshort | Llong ∗ Nlong + Lshort ∗ Nshort |
|---|---|---|---|---|---|
| 20 | 50 | 1 | 5 | 2863 | 14,365 |
| 10 | 1432 | ||||
| 20 | 716 | ||||
| 30 | 477 | ||||
| 40 | 358 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, X.; Wei, W. Adherent Moving of Polymers in Spherical Confined Binary Semiflexible Ring Polymer Mixtures. Biophysica 2022, 2, 525-537. https://doi.org/10.3390/biophysica2040044
Zhou X, Wei W. Adherent Moving of Polymers in Spherical Confined Binary Semiflexible Ring Polymer Mixtures. Biophysica. 2022; 2(4):525-537. https://doi.org/10.3390/biophysica2040044
Chicago/Turabian StyleZhou, Xiaolin, and Wan Wei. 2022. "Adherent Moving of Polymers in Spherical Confined Binary Semiflexible Ring Polymer Mixtures" Biophysica 2, no. 4: 525-537. https://doi.org/10.3390/biophysica2040044





