On the Scalability of a Membrane Unit for Ultrapure Hydrogen Separation
Abstract
:1. Introduction
2. The Experimental Setup
2.1. Rationale of the Scale-Up Tests
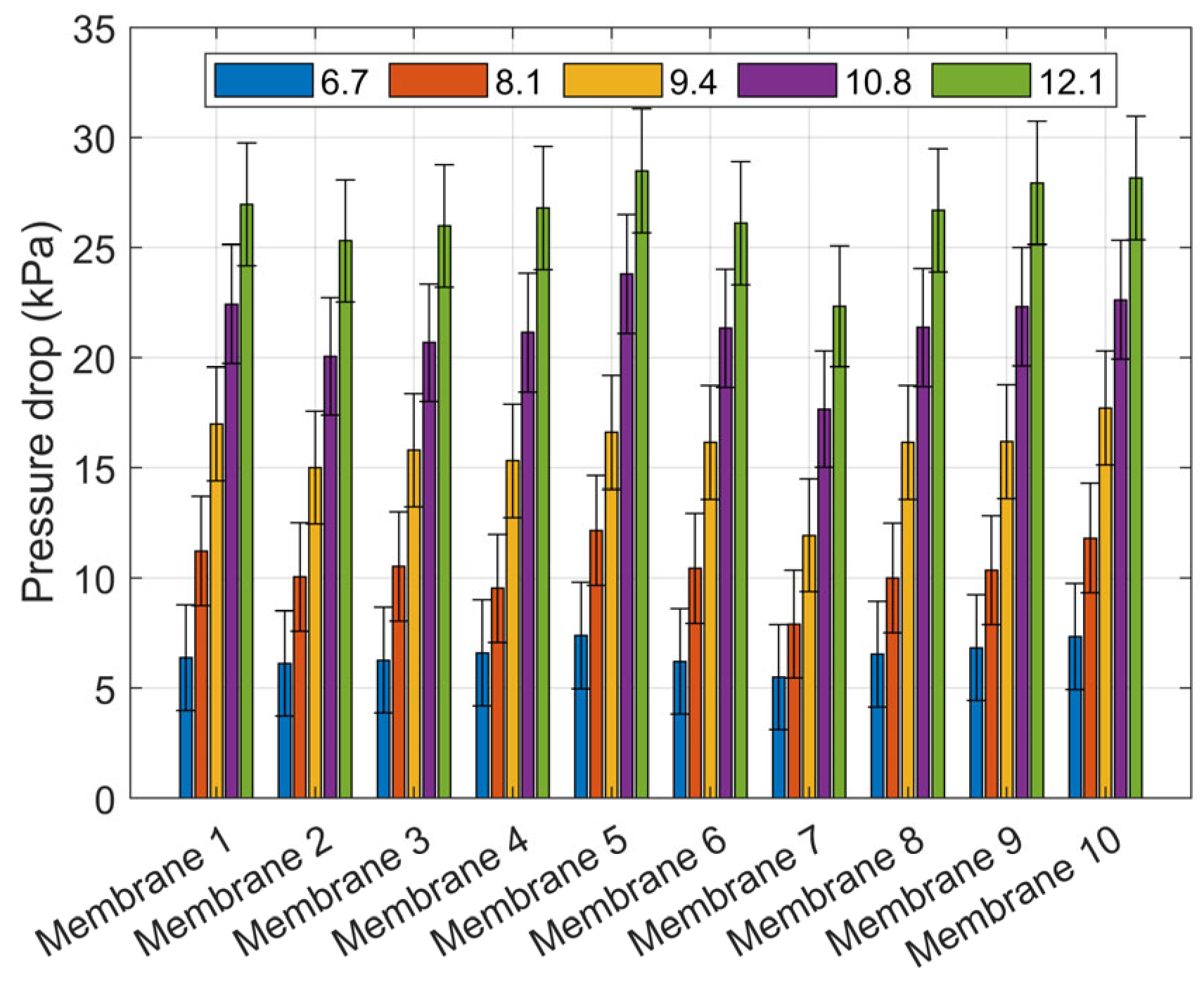
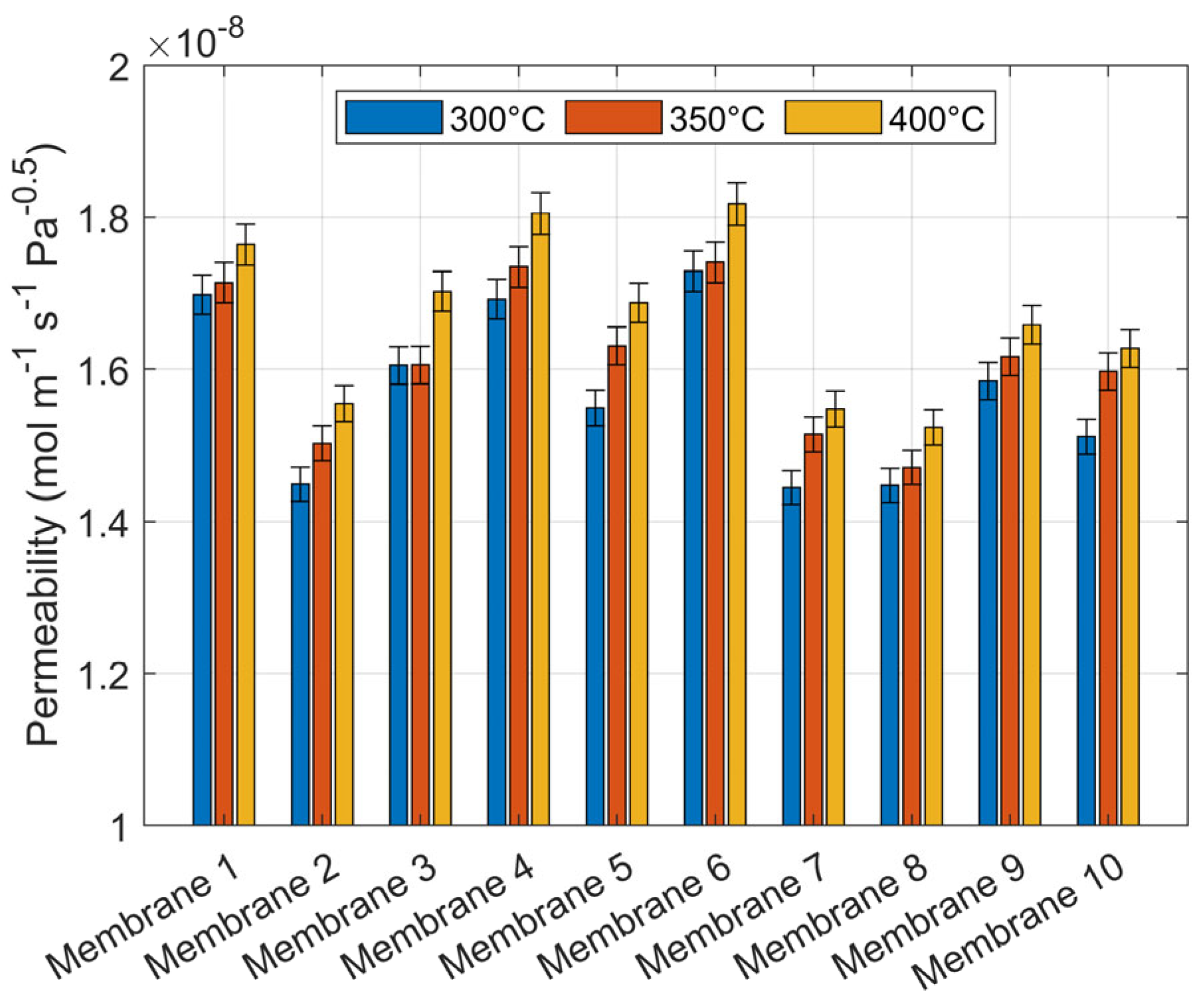
2.2. Characterization Tests
3. The Experimental Activity
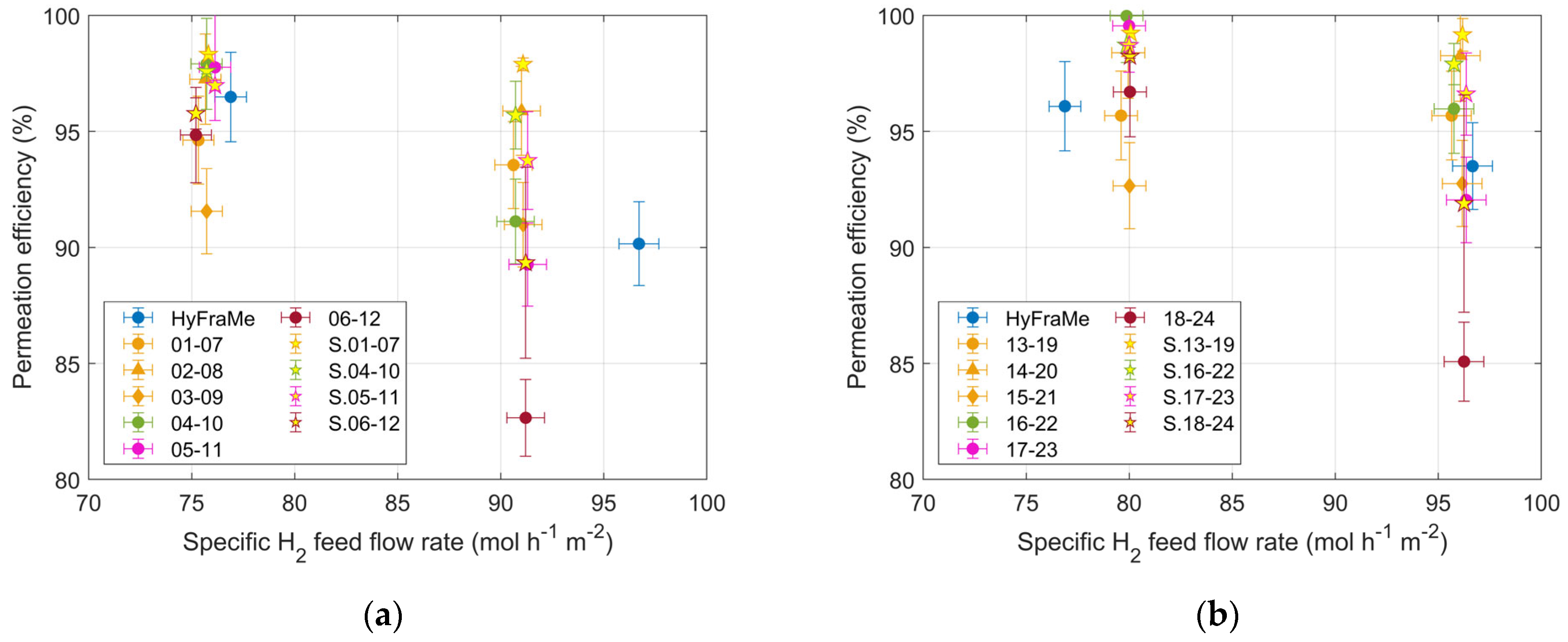
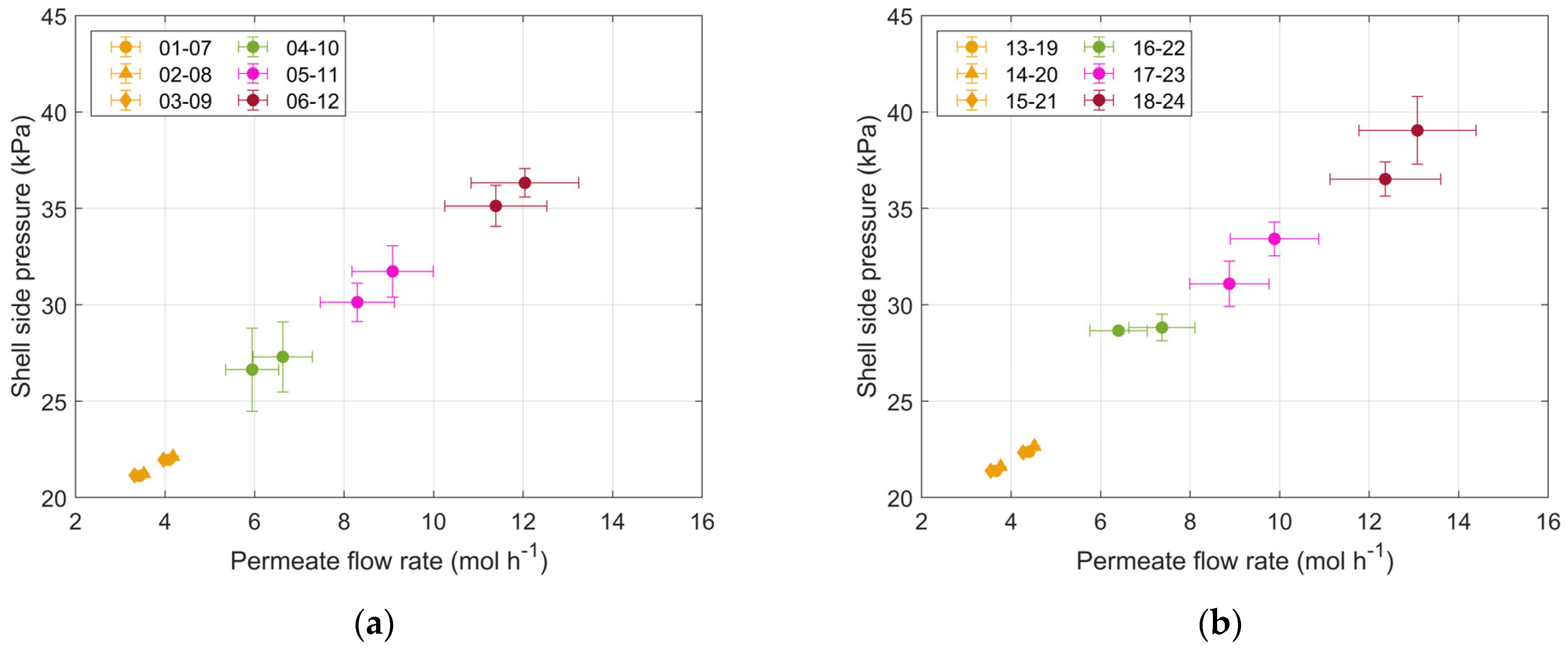
3.1. The Experimental Test Matrix
3.2. Analysis of the Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Deville, H.; Troost, L. Sur la permeabilitè du fer a haute temperature. Comptes Rend. 1863, 57, 965–967. [Google Scholar]
- Deville, H. Note sur le passage des gaz au travers des corps solides homogènes. Comptes Rend. 1864, 59, 102. [Google Scholar]
- Graham, T. On the adsorption and dialytic separation of gases by colloid septa. Philos. Trans. R. Soc. 1866, 156, 399–439. [Google Scholar] [CrossRef]
- Alique, D.; Martinez-Diaz, D.; Sanz, R.; Calles, J.A. Review of Supported Pd-Based Membranes Preparation by Electroless Plating for Ultra-Pure Hydrogen Production. Membranes 2018, 8, 5. [Google Scholar] [CrossRef] [PubMed]
- Martino, M.; Ruocco, C.; Meloni, E.; Pullumbi, P.; Palma, V. Main Hydrogen Production Processes: An Overview. Catalysts 2021, 11, 547. [Google Scholar] [CrossRef]
- Jokar, S.M.; Farokhnia, A.; Tavakolian, M.; Pejman, M.; Parvasi, P.; Javanmardi, J.; Zare, F.; Gonçalves, M.C.; Basile, A. The recent areas of applicability of palladium based membrane technologies for hydrogen production from methane and natural gas: A review. Int. J. Hydrogen Energy 2023, 48, 6451–6476. [Google Scholar] [CrossRef]
- Adams, B.D.; Chen, A. The role of palladium in a hydrogen economy. Mater. Today 2011, 14, 282–289. [Google Scholar] [CrossRef]
- Boyano, A.; Blanco-Marigorta, A.M.; Morosuk, T.; Tsatsaronis, G. Exergoenvironmental analysis of a steam methane reforming process for hydrogen production. Energy 2011, 36, 2202–2214. [Google Scholar] [CrossRef]
- Ockwig, N.W.; Nenoff, T.M. Membranes for hydrogen separation. Chem. Rev. 2007, 107, 4078–4110. [Google Scholar] [CrossRef]
- Soltani, R.; Rosen, M.A.; Dincer, I. Assessment of CO2 capture options from various points in steam methane reforming for hydrogen production. Int. J. Hydrogen Energy 2014, 39, 20266–20275. [Google Scholar] [CrossRef]
- Yuan, M.; Lee, K.; Van Campen, D.G.; Liguori, S.; Tnoey, M.F.; Wilcox, J. Hydrogen Purification in Palladium-Based Membranes: An Operando X-ray Diffraction Study. Ind. Eng. Chem. Res. 2019, 58, 926–934. [Google Scholar] [CrossRef]
- Shirasaki, Y.; Tsuneki, T.; Ota, Y.; Yasuda, I.; Tachibana, S.; Nakajima, H.; Kobayashi, K. Development of membrane reformer system for highly efficient hydrogen production from natural gas. Int. J. Hydrogen Energy 2009, 34, 4482–4487. [Google Scholar] [CrossRef]
- Khalilpour, R.; Mumford, K.; Zhai, H.; Abbas, A.; Stevens, G.; Rubin, E.S. Membrane-based carbon capture from flue gas: A review. J. Clean. Prod. 2015, 103, 286–300. [Google Scholar] [CrossRef]
- Adhikari, S.; Fernando, S. Hydrogen membrane separation techniques. Ind. Eng. Chem. Res. 2006, 45, 775–881. [Google Scholar] [CrossRef]
- Wilson, J.; Becnel, J.; Demange, D.; Rogers, B. The ITER Tokamak Exhaust Processing System Design and Substantiation. Fusion Sci. Technol. 2019, 75, 794–801. [Google Scholar] [CrossRef]
- Day, C.; Butler, B.; Giegerich, T.; Ploeckl, B.; Varaoutis, S. A smart three-loop fuel cycle architecture for DEMO. Fusion Eng. Des. 2019, 146, 2462–2468. [Google Scholar] [CrossRef]
- Helmi, A.; Fernandez, E.; Melendez, J.; Tanaka, D.A.P.; Gallucci, F.; Annaland, M.V. Fluidized Bed Membrane Reactors for Ultra Pure H2 Production-A Step forward towards Commercialization. Molecules 2016, 21, 376. [Google Scholar] [CrossRef] [PubMed]
- Santucci, A.; Tosti, S. Membrane technology for tritium recovery in fusion power plants. In Current Trends and Future Developments on (Bio-) Membranes: Advances on Membrane Engineering; Basile, A., Lipnizki, F., Rahimpour, M.R., Piemonte, V., Eds.; Elsevier: Amsterdam, The Netherlands, 2024; pp. 445–470. [Google Scholar]
- Craft, A. A Case Study Approach: Summary of Some Results on the Effects of Hydrogen Exposure on the Mechanical Properties of Palladium and the Alloy Systems Pd1−xMx, M = Ag, Cu, Mn; x = 5 − 0.25. Hydrogen 2023, 4, 237–256. [Google Scholar] [CrossRef]
- Fernandez, E.; Gallucci, F.; Pacheco Tanaka, D.A. Palladium-Based Membrane (Palladium Alloy Membrane). In Encyclopedia of Membranes; Drioli, E., Giorno, L., Eds.; Springer: Berlin/Heidelberg, Germany, 2015; pp. 1–4. [Google Scholar]
- Livshits, A.I. The hydrogen transport through the metal alloy membranes with a spatial variation of the alloy composition: Potential diffusion and enhanced permeation. Int. J. Hydrogen Energy 2017, 42, 13111–13119. [Google Scholar] [CrossRef]
- Plazaola, A.A.; Pacheco Tanaka, D.A.; Van Sint Annaland, M.; Gallucci, F. Recent Advances in Pd-Based Membranes for Membrane Reactors. Molecules 2017, 22, 51. [Google Scholar] [CrossRef] [PubMed]
- Narcisi, V.; Tamborrini, L.; Farina, L.; Cortese, G.; Romanelli, F.; Santucci, A. Experimental and Numerical Analysis of a Pd–Ag Membrane Unit for Hydrogen Isotope Recovery in a Solid Blanket. Membranes 2023, 13, 578. [Google Scholar] [CrossRef] [PubMed]
- Antunes, R.; Frances, L.; Incelli, M.; Santucci, A. Numerical study and experimental verification of protium permeation through Pd/Ag membranes for fusion applications. Fusion Eng. Des. 2019, 146, 1286–1290. [Google Scholar] [CrossRef]
- Barbiere, G.; Brunetti, A.; Tricoli, G.; Drioli, E. An innovative configuration of a Pd-based membrane reactor for the production of pure hydrogen: Experimental analysis of water gas shift. J. Power Sources 2008, 182, 160–167. [Google Scholar] [CrossRef]
- Incelli, M.; Santucci, A.; Tosti, S.; Sansovini, M.; Carlini, M. Heavy water decontamination tests through a Pd-Ag membrane reactor: Water Gas Shift and Isotopic Swamping performances. Fusion Eng. Des. 2017, 124, 692–695. [Google Scholar] [CrossRef]
- Incelli, M.; Santucci, A.; Tosti, S.; Mallozzi, F.; Sansovini, M.; Marini, F. Design and first experimental results of a new multi-tube Pd-Ag membrane module. Fusion Eng. Des. 2019, 146, 2729–2733. [Google Scholar] [CrossRef]
- Boeltken, T.; Belimov, M.; Pfeifer, P.; Peters, T.A.; Bredesen, R.; Dittmeyer, R. Fabrication and testing of a planar microstructured concept module with integrated palladium membranes. Chem. Eng. Process. 2013, 67, 136–147. [Google Scholar] [CrossRef]
- Chabot, J.; Lecomte, J.; Grumet, C.; Sannier, J. Fuel clean-up system: Poisoning of palladium-silver membranes by gaseous impurities. Fusion Technol. 1988, 14, 614–618. [Google Scholar] [CrossRef]
- Serra, E.; Kemali, M.; Perujo, A.; Ross, D.K. Hydrogen and Deuterium in Pd-25 Pct Ag alloy: Permeation, diffusion, solubilization, and surface reaction. Metall. Mater. Trans. A 1998, 29, 1023–1028. [Google Scholar] [CrossRef]
- Scura, F.; Barbieri, G.; Drioli, E. H2 for PEM-FC: Effect of CO in the purification by means of Pd-based membranes. Desalination 2006, 200, 239–241. [Google Scholar] [CrossRef]
- Federici, G. Testing needs for the development and qualification of a breeding blanket for DEMO. Nucl. Fusion 2023, 63, 125002. [Google Scholar] [CrossRef]
- Hernández, F.A.; Pereslavtsev, P.; Zhou, G.; Kanga, Q.; D’Amico, S.; Neubergera, H.; Boccaccini, L.V.; Kiss, B.; Nádasic, G.; Maqueda, L.; et al. Consolidated design of the HCPB Breeding Blanket for the pre-Conceptual Design Phase of the EU DEMO and harmonization with the ITER HCPB TBM program. Fus. Eng. Des. 2020, 157, 111614. [Google Scholar] [CrossRef]
- Palermo, I.; Hernández, F.A.; Pereslavtsev, P.; Rapisarda, D.; Zhou, G. Shielding Design Optimization of the Helium-Cooled Pebble Bed Breeding Blanket for the EU DEMO Fusion Reactor. Energies 2022, 15, 5734. [Google Scholar] [CrossRef]
- Qi, Q.; Zhao, M.; Sun, F.; Zhang, Y.; Gu, S.; Ji, B.; Zhou, H.S.; Oya, Y.; Liu, S.; Luo, G.N. Investigation on effects of tritium release behavior in Li4SiO4 pebbles. Nucl. Mater. Energy 2021, 28, 101036. [Google Scholar] [CrossRef]
- Cristescu, I.; Draghia, M. Developments on the tritium extraction and recovery system for HCPB. Fusion Eng. Des. 2020, 158, 111558. [Google Scholar] [CrossRef]

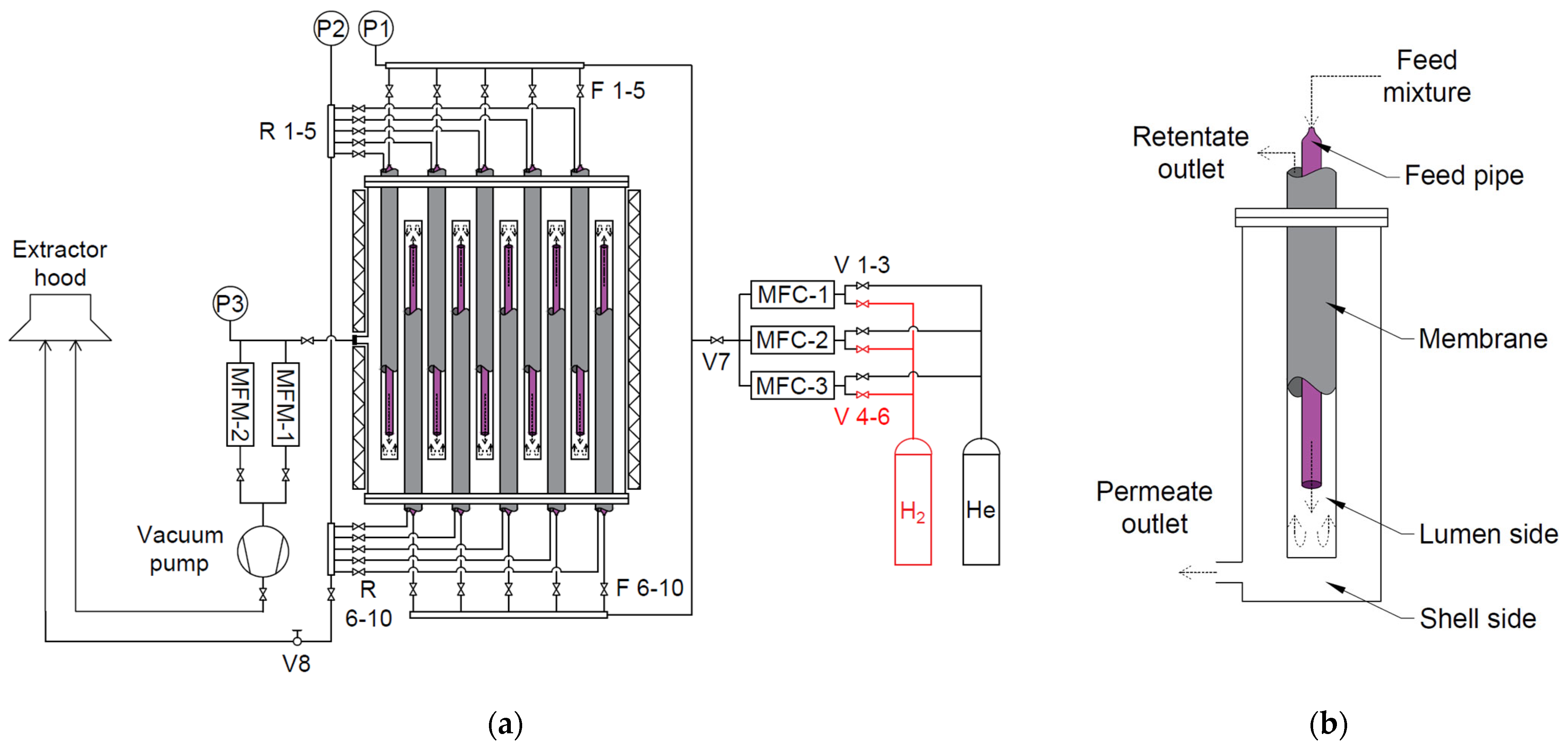

| Item | Model | Range | Accuracy |
|---|---|---|---|
| MFC-1 | MKS, GE50A series | 0–5000 sccm | ±1% of reading between 20% and 100% of full scale; ±0.2% of full scale between 2% and 20% of full scale |
| MFC-2 | 0–5000 sccm | ||
| MFC-3 | 0–1000 sccm | ||
| MFM-1 | 0–5000 sccm | ||
| MFM-2 | 0–10,000 sccm | ||
| P1 | MKS, Baratron® Type 722B | 105–106 Pa | ±0.5% of reading |
| P2 | |||
| P3 | PFEIFFER, CMR 271 | 101–1.1 × 105 | ±0.15% of reading |
| Parameter | HyFraMe | MeSMeR |
|---|---|---|
| Heating mode | Direct heating via the Joule effect | Thermal radiation through shell walls |
| Temperature monitoring | Three thermocouples installed on the outside surface of the membrane | Three thermocouples measuring bulk temperature on the shell side |
| Flow distribution and distortion | No distortion | Total flow rate elaborated through MFCs; possible distortion in the flow distribution through parallel membranes |
| Manufacture distortion | No distortion | Distortion due to manufacturing discrepancies in membranes |
| Membrane | Length (mm) | Inner Diameter (mm) | Thickness (µm) |
|---|---|---|---|
| 1 | 510 | 9.99 | 120 |
| 2 | 507 | 10.13 | 110 |
| 3 | 507 | 10.01 | 110 |
| 4 | 509 | 10.00 | 120 |
| 5 | 512 | 10.05 | 113 |
| 6 | 508 | 9.95 | 115 |
| 7 | 505 | 9.97 | 105 |
| 8 | 508 | 9.95 | 115 |
| 9 | 510 | 10.10 | 118 |
| 10 | 513 | 10.06 | 130 |
| Membrane | Pe0 (mol m−1 s−1 Pa−0.5) | ΔPe0 (mol m−1 s−1 Pa−0.5) | Ea (J mol−1) | ΔEa (J mol−1) |
|---|---|---|---|---|
| 1 | 2.21 × 10−8 | ±1.74 × 10−9 | 1278.69 | ±409.10 |
| 2 | 2.41 × 10−8 | ±2.06 × 10−11 | 2442.65 | ±4.42 |
| 3 | 2.41 × 10−8 | ±5.61 × 10−9 | 2015.31 | ±1201.80 |
| 4 | 2.67 × 10−8 | ±1.71 × 10−9 | 2205.70 | ±331.55 |
| 5 | 2.87 × 10−8 | ±1.55 × 10−9 | 2961.45 | ±278.66 |
| 6 | 2.45 × 10−8 | ±3.58 × 10−9 | 1709.36 | ±756.41 |
| 7 | 2.38 × 10−8 | ±2.12 × 10−9 | 2395.26 | ±459.23 |
| 8 | 2.08 × 10−8 | ±1.75 × 10−9 | 1769.22 | ±434.02 |
| 9 | 2.20 × 10−8 | ±6.05 × 10−10 | 1587.14 | ±142.23 |
| 10 | 2.58 × 10−8 | ±3.38 × 10−9 | 2557.39 | ±677.50 |
| Thickness (µm) | Pe0 (mol m−1 s−1 Pa−0.5) | Ea (J mol−1) | Reference |
|---|---|---|---|
| 250 | 4.95 × 10−8 | 5900.00 | [28,29] |
| 198–782 | 5.58 × 10−8 | 6300.00 | [28,30] |
| 60 | 9.7 × 10−7 | 25,800.00 | [28,31] |
| 84 | 2.95 × 10−8 | 2531.49 | [24] |
| 150 | 5.63 × 10−8 | 5456.06 | [24] |
| 113 | (2.58 ± 0.35) × 10−8 | 4013.90 ± 640.30 | [24] |
| Test ID | Feed Composition H2–He (%) | Specific Feed Flow Rate (mol h−1 m−2) | Specific H2 Feed Flow Rate (mol h−1 m−2) | Membranes |
|---|---|---|---|---|
| TCSm-01 | 90–10 | 84.2 | 75.8 | 1–3 |
| TCSm-02 | 1; 4; 6 | |||
| TCSm-03 | 2; 7; 8 | |||
| TCSm-04 | 1–5 | |||
| TCSm-05 | 1–7 | |||
| TCSm-06 | 1–10 | |||
| TCSm-07 | 101.3 | 91.2 | 1–3 | |
| TCSm-08 | 1; 4; 6 | |||
| TCSm-09 | 2; 7; 8 | |||
| TCSm-10 | 1–5 | |||
| TCSm-11 | 1–7 | |||
| TCSm-12 | 1–10 | |||
| TCSm-13 | 95–5 | 84.2 | 80.0 | 1–3 |
| TCSm-14 | 1; 4; 6 | |||
| TCSm-15 | 2; 7; 8 | |||
| TCSm-16 | 1–5 | |||
| TCSm-17 | 1–7 | |||
| TCSm-18 | 1–10 | |||
| TCSm-19 | 101.3 | 96.2 | 1–3 | |
| TCSm-20 | 1; 4; 6 | |||
| TCSm-21 | 2; 7; 8 | |||
| TCSm-22 | 1–5 | |||
| TCSm-13 | 1–7 | |||
| TCSm-14 | 1–10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Narcisi, V.; Farina, L.; Santucci, A. On the Scalability of a Membrane Unit for Ultrapure Hydrogen Separation. Hydrogen 2024, 5, 149-162. https://doi.org/10.3390/hydrogen5020010
Narcisi V, Farina L, Santucci A. On the Scalability of a Membrane Unit for Ultrapure Hydrogen Separation. Hydrogen. 2024; 5(2):149-162. https://doi.org/10.3390/hydrogen5020010
Chicago/Turabian StyleNarcisi, Vincenzo, Luca Farina, and Alessia Santucci. 2024. "On the Scalability of a Membrane Unit for Ultrapure Hydrogen Separation" Hydrogen 5, no. 2: 149-162. https://doi.org/10.3390/hydrogen5020010
APA StyleNarcisi, V., Farina, L., & Santucci, A. (2024). On the Scalability of a Membrane Unit for Ultrapure Hydrogen Separation. Hydrogen, 5(2), 149-162. https://doi.org/10.3390/hydrogen5020010






