Next Article in Journal
Multiphysics Studies of 3D Plate Fin Heat Exchanger Filled with Ortho-Para-Hydrogen Conversion Catalyst for Hydrogen Liquefaction
Previous Article in Journal
Liquid Organic Hydrogen Carriers: Hydrogenation Thermodynamics of Aromatic Esters
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Hydrogen
Volume 5
Issue 4
10.3390/hydrogen5040035
hydrogen-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Structures and Properties of MgTiHn Clusters (n ≤ 20)

by
Camryn Newland
1,
D. Balamurugan
2 and
Jonathan T. Lyon
1,*
1
Department of Chemistry, Murray State University, Murray, KY 42071, USA
2
Office of Advanced Research Computing, Rutgers University, Piscataway, NJ 08854, USA
*
Author to whom correspondence should be addressed.
Hydrogen 2024, 5(4), 669-681; https://doi.org/10.3390/hydrogen5040035
Submission received: 27 August 2024 / Revised: 26 September 2024 / Accepted: 27 September 2024 / Published: 3 October 2024
Browse Figures
Versions Notes

Abstract

:
Magnesium hydride solids doped with transition metals have received attention recently as prospective hydrogen storage materials for a green energy source and a hydrogen economy. In this study, MgTiHn (n = 1–20) clusters were investigated for the first time by employing the B3PW91 hybrid density functional theory computational chemistry technique with all electron basis sets to determine precise cluster structures and the maximum hydrogen capacity for this model system. We find that hydrogen atoms bind to the metal cluster core until a MgTiH14 saturation limit is reached, with hydrogen dissociation from this system occurring for MgTiH15 and larger cluster sizes. This MgTiH14 cluster contains a large 16.4% hydrogen by mass. This saturation size limit and hydrogen mass percent is larger than the analogous MgScHn system previously reported. The clusters relative stabilities and electronic properties are discussed along with a possible novel hydrogen dissociation pathway. MgTiH10 and MgTiH13 clusters are predicted to be especially stable species in this size range.

1. Introduction

Our modern-day society largely depends on fossil fuels as a main energy source. The continued use of these non-renewable resources raises concerns about their sustainability as well as the environmental impact of burning these fossil fuels. Carbon dioxide, a major byproduct of the combustion of fossil fuels, is a greenhouse gas causing a rise in Earth’s surface temperature [1]. In addition to climate change for future generations, burning fossil fuels can lead to health problems in the current population, such as cardiovascular and respiratory diseases [2].
The desire to reduce our current dependence on fossil fuels has led to interest in developing different clean alternative energy sources. Hydrogen has become an attractive alternative fuel candidate with a high energy density. In addition, water is the main byproduct of burning hydrogen gas. However, many challenges need to be overcome for hydrogen to be an efficient replacement for fossil fuels. One issue is that molecular hydrogen exists as a gas under ambient conditions, which has low particle density and leads to special safety and handling issues. Liquid hydrogen requires extremely low temperatures and high pressures [3]. However, solid compounds containing hydrogen are a plausible option for a safer and more efficient hydrogen storage material. Metal hydrides, in particular, have come to the forefront as a possible solid hydrogen storage material, as they can be handled more safely and are a practical alternative to other forms of hydrogen [4,5,6,7,8,9,10,11,12,13].
Magnesium hydrides have several advantages as a potential solid-state hydrogen storage metal hydride. For example, magnesium is abundant, relatively inexpensive, nontoxic, and lightweight, with a low molar mass [4,14,15,16,17]. Although the strong Mg-H bonding interactions lead to poor hydrogen sorption kinetics and thermodynamics in the solid, the hydrogen storage capacity and hydrogen sorption kinetics in magnesium hydrides have been noted to greatly improve by doping it with transition metals [18,19,20,21,22,23,24,25,26,27,28,29]. Titanium in particular is an attractive catalytic dopant in magnesium hydride systems [21,27]. MgxTiyHz systems of various compositions, for example, have shown to exhibit better (de)hydrogenation energetics and kinetics than both magnesium and titanium hydrides individually, and the hydrogen from some MgxTiyHz materials has been noted to be released from the solid at lower desorption temperatures than other solids [30,31]. However, much is still not fully understood about transition metal-doped magnesium hydrides, including the role of the metal dopant, the required and optimal dopant concentration, and specific details about the desorption mechanism. However, it has been suggested that the dopant atom migrates in the material when hydrogen is released and that the magnesium–metal interactions are critically important during the release of this molecular hydrogen [22,23,24,25,26,27,32,33]. To study real-world bulk processes in greater detail, small atomic clusters are frequently used as convenient models [34,35,36].
Recently, we have investigated small scandium–magnesium hydride clusters, i.e., MgScHn (n = 1–20), enhancing and extending the work of prior research [37]. It was noted in this investigation that these clusters became saturated at MgScH13 with a 15.9% hydrogen by mass, and that larger clusters contained dissociated H2 molecules. This dissociation was proposed to occur through a weakly bound H2 molecule coordinated to a negatively charged hydrogen atom on the cluster. Here, we investigate the impact of incorporating a different transition metal on the cluster properties, i.e., MgTiHn clusters in the same size range. This has significant importance due, in part, to the increased (de)hydrogen kinetics and energetics noted when titanium is used as a dopant in various magnesium hydride systems [21,27,30,31]. The major goals of this investigation are to explore what the hydrogen saturation size limit is for this cluster system and if it changes based on the dopant atom; to determine what the role of a different transition metal atom, specifically titanium, plays in the dissociation pathway and the preferred cluster structures; and to investigate the similarities and differences in cluster energetics and electronics with different dopant transition metal atoms as a function of increasing size.

2. Computational Methods

Candidate cluster isomers were generated using the unbiased Artificial Bee Colony algorithm implemented in the ABCluster global optimization program, were taken from previous predictions reported in the literature, or were created from our prior knowledge of likely candidate atomic cluster structures [37,38,39,40]. This ensured that both logical structures would be considered and that other candidate isomers would be located without using prior knowledge or bias. Low-energy isomers generated from the global optimization procedure, along with the additional structural isomers created for each size, were reoptimized without symmetry constraints using stringent convergence criteria via the B3PW91 density functional theory method and the 6-311++G(d,p) basis set for all atoms [41,42,43,44]. The Gaussian 16 quantum chemical package was used for all calculations [45]. This method was chosen to allow for comparison with the analogous MgScHn system studied previously at this level of theory [37]. Additionally, this theoretical approach has been shown to accurately reproduce various experimental paraments and produce the same major conclusions as higher-level ab initio methods for similar systems, and has performed better than other combinations of DFT methods and basis sets for these test cases [37]. Vibrational frequencies were calculated for each isomer to ensure that true minima structures were located. Zero-point energy corrections were included for all relative energy comparisons. All global minima isomers reported adapted the lowest possible spin multiplicity for the particular system (i.e., either singlet or doublet states depending on the number of electrons involved for the respective size). Natural Bond Orbital (NBO) analyses were performed using the NBO 7.0 program to gain further insight into the electronic and bonding properties of each cluster [46]. All calculations were performed using the Expanse high-performance computing cluster housed at the San Diego Supercomputing Center (SDSC) through the NSF-ACCESS program. Visualization of the results and pictures of each structure and the frontier molecular orbitals were generated locally using the GaussView 6 program [47]. By exploring small MgTiHn clusters in detail here, the results will provide insight and further motivation for utilizing titanium-doped magnesium hydride solids as hydrogen storage materials.

3. Results and Discussion

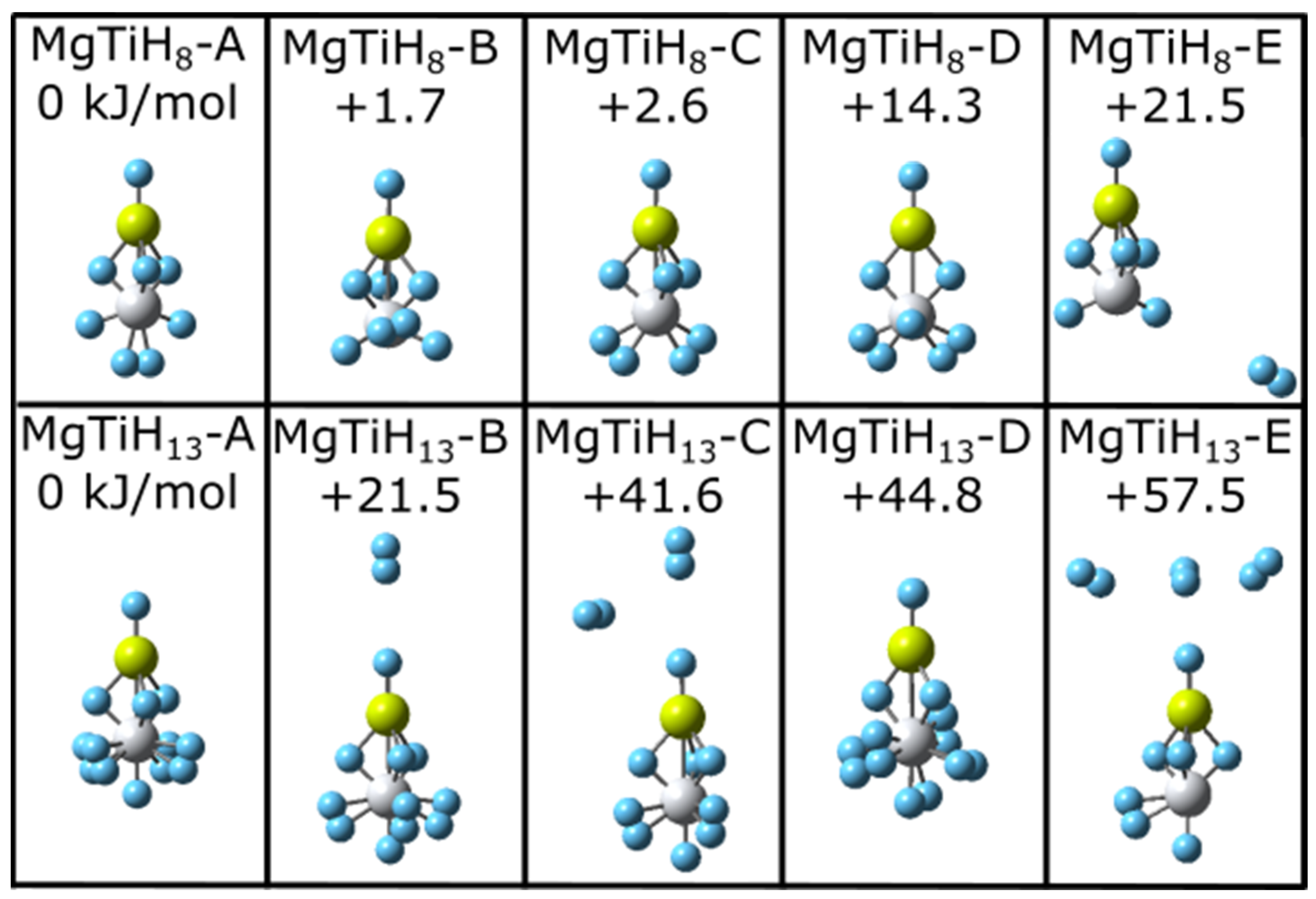
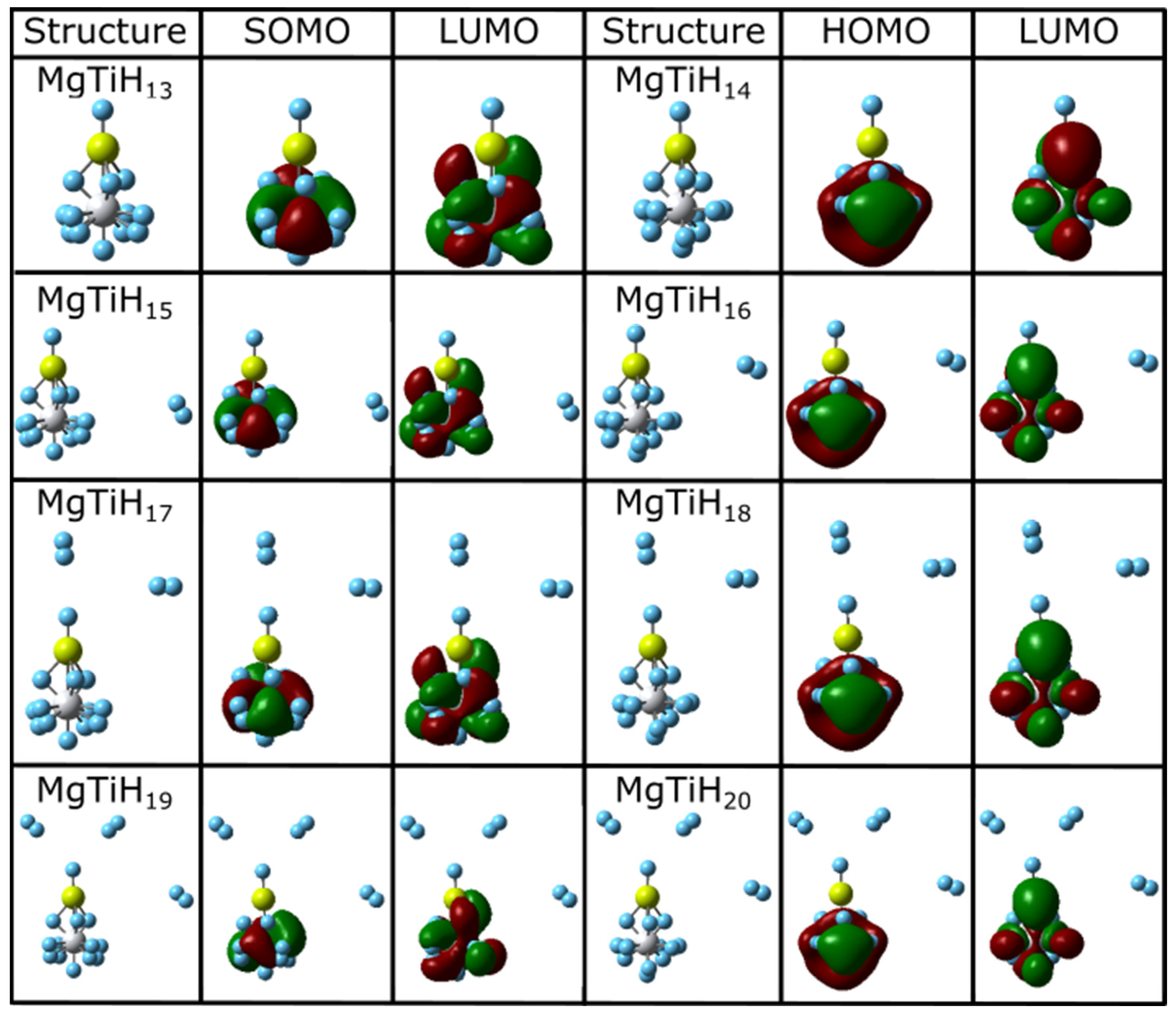
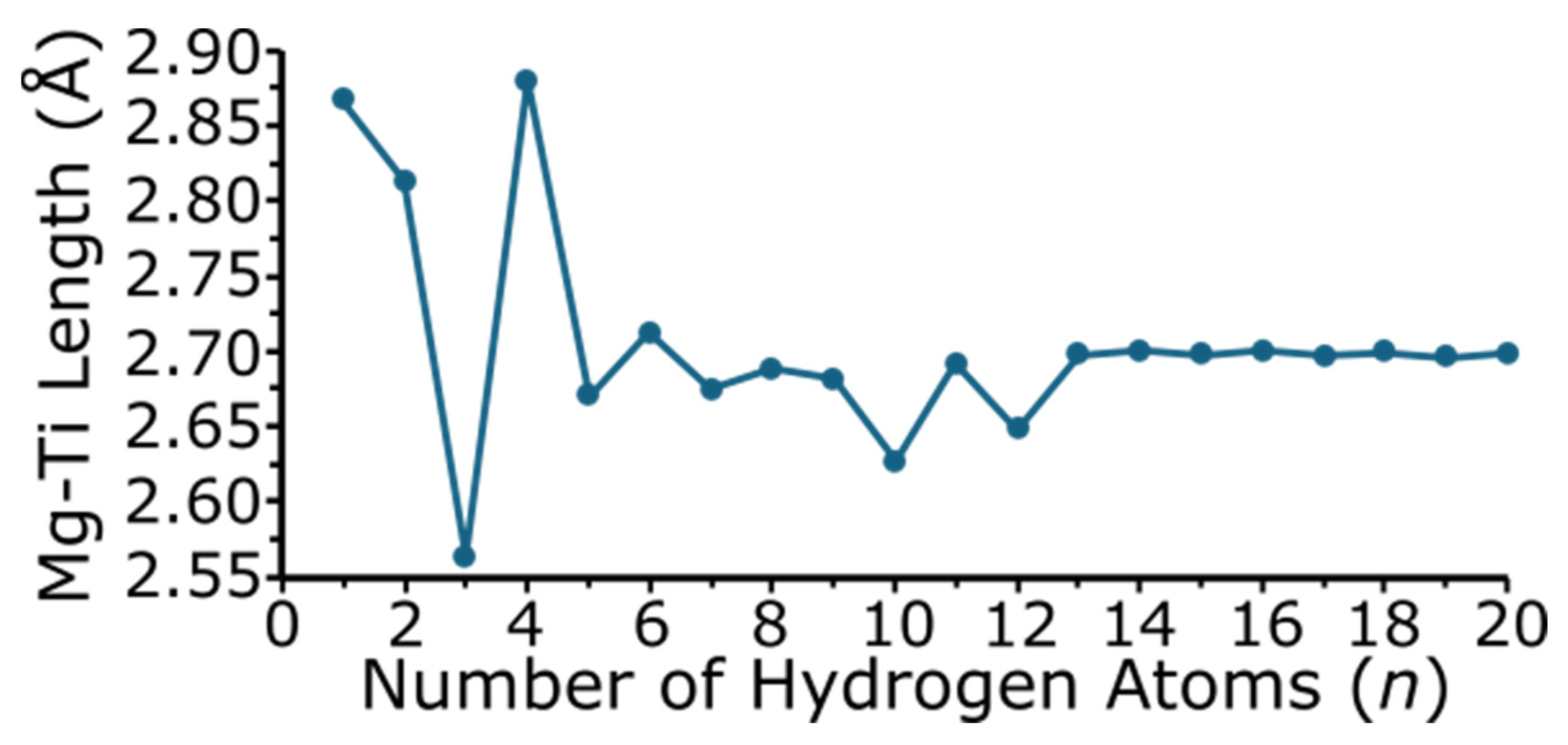
3.1. Geometric Structure Determination, Growth, and Electronic Properties

For each MgTiHn size (n = 1–20), the energies of multiple isomers were compared to determine the ground state structure. As two representative examples, five low-energy local minima structures located for MgTiH8 and MgTiH13 are shown in Figure 1. The energy of each isomer in Figure 1 is reported relative to the lowest-energy isomer for that size. The lowest-energy isomers MgTiH8-A and MgTiH13-A lie over 21 kJ/mol lower in energy than structures with a single dissociated H2 molecule (i.e., isomers MgTiH8-E and MgTiH13-B), and dissociation of additional H2 molecules leads to even higher energy geometries for these sizes (i.e., MgTiH13-C and MgTiH13-E are 41.6 and 57.5 kJ/mol higher in energy than MgTiH13-A, respectively). Note that the global minima isomers MgTiH8-A and MgTiH13-A contain an H-Mg(μ-H)3Ti subunit to their structure. Isomers without this (μ-H)3 group (i.e., MgTiH8-D and MgTiH13-D) lie higher in energy, and more is discussed about this particular group while discussing the growth pattern in the next paragraphs. Note also that MgTiH8-A is a different lowest-energy structural isomer than what was predicted for this size when Sc was used as a transition metal (i.e., isomer MgTiH8-C is analogous to the global minimum MgScH8 isomer reported previously [37]), and, interestingly, structural changes based on transition metal dopant identity can already be noted. A more detailed discussion of the relationship between MgTiHn and MgScHn clusters is presented later in Section 3.2. Calculations were also performed at the B3PW91/lanl2dz level, and they gave similar results. At this B3PW91/lanl2dz level of theory, MgTiH8-A was also predicted to be the lowest-energy MgTiH8 structure, lying 6.6 kJ/mol lower in energy than MgTiH8-B. Interestingly, at this level, MgTiH8-E with a dissociated H2 unit lay only 7.8 kJ/mol higher in energy than the global minima MgTiH8-A structure.
The ground state structures and frontier molecular orbitals of all of the lowest-energy MgTiHn isomers located for n = 1–12 and n = 13–20 are shown in Figure 2 and Figure 3, respectively. The coordinates of all of the located global minima isomers in standard xyz format are provided in the Supporting Information for this article. For small-sized MgTiH1–MgTiH4, hydrogen atoms either bind to the Ti center or act as bridging hydrogen atoms between titanium and magnesium. When an odd number of hydrogen atoms is present for these small sizes (i.e., for the MgTiH and MgTiH3 doublet species), hydrogen atoms bind exclusively as bridge-bound hydrogens. However, when an even number of hydrogen atoms are present (i.e., for the MgTiH2 and MgTiH4 singlet species), two hydrogen atoms bind to the titanium center. For MgTiH5, a single hydrogen atom binds solely to Mg, and three bridge-bonded hydrogen atoms exist. Once this H-Mg(μ-H)3Ti entity is formed in MgTiH5, the group also exists as a structural motif in the ground state geometry for all larger-sized clusters in the size range explored here. For MgTiH6–MgTiH14, hydrogen atoms continue to bind to the titanium center either as H atoms or as molecular H2. For MgTiH15 and larger cluster sizes, weakly interacting H2 molecule(s) are dissociated from the MgTiH13 or MgTiH14 cluster core, depending on whether an odd or even number of hydrogen atoms is present, respectively. This MgTiH14 size thus sets the hydrogen saturation limit on these clusters, indicating a maximum hydrogen capacity of 16.4% by mass in the MgTiHn system.
Once H2 dissociation occurs for MgTiHn (n ≥ 15), the weakly bound H2 molecule appears at different locations in the ground state structure. After the first H2 molecule in MgTiH15 and MgTiH16 appears along the cluster’s side, additional H2 molecules in MgTiHn (n = 17–20) aggregate oriented along the Ti-Mg-H backbone of the cluster. A similar observation was noted in MgScHn clusters [37]. This provides further evidence that dihydrogen may prefer to dissociate through coordinating with the metal-Mg-H backbone, particularly after the first H2 molecule dissociates. It seems likely the transition metal may aid in the release of dihydrogen from magnesium hydrides solids as the transition metal diffuses into the solid. By doing so, this may lead to a hydride with a large negative partial charge that dissociated dihydrogen can coordinate to with a temporary dipole. For example, in the ground state structure of MgTiH17, NBO charges indicate the partial charges for each atom along the Ti-Mg-H backbone to be −0.399, +1.482, and −0.654, respectively. The dissociated dihydrogen aligned with this backbone has a temporary dipole with partial charges on the two hydrogen atoms of +0.014 and −0.015. Hence, the dissociated H2 molecule is able to weakly interact with the large, negatively charged hydride (−0.654) in the cluster, which may aid in stabilizing the H2 as it dissociates from metal-doped magnesium hydride materials.
The highest occupied molecular orbital (HOMO) and lowest unoccupied molecular orbital (LUMO), which jointly make up the frontier orbitals for these clusters, are also shown in Figure 2 and Figure 3. These orbitals primarily consist of magnesium p and titanium d character. This is easily seen for smaller-sized clusters, and the frontier orbitals become increasingly complicated as the cluster size increases. For example, in the MgTiH2 cluster, the p and d orbitals of Mg and Ti, respectively, overlap constructively in these frontier orbitals. For doublet species with an odd number of electrons, the singly occupied molecule orbital (SOMO) is denoted for the HOMO, as is common, and is more descriptive for the system. Figure 2 and Figure 3 show clusters with an odd number of hydrogen (doublet systems) on the left side and clusters with an even number of hydrogen atoms (singlet systems) on the right. Thus, similarities can be noted down a column, and the HOMO and SOMO are labeled accordingly for a given column. Note, for example, that once a cluster is saturated with hydrogen atoms (i.e., for MgTiH13 and MgTiH14), the addition of dissociated H2 molecules for larger MgTiHn (n ≥ 15) clusters does not change the frontier orbitals significantly.
Table 1 presents the natural electron configurations (NECs) for magnesium and titanium atoms in MgTiHn (n = 1–20) ground state clusters. It also includes the Mullikan and natural partial charges for both metal atoms in each structure. The NECs show that titanium predominantly loses s electron density and gains d character in the cluster relative to the atomic electron configuration, whereas magnesium predominantly loses s electron density. In general, Mullikan and natural partial charges have the same sign for each metal in the cluster, but natural charges are typically predicted to be larger in magnitude for both metals. This is a similar trend to what was observed for MgScHn clusters [37]. While the partial charge of Mg is predicted to be positively charged for every ground state structure, titanium is positively charged for small-sized clusters and becomes partially negatively charged in MgTiH12 and larger sizes. At n = 5 and larger, the natural charge on magnesium remains relatively constant up to n = 20.

3.2. MgTiHn and MgScHn Comparison

In comparing the ground state structures of MgTiHn clusters assigned in the previous section and MgScHn reported previously [37], several interesting observations can be noted. First, the lowest-energy isomer of the small MgTiH8 cluster contains a TiH2(H2) group (i.e., two hydrogen atoms and an H2 molecule bound to Ti). This differs from the ground state of MgScH8, where a Sc(H2)2 group exists. The reported HMg(μ-H)3TiH2(H2) ground state structure of MgTiH8 lies 2.6 kJ/mol lower in energy than a possible HMg(μ-H)3Ti(H2)2 structure (which is the lowest-energy Sc isomer) at this level of theory. This preference for two hydrogen atoms and a hydrogen molecule bound to the metal, rather than two diatomic hydrogen molecules, likely arises from the presence of an additional electron on the Ti center compared to Sc. This difference is also important, as it impacts various parameters of the clusters. For example, the presence of terminal hydrogen atoms, as opposed to hydrogen molecules, bound to the metal has an impact on the metal–magnesium bond length. This is discussed in more detail in Section 3.3. Secondly, all hydrogen atoms remain bound to the lowest-energy MgTiH14 cluster, and MgTiH15 is the first size cluster with a dissociated H2 molecule. Hence, MgTiH14 achieves hydrogen saturation, and the maximum hydrogen capacity of this system is found to be 16.4% by mass. This is larger and differs from the analogous scandium system where MgScH13, which is 15.9% hydrogen by mass, is the largest cluster without a dissociated H2 molecule. This hydrogen dissociation at different-sized clusters for two transition metals that differ in their number of valence electrons but have similar sizes implies, at least in part, that dissociation likely occurs due to the electronic properties of the transition metal dopant rather than steric factors.

3.3. Cluster Stability and Energetics

In the discussion on cluster energetics here, we focus mainly on trends that are predicted rather than absolute values at this level of theory employed. Figure 4 shows the absolute value of the HOMO-LUMO energy gap as a function of the number of hydrogen atoms in a cluster. For doublet electronic species (i.e., species with an odd number of hydrogen atoms), the singly occupied molecular orbital (SOMO) is taken as the HOMO. This energy gap, while it should only be taken as qualitative at this level of theory, provides a relative indication of the inertness or reactivity of a specific cluster, with a higher energy gap indicating a more inert cluster. In Figure 4, it is seen that the HOMO-LUMO gap generally increases with size up to MgTiH13; is the largest for MgTiHn (n ≥ 13), where the clusters are predicted to be the most inert in this cluster size range; and remains fairly constant for larger sizes. This is appropriate, as H2 molecules begin to dissociate from the saturated clusters at this size and the general cluster structure remains relatively constant with each additional dissociated hydrogen molecule. Note that this maximum gap energy is only slightly less than the maximum calculated for MgScHn clusters [37].
Figure 5 shows the magnesium–titanium bond length as a function of the number of hydrogen atoms in the cluster. The magnesium–titanium bond length changes for the smaller MgTiHn (n = 1–5) size range, but varies to a lesser degree when n is greater than 5. For MgTiH13 and larger sizes, once hydrogen saturation occurs in the MgTiHn cluster, the Mg-Ti distance remains relatively constant. Note that MgTiH, MgTiH2, and MgTiH4, which have the longest predicted Mg-Ti bond lengths of 2.867, 2.813, and 2.880 Å, respectively, correspond to the three sized clusters where the ground state structure does not possess a full three-hydrogen-atom bridge-bound Mg(μ-H)3Ti unit. MgTiH3, which has the shortest Mg-Ti bond length of 2.564 Å, contains a full Mg(μ-H)3Ti group without any additional terminal hydrogen atoms in the cluster, and these bridging hydrogen atoms appear to lead to structures with shorter Mg-Ti distances. For MgTiH10 and MgTiH12, there is also a decreased Mg-Ti bond length compared to other clusters in this size range, although to a lesser extent than that seen for smaller sized clusters. Similarly to MgTiH3, MgTiH10 and MgTiH12 do not contain any terminal Ti-H bonds (i.e., all of the hydrogen atoms bound to Ti are either as H2 units or bridge bound Mg(μ-H)3Ti). Hence, the decreased Mg-Ti length appears to be due to the cluster containing the three bridge-bound hydrogen atoms, without any additional terminal H atoms bound to the titanium center.
Figure 6 shows the binding energy (Ebind) of the MgTiHn clusters. The binding energy, or fragmentation energy, is calculated by Equation (1) and is the sum of the energy of each individual atom making up the MgTiHn cluster minus the energy of the cluster, and is normalized for the total number of atoms in the cluster by dividing by n + 2.
E b i n d n = E M g + E T i + n E H − E M g T i H n n + 2    
Hence, a more positive Ebind value denotes a more stable cluster, and as all of the Ebind values are positive, this indicates that the clusters studied here are more stable than the individual atoms making up the cluster. The numerical values of Ebind are given in the Supporting Information. As expected, the Ebind of the cluster increases drastically as n increases for small-sized clusters, and then begins to level off and change more gradually for larger-sized clusters. The odd/even oscillation seen in Figure 6 indicates that clusters with an odd number of hydrogen atoms are, in general, more stable for this system. A larger blown-up section for n = 5–20 is shown as an insert in Figure 6. In this region, note that the energy increases up to n = 13, and then begins to decrease. Hence, MgTiH13 appears to be a more stable cluster size with regard to Ebind. For clusters with an even number of hydrogen atoms, the Ebind increases up to MgTiH10 and then begins to decrease with cluster size.
The relative stability of each cluster compares the energy of a cluster in relationship to its neighboring-sized clusters with one more and one less hydrogen atom (or two more and two less hydrogen atoms). These Estab1 and Estab2 relative energies are calculated using Equations (2) and (3), respectively.
E s t a b 1   n = 2 E M g T i H n − E M g T i H n − 1 − E M g T i H n + 1  
E s t a b 2   n = 2 E M g T i H n − E M g T i H n − 2 − E M g T i H n + 2  
Figure 7 depicts Estab1 and Estab2 as functions of cluster size and each are additional metrics to indicate the relative stability of each cluster. As these are calculated with Equations (2) and (3), a more negative stabilization energy indicates an enhanced stability relative to its neighboring-sized clusters. The numerical values of Estab1 and Estab2 are also provided in Table S1 of the Supporting Information. With Estab1, an odd/even oscillation can be seen, which again indicates that clusters with an odd number of hydrogen atoms are more stable than their even-numbered neighbors. For clusters with an odd number of hydrogen atoms, we again note that MgTiH13 is more stable (i.e., has a more a negative Estab1) than other clusters similar in size. For even-numbered hydrogens, we note that MgTiH10 is a minimum, and Estab1 indicates that this size is more stable than other MgTiHn clusters with an even number of hydrogen atoms. While Estab2 compares clusters with a similar odd or even number of hydrogen atoms, it also compares clusters that vary more in size, differing by two hydrogen atoms. However, Estab2 again indicates that, in addition to small MgTiHn clusters, MgTiH10 and MgTiH13 are more stable larger species than other clusters in this size range. As expected, Estab2 does not vary significantly once hydrogen saturation and dissociation occur for the largest clusters studies here.

4. Conclusions

In summary, we have explored the structures, growth mechanism, stability, and energetics of MgTiHn (n ≤ 20) clusters at the B3PW91 hybrid density functional level of theory with an all-electron 6-311++G(d,p) basis set for all atoms. We have shown that hydrogen atoms predominantly bind to titanium in small MgTiHn clusters. Additionally, hydrogen saturation occurs at MgTiH14 with a large 16.4% hydrogen by mass, and clusters with an odd number of hydrogen atoms are more stable than those with an even number of hydrogen atoms. Particularly, MgTiH10 and MgTiH13 are more stable clusters with even and odd numbers of hydrogen atoms, respectively. The cluster frontier orbitals are primarily made up of magnesium p and titanium d atomic orbitals. A plausible mechanism for H2 dissociation from metal-doped magnesium hydride solids, which is supported by NBO analysis in MgTiHn clusters, is described where H2 molecules desorb while weakly interacting with an anionic hydride in the solid. In the desorption process, the anionic hydride forms a weak intermolecular interaction with a temporary dipole of the dissociating H2 molecule.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/hydrogen5040035/s1. The supplementary data for this article include the determined Cartesian coordinates for the lowest-energy MgTiHn (n = 1–20) isomers calculated at the B3PW91/6-311++G(d,p) level of theory in standard xyz format. Additionally, the numerical values of Ebind, Estab1, and Estab2 shown in Figure 6 and Figure 7 are provided in the Supplementary Materials as Table S1 (Table S1: The Ebind, Estab1, and Estab2 energy calculated for MgTiHn (n = 1-20) at the B3PW91/6-311++G(d,p) level of theory by equation 1, 2, and 3, respectively.).

Author Contributions

Conceptualization, J.T.L.; methodology, C.N., D.B., and J.T.L.; validation, C.N., D.B., and J.T.L.; formal analysis, C.N. and J.T.L.; investigation, C.N. and J.T.L.; resources, J.T.L.; data curation, C.N. and J.T.L.; writing—original draft preparation, C.N. and J.T.L.; writing—review and editing, J.T.L.; visualization, C.N. and J.T.L.; supervision, D.B. and J.T.L.; project administration, C.N., D.B., and J.T.L.; funding acquisition, J.T.L. All authors have read and agreed to the published version of the manuscript.

Funding

This study utilized the Advanced Cyberinfrastructure Coordination Ecosystem: Services and Support (ACCESS) supported by the National Science Foundation awards #2138259, 2138286, 2138307, 2137603, and 2138296, with additional support through the Match Plus program. Calculations were performed on the Expanse high-performance computing cluster at the San Diego Supercomputing Center (SDSC) through allocation grant #CHE130094.

Data Availability Statement

The Cartesian coordinates of the optimized global minimum structures for each size are available in standard xyz format in the Supplementary Materials.

Acknowledgments

We acknowledge Murray State University, the Jones College of Science, Engineering, and Technology, and the Department of Chemistry for support of this research. We thank Alana Romanella (University of Colorado Boulder) for helpful discussions during this research project.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Solomon, C.G.; Salas, R.N.; Malina, D.; Sacks, C.A.; Hardin, C.C.; Prewitt, E.; Lee, T.H.; Rubin, E.J. Fossil-Fuel Pollution and Climate Change—A New Nejm Group Series. N. Engl. J. Med. 2022, 386, 2328–2329. [Google Scholar] [CrossRef] [PubMed]
  2. Koch, S.; Klitzman, R. Reliance on Fossil Fuels: Ethical Implications for Intensivists. Intensive Care Med. 2023, 49, 330–333. [Google Scholar] [CrossRef] [PubMed]
  3. Zhang, Y.; Jia, Z.; Yuan, Z.; Yang, T.; Qi, Y.; Zhao, D. Development and Application of Hydrogen Storage. J. Iron Steel Res. Int. 2015, 22, 757–770. [Google Scholar] [CrossRef]
  4. Yartys, V.A.; Lototskyy, M.V.; Akiba, E.; Albert, R.; Antonov, V.E.; Ares, J.R.; Baricco, M.; Bourgeois, N.; Buckley, C.E.; Bellosta von Colbe, J.M.; et al. Magnesium based materials for hydrogen based storage: Past, present, and future. Int. J. Hydrogen Energy 2019, 44, 7809–7859. [Google Scholar] [CrossRef]
  5. von Colbe, J.B.; Ares, J.-R.; Barale, J.; Baricco, M.; Buckley, C.; Capurso, G.; Galladat, N.; Grant, D.M.; Guzik, M.N.; Jacob, I.; et al. Application of hydrides in hydrogen storage and compression: Achievements, outlook and perspectives. Int. J. Hydrogen Energy 2019, 44, 7780–7808. [Google Scholar] [CrossRef]
  6. Zhou, W.; Jin, S.; Dai, W.; Lyon, J.T.; Lu, C. Theoretical study on the structural evolution and hydrogen storage in NbHn (n = 2–15) clusters. Int. J. Hydrogen Energy 2021, 46, 17246–17252. [Google Scholar] [CrossRef]
  7. Liao, Y.H.; Zhou, W.L.; Lyon, J.T.; Peng, F.; Lu, C. The structure of anionic NbHn− (n = 2–15) clusters and their maximum hydrogen capacity. New J. Phys. 2022, 24, 043038. [Google Scholar] [CrossRef]
  8. Schneemann, A.; White, J.L.; Kang, S.; Jeong, S.; Wan, L.F.; Cho, E.S.; Heo, T.W.; Prendergast, D.; Urban, J.J.; Wood, B.C.; et al. Nanostructure Metal Hydrides for Hydrogen Storage. Chem. Rev. 2018, 118, 10775–10839. [Google Scholar] [CrossRef]
  9. Dragassi, M.-C.; Royon, L.; Redolfi, M.; Ammar, S. Hydrogen Storage as a Key Energy Vector for Car Transportation: A Tutorial Review. Hydrogen 2023, 4, 831–861. [Google Scholar] [CrossRef]
  10. Lobo, R.F.M. A Brief on Nano-Based Hydrogen Energy Transition. Hydrogen 2023, 4, 679–693. [Google Scholar] [CrossRef]
  11. Fiorio, J.L.; Goethe, M.L.; Kohlrausch, E.C.; Zardo, M.L.; Tanaka, A.A.; de Lima, R.B.; da Silva, A.G.M.; Garcia, M.A.S.; Vidinha, P.; Machado, G. Nanoengineering of Catalysts for Enhanced Hydrogen Production. Hydrogen 2022, 3, 218–254. [Google Scholar] [CrossRef]
  12. Pistidda, C. Solid-State Hydrogen Storage for a Decarbonized Society. Hydrogen 2021, 2, 428–443. [Google Scholar] [CrossRef]
  13. Cao, Z.; Habermann, F.; Burkmann, K.; Felderhoff, M.; Mertens, F. Unstable Metal Hydrides for Possible On-Board Hydrogen Storage. Hydrogen 2024, 5, 241–279. [Google Scholar] [CrossRef]
  14. Vajeeston, P.; Ravindran, P.; Fjellvåg, H. Predicting New Materials for Hydrogen Storage Application. Materials 2009, 2, 2296–2318. [Google Scholar] [CrossRef]
  15. Zhang, J.; Li, J.; Wu, Y.; Guo, X.; Ye, J.; Yuan, B.; Wang, S.; Jiang, L. Recent advances on the thermal destabilization of Mg-based hydrogen storage materials. RSC Adv. 2019, 9, 408–428. [Google Scholar] [CrossRef] [PubMed]
  16. Hevia, E.; Mulvey, R.E. A Record-Breaking Magnesium Hydride Molecular Cluster: Implications for Hydrogen Storage. Angew. Chem. Int. Ed. 2011, 50, 9242–9243. [Google Scholar] [CrossRef]
  17. Seifert, F.; Görls, H.; Kupfer, S.; Kretschmer, R. A tetranuclear magnesium hydride cluster with a four-coordinate hydride in near square-planar geometry. Chem. Commun. 2023, 59, 7627–7630. [Google Scholar] [CrossRef]
  18. Wang, Y.; Wang, Y. Recent Advances in Additive-Enhanced Magnesium Hydride for Hydrogen Storage. Prog. Nat. Sci. Mater. Int. 2017, 27, 41–49. [Google Scholar] [CrossRef]
  19. Zhang, J.; Sun, L.Q.; Zhou, Y.C.; Peng, P. Dehydrogenation Thermodynamics of Magnesium Hydride Doped with Transition Metals: Experimental and Theoretical Studies. Comput. Mater. Sci. 2015, 98, 211–219. [Google Scholar] [CrossRef]
  20. El Khatabi, M.; Bhihi, M.; Naji, S.; Labrim, H.; Benyoussef, A.; El Kenz, A.; Loulidi, M. Study of Doping Effects with 3d and 4d-Transition Metals on the Hydrogen Storage Properties of MgH2. Int. J. Hydrogen Energy 2016, 41, 4712–4718. [Google Scholar] [CrossRef]
  21. Zhou, C.; Zhang, J.; Bowman, R.C.; Fang, Z.Z. Roles of Ti-Based Catalysts on Magnesium Hydride and Its Hydrogen Storage Properties. Inorganics 2021, 9, 36. [Google Scholar] [CrossRef]
  22. Larsson, P.; Araúo, C.M.; Larsson, J.A.; Jena, P.; Ahuja, R. Role of catalysts in dehydrogenation of MgH2 nanoclusters. Proc. Natl. Acad. Sci. USA 2008, 105, 8227–8231. [Google Scholar] [CrossRef] [PubMed]
  23. Bazzanella, N.; Checchetto, R.; Miotello, A. Atoms and Nanoparticles of Transition Metals as Catalysts for Hydrogen Desorption from Magnesium Hydride. J. Nanomater. 2011, 2011, 865969. [Google Scholar] [CrossRef]
  24. Li, Q.; Yan, M.; Xu, Y.; Zhang, X.L.; Lau, K.T.; Sun, C.; Jia, B. Computational Investigation of MgH2/NbOx for Hydrogen Storage. J. Phys. Chem. C 2021, 125, 8862–8868. [Google Scholar] [CrossRef]
  25. Sun, Z.; Lu, X.; Nyahuma, F.M.; Yan, N.; Xiao, J.; Su, S.; Zhang, L. Enhancing Hydrogen Storage Properties of MgH2 by Transition Metals and Carbon Materials: A Brief Review. Front. Chem. 2020, 8, 552. [Google Scholar] [CrossRef] [PubMed]
  26. Charkin, O.P.; Maltsev, A.P. Density Functional Theory Modeling of Reactions of Addition of H2 Molecules to Magnesium Clusters Mg17M Doped with Atoms M of Transition 3d Elements. J. Phys. Chem. A 2021, 125, 2308–2315. [Google Scholar] [CrossRef]
  27. Jangir, M.; Jain, I.P.; Gattia, D.M. Effect of Ti-Based Additives on the Hydrogen Storage Properties of MgH2: A Review. Hydrogen 2023, 4, 523–541. [Google Scholar] [CrossRef]
  28. Yuan, Q.; Peng, C.; Yang, C.; Li, Y.; Zhang, Q.; Lv, Y.; Liu, G.; Liu, D. Facilitated hydrogen storage properties of MgH2 by Ni nanoparticles anchored on Mo2C@C nanosheets. Int. J. Hydrogen Energy 2024, 85, 12–19. [Google Scholar] [CrossRef]
  29. Khandelwal, P.; Rathi, B.; Agarwal, S.; Juyal, S.; Gill, F.S.; Kumar, M.; Saini, P.; Dixit, A.; Ichikawa, T.; Jain, A. Core-shell structured Ni@C based additive for magnesium hydride system towards efficient hydrogen sorption kinetics. Int. J. Hydrogen Energy, 2024; in press. [Google Scholar] [CrossRef]
  30. Er, S.; van Setten, M.J.; de Wijs, G.A.; Brocks, G. First-principles modelling of magnesium titanium hydrides. J. Phys. Condens. Matter 2010, 22, 074208. [Google Scholar] [CrossRef]
  31. Kyoi, D.; Sato, T.; Rönnebro, E.; Kitamura, N.; Ueda, A.; Ito, M.; Katsuyama, S.; Hara, S.; Noréus, D.; Sakai, T. A new ternary magnesium-titanium hydride Mg7TiHx with hydrogen desorption properties better than both binary magnesium and titanium hydrides. J. Alloys Compd. 2004, 372, 213–217. [Google Scholar] [CrossRef]
  32. Luo, X.; Grant, D.M.; Walker, G.S. Hydrogen storage properties of nano-structured 0.65MgH2/0.35ScH2. Int. J. Hydrogen Energy 2013, 38, 153–161. [Google Scholar] [CrossRef]
  33. Garçon, M.; Bakewell, C.; Sackman, G.A.; White, A.J.P.; Cooper, R.I.; Edwards, A.J.; Crimmin, M.R. A hexagonal planar transition-metal complex. Nature 2019, 574, 390–393. [Google Scholar] [CrossRef] [PubMed]
  34. Dore, E.M.; Lyon, J.T. The Structures of Silicon Clusters Doped with Two Gold Atoms, SinAu2 (n = 1–10). J. Clust. Sci. 2016, 27, 1365–1381. [Google Scholar] [CrossRef]
  35. Lyon, J.T. Building Blocks: Investigating the Structures, Properties, and Reactivity of Strongly Bound Atomic Clusters at a PUI. In Physical Chemistry Research at Undergraduate Institutions; Hopkins, T., Parish, C.A., Eds.; ACS Books: Washington, DC, USA, 2022; Volume 2, Chapter 10; pp. 165–179. [Google Scholar] [CrossRef]
  36. Lyon, J.T.; Gruene, P.; Fielicke, A.; Meijer, G.; Rayner, D.M. Probing C-O bond activation on gas-phase transition metal clusters: Infrared multiple photon dissociation spectroscopy of Fe, Ru, Re, and W cluster CO complexes. J. Chem. Phys. 2009, 131, 184706. [Google Scholar] [CrossRef]
  37. Lyon, J.T. Hydrogen Binding and Dissociation in MgScHn Clusters (n ≤ 20). Int. J. Hydrogen Energy 2021, 46, 36872–36877. [Google Scholar] [CrossRef]
  38. Zhang, J.; Dolg, M. ABCLUSTER: The Artificial Bee Colony Algorithm for Cluster Global Optimization. Phys. Chem. Chem. Phys. 2015, 17, 24173–24181. [Google Scholar] [CrossRef]
  39. Zhang, J.; Dolg, M. Global Optimization of Clusters of Rigid Molecules Using the Artificial Bee Colony Algorithm. Phys. Chem. Chem. Phys. 2016, 18, 3003–3010. [Google Scholar] [CrossRef]
  40. Chen, H.; Liang, H.; Dai, W.; Lu, C.; Ding, K.; Bi, J.; Zhu, B. MgScH15: A highly stable cluster for hydrogen storage. Int. J. Hydrogen Energy 2020, 45, 32260–32268. [Google Scholar] [CrossRef]
  41. Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
  42. Perdew, J.P.; Burke, K.; Wang, Y. Generalized gradient approximation for the exchange-correlation hole of a many-electron system. Phys. Rev. B. 1996, 54, 16533–16539. [Google Scholar] [CrossRef] [PubMed]
  43. McLean, A.D.; Chandler, G.S. Contracted Gaussian-basis sets for molecular calculations. 1. 2nd row atoms, Z = 11–18. J. Chem. Phys. 1980, 72, 5639–5648. [Google Scholar] [CrossRef]
  44. Raghavachari, K.; Trucks, G.W. Highly correlated systems: Excitation energies of first row transition metals Sc-Cu. J. Chem. Phys. 1989, 91, 1062–1065. [Google Scholar] [CrossRef]
  45. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision C.01; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
  46. Glendening, E.D.; Badenhoop, J.K.; Reed, A.E.; Carpenter, J.E.; Bohmann, J.A.; Morales, C.M.; Karafiloglou, P.; Landis, C.R.; Weinhold, F. NBO, 7.0; Theoretical Chemistry Institute, University of Wisconsin: Madison, WI, USA, 2018. [Google Scholar]
  47. Dennington, R.; Keith, T.A.; Millam, J.M. GaussView, Version 6.0.16; Semichem Inc.: Shawnee Mission, KS, USA, 2016. [Google Scholar]
Hydrogen 05 00035 g001
Figure 1. Low-energy structural isomers of MgTiH8 (top) and MgTiH13 (bottom). For each structure, magnesium atoms are colored yellow, titanium atoms are grey, and hydrogen atoms are blue. Relative energy values are listed in kJ/mol.
Figure 1. Low-energy structural isomers of MgTiH8 (top) and MgTiH13 (bottom). For each structure, magnesium atoms are colored yellow, titanium atoms are grey, and hydrogen atoms are blue. Relative energy values are listed in kJ/mol.
Hydrogen 05 00035 g001
Hydrogen 05 00035 g002
Figure 2. Lowest-energy isomers and frontier orbitals of MgTiHn (n = 1–12). For each structure, magnesium atoms are colored yellow, titanium atoms are grey, and hydrogen atoms are blue.
Figure 2. Lowest-energy isomers and frontier orbitals of MgTiHn (n = 1–12). For each structure, magnesium atoms are colored yellow, titanium atoms are grey, and hydrogen atoms are blue.
Hydrogen 05 00035 g002
Hydrogen 05 00035 g003
Figure 3. Lowest-energy isomers and frontier orbitals of MgTiHn (n = 13–20). For each structure, magnesium atoms are colored yellow, titanium atoms are grey, and hydrogen atoms are blue.
Figure 3. Lowest-energy isomers and frontier orbitals of MgTiHn (n = 13–20). For each structure, magnesium atoms are colored yellow, titanium atoms are grey, and hydrogen atoms are blue.
Hydrogen 05 00035 g003
Hydrogen 05 00035 g004
Figure 4. Frontier molecular orbital energy gap as a function of the number of hydrogen atoms in MgTiHn clusters.
Figure 4. Frontier molecular orbital energy gap as a function of the number of hydrogen atoms in MgTiHn clusters.
Hydrogen 05 00035 g004
Hydrogen 05 00035 g005
Figure 5. Bond length between magnesium and titanium as a function of the number of hydrogen atoms in each MgTiHn cluster.
Figure 5. Bond length between magnesium and titanium as a function of the number of hydrogen atoms in each MgTiHn cluster.
Hydrogen 05 00035 g005
Hydrogen 05 00035 g006
Figure 6. Binding (fragmentation) energy as a function of the number of hydrogen atoms in MgTiHn clusters (n = 1–20). The insert shows a blown-up portion of the overall plot for n = 5–20.
Figure 6. Binding (fragmentation) energy as a function of the number of hydrogen atoms in MgTiHn clusters (n = 1–20). The insert shows a blown-up portion of the overall plot for n = 5–20.
Hydrogen 05 00035 g006
Hydrogen 05 00035 g007
Figure 7. Relative energy between MgTiHn clusters as a function of the number of hydrogen atoms per cluster. Estab1 (blue circles) compares the energy of a cluster with that containing one more and less hydrogen atom, whereas Estab2 (green squares) compares the energy of a cluster with that containing two more and less hydrogen atoms. See text for additional details.
Figure 7. Relative energy between MgTiHn clusters as a function of the number of hydrogen atoms per cluster. Estab1 (blue circles) compares the energy of a cluster with that containing one more and less hydrogen atom, whereas Estab2 (green squares) compares the energy of a cluster with that containing two more and less hydrogen atoms. See text for additional details.
Hydrogen 05 00035 g007
Table 1. The natural electron configuration (NEC), Mullikan charges, and natural charges for the metal atoms in MgTiHn (n = 1–20) clusters.
Table 1. The natural electron configuration (NEC), Mullikan charges, and natural charges for the metal atoms in MgTiHn (n = 1–20) clusters.
H Atoms (n)NECMullikan Charge (Mg/Ti)Natural Charge (Mg/Ti)
1Mg:3s1.683p0.045s0.010.146/0.04690.265/0.303
Ti:4s1.033d2.594p0.064d0.01
2Mg:3s1.68 3p0.060.147/0.3360.253/0.711
Ti:4s0.533d2.614p0.134d0.01
3Mg:3s0.99 3p0.06 4s0.01 3d0.010.374/0.5320.921/0.774
Ti:4s0.383d2.804p0.035s0.014d0.01
4Mg:3s1.53 3p0.01 4s0.010.290/0.6170.449/0.961
Ti:4s0.643d2.324p0.074d0.01
5Mg:3s0.53 3d0.015p0.040.831/0.4561.422/1.179
Ti:4s0.373d2.394p0.034d0.02
6Mg:3s0.515p0.050.602/0.5131.437/0.911
Ti:4s0.453d2.584p0.044d0.02
7Mg:3s0.514p0.040.783/0.3241.437/0.861
Ti:4s0.373d2.724p0.024d0.03
8Mg:3s0.503p0.040.683/0.2441.454/0.484
Ti:4s0.413d3.054p0.024d0.03
9Mg:3s0.505p0.040.724/0.1441.451/0.490
Ti:4s0.363d3.094p0.024d0.04
10Mg:3s0.503p0.040.670/−0.0361.459/0.011
Ti:4s0.373d3.584p0.014d0.02
11Mg:3s0.496p0.040.689/−0.06991.467/0.039
Ti:4s0.363d3.534p0.024d0.05
12Mg:3s0.496p0.040.566/−0.04581.467/−0.379
Ti:4s0.373d3.974d0.025p0.02
13Mg:3s0.486p0.040.607/−0.2471.476/−0.399
Ti:3d3.954p0.025s0.384d0.05
14Mg:3s0.476p0.040.547/−0.5841.482/−1.013
Ti:4s0.363d4.624d0.015p0.01
15Mg:3s0.486p0.040.608/−0.2481.475/−0.400
Ti:3d3.954p0.025s0.384d0.05
16Mg:3s0.475p0.040.547/−0.5811.481/−1.013
Ti:4s0.363d4.624d0.015p0.01
17Mg:3s0.485p0.040.539/−0.1221.482/−0.399
Ti:4s0.383d3.954p0.024d0.05
18Mg:3s0.476p0.040.457/−0.4331.487/−1.012
Ti:4s0.363d4.624d0.015p0.01
19Mg:3s0.475p0.040.576/−0.1951.482/−0.399
Ti:4s0.383d3.954p0.024d0.05
20Mg:3s0.474p0.040.522/−0.5511.488/−1.012
Ti:3d4.625s0.364d0.015p0.01
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Newland, C.; Balamurugan, D.; Lyon, J.T. Structures and Properties of MgTiHn Clusters (n ≤ 20). Hydrogen 2024, 5, 669-681. https://doi.org/10.3390/hydrogen5040035

AMA Style

Newland C, Balamurugan D, Lyon JT. Structures and Properties of MgTiHn Clusters (n ≤ 20). Hydrogen. 2024; 5(4):669-681. https://doi.org/10.3390/hydrogen5040035

Chicago/Turabian Style

Newland, Camryn, D. Balamurugan, and Jonathan T. Lyon. 2024. "Structures and Properties of MgTiHn Clusters (n ≤ 20)" Hydrogen 5, no. 4: 669-681. https://doi.org/10.3390/hydrogen5040035

APA Style

Newland, C., Balamurugan, D., & Lyon, J. T. (2024). Structures and Properties of MgTiHn Clusters (n ≤ 20). Hydrogen, 5(4), 669-681. https://doi.org/10.3390/hydrogen5040035

Article Metrics

Back to TopTop