Domination Number in the Context of Some New Graphs ^†

Abstract

Let G = (V, E) be a graph, where D is a subset of V, such that the set of vertices in the set (V-D) is adjacent to at least one vertex in set D. Then, set D is known as the domination set. In other words, a dominating set in graph G is a set of vertices S, such that every vertex in the graph either belongs to S or is adjacent to at least one vertex in S. In this paper, we investigate the domination number for some different types of graphs like antiprism graph A_n, alternate pentagonal snake A(PS_n), cycle with one chord, and m-copies of cycle C_n with one chord. The domination number is frequently employed in computer science for tasks such as to optimize network design, algorithm design, security analysis, etc. Complex computational and network related challenges can be solved by domination number.

1. Introduction

In this study, we have considered finite and undirected graphs with no loop and multiple edges. A graph G(V, E) is composed of vertices (or nodes) and edges. The vertices are elements of non-empty set V, and the edges are unordered pairs of distinct vertices from V, forming set E. Each vertex has a degree, which represents the count of edges connected to that vertex, with loops (edges connecting a vertex to itself) counted twice. The open neighborhood of a vertex v, denoted as N(v), consists of all the vertices adjacent to v, i.e., connected to v by an edge. The closed neighborhood of v, denoted as N[v], includes both v and its open neighborhood. The domination number of a graph G is defined as the minimum cardinality (i.e., the smallest size) of a dominating set in G. It represents the minimum number of vertices needed to ensure that each vertex within the graph must either belong to the dominating set or be connected to a vertex within the dominating set. Domination in graphs is also discussed by Ambily et al. [1]. The notion of dominating sets within the field of graph theory can be traced back to the game of chess, where the objective is to strategically position chess pieces to dominate specific squares on the chessboard. The quest for calculating the domination numbers of graphs dates back to 1862 when De Jaenisch embarked on a quest to ascertain the smallest number of queens required on a chessboard. The goal was to ensure that every square was either occupied by a queen or within the reach of a queen’s single move. The concept of domination goes beyond the game of chess and has wide-ranging applications in different domains. In the field of chemistry, domination theory serves as a valuable tool for analyzing chemical structures. Moreover, domination theory finds practical uses in areas such as wireless communication networks, business networks, radio stations, and decision-making processes, as discussed by Gupta [2]. These practical applications highlight the importance and versatility of domination theory beyond its original association with chess, establishing it as a valuable concept for various fields of study and problem-solving. Jayachandran et al. [3] have discussed the domination number of graphs like fan F_m,2, diamond snake D_n, banana tree B(m, n), coconut tree CT (m, n) and firecracker F(m, n). Vaidya et al. [4] have given us the idea of the strong domination number of various graphs. Abdul et al. [5] have shown an Independent Domination Number by using 6-Alternative Snake graphs. Independent Domination Number by using a triangular and quadrilateral snake graph has been discussed by Meenakshi et al. [6]. Recently signed product cordial labeling has been studied on pan graphs by Sadawarte [7] and on some square cycle graphs by Sadawarte [8]. Bhuvaneswari et al. [9] have discussed domination integrity of snake and sunlet graphs. Wheel graphs and its locating edge domination number has been explained by Adawiyah et al. [10]. Similarly, we can extend this study by considering different types of graphs like antiprism graph A_n, alternate pentagonal snake A(PS_n), cycle with one chord and m-copies of cycle C_n with one chord. The concept of domination number has various applications in computer science. This concept is used in network design, wireless communication, social network analysis, security etc. Calculating the domination number is valuable for crafting effective network configurations, like for sensor networks and communication networks, where ensuring network connectivity or coverage with the fewest possible nodes to be managed or monitored is essential. In social network analysis, the dominating sets can uncover important individuals or nodes within a network, contributing to strategies like viral marketing and efforts to maximize influence.

2. Definitions

Definition 1. 

Within a graph G = (V, E), a set of vertices, denoted as D, is termed a dominating set if every vertex not included in D (belonging to V-D) is connected to at least one vertex within D. The graph’s domination number, denoted as γ(G), signifies the minimum size of a dominating set necessary to encompass all the vertices in G.

Definition 2. 

An antiprism graph A_n is a polyhedral and planar graph that represents the skeleton of an antiprism. The number of vertices and edges in an n-antiprism graph is 2n and 4n, respectively. It is isomorphic to the circulant graph.

Definition 3. 

The alternate pentagonal snake A(PS_n) is constructed by transforming a path p₁, p₂, …, p_k. This transformation involves adding two new vertices u_i and w_i between each pair of consecutive vertices p_i and p_(i+1). Additionally, a new vertex x_i is connected to the vertices u_i and w_i. In this way, every alternate edge of the original path is replaced by a cycle C₅.

Definition 4. 

A chord of a cycle C_n is an edge that connects two vertices of the cycle C_n, where the two vertices are not adjacent to each other.

Definition 5. 

Cycle with one chord can be defined as a cycle with a chord, such that there exist two vertices that are adjacent to each other, but not consecutive along the cycle.

Definition 6. 

The floor function, denoted as ⌊x⌋, is a mathematical function that returns the largest integer less than or equal to a given real number x. It is commonly referred to as the greatest integer function or integer value. If we have a real number x such that n ≤ x < n + 1, where n is an integer, then the floor of x ⌊x⌋ is equal to n.

Definition 7. 

The ceiling function, also known as the least integer function, is defined as the mathematical operation that finds the smallest integer greater than or equal to a given real number x. It is denoted by ⌈x⌉ or x. If we have a real number x such that n − 1 < x ≤ n, where n is an integer, then the ceiling of x ⌈x⌉ is equal to n.

3. Main Results

Theorem 1. 

For any antiprism graph, A_n n ≥ 5 domination number always exists.

Proof. 

Let G be a graph which is an antiprism graph A_n with 2n—vertices and 4n—edges. The following explanation delineates the minimal dominating set and its cardinality, referred to as the domination number, i.e., γ(G):

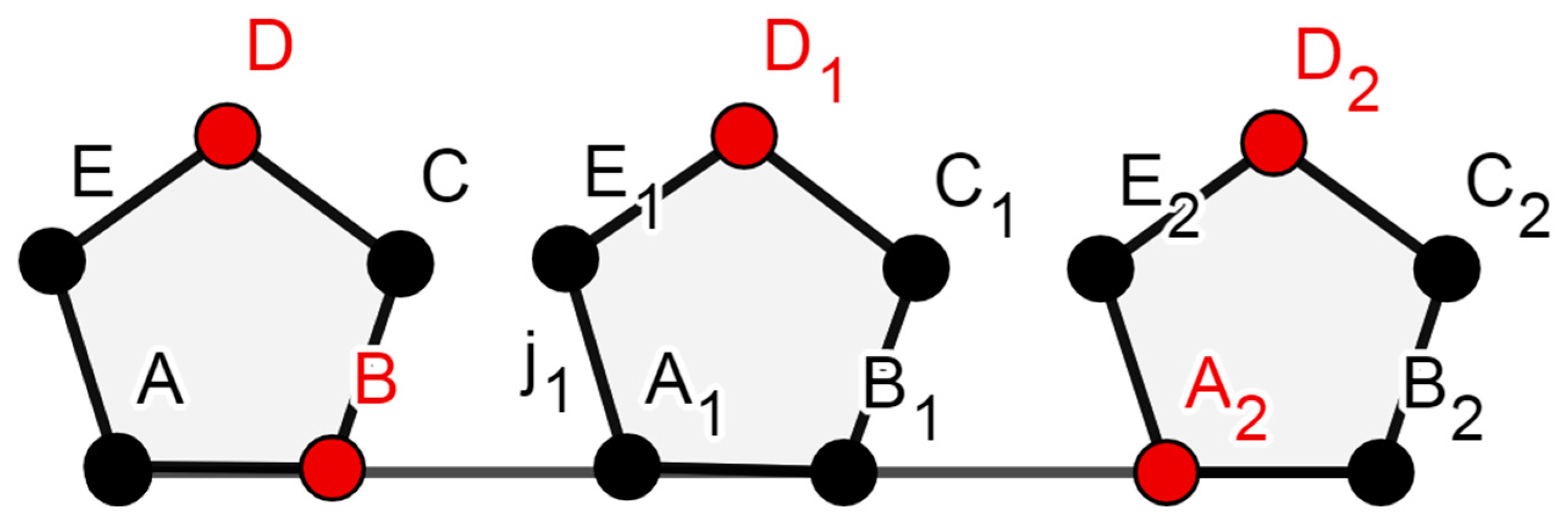
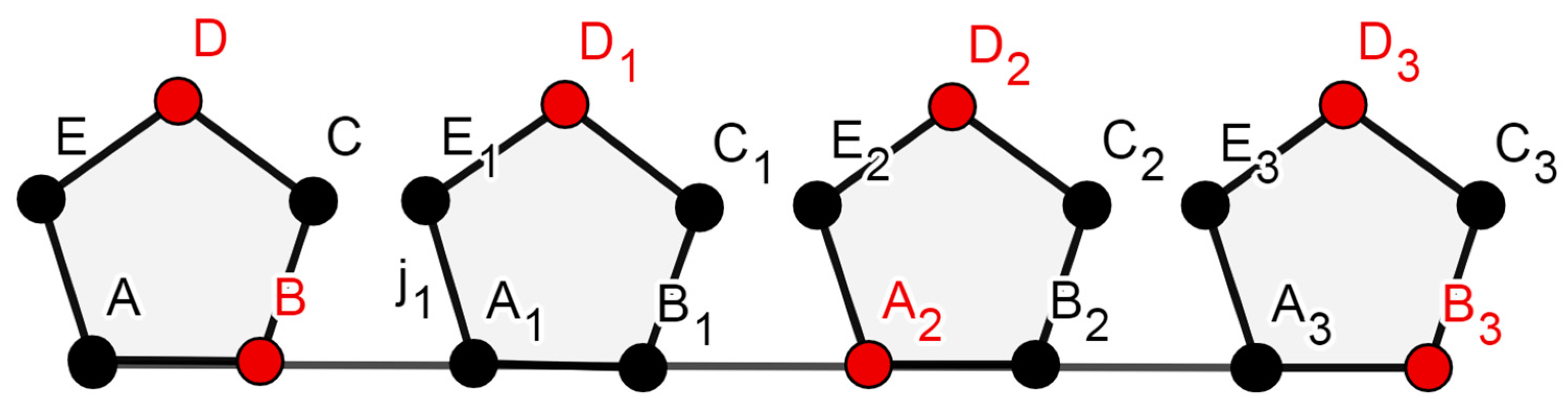
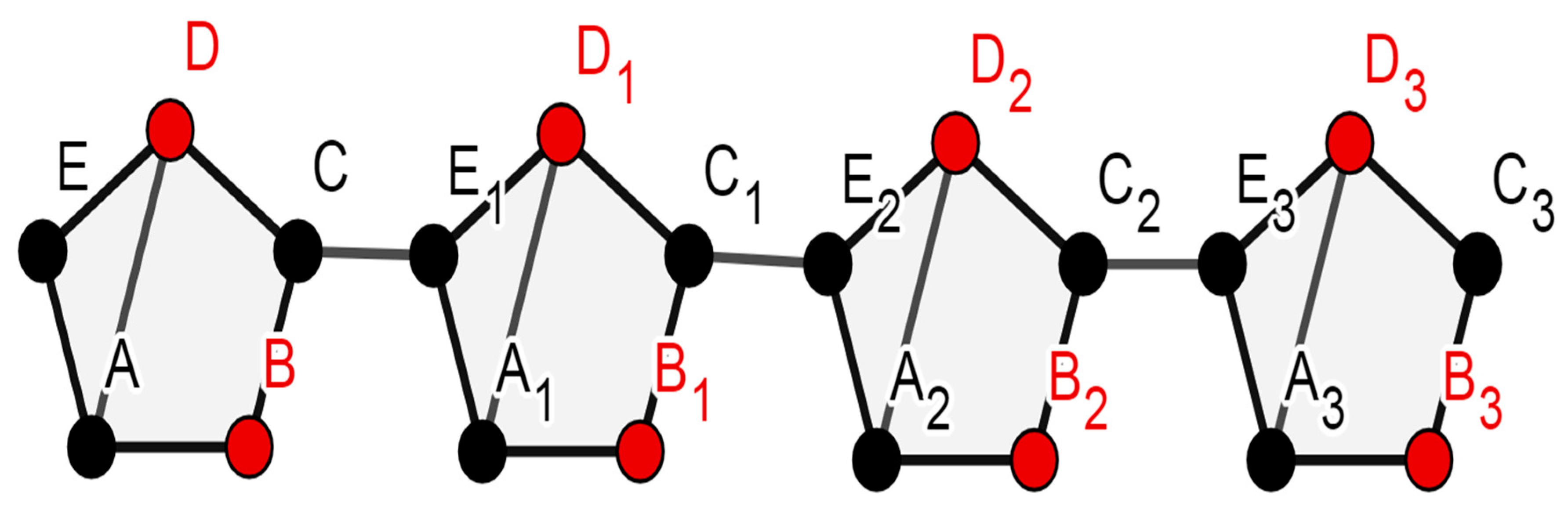
• Condition I: n ≡ 0 mod5, γ(G) = 0.4n is the domination number.
• Condition II: n ≡ 1 mod5, γ(G) = ⌈0.4n + 0.6⌉ is the domination number.
• Condition III: n ≡ 2 mod5, γ(G) = ⌊0.4n + 0.5⌋ is the domination number.
• Condition IV: n ≡ 3 mod5, γ(G) = 0.4n + 0.8 is the domination number.
• Condition V: n ≡ 4 mod5, γ(G) = ⌊0.4n + 0.8⌋ is the domination number. □

Example:

(1)

The domination number of an antiprism graph A₇ is 3, as shown in Figure 1.

In the above Figure 1 the set of red vertices form the dominating set.

(2)

The domination number of an antiprism graph A₁₀ is 4, as shown in Figure 2.

In the above Figure 2 the set of red vertices form the dominating set.

Theorem 2. 

For any alternate pentagonal snake A(PS_n), the domination number exists ∀ n ≥ 3.

Proof. 

Let G be an alternate pentagonal snake graph with 5n vertices and 5n + (n−1) edges. The following explanation delineates the minimal dominating set and its cardinality, referred to as the domination number, i.e., γ(G):

• Condition I: n ≡ 0 mod3, γ(G) = ⌊$\frac{5n}{3}$⌋ is the domination number.

• Condition II: n ≡ 1 mod3, γ(G) = $\frac{5n}{3}$ + $\frac{1}{3}$ is the domination number.

• Condition III: n ≡ 2 mod3, γ(G) = $\frac{5n}{3}$ + $\frac{2}{3}$ is the domination number. □

Example:

(1)

The domination number of an alternate pentagonal snake graph A(PS₃) is 5, as shown in Figure 3.

In the above Figure 3 the set of red vertices form the dominating set.

(2)

The domination number of an alternate pentagonal snake graph A(PS₄) is 7, as shown in Figure 4.

In the above Figure 4 the set of red vertices form the dominating set.

Theorem 3. 

Domination number exists for cycle C_n with one chord ∀ n ≥ 4.

Proof. 

Let G be a cyclic graph C_n with one chord having n vertices and n + 1 edges. The following explanation delineates the minimal dominating set and its cardinality, referred to as the domination number, i.e., γ(G):

• Condition I: n ≡ 0 mod3, γ(G) = $\frac{1n}{3}$ is the domination number.

• Condition II: n ≡ 1 mod3, γ(G) = $\frac{1n}{3}$ − $\frac{1}{3}$ is the domination number.

• Condition III: n ≡ 2 mod3, γ(G) = $\frac{1n}{3}$ + $\frac{1}{3}$ is the domination number. □

Example:

(1)

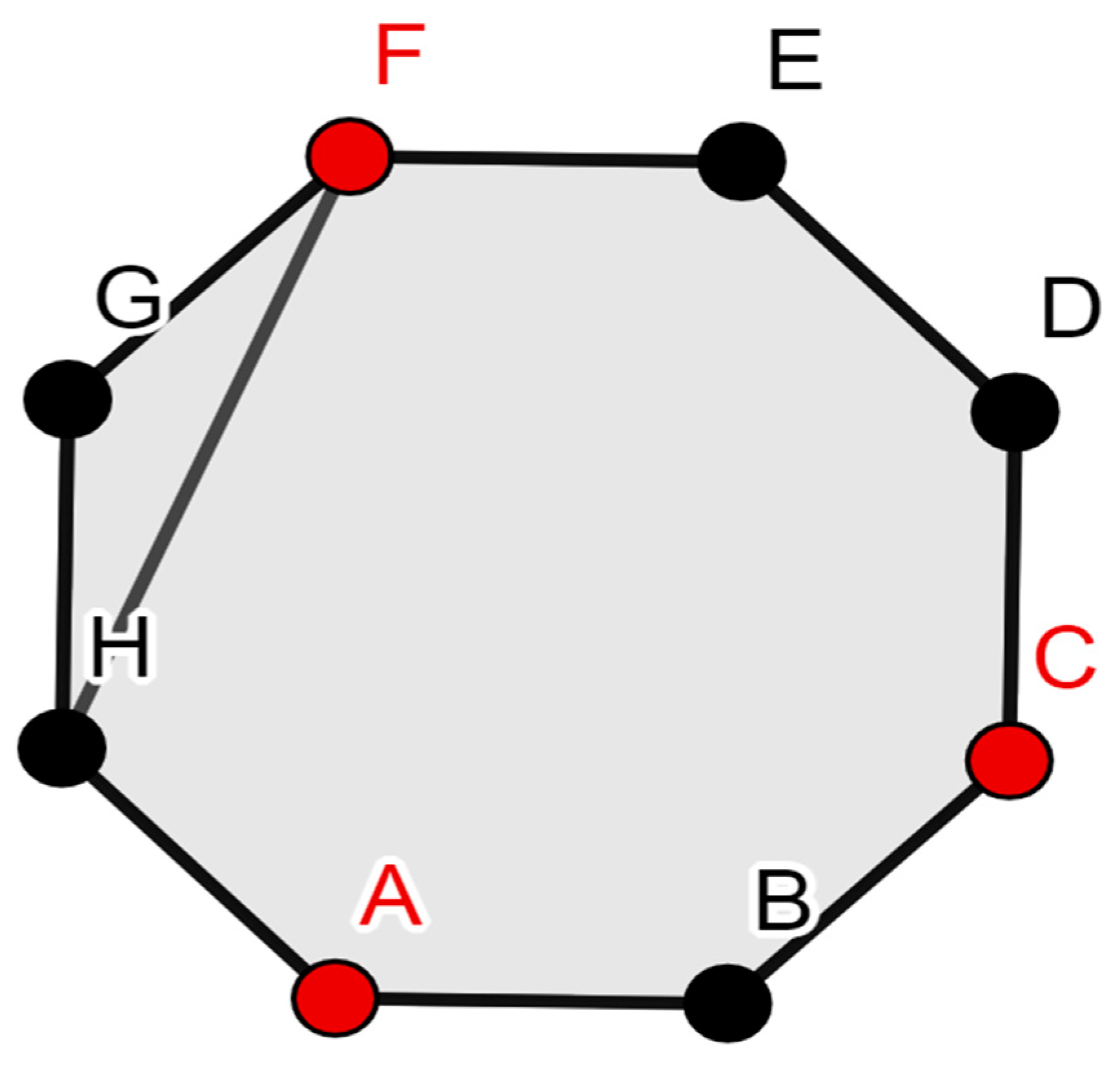
The domination number of a cycle C₈ with one chord is 3, as shown in Figure 5.

In the above Figure 5 the set of red vertices form the dominating set.

(2)

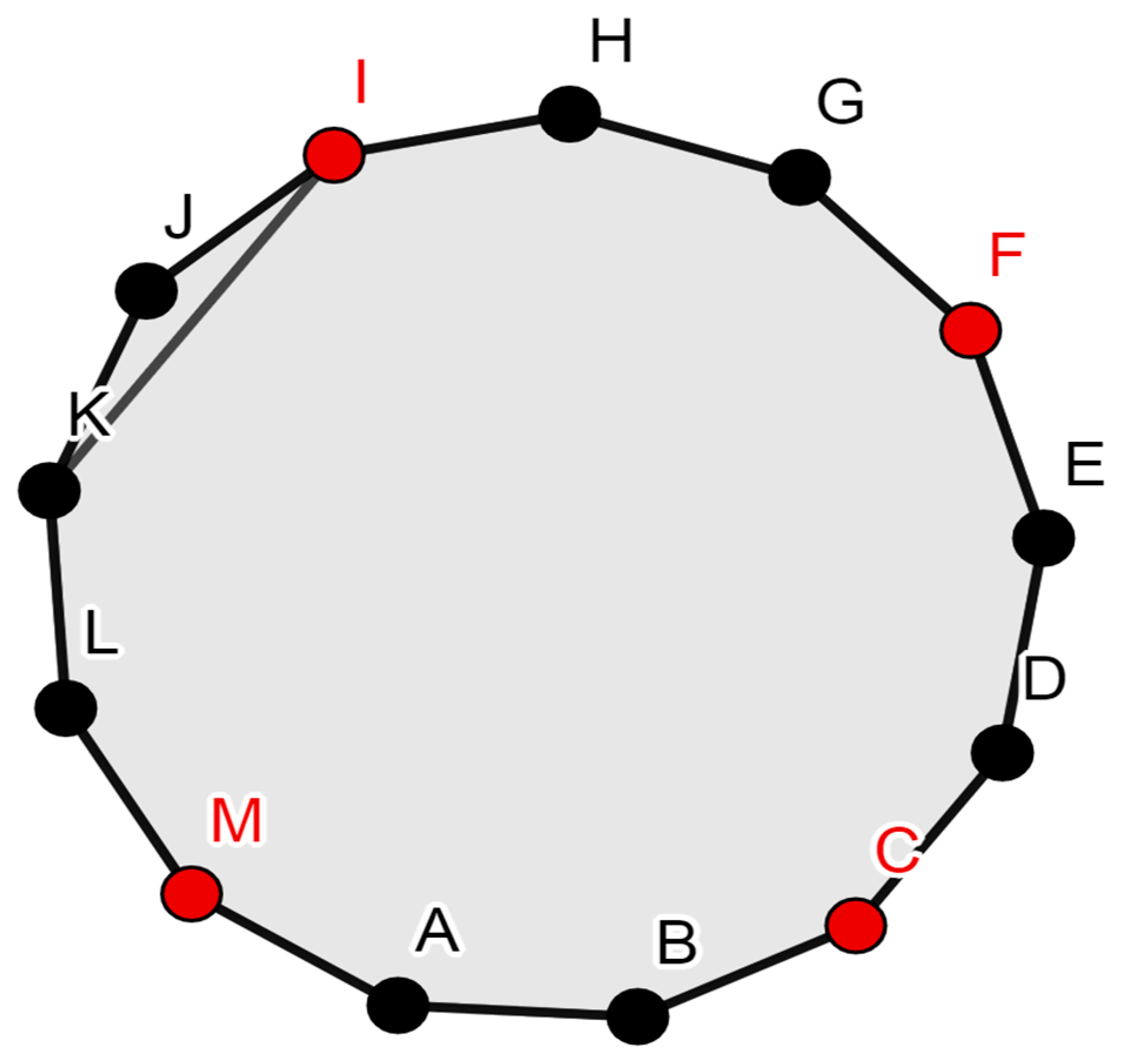
The domination number of a cycle C₁₃ with one chord is 4, as shown in Figure 6.

In the above Figure 6 the set of red vertices form the dominating set.

Theorem 4. 

Domination number exists for m-copies of cycle C_n with one chord ∀ n ≥ 4 and m ∊ N.

Proof. 

Let G be a cyclic graph C_n with one chord having m-copies with mn vertices and m(n + 1) + (m−1) edges. The following explanation delineates the minimal dominating set and its cardinality, referred to as the domination number, i.e., γ(G):

• Condition I: n ≡ 0 mod3, γ(G) = $\frac{1n}{3}$m is the domination number.

• Condition II: n ≡ 1 mod3, γ(G) = ($\frac{1n}{3}$ − $\frac{1}{3}$)m is the domination number.

• Condition III: n ≡ 2 mod3, γ(G) = ($\frac{1n}{3}$ + $\frac{1}{3}$)m is the domination number. □

Example:

(1)

The domination number of 4-copies of cycle C₅ with one chord is 8, as shown in Figure 7.

In the above Figure 7 the set of red vertices form the dominating set.

(2)

The domination number of 3-copies of cycle C₆ with one chord is 6, as shown in Figure 8.

In the above Figure 8 the set of red vertices form the dominating set.

4. Concluding Remarks

We have proved the existence of domination number for graphs like antiprism graph A_n, alternate pentagonal snake A(PS_n), cycle with one chord and m-copies of cycle C_n with one chord. This versatile approach not only facilitates graph duplication, merging, and reconstruction but also opens up avenues for advanced graph operations in the future. Researchers are actively exploring ways to improve result comparability across diverse graphs, making it an area of ongoing investigation and potential breakthroughs.