Hydrological 2D Modelling of Lithaios River Flows (Greece) Using GIS and Geostatistics for Environmental and Agricultural Water Resources Administration ^†

Abstract

The goal of our investigation is the hydrological 2D modelling of Lithaios River’s (Central Greece) streamflow, using GIS and geostatistics for studying water velocity and discharge, stage elevation, and hydraulic features (streamflow depth, water flow area, wettable circumference, hydraulic radius and depth, n Manning’s coefficient, Chow’s composite n, Froude number, etc.). Moreover, compilations and validations of rating curves (RC) were performed from a series of stage h(t)–discharge Q(t) couples metrics, aiming to use these as a river toolkit to aid environmental and agriculture surface water resources management and help environmental flows calculation, streamflow tracking, and irrigation programming in the regional basin range. The statistical results showed that the Froude number during the study period was Fr < 1 showing that Lithaios River’s streamflow is classified as subcritical. The models’ validation outcomes by using various statistics and geostatistical alternative methods, model simulations and statistics errors criterions, were correlated with the retrieved power models’ streamflow data matching for the RC curves and 2D GIS modelling and mapping of river velocity and discharge relationships and were highly satisfying since the stabilities of the deployed relationships were solid. The outcomes of the study results are recommended to provide a hydrological serving toolkit for environmental water resources administration and irrigation programming. This toolkit could assist water supply principalities to rapidly and precisely calculate streamflow volumes and features with a minimal cost rate and workload, and it could be engaged in water supply and agricultural watering administration, the calculation of environmental flows, flood protection, groundwater recharge, and other objectives.

1. Introduction

Streamflow velocity and discharge rate, water elevation, hydraulic deep, and flow form are the main themes in hydrology and are closely linked to water supply, quality and administration, flood protection, dewatering, irrigation, dam construction, and other related themes [1,2,3]. The streamflow velocity and discharge rate have a significant effect on water’s retention period and quality [2,4,5]. Thus, these variables are typically needed for hydro-systems modelling. Unfortunately, the ongoing streamflow monitoring on a river’s cross-section is commonly unfeasible or very costly [2,3,4,5,6]. Fast and accurate discharges calculation is of high importance for a great number of environmental engineering projects (real-time flood forecasting, water resources administration, etc.) [2,5,6,7]. The goal of our research is hydrological 2D streamflow modelling of Lithaios River (Central Greece), using GIS, and geostatistics for studying water velocity and discharge, stage elevation, and hydraulic features (streamflow depth, water flow area, wettable circumference, hydraulic radius and depth, n Manning’s coefficient, Chow’s composite n, Froude number, etc.).

2. Materials and Methods

2.1. Lithaios River Measurements, Instruments Used, and Specifications

The study was conducted in Lithaios River (top width = 15 m) at Trikala monitoring station (M-S), in the region of Thessaly in Central Greece. A propeller current flow [7,8] meter (OTT) was employed together with a modern electronic metering system including a flow computer, data logger, and a real-time display monitor, all calibrated by the manufacturer. River flow data were computed by averaging over a 60 + 60 s measured couple. Vertical measurements of water depths and velocities were performed for temporal monitoring of the cross-section’s velocity and discharge variation [8].

2.2. Hydrological Methodology

The river’s streamflow velocity and the depths and widths of the defined segments were measured and engaged for the estimation of the cross-section’s mean discharge of every segment [2,3,4,5,6,7,8]. The overall discharge [2,3,4,5,6,7,8] was estimated by the mid-section methodology [5,6,7,8]. The features of the cross-section, the water flow velocity of the defined segments and the overall mean flow velocity, were metered, computed, modeled, and depicted in diagrams and GIS maps, respectively, building up a hydrological toolkit for Lithaios River. Water stage elevation and flow measurements were taken monthly for a period of 1 year (January to December). In addition, more measurable variables (streamflow depth, the defined segments’ width, overall river width, and water stage elevation) were measured, and more hydraulic features (streamflow depth, water flow area, wettable circumference, hydraulic radius and depth, n Manning’s coefficient, Chow’s composite n, Froude number, etc.) were computed and depicted in diagrams and saved in the hydrological toolkit. Equation (1) was applied in order to calculate the river flow velocities.

$$V_{i=1}^n=a+(b_{eq}\times N_{eq})$$

(1)

where $V_{i=1}^n=\mathrm{s}\mathrm{t}\mathrm{r}\mathrm{e}\mathrm{a}\mathrm{m}$ flow velocity (m s⁻¹), n is the number of cross-section segments, $a$ = the initial speed to overcome mechanical resistance, b_eq = the system’s calibration constant, and N_eq = the equipment’s rotations per second.

Equation (2) was used for the river’s cross-section total discharge.

$$Q_T=\sum _{g=1}^n{V}_{i=1}^n{A}_{J=1}^n$$

(2)

where $Q_T$ = total discharge (m³ s⁻¹) of the river’s cross-section, g = 1…n is the number of cross-section segments, $V_{i=1}^n$ = the mean flow velocity of each cross-section segment (m s⁻¹), and $A_{J=1}^n=$ the wet flow area of each cross-section segment (m²).

Couples of stage water elevation h(t) and discharge Q(t) measurements were utilized to develop mathematical relationships between them. Lithaios River rating curves (h(t) − Q(t)) [2,3,4,5,6,7,8] and changes in the riverbed were computed on the basis of the measured variables using various model equations for regression, ANOVA statistical analysis, and the model’s fit F test by utilizing the IBM SPSS v.26 statistics software [2,3,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25].

2.3. Statistical and Geostatistical Data Analysis, Flow Velocity and Discharge Modelling, and 2D Mapping Methodology

The data were analyzed through the use of IBM SPSS v.26 [2,9,11,12] statistics software. The results are the observations’ averages. ANOVA (analysis of variance) [2,3,9,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25] was used to assess velocity ${(V}_{i=1}^n$), discharge ($Q_T$), and hydraulic depth effects. In the present study, we used geostatistics (the Kriging method with power model) [2,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25] for modelling and GIS (geographical information system) hydrological 2D mapping of Lithaios River’s water velocity and discharge. Furthermore, the validation of $Q_T$ and $V_{i=1}^n$ involves analysis of residual errors, which is the gap between predicted and observed data values and the bias forecast between over- and underestimates. For this purpose, we applied the statistical criteria described by other studies [2,11,12,13,14,15,16,18,19,20,22,23,24,25,26,27,28], such as the equations for residual sum squares (RSS), standard error (SE), and root mean square error (RMSE).

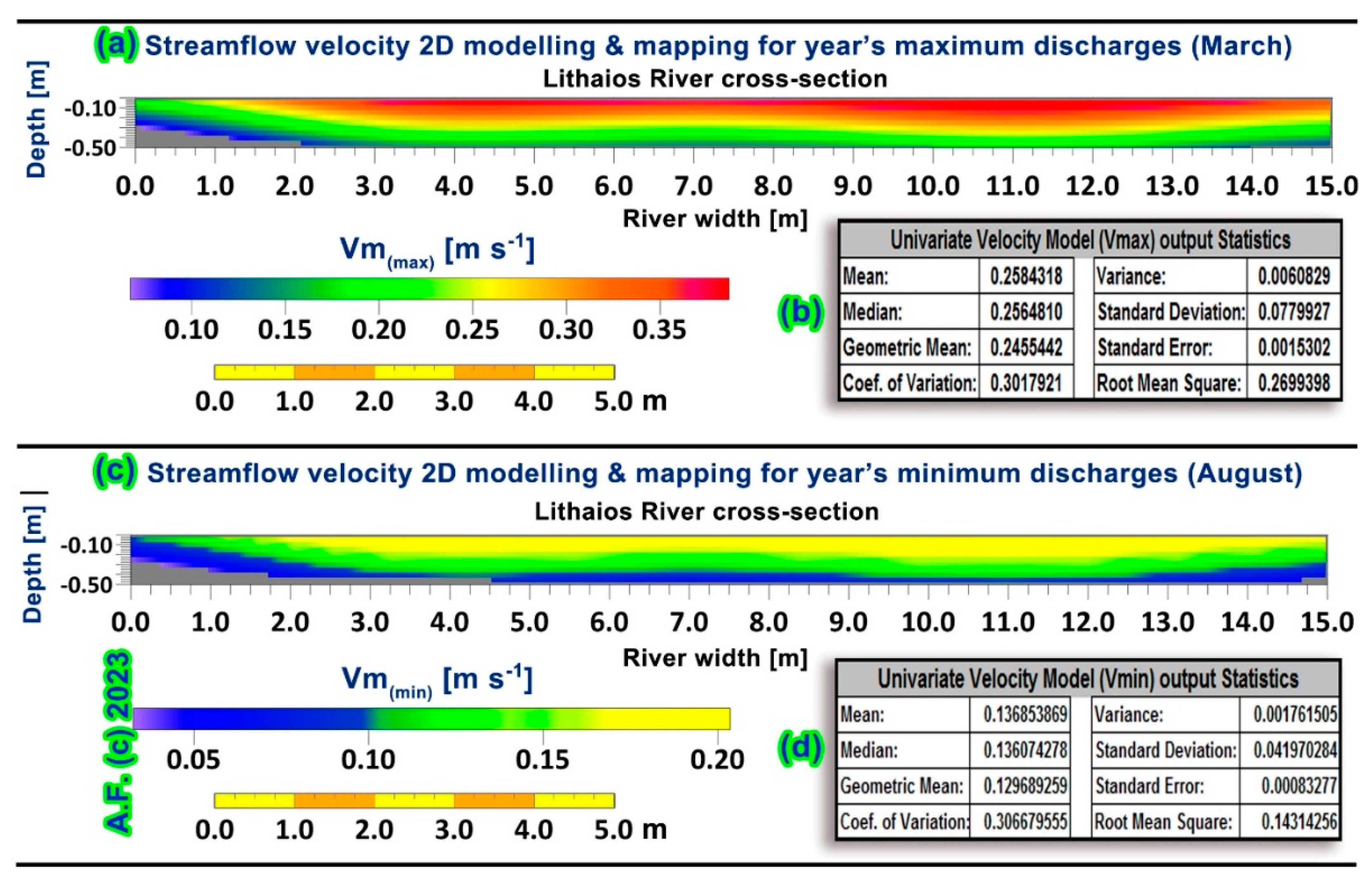
3. Results and Discussion

The streamflow velocity 2D modelling [2,27] results of the Lithaios River cross-section for the year’s maximum (March) and minimum (August) water discharges and the univariate velocity model output statistics are depicted in Figure 1a–d. Lithaios River mean water flow velocity ${(V}_{i=1}^n$) of the cross-section segments for the year’s maximum (March) and minimum (August) water discharge results show that $V_{i=1}^n$(max) ranged 0.199–0.329 (m s⁻¹) and $V_{i=1}^n$(min) ranged 0.098–0.177 (m³ s⁻¹).

The flow velocity ${(V}_{i=1}^n$) statistics [$\stackrel{-}{x}$ (mean), median, geometric mean, coefficient of variation (CV), s² (variance), and s (standard deviation)] for the year’s maximum discharges (March) are presented in Figure 1b and the year’s minimum discharges (August) are presented in Figure 1d. Velocity fluctuation of a river’s cross-section can be specified by means of descriptive statistics [2,3,9,27,28,29], and of all the descriptive statistics, the coefficient of variation (CV) is the most important measure. [2,9]. The results for both CVs’ of the cross-sections velocity variability for the year’s maximum (March) (CV = 0.302) and minimum (August) (CV = 0.307) discharges were classified as moderate variability $V_{i=1}^n$. The resulting spatial distribution of water flow velocities obtained using river cross-section measurements was best fitted using the Kriging with power model, which resulted in minimum residual sum squares (RSS = 0.0001694), and the RSS used as one of the criteria to choose the greatest model. The other criteria used included the standard error (SE) and root mean square error (RMSE), as in other studies. [2,27]. The best SE for March’s velocity modelling was the one using the Kriging with power model (SE = 0.0002236) and for August, it was also the same model (SE = 0.0008328). The RMSE using the Kriging with power model for March’s velocity modelling was found to be the best, RMSE = 0.0406329, and for August, it was found to be the best, RMSE = 0.1431426. These results are acceptable since the SE and RMSE scores should be close to zero for accurate prediction and they classified the Kriging with power model as the best model. The abovementioned outcomes, prove the validity and accuracy of the generated 2D digital velocity maps (Figure 1a,c). The relationships between the n Manning’s coefficient [2,3,5,7], the Chow’s composite n coefficient [5], and the river’s water discharges modelling (power model) resulted in high coefficients of determination (R²) [2,9,12,13,16] for the 12-month measurement study period (Figure 2a,b). The diagrams of the discharges power model (which resulted in being the best model), the Darcy–Weisbach f coefficient multinomial model, and the shear linear model for year’s maximum (March) and minimum (August) water discharges are depicted in Figure 2c,d. The R-squared gives a measure of how accurately the observable outputs are reproduced by the model based on the percentage of the total variance that is explained by the model [2,9].

The R squared output results for the n Manning’s coefficient vs. discharge showed a high R² = 0.8576 and the Chow’s composite n coefficient vs. discharge also resulted in a high R² = 0.8927. The n Manning’s coefficient and the Chow’s composite n coefficient results show a high degree of correlation with the river’s water discharges, with the Chow’s composite n coefficient found to have a higher correlation. These results indicate that Chow’s composite n [5]—which is built on the hypothesis that the overall force resisting the streamflow in the cross-section is equivalent to the summation of the resisting forces of streamflow in each of the defined segment’s regions [2,5]—more accurately approximates the force resisting water flow in Lithaios River. Finally, the statistical results showed that the Froude number during the study period was Fr < 1, showing that the Lithaios streamflow is classified as subcritical [2,3,5].

4. Conclusions

The RSS and the prediction error (SE and RMSE) results of spatial and geostatistical 2-dimensional modelling, mapping, and validation of Lithaios River water flows confirmed the validity and accuracy of the generated 2D digital GIS velocity maps of the river’s cross-sections. These outcomes have proven that the Kriging with power model had good performance and that it is regarded as very appropriate for 2-D streamflow modelling and digital mapping, as well as being suitable for other hydraulic parameters (n Manning’s coefficient, Chow’s composite n coefficient, Froude number, shear, Darcy–Weisbach f coefficient, hydraulic radius, etc.). The outcomes of the study are recommended to provide a hydrological toolkit for environmental water resources administration and irrigation programming. This toolkit could assist water supply principalities to rapidly and precisely calculate streamflow volumes and the river’s features with a minimal cost rate and workload, and it could be engaged in water supply and agricultural watering administration, the calculation of environmental flows, flood protection, groundwater recharge, and other objectives.