Modeling of Coalmine Methane Flows to Estimate the Spacing of Primary Roof Breaks
Abstract
:1. Introduction
2. Materials and Methods
3. Results
- -
- The definition domain of the points (orthogonality interval of the approximating polynomials) corresponds to S = S′ for all S′ ∈ [0, π] and L = L′ for all corresponds to S = S′ for all S′ ∈ [0, π] and L = L′ for all L′ ∈ [0, π].
4. Discussion
5. Conclusions
- Deformation-wave processes in geo-environments produce cyclic non-linearities in the nature of the air–gas regime of mine methane emissions into anthropogenic rock masses, while only a part of the gas flows is captured by the degassing network in the extraction area.
- It has been established for the first time that a decrease in the distance of the stoping face line from the start of mining of the extraction column S = from 1340 to 1120 m and the distance in front of the longwall L = from −120 to 0 m leads to undulating changes in gas release (in wells No. 2), according to a polynomial dependence.
- The influence of situational geomechanical conditions of reserve mining on the area and the shape of the local extrema of the models was clarified. These models are transformed in proportion to the development of the stoping front and are displaced at certain angles to the alignment with the longwall.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Saunois, M.; Jackson, R.B.; Bousquet, P.; Poulter, B.; Canadell, J.G. The growing role of methane in anthropogenic climate change. Environ. Res. Lett. 2016, 11, 120207. [Google Scholar] [CrossRef] [Green Version]
- Shukla, P.R.; Skea, J.; Slade, R.; Khourdajie, A.A.; van Diemen, R.; McCollum, D.; Pathak, M.; Some, S.; Vyas, P.; Fradera, R.; et al. IPCC, 2022: Climate Change 2022: Mitigation of Climate Change. Contribution of Working Group III to the Sixth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2022; p. 2913. [Google Scholar] [CrossRef]
- Silvia, F.; Talia, V.; Di Matteo, M. Coal mining and policy responses: Are externalities appropriately addressed? A meta-analysis. Environ. Sci. Policy 2021, 126, 39–47. [Google Scholar] [CrossRef]
- Rylnikova, M.V. The Harmonious Development of Mining, Science and Higher Education—A Guarantee of Stable State of Mining Regions in Russia. Sustain. Dev. Mt. Territ. 2020, 12, 154–161. (In Russian) [Google Scholar] [CrossRef]
- Golik, V.I.; Razorenov, Y.I.; Dmitrak, Y.V.; Gabaraev, O.Z. Safety improvement of the underground ore extraction considering mass geodynamics. Occup. Saf. industry. 2019, 8, 36–42. (In Russian) [Google Scholar] [CrossRef]
- Rybak, J.; Tyulyaeva, Y.; Kongar-Syuryun, C.; Khayrutdinov, A.M.; Akinshin, I. Geomechanical substantiation of parameters of technology for mining salt deposits with a backfill. Min. Sci. 2021, 28, 19–32. [Google Scholar] [CrossRef]
- Amrani, M.; Taha, Y.; El Haloui, Y.; Benzaazoua, M.; Hakkou, R. Sustainable Reuse of Coal Mine Waste: Experimental and Economic Assessments for Embankments and Pavement Layer Applications in Morocco. Minerals 2020, 10, 851. [Google Scholar] [CrossRef]
- Kongar-Syuryun, C.B.; Faradzhov, V.V.; Tyulyaeva, Y.S.; Khayrutdinov, A.M. Effect of activating treatment of halite flotation waste in backfill mixture preparation. Min. Inf. Anal. Bull. 2021, 1, 43–57. (In Russian) [Google Scholar] [CrossRef]
- Gabaraev, O.Z.; Konovalova, T.G.; Turluev, R.R. Ecology and utilization of ore dressing tailings. IOP Conf. Ser. EarthEnviron. Sci. 2022, 1021. [Google Scholar] [CrossRef]
- Khayrutdinov, A.; Paleev, I.; Artemov, S. Replacement of traditional components of the backfill mixture with man-made waste. IOP Conf. Ser. Earth Environ. Sci. 2021, 904, 012005. [Google Scholar] [CrossRef]
- Dzhioeva, A.K. Prospects for mining ecologization to reduce harmful emissions into the atmosphere. Ugol’ 2022, 10, 29–32. (In Russian) [Google Scholar] [CrossRef]
- Rybak, J.; Khayrutdinov, M.M.; Kuziev, D.A.; Kongar-Syuryun, C.B.; Babyr, N.V. Prediction of the geomechanical state of the rock mass when mining salt deposits with stowing. J. Min. Inst. 2022, 253, 61–70. (In Russian) [Google Scholar] [CrossRef]
- Adero, N.J.; Drebenstedt, C.; Prokofeva, E.N.; Vostrikov, A.V. Spatial data and technologies for geomonitoring of land use under aspect of mineral resource sector development. Eurasian Min. 2020, 1, 69–74. [Google Scholar] [CrossRef]
- Mohammadi, S.; Ataei, M.; Kakaie, R.; Mirzaghorbanali, A.; Aziz, N. A Probabilistic Model to Determine Main Caving Span by Evaluating Cavability of Immediate Roof Strata in Longwall Mining. Geotech. Geol. Eng. 2021, 39, 2221–2237. [Google Scholar] [CrossRef]
- Mohammadi, S.; Ataei, M.; Kakaie, R.; Mirzaghorbanali, A. A New Roof Strata Cavability Index (RSCi) for Longwall Mining Incorporating New Rating System. Geotech. Geol. Eng. 2019, 37, 3619–3636. [Google Scholar] [CrossRef]
- Khanal, M.; Qu, Q.; Zhu, Y.; Xie, J.; Zhu, W.; Hou, T.; Song, S. Characterization of Overburden Deformation and Subsidence Behavior in a Kilometer Deep Longwall Mine. Minerals. 2022, 12, 543. [Google Scholar] [CrossRef]
- Islavath, S.; Deb, D. Interaction of a Shield Structure with Surrounding Rock Strata Under Geo-static and Fatigue Loadings. Geotech. Geol. Eng. 2022, 40, 2949–2965. [Google Scholar] [CrossRef]
- Arasteh, H.; Esmaeili, K.; Saeedi, G.; Farsangi, M.A.E. Discontinuous Modeling of Roof Strata Caving in a Mechanized Longwall Mine in Tabas Coal Mine. Int. J. Geomech. 2022, 22, 04022040. [Google Scholar] [CrossRef]
- Rasouli, H.; Shahriar, K.; Madani, S.H. Prediction of the Height of Fracturing via Gene Expression Programming in Australian Longwall Panels: A Comparative Study. Rud. GeoloskoNaft. Zb. 2022, 37, 91–104. [Google Scholar] [CrossRef]
- Zhu, Z.; Wu, Y.; Liang, Z. Mining-Induced Stress and Ground Pressure Behavior Characteristics in Mining a Thick Coal Seam With Hard Roofs. Front. Earth Sci. 2022, 10, 843191. [Google Scholar] [CrossRef]
- Polevshchikov, G.Y. Deformation-wave processes under production face advance in coal and rocks. J. Min. Sci. 2013, 49, 715–723. [Google Scholar] [CrossRef]
- Shemyakin, E.I.; Fisenko, G.L.; Kurlenya, M.V.; Oparin, V.N.; Reva, V.N.; Glushikhin, F.P.; Rosenbaum, M.A.; Tropp, E.A.; Kuznetsov, Y.S. The effect of zonal disintegration of rocks around underground workings. Doklady Akademii Nauk 1986, 1, 289. [Google Scholar]
- Shemyakin, E.I.; Fisenko, G.L.; Kurlenya, M.V.; Oparin, V.N.; Reva, V.N.; Glushikhin, F.P.; Rozenbaum, M.A.; Tropp, E.A.; Kuznetsov, Y.S. Zonal Disintegration of Rocks and Underground Workings Stability; Siberian Branch of the Russian Academy of Sciences: Novosibirsk, Russia, 2008; p. 276. [Google Scholar]
- Young, R.P.; Nasseri, M.H.B.; Sehizadeh, M. Mechanical and seismic anisotropy of rocks from the ONKALO underground rock characterization facility. Int. J. Rock Mech. Min. 2020, 126, 104190. [Google Scholar] [CrossRef]
- Ghaffari, H.; Nasseri, M.; Young, R. Faulting of Rocks in a Three-Dimensional Stress Field by Micro-Anticracks. Sci. Rep. 2014, 4, 5011. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, Z.; Zhang, R.; Cao, Z.; Gao, M.; Zhang, Y.; Xie, J. Mechanical Behavior and Permeability Evolution of Coal under Different Mining Induced Stress Conditions and Gas Pressures. Energies 2020, 13, 2677. [Google Scholar] [CrossRef]
- Karimpouli, S.; Tahmasebi, P.; Ramandi, H.L. A review of experimental and numerical modeling of digital coalbed methane: Imaging, segmentation, fractures modeling and permeability prediction. Int. J. Coal Geol. 2020, 228, 103552. [Google Scholar] [CrossRef]
- Szlazak, N.; Obracaj, D.; Korzec, M. Estimation of Gas Loss in Methodology for Determining Methane Content of Coal Seams. Energies 2021, 14, 982. [Google Scholar] [CrossRef]
- Brigida, V.S.; Zinchenko, N.N. Methane Release in Drainage Holes Ahead of Coal Face. J. Min. Sci. 2014, 50, 60–64. [Google Scholar] [CrossRef]
- Gryazev, M.V.; Kachurin, N.M.; Vorob’ev, S.A. Mathematical Models of Gas-Dynamic and Thermophysical Processes in Underground Coal Mining at Different Stages of Mine Development. J. Min. Inst. 2017, 223, 99–108. [Google Scholar] [CrossRef]
- Qu, Q.; Shi, J.; Wilkins, A. A Numerical Evaluation of Coal Seam Permeability Derived from Borehole Gas Flow Rate. Energies 2022, 15, 3828. [Google Scholar] [CrossRef]
- Cheng, Y.P.; Wang, L.; Zhang, X.L. Environmental impact of coal mine methane emissions and responding strategies in China. Int. J. Greenh. Gas Control. 2011, 5, 157–166. [Google Scholar] [CrossRef]
- Jiangfu, H.; Wenchao, H.; Chengpeng, Z.; Zhongguang, S.; Xiaoyi, S. Numerical simulation on the deformation characteristics of borehole failure in gas-bearing coal seams considering the effective stress principle under complicated stress path conditions. Geomech. Geophys. Geo-Energy Geo-Resour. 2022, 8, 95. [Google Scholar] [CrossRef]
- Hou, X.; Liu, S.; Zhu, Y.; Yang, Y. Evaluation of gas contents for a multi-seam deep coalbed methane reservoir and their geo-logical controls: In situ direct method versus indirect method. Fuel 2020, 265, 116917. [Google Scholar] [CrossRef]
- Bosikov, I.I.; Klyuev, R.V.; Khetagurov, V.N.; Azhmukhamedov, I.M. Development of methods and controls for aerogasodynamic processes at mining sites. Sustain. Dev. Mt. Territ. 2021, 1, 77–83. (In Russian) [Google Scholar] [CrossRef]
- Kadochnikov, V.G.; Dvoynikov, M.V. Development of Technology for Hydromechanical Breakdown of Mud Plugs and Improvement of Well Cleaning by Controlled Buckling of the Drill String. Appl. Sci. 2022, 12, 6460. [Google Scholar] [CrossRef]
- Bosikov, I.I.; Klyuev, R.V.; Azhmukhamedov, I.M.; Revazov, V.C. Assessment of coal mine ventilation control using statistical dynamics methods. Min. Inf. Anal. Bull. 2021, 11, 123–135. (In Russian) [Google Scholar] [CrossRef]
- Yao, H.; Peng, H.; Hong, B.; Guo, Q.; Ding, H.; Hong, Y.; Zhu, Y.; Cai, C.; Chi, J. Environmental Controls on Multi-Scale Dynamics of Net Carbon Dioxide Exchange From an Alpine Peatland on the Eastern Qinghai-Tibet Plateau. Front. Plant Sci. 2022, 12, 791343. [Google Scholar] [CrossRef]
- Dzhioeva, A.K.; Brigida, V.S. Spatial non-linearity of methane release dynamics in underground boreholes for sustainable mining. J. Min. Inst. 2020, 245, 522–530. [Google Scholar] [CrossRef]
- Qu, Q.; Balusu, R.; Belle, B. Specific gas emissions in Bowen Basin longwall mines, Australia. International. Int. J. Coal Geol. 2022, 261, 104076. [Google Scholar] [CrossRef]
- Borowski, M.; Zyczkowski, P.; Cheng, J.; Luczak, R.; Zwolinska, K. The Combustion of Methane from Hard Coal Seams in Gas Engines as a Technology Leading to Reducing Greenhouse Gas Emissions—Electricity Prediction Using ANN. Energies 2020, 13, 4429. [Google Scholar] [CrossRef]
- Alipenhani, B.; Majdi, A.; Amnieh, H.B. Determination of Caving Hydraulic Radius of Rock Mass in Block Caving Method using Numerical Modeling and Multivariate Regression. J. Min. Environ. 2022, 13, 217–233. [Google Scholar] [CrossRef]
- Khanal, M.; Adhikary, D.; Balusu, R.; Wilkins, A.; Belle, B. Mechanical study of shear failure of vertical goaf drainage hole. Geotech. Geol. Eng. 2022, 40, 1899–1920. [Google Scholar] [CrossRef]
- Niu, Y.; Zhang, X.; Wang, E.; Li, Z.; Cheng, Z.; Duan, X.; Li, H.; Wei, Y.; Qian, J.; Cai, G.; et al. A new method of monitoring the stability of boreholes for methane drainage from coal seams. Meas. J. Int. Meas. Confed. 2020, 15, 107521. [Google Scholar] [CrossRef]
- Brigida, V.S.; Golik, V.I.; Dmitrak, Y.V.; Gabaraev, O.Z. Ensuring Stability of Undermining Inclined Drainage Holes During Intensive Development of Multiple Gas-Bearing Coal Layers. J. Min. Inst. 2019, 239, 497–501. [Google Scholar] [CrossRef]
- Qu, Q.; Guo, H.; Balusu, R. Methane emissions and dynamics from adjacent coal seams in a high permeability multi-seam mining environment. Int. J. Coal Geol. 2022, 253, 103969. [Google Scholar] [CrossRef]
- Li, Y.; Wu, S.; Nie, B.; Ma, Y. A new pattern of underground space-time Tridimensional gas drainage: A case study in Yuwu coal mine, China. Energy Sci. Eng. 2019, 7, 399–410. [Google Scholar] [CrossRef] [Green Version]
- Dzhioeva, A.K.; Tekhov, A.V. Reduction of mine methane emissions for ensuring sustainable development of geotechnologies in the transition to Industry 3.0. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1064, 012008. [Google Scholar] [CrossRef]
- Qin, B.; Shi, Z.S.; Hao, J.F.; Ye, D.L.; Liang, B.; Sun, W.J. Analysis of the Space–Time Synergy of Coal and Gas Co-mining. ACS Omega 2022, 7, 13737–13749. [Google Scholar] [CrossRef]
- Shi, Z.; Ye, D.; Hao, J.; Qin, B.; Li, G. Research on Gas Extraction and Cut Flow Technology for Lower Slice Pressure Relief Gas under Slice Mining of Extra-thick Coal Seam. ACS Omega 2022, 7, 24531–24550. [Google Scholar] [CrossRef]
- Zhang, C.; Tu, S.; Bai, Q.; Yang, G.; Zhang, L. Evaluating pressure-relief mining performances based on surface gas vent hole extraction data in longwall coal mines. J. Nat. Gas Eng. 2015, 24, 431–440. [Google Scholar] [CrossRef]
- Liu, T.; Zhao, Y.; Kong, X.; Lin, B.; Zou, Q. Dynamics of coalbed methane emission from coal cores under various stress paths and its application in gas extraction in mining-disturbed coal seam. J. Nat. Gas Eng. 2022, 104, 104677. [Google Scholar] [CrossRef]
- Khanal, M.; Poulsen, B.; Adhikary, D.; Balusu, R.; Wilkins, A.; Belle, B. Numerical study of stability and connectivity of vertical goaf drainage holes. Geotech. Geol. Eng. 2021, 39, 2669–2679. [Google Scholar] [CrossRef]
- Zhou, J.; Lin, H.; Jin, H.; Li, S.; Yan, Z.; Huang, S. Cooperative prediction method of gas emission from mining face based on feature selection and machine learning. Int. J. Coal Sci. Technol. 2022, 9, 51. [Google Scholar] [CrossRef]
- Zhong, S.; Lin, D. Evaluation of the Coordination Degree of Coal and Gas Co-Mining System Based on System Dynamics. Sustainability. 2022, 14, 16434. [Google Scholar] [CrossRef]

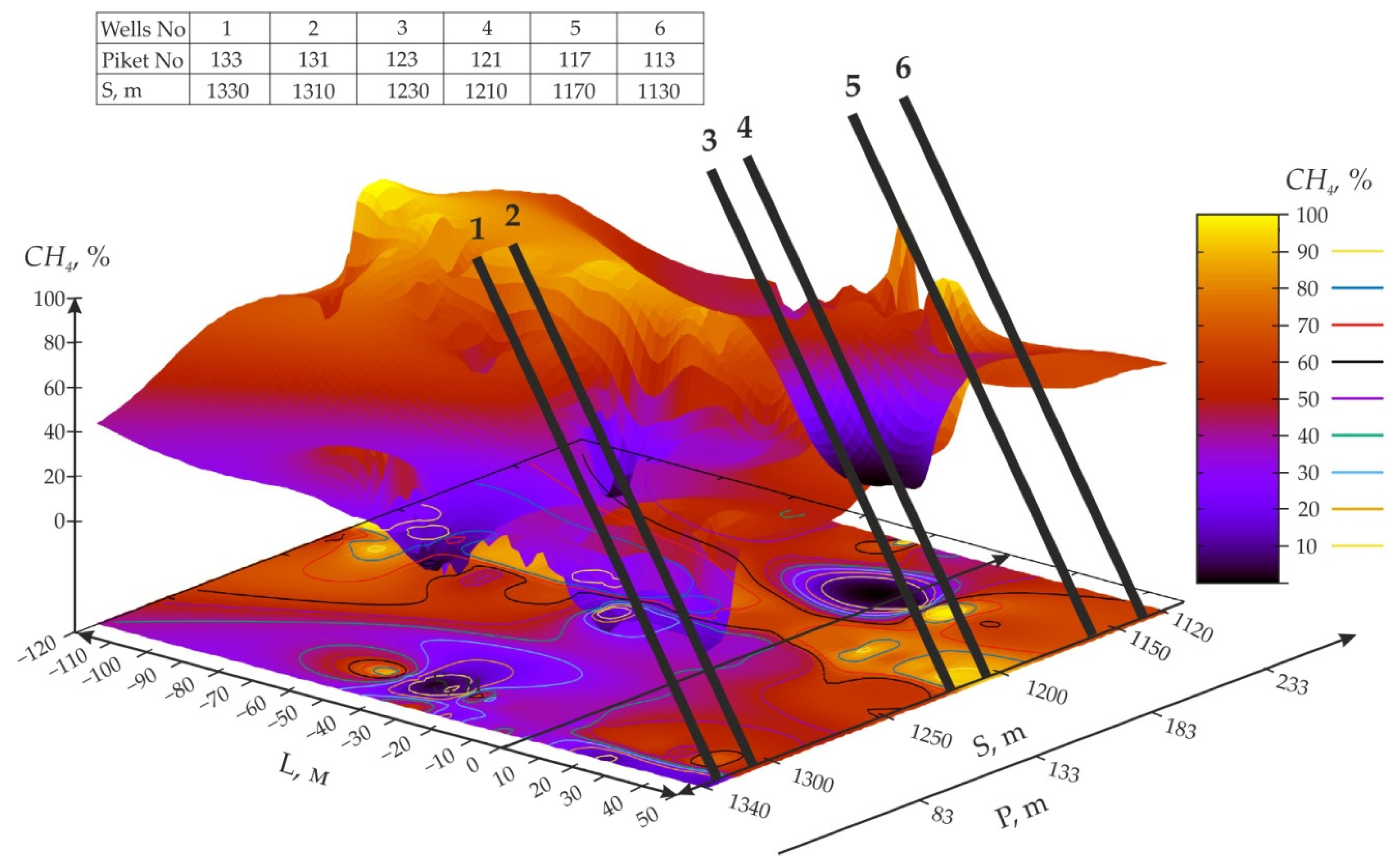
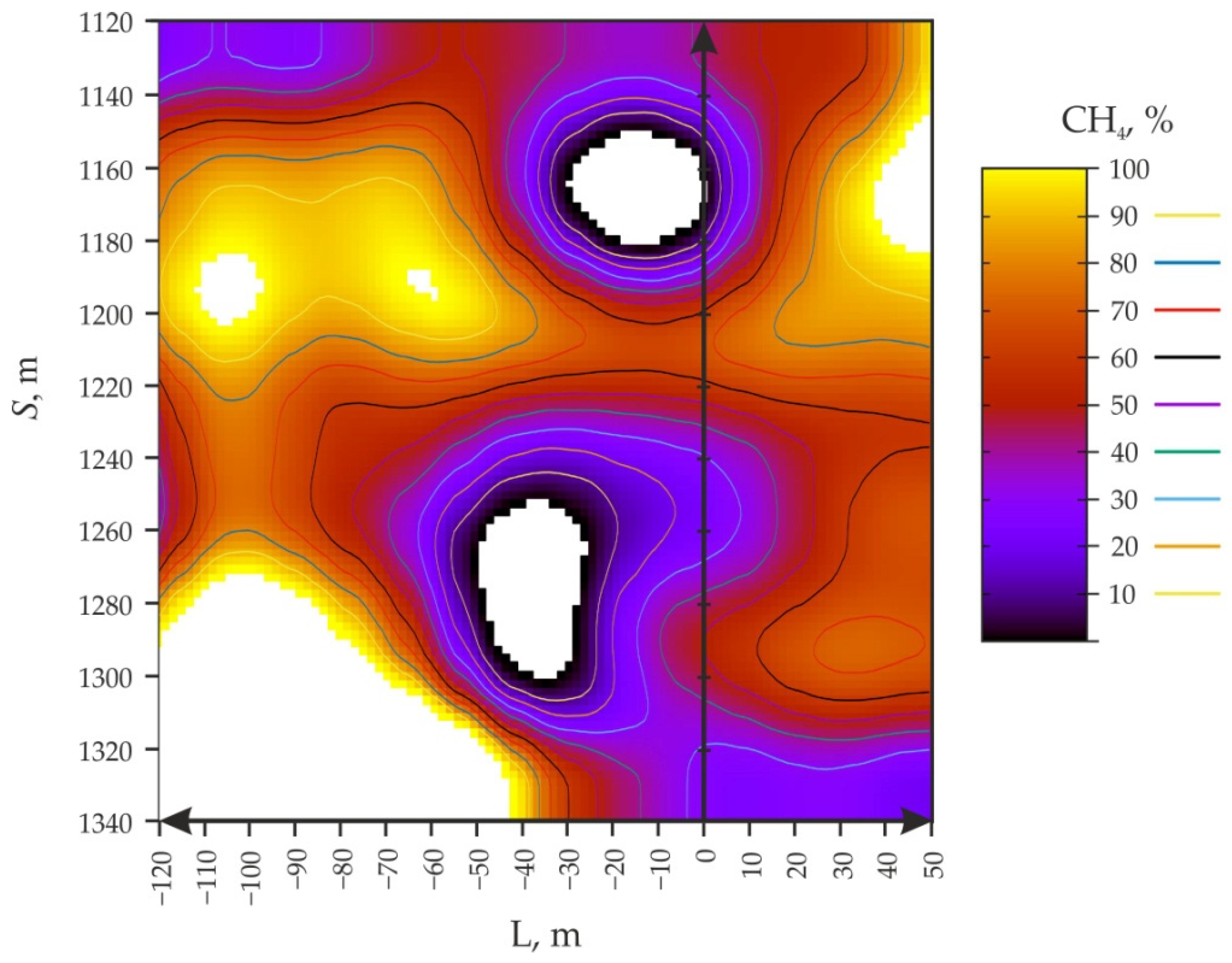
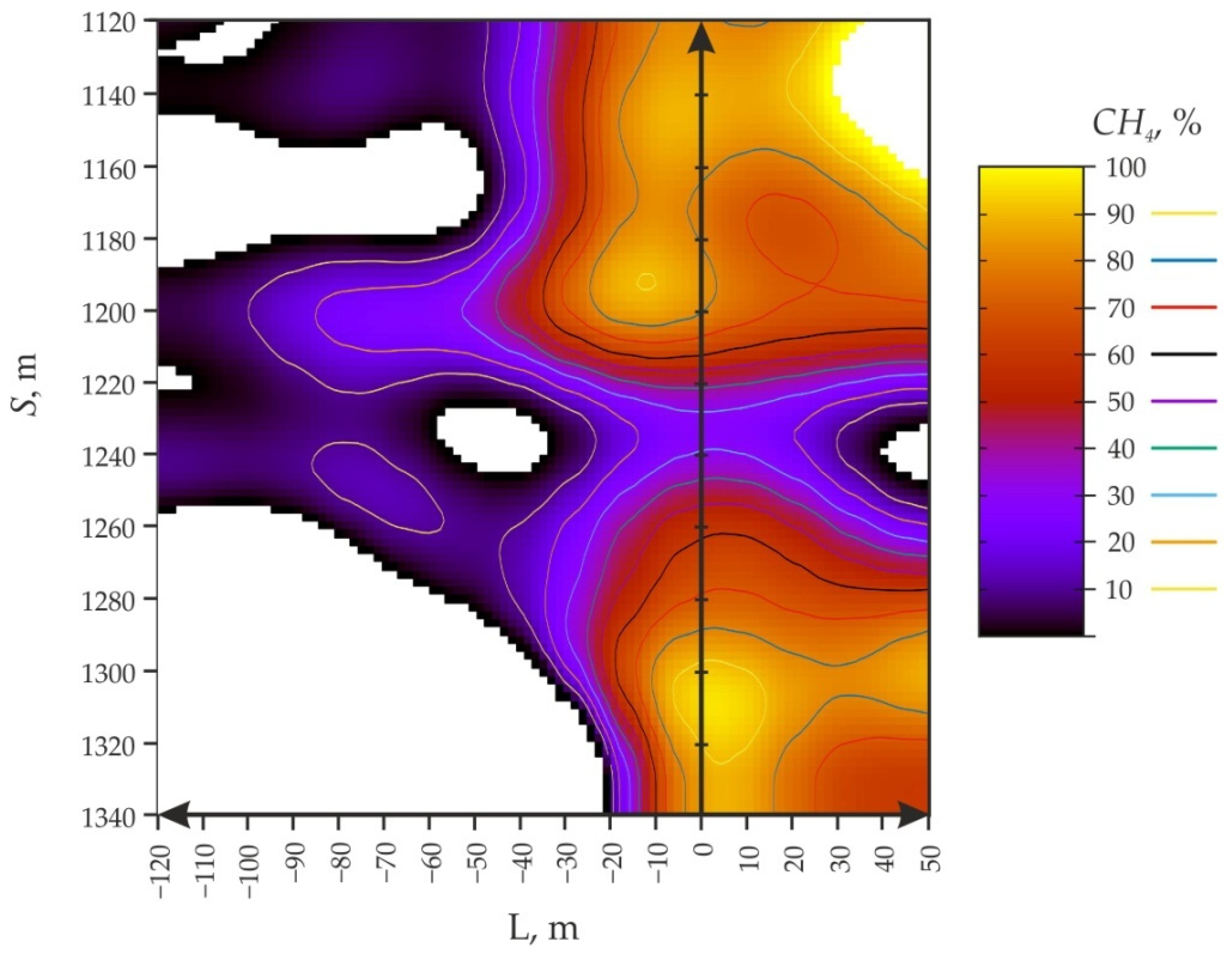
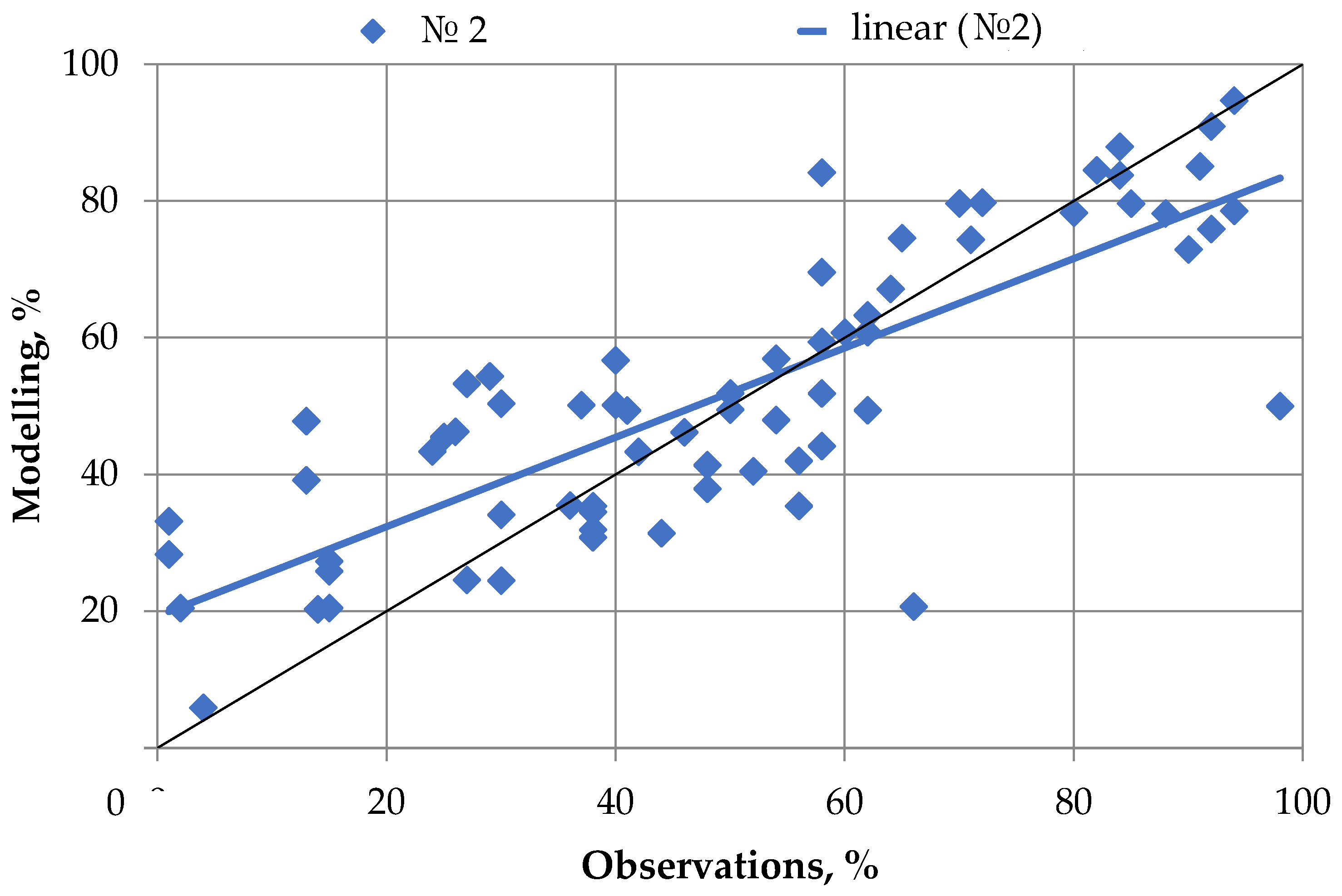

| N | Wells No. 4 | Wells No. 2 | ||||
|---|---|---|---|---|---|---|
| L, m | S, m | Measurements CH4, % | L, m | S, m | Measurements CH4, % | |
| 1 | −20 | 1330 | 2 | −30 | 1330 | 60 |
| 2 | −18 | 1330 | 10 | −28 | 1330 | 40 |
| 3 | −6 | 1330 | 100 | −26 | 1330 | 27 |
| 4 | 8 | 1330 | 88 | −24 | 1330 | 30 |
| 5 | 10 | 1330 | 86 | −22 | 1330 | 13 |
| 6 | 16 | 1330 | 86 | −20 | 1330 | 25 |
| 7 | 28 | 1330 | 65 | −18 | 1330 | 24 |
| 8 | 46 | 1330 | 48 | −6 | 1330 | 44 |
| 9 | −26 | 1310 | 15 | 8 | 1330 | 30 |
| 10 | −12 | 1310 | 72 | 10 | 1330 | 27 |
| 11 | −10 | 1310 | 100 | 16 | 1330 | 15 |
| 12 | −4 | 1310 | 95 | 28 | 1330 | 15 |
| 13 | 8 | 1310 | 90 | 46 | 1330 | 15 |
| 14 | 26 | 1310 | 80 | 50 | 1330 | 14 |
| 15 | 34 | 1310 | 72 | −50 | 1310 | 90 |
| N | Wells No. 4 | Wells No. 2 | ||
|---|---|---|---|---|
| Model, % | Measurements, % | Model, % | Measurements, % | |
| 1 | 6.51 | 2 | 60.77 | 60 |
| 2 | 20.19 | 10 | 56.72 | 40 |
| 3 | 76.19 | 100 | 53.30 | 27 |
| 4 | 87.34 | 88 | 50.37 | 30 |
| 5 | 85.77 | 86 | 47.82 | 13 |
| 6 | 79.08 | 86 | 45.51 | 25 |
| 7 | 65.99 | 65 | 43.36 | 24 |
| 8 | 60.70 | 48 | 31.43 | 44 |
| 9 | 5.62 | 15 | 24.47 | 30 |
| 10 | 73.84 | 72 | 24.57 | 27 |
| 11 | 79.95 | 100 | 25.89 | 15 |
| 12 | 92.23 | 95 | 27.30 | 15 |
| 13 | 94.23 | 90 | 20.46 | 15 |
| 14 | 79.06 | 80 | 20.29 | 14 |
| 15 | 77.16 | 72 | 72.92 | 90 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brigida, V.S.; Golik, V.I.; Dzeranov, B.V. Modeling of Coalmine Methane Flows to Estimate the Spacing of Primary Roof Breaks. Mining 2022, 2, 809-821. https://doi.org/10.3390/mining2040045
Brigida VS, Golik VI, Dzeranov BV. Modeling of Coalmine Methane Flows to Estimate the Spacing of Primary Roof Breaks. Mining. 2022; 2(4):809-821. https://doi.org/10.3390/mining2040045
Chicago/Turabian StyleBrigida, Vladimir Sergeevich, Vladimir Ivanovich Golik, and Boris Vitalievich Dzeranov. 2022. "Modeling of Coalmine Methane Flows to Estimate the Spacing of Primary Roof Breaks" Mining 2, no. 4: 809-821. https://doi.org/10.3390/mining2040045
APA StyleBrigida, V. S., Golik, V. I., & Dzeranov, B. V. (2022). Modeling of Coalmine Methane Flows to Estimate the Spacing of Primary Roof Breaks. Mining, 2(4), 809-821. https://doi.org/10.3390/mining2040045







