Comparative Analysis of Non-Pneumatic Tire Spoke Designs for Off-Road Applications: A Smoothed Particle Hydrodynamics Perspective
Abstract
1. Introduction
2. Non-Pneumatic Tire Spokes Modeling
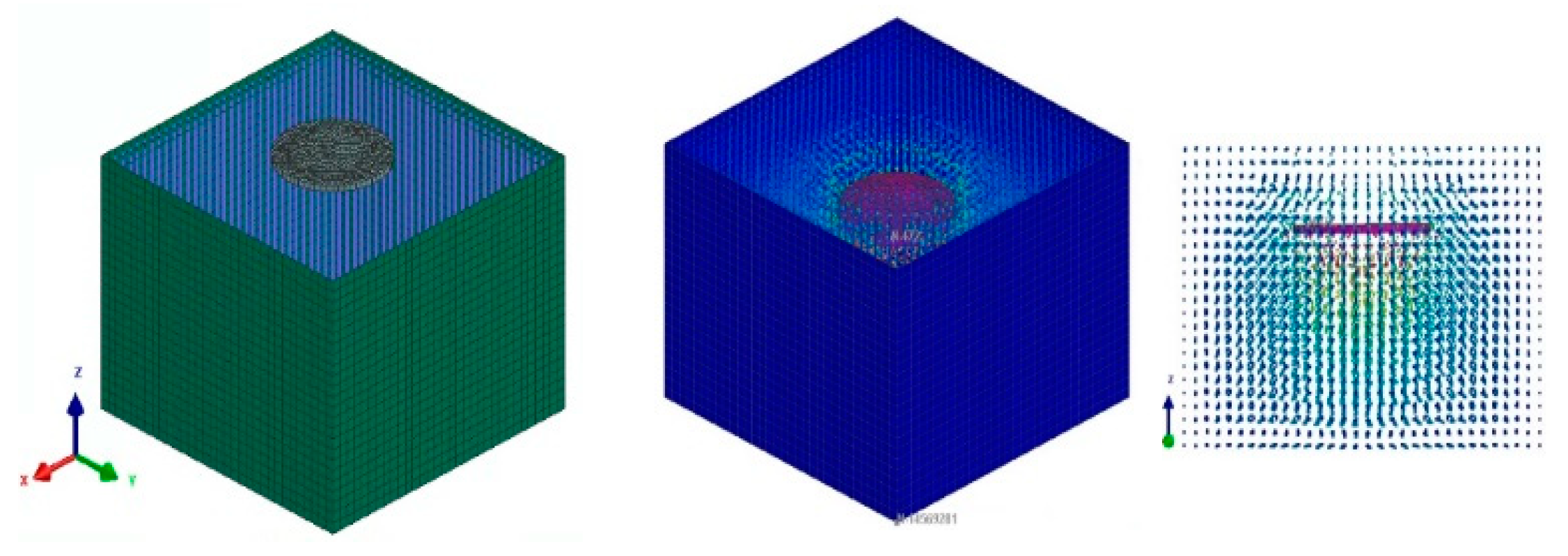
3. Soil Modeling and Calibration
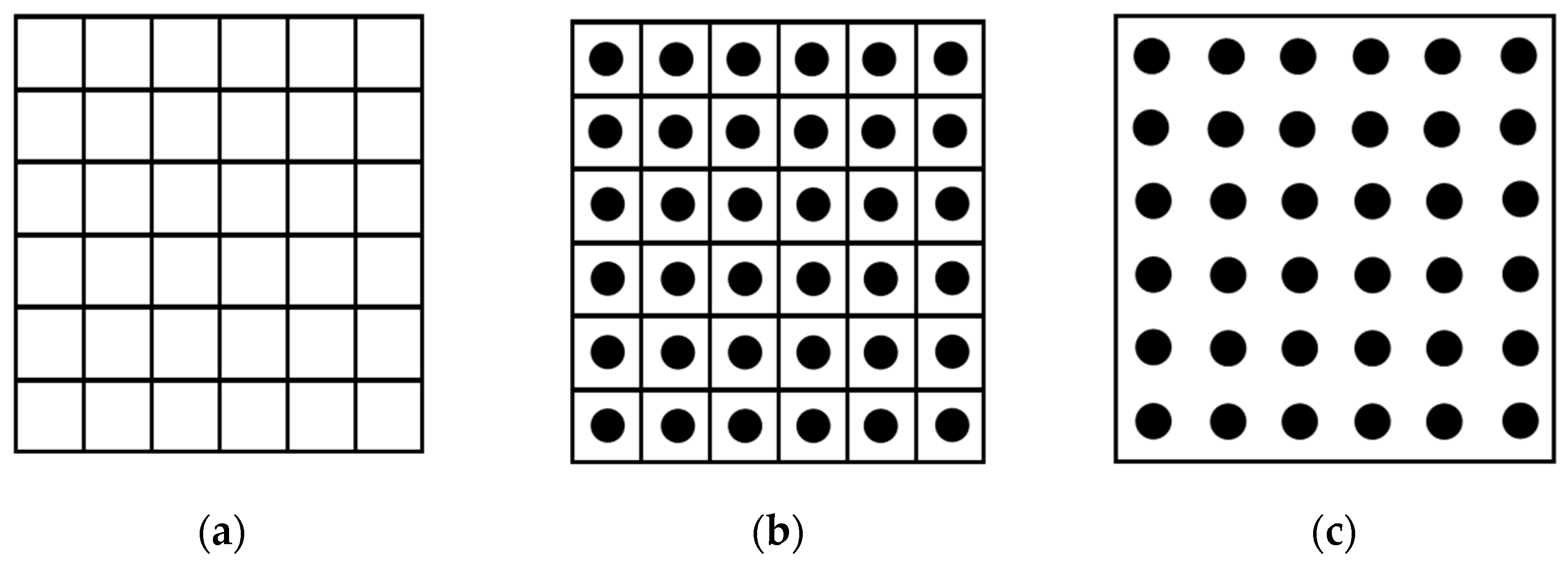
3.1. Pressure–Sinkage Test and Results
3.2. Shear Strength Test and Results
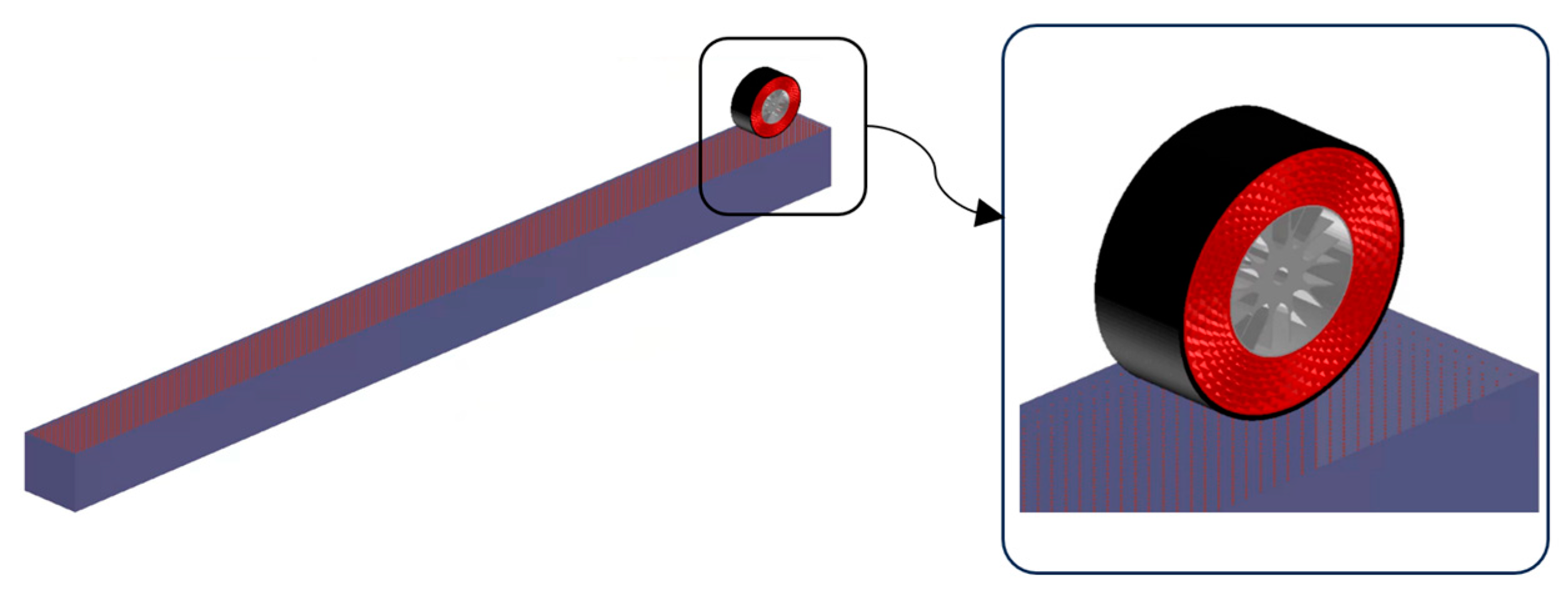
4. Non-Pneumatic Tire–Soil Interaction
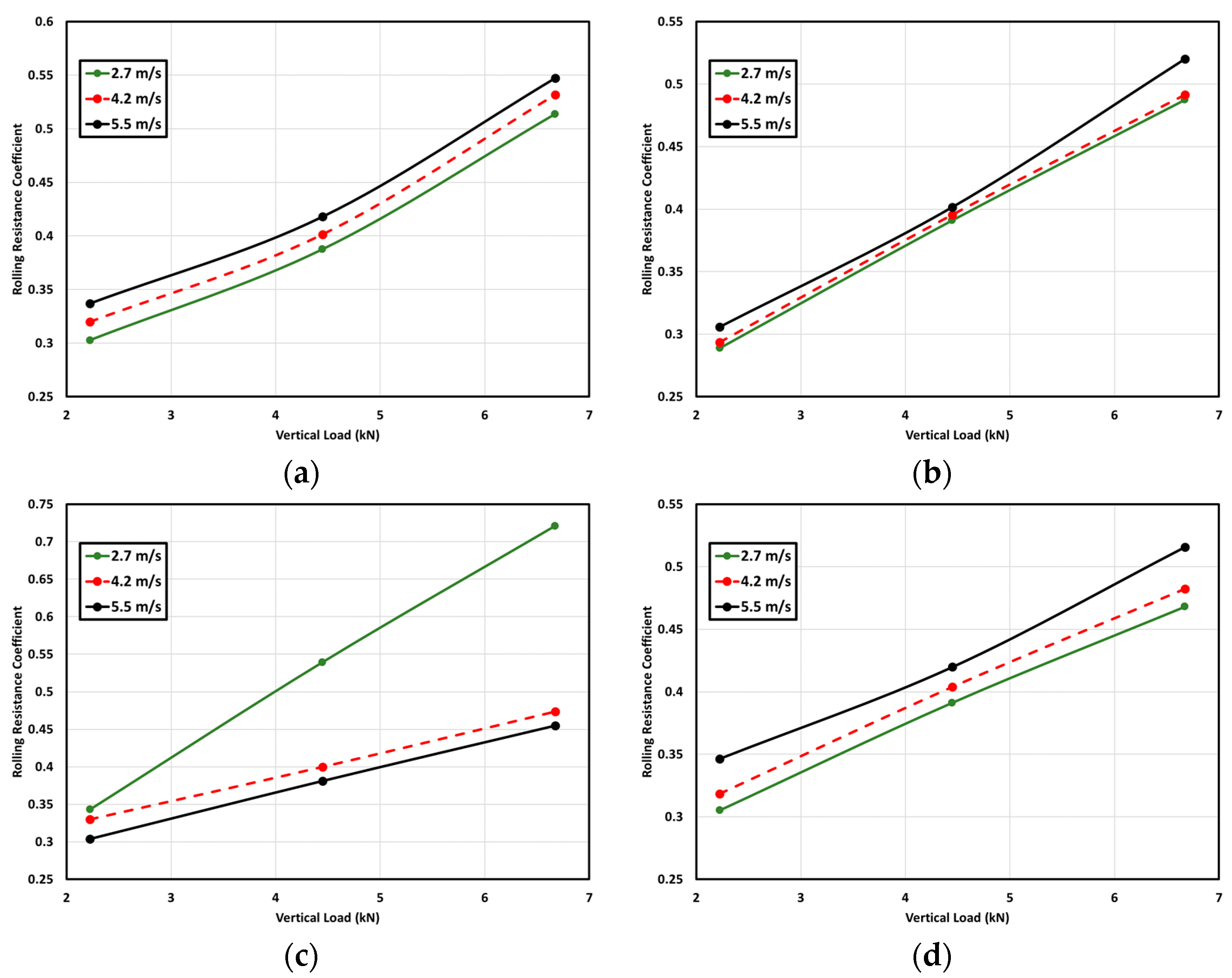
4.1. Effect of Normal Force and Velocity
4.2. Tire Penetration in Soil
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sidhu, C.; El-Sayegh, Z.; El-Gindy, M. Non-Pneumatic Tire-Road Interaction Using Finite Element Analysis. Int. J. Veh. Syst. Model. Test. 2023, 17, 52–66. [Google Scholar]
- Sidhu, C.; El-Sayegh, Z.; Ly, A. Non-pneumatic Tire-Mars Soil Interaction Using Advanced Computational Techniques. In Proceedings of the WCX SAE World Congress Experience, Detroit, MI, USA, 18–20 April 2023. [Google Scholar]
- Wong, J.Y. Theory of Ground Vehicles, 5th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2022. [Google Scholar]
- El-Sayegh, Z.; El-Gindy, M.; Johansson, I.; Öijer, F. Improved tire-soil interaction model using FEA-SPH simulation. J. Terramech. 2018, 78, 53–62. [Google Scholar] [CrossRef]
- El-Sayegh, Z.; El-Gindy, M.; Johansson, I.; Öijer, F. Off-Road Soft Terrain Modeling Using Smoothed Particle Hydrodynamics Technique. In Proceedings of the ASME 2018 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Quebec City, QC, Canada, 26–29 August 2018. Volume 3: 20th International Conference on Advanced Vehicle Technologies; 15th International Conference on Design Education, V003T01A030. [Google Scholar] [CrossRef]
- Phromjan, J.; Suvanjumrat, C. Non-pneumatic tire with curved isolated spokes for agricultural machinery in agricultural fields: Empirical and numerical study. Heliyon 2023, 9, e18984. [Google Scholar] [CrossRef] [PubMed]
- Sardinha, M.; Fátima Vaz, M.; Ramos, T.R.P.; Reis, L. Design, properties, and applications of non-pneumatic tires: A review. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2023, 237, 2277–2297. [Google Scholar] [CrossRef]
- Islam, M.R.I.; Ganesh, K.V.; Patra, P.K. On the equivalence of Eulerian Smoothed Particle Hydrodynamics, Total Lagrangian Smoothed Particle Hydrodynamics and molecular dynamics simulations for solids. Comput. Methods Appl. Mech. Eng. 2022, 391, 114591. [Google Scholar] [CrossRef]
- Andriya, N.; Dutta, V.; Vani, V.V. Study on 3D printed auxetic structure-based non-pneumatic tyres (NPT’S). Mater. Manuf. Process. 2022, 37, 1280–1297. [Google Scholar] [CrossRef]
- Liang, C.; Wei, W.; Mousavi, H.; Chen, K.; Asafo-Duho, B.; Wang, G. Investigation on static grounding analysis model of non-pneumatic tire with nonlinear spokes. AIP Adv. 2021, 11, 015303. [Google Scholar] [CrossRef]
- Sim, J.; Hong, J.; Cho, I.; Lee, J. Analysis of Vertical Stiffness Characteristics Based on Spoke Shape of Non-Pneumatic Tire. Appl. Sci. 2021, 11, 2369. [Google Scholar] [CrossRef]
- Wang, J.; Yang, B.; Lin, X.; Gao, L.; Liu, T.; Lu, Y.; Wang, R. Research of TPU Materials for 3D Printing Aiming at Non-Pneumatic Tires by FDM Method. Polymers 2020, 12, 2492. [Google Scholar] [CrossRef] [PubMed]
- Phromjan, J.; Suvanjumrat, C. The modification of steel belt layer of airless tire for finite element analysis. IOP Conf. Ser. Mater. Sci. Eng. 2020, 773, 012047. [Google Scholar] [CrossRef]
- Du, X.; Zhao, Y.; Wang, Q.; Fu, H.; Lin, F. Grounding Characteristics of a Non-Pneumatic Mechanical Elastic Tire in a Rolling State with a Camber Angle. J. Mech. Eng./Stroj. Vestn. 2019, 65, 10. [Google Scholar] [CrossRef]
- Jin, X.; Hou, C.; Fan, X.; Sun, Y.; Lv, J.; Lu, C. Investigation on the static and dynamic behaviors of non-pneumatic tires with honeycomb spokes. Compos. Struct. 2018, 187, 27–35. [Google Scholar] [CrossRef]
- Mohan, A.; Johny, C.A.; Tamilarasu, A.; Bhasker, J.P.; Ravi, K. Design and analysis of non-pneumatic tyre. IOP Conf. Ser. Mater. Sci. Eng. 2017, 263, 062061. [Google Scholar] [CrossRef]
- Sugiura, K.; Inutsuka, S.-i. An extension of Godunov SPH II: Application to elastic dynamics. J. Comput. Phys. 2017, 333, 78–103. [Google Scholar] [CrossRef]
- Marrone, S.; Di Mascio, A.; Le Touzé, D. Coupling of Smoothed Particle Hydrodynamics with Finite Volume method for free-surface flows. J. Comput. Phys. 2016, 310, 161–180. [Google Scholar] [CrossRef]
- Valizadeh, A.; Monaghan, J.J. A study of solid wall models for weakly compressible SPH. J. Comput. Phys. 2015, 300, 5–19. [Google Scholar] [CrossRef]
- Monaghan, J.J.; Kajtar, J.B. SPH particle boundary forces for arbitrary boundaries. Comput. Phys. Commun. 2009, 180, 1811–1820. [Google Scholar] [CrossRef]
- Morris, J.P.; Fox, P.J.; Zhu, Y. Modeling Low Reynolds Number Incompressible Flows Using SPH. J. Comput. Phys. 1997, 136, 214–226. [Google Scholar] [CrossRef]
- Adami, S.; Hu, X.Y.; Adams, N.A. A generalized wall boundary condition for smoothed particle hydrodynamics. J. Comput. Phys. 2012, 231, 7057–7075. [Google Scholar] [CrossRef]
- Molteni, D.; Colagrossi, A. A simple procedure to improve the pressure evaluation in hydrodynamic context using the SPH. Comput. Phys. Commun. 2009, 180, 861–872. [Google Scholar] [CrossRef]
- Antuono, M.; Colagrossi, A.; Marrone, S. Numerical diffusive terms in weakly-compressible SPH schemes. Comput. Phys. Commun. 2012, 183, 2570–2580. [Google Scholar] [CrossRef]
- Litvinov, S.; Hu, X.Y.; Adams, N.A. Towards consistence and convergence of conservative SPH approximations. J. Comput. Phys. 2015, 301, 394–401. [Google Scholar] [CrossRef]
- Adami, S.; Hu, X.Y.; Adams, N.A. A transport-velocity formulation for smoothed particle hydrodynamics. J. Comput. Phys. 2013, 241, 292–307. [Google Scholar] [CrossRef]
- Swegle, J.W.; Hicks, D.L.; Attaway, S.W. Smoothed Particle Hydrodynamics Stability Analysis. J. Comput. Phys. 1995, 116, 123–134. [Google Scholar] [CrossRef]
- Rhyne, T.B.; Cron, S.M. Development of a Non-Pneumatic Wheel. Tire Sci. Technol. 2006, 34, 150–169. [Google Scholar] [CrossRef]
- Dhillon, R.S. Development of Truck Tire-Terrain Finite Element Analysis Models. Master’s Thesis, University of Ontario Institute of Technology, Oshawa, ON, Canada, 2013. [Google Scholar]
- Bui, H.; Fukagawa, R.; Sako, K.; Ohno, S. Lagrangian mesh-free particle method (SPH) for large deformation and post-failure of geomaterial using elastic-plastic soil constitutive model. Int. J. Numer. Anal. Methods Geomech. 2008, 32, 1537–1570. [Google Scholar] [CrossRef]
- Bekker, M.G. Introduction to Terrain-Vehicle Systems. Part I: The Terrain. Part II: The Vehicle; DTIC Document; University of Michigan Press: Ann Arbor, MI, USA, 1969. [Google Scholar]







| Spoke Design | Critical Frequency (Hz) | Damping Coefficient |
|---|---|---|
| Honeycomb spokes | 25 | 1.57 |
| Modified honeycomb | 32 | 2.01 |
| Re-entrant honeycomb | 22 | 1.38 |
| Straight spoke Tweel | 48 | 3.02 |
| Material Type | (ton/mm3) | σ (MPa) | E (MPa) | K (MPa) | G (MPa) |
|---|---|---|---|---|---|
| Loose soil | 1.44 × 10−9 | 0.016 | 17 | 11 | 7 |
| Soil Type | n | kc | kθ | c | |
|---|---|---|---|---|---|
| (Units) | kPa | deg | |||
| Loose soil | 1.1 | 0.99 | 1528.43 | 1.04 | 28 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sidhu, C.; El-Sayegh, Z. Comparative Analysis of Non-Pneumatic Tire Spoke Designs for Off-Road Applications: A Smoothed Particle Hydrodynamics Perspective. Geotechnics 2024, 4, 549-563. https://doi.org/10.3390/geotechnics4020030
Sidhu C, El-Sayegh Z. Comparative Analysis of Non-Pneumatic Tire Spoke Designs for Off-Road Applications: A Smoothed Particle Hydrodynamics Perspective. Geotechnics. 2024; 4(2):549-563. https://doi.org/10.3390/geotechnics4020030
Chicago/Turabian StyleSidhu, Charanpreet, and Zeinab El-Sayegh. 2024. "Comparative Analysis of Non-Pneumatic Tire Spoke Designs for Off-Road Applications: A Smoothed Particle Hydrodynamics Perspective" Geotechnics 4, no. 2: 549-563. https://doi.org/10.3390/geotechnics4020030
APA StyleSidhu, C., & El-Sayegh, Z. (2024). Comparative Analysis of Non-Pneumatic Tire Spoke Designs for Off-Road Applications: A Smoothed Particle Hydrodynamics Perspective. Geotechnics, 4(2), 549-563. https://doi.org/10.3390/geotechnics4020030







