Prediction of Hydrogen-Bonding Interaction Free Energies with Two New Molecular Descriptors
Abstract
1. Introduction
2. The Predictive Method for HB Interaction Free Energies
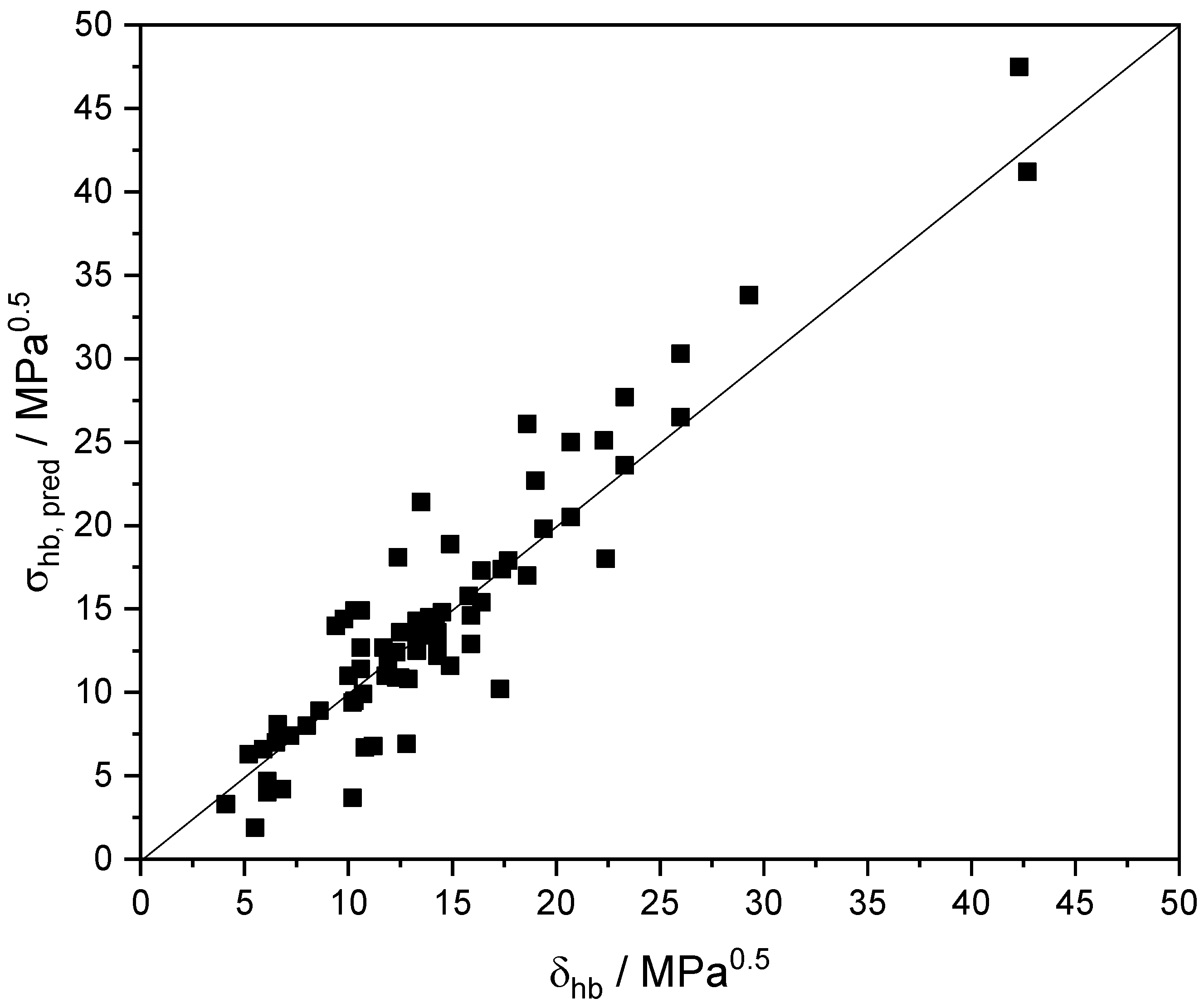
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zuburtikudis, I.; Acree, W.E.; Panayiotou, C. Prediction of hydrogen-bonding interaction energies with new COSMO-based molecular descriptors. J. Mol. Liq. 2025, 422, 126907. [Google Scholar] [CrossRef]
- Hirata, F. Exploring Life Phenomena with Statistical Mechanics of Molecular Liquids; CRC Press: Boca Raton, FL, USA, 2020. [Google Scholar]
- Joesten, M.D.; Schaad, L. Hydrogen Bonding; Marcel Dekker: New York, NY, USA, 1974. [Google Scholar]
- Gilli, G.; Gilli, P. The Nature of the Hydrogen Bond; Oxford University Press: Oxford, UK, 2009. [Google Scholar]
- Baev, A.K. Specific Intermolecular Interactions of Nitrogenated and Bioorganic Compounds; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Ben-Naim, A. Solvation Thermodynamics; Plenum Press: New York, NY, USA; Springer: Berlin/Heidelberg, Germany, 1987. [Google Scholar]
- Car, R.; Parrinello, M. Unified approach for molecular dynamics and density functional theory. Phys. Rev. Lett. 1985, 55, 2471. [Google Scholar] [CrossRef] [PubMed]
- Silvestrelli, P.L.; Parrinello, M. Structural, electronic, and bonding properties of liquid water from first principles. J. Chem. Phys. 1999, 111, 3572–3580. [Google Scholar] [CrossRef]
- Sapir, L.; Harries, D. Revisiting Hydrogen Bond Thermodynamics in Molecular Simulations. J. Chem. Theory Comput. 2017, 13, 2851–2857. [Google Scholar] [CrossRef]
- Matos, G.D.R.; Kyu, D.Y.; Loeffler, H.H.; Chodera, J.D.; Shirts, M.R.; Mobley, D.L. Approaches for Calculating Solvation Free Energies and Enthalpies Demonstrated with an Update of the FreeSolv Database. J. Chem. Eng. Data 2017, 62, 1559–1569. [Google Scholar] [CrossRef]
- Tillotson, M.J.; Diamantonis, N.I.; Buda, C.; Bolton, L.W.; Muller, E.A. Molecular modelling of the thermophysical properties of fluids: Expectations, limitations, gaps and opportunities. Phys. Chem. Chem. Phys. 2023, 25, 12607. [Google Scholar] [CrossRef]
- Vera, J.H.; Wiltzek-Vera, G.; Oliveira Fuentes, C.; Panayiotou, C. Classical and Molecular Thermodynamics of Fluid Systems; CRC Press: Boca Raton, FL, USA, 2024. [Google Scholar]
- Dolezalek, F. Zur theorie der binaren gemische und konzentrierten losungen. Z. Phys. Chem. 1908, 64, 727–747. [Google Scholar] [CrossRef]
- Katritzky, A.R.; Fara, D.C.; Yang, H.; Tamm, K.; Tamm, T.; Karelson, M. Quantitative Measures of Solvent Polarity. Chem. Rev. 2004, 104, 175–198. [Google Scholar] [CrossRef]
- Laurence, C.; Gal, J.-F.; Lewis, J.-F. Basicity and Affinity Scales: Data and Measurements; Wiley: New York, NY, USA, 2010. [Google Scholar]
- Sedov, L.A.; Solomonov, B.N. Hydrogen bonding in neat aliphatic alcohols: The Gibbs free energy of self-association and molar fraction of monomer. J. Mol. Liq. 2012, 167, 47–51. [Google Scholar] [CrossRef]
- Sinha, S.; Yang, C.; Wu, E.; Acree, W.E. Abraham Solvation Parameter Model: Examination of Possible Intramolecular Hydrogen-Bonding using calculated solute descriptors. Liquids 2022, 2, 131–146. [Google Scholar] [CrossRef]
- Moriguchi, I. Quantitative Structure-Activity Studies I. Parameters Relating to Hydrophobicity. Chem. Pharm. Bull. 1975, 23, 247–257. [Google Scholar] [CrossRef]
- Kamlet, M.J.; Abboud, J.L.M.; Taft, R.W. An Examination of Linear Solvation Energy Relationships. Proc. Phys. Org. Chem. 1981, 13, 485–630. [Google Scholar]
- Kamlet, M.J.; Doherty, R.M.; Abboud, J.-L.; Abraham, M.H.; Taft, R.W. Solubility: A new look. Chemtech 1986, 16, 566–576. [Google Scholar]
- Abraham, M.H.; Doherty, R.M.; Kamlet, M.J.; Taft, R.W. A new look at acids and bases. Chemical. Brit. 1986, 22, 551–554. [Google Scholar]
- Kamlet, M.J.; Doherty, R.M.; Abraham, M.H.; Veith, G.D.; Abraham, D.J.; Taft, R.W. Solubility Properties in Polymers and Biological Media. 8. An Analysis of the Factors that Influence Toxicities of Organic Nonelectrolytes to the Golden Orfe Fish (Leuciscus idus melanotus). Environ. Sci. Technol. 1987, 21, 149–155. [Google Scholar] [CrossRef]
- Schuurmann, G. Quantitative Structure-Property Relationships for the Polarizability, Solvatochromic Parameters and Lipophilicity. Quant. Struct. Act. Relat. 1990, 9, 326–333. [Google Scholar] [CrossRef]
- Dohnal, V. New QSPR molecular descriptors based on low-cost quantum chemistry computations using DFT/COSMO approach. J. Mol. Liq. 2024, 407, 125256. [Google Scholar] [CrossRef]
- Kontogeorgis, G.M.; Folas, G.K. Thermodynamic Models for Industrial Applications. From Classical and Advanced Mixing Rules to Association Theories; John Wiley and Sons, Ltd.: Chichester, UK, 2010. [Google Scholar]
- Wilson, L.Y.; Famini, G.R. Using Theoretical Descriptors in Quantitative Structure-Activity Relationships: Some Toxicological Indices. J. Med. Chem. 1991, 34, 1668–1674. [Google Scholar] [CrossRef]
- Dearden, J.C.; Ghafourian, T. Investigation of Calculated Hydrogen Bonding Parameters for QSAR. In QSAR and Molecular Modelling: Concepts, Computational Tools and Biological Applications; Sanz, F., Giraldo, J., Manaut, F., Eds.; Prous Science Publishers: Barcelona, Spain, 1995; pp. 117–119. [Google Scholar]
- Abraham, M.H.; McGowan, J.C. The use of characteristic volumes to measure cavity terms in reversed phase liquid chromatography. Chromatographia 1987, 23, 243–246. [Google Scholar] [CrossRef]
- Abraham, M.H. Scales of solute hydrogen-bonding: Their construction and application to physicochemical and biochemical processes. Chem. Soc. Rev. 1993, 22, 73–83. [Google Scholar] [CrossRef]
- Abraham, M.H.; Ibrahim, A.; Zissimos, A.M. Determination of sets of solute descriptors from chromatographic measurements. J. Chromatogr. A 2004, 1037, 29–47. [Google Scholar] [CrossRef] [PubMed]
- Abraham, M.H.; Smith, R.E.; Luchtefeld, R.; Boorem, A.J.; Luo, R.; Acree, W.E., Jr. Prediction of solubility of drugs and other compounds in organic solvents. J. Pharm. Sci. 2010, 99, 1500–1515. [Google Scholar] [CrossRef] [PubMed]
- Goss, K.-U. Predicting the equilibrium partitioning of organic compounds using just one linear solvation energy relationship (LSER). Fluid Phase Equilibr. 2005, 233, 19–22. [Google Scholar] [CrossRef]
- Mintz, C.; Ladlie, T.; Burton, K.; Clark, M.; Acree, W.E., Jr.; Abraham, M.H. Enthalpy of solvation correlations for gaseous solutes dissolved in alcohol solvents based on the Abraham model. QSAR Comb. Sci. 2008, 27, 627–635. [Google Scholar] [CrossRef]
- Endo, S.; Watanabe, N.; Ulrich, N.; Bronner, G.; Goss, K.-U. UFZ-LSER Database v 2.1; Helmholtz Centre for Environmental Research-UFZ: Leipzig, Germany, 2015; Available online: https://www.ufz.de/index.php?en=31698&contentonly=1&m=0&lserd_data[mvc]=Public/start (accessed on 12 November 2024).
- Panayiotou, C.; Zuburtikudis, I.; Abu Khalifeh, H. Linear Free-Energy Relationships (LFER) and Solvation Thermodynamics: The Thermodynamic Basis of LFER Linearity. Ind. Eng. Chem. Res. 2023, 62, 2989–3000. [Google Scholar] [CrossRef]
- Raevsky, O.A.; Grigor’ev, V.; Mednikova, E. QSAR H-Bonding Descriptions. In Trends in QSAR and Molecular Modelling 92; Wermuth, C.G., Ed.; ESCOM: Leiden, The Netherlands, 1993; pp. 116–119. [Google Scholar]
- Panayiotou, C. Quantum Chemical (QC) Calculations and Linear Solvation Energy Relationships (LSER): Hydrogen-Bonding Calculations with New QC-LSER Molecular Descriptors. Liquids 2024, 4, 663–688. [Google Scholar] [CrossRef]
- Klamt, A. Conductor-like Screening Model for Real Solvents: A New Approach to the Quantitative Calculation of Solvation Phenomena. J. Phys. Chem. 1995, 99, 2224–2235. [Google Scholar] [CrossRef]
- Klamt, A. COSMO-RS from Quantum Chemistry to Fluid Phase Thermodynamics and Drug Design; Elsevier: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Lin, S.T.; Sandler, S.I. A priori phase equilibrium prediction from a segment contribution solvation model. Ind. Eng. Chem. Res. 2002, 41, 899–913. [Google Scholar] [CrossRef]
- Grensemann, H.; Gmehling, J. Performance of a conductor-like screening model for real solvents model in comparison to classical group contribution methods. Ind. Eng. Chem. Res. 2005, 44, 1610–1624. [Google Scholar] [CrossRef]
- Pye, C.C.; Ziegler, T.; van Lenthe, E.; Louwen, J.N. An implementation of the conductor-like screening model of solvation within the Amsterdam density functional package, Part II. COSMO for real solvents. Can. J. Chem. 2009, 87, 790–797. [Google Scholar] [CrossRef]
- Klamt, A.; Eckert, F.; Arlt, W. COSMO-RS: An alternative to simulation for calculating thermodynamic properties of liquid mixtures. Annu. Rev. Chem. Biomol. Eng. 2010, 1, 101–122. [Google Scholar] [CrossRef] [PubMed]
- COSMObase, ver. 2019; COSMOlogic GmbH &CoKG (now, BIOVIA Dassault Systemes): Leverkusen, Germany, 2019.
- Bell, I.H.; Mickoleit, E.; Hsieh, C.-M.; Lin, S.-T.; Vrabec, J.; Breitkopf, C.; Jager, A. A Benchmark Open-Source Implementation of COSMO-SAC. J. Chem. Theory Comput. 2020, 16, 2635–2646. [Google Scholar] [CrossRef] [PubMed]
- TURBOMOLE V7.5 2020, a Development of University of Karlsruhe and Forschungszentrum Karlsruhe GmbH, 1989–2007, TURBOMOLE GmbH, Since 2007. Available online: http://www.turbomole.com (accessed on 8 April 2025).
- Available online: https://www.3ds.com/products/biovia/materials-studio (accessed on 8 April 2025).
- Available online: https://www.scm.com/product/cosmo-rs/ (accessed on 8 April 2025).
- Tsivintzelis, I.; Kontogeorgis, G.Μ.; Panayiotou, C. Dimerization of carboxylic acids: An equation of state approach. J. Phys. Chem. B 2017, 121, 2153–2163. [Google Scholar] [CrossRef] [PubMed]
- Abbott, S.; Yamamoto, H.; Hansen, C.M. Hansen Solubility Parameters in Practice, Complete with Software, Data and Examples, 3rd ed.; Version 3.1.20 Ebook. Available online: www.hansen-solubility.com (accessed on 8 April 2025).
- Mastrogeorgopoulos, S.; Hatzimanikatis, V.; Panayiotou, C. Toward a Simple Predictive Molecular Thermodynamic Model for Bulk Phases and Interfaces. Ind. Eng. Chem. Res. 2017, 56, 10900–10910. [Google Scholar] [CrossRef]
- Panayiotou, C.; Zuburtikudis, I.; Abu Khalifeh, H.; Hatzimanikatis, V. Linear Solvation—Energy Relationships (LSER) and Equation-of-State Thermodynamics: On the Extraction of Thermodynamic Information from the LSER Database. Liquids 2023, 3, 66–89. [Google Scholar] [CrossRef]
- Qiu, X.; Li, H.; Ver Steeg, G.; Godzik, A. Advances in AI for Protein Structure Prediction: Implications for Cancer Drug Discovery and Development. Biomolecules 2024, 14, 339. [Google Scholar] [CrossRef]
- Pereyaslavets, L.; Kamath, G.; Butin, O.; Illarionov, A.; Olevanov, M.; Kurnikov, I.; Sakipov, S.; Leontyev, I.; Voronina, E.; Gannon, T.; et al. Accurate determination of solvation free energies of neutral organic compounds from first principles. Nat. Commun. 2022, 13, 414. [Google Scholar] [CrossRef]
- Jumper, J.; Evans, R.; Pritzel, A.; Green, T.; Figurnov, M.; Ronneberger, O.; Tunyasuvunakool, K.; Bates, R.; Žídek, A.; Potapenko, A.; et al. Highly accurate protein structure prediction with AlphaFold. Nature 2021, 596, 583–589. [Google Scholar] [CrossRef]
- Khaliullin, R.Z.; Bell, A.T.; Head-Gordon, M. Analysis of charge transfer effects in molecular complexes based on absolutely localized molecular orbitals. J. Chem. Phys. 2008, 128, 184112. [Google Scholar] [CrossRef]
- Mao, Y.; Loipersberger, M.; Horn, P.R.; Das, A.; Demerdash, O.; Levine, D.S.; Veccham, S.P.; Head-Gordon, T.; Head-Gordon, M. From Intermolecular Interaction Energies and Observable Shifts to Component Contributions and Back Again: A Tale of Variational Energy Decomposition Analysis. Annu. Rev. Phys. Chem. 2021, 72, 641–666. [Google Scholar] [CrossRef]
- Bistoni, G.; Altun, A.; Wang, Z.; Neese, F. Local Energy Decomposition Analysis of London Dispersion Effects: From Simple Model Dimers to Complex Biomolecular Assemblies. Acc. Chem. Res. 2024, 57, 1411–1420. [Google Scholar] [CrossRef] [PubMed]
- Becke, A.D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef] [PubMed]
- Perdew, J.P. Density-functional approximation for the correlation-energy of the inhomogenous electron gas. Phys. Rev. B 1986, 33, 8822–8824. [Google Scholar] [CrossRef] [PubMed]
- Delley, B. An All-Electron Numerical Method for Solving the Local Density Functional for Polyatomic Molecules. J. Chem. Phys. 1990, 92, 508–517. [Google Scholar] [CrossRef]
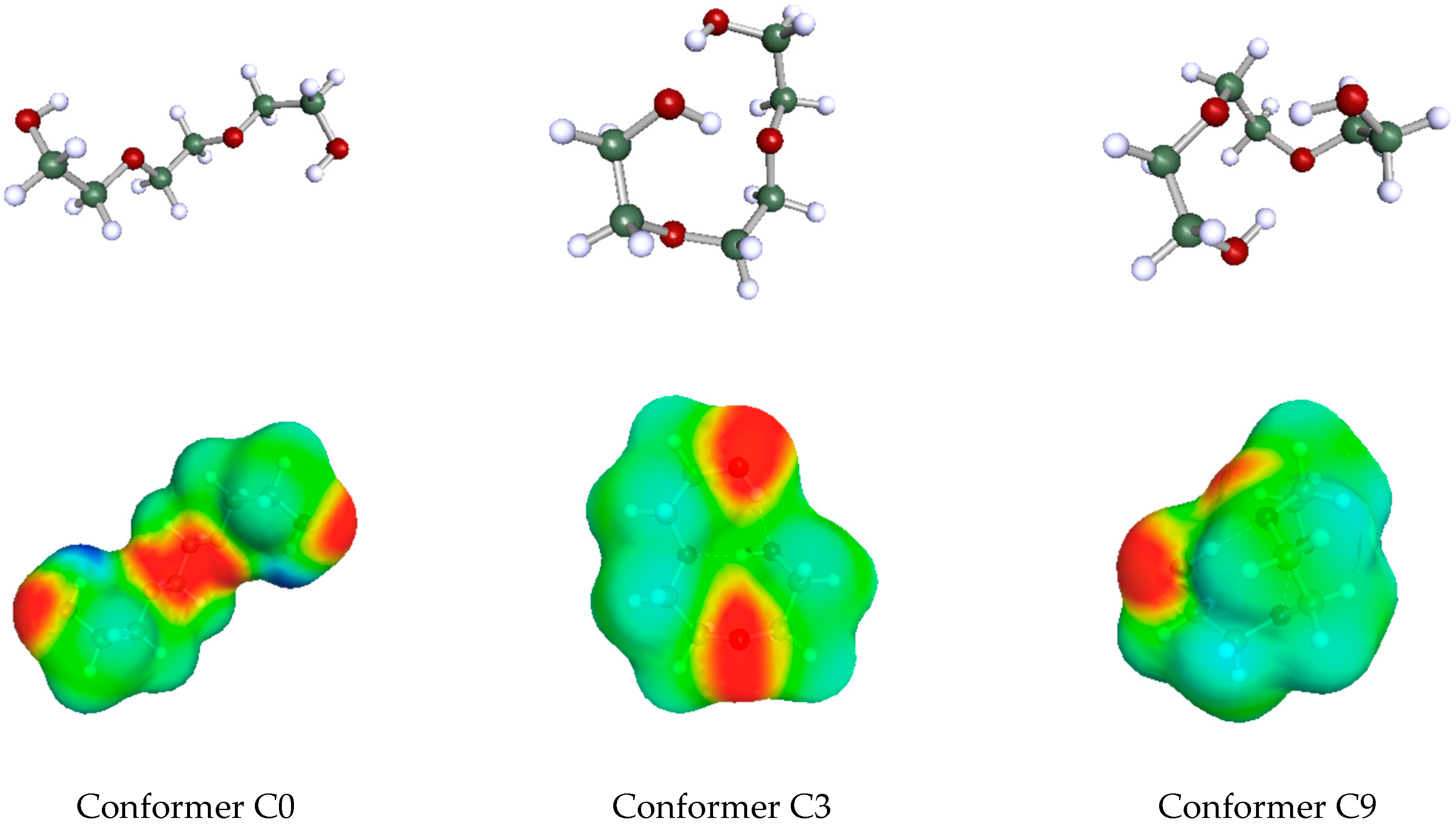

| SOLUTE | Ah | Bh | fGA | fGB | αG | βG |
|---|---|---|---|---|---|---|
| 1-PENTYNE | 0.49 | 0.16 | 0.54 | 0.85 | 0.26 | 0.14 |
| 1-HEXYNE | 0.48 | 0.17 | 0.54 | 0.85 | 0.26 | 0.14 |
| 3-HEXYNE | 0.00 | 0.34 | 0.00 | 0.85 | 0.00 | 0.29 |
| DICHLOROMETHANE | 0.94 | 0.00 | 0.37 | 0.00 | 0.35 | 0.00 |
| CHLOROFORM | 1.14 | 0.00 | 0.37 | 0.00 | 0.42 | 0.00 |
| DIETHYL ETHER | 0.00 | 1.81 | 0.00 | 0.70 | 0.00 | 1.27 |
| DI-n-PROPYL ETHER | 0.00 | 1.79 | 0.00 | 0.70 | 0.00 | 1.25 |
| DI-ISOPROPYL ETHER | 0.00 | 1.74 | 0.00 | 0.70 | 0.00 | 1.22 |
| DI-n-BUTYL ETHER | 0.00 | 1.79 | 0.00 | 0.70 | 0.00 | 1.25 |
| DI-n-PENTYL ETHER | 0.00 | 1.71 | 0.00 | 0.70 | 0.00 | 1.20 |
| ANISOLE | 0.01 | 0.58 | 0.54 | 0.85 | 0.01 | 0.49 |
| 12-CROWN-4 | 0.00 | 5.44 | 0.00 | 0.50 | 0.00 | 2.72 |
| 15-CROWN-5 | 0.00 | 7.89 | 0.00 | 0.50 | 0.00 | 3.95 |
| DIGLYME | 0.00 | 5.13 | 0.00 | 0.50 | 0.00 | 2.57 |
| TRIGLYME | 0.00 | 5.24 | 0.00 | 0.50 | 0.00 | 2.62 |
| FURAN | 0.24 | 0.13 | 0.54 | 0.70 | 0.13 | 0.09 |
| TETRAHYDROFURAN | 0.00 | 2.15 | 0.00 | 0.70 | 0.00 | 1.51 |
| 1,3-DIOXANE | 0.00 | 3.12 | 0.00 | 0.55 | 0.00 | 1.72 |
| 1,4-DIOXANE | 0.00 | 3.12 | 0.00 | 0.55 | 0.00 | 1.72 |
| TETRAHYDROPYRAN | 0.00 | 1.97 | 0.00 | 0.70 | 0.00 | 1.38 |
| METHYL FORMATE | 0.19 | 2.21 | 0.00 | 0.49 | 0.00 | 1.08 |
| ETHYL FORMATE | 0.15 | 2.36 | 0.00 | 0.49 | 0.00 | 1.16 |
| METHYL ACETATE | 0.01 | 2.67 | 0.00 | 0.49 | 0.00 | 1.31 |
| ETHYL ACETATE | 0.00 | 2.81 | 0.00 | 0.49 | 0.00 | 1.38 |
| n-PROPYL ACETATE | 0.00 | 2.80 | 0.00 | 0.49 | 0.00 | 1.37 |
| ISOPROPYL ACETATE | 0.00 | 2.68 | 0.00 | 0.49 | 0.00 | 1.31 |
| n-BUTYL ACETATE | 0.00 | 2.81 | 0.00 | 0.49 | 0.00 | 1.38 |
| ΜETHYL PROPIONATE | 0.00 | 2.55 | 0.00 | 0.49 | 0.00 | 1.25 |
| ETHYL PROPIONATE | 0.00 | 2.66 | 0.00 | 0.49 | 0.00 | 1.30 |
| ETHYL n-BUTYRATE | 0.00 | 2.65 | 0.00 | 0.49 | 0.00 | 1.30 |
| n-PROPYL PROPIONATE | 0.00 | 2.69 | 0.00 | 0.49 | 0.00 | 1.32 |
| PROPYLENE CARBONATE | 0.08 | 2.86 | 0.54 | 0.35 | 0.04 | 1.00 |
| DIETHYL CARBONATE | 0.00 | 2.78 | 0.00 | 0.37 | 0.00 | 1.03 |
| DIMETHYL CARBONATE | 0.01 | 2.46 | 0.00 | 0.40 | 0.00 | 0.98 |
| TRIBUTYL PHOSPHATE | 0.00 | 4.46 | 0.00 | 0.55 | 0.00 | 2.45 |
| ACETONE | 0.02 | 2.95 | 0.54 | 0.49 | 0.01 | 1.45 |
| METHYL ETHYL KETONE | 0.00 | 2.78 | 0.00 | 0.49 | 0.00 | 1.36 |
| 2-PENTANONE | 0.00 | 2.81 | 0.00 | 0.49 | 0.00 | 1.38 |
| 3-PENTANONE | 0.00 | 2.58 | 0.00 | 0.49 | 0.00 | 1.26 |
| CYCLOPENTANONE | 0.00 | 3.02 | 0.00 | 0.49 | 0.00 | 1.48 |
| 2-HEXANONE | 0.00 | 2.82 | 0.00 | 0.49 | 0.00 | 1.38 |
| 3-HEXANONE | 0.00 | 2.62 | 0.00 | 0.49 | 0.00 | 1.28 |
| 2-HEPTANONE | 0.00 | 2.84 | 0.00 | 0.49 | 0.00 | 1.39 |
| 2-OCTANONE | 0.00 | 2.82 | 0.00 | 0.49 | 0.00 | 1.38 |
| ACETOPHENONE | 0.01 | 2.44 | 0.00 | 0.49 | 0.00 | 1.20 |
| 2-NONANONE | 0.00 | 2.80 | 0.00 | 0.49 | 0.00 | 1.37 |
| FORMALDEHYDE | 0.00 | 1.55 | 0.00 | 0.45 | 0.00 | 0.70 |
| ACETALDEHYDE | 0.00 | 2.36 | 0.00 | 0.45 | 0.00 | 1.06 |
| PROPANAL | 0.00 | 2.33 | 0.00 | 0.45 | 0.00 | 1.05 |
| BUTANAL | 0.00 | 2.32 | 0.00 | 0.45 | 0.00 | 1.04 |
| PENTANAL | 0.00 | 2.33 | 0.00 | 0.45 | 0.00 | 1.05 |
| HEXANAL | 0.00 | 2.32 | 0.00 | 0.45 | 0.00 | 1.04 |
| OCTANAL | 0.00 | 2.33 | 0.00 | 0.45 | 0.00 | 1.05 |
| BENZALDEHYDE | 0.01 | 2.20 | 0.54 | 0.45 | 0.01 | 0.99 |
| PYRIDINE | 0.00 | 2.11 | 0.00 | 0.63 | 0.00 | 1.33 |
| 2-METHYLPYRIDINE | 0.01 | 2.12 | 0.54 | 0.63 | 0.01 | 1.34 |
| 3-METHYLPYRIDINE | 0.01 | 2.23 | 0.54 | 0.63 | 0.01 | 1.40 |
| 4-METHYLPYRIDINE | 0.01 | 2.31 | 0.54 | 0.63 | 0.01 | 1.46 |
| TRIMETHYLAMINE | 0.00 | 1.49 | 0.00 | 0.63 | 0.00 | 0.94 |
| METHANOL | 1.39 | 2.39 | 0.54 | 0.65 | 0.75 | 1.55 |
| ETHANOL | 1.23 | 2.46 | 0.54 | 0.65 | 0.66 | 1.60 |
| 1-PROPANOL | 1.23 | 2.44 | 0.54 | 0.65 | 0.66 | 1.59 |
| 1-BUTANOL | 1.22 | 2.47 | 0.54 | 0.65 | 0.66 | 1.61 |
| 1-PENTANOL | 1.22 | 2.46 | 0.54 | 0.65 | 0.66 | 1.60 |
| 1-HEXANOL | 1.22 | 2.48 | 0.54 | 0.65 | 0.66 | 1.61 |
| 1-HEPTANOL | 1.21 | 2.48 | 0.54 | 0.65 | 0.65 | 1.61 |
| 1-OCTANOL | 1.19 | 2.46 | 0.54 | 0.65 | 0.64 | 1.60 |
| 1-NONANOL | 1.21 | 2.47 | 0.54 | 0.65 | 0.65 | 1.61 |
| 1-DECANOL | 1.23 | 2.47 | 0.54 | 0.65 | 0.66 | 1.61 |
| 2-METHYL-1-PROPANOL | 1.20 | 2.22 | 0.54 | 0.65 | 0.65 | 1.44 |
| 2-METHYL-1-BUTANOL | 1.19 | 2.19 | 0.54 | 0.65 | 0.64 | 1.42 |
| 3-METHYL-1-BUTANOL | 1.20 | 2.47 | 0.54 | 0.65 | 0.65 | 1.61 |
| 2-ETHYL-1-HEXANOL | 1.18 | 2.18 | 0.54 | 0.65 | 0.64 | 1.42 |
| ISOPROPANOL | 1.18 | 2.52 | 0.54 | 0.66 | 0.64 | 1.66 |
| 2-METHYL-2-PROPANOL | 1.04 | 2.49 | 0.54 | 0.66 | 0.56 | 1.64 |
| 2-BUTANOL | 1.15 | 2.21 | 0.54 | 0.68 | 0.62 | 1.50 |
| 2-PENTANOL | 1.14 | 2.22 | 0.54 | 0.68 | 0.62 | 1.51 |
| 2-HEXANOL | 1.15 | 2.24 | 0.54 | 0.68 | 0.62 | 1.52 |
| 2-METHYL-2-BUTANOL | 1.00 | 2.24 | 0.54 | 0.68 | 0.54 | 1.52 |
| 4-METHYL-2-PENTANOL | 1.15 | 2.17 | 0.54 | 0.68 | 0.62 | 1.48 |
| CYCLOPENTANOL | 1.16 | 2.29 | 0.54 | 0.65 | 0.63 | 1.49 |
| CYCLOHEXANOL | 1.13 | 2.55 | 0.54 | 0.65 | 0.61 | 1.66 |
| 1-METHYLCYCLOHEXANOL | 0.97 | 2.07 | 0.54 | 0.65 | 0.52 | 1.35 |
| 1-ADAMANTANOL | 1.00 | 2.56 | 0.54 | 0.65 | 0.54 | 1.66 |
| BENZYL ALCOHOL | 1.23 | 2.07 | 0.54 | 0.65 | 0.66 | 1.35 |
| PHENOL | 2.03 | 0.88 | 0.54 | 0.65 | 1.10 | 0.57 |
| o-CRESOL | 1.99 | 0.77 | 0.54 | 0.65 | 1.07 | 0.50 |
| m-CRESOL | 1.98 | 0.93 | 0.54 | 0.65 | 1.07 | 0.61 |
| p-CRESOL | 1.96 | 0.96 | 0.54 | 0.65 | 1.06 | 0.63 |
| 2,3-XYLENOL | 1.88 | 0.69 | 0.54 | 0.65 | 1.02 | 0.45 |
| o-ETHYLPHENOL | 1.95 | 0.55 | 0.54 | 0.65 | 1.05 | 0.35 |
| m-ETHYLPHENOL | 1.98 | 0.93 | 0.54 | 0.65 | 1.07 | 0.60 |
| p-ETHYLPHENOL | 1.95 | 0.53 | 0.54 | 0.65 | 1.05 | 0.34 |
| 1-NAPHTHOL | 2.12 | 0.50 | 0.54 | 0.65 | 1.14 | 0.33 |
| THYMOL | 1.88 | 0.68 | 0.54 | 0.65 | 1.02 | 0.44 |
| FORMIC ACID | 2.67 | 2.10 | 0.54 | 0.50 | 1.44 | 1.05 |
| ACETIC ACID | 2.04 | 2.70 | 0.54 | 0.50 | 1.10 | 1.35 |
| PROPIONIC ACID | 1.99 | 2.62 | 0.54 | 0.50 | 1.07 | 1.31 |
| n-BUTYRIC ACID | 1.98 | 2.62 | 0.54 | 0.50 | 1.07 | 1.31 |
| n-PENTANOIC ACID | 1.97 | 2.62 | 0.54 | 0.50 | 1.06 | 1.31 |
| n-HEXANOIC ACID | 2.00 | 2.63 | 0.54 | 0.50 | 1.08 | 1.32 |
| ACRYLIC ACID | 2.17 | 2.40 | 0.54 | 0.50 | 1.17 | 1.20 |
| BENZOIC ACID | 2.09 | 2.14 | 0.54 | 0.50 | 1.13 | 1.07 |
| METHYLAMINE | 0.50 | 2.69 | 0.54 | 0.63 | 0.27 | 1.69 |
| ETHYLAMINE | 0.43 | 2.73 | 0.54 | 0.63 | 0.23 | 1.72 |
| n-PROPYLAMINE | 0.44 | 2.74 | 0.54 | 0.63 | 0.24 | 1.73 |
| ISOPROPYLAMINE | 0.41 | 2.61 | 0.54 | 0.63 | 0.22 | 1.64 |
| n-BUTYLAMINE | 0.43 | 2.73 | 0.54 | 0.63 | 0.23 | 1.72 |
| n-PENTYLAMINE | 0.43 | 2.73 | 0.54 | 0.63 | 0.23 | 1.72 |
| n-HEXYLAMINE | 0.43 | 2.75 | 0.54 | 0.63 | 0.23 | 1.73 |
| n-HEPTYLAMINE | 0.43 | 2.75 | 0.54 | 0.63 | 0.23 | 1.73 |
| n-OCTYLAMINE | 0.43 | 2.76 | 0.54 | 0.63 | 0.23 | 1.74 |
| DIMETHYLAMINE | 0.32 | 2.13 | 0.54 | 0.63 | 0.17 | 1.34 |
| DIETHYLAMINE | 0.20 | 2.02 | 0.54 | 0.63 | 0.11 | 1.27 |
| DI-n-PROPYLAMINE | 0.19 | 2.02 | 0.54 | 0.63 | 0.10 | 1.27 |
| ANILINE | 1.62 | 0.78 | 0.45 | 0.95 | 0.73 | 0.74 |
| N-METHYLANILINE | 0.87 | 0.28 | 0.45 | 0.95 | 0.39 | 0.27 |
| N-ETHYLANILINE | 0.67 | 0.31 | 0.45 | 0.95 | 0.30 | 0.29 |
| FORMAMIDE | 3.03 | 4.23 | 0.35 | 0.45 | 1.06 | 1.90 |
| ACETAMIDE | 2.61 | 4.74 | 0.35 | 0.45 | 0.91 | 2.13 |
| N-METHYL FORMAMIDE | 1.58 | 4.20 | 0.35 | 0.45 | 0.55 | 1.89 |
| DIMETHYL SULFOXIDE | 0.10 | 5.24 | 0.43 | 0.35 | 0.04 | 1.83 |
| N,N-DIMETHYLFORMAMIDE | 0.00 | 4.17 | 0.00 | 0.45 | 0.00 | 1.88 |
| NITROMETHANE | 0.74 | 1.14 | 0.43 | 0.35 | 0.32 | 0.40 |
| NITROETHANE | 0.39 | 1.21 | 0.43 | 0.35 | 0.17 | 0.42 |
| NITROBENZENE | 0.11 | 1.02 | 0.43 | 0.35 | 0.05 | 0.36 |
| ACETONITRILE | 0.31 | 2.00 | 0.43 | 0.35 | 0.13 | 0.70 |
| PROPIONITRILE | 0.08 | 2.02 | 0.43 | 0.35 | 0.04 | 0.71 |
| ACRYLONITRILE | 0.43 | 1.52 | 0.43 | 0.35 | 0.18 | 0.53 |
| BUTYRONITRILE | 0.06 | 2.04 | 0.43 | 0.35 | 0.03 | 0.71 |
| HEXANENITRILE | 0.06 | 2.06 | 0.43 | 0.35 | 0.03 | 0.72 |
| OCTANENITRILE | 0.06 | 2.06 | 0.43 | 0.35 | 0.03 | 0.72 |
| DECANITRILE | 0.06 | 2.06 | 0.43 | 0.35 | 0.03 | 0.72 |
| DODECANITRILE | 0.06 | 2.06 | 0.43 | 0.35 | 0.03 | 0.72 |
| BENZONITRILE | 0.11 | 1.58 | 0.43 | 0.35 | 0.05 | 0.55 |
| 2-NITROPHENOL | 0.43 | 1.09 | 0.54 | 0.63 | 0.23 | 0.69 |
| 4-NITROPHENOL | 2.97 | 1.80 | 0.54 | 0.63 | 1.60 | 1.13 |
| 4-CHLOROPHENOL | 2.28 | 0.72 | 0.65 | 0.65 | 1.48 | 0.47 |
| PIPERIDINE | 0.29 | 2.14 | 0.54 | 0.63 | 0.16 | 1.35 |
| PYRAZOLE | 2.19 | 2.34 | 0.54 | 0.45 | 1.18 | 1.05 |
| PYRROLIDINE | 0.28 | 2.45 | 0.54 | 0.63 | 0.15 | 1.54 |
| PYRROLE | 1.68 | 0.34 | 0.54 | 0.63 | 0.91 | 0.21 |
| INDOLE | 1.78 | 0.01 | 0.54 | 0.63 | 0.96 | 0.01 |
| 2-PYRROLIDONE | 1.26 | 4.92 | 0.35 | 0.38 | 0.44 | 1.87 |
| N-METHYLIMIDAZOLE | 0.21 | 3.42 | 0.35 | 0.45 | 0.07 | 1.54 |
| ACETOL | 0.33 | 3.37 | 0.54 | 0.65 | 0.18 | 2.19 |
| 2-METHOXYETHANOL c0 * | 0.77 | 3.13 | 0.54 | 0.70 | 0.41 | 2.19 |
| 2-METHOXYETHANOL c7 | 1.39 | 3.19 | 0.55 | 0.73 | 0.76 | 2.33 |
| 2-ETHOXYETHANOL solvent/solute | 0.63 | 3.13 | 0.54 | 0.70 | 0.34 | 2.19 |
| 2-ETHOXYETHANOL self-solv | 1.35 | 4.23 | 0.33 | 0.44 | 0.44 | 1.86 |
| 2-ETHOXYETHANOL c9 | 1.39 | 3.28 | 0.55 | 0.73 | 0.76 | 2.39 |
| 2-METHOXY PROPANOL-1 | 0.52 | 3.19 | 0.54 | 0.70 | 0.28 | 2.23 |
| 2-BUTOXYETHANOL | 0.67 | 3.11 | 0.54 | 0.70 | 0.36 | 2.18 |
| 2-METHOXYPHENOL C0 | 0.90 | 0.91 | 0.54 | 0.70 | 0.49 | 0.64 |
| 2-METHOXYPHENOL C1 | 2.07 | 1.96 | 0.54 | 0.70 | 1.12 | 1.37 |
| 4-METHOXYPHENOL C0 | 1.95 | 1.70 | 0.54 | 0.70 | 1.05 | 1.19 |
| ETHYLENE GLYCOL solvent | 2.13 | 3.87 | 0.54 | 0.50 | 1.15 | 1.94 |
| ETHYLENE GLYCOL solute | 2.13 | 3.87 | 0.54 | 0.65 | 1.15 | 2.52 |
| ETHYLENE GLYCOL c3 | 2.83 | 4.89 | 0.54 | 0.65 | 1.53 | 3.18 |
| ETHYLENE GLYCOL c6 | 2.97 | 4.11 | 0.54 | 0.65 | 1.60 | 2.67 |
| 1,2-PROPYLENE GLYCOL solvent | 1.95 | 3.82 | 0.47 | 0.55 | 0.92 | 2.10 |
| 1,2-PROPYLENE GLYCOL solute | 1.95 | 3.82 | 0.54 | 0.65 | 1.06 | 2.48 |
| DIETHYLENE GLYCOLsolute | 1.47 | 4.55 | 0.54 | 0.65 | 0.79 | 2.96 |
| DIETHYLENE GLYCOLsolvent | 1.47 | 4.55 | 0.49 | 0.49 | 0.72 | 2.23 |
| DIPROPYLENE GLYCOL solute | 1.01 | 4.67 | 0.65 | 0.75 | 0.66 | 3.51 |
| TRIETHYLENE GLYCOL solute | 1.41 | 6.20 | 0.49 | 0.49 | 0.69 | 3.04 |
| TRIETHYLENE GLYCOL solvent | 1.41 | 6.20 | 0.30 | 0.27 | 0.42 | 1.68 |
| 1,2-BUTANEDIOL | 1.73 | 3.66 | 0.54 | 0.65 | 0.93 | 2.38 |
| 1,3-BUTANEDIOL | 1.60 | 3.96 | 0.54 | 0.65 | 0.86 | 2.57 |
| 1,4-BUTANEDIOL | 1.63 | 3.98 | 0.54 | 0.65 | 0.88 | 2.59 |
| 1,3-DIHYDROXYBENZENE | 4.03 | 1.73 | 0.54 | 0.75 | 2.18 | 1.30 |
| GLYCEROL | 2.98 | 5.26 | 0.54 | 0.40 | 1.61 | 2.10 |
| HYDROGEN PEROXIDE | 3.81 | 2.09 | 0.50 | 0.45 | 1.91 | 0.94 |
| WATER | 2.89 | 2.99 | 0.54 | 0.54 | 1.56 | 1.61 |
| Conformer | Ah | Ap | Bp | Bh | fGA | fGB | αG | βG |
|---|---|---|---|---|---|---|---|---|
| C0 | 1.41 | 2.32 | 1.00 | 6.20 | 0.49 | 0.49 | 0.69 | 3.04 |
| C1 | 1.30 | 2.30 | 1.00 | 6.01 | 0.53 | 0.51 | 0.69 | 3.04 |
| C2 | 1.34 | 2.30 | 1.02 | 6.02 | 0.52 | 0.50 | 0.69 | 3.04 |
| C3 | 0.00 | 2.47 | 0.95 | 5.05 | 0.60 | 0.69 | 3.04 | |
| C4 | 2.15 | 2.28 | 0.91 | 7.22 | 0.32 | 0.42 | 0.69 | 3.04 |
| C5 | 0.00 | 2.56 | 0.96 | 5.10 | 0.60 | 0.69 | 3.04 | |
| C6 | 0.77 | 2.50 | 0.95 | 5.84 | 0.90 | 0.52 | 0.69 | 3.04 |
| C7 | 0.59 | 2.07 | 0.95 | 4.96 | 1.17 | 0.61 | 0.69 | 3.04 |
| C8 | 0.00 | 2.51 | 0.90 | 5.21 | 0.58 | 0.69 | 3.04 | |
| C9 | 0.00 | 2.67 | 0.90 | 5.37 | 0.57 | 0.69 | 3.04 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Acree, W.E., Jr.; Panayiotou, C. Prediction of Hydrogen-Bonding Interaction Free Energies with Two New Molecular Descriptors. Liquids 2025, 5, 12. https://doi.org/10.3390/liquids5020012
Acree WE Jr., Panayiotou C. Prediction of Hydrogen-Bonding Interaction Free Energies with Two New Molecular Descriptors. Liquids. 2025; 5(2):12. https://doi.org/10.3390/liquids5020012
Chicago/Turabian StyleAcree, William E., Jr., and Costas Panayiotou. 2025. "Prediction of Hydrogen-Bonding Interaction Free Energies with Two New Molecular Descriptors" Liquids 5, no. 2: 12. https://doi.org/10.3390/liquids5020012
APA StyleAcree, W. E., Jr., & Panayiotou, C. (2025). Prediction of Hydrogen-Bonding Interaction Free Energies with Two New Molecular Descriptors. Liquids, 5(2), 12. https://doi.org/10.3390/liquids5020012








