Digital Impedance Bridge for Four-Terminal-Pair AC Resistor Calibration up to 20 kHz
Abstract
1. Introduction
- The range of resistors to be calibrated. In the first paper [18], the resistors range from 400 Ω to 2 MΩ. In the present paper, the calibration system is developed for low resistance values of between 100 mΩ and 400 Ω.
- The type of resistors. In the first paper [18], the resistors are defined in a 2TP configuration. In this paper, the measured resistors are defined in a 4TP configuration, which requires triaxial connections to limit the internal leakage (internal guard).
- Although the power source and voltage injection system are the same as in the first paper [18], the calibration system presented in this paper has been improved to measure low resistance values. In the new calibration bench, two developed high-impedance stages and standard capacitors have been used to ensure bridge balance for resistors defined in a 4TP configuration. Consequently, the calibration equations to define the frequency variation of the calibrated resistors are different from those in the first paper [18].
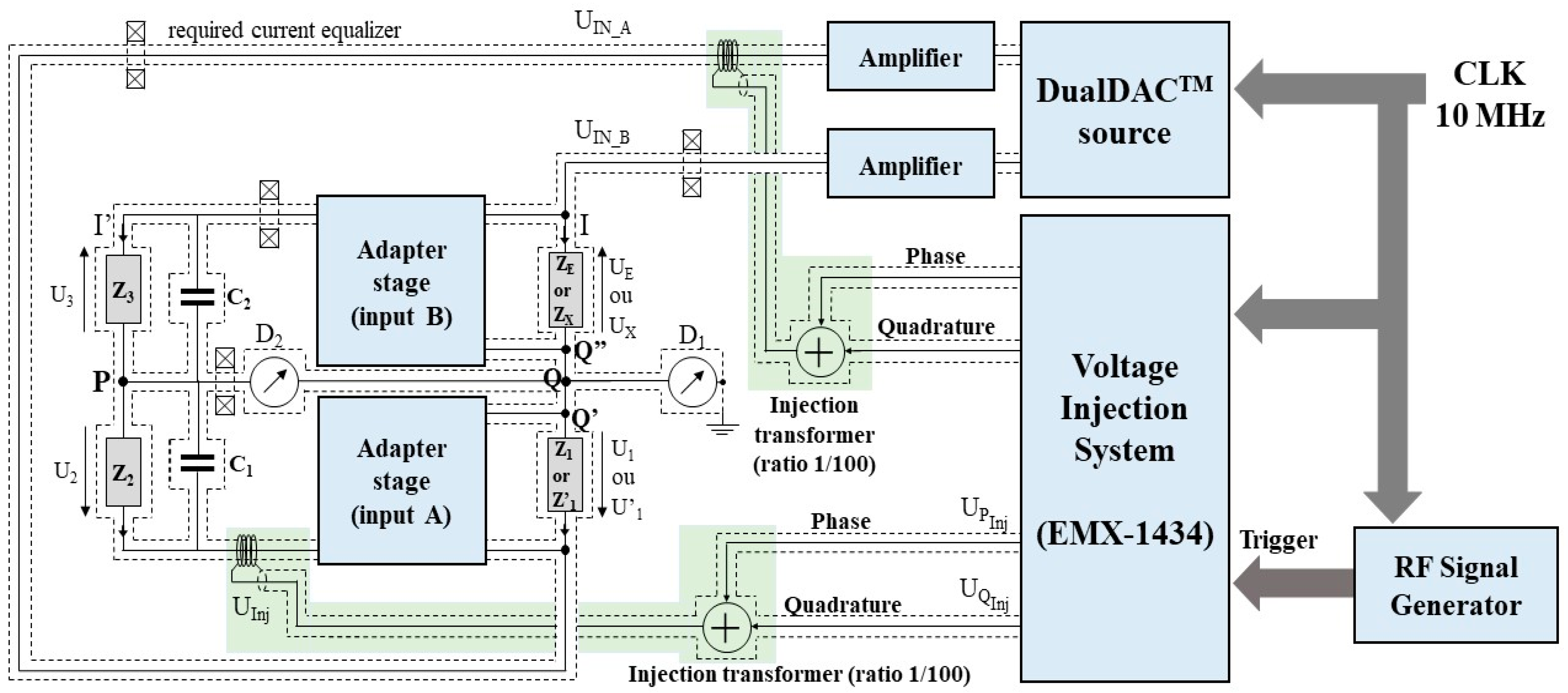
2. Description of the Impedance Bridge
- To ensure a 4TP configuration for impedances ZE (or ZX) and Z1 (or Z’1) and a 2TP configuration for impedances Z2 and Z3;
- To eliminate the influence of parasitic impedances between the P and Q nodes and the ground;
- To eliminate the influence of the common mode on the main null detector, D2.
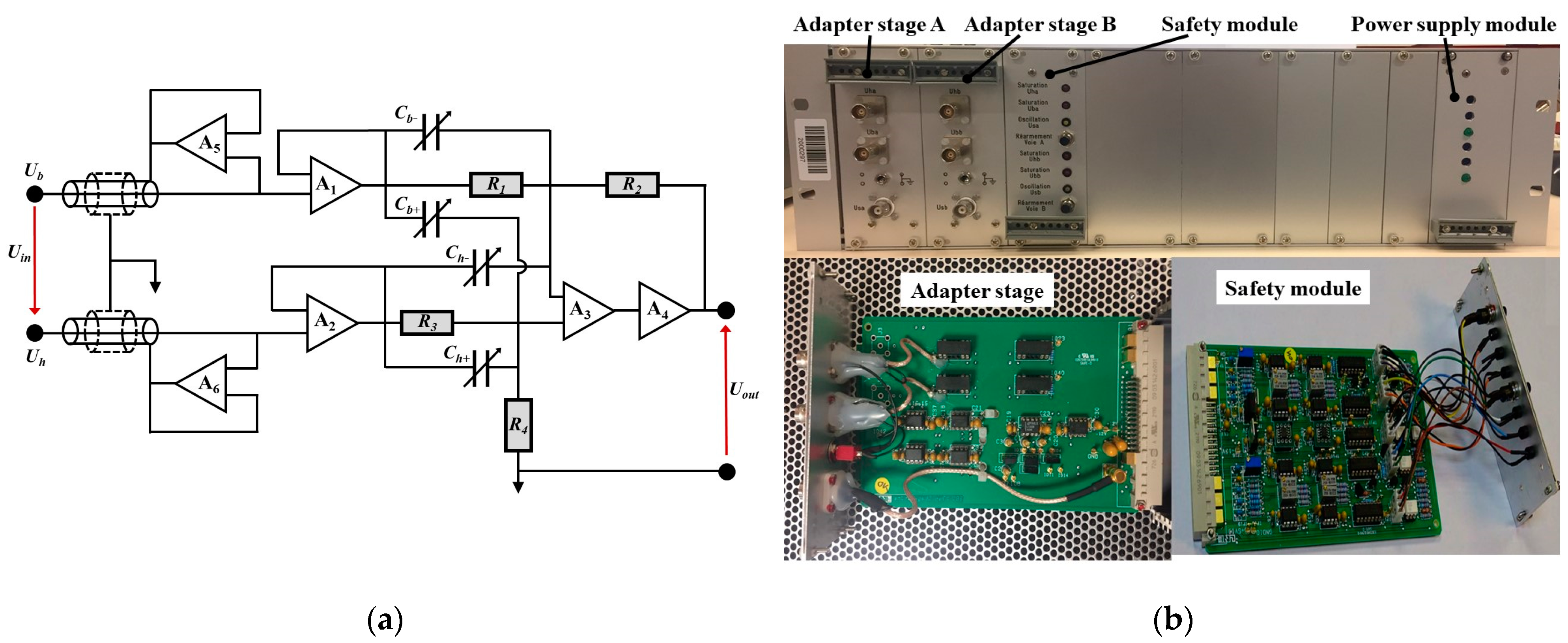
- Two adapter stages A and B, representing the main module of the high-impedance stages.
- A safety module to protect and warn against voltage limits or saturation levels for the input module amplifiers (3 V).
- Power supply module to supply power to the high-impedance stages (±12 V).
- Follower configuration: voltage is applied to the high input Uh and grounded low input Ub.
- Inverter configuration: voltage is applied to the low input Ub and grounded to the high input Uh.
- Differential configuration: the same voltage is applied to the low input Ub and high input Uh.
- Construction of the impedance bridge with impedances Z1, Z2, Z3, and ZE;
- Generation of the supply voltages UIN_A and UIN_B by the DualDAC™ source, based on the bridge ratio and the maximum input voltage of the adapter stages (3 V);
- Correction of phase errors between the DualDAC™ source and the EMX-1434 module;
- Adjustment by successive iterations of balances on detectors D1 and D2, using the voltage injection system and the capacitor C1 (or C2);
- Repetition of steps 2 to 4 for each measurement frequency;
- Substitution of Z1 and ZE by Z′1 and ZX, respectively (an electrical circuit with impedances Z′1, Z2, Z3 and ZX);
- Repetition of steps 2 to 5.
3. AC Resistor Calibration
4. Uncertainty Budget
- Influence of adapter stages (BL1): This error relates to the calibration and adjustment of the differential inputs of the adapter stages. The errors on variation Δs are corrected. The associated uncertainty (k = 1) can be increased by a component equal to 1 µV/V up to 20 kHz. For phase shift measurements, considering a rectangular distribution law, the estimated uncertainty (k = 1) is less than 4.1 µrad up to 20 kHz.
- Influence of parasitic impedances (BL2): The leakage impedances to the ground are eliminated by the Wagner arm. The input impedance of the adapters is likely to interfere with the measurements. This component is given according to the resistor values:
- ▪
- For the relative variation, Δs: The values that were estimated for impedance Zp during the calibration of the adapter stages were used to apply a correction term. The uncertainty (k = 1) associated with the internal resistance value Rp of the impedance Zp of the adapter stages can be considered to be less than 1 GΩ. The correction term β∆s_Z, related to the influence of the parasitic impedance Zp on the variation Δs, is equal to:
- ▪
- For the phase shift ωt, the uncertainty (k = 1) associated with the capacitance value, Cp, is less than 1 fF; thus, the applied correction term is equal to (Rx − R′1).Cp.2πfs. The uncertainty component BL2 is given by:
- Mutual induction effect (BL3): This component corresponds to the error due to mutual parasitic coupling between a current circuit and a voltage circuit. It is equal to:
5. AC Resistance Standards Measurement Chain
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Callegaro, L. Traceable Measurements of Electrical Impedance. IEEE Instrum. Meas. Mag. 2015, 18, 42–46. [Google Scholar] [CrossRef]
- Sindjui, R. Réalisation et Caractérisation de Dispositifs de Mesure Associés à la Détermination de la Constante de von Klitzing à Partir d’un Condensateur Calculable Etalon dit de Thompson-Lampard. Ph.D. Thesis, Université Paris Saclay (COmUE), Paris, France, 2016. [Google Scholar]
- Budovsky, I. Measurement of Phase Angle Errors of Precision Current Shunts in the Frequency Range From 40 Hz to 200 kHz. IEEE Trans. Instrum. Meas. 2007, 56, 284–288. [Google Scholar] [CrossRef]
- Overney, F.; Flowers-Jacobs, N.E.; Jeanneret, B.; Rüfenacht, A.; Fox, A.E.; Dresselhaus, P.D.; Benz, S.P. Dual Josephson Impedance Bridge: Towards a Universal Bridge for Impedance Metrology. Metrologia 2020, 57, 065014. [Google Scholar] [CrossRef] [PubMed]
- Kucera, J.; Sedlacek, R.; Bohacek, J. An HF Coaxial Bridge for Measuring Impedance Ratios up to 1 MHz. Meas. Sci. Technol. 2012, 23, 085004. [Google Scholar] [CrossRef]
- Rybski, R.; Kaczmarek, J.; Koziol, M. A PXI-Based Calibration System for Low-Value AC Resistors. IEEE Trans. Instrum. Meas. 2018, 67, 905–911. [Google Scholar] [CrossRef]
- Rybski, R.; Kaczmarek, J.; Koziol, M. Characterization of a digitizer for a low value resistor calibration system in the audio-frequency band. In Proceedings of the 2012 Conference on Precision Electromagnetic Measurements, Washington, DC, USA, 1–6 July 2012; pp. 580–581. [Google Scholar]
- Zhu, X.; Zhang, L.; Guo, Y.; Wei, P.; Zai, R.; Drewniak, J.; Pommerenke, D. I-V Method Based PDN Impedance Measurement Technique and Associated Probe Design. In Proceedings of the 2019 IEEE International Symposium on Electromagnetic Compatibility, Signal & Power Integrity (EMC+SIPI), New Orleans, LA, USA, 22–26 July 2019; pp. 30–33. [Google Scholar]
- Overney, F.; Jeanneret, B. Calibration of an $LCR$ -Meter at Arbitrary Phase Angles Using a Fully Automated Impedance Simulator. IEEE Trans. Instrum. Meas. 2017, 66, 1516–1523. [Google Scholar] [CrossRef]
- Istrate, D.; Blanc, I.; Fortuné, D. Development of a Measurement Setup for High Impulse Currents. IEEE Trans. Instrum. Meas. 2013, 62, 1473–1478. [Google Scholar] [CrossRef]
- Sordi, F.; Capineri, L.; Carobbi, C. Lumped Circuit Model and VNA Measurement of the RF Impedance of a Bypass Network. In Proceedings of the 2021 IEEE International Joint EMC/SI/PI and EMC Europe Symposium, Raleigh, NC, USA, 26 July–13 August 2021; pp. 196–201. [Google Scholar]
- Callegaro, L. Electrical Impedance: Principles, Measurement, and Applications, 1st ed.; CRC Press: Boca Raton, FL, USA, 2012. [Google Scholar]
- Hague, B. Alternating Current Bridge Methods for the Measurement of Inductance Capacitance. and Effective Resistance at Low and Telephonic Frequencies: A Theoretical and Practical Handbook for the Use of Advanced Students. Nature 1930, 113, 530. [Google Scholar]
- Christensen, A.E. A versatile electrical impedance calibration laboratory based on a digital impedance bridge. In Proceedings of the 19th International Congress of Metrology (CIM2019), Porte de Versailles, France, 24–26 September 2019. [Google Scholar]
- Sindjui, R. Realization and Characterization of Measuring Devices Associated with the Determination of the von Klitzing Constant from a Calculable Standard Capacitor Called Thompson-Lampard. Ph.D. Thesis, Université Paris-Saclay, Paris, France, 2017. [Google Scholar]
- Kibble, B.P. Four Terminal-Pair to Anything Else! In Proceedings of the IEE Colloquium on Interconnections from DC to Microwaves (Ref. No. 1999/019), London, UK, 18 February 1999. [Google Scholar]
- Callegaro, L. Four Terminal-Pair Impedance Comparisons at High Frequency. In Proceedings of the 21st IEEE Instrumentation and Measurement Technology Conference (IEEE Cat. No.04CH37510), Como, Italy, 18–20 May 2004; Volume 1, pp. 383–386. [Google Scholar]
- Ouameur, M.; Vasconcellos, R.; Agazar, M.; Thévenot, O.; Patois, E. New Impedance Bridge at LNE for the Two Terminal-Pair AC Resistor Calibration up to 20 kHz. Metrologia 2022, 59, 055005. [Google Scholar] [CrossRef]
- Consejo, C.; Thevenot, O.; Lahousse, L.; Piquemal, F.; David, J.M. Improvements of the Measurement Chain for the Determination of the von Klitzing Constant K. IEEE Trans. Instrum. Meas. 2009, 58, 902–907. [Google Scholar] [CrossRef]
- Delmas, J.; Knosp, R. Etalonnage de Résistances et de Shunts En Courant Alternatif; LNE internal project n° 81.2.46.0007 study n° 697311, report n° 941. 1983. [Google Scholar]
- Agazar, M.; Ouameur, M. Compensated High Input Impedance Stage for the Measurements of Four Terminals Resistors up to 20 kHz. In Proceedings of the 2020 Conference on Precision Electromagnetic Measurements (CPEM), Denver, CO, USA, 24–28 August 2020; pp. 1–2. [Google Scholar]
- Information Published on the Manufacturer’s Website about the DualDAC Source. Available online: https://aivon.fi/wordpress/dualdac-3/ (accessed on 5 October 2023).
- Ouameur, M.; Vasconcellos, R.; Agazar, M. A Double Precision Arbitrary Waveform Generator Based Calibration System for Low-Value AC Resistors up to 20 kHz. In Proceedings of the 2021 IEEE 12th Annual Information Technology, Electronics and Mobile Communication Conference (IEMCON), Vancouver, BC, Canada, 27–30 October 2021; pp. 0796–0798. [Google Scholar]
- EMX-1434. Smart PXI Express 4 Channel 204.8 kSa/s Arbitrary Waveform Generator. Available online: https://www.vtiinstruments.com/products/functional-test/emx-series/emx-1434 (accessed on 5 October 2023).
- Ouameur, M.; Vasconcellos, R.; Agazar, M. A PXI Modules-Based Voltage Injection System for AC Resistors Calibration. In Proceedings of the 2021 IEEE 7th International Conference on Smart Instrumentation, Measurement and Applications (ICSIMA), Bandung, Indonesia, 23–25 August 2021; pp. 257–260. [Google Scholar]
- Sindjui, R.; Gournay, P.; Thevenot, O.; Thuillier, G. Fabrication of a standard two-stage autotransformer at LNE. In Proceedings of the 29th Conference on Precision Electromagnetic Measurements (CPEM 2014), Rio de Janeiro, Brazil, 24–29 August 2014. [Google Scholar]
- Schurr, J.; Melcher, J. Unequalized currents in coaxial AC bridges. IEEE Trans. Instrum. Meas. 2004, 53, 807–811. [Google Scholar] [CrossRef]
- Thevenot, O. Calibration of Standard Transformers. Master’s Thesis, Conservatoire National des Arts et Métiers (CNAM), Paris, France, 2003. [Google Scholar]
- Manceau, J. Characterization of Standard Resistors in Sinusoidal Alternating Current; LNE internal project n° 81.2.46.0007 study n° 2.03, report n° PQ/96-EM-8; 2010.
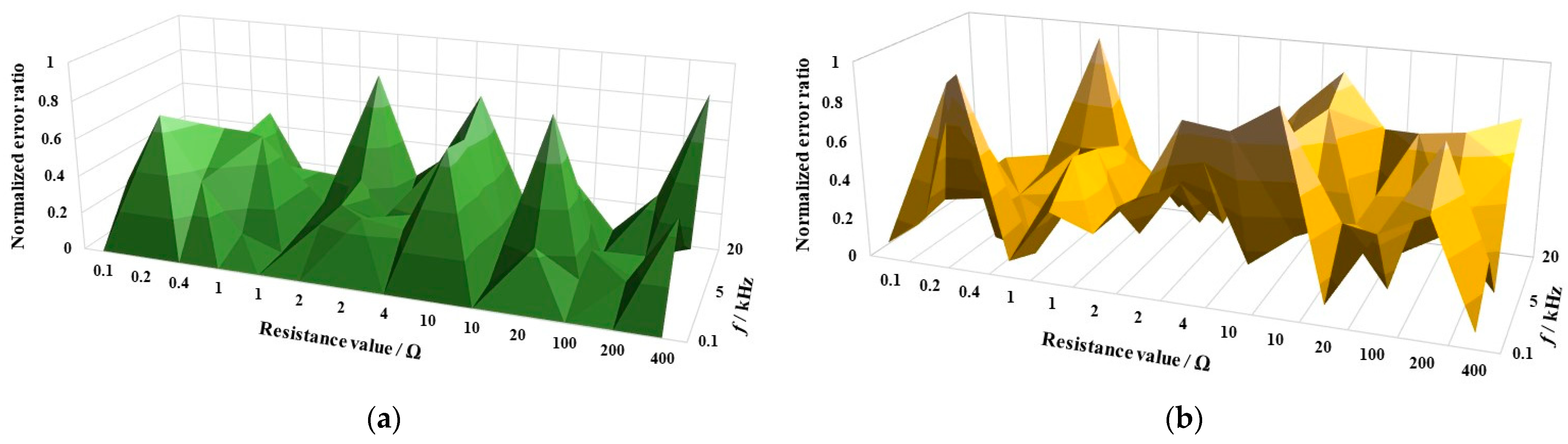
- Lee, Y.S. Applying Normalized Error Ratio in the Vector Measurement Quantities. In Proceedings of the 74th ARFTG Microwave Measurement Conference, Broomfield, CO, USA, 30 November–4 December 2009; pp. 1–6. [Google Scholar]


| Frequency (kHz) | Adapter Stage A | Adapter Stage A | ||
|---|---|---|---|---|
| Capacitance Cp (fF) | Resistance Rp (GΩ) | Capacitance Cp (fF) | Resistance Rp (GΩ) | |
| 0.1 | 95.1 ± 0.5 | 3011.4 ± 0.9 | 95.1 ± 0.5 | 2589.2 ± 0.9 |
| 0.2 | 95.0 ± 0.4 | 2055.2 ± 0.9 | 95.0 ± 0.4 | 2375.3 ± 0.9 |
| 0.5 | 95.1 ± 0.4 | 1971.3 ± 0.9 | 95.0 ± 0.4 | 2347.6 ± 0.9 |
| 1 | 95.2 ± 0.2 | 922.9 ± 0.9 | 95.2 ± 0.2 | 753.1 ± 0.9 |
| 2 | 95.1 ± 0.4 | 235.3 ± 0.9 | 95.1 ± 0.4 | 217.6 ± 0.9 |
| 5 | 95.0 ± 0.8 | 43.1 ± 0.9 | 95.0 ± 0.8 | 41.9 ± 0.9 |
| 10 | 95.1 ± 1.6 | 11.5 ± 0.9 | 95.0 ± 1.6 | 11.5 ± 0.9 |
| 20 | 95.2 ± 1.6 | 3.1 ± 0.9 | 95.2 ± 1.6 | 3.1 ± 0.9 |
| Resistance Defined in 4TP Configuration | Frequency (kHz) | Estimated Uncertainty (k = 1) of the Different Uncertainty Components of the Wheatstone Bridge Due to: | Estimated Uncertainty (k = 2) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Impedances ZE, Z1 et Z’1 | Complex Transfer Coefficients kinj and k’inj | Influence of Temperature on the Stability of the Bridge (UB1) | Accuracy Error Associated with the Adapter Stages (BL1) | Influence of Parasitic Impedances (BL2) | Mutual Induction Effect (BL3) | |||||||||
| Δs (µΩ/Ω) | ωt (µrad) | Δs (µΩ/Ω) | ωt (µrad) | Δs (µΩ/Ω) | ωt (µrad) | Δs (µΩ/Ω) | ωt (µrad) | Δs (µΩ/Ω) | ωt (µrad) | ωt (µrad) | Δs (µΩ/Ω) | ωt (µrad) | ||
| SC1 (1 Ω) | 0.1 | 3.6 | 3.6 | 0.6 | 0.6 | 2.4 | 2.4 | 1.0 | 1.0 | 0.6 | 0.1 | 0.6 | 9.1 | 9.2 |
| 1 | 5.0 | 5.1 | 0.6 | 0.6 | 2.4 | 2.4 | 1.0 | 1.0 | 0.6 | 1.4 | 0.6 | 11.5 | 11.9 | |
| 5 | 11.6 | 11.6 | 0.6 | 0.6 | 2.4 | 2.4 | 1.0 | 1.4 | 0.6 | 7.2 | 3.0 | 23.9 | 28.6 | |
| 10 | 19.9 | 20.0 | 0.6 | 0.6 | 2.4 | 2.4 | 1.0 | 2.2 | 0.6 | 14.4 | 6.0 | 40.2 | 51.2 | |
| 20 | 34.9 | 19.2 | 0.8 | 0.8 | 2.4 | 2.4 | 1.0 | 4.1 | 0.6 | 28.8 | 12.0 | 70.1 | 73.9 | |
| SC0.1 (100 mΩ) | 0.1 | 5.5 | 6.0 | 0.4 | 0.4 | 2.8 | 2.8 | 1.0 | 1.0 | 0.0 | 0.0 | 3.2 | 12.5 | 14.8 |
| 1 | 7.2 | 13.0 | 0.3 | 0.3 | 2.8 | 2.8 | 1.0 | 1.0 | 0.0 | 0.0 | 3.2 | 15.6 | 27.4 | |
| 5 | 15.2 | 46.6 | 0.3 | 0.3 | 2.8 | 2.8 | 1.0 | 1.4 | 0.0 | 0.1 | 15.8 | 31.1 | 98.5 | |
| 10 | 25.6 | 88.7 | 0.3 | 0.3 | 2.8 | 2.8 | 1.0 | 2.2 | 0.0 | 0.2 | 31.6 | 51.5 | 188.5 | |
| 20 | 45.3 | 170.5 | 0.5 | 0.5 | 2.8 | 2.8 | 1.0 | 4.1 | 0.0 | 0.3 | 63.2 | 90.8 | 363.9 | |
| Frequency (kHz) | Parameters | Year of Calibration | ||
|---|---|---|---|---|
| 1983 | 2009 | 2022 | ||
| 0.1 | Variation Δs (µΩ/Ω) | 0 ± 11 | 0 ± 11 | 0 ± 13 |
| Phase shift ωt (mrad) | 0.23 ± 0.01 | 0.23 ± 0.01 | 0.23 ± 0.01 | |
| 1 | Variation Δs (µΩ/Ω) | 26 ± 15 | 28 ± 15 | 32 ± 16 |
| Phase shift ωt (mrad) | 2.32 ± 0.03 | 2.32 ± 0.03 | 2.32 ± 0.03 | |
| 5 | Variation Δs (µΩ/Ω) | 260 ± 33 | 260 ± 33 | 261 ± 31 |
| Phase shift ωt (mrad) | 11.3 ± 0.1 | 11.3 ± 0.1 | 11.3 ± 0.1 | |
| 10 | Variation Δs (µΩ/Ω) | 470 ± 56 | 459 ± 56 | 461 ± 52 |
| Phase shift ωt (mrad) | 22.3 ± 0.3 | 22.3 ± 0.3 | 22.3 ± 0.2 | |
| 20 | Variation Δs (µΩ/Ω) | 840 ± 100 | 813 ± 100 | 812 ± 91 |
| Phase shift ωt (mrad) | 44.3 ± 0.5 | 44.3 ± 0.5 | 44.3 ± 0.4 | |
| Frequency (kHz) | Parameters | Year of Calibration | ||
|---|---|---|---|---|
| 1983 | 2009 | 2022 | ||
| 0.1 | Variation Δs (µΩ/Ω) | 0 ± 10 | 0 ± 10 | 0 ± 10 |
| Phase shift ωt (mrad) | 0.19 ± 0.01 | 0.19 ± 0.01 | 0.18 ± 0.01 | |
| 1 | Variation Δs (µΩ/Ω) | 25 ± 13 | 24 ± 13 | 38 ± 13 |
| Phase shift ωt (mrad) | 1.86 ± 0.03 | 1.90 ± 0.03 | 1.89 ± 0.03 | |
| 5 | Variation Δs (µΩ/Ω) | 125 ± 28 | 101 ± 28 | 131 ± 28 |
| Phase shift ωt (mrad) | 9.1 ± 0.1 | 9.2 ± 0.1 | 9.1 ± 0.1 | |
| 10 | Variation Δs (µΩ/Ω) | 225 ± 46 | 205 ± 46 | 218 ± 46 |
| Phase shift ωt (mrad) | 18.2 ± 0.2 | 18.1 ± 0.2 | 18.1 ± 0.2 | |
| 20 | Variation Δs (µΩ/Ω) | 400 ± 83 | 404 ± 83 | 417 ± 83 |
| Phase shift ωt (mrad) | 36.2 ± 0.4 | 36.3 ± 0.4 | 36.3 ± 0.4 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ouameur, M.; Vasconcellos, R.; Agazar, M. Digital Impedance Bridge for Four-Terminal-Pair AC Resistor Calibration up to 20 kHz. Metrology 2024, 4, 1-14. https://doi.org/10.3390/metrology4010001
Ouameur M, Vasconcellos R, Agazar M. Digital Impedance Bridge for Four-Terminal-Pair AC Resistor Calibration up to 20 kHz. Metrology. 2024; 4(1):1-14. https://doi.org/10.3390/metrology4010001
Chicago/Turabian StyleOuameur, Mohamed, Renata Vasconcellos, and Mohamed Agazar. 2024. "Digital Impedance Bridge for Four-Terminal-Pair AC Resistor Calibration up to 20 kHz" Metrology 4, no. 1: 1-14. https://doi.org/10.3390/metrology4010001
APA StyleOuameur, M., Vasconcellos, R., & Agazar, M. (2024). Digital Impedance Bridge for Four-Terminal-Pair AC Resistor Calibration up to 20 kHz. Metrology, 4(1), 1-14. https://doi.org/10.3390/metrology4010001






