An Update to The Demagnetizing Factor Dataset Calculated for The General Ellipsoid by Osborn
Abstract
:1. Introduction
2. Demagnetizing Factors of the General Ellipsoid
3. New Calculated Data for the Demagnetizing Factors of the General Ellipsoid
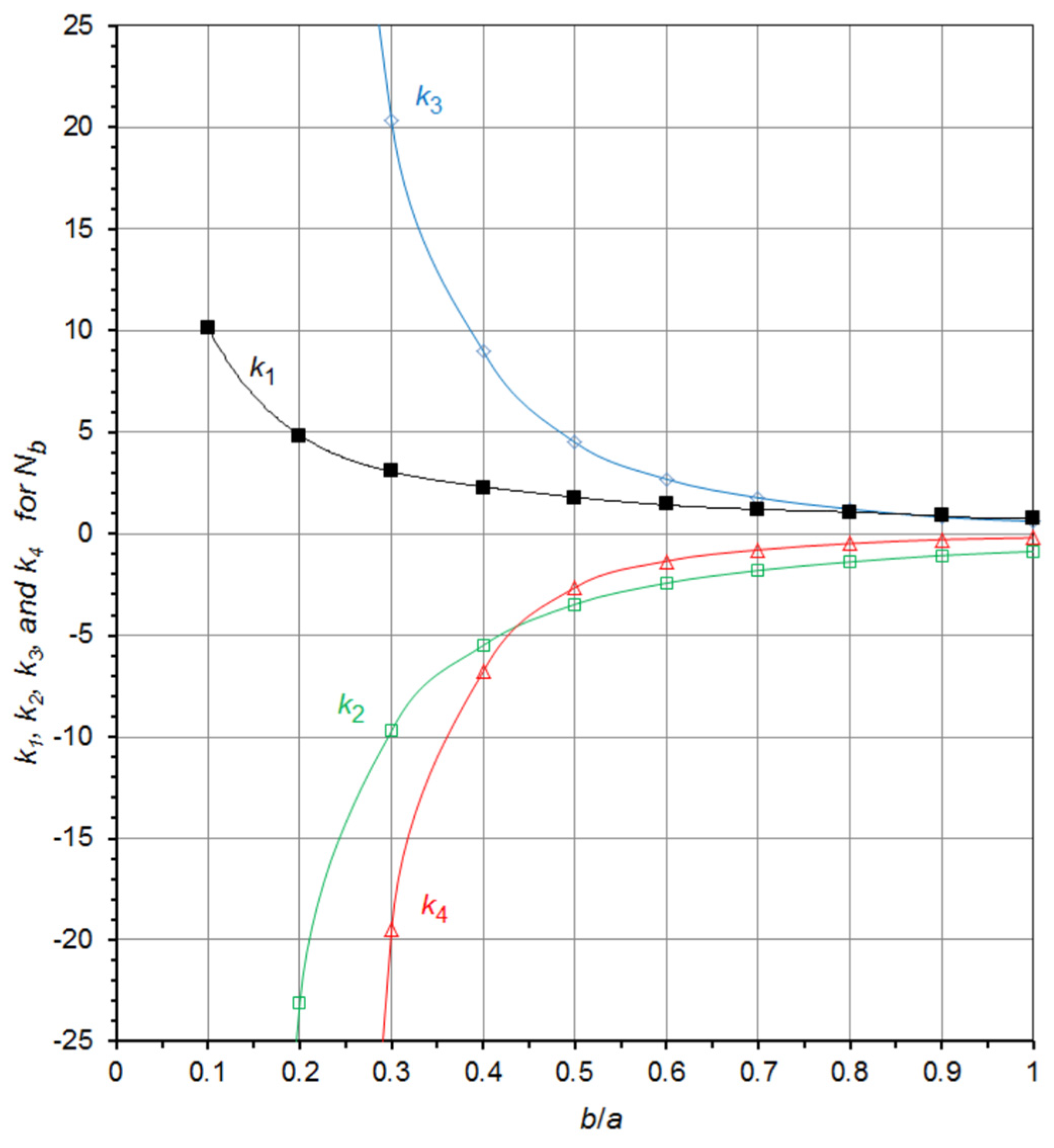
4. A Useful Graphical Representation of the Demagnetizing Factor Data for the General Ellipsoid
5. Summary
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- McGuire, T.R.; Potter, R.I. Anisotropic magnetoresistance in ferromagnetic 3d alloys. IEEE Trans. Magn. 1975, 11, 1018–1038. [Google Scholar] [CrossRef]
- Campbell, I.A.; Fert, A. Chapter 9 Transport Properties of Ferromagnets. In Handbook of Ferromagnetic Materials; Wohlfarth, E.P., Ed.; North-Holland: Amsterdam, The Netherland, 1982; Volume 3. [Google Scholar]
- Bakonyi, I. Guidelines for the evaluation of magnetotransport parameters from measurements on thin strip-shaped samples of bulk metallic ferromagnets with finite residual resistivity. Eur. Phys. J. Plus 2018, 133, 521. [Google Scholar] [CrossRef]
- Isnaini, V.A.; Kolonits, T.; Czigány, Z.; Gubicza, J.; Zsurzsa, S.; Varga, L.K.; Tóth-Kádár, E.; Pogány, L.; Péter, L.; Bakonyi, I. Room-temperature magnetoresistance of nanocrystalline Ni metal with various grain sizes. Eur. Phys. J. Plus 2020, 135, 39. [Google Scholar] [CrossRef]
- Bakonyi, I.; Czeschka, F.D.; Kiss, L.F.; Isnaini, V.A.; Krupp, A.T.; Palotás, K.; Zsurzsa, S.; Péter, L. High-field magnetoresistance of microcrystalline and nanocrystalline Ni metal at 3 K and 300 K. Eur. Phys. J. Plus 2022, 137, 871. [Google Scholar] [CrossRef]
- El-Tahawy, M.; Péter, L.; Kiss, L.F.; Gubicza, J.; Czigány, Z.; Molnár, G.; Bakonyi, I. Anisotropic magnetoresistance (AMR) of cobalt: hcp-Co vs. fcc-Co. J. Magn. Magn. Mater. 2022, 560, 169660. [Google Scholar] [CrossRef]
- El-Tahawy, M.; Péter; Gubicza, J.; Molnár, G.; Li, C.; Vitos, L.; Bakonyi, I. Metastable Phase Formation in Electrodeposited Co-Rich Co-Cu and Co-Ni Alloys. J. Electrochem. Soc. 2023, 170, 062507. [Google Scholar] [CrossRef]
- Bakonyi, I.; Czeschka, F.D.; Isnaini, V.A.; Krupp, A.T.; Gubicza, J.; Varga, L.K.; Péter, L. High-field magnetoresistance measurements on Ni75Co25 and Ni40Co60 alloys at 3 K and 300 K. J. Magn. Magn. Mater. 2024, 591, 171683. [Google Scholar] [CrossRef]
- Kiss, L.F.; Varga, L.K.; Gubicza, J.; Péter, L.; Bakonyi, I. Influence of demagnetizing effects on the magnetization curves of finite-size rectangular slabs (in preparation).
- Osborn, J.A. Demagnetizing Factors of the General Ellipsoid. Phys. Rev. 1945, 67, 351. [Google Scholar] [CrossRef]
- Stoner, E.C. XCVII. The demagnetizing factors for ellipsoids. Philos. Mag. 1945, 36, 803. [Google Scholar] [CrossRef]
- Cronemeyer, D.C. Demagnetization factors for general ellipsoids. J. Appl. Phys. 1991, 70, 2911–2914, Erratum in J. Appl. Phys. 1991, 70, 7660. [Google Scholar] [CrossRef]
- Chen, D.-X.; Brug, J.A.; Goldfarb, R.B. Demagnetizing factors for cylinders. IEEE Trans. Magn. 1991, 27, 3601. [Google Scholar] [CrossRef]
- Zheng, G.; Pardavi-Horváth, M.; Huang, X.; Keszei, B.; Vandlik, J. Experimental determination of an effective demagnetization factor for nonellipsoidal geometries. J. Appl. Phys. 1996, 79, 5742. [Google Scholar] [CrossRef]
- Aharoni, A. Demagnetizing factors for rectangular ferromagnetic prisms. J. Appl. Phys. 1998, 83, 3432. [Google Scholar] [CrossRef]
- Pardavi-Horvath, M. Shape and size effects in nanostructured 2D magnetic systems. J. Magn. Magn. Mater. 1999, 198–199, 219–221. [Google Scholar] [CrossRef]
- Chen, D.-X.; Pardo, E.; Sanchez, A. Demagnetizing factors of rectangular prisms and ellipsoids. IEEE Trans. Magn. 2002, 38, 1742–1752. [Google Scholar] [CrossRef]
- Chen, D.-X.; Prados, C.; Pardo, E.; Sanchez, A.; Hernando, A. Transverse demagnetizing factors of long rectangular bars: I. Analytical expressions for extreme values of susceptibility. J. Appl. Phys. 2002, 91, 5254–5259. [Google Scholar] [CrossRef]
- Chen, D.-X.; Pardo, E.; Sanchez, A. Demagnetizing factors for rectangular prisms. IEEE Trans. Magn. 2005, 41, 2077–2088. [Google Scholar] [CrossRef]
- Beleggia, M.; De Graef, M.; Millev, Y. Demagnetization factors of the general ellipsoid: An alternative to the Maxwell approach. Philos. Mag. 2006, 86, 2451. [Google Scholar] [CrossRef]
- Smith, A.; Nielsen, K.K.; Christensen, D.V.; Bahl, C.R.H.; Bjørk, R.; Hattel, J. The demagnetizing field of a nonuniform rectangular prism. J. Appl. Phys. 2010, 107, 103910. [Google Scholar] [CrossRef]
- Cullity, B.D.; Graham, C.D. Introduction to Magnetic Materials, 2nd ed.; IEEE Press–Wiley: Hoboken, NJ, USA, 2009; p. 54. [Google Scholar]


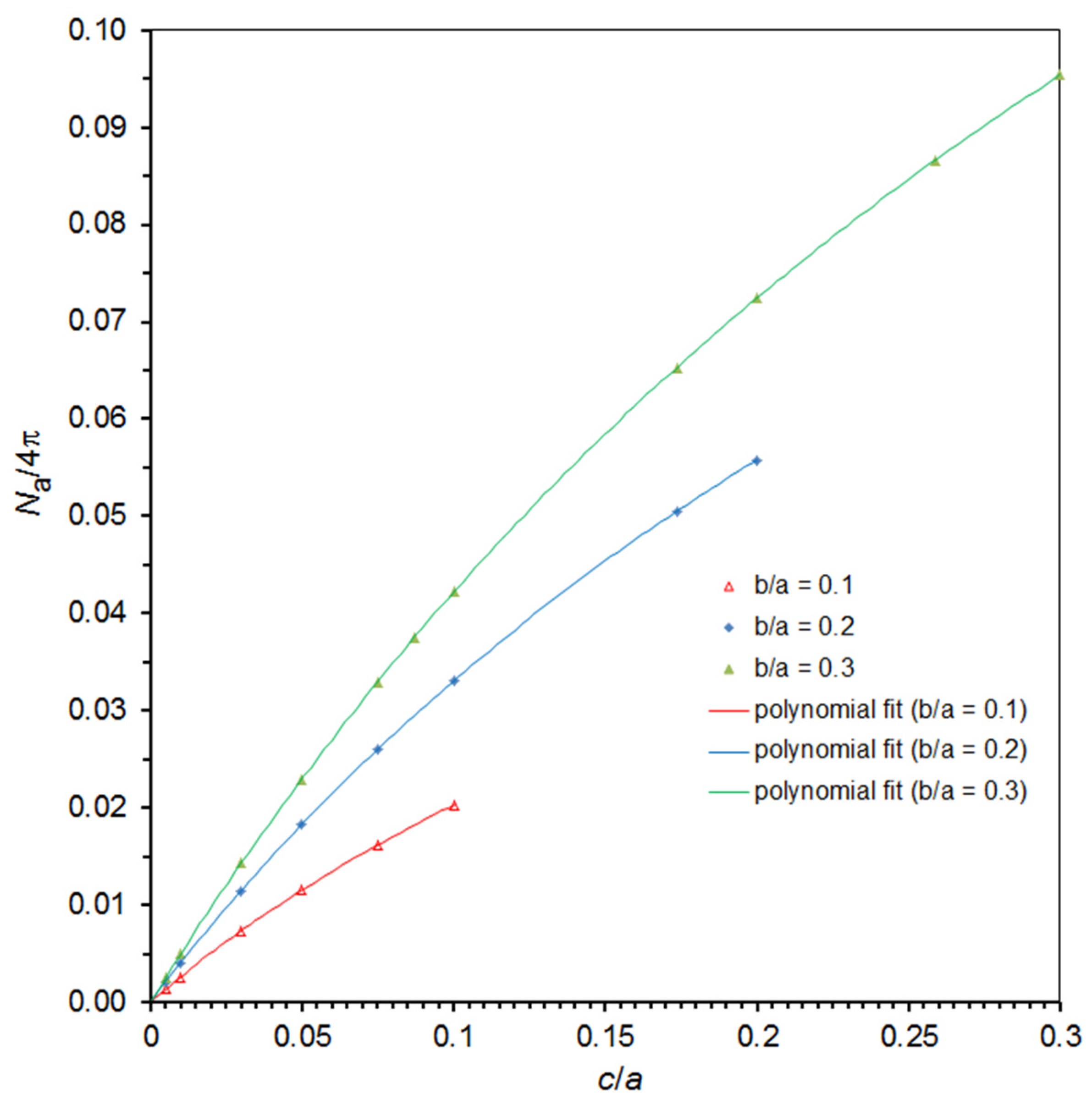
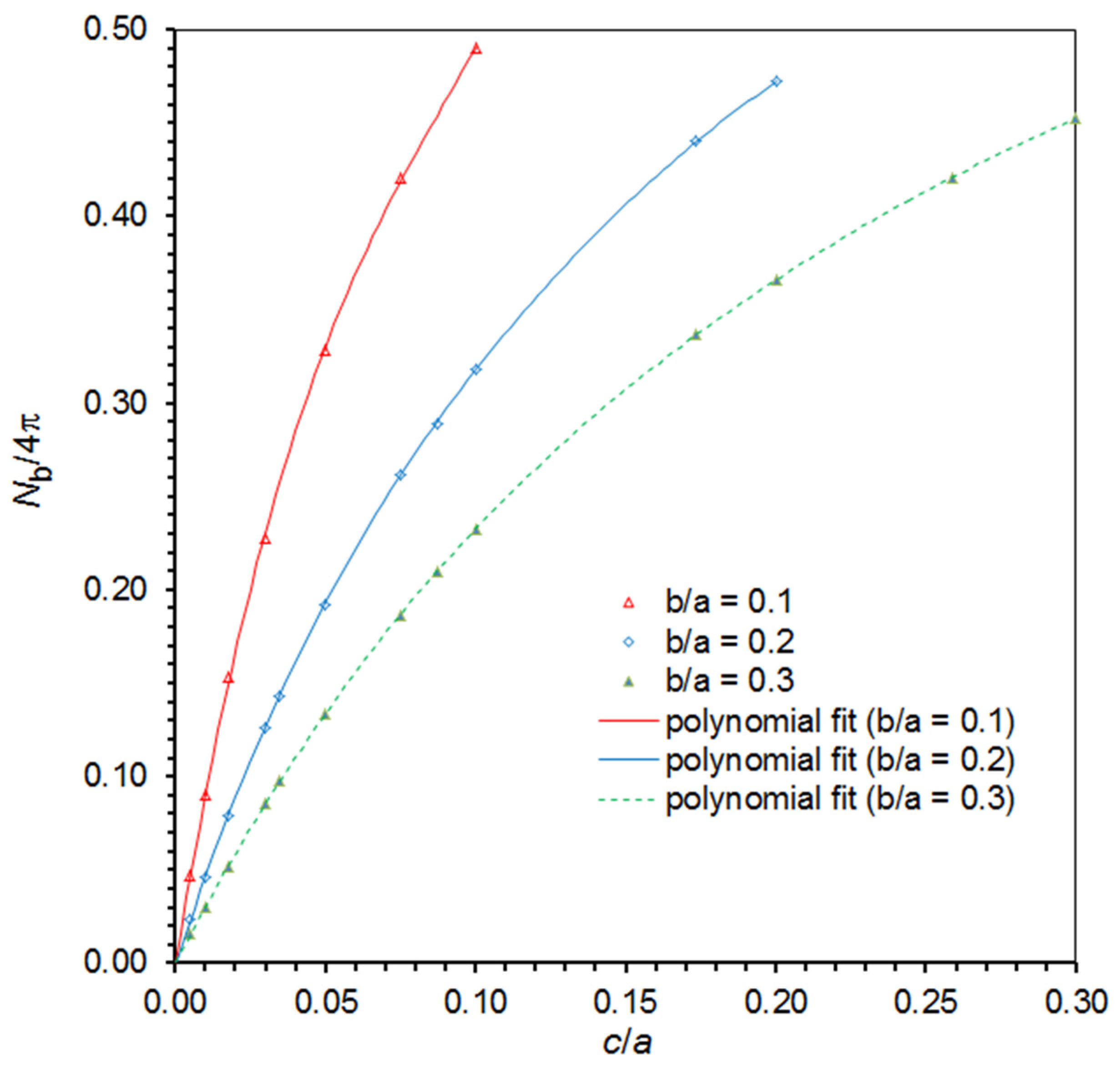

| b/a | c/a | Na/4π | Nb/4π | Nc/4π | b/a | c/a | Na/4π | Nb/4π | Nc/4π |
|---|---|---|---|---|---|---|---|---|---|
| 0.1 | 0.005 | 0.001329 | 0.047066 | 0.951605 | 0.6 | 0.005 | 0.003345 | 0.007197 | 0.989457 |
| 0.01 | 0.002612 | 0.089804 | 0.907585 | 0.01 | 0.006642 | 0.014259 | 0.979099 | ||
| 0.03 | 0.007338 | 0.227495 | 0.765167 | 0.03 | 0.019357 | 0.041225 | 0.939419 | ||
| 0.05 | 0.011528 | 0.327974 | 0.660498 | 0.05 | 0.031364 | 0.066289 | 0.902346 | ||
| 0.075 | 0.016171 | 0.420749 | 0.563080 | 0.075 | 0.045462 | 0.095223 | 0.859315 | ||
| 0.1 | 0.0203 | 0.4899 | 0.4898 | 0.1 | 0.0586 | 0.1218 | 0.8196 | ||
| 0.2 | 0.005 | 0.002023 | 0.023606 | 0.974372 | 0.7 | 0.005 | 0.003524 | 0.006012 | 0.990464 |
| 0.01 | 0.003998 | 0.046050 | 0.949952 | 0.01 | 0.006998 | 0.011925 | 0.981077 | ||
| 0.03 | 0.011455 | 0.125752 | 0.862793 | 0.03 | 0.020424 | 0.034624 | 0.944952 | ||
| 0.05 | 0.018279 | 0.192259 | 0.789461 | 0.05 | 0.033138 | 0.055900 | 0.910962 | ||
| 0.075 | 0.026049 | 0.261274 | 0.712676 | 0.075 | 0.048107 | 0.080677 | 0.871216 | ||
| 0.1 | 0.0331 | 0.3183 | 0.6486 | 0.1 | 0.0621 | 0.1036 | 0.8343 | ||
| 0.3 | 0.005 | 0.002499 | 0.015477 | 0.982023 | 0.8 | 0.005 | 0.003671 | 0.005128 | 0.991201 |
| 0.01 | 0.004950 | 0.030426 | 0.964623 | 0.01 | 0.007294 | 0.010179 | 0.982527 | ||
| 0.03 | 0.014297 | 0.085430 | 0.900273 | 0.03 | 0.021311 | 0.029650 | 0.949039 | ||
| 0.05 | 0.022975 | 0.133772 | 0.843253 | 0.05 | 0.034611 | 0.048018 | 0.917371 | ||
| 0.075 | 0.032988 | 0.186493 | 0.780519 | 0.075 | 0.050305 | 0.069552 | 0.880143 | ||
| 0.1 | 0.0422 | 0.2322 | 0.7256 | 0.1 | 0.0651 | 0.0896 | 0.8453 | ||
| 0.4 | 0.005 | 0.002853 | 0.011351 | 0.985796 | 0.9 | 0.005 | 0.003796 | 0.004444 | 0.991760 |
| 0.01 | 0.005657 | 0.022402 | 0.971941 | 0.01 | 0.007543 | 0.008829 | 0.983629 | ||
| 0.03 | 0.016410 | 0.063814 | 0.919776 | 0.03 | 0.022058 | 0.02578 | 0.952162 | ||
| 0.05 | 0.026474 | 0.101225 | 0.872300 | 0.05 | 0.035853 | 0.041849 | 0.922298 | ||
| 0.075 | 0.038182 | 0.143158 | 0.818660 | 0.075 | 0.052157 | 0.060793 | 0.887050 | ||
| 0.1 | 0.049 | 0.1805 | 0.7705 | 0.1 | 0.0675 | 0.0786 | 0.8539 | ||
| 0.5 | 0.005 | 0.003127 | 0.008860 | 0.988013 | 1 | 0.005 | 0.003902 | 0.003902 | 0.992196 |
| 0.01 | 0.006205 | 0.017526 | 0.976269 | 0.01 | 0.007755 | 0.007755 | 0.98449 | ||
| 0.03 | 0.018049 | 0.050368 | 0.931583 | 0.03 | 0.022693 | 0.022693 | 0.954615 | ||
| 0.05 | 0.029193 | 0.080545 | 0.890262 | 0.05 | 0.036909 | 0.036909 | 0.926181 | ||
| 0.075 | 0.042225 | 0.114965 | 0.842810 | 0.075 | 0.053738 | 0.053738 | 0.892524 | ||
| 0.1 | 0.0544 | 0.1462 | 0.7994 | 0.1 | 0.069598 | 0.069598 | 0.860804 |
| Demagnetizing Factor | b/a | Fitted Polynomials for Na/4π and Nb/4π vs. c/a Data |
|---|---|---|
| Na/4π | 0.1 | y = −8.213325x4 + 3.683730x3 − 0.959648x2 + 0.270340x |
| 0.2 | y = −2.056457x4 + 1.893922x3 − 0.943486x2 + 0.408386x | |
| 0.3 | y = −1.036537x4 + 1.383364x3 − 0.937688x2 + 0.502749x | |
| 0.4 | y = −0.649740x4 + 1.058068x3 − 0.901723x2 + 0.570613x | |
| 0.5 | y = −0.634477x4 + 1.093231x3 − 0.950133x2 + 0.628057x | |
| 0.6 | y = −0.416283x4 + 0.892748x3 − 0.918148x2 + 0.669353x | |
| 0.7 | y = −0.323111x4 + 0.789552x3 − 0.901536x2 + 0.703562x | |
| 0.8 | y = −0.277554x4 + 0.737396x3 − 0.896990x2 + 0.732554x | |
| 0.9 | y = −0.226527x4 + 0.664125x3 − 0.875293x2 + 0.754415x | |
| 1.0 | y = −0.190013x4 + 0.607244x3 − 0.857422x2 + 0.773218x | |
| Nb/4π | 0.1 | y = −2725.800x4 + 797.2283x3 − 104.9405x2 + 10.14657x |
| 0.2 | y = −122.1111x4 + 78.27606x3 − 23.08401x2 + 4.822780x | |
| 0.3 | y = −19.49980x4 + 20.34586x3 − 9.691984x2 + 3.109993x | |
| 0.4 | y = −6.783389x4 + 8.992644x3 − 5.484144x2 + 2.269712x | |
| 0.5 | y = −2.650351x4 + 4.504643x3 − 3.476309x2 + 1.769546x | |
| 0.6 | y = −1.337034x4 + 2.687582x3 − 2.427738x2 + 1.436237x | |
| 0.7 | y = −0.782321x4 + 1.770638x3 − 1.798673x2 + 1.199285x | |
| 0.8 | y = −0.475207x4 + 1.211208x3 − 1.377362x2 + 1.022217x | |
| 0.9 | y = −0.278778x4 + 0.814805x3 − 1.057882x2 + 0.880707x | |
| 1.0 | y = −0.190013x4 + 0.607244x3 − 0.857422x2 + 0.773218x |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kiss, L.F.; Bakonyi, I. An Update to The Demagnetizing Factor Dataset Calculated for The General Ellipsoid by Osborn. Magnetism 2024, 4, 173-182. https://doi.org/10.3390/magnetism4030012
Kiss LF, Bakonyi I. An Update to The Demagnetizing Factor Dataset Calculated for The General Ellipsoid by Osborn. Magnetism. 2024; 4(3):173-182. https://doi.org/10.3390/magnetism4030012
Chicago/Turabian StyleKiss, László F., and Imre Bakonyi. 2024. "An Update to The Demagnetizing Factor Dataset Calculated for The General Ellipsoid by Osborn" Magnetism 4, no. 3: 173-182. https://doi.org/10.3390/magnetism4030012
APA StyleKiss, L. F., & Bakonyi, I. (2024). An Update to The Demagnetizing Factor Dataset Calculated for The General Ellipsoid by Osborn. Magnetism, 4(3), 173-182. https://doi.org/10.3390/magnetism4030012







