Existence in the Large for Caputo Fractional Multi-Order Systems with Initial Conditions
Abstract
:1. Introduction
2. Preliminaries
3. Auxiliary Results
4. Main Result
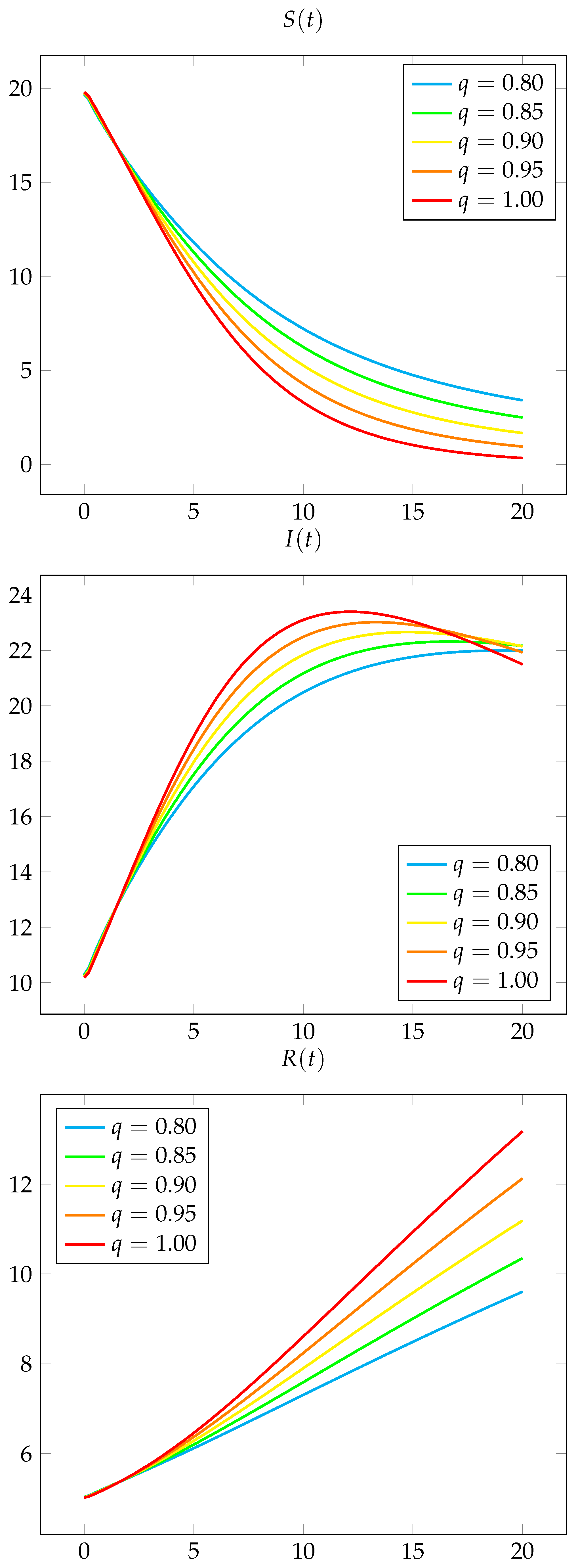
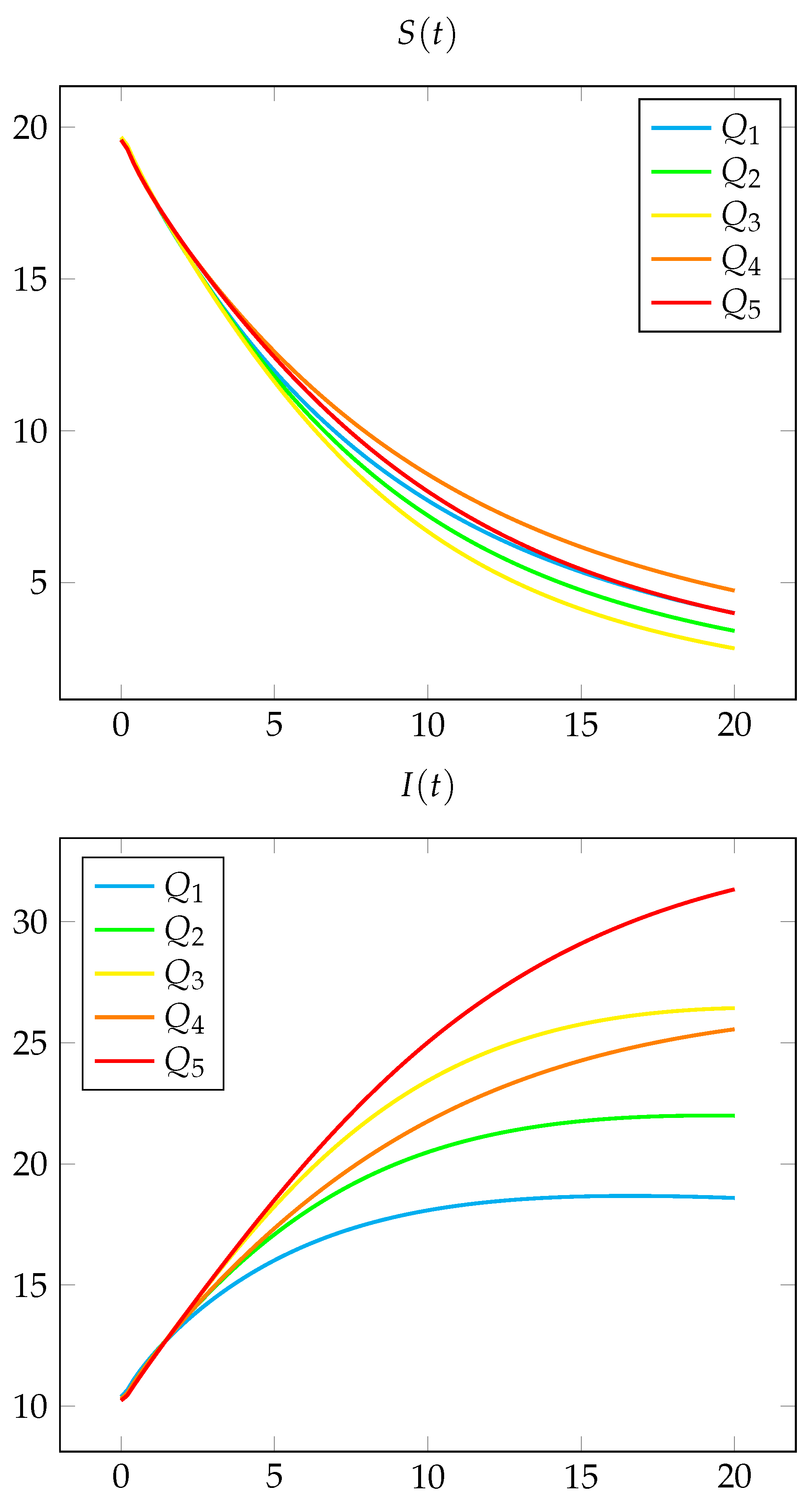
5. Applications
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| SIR | Susceptible, Infected, and Recovered |
References
- Diethelm, K.; Freed, A. On the solution of nonlinear fractional differential equations used in the modeling of viscoplasticity. In Scientific Computing in Chemical Engineering II: Computational Fluid Dynamics, Reaction Engineering, and Molecular Properties; Keil, F., Mackens, W., Vob, H., Werther, J., Eds.; Springer: Berlin/Heidelberg, Germany, 1999; pp. 217–224. [Google Scholar]
- Glöckle, W.; Nonnenmacher, T. A fractional calculus approach to self similar protein dynamics. Biophy. J. 1995, 68, 46–53. [Google Scholar] [CrossRef] [PubMed]
- Metzler, R.; Schick, W.; Kilian, H.; Nonnenmacher, T. Relaxation in filled polymers: A fractional calculus approach. J. Chem. Phy. 1995, 103, 7180–7186. [Google Scholar] [CrossRef]
- Oldham, B.; Spanier, J. The Fractional Calculus; Academic Press: New York, NY, USA; London, UK, 2002. [Google Scholar]
- Zhou, Y.; He, J.W. A Cauchy problem for fractional evolution equations with Hilfer’s fractional derivative on semi-infinite interval. Fract. Calc. Appl. Anal. 2022, 25, 924–961. [Google Scholar] [CrossRef]
- Sivasankar, S.; Udhayakumar, R. Discussion on Existence of Mild Solutions for Hilfer Fractional Neutral Stochastic Evolution Equations Via Almost Sectorial Operators with Delay. Qual. Theory Dyn. Syst. 2023, 22, 67. [Google Scholar] [CrossRef]
- Sunthrayuth, P.; Shah, R.; Zidan, A.; Khan, S.; Kafle, J. The analysis of fractional-order Navier-Stokes model arising in the unsteady flow of a viscous fluid via Shehu transform. J. Funct. Spaces 2021, 2021, 1–15. [Google Scholar] [CrossRef]
- Aljahdaly, N.H.; Akgül, A.; Shah, R.; Mahariq, I.; Kafle, J. A comparative analysis of the fractional-order coupled Korteweg–De Vries equations with the Mittag–Leffler law. J. Math. 2022, 2022, 1–30. [Google Scholar] [CrossRef]
- Yang, W.; Wang, D.; Yang, L. A stable numerical method for space fractional Landau–Lifshitz equations. Appl. Math. Lett. 2016, 61, 149–155. [Google Scholar] [CrossRef]
- Sunthrayuth, P.; Ullah, R.; Khan, A.; Shah, R.; Kafle, J.; Mahariq, I.; Jarad, F. Numerical analysis of the fractional-order nonlinear system of Volterra integro-differential equations. J. Funct. Spaces 2021, 2021, 1–10. [Google Scholar] [CrossRef]
- Naeem, M.; Yasmin, H.; Shah, N.A.; Kafle, J.; Nonlaopon, K. Analytical Approaches for Approximate Solution of the Time-Fractional Coupled Schrödinger–KdV Equation. Symmetry 2022, 14, 2602. [Google Scholar] [CrossRef]
- Kilbas, A.; Srivastava, H.; Trujillo, J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Kiryakova, V. Generalized Fractional Calculus and Applications; Pitman Research Notes in Mathematics Series; Longman-Wiley: New York, NY, USA, 1994; Volume 301. [Google Scholar]
- Almeida, R.; Bastos, N.R.O.; Monteiro, M.T.T. Modeling some real phenomena by fractional differential equations. Math. Methods Appl. Sci. 2016, 39, 4846–4855. [Google Scholar] [CrossRef]
- Denton, Z. Monotone method for multi-order 2-systems of Riemann-Liouville fractional differential equations. Commun. Appl. Anal. 2015, 19, 353–368. [Google Scholar]
- Denton, Z. Monotone Method for Riemann-Liouville Multi-Order Fractional Differential Systems. Opusc. Math. 2016, 36, 189–206. [Google Scholar] [CrossRef]
- Denton, Z.; Ramírez, J. Generalized Monotone Method for Multi-Order 2-Systems of Riemann-Liouville Fractional Differential Equations. Nonlinear Dyn. Syst. Theory 2016, 16, 246–259. [Google Scholar]
- Chhetri, P.; Vatsala, A. Existence of the Solution in the Large for Caputo Fractional Reaction Diffusion Equation by Picard’s Method. Dyn. Syst. Appl. 2018, 27, 837–851. [Google Scholar] [CrossRef]
- Pageni, G.; Vatsala, A.S. Study of two system of Caputo fractional differential equations with initial conditions via Laplace transform method. Neural Parallel Sci. Comput. 2021, 29, 69–83. [Google Scholar] [CrossRef]
- Pageni, G.; Vatsala, A. Study of Three Systems of Non-linear Caputo Fractional Differential Equations with Initial Conditions and Applications. Neural Parallel Sci. Comput. 2021, 29, 211–229. [Google Scholar] [CrossRef]
- Lakshmikantham, V.; Vatsala, A. Theory of fractional differential inequalities and Applications. Commun. Appl. Anal. 2007, 11, 395–402. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Gorenflo, R.; Kilbas, A.A.; Mainardi, F.; Rogosin, S.V. Mittag-Leffler Functions, Related Topics and Applications; Springer: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Almezel, S.; Ansari, Q.H.; Khamsi, M.A. Topics in Fixed Point Theory; Springer: Berlin/Heidelberg, Germany, 2014; Volume 5. [Google Scholar]
- Caccioppoli, R. Un teorema generale sull’esistenza di elementi uniti in una trasformazione funzionale. Rend. Accad. Naz. Lincei 1930, 11, 794–799. [Google Scholar]
- Vatsala, A.S.; Pageni, G.; Vijesh, V.A. Analysis of Sequential Caputo Fractional Differential Equations versus Non-Sequential Caputo Fractional Differential Equations with Applications. Foundations 2022, 2, 1129–1142. [Google Scholar] [CrossRef]
- Vatsala, A.; Pageni, G. Caputo Sequential Fractional Differential Equations with Applications. In Synergies in Analysis, Discrete Mathematics, Soft Computing and Modelling; Springer: Berlin/Heidelberg, Germany, 2023; pp. 83–102. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Denton, Z.; Vatsala, A.S. Existence in the Large for Caputo Fractional Multi-Order Systems with Initial Conditions. Foundations 2023, 3, 260-274. https://doi.org/10.3390/foundations3020021
Denton Z, Vatsala AS. Existence in the Large for Caputo Fractional Multi-Order Systems with Initial Conditions. Foundations. 2023; 3(2):260-274. https://doi.org/10.3390/foundations3020021
Chicago/Turabian StyleDenton, Zachary, and Aghalaya S. Vatsala. 2023. "Existence in the Large for Caputo Fractional Multi-Order Systems with Initial Conditions" Foundations 3, no. 2: 260-274. https://doi.org/10.3390/foundations3020021
APA StyleDenton, Z., & Vatsala, A. S. (2023). Existence in the Large for Caputo Fractional Multi-Order Systems with Initial Conditions. Foundations, 3(2), 260-274. https://doi.org/10.3390/foundations3020021




