Structure Functions and Tau Neutrino Cross Section at DUNE Far Detector †
Abstract
:1. Introduction
2. DIS -CC cross Section
2.1. Tau Neutrino Interactions
2.2. Structure Functions
3. Preliminary Results and Outlook
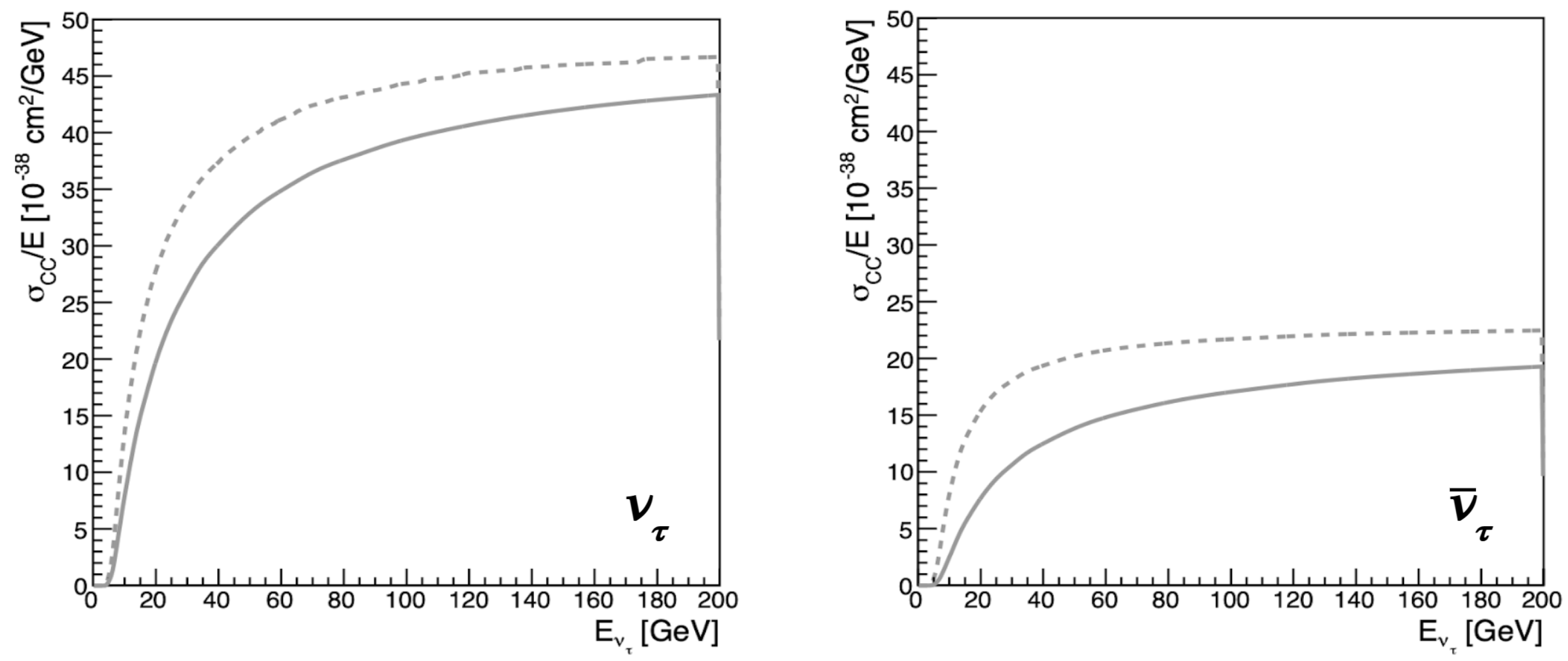
Nature
4. Discussion
- Kinematic changes in and due to the presence of .
- The contributions due to the additional nucleon structure functions and in the presence of .
- As a function of , there is an enhancement that does not come just from a normalization, but due to the changes in the shape of the presence of .
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Abraham, R.M.; Alvarez-Muniz, J.; Argüelles, C.A.; Ariga, A.; Ariga, T.; Aurisano, A.; Autiero, D.; Bishai, M.; Bostan, N.; Bustamante, M.; et al. Tau neutrinos in the next decade: From GeV to EeV. J. Phys. G Nucl. Part. Phys. 2022, 11, 110501. [Google Scholar] [CrossRef]
- Machado, P.; Schulz, H.; Turner, J. Tau neutrinos at DUNE: New strategies, new opportunities. Phys. Rev. D 2020, 10, 053010. [Google Scholar] [CrossRef]
- De Gouvêa, A.; Kelly, K.J.; Stenico, G.V.; Pasquini, P. Physics with Beam Tau-Neutrino Appearance at DUNE. Phys. Rev. D 2019, 1, 016004. [Google Scholar] [CrossRef]
- Albright, C.H.; Jarlskog, C. Neutrino production of M+ and E+ heavy leptons. Nucl. Phys. B 1975, 2, 467–492. [Google Scholar] [CrossRef]
- Jeong, Y.S.; Reno, M.H. Tau neutrino and antineutrino cross sections. Phys. Rev. D 2010, 82, 033010. [Google Scholar] [CrossRef]
- Kretzer, S.; Reno, M.H. Tau neutrino deep inelastic charged current interactions. Phys. Rev. D 2002, 66, 113007. [Google Scholar] [CrossRef]
- Andreopoulos, C.; Barry, C.; Dytman, S.; Gallagher, H.; Golan, T.; Hatcher, R.; Perdue, G.; Yarba, J. The GENIE Neutrino Monte Carlo Generator: Physics and User Manual. arXiv 2015, arXiv:1510.05494. [Google Scholar]
- Kirillov, A.; He, K.; Girshick, R.; Rother, C.; Dollár, P. Panoptic Segmentation. arXiv 2019, arXiv:1801.00868. [Google Scholar]




| Decay Mode | Branching Ratio (%) |
|---|---|
| Leptonic | 35.2 |
| 17.3 | |
| 17.4 | |
| Hadronic | 64.8 |
| 25.5 | |
| 10.8 | |
| 9.3 | |
| 9.0 | |
| 4.5 | |
| other | 5.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yaeggy, B. Structure Functions and Tau Neutrino Cross Section at DUNE Far Detector. Phys. Sci. Forum 2023, 8, 64. https://doi.org/10.3390/psf2023008064
Yaeggy B. Structure Functions and Tau Neutrino Cross Section at DUNE Far Detector. Physical Sciences Forum. 2023; 8(1):64. https://doi.org/10.3390/psf2023008064
Chicago/Turabian StyleYaeggy, Barbara. 2023. "Structure Functions and Tau Neutrino Cross Section at DUNE Far Detector" Physical Sciences Forum 8, no. 1: 64. https://doi.org/10.3390/psf2023008064
APA StyleYaeggy, B. (2023). Structure Functions and Tau Neutrino Cross Section at DUNE Far Detector. Physical Sciences Forum, 8(1), 64. https://doi.org/10.3390/psf2023008064






