Geometry Change of 1,3-Dicyanobenzene upon Electronic Excitation from a Franck–Condon Fit of Several Fluorescence Emission Spectra ^†

Abstract

The change in the geometry of 1,3-dicyanobenzene upon electronic excitation to the lowest excited singlet state has been elucidated by simultaneous Franck–Condon (FC) fits of the fluorescence emission spectra originating from the vibrationless origin and from four vibronic bands. The geometry changes obtained from the FC fits were compared to the results of ab initio calculations at the SCS-CC2/cc-pVTZ level of theory. We found close agreement between the spectral determination and the theoretical prediction of the geometry changes upon excitation. The aromatic ring opens upon excitation, resulting in a symmetrically distorted structure in the excited state.

1. Introduction

Although the determination of ground-state structures of stable molecules is straightforward using a large variety of methods, considerably less work has been performed on molecules in their electronically excited states.

For molecules in the electronic ground state, structure determination can be achieved through methods that use the inertial moments of the molecules, such as microwave spectroscopy or rotationally resolved infrared spectroscopy. Alternatively, diffraction-based methods, such as single-crystal X-ray diffraction (sc-XRD) and electron diffraction (ED) [1] are applicable. Although X-ray diffraction generally requires crystalline samples, both sc-XRD and ED can also be applied in the gas phase using strongly aligned probes. X-ray diffraction of aligned molecules has been performed using femtosecond X-rays from the Linac Coherent Light Source at the SLAC National Accelerator Laboratory [2].

Inertia-based structural determination can also be used to determine excited-state structures via rotationally resolved UV spectroscopy, pioneered by Meerts [3] and Pratt [4], and by coherent beat techniques in the time domain, introduced by the Felker group [5] and later improved by Riehn [6]. Ultrafast electron diffraction opened the gate to structures of excited states [7], especially of dark states, that cannot be encountered by optical methods.

A complementary approach uses vibronic intensities in fluorescence emission spectra and a fit of these intensities to geometry changes within the Franck–Condon (FC) or, when applicable, the Franck–Condon–Herzberg–Teller (FCHT) approximations [8].

Dicyanobenzene-based photocatalysts have found wide interest in recent years as organic dyes, with a wide range of redox potentials [9], and are widely used as electron acceptors in thermally activated delayed fluorescence (TADF) materials [10].

Several cyano-substituted aromatic species have been detected in the interstellar medium. These comprise singly substituted aromatics like cyanobenzene, 1-cyanonaphthalene, and 2-cyanonaphthalene [11,12], as well as disubstituted aromatics, like 1,2-dicyanobenzene (1,2-DCB) and 1,3-dicyanobenzene (1,3-DCB) [13].

Chitarra et al. performed centimeter- and millimeter-wave rotational spectroscopy of 1,2-DCB and 1,3-DCB to determine the inertial parameters, the quartic and sextic centrifugal constants, and the nuclear quadrupole coupling constants [13].

Recently, Zajonz et al. determined the excited-state dipole moments of 1,2-DCB and 1,3-DCB from thermochromic shifts in solution [14].

In the following, we perform an FC analysis of several fluorescence emission spectra of 1,3-dicyanobenzene to elucidate the structural changes upon electronic excitation to the lowest excited singlet state.

2. Computational Methods

2.1. Quantum Chemical Calculations

The molecular structures of 1,2- and 1,3-DCB in their electronic ground (${\mathrm{S}}_0$) and lowest excited singlet states (${\mathrm{S}}_1$) have been optimized, employing Dunning’s correlation-consistent polarized valence triple zeta (cc-pVTZ) basis set from the Turbomole library [15,16]. The equilibrium geometries of the ${\mathrm{S}}_0$- and ${\mathrm{S}}_1$-states were computed using the approximate coupled cluster singles and doubles model (CC2) employing the resolution-of-the-identity (RI) approximation and spin-component scaling (SCS) [17,18,19,20]. The Hessians and harmonic vibrational frequencies of both electronic states, which are a prerequisite for the Franck–Condon fits, described in the following section were obtained from numerical second derivatives using the NumForce script [21]. Additionally, these vibrational frequencies were used to compute the zero-point corrections to the adiabatic excitation energies. The main reason for using SCS-CC2 was that for this method the Hessian is available at the same level of theory for both states. This, of course, is also true for DFT/TD-DFT, which, however, do not compare very well with the experimental vibrational frequencies. MP2 for the ground state is of comparable quality, but lacks a good S1 counterpart. The spin-component scaled (SCS) version of CC2 has to be used for aromatics that contain heteroatoms. Pristine CC2 has large deficiencies, even for the correct order of excited states, which are removed by spin scaling. Other scaling methods like spin-only scaling (SOS) perform equally well compared to SCS.

2.2. Franck–Condon Fits

According to the Franck–Condon principle, the intensities of vibronic transitions, both in absorption and emission, depend on the overlap of the electronic wavefunctions of the different vibronic levels in the ground and excited states. With the FCFit program, developed in our group and described before [22,23], the fit of vibronic intensities of several bands in emission was performed. It was based on the recursion formula of Doktorov, Malkin, and Man’ko for computation of multidimensional FC integrals [24,25]. The ground-state geometry was distorted along a set of normal modes, in order to minimize the differences between the experimentally observed and calculated intensities. A local optimizer was used, based on a Levenberg–Marquardt variant [26,27]. Technical details of the program are given in the Supporting Material.

3. Experimental Methods

The 1,3-DCB (≥$98\%$) was purchased from BLDpharm and was used without further purification. The apparatus, which was used to record the disperse fluorescence (DF) spectra has been described in detail before [28,29]. Shortly, 1,3-DCB was evaporated in a home-built oven at ${\mathrm{T}}_0$ = 453 K and co-expanded, with helium as the carrier gas, into the measuring chamber, which was kept at ${10}^{-5}$ mbar, through a commercial pulsed nozzle (General Valve, 500 $\mu$m orifice). The nozzle was kept at a slightly higher temperature (${\mathrm{T}}_0$ + 10 K) in order to avoid condensation of the substance within the nozzle. The frequency-doubled output of a Nd:YAG (SpectraPhysics, INDI, Darmstadt, Germany) pumped dye laser (Lambda-Physik, FL3002, Göttingen, Germany ) was crossed with the molecular beam. The fluorescence light was dispersed using a monochromator (Jobin Yvon, Oberursel, Germany, f = 1 m) and the spectrum was recorded on an intensified UV-sensitive CMOS detector (LaVision, Göttingen, Germany, IRO X). The relative emission band intensities obtained from integration over the pixel intensities on the CMOS chip were normalized to the strongest band in the respective spectrum. The intensity of the resonantly excited band, which contains stray light from the exciting laser, was omitted from the FC analysis.

4. Results and Discussion

4.1. Computational Results

According to the CC2/cc-pVTZ-optimized structures, 1,3-DCB has ${\mathrm{C}}_{2v}$-symmetry in its electronic ground state and first excited singlet state, respectively. The first electronically excited singlet state, ${\mathrm{S}}_1$, is of ${\mathrm{A}}_1$-symmetry, and arises from a mixed 0.52 (LUMO ← HOMO-1) + 0.43 (LUMO+1 ← HOMO) transition, cf. Figure 1, and can be classified as L_b-state. The ${\mathrm{S}}_2$-state arises mainly from a LUMO ← HOMO excitation and represents an ${\mathrm{L}}_a$-state. The symmetry of the lowest two occupied orbitals, ${\mathrm{A}}_2$ and ${\mathrm{B}}_1$, has been confirmed from a combination of ab initio calculations and photoelectron spectroscopy for 1,3-DCB [30].

The transition dipole moment (TDM) of the ${\mathrm{S}}_1$-state of 1,3-DCB is calculated to be oriented along the a-axis and perpendicular to the c-axis, as shown in Figure 2. The TDM of the ${\mathrm{S}}_2$-state is oriented along the inertial b-axis. Both orientations can be unambiguously derived from the molecular orbitals and leading contributions to the electronic state transitions, shown in Figure 1.

The 36 molecular vibrations of 1,3-DCB transform like ${\Gamma }_{ired}$ = 13${\mathrm{a}}_1$ + 4${\mathrm{a}}_2$ + 7${\mathrm{b}}_1$ + 12${\mathrm{b}}_2$ in ${\mathrm{C}}_{2v}$. The complete Duschinsky matrix for all ground- and excited-state vibrations and the respective symmetries are given in Table S4 of the online Supplementary Material.

Table 1. Calculated and experimental inertial parameters A, B, and C; the resulting inertial defects $\Delta I$; angle of the transition moment vector in the molecular fixed frame with the inertial a-axis $\theta$; and the adiabatic excitation energies ${\nu }_0$ of 1,3-DCB. Doubly primed parameters refer to the ground state, single primed to the excited state. Experimental values of ground-state rotational constants from millimeter- and centimeter-wave spectra [13] for the excited state from the FC analysis of this work.

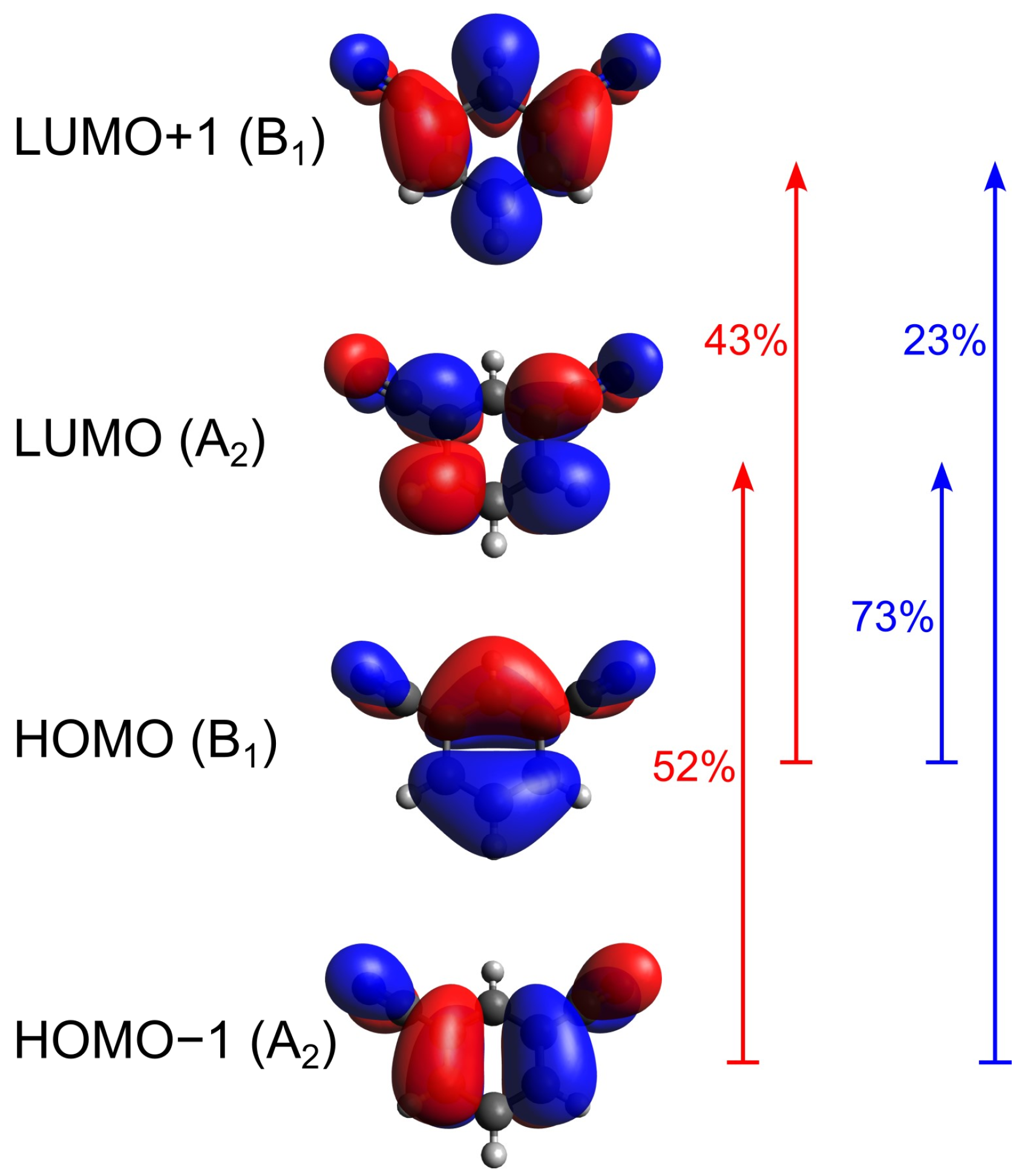
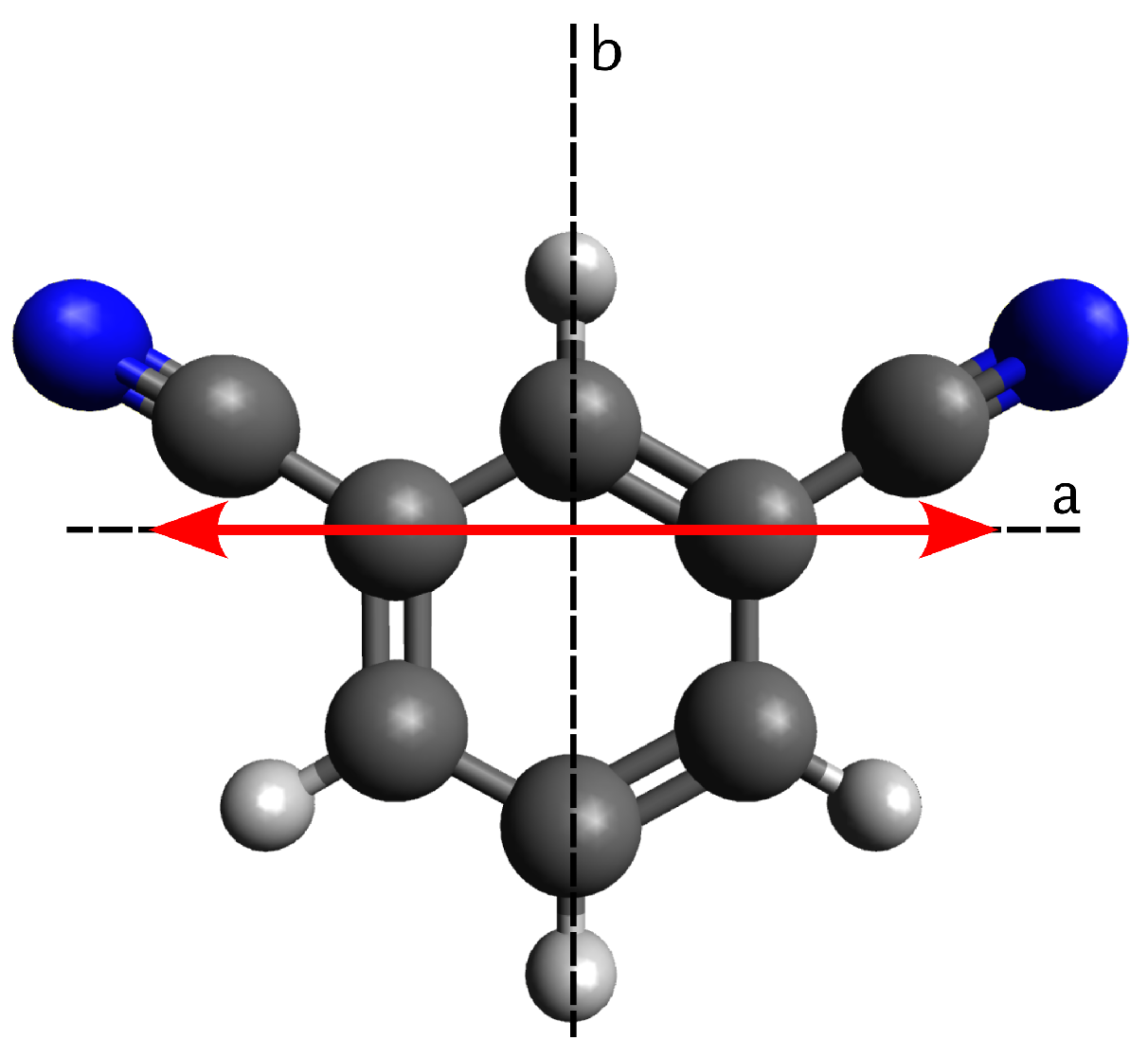
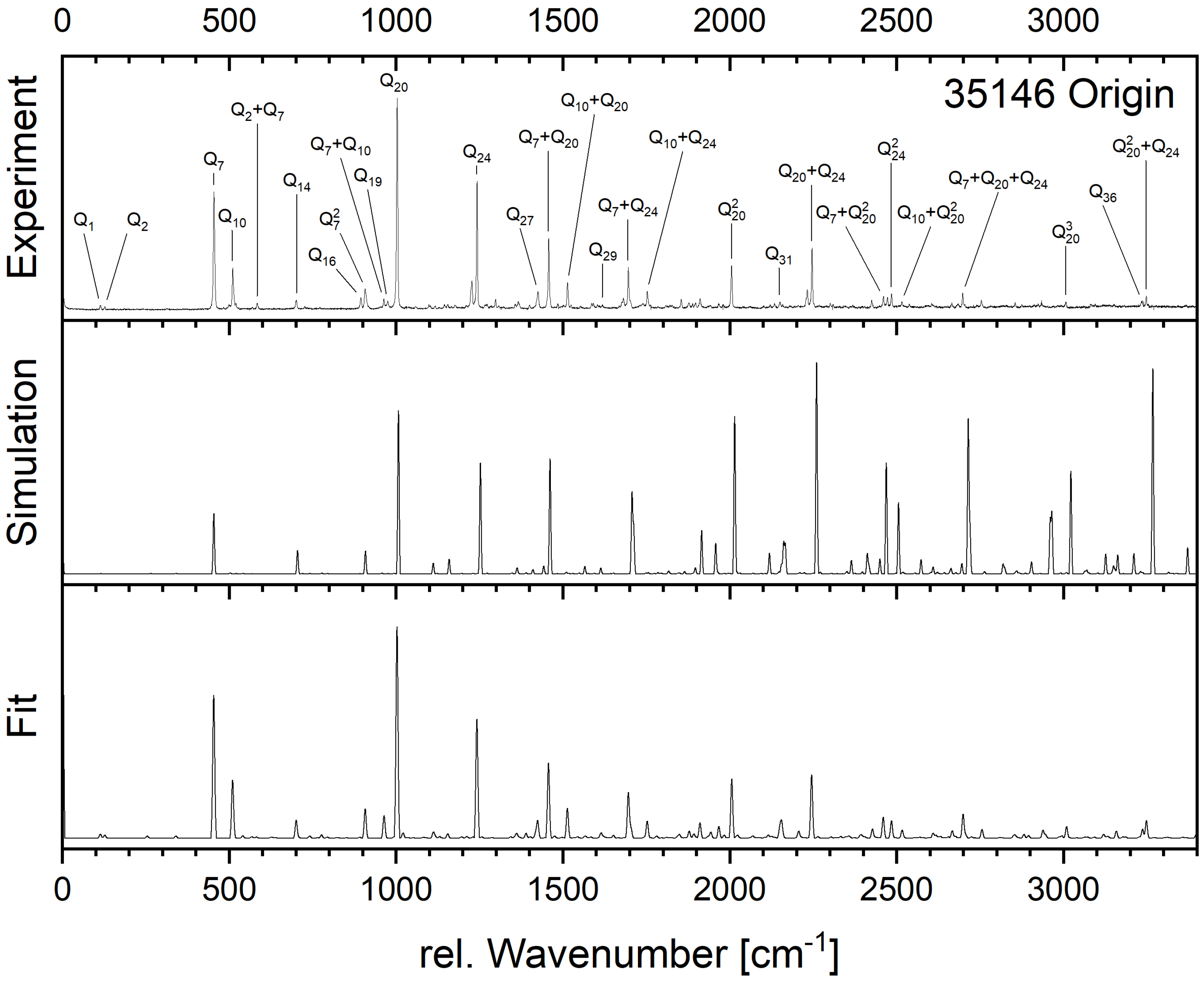
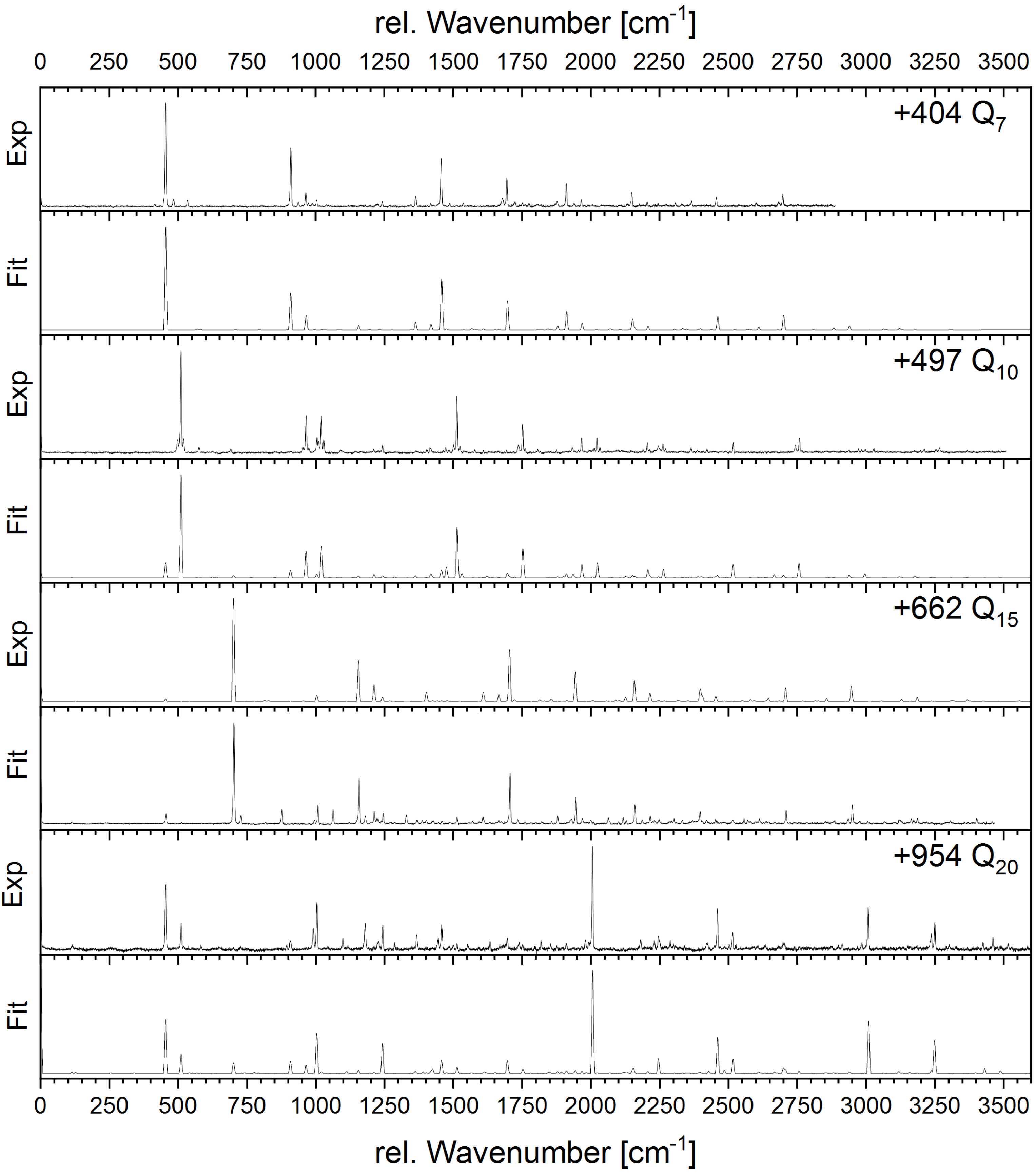
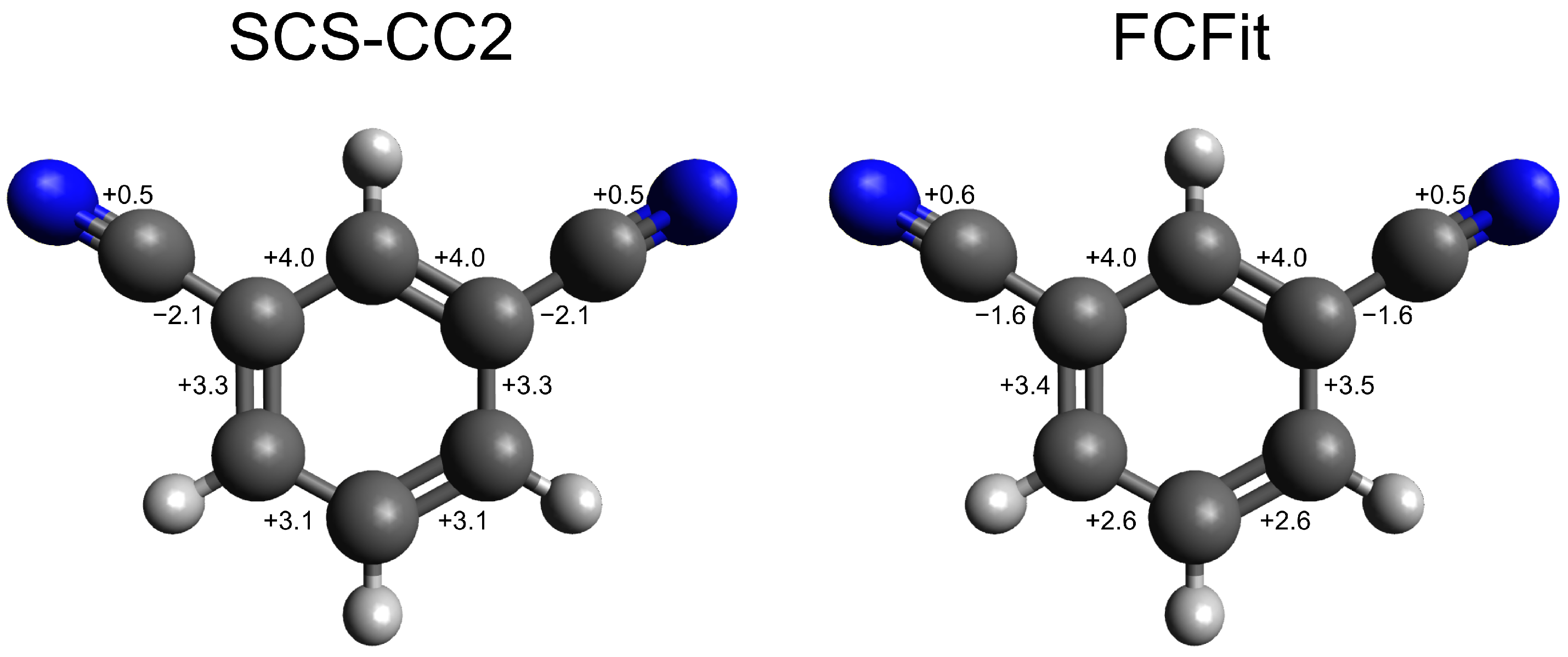
	SCS-CC2/cc-pVTZ	Experiment	FCFit
${\mathrm{A}}^{″}$ /MHz	2706.7	2723.018609(46) 1	2706.7 2
${\mathrm{B}}^{″}$ /MHz	899.9	906.419893(21) 1	899.9 2
${\mathrm{C}}^{″}$ /MHz	675.4	679.859840(15) 1	675.4 2
$\Delta$${\mathrm{I}}^{″}$ /amu Å2	0.0	0.207	0.0
${\mathrm{A}}^{\prime }$(${\mathrm{S}}_1$)/MHz	2632.9	-	2618.3
${\mathrm{B}}^{\prime }$(${\mathrm{S}}_1$)/MHz	885.9	-	884.3
${\mathrm{C}}^{\prime }$(${\mathrm{S}}_1$)/MHz	662.9	-	661.1
$\Delta$${\mathrm{I}}^{\prime }$(${\mathrm{S}}_1$) /amu Å2	0.0	-	−0.014
$\Delta$A(${\mathrm{S}}_1$-${\mathrm{S}}_0$)/MHz	−73.8	-	−88.4
$\Delta$B(${\mathrm{S}}_1$-${\mathrm{S}}_0$)/MHz	−14.0	-	−15.6
$\Delta$C(${\mathrm{S}}_1$-${\mathrm{S}}_0$)/MHz	−12.5	-	−14.3
$\theta$(${\mathrm{S}}_1$) /∘	0.0	-	-
${\nu }_0$(${\mathrm{S}}_1$) /${\mathrm{cm}}^{-1}$	35,958.0	35,146 3	-

¹ Results from millimeter- and centimeter-wave spectra; ref. [13]. ² The ab initio-calculated ${\mathrm{S}}_0$ structure is the reference state in the FC fits. ³ From the LIF spectrum in this study.

compares the ab initio-calculated rotational constants in the ground and excited states with experimental ground-state results from millimeter- and centimeter-wave spectra [13]. The small deviations of less than 0.6% are due to the difference between the constants of the $r_0$-structure given in ref. [13] and the $r_e$-structure derived from our ab initio calculations. The Cartesian geometries of 1,3-DCB in both electronic states are given in Tables S1 and S2 of the online Supporting Information.

No experimental rotational constants for the lowest excited singlet state are available to date.

4.2. Experimental Results

4.2.1. The LIF Spectrum of 1,3-Dicyanobenzene

The laser-induced fluorescence spectrum of 1,3-DCB is shown in Figure 3. The bands at 404, 497, 662, and 954 ${\mathrm{cm}}^{-1}$ relative to the electronic origin, which were excited to record the fluorescence excitation spectra, are marked with their wavenumber, along with their assignment, guided by the results of the ab initio-calculated vibrational frequencies in the ${\mathrm{S}}_1$-state. The vibrational frequencies and assignments in the ${\mathrm{S}}_1$-state along with the largest elements of the Duschinsky matrix are summarized in Table S4 of the online Supplementary Material. The magnitude of the (3N-6)(3N-6) elements of the Duschinsky matrix show which modes in the ground state resemble most those of the excited state. Matrix elements with a value close to 1 signify that the respective mode in the excited state is comprised solely of the same mode in the ground state. A graphical representation of the Duschinsky matrix is given in Figure S2 of the online Supporting Material. The excited modes, and those along which the ground-state geometry has been distorted, are depicted in Figure S1 of the online Supplementary Material; the numerical values of the displacements are given in Table S3.

A word on the notation of the vibrations used here. There are several labeling schemes for vibrations of substituted benzenes, which have evolved over several decades. The most prominent are the Wilson-mode labels [31], the scheme according to Varsányi [32], and the Mulliken [33] or Herzberg [34] labels. More recently, Wright pointed out, that for meta-disubstituted benzene derivatives these nomenclatures result in contradicting assignments, depending on the mass of the substituents [35]. Since the present work intends to work out the structural changes after electronic excitation from the FC intensity pattern of several emission spectra, we adopt a more simplistic scheme in which the modes are solely sorted by increasing energy, irrespective of their symmetry.

4.2.2. Fluorescence Emission

Dispersed fluorescence (DF) spectra were recorded by pumping the vibrationless origin at 35.146 ${\mathrm{cm}}^{-1}$ and the vibronic bands at +404, +497, +662, and +954 ${\mathrm{cm}}^{-1}$ relative to the electronic origin. The upper trace of Figure 4 shows the DF spectrum, obtained through the electronic origin. The assignment of the electronic ground state is guided by the normal-mode analysis from the CC2/cc-pVTZ calculations, shown in the middle trace of Figure 4. The lowest trace of Figure 4 presents the Franck–Condon fit of the emission spectrum, using the vibronic intensities given in Table S5 of the online Supporting Material.

The vibronic emission spectrum, which is obtained by excitation of the vibrationless origin, is mainly governed by overtones and combination bands of the modes ${\mathrm{Q}}_7$, ${\mathrm{Q}}_{20}$, and ${\mathrm{Q}}_{24}$. These modes are depicted in Figure S1 of the Supplementary Material available online. It is remarkable how the fit of the geometry changes improves the intensity features compared to the simulation performed at the ab initio geometry of the excited state. This is especially true for the overtone of mode ${\mathrm{Q}}_{20}$ and combination bands of mode ${\mathrm{Q}}_{20}$ with ${\mathrm{Q}}_{24}$. Obviously, the ab initio geometry is considerably distorted along these modes compared to the ground state in contrast to the experimental finding.

Figure 5 summarizes the results of simultaneous FC fits of the bands obtained from excitation of 0,0+404, 0,0+497, 0,0+662, and 0,0+954 ${\mathrm{cm}}^{-1}$. In all cases a good overall agreement is obtained between the experimental intensity distribution and the fitted intensities.

4.3. Franck–Condon Fit of Geometry Changes upon Electronic Excitation

We determined the structural changes of 1,3-DCB after electronic excitation from the ground (${\mathrm{S}}_0$) to the lowest excited singlet state (${\mathrm{S}}_1$) from a simultaneous fit of the intensities of 74 vibronic bands in five fluorescence emission spectra. The SCS-CC2/cc-pVTZ-optimized ground-state structure was distorted along 12 modes in order to fit the emission intensities. The rotational constants of the resulting excited-state structure are reported in the last column of Table 1. They are close to the ab initio-predicted values for the ${\mathrm{S}}_1$-state from the first column of Table 1.

The bond length changes in the chromophore upon electronic excitation are presented in

Table 2. SCS-CC2/cc-pVTZ- and FC fit-calculated geometry changes in pm of 1,3-DCB.

State	S0		S1		$\mathbf{\Delta }$(S1-S0)
Method	SCS-CC2	FCFit	SCS-CC2	FCFit	SCS-CC2	FCFit
C1-C2	139.4	139.4	142.5	142.0	+3.1	+2.6
C2-C3	140.2	140.2	143.5	143.7	+3.3	+3.5
C3-C4	139.9	139.9	143.9	143.9	+4.0	+4.0
C4-C5	139.9	139.9	143.9	143.9	+4.0	+4.0
C5-C6	140.2	140.2	143.5	143.6	+3.3	+3.4
C6-C1	139.4	139.4	142.5	142.0	+3.1	+2.6
C3-C8	143.8	143.8	141.7	142.2	−2.1	−1.6
C8-N2	117.6	117.6	118.1	118.1	+0.5	+0.5
C5-C7	143.8	143.8	141.7	142.2	−2.1	−1.6
C7-N1	117.6	117.6	118.1	118.2	+0.5	+0.6

and Figure 6. Comparison of the ab initio-predicted bond length changes at the SCS-CC2/cc-pVTZ level and the experimental fit results shows a general good agreement. The changes in bond length are symmetric with respect to the ${\mathrm{C}}_2$ axis and therefore retain the ${\mathrm{C}}_{2v}$-symmetry of the molecule. Both CN bond lengths increase (by 0.5 pm for ab initio and 0.6 pm for FCFit) upon excitation. The CC bond between the aromatic ring and the nitrile group decreases by 1.6 pm (FCFit). All ring CC bond lengths increase between 2.6 and 4.0 pm, which can be expected for a $\pi {\pi }^{*}$ excitation. The largest differences between the ab initio-calculated and experimentally determined ring bond lengths are found for the C(4)-C(5) and C(5)-C(6) bonds. Here, the theoretical and measured bond lengths differ by 5 pm, the former being longer than the latter. It is these differences that can be compensated for by a combination of the modes ${\mathrm{Q}}_{20}$ and ${\mathrm{Q}}_{24}$.

5. Conclusions

FC theory allows for the determination of the geometry changes of 1,3-DCB upon excitation to the lowest excited singlet state. The aromatic ring CC bond lengths increase symmetrically, leading to an overall ring extension. Based on the results of an FC fit of the fluorescence emission spectra of 1,3-DCB, we predict that the changes in the rotational constants upon electronic excitation are $\Delta A$ = −88.4 MHz, $\Delta B$ = −15.6 MHz, and $\Delta C$ = −14.3 MHz. These changes are up to 15% larger than the predicted SCS-CC2/cc-pVTZ changes. The ab initio calculations predict the ${\mathrm{S}}_1$-state to be the ${\mathrm{L}}_b$-state, with a transition dipole moment orientation along the inertial a-axis, i.e., the $S_1\leftarrow S_0$ as pure a-type spectrum. The reported symmetric geometry changes upon electronic excitation support the assignment of the ${\mathrm{S}}_1$-state to an ${\mathrm{L}}_b$-state. The bond length increases in the aromatic ring C-C bonds after electronic excitation are also reflected in the lower vibrational frequencies in the ${\mathrm{S}}_1$-state compared to the ground state.