Silicon Carbide and MRI: Towards Developing a MRI Safe Neural Interface
Abstract
:1. Introduction
2. Materials and Methods
2.1. Sample Microfabrication
2.2. Modeling and Simulation
2.2.1. Magnetic Perturbation Simulations
2.2.2. Finite Element Method Simulations
2.3. MRI Experiments
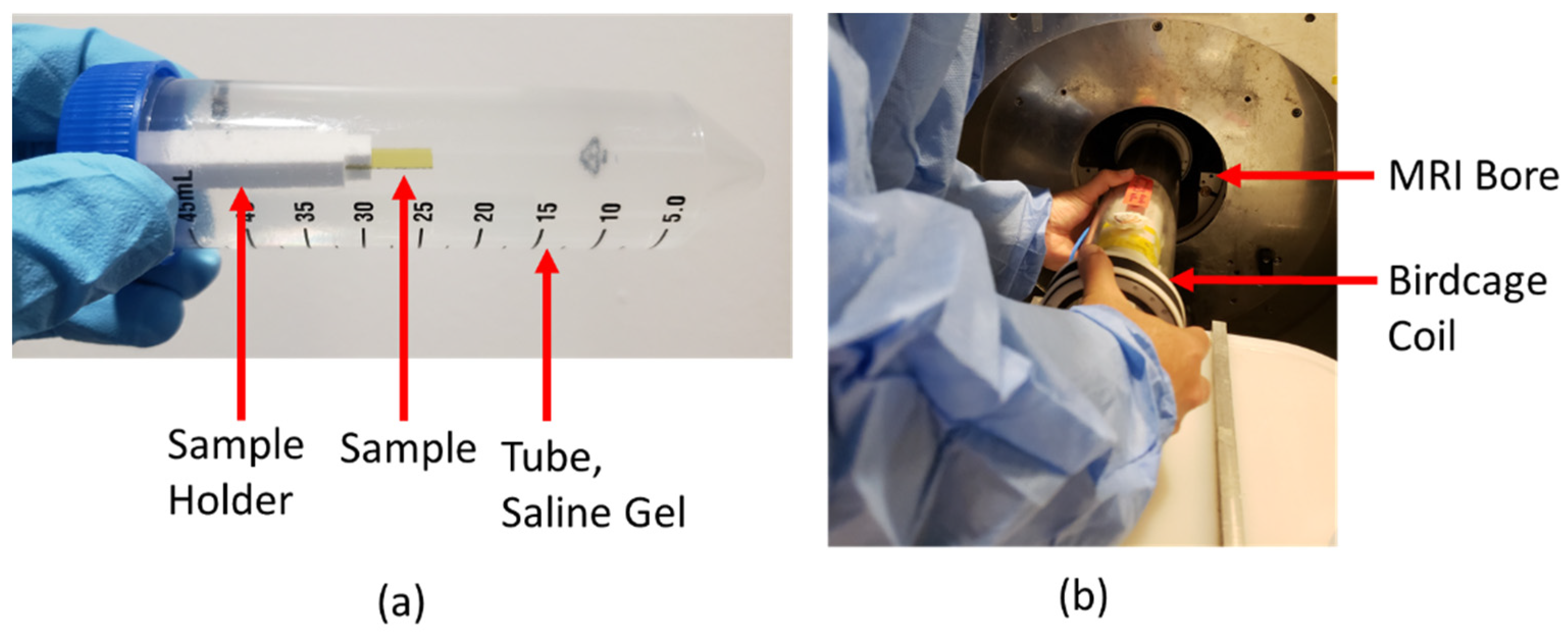
2.3.1. Saline Gel Phantom Preparation
2.3.2. MRI Image Artifact Experiments
2.3.3. MRI Induced Heat Experiments
3. Results
3.1. Image Artifacts
3.2. Induced Heat and SAR
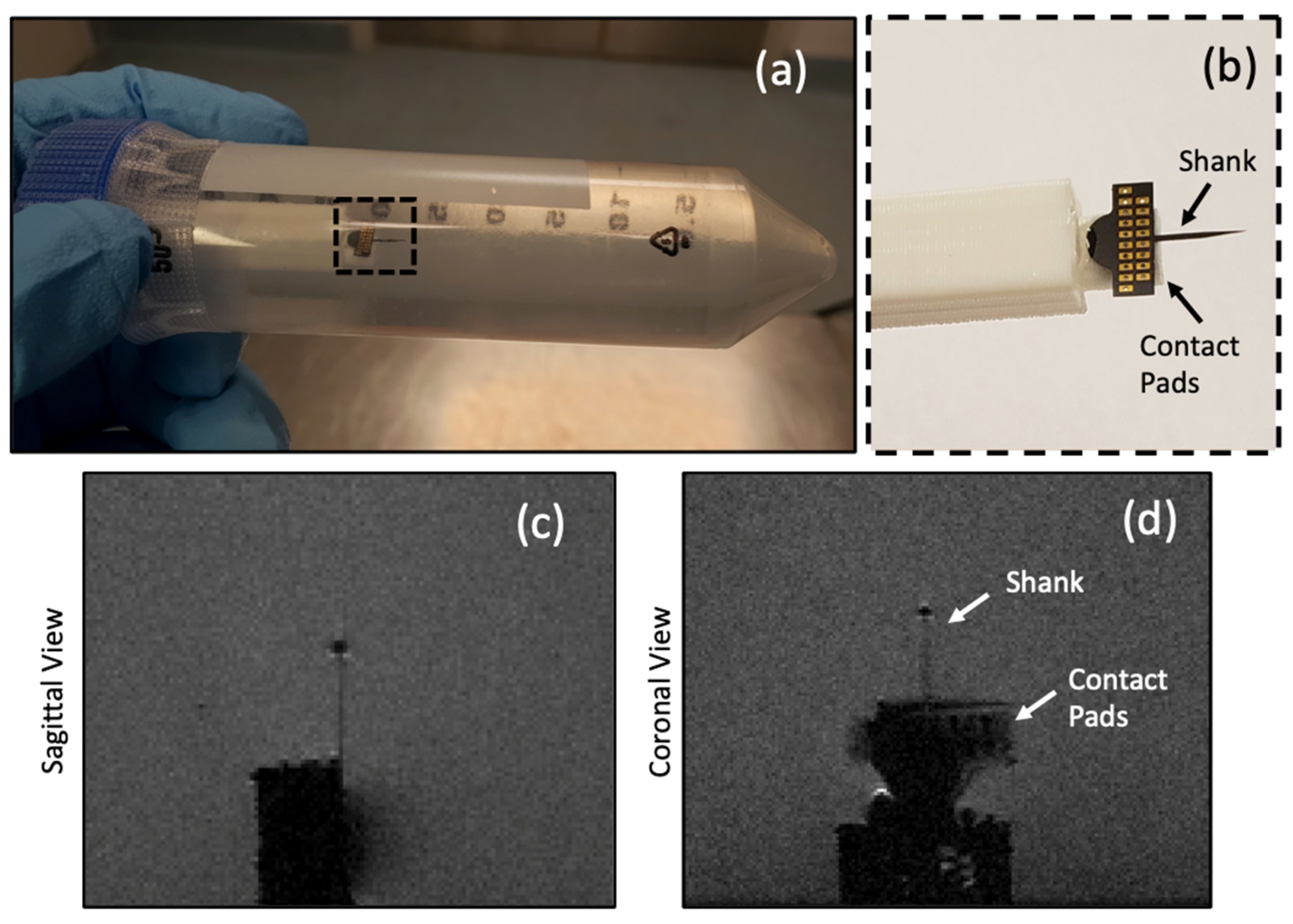
3.3. C-SiC Neural Probe under 7 T MRI
4. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Rad, L.G.; Angelone, L.M.; Kirsch, J.; Downs, S.; Keil, B.; Bonmassar, G.; Wald, L.L. Reducing RF-Induced Heating Near Implanted Leads Through High-Dielectric Capacitive Bleeding of Current (CBLOC). IEEE Trans. Microw. Theory Tech. 2019, 67, 1265–1273. [Google Scholar] [CrossRef]
- Guerin, B.; Serano, P.; Iacono, M.I.; Herrington, T.M.; Widge, A.S.; Dougherty, D.D.; Bonmassar, G.; Angelone, L.M.; Wald, L.L. Realistic modeling of deep brain stimulation implants for electromagnetic MRI safety studies. Phys. Med. Biol. 2018, 63, 095015. [Google Scholar] [CrossRef] [PubMed]
- Nyenhuis, J.; Park, S.-M.; Kamondetdacha, R.; Amjad, A.; Shellock, F.; Rezai, A. MRI and implanted medical devices: Basic interactions with an emphasis on heating. IEEE Trans. Device Mater. Reliab. 2005, 5, 467–480. [Google Scholar] [CrossRef]
- Medtronic PERCEPT™ PC NEUROSTIMULATOR. Available online: https://www.medtronic.com/us-en/healthcare-professionals/products/neurological/deep-brain-stimulation-systems/percept-pc.html (accessed on 20 December 2020).
- Ladd, M.E.; Bachert, P.; Meyerspeer, M.; Moser, E.; Nagel, A.M.; Norris, D.G.; Schmitter, S.; Speck, O.; Nagel, A.M.; Zaiss, M. Pros and cons of ultra-high-field MRI/MRS for human application. Prog. Nucl. Magn. Reson. Spectrosc. 2018, 109, 1–50. [Google Scholar] [CrossRef] [PubMed]
- Erhardt, J.B.; Fuhrer, E.; Gruschke, O.G.; Leupold, J.; Wapler, M.C.; Hennig, J.; Stieglitz, T.; Korvink, J.G. Should patients with brain im-plants undergo MRI? J. Neural. Eng. 2018, 15, 041002. [Google Scholar] [CrossRef] [PubMed]
- Golestanirad, L.; Angelone, L.M.; Iacono, M.I.; Katnani, H.; Wald, L.L.; Bonmassar, G. Local SAR near deep brain stimulation (DBS) electrodes at 64 and 127 MHz: A simulation study of the effect of extracranial loops. Magn. Reson. Med. 2017, 78, 1558–1565. [Google Scholar] [CrossRef] [PubMed]
- Bhusal, B.; Nguyen, B.T.; Sanpitak, P.P.; Vu, J.; Elahi, B.; Rosenow, J.; Nolt, M.J.; Lopez-Rosado, R.; Pilitsis, J.; DiMarzio, M.; et al. Effect of Device Configuration and Patient’s Body Composition on the RF Heating and Nonsusceptibility Artifact of Deep Brain Stimulation Implants During MRI at 1.5 T and 3T. J. Magn. Reson. Imaging 2020, 53, 599–610. [Google Scholar] [CrossRef]
- Panych, L.P.; Madore, B. The physics of MRI safety. J. Magn. Reson. Imaging 2018, 47, 28–43. [Google Scholar] [CrossRef]
- Shellock, F.G. Radiofrequency Energy-Induced Heating During MR Procedures: A Review. J. Magn. Reson. Imaging 2000, 12, 30–36. [Google Scholar] [CrossRef]
- McKinstry, R.C., III; Jarrett, D.Y. Magnetic susceptibility artifacts on MRI: A hairy situation. Am. J. Roentgenol. 2004, 182, 532. [Google Scholar] [CrossRef]
- Schramm, G.; Ladefoged, C.N. Metal artifact correction strategies in MRI-based attenuation correction in PET/MRI. BJR Open 2019, 1, 20190033. [Google Scholar] [CrossRef] [PubMed]
- Scherberger, H.; Fineman, I.; Musallam, S.; Dubowitz, D.J.; Bernheim, K.A.; Pesaran, B.; Corneil, B.D.; Gilliken, B.; Andersen, R.A. Magnetic resonance image-guided implantation of chronic recording electrodes in the macaque intraparietal sulcus. J. Neurosci. Methods 2003, 130, 1–8. [Google Scholar] [CrossRef]
- Nimbalkar, S.; Fuhrer, E.; Silva, P.; Nguyen, T.; Sereno, M.I.; Kassegne, S.; Korvink, J.G. Glassy carbon microelectrodes minimize induced voltages, mechanical vibrations, and artifacts in magnetic resonance imaging. Microsystems Nanoeng. 2019, 5, 1–11. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Oh, S.; Ryu, Y.-C.; Carluccio, G.; Sica, C.T.; Collins, C.M. Measurement of SAR-induced temperature increase in a phantom and in vivo with comparison to numerical simulation. Magn. Reson. Med. 2014, 71, 1923–1931. [Google Scholar] [CrossRef] [Green Version]
- Golestanirad, L.; Iacono, M.I.; Keil, B.; Angelone, L.M.; Bonmassar, G.; Fox, M.D.; Herrington, T.; Adalsteinsson, E.; Lapierre, C.; Mareyam, A.; et al. Construction and modeling of a reconfigurable MRI coil for lowering SAR in patients with deep brain stimulation implants. NeuroImage 2017, 147, 577–588. [Google Scholar] [CrossRef] [Green Version]
- Bottomley, P.A.; Kumar, A.; Edelstein, W.A.; Allen, J.M.; Karmarkar, P.V. Designing passive MRI-safe implantable conducting leads with electrodes. Med. Phys. 2010, 37, 3828–3843. [Google Scholar] [CrossRef]
- Li, D.; Ji, X.; Zheng, J.; Pan, C.; Kainz, W.; Chen, J. A Novel Design of Implantable Esophageal Stent to Reduce the MRI RF-Induced Heating. IEEE Trans. Electromagn. Compat. 2016, 59, 1–8. [Google Scholar] [CrossRef]
- Bottomley, P.A.; Karmarkar, P.V.; Allen, J.M.; Edelstein, W.A. MRI and RF Compatible Leads and Related Methods of Operating and Fabricating Leads. U.S. Patent 10,391,307, 27 August 2019. [Google Scholar]
- Ho, H.S. Safety of metallic implants in magnetic resonance imaging. J. Magn. Reson. Imaging 2001, 14, 472–477. [Google Scholar] [CrossRef]
- Bonmassar, G.; Fujimoto, K.; Golby, A.J. PTFOS: Flexible and Absorbable Intracranial Electrodes for Magnetic Resonance Imaging. PLoS ONE 2012, 7, e41187. [Google Scholar] [CrossRef] [Green Version]
- Mohsin, S.A.; Nyenhuis, J.A.; Masood, R. INTERACTION OF MEDICAL IMPLANTS WITH THE MRI ELECTROMAGNETIC FIELDS. Prog. Electromagn. Res. C 2010, 13, 195–202. [Google Scholar] [CrossRef] [Green Version]
- Yeung, C.J.; Susil, R.C.; Atalar, E. RF safety of wires in interventional MRI: Using a safety index. Magn. Reson. Med. Off. J. Int. Soc. Magn. Reson. Med. 2002, 47, 187–193. [Google Scholar] [CrossRef]
- Zhao, S.; Liu, X.; Xuefeng, F.; Ren, H.; Deng, B.; Tang, M.; Lu, L.; Fu, X.; Peng, H.; Liu, Z.; et al. Graphene Encapsulated Copper Microwires as Highly MRI Compatible Neural Electrodes. Nano Lett. 2016, 16, 7731–7738. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Angelone, L.M.; Ahveninen, J.; Belliveau, J.W.; Bonmassar, G. Analysis of the Role of Lead Resistivity in Specific Absorption Rate for Deep Brain Stimulator Leads at 3T MRI. IEEE Trans. Med. Imaging 2010, 29, 1029–1038. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Park, S.; Kamondetdacha, R.; Amjad, A.; Nyenhuis, J. MRI safety: RF-induced heating near straight wires. IEEE Trans. Magn. 2005, 41, 4197–4199. [Google Scholar] [CrossRef]
- Smith, C.D.; Kildishev, A.V.; Nyenhuis, J.A.; Foster, K.S.; Bourland, J.D. Interactions of magnetic resonance imaging radio frequency magnetic fields with elongated medical implants. J. Appl. Phys. 2000, 87, 6188–6190. [Google Scholar] [CrossRef]
- Nitz, W.R.; Oppelt, A.; Renz, W.; Manke, C.; Lenhart, M.; Link, J. On the heating of linear conductive structures as guide wires and catheters in interventional MRI. J. Magn. Reson. Imaging 2001, 13, 105–114. [Google Scholar] [CrossRef]
- Liu, C.-Y.; Farahani, K.; Lu, D.S.; Duckwiler, G.; Oppelt, A. Safety of MRI-guided endovascular guidewire applications. J. Magn. Reson. Imaging 2000, 12, 75–78. [Google Scholar] [CrossRef]
- Ladd, M.E.; Quick, H.H.; Boesiger, P.; Mckinnon, G.C. RF heating of actively visualized catheters and guidewires. Proc. Intl. Soc. Mag. Reson. Med. 1998, 6, 473. [Google Scholar]
- Golestanirad, L.; Keil, B.; Angelone, L.M.; Bonmassar, G.; Mareyam, A.; Wald, L.L. Feasibility of using linearly polarized rotating birdcage transmitters and close-fitting receive arrays in MRI to reduce SAR in the vicinity of deep brain simulation implants. Magn. Reson. Med. 2017, 77, 1701–1712. [Google Scholar] [CrossRef]
- Martinez-Santiesteban, F.M.; Swanson, S.D.; Noll, D.C.; Anderson, D.J. Magnetic field perturbation of neural recording and stimulating microelectrodes. Phys. Med. Biol. 2007, 52, 2073–2088. [Google Scholar] [CrossRef] [Green Version]
- Saddow, S.E.; Frewin, C.L.; Reyes, M.; Register, J.; Nezafati, M.; Thomas, S. 3C-SiC on Si: A biocompatible material for advanced bioelectronic devices. ECS Trans. 2014, 61, 101–111. [Google Scholar] [CrossRef]
- Saddow, S.E. Silicon Carbide Biotechnology: A Biocompatible Semiconductor for Advanced Biomedical Devices and Applications; Elsevier: Amsterdam, The Netherlands, 2012. [Google Scholar]
- Beygi, M.; Bentley, J.T.; Frewin, C.L.; Kuliasha, C.A.; Takshi, A.; Bernardin, E.K.; La Via, F.; Saddow, S.E. Fabrication of a Monolithic Implantable Neural Interface from Cubic Silicon Carbide. Micromachines 2019, 10, 430. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Frewin, C.L.; Locke, C.; Mariusso, L.; Weeber, E.J.; Saddow, S.E. Silicon Carbide Neural Implants: In Vivo Neural Tissue Reaction. In Proceedings of the 2013 6th International IEEE/EMBS Conference on Neural Engineering (NER), San Diego, CA, USA, 6–8 November 2013; IEEE: Piscataway Township, NJ, USA, 2013; pp. 661–664. [Google Scholar]
- Phan, H.-P.; Zhong, Y.; Nguyen, T.-K.; Park, Y.; Dinh, T.; Song, E.; Vadivelu, R.K.; Masud, M.K.; Li, J.; Shiddiky, M.J.A.; et al. Long-Lived, Transferred Crystalline Silicon Carbide Nanomembranes for Implantable Flexible Electronics. ACS Nano 2019, 13, 11572–11581. [Google Scholar] [CrossRef]
- Knaack, G.L.; McHail, D.G.; Borda, G.; Koo, B.; Peixoto, N.; Cogan, S.F.; Dumas, T.C.; Pancrazio, J.J. In vivo Characterization of Amorphous Silicon Carbide As a Biomaterial for Chronic Neural Interfaces. Front. Neurosci. 2016, 10, 301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Deku, F.; Frewin, C.L.; Stiller, A.M.; Cohen, Y.; Aqeel, S.; Joshi-Imre, A.; Black, B.J.; Gardner, T.J.; Pancrazio, J.J.; Cogan, S.F. Amorphous Silicon Carbide Platform for Next Generation Penetrating Neural Interface Designs. Micromachines 2018, 9, 480. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Knaack, G.L.; Charkhkar, H.; Cogan, S.F.; Pancrazio, J.J. Amorphous Silicon Carbide for Neural Interface Applications; Elsevier BV: Amsterdam, The Netherlands, 2016; pp. 249–260. [Google Scholar]
- Lei, X.; Kane, S.; Cogan, S.; Lorach, H.; Galambos, L.; Huie, P.; Mathieson, K.; Kamins, T.; Harris, J.; Palanker, D. SiC protective coating for photovoltaic retinal prosthesis. J. Neural Eng. 2016, 13, 046016. [Google Scholar] [CrossRef] [Green Version]
- Kimoto, T.; Cooper, J.A. Fundamentals of Silicon Carbide Technology: Growth, Characterization, Devices and Applications; Wiley: Singapore, 2014. [Google Scholar]
- Sarachik, M.P.; He, D.R.; Li, W.; Levy, M.; Brooks, J.S. Magnetic properties of boron-doped silicon. Phys. Rev. B 1985, 31, 1469–1477. [Google Scholar] [CrossRef] [PubMed]
- Schlager, H.G.; Löhneysen, H.V. Susceptibility of local magnetic moments in phosphorus-doped silicon near the met-al-insulator transition. Europhys. Lett. 1997, 40, 661–666. [Google Scholar] [CrossRef]
- Duyn, J.H.; Schenck, J. Contributions to magnetic susceptibility of brain tissue. NMR Biomed. 2017, 30, e3546. [Google Scholar] [CrossRef] [Green Version]
- Maeder, T.; Sagalowicz, L.; Muralt, P. Stabilized Platinum Electrodes for Ferroelectric Film Deposition using Ti, Ta and Zr Adhesion Layers. Jpn. J. Appl. Phys. 1998, 37, 2007–2012. [Google Scholar] [CrossRef]
- Marques, J.; Bowtell, R. Application of a Fourier-based method for rapid calculation of field inhomogeneity due to spatial variation of magnetic susceptibility. Concepts Magn. Reson. Part B Magn. Reson. Eng. 2005, 25, 65–78. [Google Scholar] [CrossRef]
- Bouwman, J.G.; Bakker, C.J.G. Alias subtraction more efficient than conventional zero-padding in the Fourier-based calculation of the susceptibility induced perturbation of the magnetic field in MR. Magn. Reson. Med. 2012, 68, 621–630. [Google Scholar] [CrossRef] [PubMed]
- ASTM F2182-19e2, Standard Test Method for Measurement of Radio Frequency Induced Heating on or Near Passive Implants during Magnetic Resonance Imaging, ASTM International, West Conshohocken, PA. 2019. Available online: www.astm.org (accessed on 25 January 2021).
- Fantasia, M.; Galante, A.; Maggiorelli, F.; Retico, A.; Fontana, N.; Monorchio, A.; Alecci, M. Numerical and Workbench Design of 2.35 T Double-Tuned (¹H/²³Na) Nested RF Birdcage Coils Suitable for Animal Size MRI. IEEE Trans. Med. Imaging 2020, 39, 3175–3186. [Google Scholar] [CrossRef] [PubMed]
- Gubareff, G.G.; Janssen, J.E.; Torborg, R.H. Thermal Radiation Properties Survey: A Review of the Literature; Honeywell Research Center: Minneapolis, MN, USA, 1960. [Google Scholar]
- Lide, D.R. (Ed.) CRC Handbook of Chemistry and Physics; CRC Press: Boca Raton, FL, USA, 2004; Volume 85. [Google Scholar]
- Sasaki, W.; Kinoshita, J. Piezoresistance and Magnetic Susceptibility in Heavily Doped n-Type Silicon. J. Phys. Soc. Jpn. 1968, 25, 1622–1629. [Google Scholar] [CrossRef]
- Frewin, C.L.; Register, J.J.; Saddow, S.E.; Reyes-Natal, M.; Pancrazio, J.J. Magnetic Resonant Imaging Safe Stylus. U.S. Patent 10,046,165, 14 August 2018. [Google Scholar]

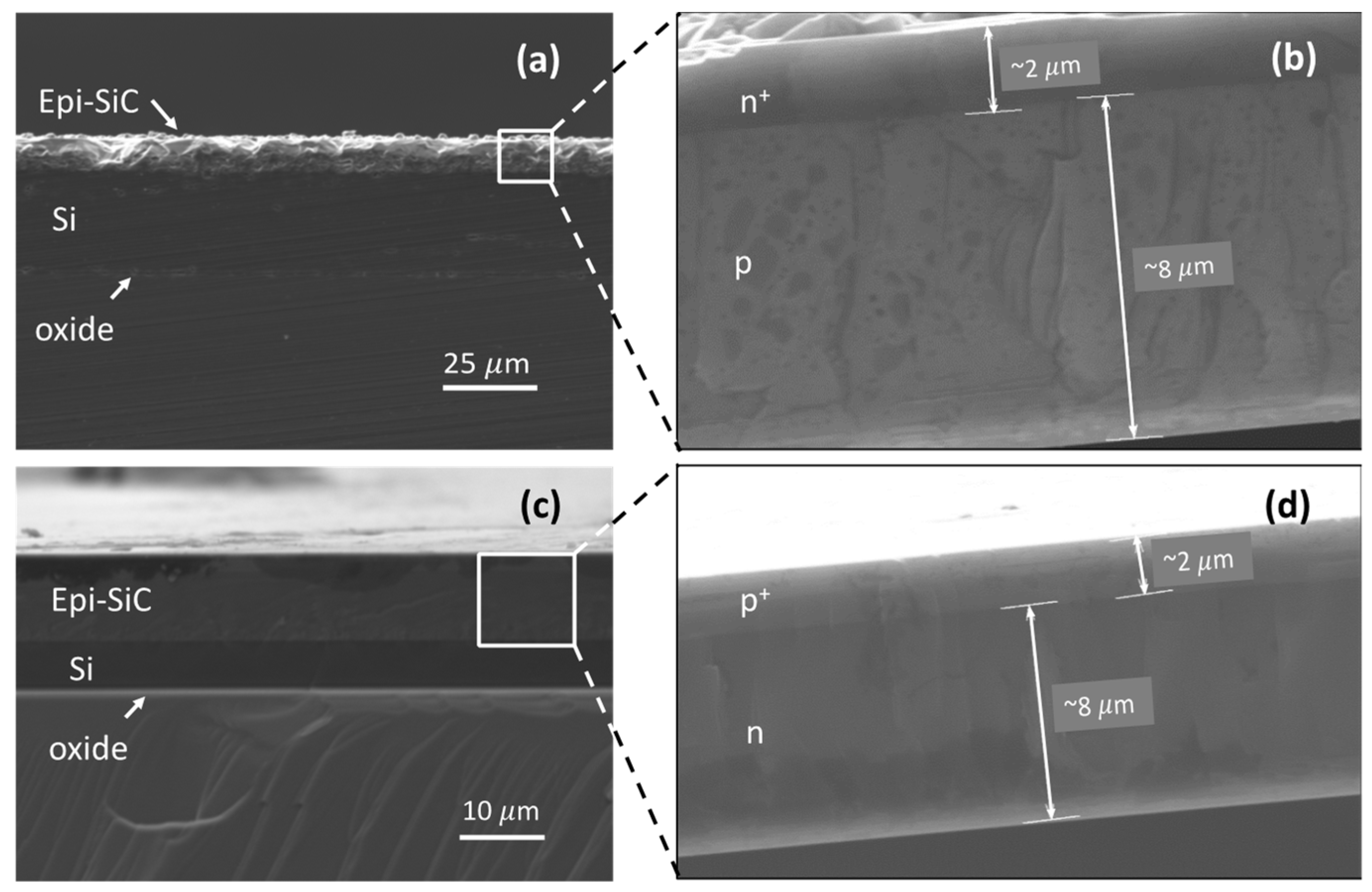





| Material | Electric Conductivity (×106 S/m) | Thermal Conductivity (W/m-K) | Magnetic Susceptibility χv (ppm) |
|---|---|---|---|
| Human Tissue | 0.5 × 10−6 | 0.4 | –9.05 |
| Doped SiC (~1019 dopants/cm3) | ~0.17 | 360 | –12.87 |
| Doped Si (~1018 dopants/cm3) | ~0.1 | 148 | –4.2 |
| Platinum | 9.3 | 71 | 279 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Beygi, M.; Dominguez-Viqueira, W.; Feng, C.; Mumcu, G.; Frewin, C.L.; La Via, F.; Saddow, S.E. Silicon Carbide and MRI: Towards Developing a MRI Safe Neural Interface. Micromachines 2021, 12, 126. https://doi.org/10.3390/mi12020126
Beygi M, Dominguez-Viqueira W, Feng C, Mumcu G, Frewin CL, La Via F, Saddow SE. Silicon Carbide and MRI: Towards Developing a MRI Safe Neural Interface. Micromachines. 2021; 12(2):126. https://doi.org/10.3390/mi12020126
Chicago/Turabian StyleBeygi, Mohammad, William Dominguez-Viqueira, Chenyin Feng, Gokhan Mumcu, Christopher L. Frewin, Francesco La Via, and Stephen E. Saddow. 2021. "Silicon Carbide and MRI: Towards Developing a MRI Safe Neural Interface" Micromachines 12, no. 2: 126. https://doi.org/10.3390/mi12020126
APA StyleBeygi, M., Dominguez-Viqueira, W., Feng, C., Mumcu, G., Frewin, C. L., La Via, F., & Saddow, S. E. (2021). Silicon Carbide and MRI: Towards Developing a MRI Safe Neural Interface. Micromachines, 12(2), 126. https://doi.org/10.3390/mi12020126









