Statistical Optimisation of Chemical Stability of Hybrid Microwave-Sintered Alumina Ceramics in Nitric Acid
Abstract
:1. Introduction
2. Materials and Methods
2.1. Preparation of Alumina Ceramics
2.2. Characterisation of Alumina Ceramics
2.3. Monitoring of Alumina Corrosion in an Aqueous Solution of Nitric Acid
2.4. Design of Experiments of Monitored Alumina Corrosion Resistance
3. Results and Discussion
3.1. Properties of Alumina Ceramics
| Sample | ρ, g cm−3 | HV1 | c/a | KIC, MPa m1/2 | ||
|---|---|---|---|---|---|---|
| Casellas [48] | Niihara et al. [48] | Shetty et al. [48] | ||||
| Al2O3 | 3.784 ± 0.028 | 1815 ± 51 | 1.96 ± 0.26 | 6.45 ± 1.43 | 4.91 ± 0.81 | 5.15 ± 0.89 |
3.2. Modelling of the Amount of Eluted Ions and Alumina Density
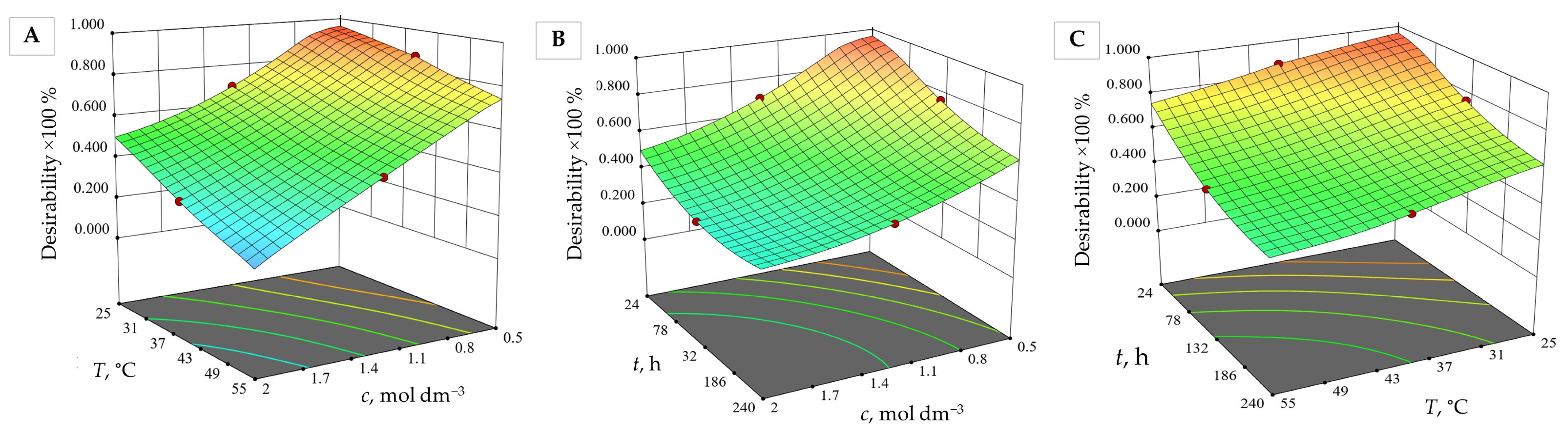
3.3. Optimisation and Verification of Alumina Ceramics Corrosion Resistance in Nitric Acid
4. Conclusions
- The Box–Behnken design was applied to conduct an experiment on the chemical stability of alumina ceramics sintered by means of a hybrid microwave sintering process.
- The corrosion resistance of alumina ceramics to 0.5, 1.25, and 2.00 mol dm−3 nitric acid at 25, 40, and 55 °C for up to 10 days was investigated.
- The inverse behaviour of the density values in relation to the amounts of eluted ions was demonstrated by regression models accompanied by surface plots.
- The optimal corrosion resistance parameters obtained for the investigated alumina are the minimum exposure time (24 h) of alumina to the 0.50 mol dm−3 nitric acid at 25 °C with a desirability of 96%. After 24 h of alumina exposure to the 0.50 mol dm−3 nitric acid at 40 °C, a second optimum, with a lower, but still acceptable desirability (88%) occurred.
- The amounts of eluted ions from the alumina ceramics are in the following order: Fe3+ < Mg2+ < Ca2+ < Na+ < Al3+.
- The highest amounts of eluted ions, Al3+ (14.805 µg cm−2), Ca2+ (7.079 µg cm−2), Fe3+ (0.361 µg cm− 2), Mg2+ (3.654 µg cm−2), and Na+ (13.261 µg cm−2), were obtained at 55 °C in 2 mol dm−3 nitric acid. The amount of eluted Si4+ ions is below the detection limit of ICP-AES.
- The change in alumina ceramic density during the corrosion test was negligible.
- In general, it can be concluded that the corrosion of alumina ceramics sintered in hybrid microwave kilns shows good chemical stability under test conditions. Corrosion can be mostly attributed to the dissolution of segregated impurities (calcium oxide, ferric oxide, sodium oxide, and silicon dioxide) and the sintering aid (magnesium oxide) from the grain boundaries of the alumina ceramics.
- This study demonstrated that an unconventional sintering process (hybrid microwave) can produce high-purity alumina ceramics with a favourable microstructure for use in a corrosive nitric acid medium.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Barsoum, M.W. Fundamentals of Ceramics, 2nd ed.; Institute of Physics Publishing: Bristol, UK; Philadephia, PA, USA, 2003; ISBN 978103233. [Google Scholar]
- Figiel, P.; Rozmus, M.; Smuk, B. Properties of Alumina Ceramics Obtained by Conventional and Non-Conventional Methods for Sintering Ceramics. J. Achiev. Mater. Manuf. Eng. 2011, 48, 29–34. [Google Scholar]
- Kachaev, A.A.; Grashchenkov, D.V.; Lebedeva, Y.E.; Solntsev, S.S.; Khasanov, O.L. Optically Transparent Ceramic (Review). Glas. Ceram. 2016, 73, 117–123. [Google Scholar] [CrossRef]
- Ayode Otitoju, T.; Ugochukwu Okoye, P.; Chen, G.; Li, Y.; Onyeka Okoye, M.; Li, S. Advanced Ceramic Components: Materials, Fabrication, and Applications. J. Ind. Eng. Chem. 2020, 85, 34–65. [Google Scholar] [CrossRef]
- Rahaman, M.N. Sintering of Ceramics, 1st ed.; CRC Press: Boca Raton, MA, USA, 2007; ISBN 9781420007053. [Google Scholar]
- Rybakov, K.; Olevsky, A.E.; Krikun, V.E. Microwave Sintering: Fundamentals and Modeling. J. Am. Ceram. Soc. 2013, 96, 1003–1020. [Google Scholar] [CrossRef]
- Curto, H.; Thuault, A.; Jean, F.; Violier, M.; Dupont, V.; Hornez, J.C.; Leriche, A. Coupling Additive Manufacturing and Microwave Sintering: A Fast Processing Route of Alumina Ceramics. J. Eur. Ceram. Soc. 2020, 40, 2548–2554. [Google Scholar] [CrossRef]
- Ropuš, I.; Ćurković, L.; Mandić, V.; Mustafa, M.K.; Gabelica, I. Conventional and Non-Conventional Sintering Techniques of High Purity Alumina Ceramics. Tech. Gaz. 2021, 28, 1526–1531. [Google Scholar]
- Ćurković, L.; Ropuš, I.; Žmak, I.; Gabelica, I.; Švagelj, Z. Comparison of Mechanical Properties of Conventionally and Non-Conventionally Sintered Cold Isostatically Pressed Al2O3 Ceramics. Eng. Power Bull. Croat. Acad. Eng. 2021, 16, 7–15. [Google Scholar]
- Gavrilov, K.L.; Bennison, S.J.; Mikeska, K.R.; Levi-Setti, R. Grain Boundary Chemistry of Alumina by High-Resolution Imaging SIMS. Acta Mater. 1999, 47, 4031–4039. [Google Scholar] [CrossRef]
- Ćurković, L.; Fudurić Jelača, M. Dissolution of Alumina Ceramics in HCl Aqueous Solution. Ceram. Int. 2009, 35, 2041–2045. [Google Scholar] [CrossRef]
- Medvedovski, E. Influence of Corrosion and Mechanical Loads on Advanced Ceramic Components. Ceram. Int. 2013, 39, 2723–2741. [Google Scholar] [CrossRef]
- Sudha, P.N.; Sangeetha, K.; Jisha Kumari, A.V.; Vanisri, N.; Rani, K. Corrosion of Ceramic Materials. In Fundamental Biomaterials: Ceramics; Elsevier: Amsterdam, The Netherlands, 2018; pp. 223–250. ISBN 9780081022047. [Google Scholar]
- Gogotsi, Y.G.; Lavrenko, V.A. Corrosion of High-Performance Ceramics, 1st ed.; Kubler, A., Ed.; Springer: Berlin/Heidelberg, Germany, 1992; ISBN 9783642773921. [Google Scholar]
- Breviary Technical Ceramics; 2004; ISBN 3-924158-77-0. Available online: https://www.nonmet.mat.ethz.ch/education/courses/Materialwissenschaft_2/brevier.pdf (accessed on 4 December 2022).
- Schacht, M.; Boukis, N.; Dinjus, E. Corrosion of Alumina Ceramics in Acidic Aqueous Solutions at High Temperatures and Pressures. J. Mater. Sci. 2000, 5, 6251–6258. [Google Scholar] [CrossRef]
- Schilm, J.; Gruner, W.; Herrmann, M.; Michael, G. Corrosion of Si3N4-Ceramics in Aqueous Solutions Part I: Characterisation of Starting Materials and Corrosion in 1N H2SO4. J. Eur. Ceram. Soc. 2006, 26, 3909–3917. [Google Scholar] [CrossRef]
- Wang, Z.G.B.; Yin, J. Corrosion Behavior of Alumina - Based Ceramic Core Materials in Caustic Alkali Solutions. Int. J. Appl. Ceram. Technol. 2019, 16, 335–345. [Google Scholar]
- Gutiérrez, A.V.; Cuevas, J.L.; Ángeles, A.G.; Pilalua, N. Addition of Ceramics Materials to Improve the Corrosion Resistance of Alumina Refractories. SN Appl. Sci. 2019, 1, 784. [Google Scholar] [CrossRef]
- Herrmann, M.; Toma, F.L.; Berger, L.M.; Kaiser, G.; Stahr, C.C. Comparative Study of the Corrosion Resistance of Thermally Sprayed Ceramic Coatings and Their Bulk Ceramic Counterparts. J. Eur. Ceram. Soc. 2014, 34, 493–504. [Google Scholar] [CrossRef]
- Sato, T.; Tokunaga, Y.; Endo, T.; Shimada, M.; Komeya, K.; Nishida, K.; Komatsu, M.; Kameda, T. Corrosion of Silicon Nitride Ceramics in Aqueous HF Solutions. J. Mater. Sci. 1988, 23, 3440–3446. [Google Scholar] [CrossRef]
- Mikeska, K.R.; Bennison, S.J.; Grise, S.L. Corrosion of Ceramics in Aqueous Hydrofluoric Acid. J. Am. Ceram. Soc. 2000, 83, 1160–1164. [Google Scholar] [CrossRef]
- Ćurković, L.; Fudurić Jelača, M.; Kurajica, S. Corrosion Behavior of Alumina Ceramics in Aqueous HCl and H2SO4 Solutions. Corros. Sci. 2008, 50, 872–878. [Google Scholar] [CrossRef]
- Ropuš, I.; Ćurkoviić, L.; Cajner, H.; Rončević, S. Optimization of Alumina Ceramics Corrosion Resistance in Nitric Acid. Materials 2022, 15, 2579. [Google Scholar] [CrossRef]
- Mäkelä, M. Experimental Design and Response Surface Methodology in Energy Applications: A Tutorial Review. Energy Convers. Manag. 2017, 151, 630–640. [Google Scholar] [CrossRef]
- Keramat, E.; Hashemi, B. Modelling and Optimizing the Liquid Phase Sintering of Alumina/CaO–SiO2–Al2O3 Ceramics Using Response Surface Methodology. Ceram. Int. 2021, 47, 3159–3172. [Google Scholar] [CrossRef]
- Unal, F.; Kaya, F. Modelling of Relation between Synthesis Parameters and Average Crystallite Size of Yb2O3 Nanoparticles Using Box-Behnken Design. Ceram. Int. 2020, 46, 26800–26808. [Google Scholar] [CrossRef]
- Alam, M.A.; Ya, H.H.; Yusuf, M.; Sivraj, R.; Mamat, O.B.; Sapuan, S.M.; Masood, F.; Parveez, B.; Sattar, M. Modeling, Optimization and Performance Evaluation of Tic/Graphite Reinforced Al 7075 Hybrid Composites Using Response Surface Methodology. Materials 2021, 14, 4703. [Google Scholar] [CrossRef]
- Montgomery, D.C. Design and Analysis of Experiments, 8th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2013; ISBN 978-1-118-14692-7. [Google Scholar]
- Ferreira, S.L.C.; Bruns, R.E.; Ferreira, H.S.; Matos, G.D.; David, J.M.; Brandão, G.C.; da Silva, E.G.P.; Portugal, L.A.; dos Reis, P.S.; Souza, A.S.; et al. Box-Behnken Design: An Alternative for the Optimization of Analytical Methods. Anal. Chim. Acta 2007, 597, 179–186. [Google Scholar] [CrossRef] [PubMed]
- Chaker, H.; Ameur, N.; Saidi-Bendahou, K.; Djennas, M.; Fourmentin, S. Modeling and Box- Behnken design optimization of photocatalytic parameters for efficient removal of dye by lanthanum-doped mesoporous TiO2. J. Environ. Chem. Eng. 2020, 9, 104584. [Google Scholar] [CrossRef]
- Cho, K.; Min, J.; Lee, H.; Kim, H.; Kim, H. Reducing the density deviation in alumina by pressure-vacuum hybrid slip casting by employing powders with different particle sizes. J. Asian Ceram. Soc. 2020, 8, 407–415. [Google Scholar] [CrossRef] [Green Version]
- Anstis, G.R.; Chantikul, P.; Lawn, B.R.; Marshall, D.B. A Critical Evaluation of Indentation Techniques for Measuring Fracture Toughness: I, Direct Crack Measurements. J. Am. Ceram. Soc. 1981, 64, 533–538. [Google Scholar] [CrossRef]
- Casellas, D.; Nagl, M.M.; Llanes, L.; Anglada, M. Growth of Small Surface Indentation Cracks in Alumina and Zirconia Toughened Alumina. Key Eng. Mater. 1997, 127–131, 895–902. [Google Scholar] [CrossRef]
- Casellas, D.; Nagl, M.M.; Llanes, L.; Anglada, M. Microstructural Coarsening of Zirconia-Toughened Alumina Composites. J. Am. Ceram. Soc. 2005, 88, 1958–1963. [Google Scholar] [CrossRef]
- Moraes, M.C.C.D.S.; Elias, C.N.; Duailibi Filho, J.; Oliveira, L.G.D. Mechanical Properties of Alumina-Zirconia Composites for Ceramic Abutments. Mater. Res. 2004, 7, 643–649. [Google Scholar] [CrossRef]
- Souza, A.L.; Lemos, S.G.; Oliveira, P.V. A Method for Ca, Fe, Ga, Na, Si and Zn Determination in Alumina by Inductively Coupled Plasma Optical Emission Spectrometry after Aluminum Precipitation. Spectrochim. Acta—Part B At. Spectrosc. 2011, 66, 383–388. [Google Scholar] [CrossRef]
- Anfar, Z.; Ait Ahsaine, H.; Zbair, M.; Amedlous, A.; Ait El Fakir, A.; Jada, A.; El Alem, N. Recent Trends on Numerical Investigations of Response Surface Methodology for Pollutants Adsorption onto Activated Carbon Materials: A Review. Crit. Rev. Environ. Sci. Technol. 2020, 50, 1043–1084. [Google Scholar] [CrossRef]
- Kurajica, S.; Mandić, V.; Ćurković, L. Mullite Ceramics Acid Corrosion Kinetics as a Function of Gel Homogeneity. Biointerface Res. Appl. Chem. 2017, 7, 2295–2299. [Google Scholar]
- Kurajica, S.; Ćurković, L.; Tkalčec, E.; Mandić, V. Acid Corrosion Behavior of Sol—Gel-Prepared Mullite Ceramics with and without Addition of Lanthanum. J. Am. Ceram. Soc. 2013, 96, 923–927. [Google Scholar] [CrossRef]
- Mendelson, M.I. Average Grain Size in Polycrystalline Ceramics. J. Am. Ceram. Soc. 1969, 52, 443–446. [Google Scholar] [CrossRef]
- Wurst, J.C.; Nelson, J.A. Lineal Intercept Technique for Measuring Grain Size in Two-phase Polycrystalline Ceramics. J. Am. Ceram. Soc. 1972, 55, 109. [Google Scholar] [CrossRef]
- Bundschuh, W.; Zimmerlin, B. Effect of Grain Size on Friction and Sliding Wear of Oxide Ceramics. Wear 1993, 162–164, 269–279. [Google Scholar]
- Zuo, F.; Badev, A.; Saunier, S.; Goeuriot, D.; Heuguet, R.; Marinel, S. Microwave versus Conventional Sintering: Estimate of the Apparent Activation Energy for Densification of α-Alumina and Zinc Oxide. J. Eur. Ceram. Soc. 2014, 34, 3103–3110. [Google Scholar] [CrossRef]
- Madhan, M.; Prabhakaran, G. Microwave versus Conventional Sintering: Microstructure and Mechanical Properties of Al2O3—SiC Ceramic Composites. Boletín la Soc. Española Cerámica y Vidr. 2019, 58, 14–22. [Google Scholar] [CrossRef]
- Chinn, R.E. Ceramography; Preparation and Analysis of Ceramic Microstructures, 1st ed.; ASM International: Materials Park, OH, USA, 2002; ISBN 0871707705. [Google Scholar]
- Žmak, I.; Ćorić, D.; Mandić, V.; Ćurković, L. Hardness and Indentation Fracture Toughness of Slip Cast Alumina and Alumina-Zirconia Ceramics. Materials 2020, 13, 122. [Google Scholar] [CrossRef] [Green Version]
- Kokubo, T. Bioceramics and Their Clinical Applications, 1st ed.; Woodhead Publishing Limited: Abingdon, UK, 2008; ISBN 9781845694227. [Google Scholar]
- Almeida, M.; Erthal, R.; Padua, E.; Silveira, L.; Am, L. Response Surface Methodology (RSM) as a Tool for Optimization in Analytical Chemistry. Talanta 2008, 76, 965–977. [Google Scholar]
- Ngan, C.L.; Basri, M.; Lye, F.F.; Fard Masoumi, H.R.; Tripathy, M.; Abedi Karjiban, R.; Abdul-Malek, E. Comparison of Box-Behnken and Central Composite Designs in Optimization of Fullerene Loaded Palm-Based Nano-Emulsions for Cosmeceutical Application. Ind. Crops Prod. 2014, 59, 309–317. [Google Scholar] [CrossRef]
- Greenwood, N.N.; Earnshaw, A. Chemistry of the Elements, 2nd ed.; Butterworth-Heinemann: Oxford, UK, 2012; ISBN 9780750633659. [Google Scholar]


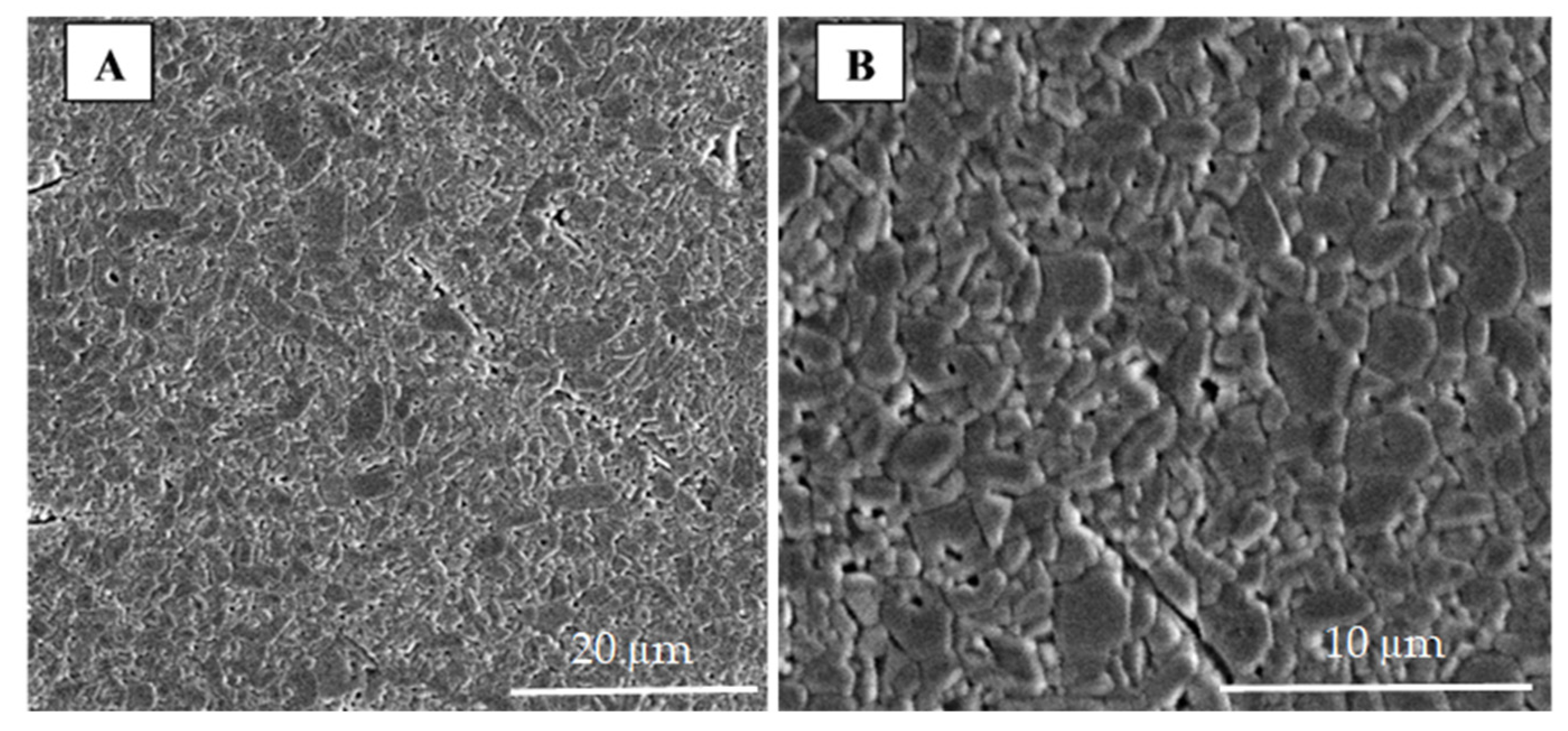
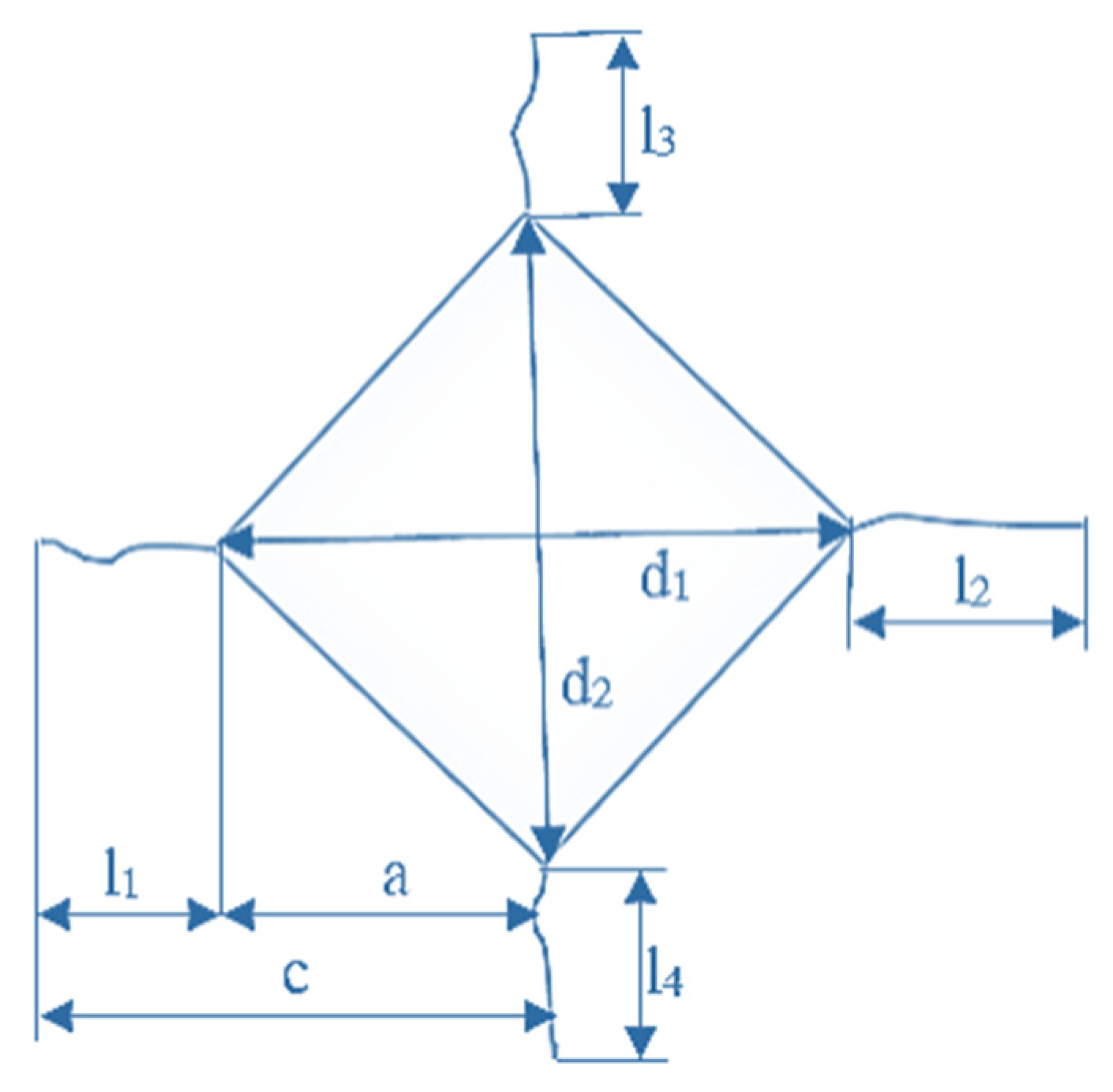

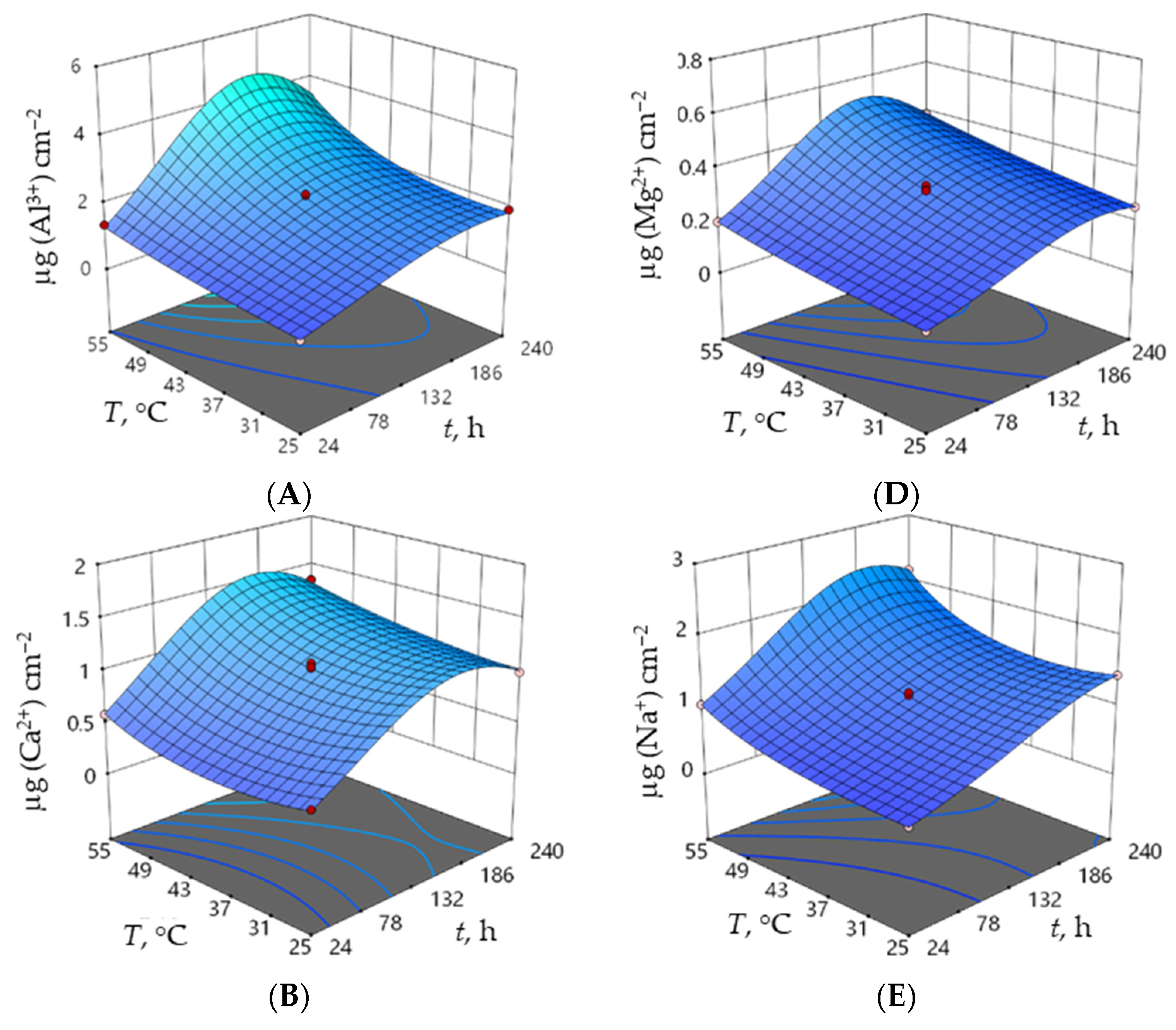

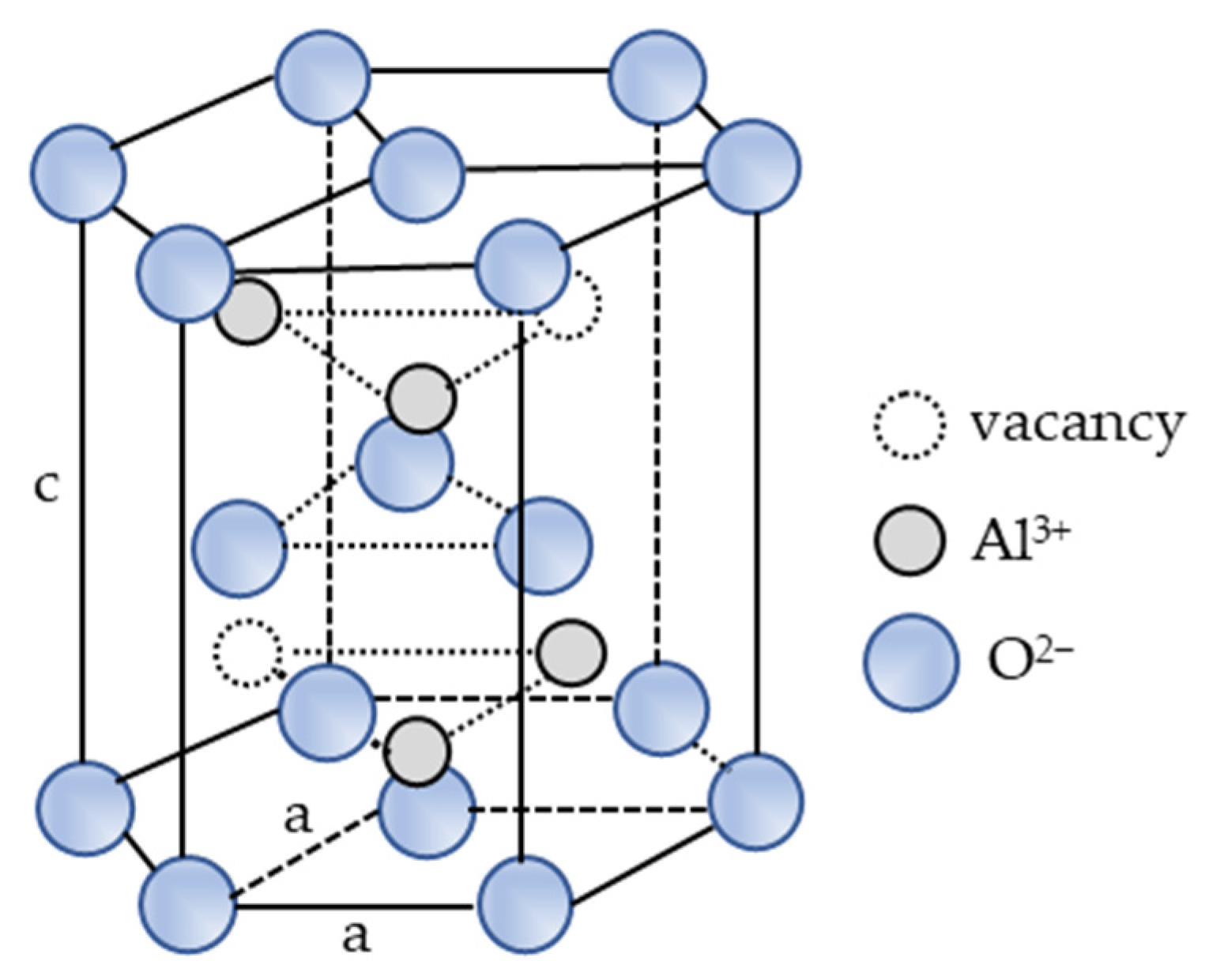
| Component | Fe2O3 | CaO | SiO2 | MgO | Na2O | Al2O3 |
|---|---|---|---|---|---|---|
| wt.% | 0.018 | 0.02 | 0.0325 | 0.045 | 0.05 | balance |
| Independent Variable | −1 Level | 0 | +1 Level |
|---|---|---|---|
| c (HNO3), mol dm−3 | 0.50 | 1.25 | 2.00 |
| T, °C | 25 | 40 | 55 |
| t, h | 24 | 132 | 240 |
| No | c (HNO3), mol dm−3 | T, °C | t, h |
|---|---|---|---|
| 1 | 0.50 | 55 | 132 |
| 2 | 2.00 | 55 | 132 |
| 3 | 1.25 | 25 | 240 |
| 4 | 1.25 | 40 | 132 |
| 5 | 0.50 | 40 | 240 |
| 6 | 1.25 | 40 | 132 |
| 7 | 1.25 | 40 | 132 |
| 8 | 1.25 | 40 | 132 |
| 9 | 1.25 | 40 | 132 |
| 10 | 0.50 | 40 | 24 |
| 11 | 1.25 | 25 | 24 |
| 12 | 2.00 | 25 | 132 |
| 13 | 1.25 | 55 | 240 |
| 14 | 2.00 | 40 | 240 |
| 15 | 0.50 | 25 | 132 |
| 16 | 2.00 | 40 | 24 |
| 17 | 1.25 | 55 | 24 |
| Run | c, | T, | t, | Al3+, | Ca2+, | Fe3+, | Mg2+, | Na+, | ρ, |
|---|---|---|---|---|---|---|---|---|---|
| mol dm−3 | °C | h | µg cm−2 | µg cm−2 | µg cm−2 | µg cm−2 | µg cm−2 | g cm−3 | |
| 1 | 0.50 | 55 | 132 | 0.775 | 0.690 | 0.021 | 0.113 | 0.910 | 3.781 |
| 2 | 2.00 | 55 | 132 | 14.805 | 7.079 | 0.361 | 3.654 | 13.261 | 3.767 |
| 3 | 1.25 | 25 | 240 | 1.918 | 0.990 | 0.029 | 0.253 | 1.440 | 3.783 |
| 4 | 1.25 | 40 | 132 | 2.146 | 0.982 | 0.025 | 0.311 | 1.079 | 3.772 |
| 5 | 0.50 | 40 | 240 | 0.763 | 0.731 | 0.015 | 0.100 | 0.892 | 3.776 |
| 6 | 1.25 | 40 | 132 | 2.189 | 1.004 | 0.024 | 0.310 | 1.128 | 3.779 |
| 7 | 1.25 | 40 | 132 | 2.239 | 1.040 | 0.024 | 0.315 | 1.135 | 3.774 |
| 8 | 1.25 | 40 | 132 | 2.294 | 1.029 | 0.023 | 0.335 | 1.151 | 3.779 |
| 9 | 1.25 | 40 | 132 | 2.338 | 1.079 | 0.023 | 0.316 | 1.189 | 3.769 |
| 10 | 0.50 | 40 | 24 | 0.310 | 0.101 | 0.006 | 0.038 | 0.250 | 3.785 |
| 11 | 1.25 | 25 | 24 | 0.645 | 0.463 | 0.011 | 0.095 | 0.472 | 3.792 |
| 12 | 2.00 | 25 | 132 | 3.982 | 1.301 | 0.041 | 0.495 | 1.839 | 3.796 |
| 13 | 1.25 | 55 | 240 | 3.352 | 1.340 | 0.040 | 0.388 | 2.154 | 3.771 |
| 14 | 2.00 | 40 | 240 | 3.633 | 2.144 | 0.075 | 0.841 | 2.221 | 3.771 |
| 15 | 0.50 | 25 | 132 | 0.402 | 0.618 | 0.008 | 0.067 | 0.396 | 3.809 |
| 16 | 2.00 | 40 | 24 | 1.690 | 1.688 | 0.025 | 0.663 | 1.415 | 3.778 |
| 17 | 1.25 | 55 | 24 | 1.353 | 0.582 | 0.019 | 0.195 | 1.014 | 3.784 |
| Source | Sum of Squares | df | Mean Square | F-Value | p-Value(Prob > F) |
|---|---|---|---|---|---|
| Model | 14.06 | 10 | 1.41 | 477.06 | <0.0001 * |
| A-Concentration | 2.65 | 1 | 2.65 | 900.17 | <0.0001 |
| B-Temperature | 0.97 | 1 | 0.97 | 329.43 | <0.0001 |
| C-Time | 0.69 | 1 | 0.69 | 235.56 | <0.0001 |
| AB | 0.11 | 1 | 0.11 | 36.54 | 0.0009 |
| A2 | 0.18 | 1 | 0.18 | 62.07 | 0.0002 |
| B2 | 0.07 | 1 | 0.07 | 23.96 | 0.0027 |
| C2 | 1.07 | 1 | 1.07 | 364.30 | <0.0001 |
| AB2 | 0.49 | 1 | 0.49 | 167.27 | <0.0001 |
| B2C | 0.01 | 1 | 0.01 | 4.65 | 0.0745 |
| BC2 | 0.06 | 1 | 0.06 | 19.14 | 0.0047 |
| Residual | 0.018 | 6 | 0.003 | ||
| Lack of Fit | 0.013 | 2 | 0.007 | 5.41 | 0.0728 ** |
| Pure Error | 0.005 | 4 | 0.001 | ||
| Cor Total | 14.07 | 16 |
| Response | Regression Equations |
|---|---|
| ln (µg Al3+ cm−2) | 0.81 + 0.81A + 0.49B + 0.42C + 0.16AB − 0.21A2 + 0.13B2 − 0.51C2 + 0.50AB2 + 0.08B2C − 0.17BC2 |
| log (µg Ca2+ cm−2) | 0.011 + 0.42A + 0.06B + 0.24C + 0.17AB − 0.19AC + 0.05A2 + 0.09B2 − 0.21C2 + 0.14A2B − 0.09AB2 − 0.07B2C |
| (µg Fe3+ cm−2)−0.5 | 6.49 − 2.89A − 0.81B − 1.54C + 0.31AB + 0.56AC + 0.36BC + 0.32A2 − 0.59B2 + 1.06C2− 1.14A2B − 0.38A2C |
| (µg Mg2+ cm−2)−0.5 | 1.78 − 1.50A − 0.34B − 0.48C + 0.46AC + 0.15BC + 0.40A2 + 0.48C2 − 0.10A2B − 0.05A2C + 0.28AB2 |
| (µg Na+ cm−2)−0.5 | 0.94 − 0.40A − 0.15B − 0.23C + 0.19AC + 0.08BC + 0.06A2 − 0.09B2 + 0.14C2 − 0.10A2B − 0.05A2C |
| ρ | 3.78 − 0.01A − 0.01B − 0.01C + 0.01B2 |
| Cation | Al3+ | Na+ | Ca2+ | Mg2+ | Fe3+ | Si4+ |
|---|---|---|---|---|---|---|
| rion, pm | 53.5 | 102 | 100 | 72 | 64.5 | 40 |
| No. of Verifications | Response | Experimental Values | Mean Predicted Value | Low CI (95%) | High CI (95%) |
|---|---|---|---|---|---|
| 1 | Experimental parameters: 0.50 mol dm−3 HNO3, 25 °C, 132 h | ||||
| µg (Al3+) cm−2 | 0.402 | 0.402 | 0.352 | 0.460 | |
| µg (Ca2+) cm−2 | 0.618 | 0.618 | 0.564 | 0.678 | |
| µg (Fe3+) cm−2 | 0.008 | 0.008 | 0.007 | 0.008 | |
| µg (Mg2+) cm−2 | 0.067 | 0.067 | 0.066 | 0.069 | |
| µg (Na+) cm−2 | 0.396 | 0.411 | 0.388 | 0.437 | |
| ρ, g cm−3 | 3.809 | 3.800 | 3.792 | 3.807 | |
| 2 | Experimental parameters: 0.50 mol dm−3 HNO3, 40 °C, 240 h | ||||
| µg (Al3+) cm−2 | 0.763 | 0.738 | 0.658 | 0.829 | |
| µg (Ca2+) cm−2 | 0.731 | 0.731 | 0.667 | 0.802 | |
| µg (Fe3+) cm−2 | 0.015 | 0.015 | 0.014 | 0.016 | |
| µg (Mg2+) cm−2 | 0.100 | 0.099 | 0.095 | 0.103 | |
| µg (Na+) cm−2 | 0.892 | 0.878 | 0.795 | 0.974 | |
| ρ, g cm−3 | 3.776 | 3.776 | 3.769 | 3.783 | |
| 3 | Experimental parameters: 1.25 mol dm−3 HNO3, 25 °C, 240 h | ||||
| µg (Al3+) cm−2 | 1.918 | 1.833 | 1.636 | 2.059 | |
| µg (Ca2+) cm−2 | 0.990 | 1.009 | 0.932 | 1.094 | |
| µg (Fe3+) cm−2 | 0.029 | 0.029 | 0.026 | 0.032 | |
| µg (Mg2+) cm−2 | 0.253 | 0.255 | 0.239 | 0.273 | |
| µg (Na+) cm−2 | 1.440 | 1.440 | 1.262 | 1.667 | |
| ρ, g cm−3 | 3.783 | 3.790 | 3.783 | 3.798 | |
| 4 | Experimental parameters: 1.25 mol dm−3 HNO3, 55 °C, 24 h | ||||
| µg (Al3+) cm−2 | 1.353 | 1.294 | 1.154 | 1.452 | |
| µg (Ca2+) cm−2 | 0.582 | 0.594 | 0.548 | 0.643 | |
| µg (Fe3+) cm−2 | 0.019 | 0.019 | 0.017 | 0.020 | |
| µg (Mg2+) cm−2 | 0.195 | 0.197 | 0.186 | 0.209 | |
| µg (Na+) cm−2 | 1.014 | 1.016 | 0.907 | 1.146 | |
| ρ, g cm−3 | 3.784 | 3.781 | 3.773 | 3.788 | |
| 5 | Experimental parameters: 2.00 mol dm−3 HNO3, 40 °C, 24 h | ||||
| µg(Al3+) cm−2 | 1.691 | 1.636 | 1.459 | 1.836 | |
| µg (Ca2+) cm−2 | 1.688 | 1.689 | 1.540 | 1.853 | |
| µg (Fe3+) cm−2 | 0.025 | 0.025 | 0.023 | 0.027 | |
| µg (Mg2+) cm−2 | 0.663 | 0.653 | 0.590 | 0.728 | |
| µg (Na+) cm−2 | 1.415 | 1.451 | 1.279 | 1.661 | |
| ρ, g cm−3 | 3.778 | 3.776 | 3.768 | 3.783 | |
| No. of Verifications | Response | Experimental Values | Mean Predicted Values | Low CI (95%) | High CI (95%) |
|---|---|---|---|---|---|
| 1 | Experimental parameters: 0.50 mol dm−3 HNO3, 25 °C, 24 h, desirability 96% | ||||
| µg (Al3+) cm−2 | 0.157 | 0.174 | 0.150 | 0.202 | |
| µg (Ca2+) cm−2 | 0.278 | 0.167 | 0.149 | 0.186 | |
| µg (Fe3+) cm−2 | 0.003 | 0.004 | 0.004 | 0.005 | |
| µg (Mg2+) cm−2 | 0.021 | 0.033 | 0.032 | 0.034 | |
| µg (Na+) cm−2 | 0.108 | 0.198 | 0.186 | 0.210 | |
| ρ, g cm−3 | 3.815 | 3.805 | 3.796 | 3.813 | |
| 2 | Experimental parameters: 0.50 mol dm−3 HNO3, 40 °C, 24 h, desirability 88% | ||||
| µg (Al3+) cm−2 | 0.310 | 0.321 | 0.286 | 0.360 | |
| µg (Ca2+) cm−2 | 0.101 | 0.101 | 0.092 | 0.111 | |
| µg (Fe3+) cm−2 | 0.006 | 0.006 | 0.005 | 0.006 | |
| µg (Mg2+) cm−2 | 0.038 | 0.038 | 0.037 | 0.039 | |
| µg (Na+) cm−2 | 0.250 | 0.247 | 0.235 | 0.261 | |
| ρ, g cm−3 | 3.775 | 3.786 | 3.778 | 3.793 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ćurković, L.; Ropuš, I.; Cajner, H.; Rončević, S.; Gabelica, I. Statistical Optimisation of Chemical Stability of Hybrid Microwave-Sintered Alumina Ceramics in Nitric Acid. Materials 2022, 15, 8823. https://doi.org/10.3390/ma15248823
Ćurković L, Ropuš I, Cajner H, Rončević S, Gabelica I. Statistical Optimisation of Chemical Stability of Hybrid Microwave-Sintered Alumina Ceramics in Nitric Acid. Materials. 2022; 15(24):8823. https://doi.org/10.3390/ma15248823
Chicago/Turabian StyleĆurković, Lidija, Ivana Ropuš, Hrvoje Cajner, Sanda Rončević, and Ivana Gabelica. 2022. "Statistical Optimisation of Chemical Stability of Hybrid Microwave-Sintered Alumina Ceramics in Nitric Acid" Materials 15, no. 24: 8823. https://doi.org/10.3390/ma15248823
APA StyleĆurković, L., Ropuš, I., Cajner, H., Rončević, S., & Gabelica, I. (2022). Statistical Optimisation of Chemical Stability of Hybrid Microwave-Sintered Alumina Ceramics in Nitric Acid. Materials, 15(24), 8823. https://doi.org/10.3390/ma15248823







