Effect and Mechanism of Metakaolin Powder (MP) on Rheological and Mechanical Properties of Cementitious Suspension
Abstract
:1. Introduction
- (i)
- The effects of metakaolin powder (MP) on the macroscopic flow parameters (flow rate and flow spread), microscopic rheological parameters (thixotropy, plastic viscosity, yield stress) and mechanical properties of cementitious suspension under various water–cement ratios were studied, which provided guidance for the application of MP in engineering.
- (ii)
- The bonding strength coefficients and water film thickness were calculated to explain the changes in macroscopic flowability and microrheological properties, which analyzed the mechanism of MP on flow rate, flow spread, thixotropy, plastic viscosity, yield stress from the perspective of particle distance and flocculent structure and built a new evaluation system.
- (iii)
- The improvement of mechanical properties by MP was explained from the perspective of water absorption kinetics and the hydration activity contribution rate.
2. Materials and Methods
2.1. Materials
2.2. Experimental Program
2.3. Test Methods
2.3.1. Flow Spread
- (i)
- The slump cone was placed in the center of the flat steel plate.
- (ii)
- The cementitious suspension was slowly poured into the slump cone until the slump cone was completely filled;
- (iii)
- The slump cone was lifted to allow the suspension to spread until it stopped.
- (iv)
- The diameter of the suspension cake was measured in two orthogonal directions, and the average diameter was calculated. The flow spread of the cementitious suspension was the mean diameter that reduced the bottom diameter of the slump cone.
2.3.2. Flow Rate
2.3.3. Plastic Viscosity, Yield Stress and Thixotropy
2.3.4. Compressive Strength
2.3.5. Water Film Thickness (WFT)
2.3.6. Bonding Strength Coefficient
2.3.7. Water Absorption Dynamics
3. Results and Discussion
3.1. Experimental Result
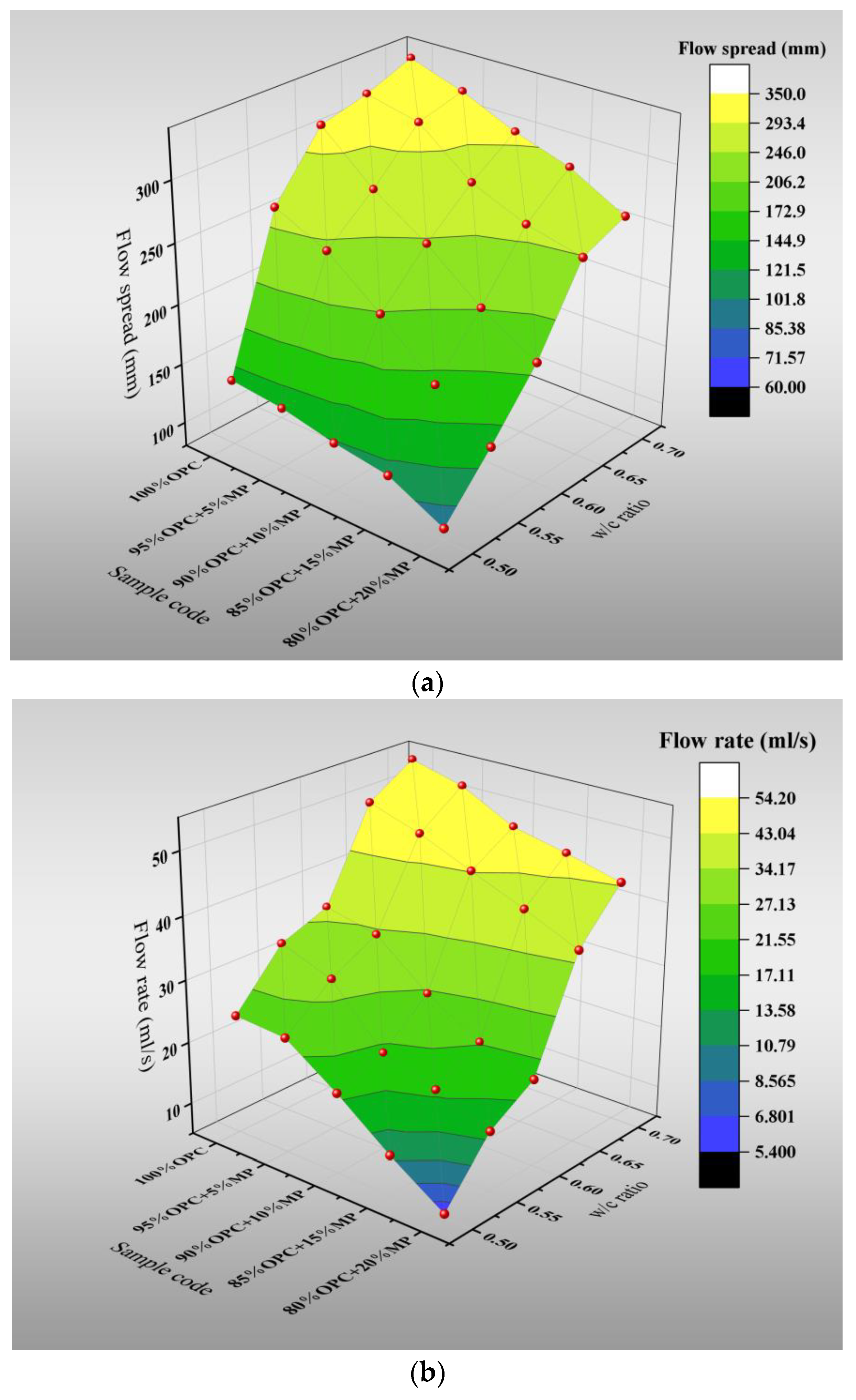
3.1.1. Flow Parameters
3.1.2. Rheological Parameters
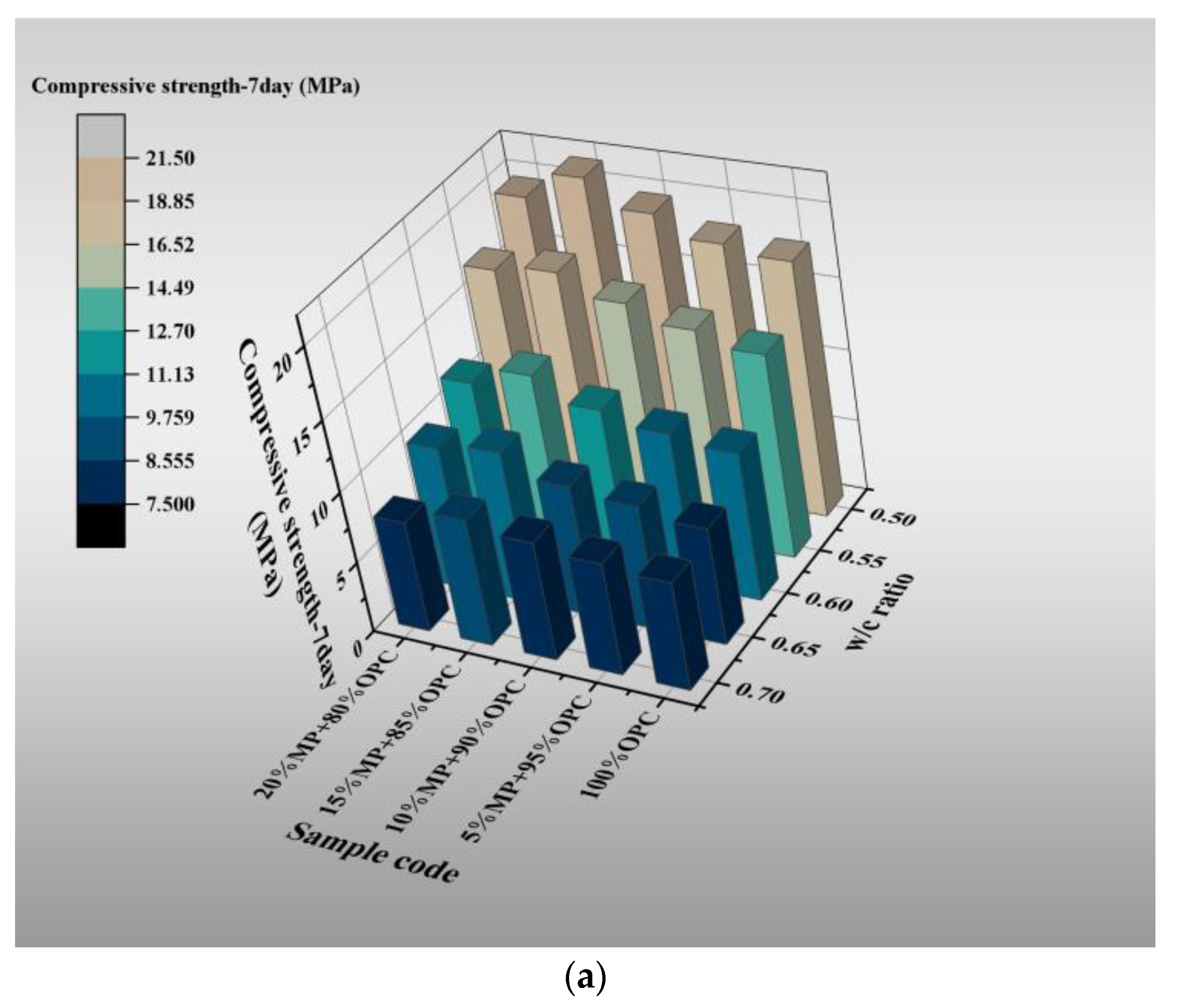
3.1.3. Compressive Strength
3.2. Mechanism of MP on Rheology and Flowability of Cementitious Suspension
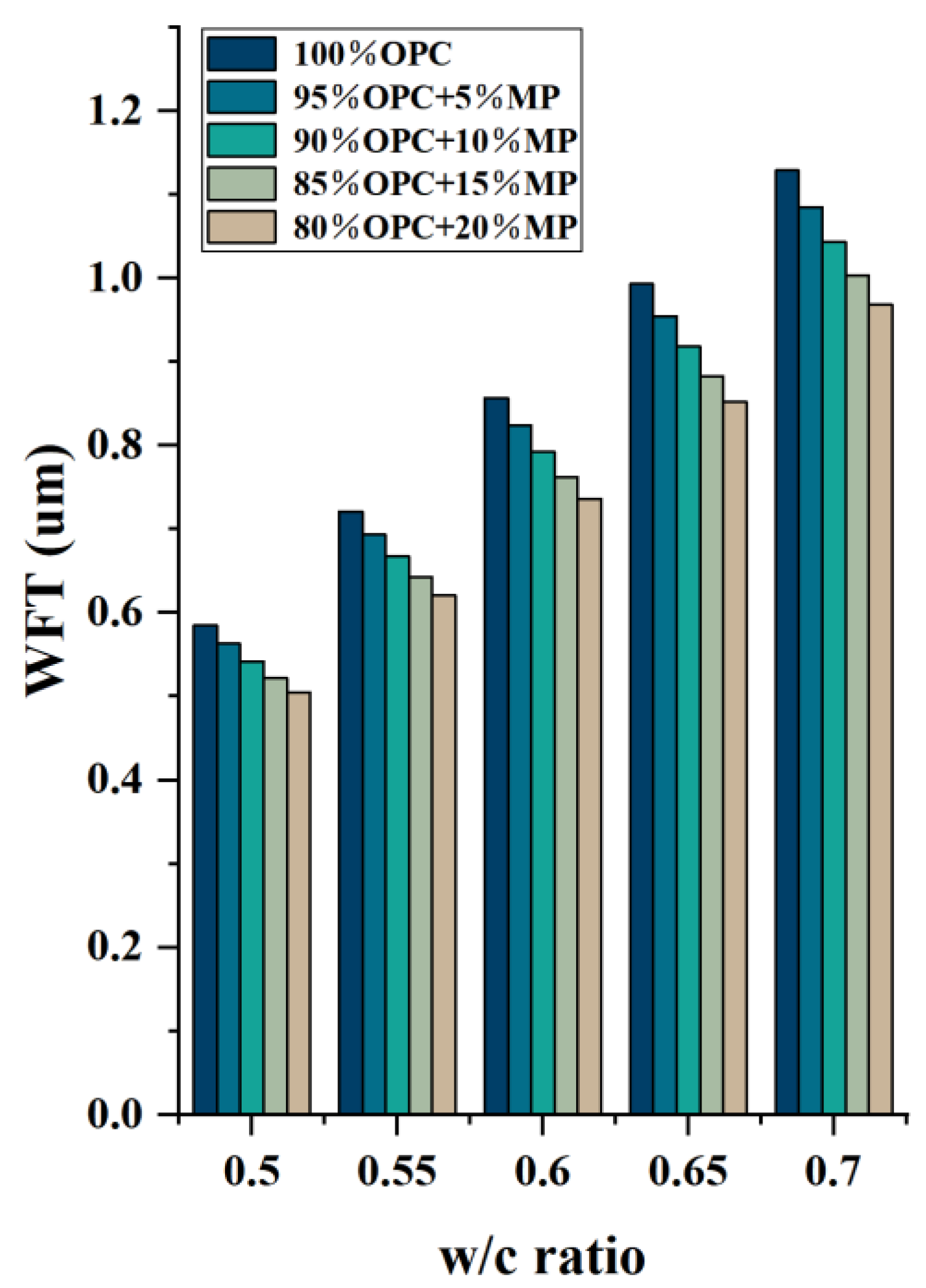
3.2.1. Water Film Thickness (WFT)
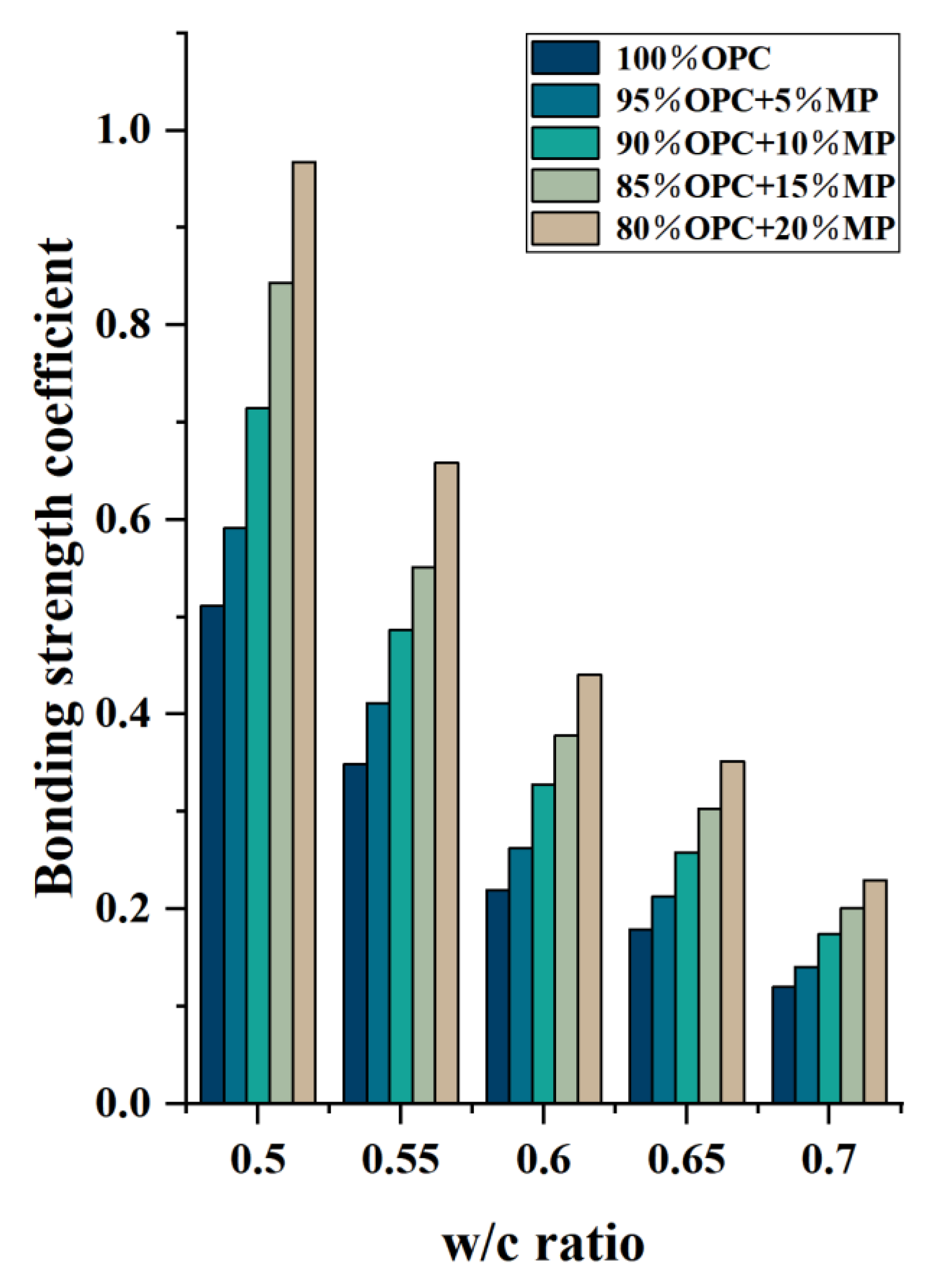
3.2.2. Bonding Strength Coefficient
3.2.3. Evaluation of the Relationship between Micro Rheological Parameters, Macro Flow Parameters and Bonding Strength Coefficient and WFT
3.3. Mechanism of MP on Compressive Strength of Hardened Cementitious Suspension
3.3.1. Hydration Activity Contribution Rate
3.3.2. Mass Water Absorption W, Pore Uniformity α and Average Pore Diameter λ
4. Conclusions
- The addition of MP can reduce the macro flow parameters of cementitious suspension and increase the micro rheological parameters. As MP replacement increased from 5% to 20%, the flow spread decreased in the range of 2.4–48.8%, the flow rate decreased in the range of 2.1–76.7%, the yield stress increased in the range of 5.1–234.2%, the plastic viscosity increased in the range of 1.2–661.3%, the thixotropy increased in the range of 1.8–146.69%, and the compressive strength increased in the range of 2.8–31.5%.
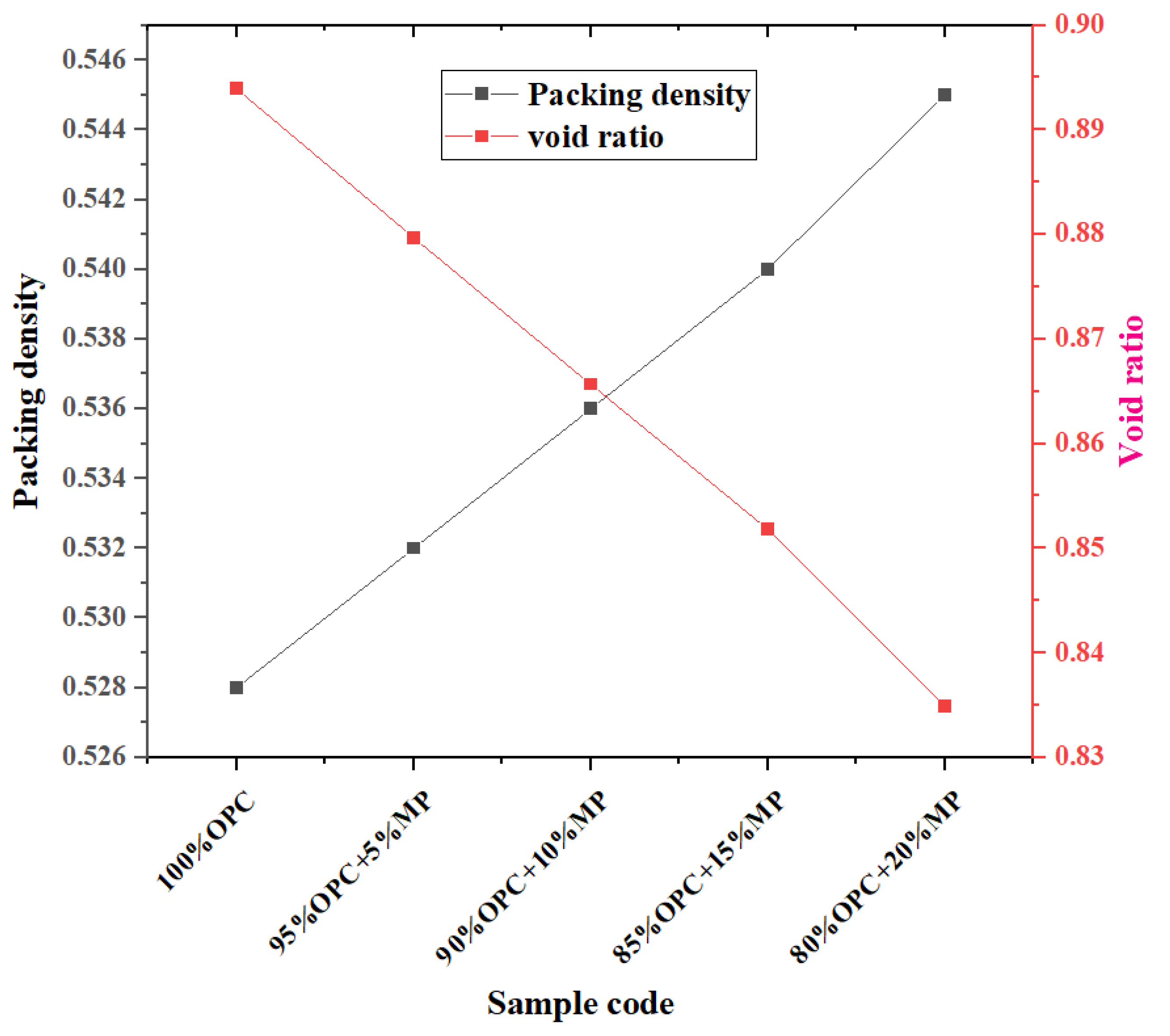
- With the addition of MP from 5% to 20%, the packing density increased by 0.75%, 1.5%, 2.2%, and 3.2%, while the void ratio decreased by 1.6%, 3.2%, 4.7%, and 6.6%. Considering the net effect of the filling effect and surface effect, the addition of MP reduces the WFT of the particles, which explained the mechanism of MP affecting microscopic rheological parameters and macroscopic flow parameters from the particle distance.
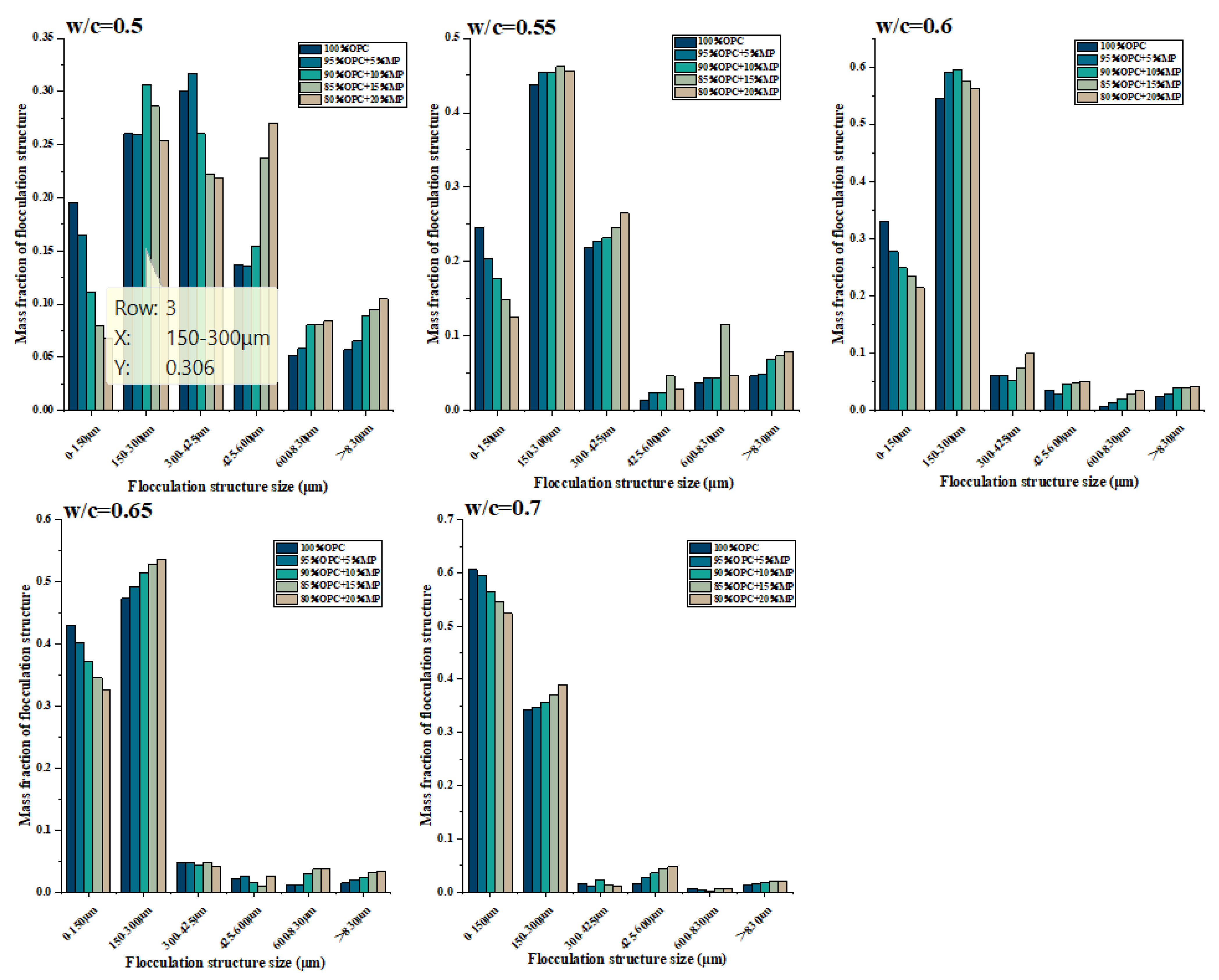
- The addition of MP increased the flocculent structure’s size and strength, and this effect was enhanced with the increase in MP replacement. The incorporation of MP decreased the flocculent structure in the <150 μm range and increased the flocculent structure in the >830 μm range at various W/CM ratios. The mechanism of MP affecting microscopic rheological parameters and macroscopic flow parameters was explained from the flocculent structure.
- The addition of MP reduced the average pore diameter λ and mass water absorption W and increased pore uniformity α and the hydration activity contribution rate, which explained the improvement of MP’s compressive strength from the perspective of water absorption kinetics and hydration. Among them, 15% MP had the best mechanical and microscopic improvement effect.
- The WFT and bonding strength coefficient can effectively characterize flow parameters and rheological parameters, but only mathematical models were established. Based on this evaluation system, a rheological theoretical physical model can be established based on further studies on hydration kinetics, collision mechanics and kinetics. This part of the content is currently in the blank stage of the research field and can be completed in the future.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Raheem, A.A.; Abdulwahab, R.; Kareem, M.A. Incorporation of metakaolin and nanosilica in blended cement mortar and concrete—A review. J. Clean. Prod. 2021, 290, 125852. [Google Scholar] [CrossRef]
- Homayoonmehr, R.; Ramezanianpour, A.A.; Mirdarsoltany, M. Influence of metakaolin on fresh properties, mechanical properties and corrosion resistance of concrete and its sustainability issues: A review. J. Build. Eng. 2021, 44, 103011. [Google Scholar] [CrossRef]
- Zhan, P.-M.; He, Z.-H.; Ma, Z.-M.; Liang, C.-F.; Zhang, X.-X.; Abreham, A.A.; Shi, J.-Y. Utilization of nano-metakaolin in concrete: A review. J. Build. Eng. 2020, 30, 101259. [Google Scholar] [CrossRef]
- Fang, Y.; Wang, J.; Wang, X.; Amaral, M.L.D.; Kniffin, H.; Reed, M.; Wang, L.; Qian, X. Bio-Based Admixture (Black Tea Extraction) for Better Performance of Metakaolin Blended Cement Mortars. Materials 2022, 15, 3994. [Google Scholar] [CrossRef] [PubMed]
- Huang, J.; Zhou, M.; Yuan, H.; Sabri, M.M.S.; Li, X. Prediction of the Compressive Strength for Cement-Based Materials with Metakaolin Based on the Hybrid Machine Learning Method. Materials 2022, 15, 3500. [Google Scholar] [CrossRef] [PubMed]
- Dai, S.; Wang, H.; An, S.; Yuan, L. Mechanical Properties and Microstructural Characterization of Metakaolin Geopolymers Based on Orthogonal Tests. Materials 2022, 15, 2957. [Google Scholar] [CrossRef] [PubMed]
- Yu, Q.; Li, X.; Wang, Z.; Xue, J. Characterization and Performance Evaluation of Metakaolin-Based Geopolymer Foams Obtained by Adding Palm Olein as the Foam Stabilizer. Materials 2022, 15, 2570. [Google Scholar] [CrossRef]
- Kovářík, T.; Bělský, P.; Novotný, P.; Říha, J.; Savková, J.; Medlín, R.; Rieger, D.; Holba, P. Structural and physical changes of re-calcined metakaolin regarding its reactivity. Constr. Build. Mater. 2015, 80, 98–104. [Google Scholar] [CrossRef]
- Li, C.; Sun, H.; Li, L. A review: The comparison between alkali-activated slag (Si+Ca) and metakaolin (Si+Al) cements. Cem. Concr. Res. 2010, 40, 1341–1349. [Google Scholar] [CrossRef]
- Qin, Z.; Zhou, S.; Ma, C.; Long, G.; Xie, Y.; Chen, B. Roles of metakaolin in magnesium phosphate cement: Effect of the replacement ratio of magnesia by metakaolin with different particle sizes. Constr. Build. Mater. 2019, 227, 116675. [Google Scholar] [CrossRef]
- Zhao, D.; Khoshnazar, R. Microstructure of cementituous suspension incorporating high volume of low-grade metakaolin. Cem. Concr. Compos. 2020, 106, 103453. [Google Scholar] [CrossRef]
- Mardani-Aghabaglou, A.; İnan Sezer, G.; Ramyar, K. Comparison of fly ash, silica fume and metakaolin from mechanical properties and durability performance of mortar mixtures view point. Constr. Build. Mater. 2014, 70, 17–25. [Google Scholar] [CrossRef]
- Poona, C.-S.; Lama, L.; Koua, S.C.; Wonga, Y.-L.; Wongb, R. Rate of pozzolanic reaction of metakaolin in high-performance cementituous suspensions. Cem. Concr. Res. 2001, 31, 1301–1306. [Google Scholar] [CrossRef]
- Wei, J.; Gencturk, B. Hydration of ternary Portland cement blends containing metakaolin and sodium bentonite. Cem. Concr. Res. 2019, 123, 105772. [Google Scholar] [CrossRef]
- Antoni, M.; Rossen, J.; Martirena, F.; Scrivener, K. Cement substitution by a combination of metakaolin and limestone. Cem. Concr. Res. 2012, 42, 1579–1589. [Google Scholar] [CrossRef]
- Tang, J.; Wei, S.; Li, W.; Ma, S.; Ji, P.; Shen, X. Synergistic effect of metakaolin and limestone on the hydration properties of Portland cement. Constr. Build. Mater. 2019, 223, 177–184. [Google Scholar] [CrossRef]
- Aiswarya, S.; Prince, A.; Narendran, A. Experimental investigation on concrete containing nano-metakaolin. IRACST. Eng. Sci. Technol. 2013, 3, 180–187. [Google Scholar]
- Norhasri, M.M.; Hamidah, M.; Fadzil, A.M.; Megawati, O. Inclusion of nano metakaolin as additive in ultra high performance concrete (UHPC). Constr. Build. Mater. 2016, 127, 167–175. [Google Scholar] [CrossRef]
- Okamura, H.; Ouchi, M. Self-compacting concrete. J. Adv. Concr. Technol. Jpn. Concr. Inst. 2003, 1, 5–15. [Google Scholar] [CrossRef]
- Sun, X.; Liu, H.; Tian, Z.; Ma, Y.; Wang, Z.; Fan, H. Feasibility and economic evaluation of grouting materials containing binary and ternary industrial waste. Constr. Build. Mater. 2021, 274, 122021. [Google Scholar] [CrossRef]
- Liu, H.; Sun, X.; Wang, Y.; Lu, X.; Du, H.; Tian, Z. Study on the Influence of Silica Fume (SF) on the Rheology, flowability, Stability, Time-Varying Characteristics, and Mechanism of cementituous suspension. Materials 2021, 15, 90. [Google Scholar] [CrossRef] [PubMed]
- Tian, Z.; Ma, Y.; Liu, H.; Sun, X.; Dong, Y. Elucidating how particle flocculating controls fresh properties of cementitious system containing attapulgite powder (AP) and polycarboxylate ether superplasticizer (PCE). Powder Technol. 2022, 399, 117163. [Google Scholar] [CrossRef]
- Rehman, S.K.U.; Ibrahim, Z.; Jameel, M.; Memon, S.A.; Javed, M.F.; Aslam, M.; Mehmood, K.; Nazar, S. Assessment of rheo-logical and piezoresistive properties of graphene based cement composites. Int. J. Concr. Struct. Mater. 2018, 12, 64. [Google Scholar]
- Kwan, A.K.H.; Wong, H.H.C. Effects of packing density, excess water and solid surface area on flowability of cementituous suspension. Adv. Cem. Res. 2008, 20, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Wong, H.H.C.; Kwan, A.K.H. Packing density of cementitious materials: Part 1—Measurement using a wet packing method. Mater. Struct. 2007, 41, 689–701. [Google Scholar] [CrossRef]
- Liu, H.; Tian, Z.; Sun, X.; Ma, Y.; Fan, H.; Dong, Y. A conceptual model focusing on internal flocculent structures and water film thickness for analyzing fresh properties of cementituous suspension containing attapulgite. Constr. Build. Mater. 2022, 325, 126836. [Google Scholar] [CrossRef]
- Son, M.; Hsu, T.-J. The effect of variable yield strength and variable fractal dimension on flocculation of cohesive sediment. Water Res. 2009, 43, 3582–3592. [Google Scholar] [CrossRef]
- Kranenburg, C. The fractal structure of cohesive sediment aggregates. Estuar. Coast. Shelf Sci. 1994, 39, 451–460. [Google Scholar] [CrossRef]
- Wang, X.C.; Jin, P.K. Fractal nature of flocs and compact floc formation. J. Xi’an Univ. Archit. Technol. 2004, 36, 253–262. [Google Scholar]
- Chen, J.J.; Kwan, A.K.H. Superfine cement for improving packing density, rheology and strength of cementituous suspension. Cem. Concr. Compos. 2012, 34, 1–10. [Google Scholar] [CrossRef]
- Chen, J.J.; Ng, P.L.; Li, L.G.; Kwan, A.K.H. Use of superfine zeolite in conjunction with silica fume—Effects on rheology and strength of cementitious suspension. Powder Technol. 2018, 328, 75–83. [Google Scholar] [CrossRef]
- Liu, H.; Sun, X.; Du, H.; Lu, H.; Ma, Y.; Shen, W.; Tian, Z. Effects and threshold of water film thickness on multi-mineral cementituous suspension. Cem. Concr. Compos. 2020, 112, 103677. [Google Scholar] [CrossRef]
- Iqbal, M.F.; Javed, M.F.; Rauf, M.; Azim, I.; Ashraf, M.; Yang, J. Sustainable utilization of foundry waste: Forecasting mechanical properties of foundry sand based concrete using multi-expression programming. Sci. Total Environ. 2021, 780, 146524. [Google Scholar] [CrossRef] [PubMed]











| Phase | Mass Percentage (%) | |
|---|---|---|
| OPC | MP | |
| SiO2 | 13.9 | 43.68 |
| Al2O3 | 3.05 | 44.96 |
| Fe2O3 | 4.80 | 1.58 |
| CaO | 72.37 | 0.33 |
| MgO | 1.07 | 4.26 |
| Na2O | 0.16 | <0.01 |
| SO3 | 2.63 | 2.64 |
| K2O | 0.97 | 0.45 |
| P2O5 | 0.23 | <0.01 |
| TiO2 | 0.35 | 1.66 |
| MnO | 0.30 | 0.04 |
| ZrO2 | 0.02 | <0.01 |
| SrO | 0.10 | <0.01 |
| Cl | 0.02 | <0.01 |
| Sample Code | w/c Ratio | Flow Spread (mm) | Flow Rate (mL/s) |
|---|---|---|---|
| 100% OPC | 0.5 | 135 | 24.1 |
| 95% OPC + 5% MP | 0.5 | 130 | 23.5 |
| 90% OPC + 10% MP | 0.5 | 120 | 17.8 |
| 85% OPC + 15% MP | 0.5 | 113 | 11.3 |
| 80% OPC + 20% MP | 0.5 | 90 | 5.6 |
| 100% OPC | 0.55 | 260 | 32.5 |
| 95% OPC + 5% MP | 0.55 | 240 | 29.5 |
| 90% OPC + 10% MP | 0.55 | 205 | 20.6 |
| 85% OPC + 15% MP | 0.55 | 165 | 17.8 |
| 80% OPC + 20% MP | 0.55 | 133 | 14.5 |
| 100% OPC | 0.6 | 310 | 35.39 |
| 95% OPC + 5% MP | 0.6 | 272 | 33.5 |
| 90% OPC + 10% MP | 0.6 | 243 | 26.7 |
| 85% OPC + 15% MP | 0.6 | 207 | 21.8 |
| 80% OPC + 20% MP | 0.6 | 180 | 18.9 |
| 100% OPC | 0.65 | 320 | 49.56 |
| 95% OPC + 5% MP | 0.65 | 310 | 46.8 |
| 90% OPC + 10% MP | 0.65 | 275 | 43.2 |
| 85% OPC + 15% MP | 0.65 | 256 | 39.65 |
| 80% OPC + 20% MP | 0.65 | 245 | 35.84 |
| 100% OPC | 0.7 | 335 | 54.1 |
| 95% OPC + 5% MP | 0.7 | 320 | 51.89 |
| 90% OPC + 10% MP | 0.7 | 300 | 47.5 |
| 85% OPC + 15% MP | 0.7 | 285 | 45.6 |
| 80% OPC + 20% MP | 0.7 | 260 | 43.4 |
| Sample Code | w/c Ratio | 7-Day Compressive Strength (MPa) | 28-Day Compressive Strength (MPa) |
|---|---|---|---|
| 100% OPC | 0.5 | 17.8 | 27.38 |
| 5% MP + 95% OPC | 0.5 | 18.3 | 28.6 |
| 10% MP + 90% OPC | 0.5 | 19.7 | 29.7 |
| 15% MP + 85% OPC | 0.5 | 21.5 | 33.6 |
| 20% MP + 80% OPC | 0.5 | 19.6 | 31.5 |
| 100% OPC | 0.55 | 14.2 | 21.38 |
| 5% MP + 95% OPC | 0.55 | 15.1 | 23.5 |
| 10% MP + 90% OPC | 0.55 | 16.2 | 25.4 |
| 15% MP + 85% OPC | 0.55 | 17.6 | 27.6 |
| 20% MP + 80% OPC | 0.55 | 17.1 | 26.8 |
| 100% OPC | 0.6 | 10.3 | 16.15 |
| 5% MP + 95% OPC | 0.6 | 10.8 | 17.8 |
| 10% MP + 90% OPC | 0.6 | 11.6 | 18.9 |
| 15% MP + 85% OPC | 0.6 | 13.2 | 20.4 |
| 20% MP + 80% OPC | 0.6 | 11.9 | 19.6 |
| 100% OPC | 0.65 | 8.1 | 13.5 |
| 5% MP + 95% OPC | 0.65 | 8.7 | 14.5 |
| 10% MP + 90% OPC | 0.65 | 9.2 | 15.8 |
| 15% MP + 85% OPC | 0.65 | 10.65 | 17.1 |
| 20% MP + 80% OPC | 0.65 | 10.1 | 16.2 |
| 100% OPC | 0.7 | 7.5 | 10.734 |
| 5% MP + 95% OPC | 0.7 | 7.8 | 11.2 |
| 10% MP + 90% OPC | 0.7 | 8.3 | 12.7 |
| 15% MP + 85% OPC | 0.7 | 8.9 | 13.8 |
| 20% MP + 80% OPC | 0.7 | 7.8 | 13.2 |
| w/c Ratio | Sample Code | Flocculation Structure Mass Fraction | ||||
|---|---|---|---|---|---|---|
| Flocculation Structure Size (μm) | ||||||
| 150 μm | 300 μm | 425 μm | 600 μm | 830 μm | ||
| C-0-0 | 0.805 | 0.545 | 0.245 | 0.108 | 0.057 | |
| C-5-0 | 0.835 | 0.576 | 0.259 | 0.123 | 0.065 | |
| 0.5 | C-10-0 | 0.889 | 0.583 | 0.323 | 0.169 | 0.089 |
| C-15-0 | 0.921 | 0.635 | 0.413 | 0.176 | 0.095 | |
| C-20-0 | 0.932 | 0.678 | 0.459 | 0.189 | 0.105 | |
| -- | 150 μm | 300 μm | 425 μm | 600 μm | 830 μm | |
| C-0-0 | 0.754 | 0.316 | 0.097 | 0.083 | 0.046 | |
| C-5-0 | 0.797 | 0.343 | 0.115 | 0.092 | 0.048 | |
| 0.55 | C-10-0 | 0.823 | 0.368 | 0.136 | 0.112 | 0.069 |
| C-15-0 | 0.851 | 0.389 | 0.143 | 0.189 | 0.073 | |
| C-20-0 | 0.875 | 0.419 | 0.154 | 0.125 | 0.079 | |
| 150 μm | 300 μm | 425 μm | 600 μm | 830 μm | ||
| C-0-0 | 0.669 | 0.125 | 0.065 | 0.031 | 0.024 | |
| C-5-0 | 0.723 | 0.132 | 0.071 | 0.042 | 0.028 | |
| 0.6 | C-10-0 | 0.751 | 0.157 | 0.105 | 0.059 | 0.039 |
| C-15-0 | 0.765 | 0.189 | 0.115 | 0.068 | 0.04 | |
| C-20-0 | 0.786 | 0.225 | 0.126 | 0.075 | 0.041 | |
| -- | 150 μm | 300 μm | 425 μm | 600 μm | 830 μm | |
| C-0-0 | 0.57 | 0.096 | 0.048 | 0.026 | 0.015 | |
| C-5-0 | 0.598 | 0.105 | 0.057 | 0.032 | 0.02 | |
| 0.65 | C-10-0 | 0.628 | 0.113 | 0.069 | 0.054 | 0.024 |
| C-15-0 | 0.654 | 0.125 | 0.078 | 0.068 | 0.031 | |
| C-20-0 | 0.675 | 0.138 | 0.097 | 0.072 | 0.034 | |
| -- | 150 μm | 300 μm | 425 μm | 600 μm | 830 μm | |
| C-0-0 | 0.394 | 0.051 | 0.0347 | 0.0187 | 0.012 | |
| C-5-0 | 0.405 | 0.057 | 0.046 | 0.019 | 0.015 | |
| 0.7 | C-10-0 | 0.435 | 0.079 | 0.057 | 0.021 | 0.019 |
| C-15-0 | 0.454 | 0.083 | 0.069 | 0.025 | 0.02 | |
| C-20-0 | 0.476 | 0.086 | 0.076 | 0.028 | 0.021 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, H.; Wang, Z.; Tian, Z.; Bu, J.; Qiu, J. Effect and Mechanism of Metakaolin Powder (MP) on Rheological and Mechanical Properties of Cementitious Suspension. Materials 2022, 15, 5797. https://doi.org/10.3390/ma15165797
Liu H, Wang Z, Tian Z, Bu J, Qiu J. Effect and Mechanism of Metakaolin Powder (MP) on Rheological and Mechanical Properties of Cementitious Suspension. Materials. 2022; 15(16):5797. https://doi.org/10.3390/ma15165797
Chicago/Turabian StyleLiu, Hengrui, Zezhu Wang, Zhenghong Tian, Jingwu Bu, and Jianchun Qiu. 2022. "Effect and Mechanism of Metakaolin Powder (MP) on Rheological and Mechanical Properties of Cementitious Suspension" Materials 15, no. 16: 5797. https://doi.org/10.3390/ma15165797






