Effects of Radiative Electro-Magnetohydrodynamics Diminishing Internal Energy of Pressure-Driven Flow of Titanium Dioxide-Water Nanofluid due to Entropy Generation
Abstract
:1. Introduction
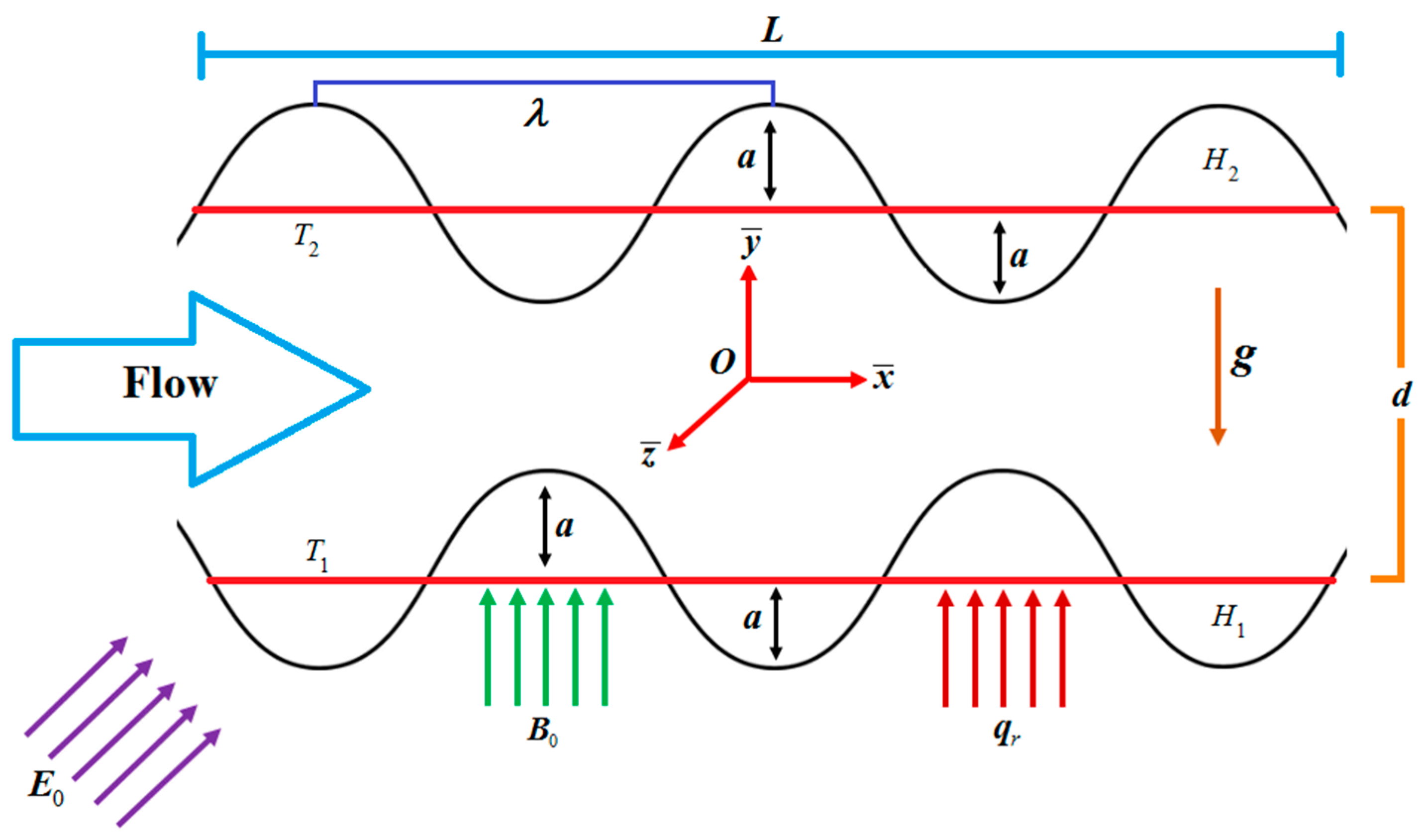
2. Problem Description
2.1. Flow Analysis
2.2. Formulation
2.3. Physical Quantities
2.3.1. Drag Force (Skin Friction)
2.3.2. Heat Transfer Ratio (Nusselt Number)
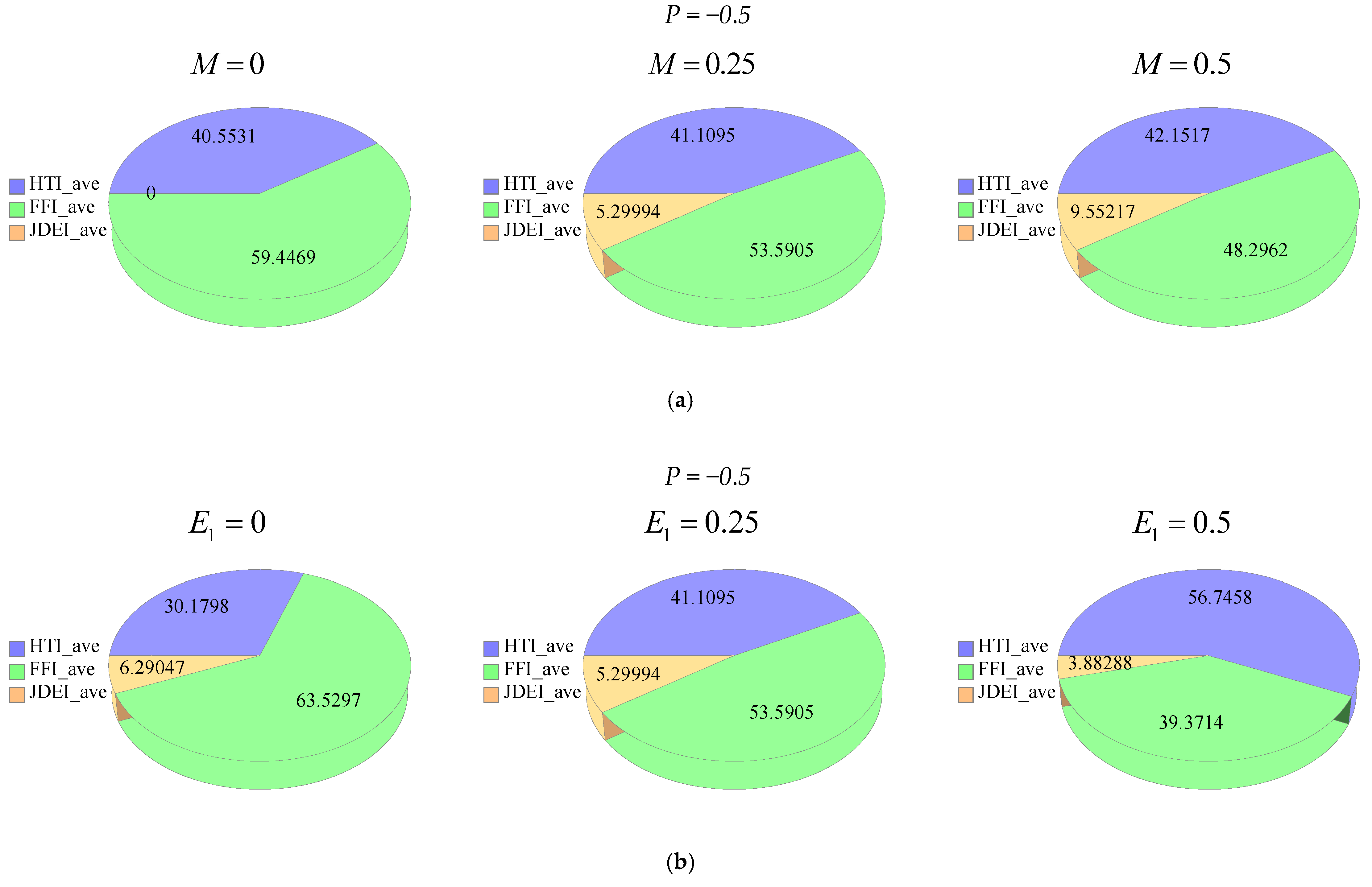
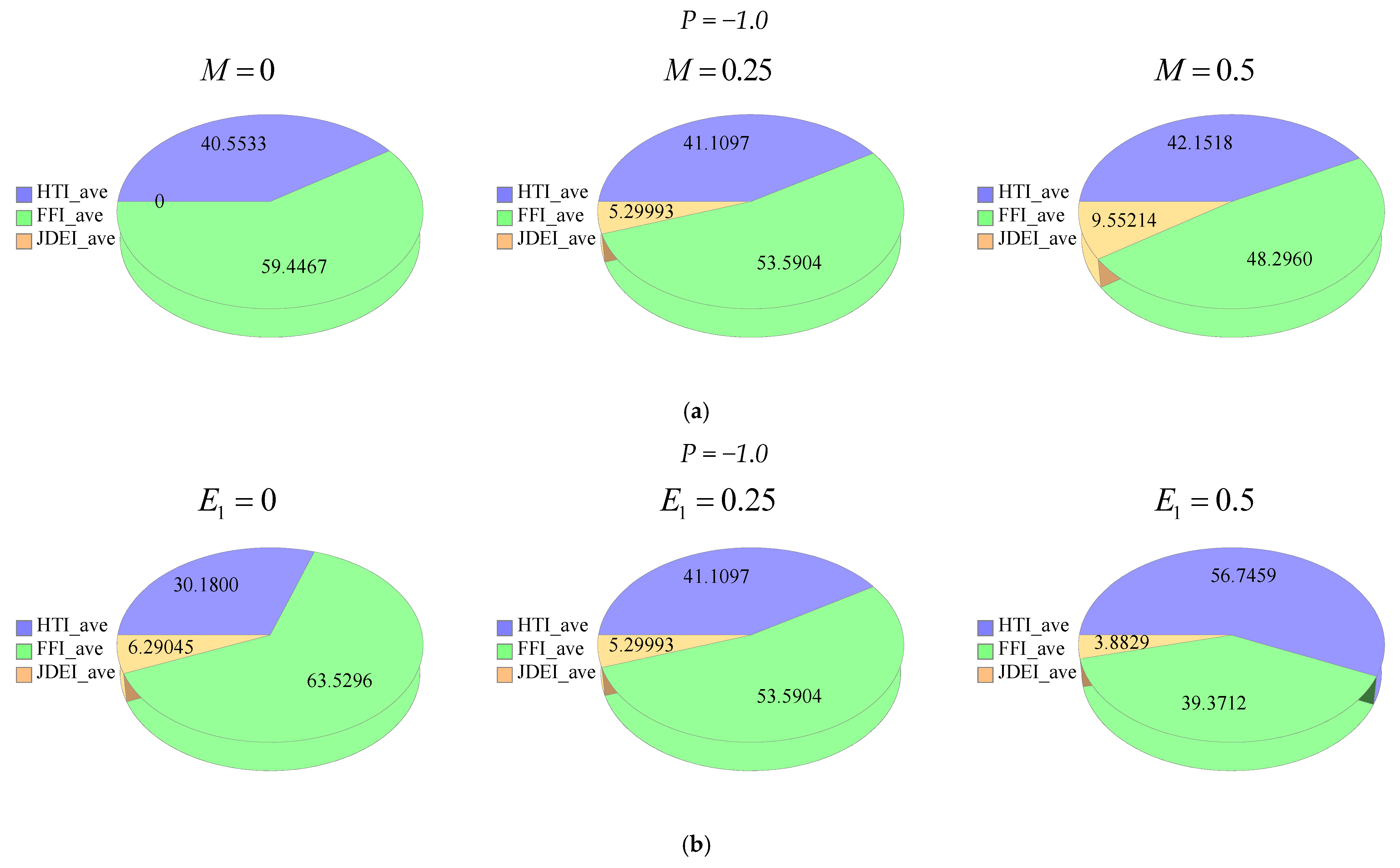
3. Analysis of Energy Loss
4. Analytical Procedure
5. Code Validation and Convergence Analysis
6. Results and Discussion
7. Conclusions
- 1)
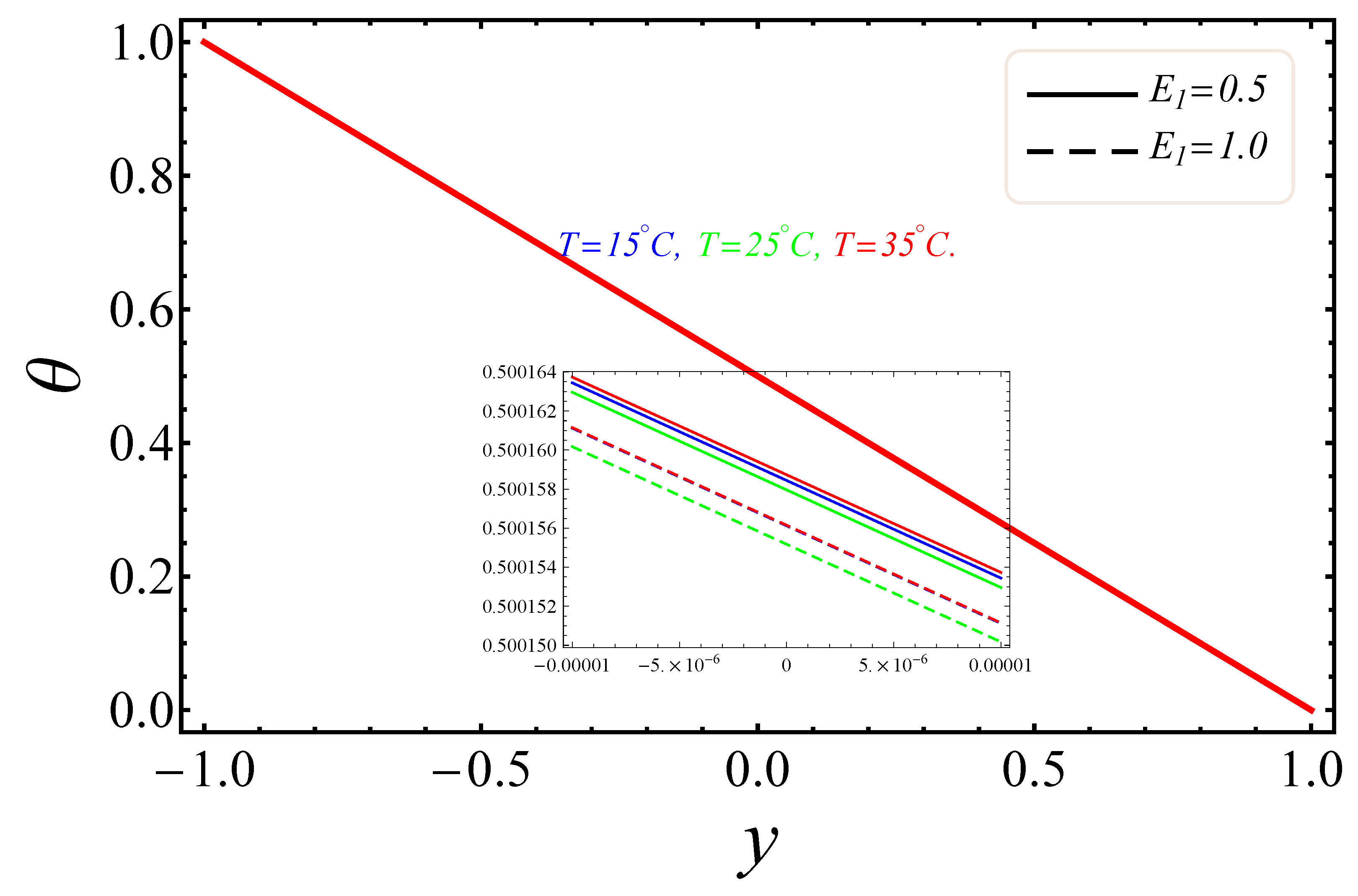
- The electric field applied on a tangential direction to the fluid affected both the velocity and temperature distributions, which produced a reduction in the temperature and an increase in the velocity.
- 2)
- The suspension of nanoparticles in the base fluid caused a slowdown in nanofluid velocity.
- 3)
- The thermal boundary layer increased against the growing radiation parameter , which was why an increase in temperature was observed.
- 4)
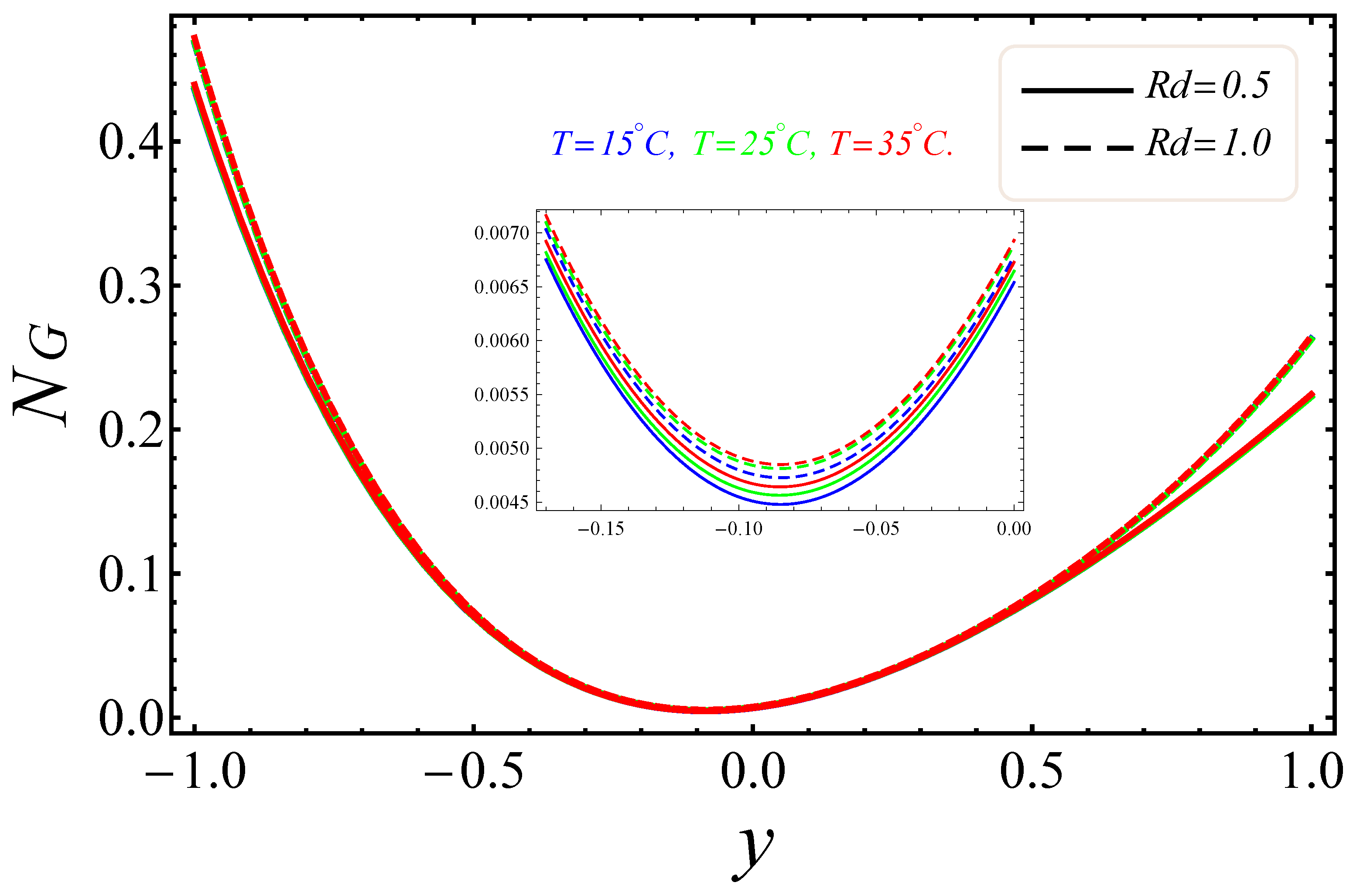
- The entropy generation near the boundary of the channel prolonged, while was very insufficient at the vicinity of the center for the electric field .
- 5)
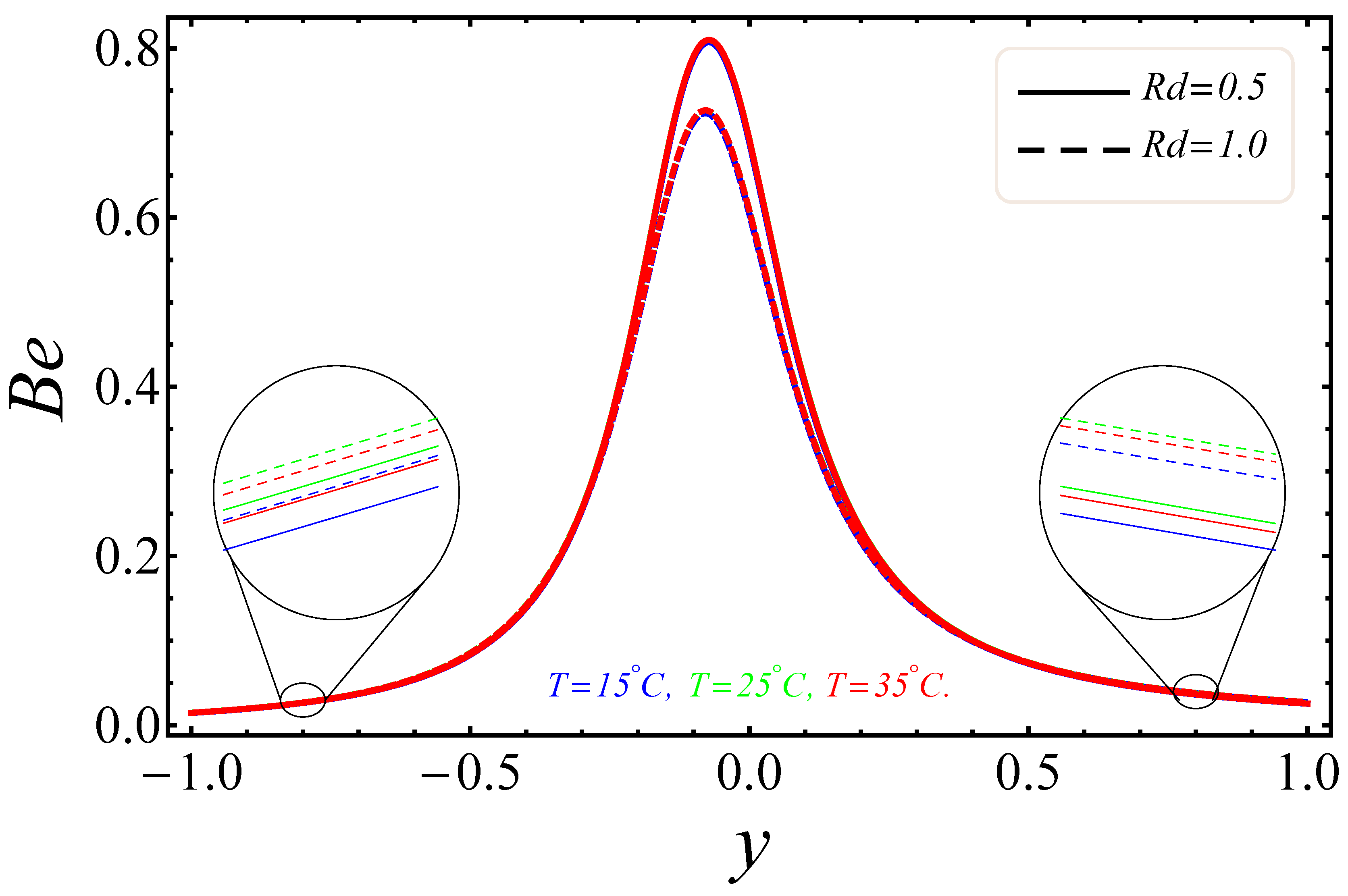
- Initially, attained a high impact near the middle of channel, but gradually it fell for a large value of the electric field parameter near the walls.
- 6)
- The entropy generation for the group parameter and the radiation parameter at the intermediate of the channel was approximately zero, while an enhancement was noted near the walls.
- 7)
- The average energy loss was due to a rise in the pressure gradient.
Author Contributions
Funding
Conflicts of Interest
References
- Chauhan, D.S.; Rastogi, P. Hall current and heat transfer effects on MHD flow in a channel partially filled with a porous medium in a rotating system. Turk. J. Eng. Environ. Sci. 2010, 33, 167–184. [Google Scholar] [CrossRef]
- Pal, D.; Mondal, H. Hydromagnetic non-Darcy flow and heat transfer over a stretching sheet in the presence of thermal radiation and Ohmic dissipation. Commun. Nonlinear Sci. Numer Simul. 2010, 15, 1197–1209. [Google Scholar] [CrossRef]
- Choi, S.U.S. Enhancing thermal conductivity of fluids with nanoparticles. ASME Publ. Fed. 1995, 231, 99–106. [Google Scholar]
- Sajadi, A.R.; Kazemi, M.H. Investigation of turbulent convective heat transfer and pressure drop of TiO2/water nanofluid in circular tube. Int. Commun. Heat Mass Transf. 2011, 38, 1474–1478. [Google Scholar] [CrossRef]
- Ropers, M.H.; Terrisse, H.; Mercier-Bonin, M.; Humbert, B. Titanium Dioxide as Food Additive. In Application of Titanium Dioxide; InTech: London, UK, 2017. [Google Scholar]
- Bhatti, M.M.; Zeeshan, A.; Ellahi, R.; Beg, A.O.; Kadir, A. Effects of coagulation on the two phase peristaltic pumping of magnetized Prandtl biofluid through an endoscopic annular geometry containing a porous medium. Chinees J. Phys. 2019, 58, 222–234. [Google Scholar] [CrossRef]
- Haq, R.U.; Soomro, F.A.; Öztop, H.F.; Mekkaoui, T. Thermal management of water-based carbon nanotubes enclosed in a partially heated triangular cavity with heated cylindrical obstacle. Int. J. Heat Mass Transf. 2019, 131, 724–736. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Haq, Ri.; Shafee, A.; Li, Z. Heat transfer behavior of nanoparticle enhanced PCM solidification through an enclosure with V shaped fins. Int. J. Heat Mass Transf. 2019, 130, 1322–1342. [Google Scholar] [CrossRef]
- Ul Haq, R.; Aman, S. Water functionalized CuO nanoparticles filled in a partially heated trapezoidal cavity with inner heated obstacle: FEM approach. Int. J. Heat Mass Transf. 2019, 129, 401–417. [Google Scholar] [CrossRef]
- Hosseini, S.M.; Safaei, M.R.; Goodarzi, M.; Alrashed, A.A.A.A.; Nguyen, T.K. New temperature, interfacial shell dependent dimensionless model for thermal conductivity of nanofluids. Int. J. Heat Mass Transf. 2017, 114, 207–210. [Google Scholar] [CrossRef]
- Nasiri, H.; Jamalabadi, M.Y.A.; Sadeghi, R.; Safaei, M.R.; Nguyen, T.K.; Shadloo, M.S. A smoothed particle hydrodynamics approach for numerical simulation of nano-fluid flows. J. Therm. Anal. Calorim. 2018, 1–9. [Google Scholar] [CrossRef]
- Safaei, M.R.; Ahmadi, G.; Goodarzi, M.S.; Shadloo, M.S.; Goshayeshi, H.R.; Dahari, M. Heat transfer and pressure drop in fully developed turbulent flows of graphene nanoplatelets–silver/water nanofluids. Fluids 2016, 1, 20. [Google Scholar] [CrossRef]
- Hassan, M.; Marin, M.; Ellahi, R.; Alamri, S.Z. Exploration of convective heat transfer and flow characteristics synthesis by Cu-Ag/water hybrid-nanofluids. Heat Transf. Res. 2018, 49, 1837–1848. [Google Scholar] [CrossRef]
- Shehzad, N.; Zeeshan, A.; Ellahi, R.; Rashidi, S. Modelling study on internal energy loss due to entropy generation for non-Darcy Poiseuille flow of silver water nanofluid: An application of purification. Entropy 2018, 20, 851. [Google Scholar] [CrossRef]
- Ellahi, R.; Zeeshan, A.; Hussain, F.; Abbas, T. Study of shiny film coating on multi-fluid flows of a rotating disk suspended with nano-sized silver and gold particles: A comparative analysis. Coatings 2018, 8, 422. [Google Scholar] [CrossRef]
- Alamri, S.Z.; Ellahi, R.; Shehzad, N.; Zeeshan, A. Convective radiative plane Poiseuille flow of nanofluid through porous medium with slip: An application of Stefan blowing. J. Mol. Liq. 2019, 273, 292–304. [Google Scholar] [CrossRef]
- Ellahi, R.; Zeeshan, A.; Hussain, F.; Asadollahi, A. Peristaltic blood flow of couple stress fluid suspended with nanoparticles under the influence of chemical reaction and activation energy. Symmetry 2019, 11, 276. [Google Scholar] [CrossRef]
- Mahmud, S.; Fraser, R.A. Analysis of mixed convection—Radiation interaction in a vertical channel: Entropy generation. Exergy Int. J. 2002, 2, 330–339. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Abelman, S.; Mehr, N.F. Entropy generation in steady MHD flow due to a rotating porous disk in a nanofluid. Int. J. Heat Mass Transf. 2013, 62, 515–525. [Google Scholar] [CrossRef]
- Cho, C.C.; Chen, C.L. Natural convection heat transfer and entropy generation in wavy-wall enclosure containing water-based nanofluid. Int. J. Heat Mass Transf. 2013, 61, 749–758. [Google Scholar] [CrossRef]
- Ranjit, N.K.; Shit, G.C. Entropy generation on electro-osmotic flow pumping by a uniform peristaltic wave under magnetic environment. Energy 2017, 128, 649–660. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Grosan, T.; Pop, I. Natural convection and entropy generation in a square cavity with variable temperature side walls filled with a nanofluid: Buongiorno’s mathematical model. Entropy 2017, 19, 337. [Google Scholar] [CrossRef]
- Jamalabadi, M.Y.A.; Safaei, M.R.; Alrashed, A.A.A.A.; Nguyen, T.K.; Filho, E.P.B. Entropy generation in thermal radiative loading of structures with distinct heaters. Entropy 2017, 19, 506. [Google Scholar] [CrossRef]
- Darbari, B.; Rashidi, S.; Abolfazli Esfahani, J. Sensitivity analysis of entropy generation in nanofluid flow inside a channel by response surface methodology. Entropy 2016, 18, 52. [Google Scholar] [CrossRef]
- Bhatti, M.M.; Abbas, T.; Rashidi, M.M.; Ali, M.E.-S. Numerical simulation of entropy generation with thermal radiation on MHD carreau nanofluid towards a shrinking sheet. Entropy 2016, 18, 200. [Google Scholar] [CrossRef]
- Bhatti, M.M.; Abbas, T.; Rashidi, M.M.; Ali, M.E.-S.; Yang, Z. Entropy generation on MHD Eyring–Powell nanofluid through a permeable stretching surface. Entropy 2016, 18, 224. [Google Scholar] [CrossRef]
- Abbas, T.; Ayub, M.; Bhatti, M.M.; Rashidi, M.M.; Ali, M.E.-S. Entropy generation on nanofluid flow through a horizontal Riga plate. Entropy 2016, 18, 223. [Google Scholar] [CrossRef]
- Ellahi, R.; Alamri, S.Z.; Basit, A.; Majeed, A. Effects of MHD and slip on heat transfer boundary layer flow over a moving plate based on specific entropy generation. J. Taibah Univ. Sci. 2018, 12, 476–482. [Google Scholar] [CrossRef]
- Liao, S.J. Beyond Perturbation: Introduction to Homotopy Analysis Method; Chapman and Hall/CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]
- Ellahi, R. The effects of MHD and temperature dependent viscosity on the flow of non-Newtonian nanofluid in a pipe: Analytical solutions. Appl. Math. Model. 2013, 37, 1451–1457. [Google Scholar] [CrossRef]
- Hussain, F.; Ellahi, R.; Zeeshan, A. Mathematical models of electro magnetohydrodynamic Multiphase Flows Synthesis with Nanosized Hafnium Particles. Appl. Sci. 2018, 8, 275. [Google Scholar] [CrossRef]
- Zeeshan, A.; Ijaz, N.; Abbas, T.; Ellahi, R. The sustainable characteristic of Bio-bi-phase flow of peristaltic transport of MHD Jeffery fluid in human body. Sustainability 2018, 10, 2671. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50. [Google Scholar] [CrossRef]
- Zeeshan, A.; Shehzad, N.; Ellahi, R.; Alamri, S.Z. Convective Poiseuille flow of Al2O3-EG nanofluid in a porous wavy channel with thermal radiation. Neural Comput. Appl. 2018, 30, 3371–3382. [Google Scholar] [CrossRef]
- Matin, M.H.; Pop, I. Forced convection heat and mass transfer flow of a nanofluid through a porous channel with a first order chemical reaction on the wall. Int. Commun. Heat Mass Transf. 2013, 46, 134–141. [Google Scholar] [CrossRef]
- Duangthongsuk, W.; Wongwises, S. Measurement of temperature-dependent thermal conductivity and viscosity of TiO2-water nanofluids. Exp. Therm. Fluid Sci. 2009, 33, 706–714. [Google Scholar] [CrossRef]
- Khanafer, K.; Vafai, K. A critical synthesis of thermophysical characteristics of nanofluids. Int. J. Heat Mass Transf. 2011, 54, 4410–4428. [Google Scholar] [CrossRef]
- Ahmed, M.A.; Shuaib, N.H.; Yusoff, M.Z.; Al-Falahi, A.H. Numerical investigations of flow and heat transfer enhancement in a corrugated channel using nanofluid. Int. Commun. Heat Mass Transf. 2011, 38, 1368–1375. [Google Scholar] [CrossRef]
- Mahian, O.; Kianifar, A.; Kleinstreuer, C.; Moh’d A, A.N.; Pop, I.; Sahin, A.Z.; Wongwises, S. A review of entropy generation in nanofluid flow. Int. J. Heat Mass Transf. 2013, 65, 514–532. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Jena, S.K.; Mahapatra, S.K. MHD convection of nanofluids: A review. J. Nanofluids 2015, 4, 271–292. [Google Scholar] [CrossRef]
- Rosseland, S. Astrophysik und Atom-Theoretische Grundlagen; Springer Verlag: Berlin, Germany, 1931; pp. 41–44. [Google Scholar]
- Ellahi, R.; Zeeshan, A.; Shehzad, N.; Alamri, S.Z. Structural impact of kerosene-Al2O3 nanoliquid on MHDpoiseuille flow with variable thermal conductivity: Application of cooling process. J. Mol. Liq. 2018, 264, 607–615. [Google Scholar] [CrossRef]
- Tippa, S.; Narahari, M.; Pendyala, R. Unsteady natural convection flow of nanofluids past a semi-infinite isothermal vertical plate. AIP Conf. Proc. 2016, 1787, 020014. [Google Scholar] [CrossRef]
- Raza, J.; Rohni, A.M.; Omar, Z. Numerical investigation of copper-water (Cu-water) nanofluid with different shapes of nanoparticles in a channel with stretching wall: Slip effects. Math. Comput. Appl. 2016, 21, 43. [Google Scholar] [CrossRef]
- Kaladhar, K.; Motsa, S.S.; Srinivasacharya, D. Mixed convection flow of couple stress fluid in a vertical channel with radiation and Soret effects. J. Appl. Fluid Mech. 2016, 9, 43–50. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Pop, I. Conjugate natural convection in a square porous cavity filled by a nanofluid using Buongiorno’s mathematical model. Int. J. Heat Mass Transf. 2014, 79, 137–145. [Google Scholar] [CrossRef]
- Van Gorder, R.A.; Vajravelu, K. On the selection of auxiliary functions, operators, and con-vergence control parameters in the application of the Homotopy Analysis Method to nonlinear differential equations: A general approach. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 4078–4089. [Google Scholar] [CrossRef]
- Liao, S.J. An analytic approximate technique for free oscillations of positively damped systems with algebraically decaying amplitude. Int. J. Non-Linear Mech. 2003, 38, 1173–1183. [Google Scholar] [CrossRef]












| Property | Water (H2O) | Titanium dioxide (TiO2) [43,44] |
|---|---|---|
| Density (kgm−3) | = 9.877 × 102 | = 4.250 × 103 |
| Heat capacity (Jkg−1 K−1) | = 4.066 × 103 | = 6.862 × 102 |
| Electrical conductivity (m−1) | = 5.0 × 10−2 | = 0.24 × 107 |
| Thermal conductivity (W m−1 K−1) | = 6.44 × 10−1 | = 8.9538 |
| Thermal expansion coefficient (K−1) | = 21 × 105 | = 0.9 × 105 |
| Order of Approximation | Time | ||
|---|---|---|---|
| 05 | 5.3818 | 4..4073 × 10−4 | 2.8357 × 10−6 |
| 10 | 9.7290 | 2.8199 × 10−8 | 4.6835 × 10−9 |
| 15 | 16.7899 | 4.0554 × 10−14 | 5.0418 × 10−14 |
| 20 | 26.9812 | 1.0687 × 10−17 | 1..0454 × 10−17 |
| 30 | 40.6344 | 1.3593 × 10−22 | 7.9903 × 10−22 |
| 0.5% | 0.0 | 0.00 | 3.80765 | −2.41647 | 3.79748 | −2.40690 | 3.80302 | −2.41211 |
| 0.25 | 3.56943 | −2.19941 | 3.55835 | −2.18901 | 3.56439 | −2.19466 | ||
| 0.50 | 3.36696 | −2.01740 | 3.35512 | −2.00632 | 3.36157 | −2.01235 | ||
| 0.5 | 0.00 | 3.80765 | −2.41647 | 3.79748 | −2.40690 | 3.80302 | −2.41211 | |
| 0.25 | 3.79915 | −2.42912 | 3.78789 | −2.41854 | 3.79402 | −2.42430 | ||
| 0.50 | 3.79582 | −2.44626 | 3.78342 | −2.43462 | 3.79017 | −2.44096 | ||
| 1.0 | 0.00 | 3.80765 | −2.41647 | 3.79748 | −2.40690 | 3.80302 | −2.41211 | |
| 0.25 | 4.02891 | −2.65888 | 4.01746 | −2.64812 | 4.02370 | −2.65398 | ||
| 0.50 | 4.22474 | −2.87519 | 4.21177 | −2.86298 | 4.21883 | −2.86962 | ||
| 1.0% | 0.0 | 0.00 | 3.79649 | −2.41100 | 3.78657 | −2.40167 | 3.79221 | −2.40697 |
| 0.25 | 3.56060 | −2.19602 | 3.54979 | −2.18588 | 3.55594 | −2.19164 | ||
| 0.50 | 3.35987 | −2.01553 | 3.34833 | −2.00473 | 3.35489 | −2.01086 | ||
| 0.5 | 0.00 | 3.79649 | −2.41100 | 3.78657 | −2.40167 | 3.79221 | −2.40697 | |
| 0.25 | 3.78872 | −2.42414 | 3.77774 | −2.41382 | 3.78398 | −2.41968 | ||
| 0.50 | 3.78594 | −2.44161 | 3.77386 | −2.43026 | 3.78073 | −2.43671 | ||
| 1.0 | 0.00 | 3.79649 | −2.41100 | 3.78657 | −2.40167 | 3.79221 | −2.40697 | |
| 0.25 | 4.01688 | −2.65231 | 4.00572 | −2.64181 | 4.01207 | −2.64777 | ||
| 0.50 | 4.21208 | −2.86774 | 4.19945 | −2.85586 | 4.20663 | −2.86261 | ||
| 1.5% | 0.0 | 0.00 | 3.78532 | −2.40553 | 3.77565 | −2.39643 | 3.78140 | −2.40183 |
| 0.25 | 3.55174 | −2.19262 | 3.54122 | −2.18273 | 3.54747 | −2.18860 | ||
| 0.50 | 3.35275 | −2.01363 | 3.34151 | −2.00311 | 3.34818 | −2.00935 | ||
| 0.5 | 0.00 | 3.78532 | −2.40553 | 3.77565 | −2.39643 | 3.78140 | −2.40183 | |
| 0.25 | 3.77827 | −2.41915 | 3.76758 | −2.40909 | 3.77393 | −2.41506 | ||
| 0.50 | 3.77605 | −2.43693 | 3.76429 | −2.42589 | 3.77127 | −2.43244 | ||
| 1.0 | 0.00 | 3.78532 | −2.40553 | 3.77565 | −2.39643 | 3.78140 | −2.40183 | |
| 0.25 | 4.00485 | −2.64573 | 3.99398 | −2.63550 | 4.00044 | −2.64157 | ||
| 0.50 | 4.19941 | −2.86030 | 4.18712 | −2.84873 | 4.19442 | −2.85559 | ||
| 0.5% | 0.0 | 0.00 | 0.510991 | 0.511551 | 0.509936 | 0.510495 | 0.506687 | 0.507244 |
| 0.25 | 0.511011 | 0.511534 | 0.509955 | 0.510479 | 0.506707 | 0.507228 | ||
| 0.50 | 0.511032 | 0.511517 | 0.509976 | 0.510461 | 0.506728 | 0.507211 | ||
| 0.5 | 0.00 | 0.510991 | 0.511551 | 0.509936 | 0.510495 | 0.506687 | 0.507244 | |
| 0.25 | 0.510989 | 0.511553 | 0.509934 | 0.510497 | 0.506685 | 0.507247 | ||
| 0.50 | 0.510987 | 0.511555 | 0.509932 | 0.510499 | 0.506683 | 0.507249 | ||
| 1.0 | 0.00 | 0.510991 | 0.511551 | 0.509936 | 0.510495 | 0.506687 | 0.507244 | |
| 0.25 | 0.510962 | 0.511613 | 0.509872 | 0.510556 | 0.506623 | 0.507306 | ||
| 0.50 | 0.510863 | 0.511672 | 0.509809 | 0.510616 | 0.506560 | 0.507365 | ||
| 1.0% | 0.0 | 0.00 | 0.511061 | 0.511618 | 0.510000 | 0.510556 | 0.506752 | 0.507306 |
| 0.25 | 0.511081 | 0.511601 | 0.510019 | 0.510540 | 0.506771 | 0.507289 | ||
| 0.50 | 0.511101 | 0.3511584 | 0.510040 | 0.510523 | 0.506791 | 0.507272 | ||
| 0.5 | 0.00 | 0.511061 | 0.511618 | 0.510000 | 0.510556 | 0.506752 | 0.507306 | |
| 0.25 | 0.511059 | 0.511620 | 0.509998 | 0.510558 | 0.506749 | 0.507308 | ||
| 0.50 | 0.511057 | 0.511622 | 0.509996 | 0.510560 | 0.506747 | 0.507310 | ||
| 1.0 | 0.00 | 0.511061 | 0.511618 | 0.510000 | 0.510556 | 0.506750 | 0.507306 | |
| 0.25 | 0.510997 | 0.511679 | 0.509936 | 0.510617 | 0.506688 | 0.507367 | ||
| 0.50 | 0.510934 | 0.511738 | 0.509873 | 0.510676 | 0.506625 | 0.507425 | ||
| 1.5% | 0.0 | 0.00 | 0.511131 | 0.511685 | 0.510000 | 0.510617 | 0.506816 | 0.507367 |
| 0.25 | 0.511150 | 0.511668 | 0.510083 | 0.510601 | 0.506835 | 0.507351 | ||
| 0.50 | 0.511171 | 0.511651 | 0.510103 | 0.510584 | 0.506855 | 0.507334 | ||
| 0.5 | 0.00 | 0.511131 | 0.511685 | 0.510064 | 0.510617 | 0.506816 | 0.507367 | |
| 0.25 | 0.511129 | 0.511687 | 0.510062 | 0.510619 | 0.506814 | 0.507369 | ||
| 0.50 | 0.511126 | 0.511689 | 0.510060 | 0.510621 | 0.5068110 | 0.507371 | ||
| 1.0 | 0.00 | 0.511131 | 0.511685 | 0.510064 | 0.510617 | 0.506816 | 0.507428 | |
| 0.25 | 0.511067 | 0.511746 | 0.510000 | 0.510678 | 0.506752 | 0.507428 | ||
| 0.50 | 0.511004 | 0.511804 | 0.509938 | 0.510736 | 0.506690 | 0.507486 | ||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zeeshan, A.; Shehzad, N.; Abbas, T.; Ellahi, R. Effects of Radiative Electro-Magnetohydrodynamics Diminishing Internal Energy of Pressure-Driven Flow of Titanium Dioxide-Water Nanofluid due to Entropy Generation. Entropy 2019, 21, 236. https://doi.org/10.3390/e21030236
Zeeshan A, Shehzad N, Abbas T, Ellahi R. Effects of Radiative Electro-Magnetohydrodynamics Diminishing Internal Energy of Pressure-Driven Flow of Titanium Dioxide-Water Nanofluid due to Entropy Generation. Entropy. 2019; 21(3):236. https://doi.org/10.3390/e21030236
Chicago/Turabian StyleZeeshan, Ahmed, Nasir Shehzad, Tehseen Abbas, and Rahmat Ellahi. 2019. "Effects of Radiative Electro-Magnetohydrodynamics Diminishing Internal Energy of Pressure-Driven Flow of Titanium Dioxide-Water Nanofluid due to Entropy Generation" Entropy 21, no. 3: 236. https://doi.org/10.3390/e21030236
APA StyleZeeshan, A., Shehzad, N., Abbas, T., & Ellahi, R. (2019). Effects of Radiative Electro-Magnetohydrodynamics Diminishing Internal Energy of Pressure-Driven Flow of Titanium Dioxide-Water Nanofluid due to Entropy Generation. Entropy, 21(3), 236. https://doi.org/10.3390/e21030236






