On The Use of Entropy to Predict Boundary Layer Stability

Abstract

Boundary layer transition is a critical parameter in the design of fluid flow systems. This situation is due to the dramatic change in both entropy production and heat transfer that accompanies it. It is well recognized that many parameters affect the location of transition onset, however, no models exist which unify all these parameters. This paper presents a new hypothesis that the driving force of boundary layer transition onset is the entropy generation rate alone, with all other parameters being functions of this higher order quantity. At present this hypothesis is speculative, but encouraging since good compatibility is found with more established transition models.

Introduction

The transition of boundary layers has both advantages and disadvantages in engineering applications. As noted by Denton [1], turbulent boundary layers have higher entropy generation rates than laminar ones and thus more work is lost in turbulent boundary layers. However, turbulent boundary layers are often an advantage in a system as they induce high rates of heat transfer, Schobeiri and Chakka [2]. Unfortunately, the physical criterion by which transition onset occurs has eluded researchers for over a century. A common approach taken at present is to predict that transition onset will occur when some predicted critical value is reached, with the nature and magnitude of this critical value being the subject of numerous experimental and theoretical investigations. Despite these efforts no universally accepted criterion has emerged for transition onset. The problem is further complicated by the various definitions of transition onset ranging from a growing instability to fully turbulent flow. Here it is the formation of turbulent spots that is taken to imply transition onset, which is usually accompanied by an increase in wall shear stress.

A number of transition models have been correlated on experimental data and thus any proposed transition model must agree with these correlations. In this paper an attempt is made to relate the second law of thermodynamics to boundary layer transition onset and to propose a new criterion for this phenomenon. The second law is applied because it is used in a number of scientific fields as a measure of stability, Kondepudi and Prigogine [3], Prigogine [4], here it will be applied as a measure of boundary layer stability, i.e. transition.

In this paper some of the primary factors that are know from experiment to affect the location of transition onset are briefly discussed. It is then demonstrated that there is a relationship between these parameters and the entropy generation rate. Well-established transition models are expressed in terms of the entropy generation rate to demonstrate the compatibility with these models. The advantage of using the entropy model is that the entropy generation rate will be sensitive to all the parameters that affect transition onset both primary and secondary. This advantage is in contrast to many of the presently used models. The hypothesis presented herein is that the driving force of boundary layer transition onset is the entropy generation rate alone, with all other parameters being functions of this higher order quantity.

Primary Factors affecting Transition Onset

Effect of Reynolds Number

The Reynolds number has been, for a number of years, considered as one of the fundamental parameters associated with transition onset. Indeed in many cases a critical Reynolds number is used to predict whether a flow is either laminar or turbulent. Some examples of these situations, which give critical Reynolds numbers at which the flow may be considered fully turbulent, are:

Re_D ≈ 2000 - Pipe Flow

Re_L ≈ 5×10⁵ - Flat Plate Flow

Re_x ≈ 3.5×10⁵ - Gas Turbine Flow

It is well recognized, however, that such simple correlations will never result in accurate predictions of transition onset on a body of arbitrary shape as (1), the Reynolds numbers in these forms contains no flow history, (2) transition onset is known to depend on a number of dimensionless parameters and (3) the transition process is not a single point event as such simple models imply. However, when adopting the approach of using Reynolds number to predict transition, a more apt approach is to consider a Reynolds number that contains flow history. Numerous authors have applied this approach to correlate transition onset data by using the Reynolds number based on momentum thickness at transition onset to collapse data. While disagreement exists as to the critical value of Reynolds number at transition onset, all authors agree that increasing Reynolds number results in an earlier transition onset in terms of wetted distance along the surface under consideration.

Effect of Turbulence Intensity

A vast amount of research has been aimed at determining the physical process by which free stream turbulence affects the laminar boundary layer. Much of this research has fallen under the term “receptivity” to describe how a laminar boundary layer interacts with free stream turbulence. The fact that the turbulence intensity has an effect on transition onset has been well established experimentally by, amongst others, Abu-Ghannam and Shaw [5], Mayle [6] and Roach and Brierley [7]. Authors such as Roach and Brierley [7] have clearly shown that fluctuations exist within the pre-transitional laminar boundary layer in the presence of free stream turbulence as shown in Figure 1 from the T3A classic flat plate data set. From these measurements Mayle and Schulz [8] note that a turbulence intensity of over 10% can exist in a “laminar boundary layer” with a free stream turbulence of 2-3% as shown in Figure 1. This phenomenon has been known for several years with a number of models being proposed to solve laminar boundary layer flows with free stream turbulence. These include Moore [9], Lighthill [10] and Ackerberg and Philips [11]. The data of Roach and Brierley [7] indicated that the mean velocity profile is also affected by free stream turbulence, with Volino and Simon [12] also showing that these fluctuations have an influence on mean velocity profiles. Understanding the effects of turbulence intensity on laminar boundary layers remains the subject of many ongoing experimental and numerical investigations but all experimental data indicates that increasing the turbulence intensity moves transition onset upstream, c.f., Abu-Ghannam and Shaw [5], Mayle [6], Roach and Brierley [7], Walsh [13].

Figure 1. Effect of Free Stream Turbulence on fluctuations in laminar boundary layer.

Figure 1. Effect of Free Stream Turbulence on fluctuations in laminar boundary layer.

Effect of Mach Number

After an analysis of available data Narasimha [14] concluded that much work was still required before the effect of Mach number on transition could be understood. It is the author’s opinion that this conclusion is still valid. Narasimha [14] did however propose from the best fit of experimental data that the effect of Mach number on the transition onset Re_θ is given by equation 1 over the range of 0.2≳M≲2.4 with percentage turbulence intensities in the free stream of 0.1-3%.

$${\mathrm{Re}}_{\theta \left(ST\right)}\propto \sqrt{1+0.38M^{0.6}}$$

1

Thus, Narasimha [14] found the effect of Mach number was a delayed transition onset. In contrast, Schook et al. [15] found the transition onset Re_θ to be consistently lower than that correlated by Mayle [6] for the incompressible range. However, Walsh [13] found reasonably good agreement with the incompressible correlations of Mayle [6] for Mach numbers of the order of 1. Clearly, the experimental evidence is contradictory. It should also be noted that transition in compressible flows is further complicated by possible shock-boundary layer interaction promoting an earlier transition.

Numerous other parameters influence transition onset in a steady flow, such as separation, surface roughness, surface curvature and heat transfer. What has been considered here are some of the primary effects on transition onset upon which a concentrated research effort has been focused.

Proposed Transition Onset Models

Several transition models have been proposed over the years in what has proved to be an almost futile attempt at obtaining a universal prediction of transition onset. Much of the experimental data is aimed at predicting the onset of transition on airfoils, with the vast majority of the experimental work done on flat plates. In these transition models, it is commonplace to find a transition onset Re_θ that is defined in terms of free stream parameters that affect the transition onset location. Such models include:

Re_θ = 400Tu^−0.625 Mayle [6]

2

Re_θ = 460Tu^−0.65 Hourmouziadis [16]

3

4

Liepmann [17] took a different approach and proposed that transition begins where the maximum Reynolds stress in the laminar boundary layer equals the wall shear stress. Thus, when

5

transition onset ensues. The transition model of Liepmann [17] differs fundamentally from those based on Re_θ, equations 2 to 4, in that equation 5 is dependent on local values within the boundary layer and not integral parameters such as the momentum thickness. These models may be considered the simple transition onset models as they originate from correlations based on semi-empirical work. A number of more complex theoretical and numerical models have been proposed which address the amplification of instabilities in boundary layers such as the eⁿ model, Schlichting [18], and Direct Numerical Simulation (DNS), Launder and Sandham [19]. All with only limited success to date.

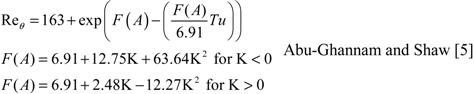
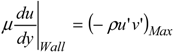
Unification of Transition Onset Parameters

Almost all transition models have been correlated on experimental data and, thus, any proposed model must agree with these data. Mayle and Schulz [8] attempted a partial unification of the factors affecting transition onset by the use of a Reynolds number with a turbulence length scale. Presently, however there is no transition model, which has the possibility of incorporating all the factors, both primary and secondary, which affect the location of transition onset. Here an attempt is made to relate the second law of thermodynamics to boundary layer transition onset and to propose a new criterion for transition onset that will allow a unification of these parameters. The second law is applied because it is used in a number of scientific fields as a measure of stability, here it will be used as measure of boundary layer stability, i.e., transition.

Entropy Generation Rate

The entropy generation rate per unit-wetted area in a steady, incompressible, two-dimensional, adiabatic boundary layer is given by O’Donnell and Davies [20] as

6

This relation may be represented in the form of a non-dimensional dissipation coefficient given by Denton [1] as

$$C_d=\frac{T{S}^{\text{'}\text{'}}}{\rho U_e^3}$$

7

Walsh [13] gives an analytical result, obtained from the integration of the Pohlhausen family of velocity profiles, for C_d in laminar boundary layers with pressure gradients as:

8

where Λ is the Pohlhausen pressure gradient parameter and ranges from a value of –12 at separation to +12 for highly accelerating flows, with a corresponding range of 0.16-0.20 for the bracketed term of equation 8. Denton [1] notes that laminar boundary layers are much more likely to exist on surfaces with favorable pressure gradients. Therefore, in accordance with equation 8, which shows the bracketed term to grow with favorable pressure gradients, he suggests a constant value of 0.2 for this term, thus giving the dissipation coefficient as simply:

9

Thus, all the transition models based on Re_θ may be expressed in terms of the entropy generation rate. For example, the transition onset model of Mayle [6], equation 2, may be reformulated in terms of the dimensionless entropy by using equation 9. This approach gives a transition onset model with the critical parameter being the non-dimensional entropy as:

$$C_{d\left(ST\right)}=0.0005{TU}^{\frac{5}{8}}$$

10

Figure 2 shows both transition onset models. While this figure only shows the same correlation expressed in a different way, C_d has the potential of being sensitive to more parameters than Re_θ. Thus, if experimentalists applied this approach, some of the scatter found from correlating the transition onset data may be reduced. It should be noted, however, that a direct measurement of C_d using equations 6 and 7 is significantly more difficult than measuring the integral parameters for the purpose of using the transition models of equations 2 to 4.

Figure 2. Comparison of Mayle [6] transition onset model, equation 2, and entropy transition onset model, equation 10

Figure 2. Comparison of Mayle [6] transition onset model, equation 2, and entropy transition onset model, equation 10

The transition onset models based upon a critical Re_θ, or any other integral parameter, implicitly suggest that transition onset is dependent on an integral of the boundary layer. Liepmann [17] took a different approach and formulated a transition onset criteria based on local values within the boundary layer. He proposed that when the maximum Reynolds stress in the boundary layer equals the wall shear stress transition onset follows, see equation 5. The Liepmann model suggests that the laminar boundary layers must first contain Reynolds stresses, a situation which has being reported by several authors including Volino and Simon [12] and Moss and Oldfield [23]. Strikingly, equation 5 becomes equivalent to stating that transition onset occurs when the entropy generated due to the mean velocity gradient at the wall equals the entropy generated due to the Reynolds stresses, when both sides of this equation are multiplied by the local velocity gradient as can be seen from equation 6.

The present transition onset hypothesis is also dependent on the Reynolds stresses in the laminar boundary layer. It is based upon the observation that nature seems to dictate that for an attached boundary layer the entropy generation rate per unit volume decreases as the distance from the wall increases. When this condition is not satisfied within a laminar boundary layer the entropy profile will become unstable, thus initiating transition by way of a turbulent spot. The result is a decreasing entropy profile with increasing distance from the wall in the form of a turbulent boundary layer. This criterion for transition onset to occur may be expressed as:

11

The entropy generation rate at any non-dimensional distance from the wall may be calculated by replacing the velocity gradient terms of equation 6 with the fourth order polynomial of Pohlhausen [24]. Applying this substitution to the case of an unsteady laminar boundary layer on a flat plate with zero pressure gradient reduces equation 6 to:

12

Thus, the distribution and magnitude of the Reynolds stresses within a laminar boundary layer play an important role in determining the location of transition onset using the proposed criterion of equation 12. An intensive experimental program is needed to determine both the magnitude and distribution of the Reynolds stresses within the unsteady laminar boundary layer to develop this hypothesis further. Interestingly, from equation 12, the entropy generation rate per unit volume in a steady laminar boundary layer is independent of viscosity, and thus independent of Reynolds number also, since the Reynolds shear stress in the second term is inherently zero. Put another way, the viscous entropy generation rate per unit volume is not directly dependent on the viscosity of the fluid. However, it is indirectly dependent on these quantities since they determine the boundary layer thickness, which appears in the definition of the non-dimensional wall distance, η.

Mach Number

It was noted earlier that the effect of Mach number on transition onset has been the subject of conflicting reports. By considering the energy integral equation, the effect of Mach number on the dimensionless entropy was shown by Walsh [13] as adapted from Schlichting [18] to be given by:

13

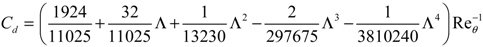
thus showing that the dissipation coefficient is dependent upon the Mach number but with its effect on the entropy generation rate being highly coupled with the type of flow field under consideration. This coupling of a number of terms may help explain why the experimental evidence is contradictory as to the effect of Mach number on transition onset.

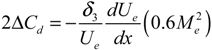
Conclusions and Future Work

• Established transition models are shown to be functions of the entropy generation rate. Further work is required to verify the new criterion with detailed measurements of the Reynolds stresses in laminar boundary layers at transition onset being required.
• For a steady laminar boundary layer, the viscous entropy production per unit volume is independent of the viscosity of the fluid.
• The relationship between Mach number and entropy production was shown to be strongly coupled to the flow field under consideration.
• For future work detailed measurements from pre-transitional laminar boundary layers to allow the implementation of the above hypothesis are needed.

Closure

The main hypothesis presented in this paper is that the driving force of boundary layer transition onset is the entropy generation rate alone, with all other parameters being functions of this higher order quantity. Following from this thought, it has been shown that many of the currently employed transition models may be expressed in terms of the entropy generation rate. The aim of this paper was to present a new idea that entropy might be the driving force in one of the great unsolved fluid mechanics problems, i.e., boundary layer transition. In closure the authors would like to leave the reader with some ideas/questions that will need to be answered before a true understanding of boundary layer transition is elucidated. Some basic research questions are, why should a regime be replaced by another? What is invariant during transition, laminar-turbulent or turbulent-laminar? What is being maximized or minimized during transition, regardless of direction? One answer, and a possible direction for inquiry is provided by Constructal Theory, Bejan [25], according to which the flow searches for and selects a configuration that maximizes access for currents.