Single Molecule Studies on Dynamics in Liquid Crystals
Abstract
:1. Introduction
2. Single Molecule Investigation of LC Cells Using Small Dye Molecules
2.1. Diffusion Anisotropy of Small Solute Molecules from SM in Nematic LC Cells
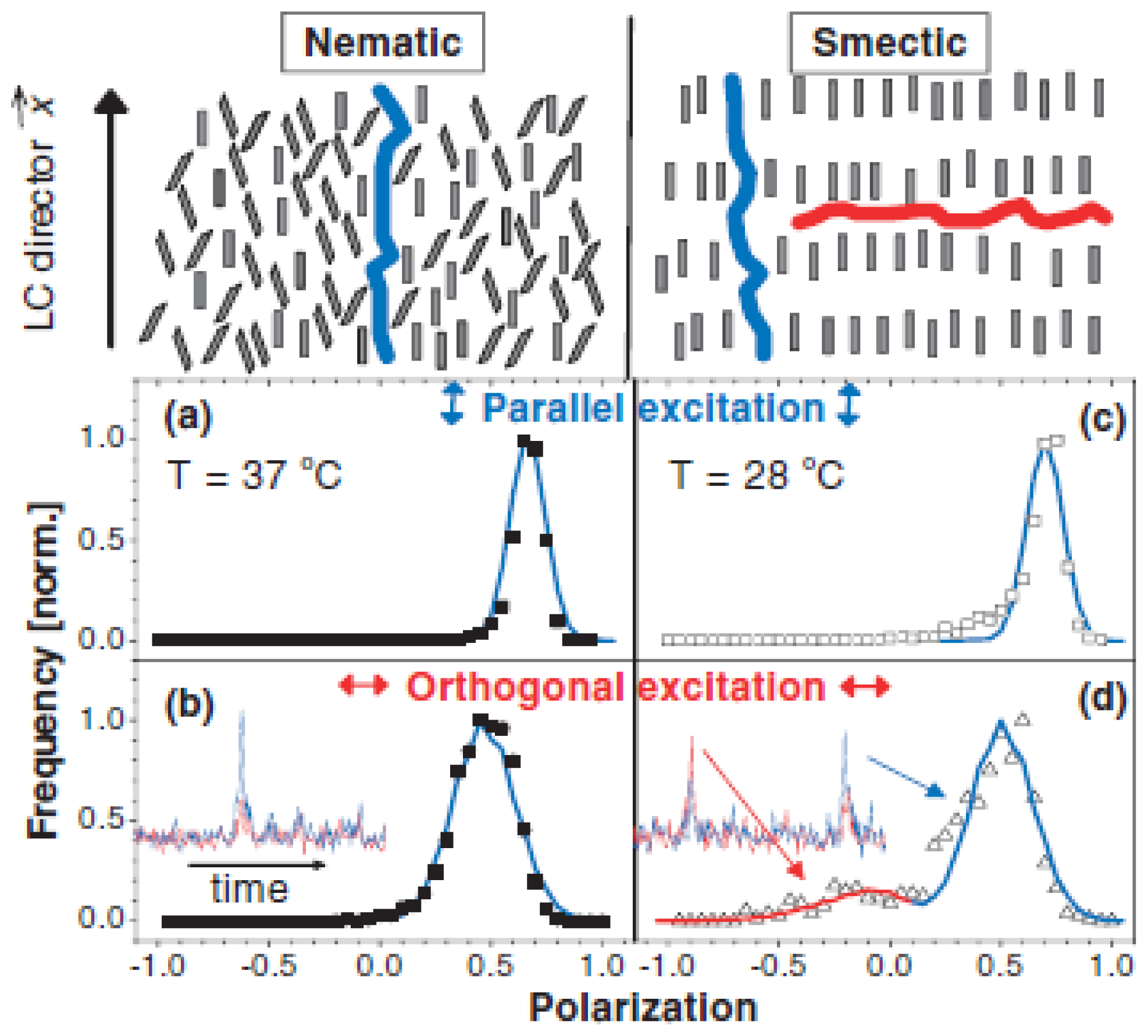
2.2. Locally Resolved Structure Related Dynamics in Nematic 5CB and Smectic 8CB Cells
3. SM Studies on Diffusion and Structure Related Dynamics in Thin Frustrated 8CB Films
4. Conjugated Polymers in Liquid Crystal Matrices
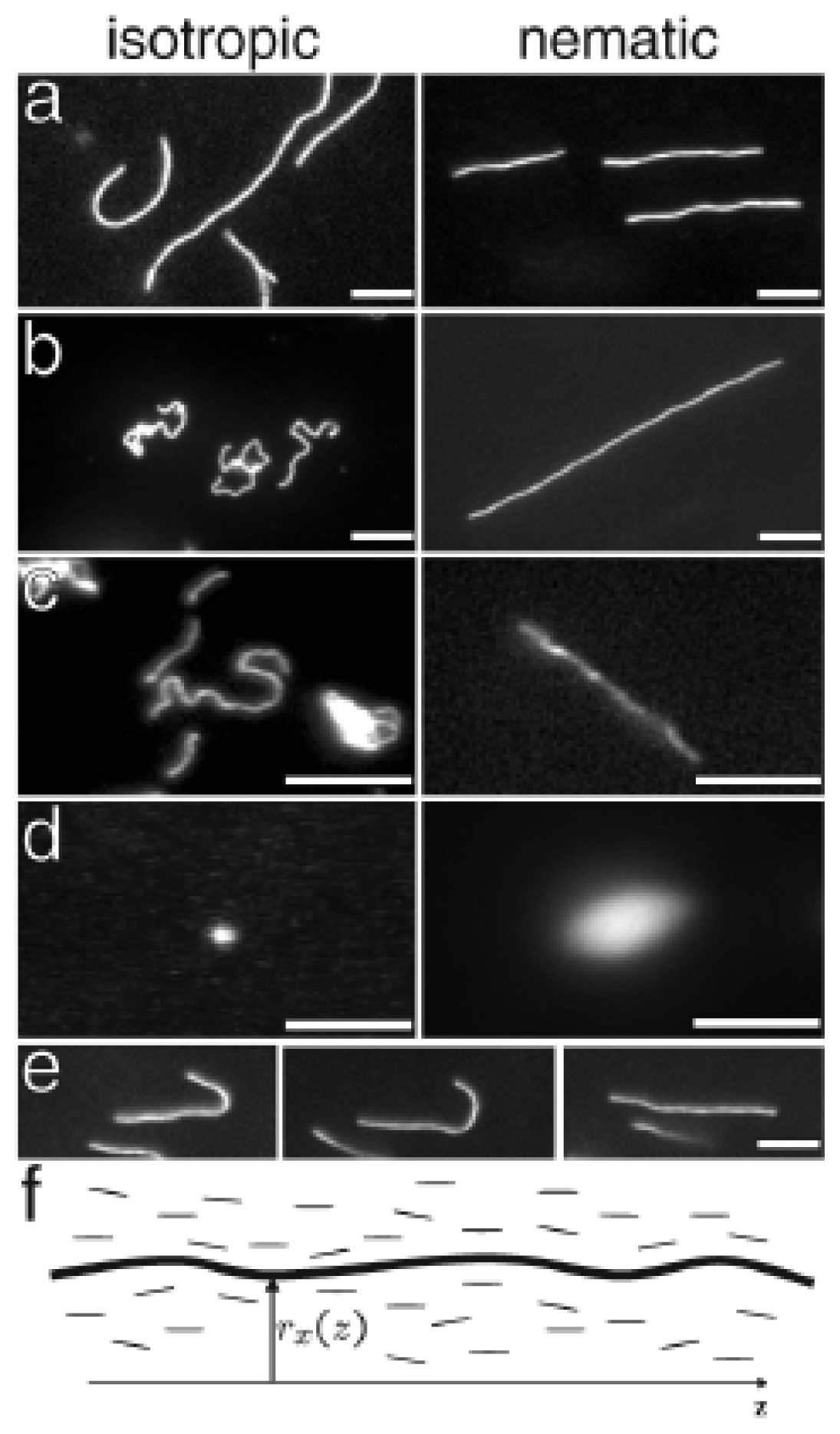
4.1. Order Parameters of MEH-PPV in Nematic 5CB
4.2. Order Parameters of MEH-PPV in Nematic/Smectic 8CB
4.3. Diffusion Anisotropy of MEH-PPV in Nematic 5CB
4.4. Local Structure Exploration in Electric Field Aligned LC by Use of MEH-PPV
5. Biological LC Materials and Their Impacts on Large Guest Molecules
6. Conclusions
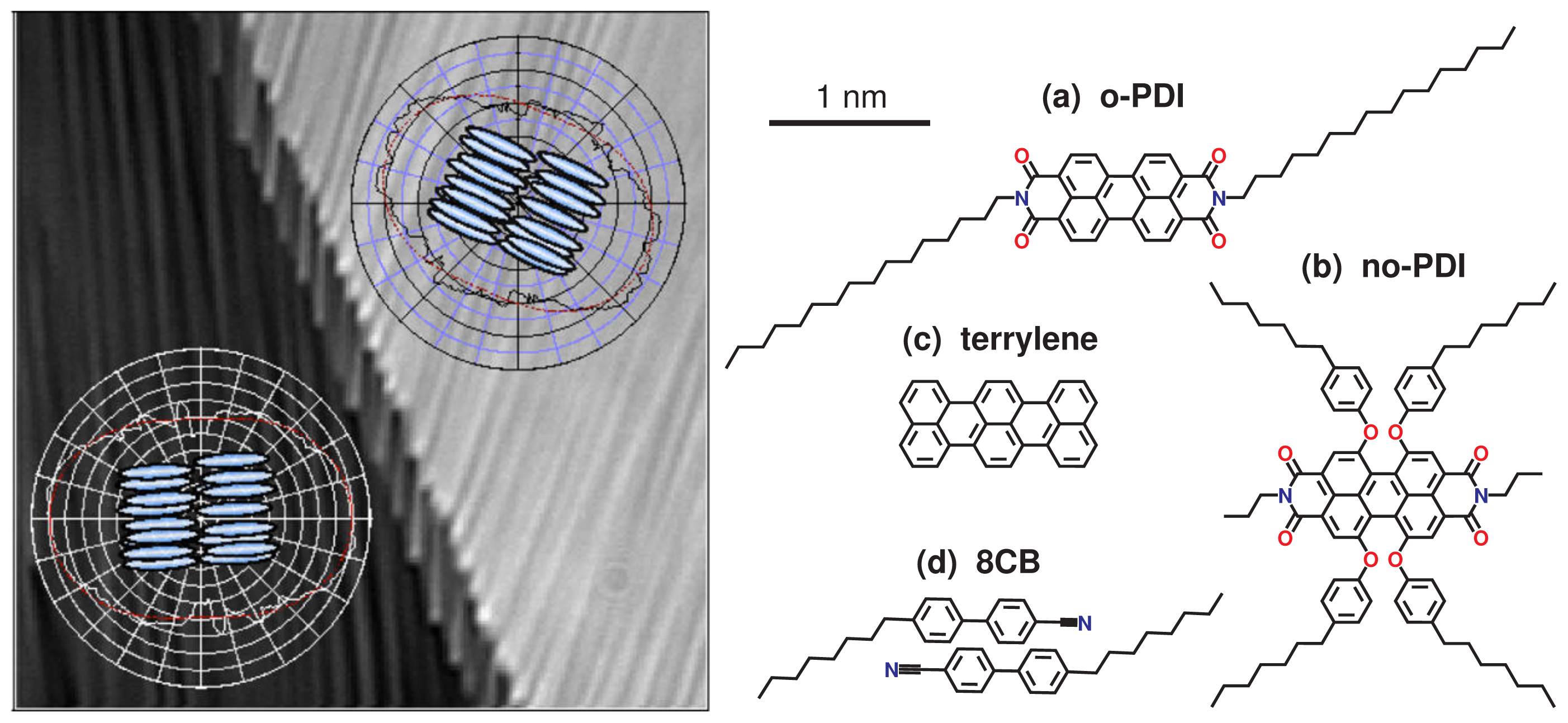
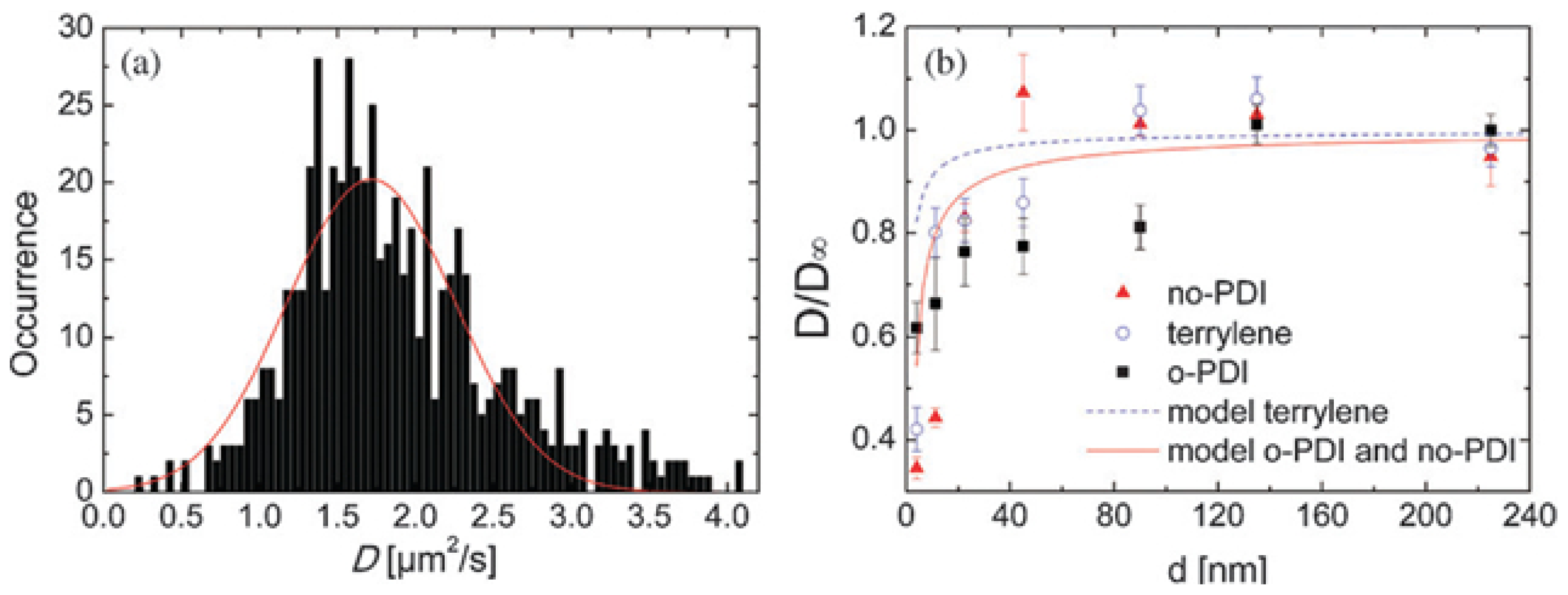


| LC | Method | Used molecule | Sample | D|| | D⊥ | D||=D⊥ |
|---|---|---|---|---|---|---|
| E7 [32] | FCS | perylene diimide | 1 μm cell | 18 | 3.0 | 4 |
| E7 [33] | FCS | antraquinones | 2 μm cell | 5 | ||
| E7 [42] | FRAP | cyanine | 100 μm cell | 1.2 ± 0.1 | ||
| 5CB [34] | SMT | perylene diimide | 1 μm cell | 8.47 ± 0.2 | 5.75 ± 0.2 | 1.5 ± 0.2 |
| 5CB [34] | FRAP | perylene diimide | D̄ = 2.6 ± 0.8 | |||
| 5CB [17] | NMR | LC self-diffusion | bulk | 50 | 20 | 2.5 |
| 5CB [43] | FRS | methyl red | 100 μm cell | 20 | 13 | 1.5 |
| 8CB [34] | SMT | perylene diimide | 0.5 μm cell, area 1 | 4.5 ± 0.2 | 2.9 ± 0.2 | 1.5 ± 0.2 |
| 8CB [34] | SMT | perylene diimide | 0.5 μm cell, area 2 | 4.3 ± 0.2 | 2.8 ± 0.2 | 1.5 ± 0.2 |
| 8CB [36] | SMT | perylene diimide | 0.2 μm film 1,2 | 3.8 ± 0.6 | 2.4 ± 0.3 | 1.6 |
| 8CB [35] | FCS | perylene diimide (no) | 0.2 μm film 1,3 | 110 ± 30 | 0.3 ± 0.1 | 360 |
| 8CB [35] | FCS | perylene diimide (o) | 0.2 μm film 1,3 | 36 ± 11 | 0.4 ± 0.3 | 90 |
| 8CB [35] | FCS | terrylene | 0.2 μm film 1,3 | 13 ± 7 | 0.4 ± 0.1 | 30 |
| 8CB [34] | FRAP | perylene diimide | D̄ = 3.2 ± 0.6 | |||
| 8CB [17] | NMR | LC self-diffusion | bulk | 25 | 14 | 1.7 |
| 8CB [43] | FRS | methyl red | 100 μm cell | 13 | 9 | 1.5 |
Acknowledgments
Conflicts of Interest
References
- Sluckin, T.J.; Dunmur, D.A.; Stegemeyer, H. Crystals that Flow: Classic Papers from the History of Liquid Crystals; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Bouligand, Y. Liquid crystals and biological morphogenesis: Ancient and new questions. Comptes Rendus Chim 2008, 11, 281–296. [Google Scholar]
- Cowin, S.C. Do liquid crystal-like flow processes occur in the supramolecular assembly of biological tissues? J. Non-Newton. Fluid Mech 2004, 119, 155–162. [Google Scholar]
- Giraud-Guille, M.M.; Mosser, G.; Belamie, E. Liquid crystallinity in collagen systems in vitro and in vivo. Curr. Opin. Colloid Interface Sci 2008, 13, 303–313. [Google Scholar]
- Aldoroty, R.A.; Garty, N.B.; April, E.W. Donnan potentials from striated muscle liquid crystals. Lattice spacing dependence. Biophys. J 1987, 51, 371–381. [Google Scholar]
- Giraud-Guille, M.M.; Belamie, E.; Mosser, G.; Helary, C.; Gobeaux, F.; Vigier, S. Liquid crystalline properties of type I collagen: Perspectives in tissue morphogenesis. Comptes Rendus Chim 2008, 11, 245–252. [Google Scholar]
- Dunmur, D.; Sluckin, T. Soap, Science, & Flat-Screen TVs—A History of Liquid Crystals; Oxford University Press: New York, NY, USA, 2011. [Google Scholar]
- Struijk, C.W.; Sieval, A.B.; Dakhorst, J.E.J.; van Dijk, M.; Kimkes, P.; Koehorst, R.B.M.; Donker, H.; Schaafsma, T.J.; Picken, S.J.; van de Craats, A.M.; et al. Liquid crystalline perylene diimides:–Architecture and charge carrier mobilities. J. Am. Chem. Soc 2000, 122, 11057–11066. [Google Scholar]
- Zentel, R.; Brehmer, M. 8.06—Electroactive Liquid Crystalline Polymers. In Polymer Science: A Comprehensive Reference; Matyjaszewski, E.i.C.K., Müller, M., Eds.; Elsevier: Amsterdam, The Netherlands, 2012; pp. 129–145. [Google Scholar]
- Hwang, J.; Song, M.H.; Park, B.; Nishimura, S.; Toyooka, T.; Wu, J.W.; Takanishi, Y.; Ishikawa, K.; Takezoe, H. Electro-tunable optical diode based on photonic bandgap liquid-crystal heterojunctions. Nat. Mater 2005, 4, 383–387. [Google Scholar]
- Alkeskjold, T.T.; Laegsgaard, J.; Bjarklev, A.; Hermann, D.S.; Anawati, A.; Broeng, J.; Li, J.; Wu, S.T. All-optical modulation in dye-doped nematic liquid crystal photonic bandgap fibers. Opt. Express 2004, 12, 5857–5871. [Google Scholar]
- Bravo-Sanchez, M.; Simmons, T.J.; Vidal, M.A. Liquid crystal behavior of single wall carbon nanotubes. Carbon 2010, 48, 3531–3542. [Google Scholar]
- Martinez-Felipe, A.; Badia, J.D.; Santonja-Blasco, L.; Imrie, C.T.; Ribes-Greus, A. A kinetic study of the formation of smectic phases in novel liquid crystal ionogens. Eur. Polym. J 2013, 49, 1553–1563. [Google Scholar]
- Lukishova, S.G. Liquid crystals under two extremes: (1) High-power laser irradiation, and (2) single-photon level. Mol. Cryst. Liquid Cryst 2012, 559, 127–157. [Google Scholar]
- Ma, J.; Ye, X.; Jin, B. Structure and application of polarizer film for thin-film-transistor liquid crystal displays. Displays 2011, 32, 49–57. [Google Scholar]
- Lagerwall, J.P.F.; Scalia, G. A new era for liquid crystal research: Applications of liquid crystals in soft matter nano-, bio- and microtechnology. Curr. Appl. Phys 2012, 12, 1387–1412. [Google Scholar]
- Dvinskikh, S.V.; Furo, I.; Zimmermann, H.; Maliniak, A. Anisotropic self-diffusion in thermotropic liquid crystals studied by 1H and 2 H pulse-field-gradient spin-echo NMR. Phys. Rev. E 2002, 65, 061701. [Google Scholar]
- Kulzer, F.; Orrit, M. Single-molecule optics. Annu. Rev. Phys. Chem 2004, 55, 585–611. [Google Scholar]
- Moerner, W.E.; Fromm, D.P. Methods of single-molecule fluorescence spectroscopy and microscopy. Rev. Sci. Instrum 2003, 74, 3597–3619. [Google Scholar]
- Barbara, P.; Chang, W.S.; Link, S.; Scholes, G.; Yethiraj, A. Structure and dynamics of conjugated polymers in liquid crystalline solvents. Ann. Rev. Phys. Chem 2007, 58, 565–584. [Google Scholar]
- Mousavi, S.H.; Koushki, E.; Haratizadeh, H. Nonlinear optical investigation of Gaussian laser beam propagating in a dye-doped nematic liquid crystal. J. Mol. Liquids 2010, 153, 124–128. [Google Scholar]
- Schuster, J.; Cichos, F.; von Borczyskowski, C. Anisotropic diffusion of single molecules in thin liquid films. Eur. Phys. J. E: Soft Matter Biol. Phys 2003, 12, 75–80. [Google Scholar]
- Schob, A.; Cichos, F.; Schuster, J.; von Borczyskowski, C. Reorientation and translation of individual dye molecules in a polymer matrix. Eur. Polym. J 2004, 40, 1019–1026. [Google Scholar]
- Araoz, B.; Täuber, D.; von Borczyskowski, C.; Aramendia, P.F. Cage effect in Poly(alkyl methacrylate) thin films studied by nile red single molecule fluorescence spectroscopy. J. Phys. Chem. C 2012, 116, 7573–7580. [Google Scholar]
- Nelson, N.; Walder, R.; Schwartz, D.K. Single molecule dynamics on hydrophobic self-assembled monolayers. Langmuir 2012, 28, 12108–12113. [Google Scholar]
- Täuber, D.; Trenkmann, I.; von Borczyskowski, C. Influence of van der Waals interactions on morphology and dynamics in ultrathin liquid films at silicon oxide interfaces. Langmuir 2013, 29, 3583–3593. [Google Scholar]
- Gerlach, F.; Täuber, D.; von Borczyskowski, C. Correlated blinking via time dependent energy transfer in single CdSe quantum dot-dye nanoassemblies. Chem. Phys. Lett. 2013, 572, 90–95. [Google Scholar]
- Zurner, A.; Kirstein, J.; Doblinger, M.; Bräuchle, C.; Bein, T. Visualizing single-molecule diffusion in mesoporous materials. Nature 2007, 450, 705–708. [Google Scholar]
- Pramanik, R.; Ito, T.; Higgins, D.A. Molecular length dependence of single molecule wobbling within surfactant- and solvent-filled silica mesopores. J. Phys. Chem. C 2013, 117, 15438–15446. [Google Scholar]
- Kowerko, D.; Schuster, J.; von Borczyskowski, C. Restricted conformation dynamics of single functionalized perylene bisimide molecules on SiO2 surfaces and in thin polymer films. Mol. Phys 2009, 107, 1911–1921. [Google Scholar]
- Krause, S.; Aramendia, P.F.; Täuber, D.; von Borczyskowski, C. Freezing single molecule dynamics on interfaces and in polymers. Phys. Chem. Chem. Phys 2011, 13, 1754–1761. [Google Scholar]
- Kawai, T.; Yoshihara, S.; Iwata, Y.; Fukaminato, T.; Irie, M. Anisotropic translational diffusion of single fluorescent perylene molecules in a nematic liquid crystal. ChemPhysChem 2004, 5, 1606–1609. [Google Scholar]
- Kawai, T.; Kubota, A.; Kawamura, K.; Tsumatori, H.; Nakashima, T. Single molecule fluorescence autocorrelation measurement on anisotropic molecular diffusion in nematic liquid crystal. Thin Solid Films 2008, 516, 2666–2669. [Google Scholar]
- Pumpa, M.; Cichos, F. Slow single-molecule diffusion in liquid crystals. J. Phys. Chem. B 2012, 116, 14487–14493. [Google Scholar]
- Schulz, B.; Täuber, D.; Friedriszik, F.; Graaf, H.; Schuster, J.; von Borczyskowski, C. Optical detection of heterogeneous single molecule diffusion in thin liquid crystal films. Phys. Chem. Chem. Phys 2010, 12, 11555–11564. [Google Scholar]
- Schulz, B.; Täuber, D.; Schuster, J.; Baumgärtel, T.; von Borczyskowski, C. Influence of mesoscopic structures on single molecule dynamics in thin smectic liquid crystal films. Soft Matter 2011, 7, 7431–7440. [Google Scholar]
- Chang, W.S.; Link, S.; Yethiraj, A.; Barbara, P.F. Single molecule spectroscopy of conjugated polymer chains in an electric field-aligned liquid crystal. J. Phys. Chem. B 2008, 112, 448–453. [Google Scholar]
- Lettinga, M.P.; Barry, E.; Dogic, Z. Self-diffusion of rod-like viruses in the nematic phase. Europhys. Lett 2005, 71, 692. [Google Scholar]
- Grelet, E.; Lettinga, M.P.; Bier, M.; van Roij, R.; van der Schoot, P. Dynamical and structural insights into the smectic phase of rod-like particles. J. Phys. Cond. Mat 2008, 20, 494213. [Google Scholar]
- Pouget, E.; Grelet, E.; Lettinga, M.P. Dynamics in the smectic phase of stiff viral rods. Phys. Rev. E 2011, 84, 041704. [Google Scholar]
- Dogic, Z.; Zhang, J.; Lau, A.W.C.; Aranda-Espinoza, H.; Dalhaimer, P.; Discher, D.E.; Janmey, P.A.; Kamien, R.D.; Lubensky, T.C.; Yodh, A.G. Elongation and fluctuations of semiflexible polymers in a nematic solvent. Phys. Rev. Lett. 2004, 92, 125503. [Google Scholar]
- Etchegoin, P. Fluorescence photobleaching recovery spectroscopy in a dye doped nematic liquid crystal. Phys. Rev. E 1999, 59, 1860–1867. [Google Scholar]
- Hara, M.; Takezoe, H.; Fukuda, A. Forced rayleigh scattering in nCB’s (n = 5-9) with methyl red and binary mass diffusion constants. Jpn. J. Appl. Phys. 1986, 25, 1756–1761. [Google Scholar]
- Stark, H.; Ventzki, D. Stokes drag of spherical particles in a nematic environment at low Ericksen numbers. Phys. Rev. E 2001, 64, 031711. [Google Scholar]
- Loudet, J.C.; Hanusse, P.; Poulin, P. Stokes drag on a sphere in a nematic liquid crystal. Science 2004, 306, 1525. [Google Scholar]
- Xu, L.; Salmeron, M.; Bardon, S. Wetting and molecular orientation of 8CB on silicon substrates. Phys. Rev. Lett. 2000, 84, 1519–1522. [Google Scholar]
- Designolle, V.; Herminghaus, S.; Pfohl, T.; Bahr, C. AFM study of defect-induced depressions of the smectic-A/Air interface. Langmuir 2006, 22, 363–368. [Google Scholar]
- Bardon, S.; Ober, R.; Valignat, M.P.; Vandenbrouck, F.; Cazabat, A.M.; Daillant, J. Organization of cyanobiphenyl liquid crystal molecules in prewetting films spreading on silicon wafers. Phys. Rev. E 1999, 59, 6808–6818. [Google Scholar]
- Lacaze, E.; Michel, J.P.; Alba, M.; Goldmann, M. Planar anchoring and surface melting in the smectic- A phase. Phys. Rev. E 2007, 76, 041702. [Google Scholar]
- Roscioni, O.M.; Muccioli, L.; Della Valle, R.G.; Pizzirusso, A.; Ricci, M.; Zannoni, C. Predicting the anchoring of liquid crystals at a solid surface: 5-cyanobiphenyl on cristobalite and glassy silica surfaces of increasing roughness. Langmuir 2013, 8950–8958. [Google Scholar]
- Wöll, D.; Braeken, E.; Deres, A.; DeSchryver, F.C.D.; Uji-i, H.; Hofkens, J. Polymers and single molecule fluorescence spectroscopy, what can we learn? Chem. Soc. Rev 2009, 38, 313–328. [Google Scholar]
- Täuber, D. Technische Universität Chemnitz: Germany, Unpublished work; 2013.
- Bras, M.; Dugas, V.; Bessueille, F.; Cloarec, J.P.; Martin, J.R.; Cabrera, M.; Chauvet, J.P.; Souteyrand, E.; Garrigues, M. Optimisation of a silicon/silicon dioxide substrate for a fluorescence DNA microarray. Biosens. Bioelectron 2004, 20, 797–806. [Google Scholar]
- Mensio, O.; Zamar, R.C.; Anoardo, E.; Acosta, R.H.; Dong, R.Y. On the role of collective and local molecular fluctuations in the relaxation of proton intrapair dipolar order in nematic 5CB. J. Chem. Phys 2005, 123, 204911–204919. [Google Scholar]
- Lacaze, E.; Michel, J.P.; Goldmann, M.; Gailhanou, M.; Boissieu, M.D.; Alba, M. Bistable nematic and smectic anchoring in the liquid crystal octylcyanobiphenyl (8CB) adsorbed on a MoS2 single crystal. Phys. Rev. E 2004, 69, 041705. [Google Scholar]
- Chakraborty, S.; Garcia, R. Optical-ellipsometric study of the nematic-to-smectic transition in 8CB films adsorbed on silicon. Phys. Rev. E 2010, 81, 031702. [Google Scholar]
- Garcia, R.; Subashi, E.; Fukuto, M. Thin-thick coexistence behavior of 8CB liquid crystalline films on silicon. Phys. Rev. Lett. 100, 01.
- Tao, J.; Tretiak, S.; Zhu, J.X. Prediction of excitation energies for conjugated oligomers and polymers from time-dependent density functional theory. Materials 2010, 3, 3430–3467. [Google Scholar]
- Lammi, R.K.; Fritz, K.P.; Scholes, G.D.; Barbara, P.F. Ordering of single conjugated polymers in a nematic liquid crystal host. J. Phys. Chem. B 2004, 108, 4593–4596. [Google Scholar]
- Link, S.; Hu, D.; Chang, W.S.; Scholes, G.D.; Barbara, P.F. Nematic solvation of segmented polymer chains. Nano Lett 2005, 5, 1757–1760. [Google Scholar]
- Link, S.; Chang, W.S.; Yethiraj, A.; Barbara, P.F. Anisotropic diffusion of elongated and aligned polymer chains in a nematic solvent. J. Phys. Chem. B 2006, 110, 19799–19803. [Google Scholar]
- Link, S.; Chang, W.S.; Yethiraj, A.; Barbara, P.F. Orthogonal orientations for solvation of polymer molecules in smectic solvents. Phys. Rev. Lett. 2006, 96, 017801. [Google Scholar]
- Durbin, S.D.; Arakelian, S.M.; Shen, Y.R. Optical-field-induced birefringence and freedericksz transition in a nematic liquid crystal. Phys. Rev. Lett. 1981, 47, 1411–1414. [Google Scholar]
- Tcherniak, A.; Solis, D.; Khatua, S.; Tangonan, A.A.; Lee, T.R.; Link, S. Chain-length dependent nematic ordering of conjugated polymers in a liquid crystal solvent. J. Am. Chem. Soc 2008, 130, 12262–12263. [Google Scholar]
- Onsager, L. The effects of shape on the interaction of colloidal particles. Ann. N. Y. Acad. Sci 1949, 51, 627–659. [Google Scholar]
- Chu, K.S.; Moroi, D.S. self-diffusion in nematic liquid crystals. J. Phys. Colloques 1975, 36, C1:9–C1:101. [Google Scholar]
- Hess, S.; Frenkel, D.; Allen, M. On the anisotropy of diffusion in nematic liquid crystals: Test of a modified affine transformation model via molecular dynamics. Mol. Phys 1991, 74, 765–774. [Google Scholar]
- Kilina, S.; Batista, E.R.; Yang, P.; Tretiak, S.; Saxena, A.; Martin, R.L.; Smith, D.L. Electronic structure of self-assembled amorphous polyfluorenes. ACS Nano 2008, 2, 1381–1388. [Google Scholar]
- Pizzirusso, A.; Berardi, R.; Muccioli, L.; Ricci, M.; Zannoni, C. Predicting surface anchoring: molecular organization across a thin film of 5CB liquid crystal on silicon. Chem. Sci 2012, 3, 573–579. [Google Scholar]
- Völtz, C.; Stannarius, R. Self-organization of isotropic droplets in smectic-C free-standing films. Phys. Rev. E 2004, 70, 061702. [Google Scholar]
- Tibirna, C.M.; Bazuin, C.G. Liquid crystalline complexes of an azo-containing surfactomesogen with oppositely charged polyelectrolytes. J. Polym. Sci. Part B 2005, 43, 3421–3431. [Google Scholar]
- Tian, Y.; Camacho, R.; Thomsson, D.; Reus, M.; Holzwarth, A.R.; Scheblykin, I.G. Organization of bacteriochlorophylls in individual chlorosomes from chlorobaculum tepidum studied by 2-dimensional polarization fluorescence microscopy. J. Am. Chem. Soc 2011, 133, 17192–17199. [Google Scholar]
- Camacho, R.; Thomsson, D.; Yadav, D.; Scheblykin, I.G. Quantitative characterization of light-harvesting efficiency in single molecules and nanoparticles by 2D polarization microscopy: Experimental and theoretical challenges. Chem. Phys 2012, 406, 30–40. [Google Scholar]
© 2013 by the authors; licensee MDPI, Basel, Switzerland This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Täuber, D.; Von Borczyskowski, C. Single Molecule Studies on Dynamics in Liquid Crystals. Int. J. Mol. Sci. 2013, 14, 19506-19525. https://doi.org/10.3390/ijms141019506
Täuber D, Von Borczyskowski C. Single Molecule Studies on Dynamics in Liquid Crystals. International Journal of Molecular Sciences. 2013; 14(10):19506-19525. https://doi.org/10.3390/ijms141019506
Chicago/Turabian StyleTäuber, Daniela, and Christian Von Borczyskowski. 2013. "Single Molecule Studies on Dynamics in Liquid Crystals" International Journal of Molecular Sciences 14, no. 10: 19506-19525. https://doi.org/10.3390/ijms141019506
APA StyleTäuber, D., & Von Borczyskowski, C. (2013). Single Molecule Studies on Dynamics in Liquid Crystals. International Journal of Molecular Sciences, 14(10), 19506-19525. https://doi.org/10.3390/ijms141019506




